Finite-Wright beams and their paraxial propagation

Abstract

In this study, we introduce a new set of light beams, which are referred to as finite-Wright beams (FWBs) and are expressed, in the initial plane, in terms of the generalized Wright function. These beams can be reduced under proper parameters conditions to well-known beams such as Mainardi beams, Hypergeometric beams, Airy beams, and Bessel beams. The analytical expression of FWBs propagating in a paraxial ABCD optical system with an annular rectangular aperture is derived based on the Huygens-Fresnel diffraction integral. The propagation properties of the considered beams in free space and through a fractional Fourier transform system are illustrated numerically as a function of the initial beam parameters and the optical system characteristics.

1 Introduction

The Airy beams (Berry and Balazs 1979) are known to display fascinating properties such as non-diffraction, transverse acceleration, and self-healing characteristics (Siviloglou et al. 2007; Siviloglou and Christodoulides 2007; Broky et al. 2008). These features allow the Airy beams to be solicited in many application fields, such as optical micromanipulations (Baumgartl et al. 2008), plasma physics (Polynkin et al. 2009; Ouahid et al. 2018a, b), optical switching (Ellenbogen et al. 2009), particles trapping (Jia et al. 2010), optical routing (Rose et al. 2013), and so on. During the last years, various models for the related-Airy beams have been introduced and their properties have been investigated, for instance, the Olver beams (Belafhal et al. 2015; Hennani et al. 2015a, b, c, 2016, 2017), Airy and Airy-Gaussian beams (Ez-Zariy et al. 2014a, 2014b, 2018; Ouahid et al. 2018c; Yaalou et al. 2019; Khonina and Ustinov 2017), and Mainardi beams (Habibi et al. 2018) among others. The cited models are non-diffracting beams and can also be reduced under specific parameters conditions to give the Airy beam. The electric field of a Mainardi beam (Habibi et al. 2018) can be expressed in terms of the Mainardi function M_ν(x), which represents a special case of the auxiliary Wright functions (Mainardi 2010). Its profile is determined by two parameters: the beam order ν which is a fractional number with 0 < v < 1, and the decay factor-a, which is associated with the exponential part and introduced to assure the finite energy of the beam. In the special case when ν = 1/q and q is an odd integer, Habibi et al. (Habibi et al. 2018) obtained the Mainardi beam, which represents the general case of Airy beams. The authors have also shown that the propagation properties of Mainardi beams through a fractional Fourier transform system (FRFT) can be controlled by adjusting the beam parameter q and the power order of the FRFT system. To extend the family of Mainardi beams, and inspired by the previous authors' idea, we propose a model based on the Wright functions. The proposed beam will be referred to as finite-Wright beam (FWB) and can be reduced by setting specific parameters conditions to give different known beams including Mainardi beams, Airy beams, Hypergeometric beams, and Bessel beams. Therefore, in the present paper, we introduce and investigate the propagation properties of a FWB in a paraxial ABCD optical system with an annular aperture. In the forthcoming section, the FWB is defined in the initial plane and its transverse intensity distribution is illustrated as a function of the beam parameters. Afterward, the propagation equation of the FWB in a paraxial ABCD optical system with an annular aperture is derived based on the Huygens-Fresnel integral and the expansion of the aperture function into Gaussian functions. The analytical expressions for a FWB propagating in free space and through FRFT are derived, and the propagation properties of the beam are presented with illustrative numerical examples in Sect. 3. In Sect. 4, a summary of the main results concludes this paper.

2 Theoretical model

2.1 Finite Wright beam in the initial plane

We begin by recalling the formula of the generalized Wright function $W_{\alpha ,\beta }^{\gamma ,\delta } \left( . \right)$ which is defined by the series expansion of the form (Mainardi 2010; El-Shahed and Salem 2015; Belafhal et al. 2022).

$$W_{{\alpha ,\beta }}^{{\gamma ,\delta }} \left( z \right) = \sum\limits_{{n = 0}}^{{ + \infty }} {\frac{{z^{n} }}{{n!a_{{\alpha ,\beta }}^{{\delta ,\gamma }} \left( n \right)}}} ,$$

(1)

where

$$a_{\alpha ,\beta }^{\delta ,\gamma } \left( n \right){\kern 1pt} {\kern 1pt} = \frac{{\left( \delta \right)_{n} }}{{\left( \gamma \right)_{n} }}\Gamma \left( {\alpha n + \beta } \right),$$

(2)

with α, β, δ and γ are complex-valued, α > 1, $\left( {{\kern 1pt} {\kern 1pt} .{\kern 1pt} {\kern 1pt} {\kern 1pt} } \right)_{n}$ is the Pochhammer symbol, and Γ(.) is the gamma function. For the sake of clarity, we have grouped in Table 1 some special functions (and their series coefficients) that are connected to the Wright function.

Table 1 Wright- related functions definitions

In mathematics, the Wright functions are known to play an important role in solving linear partial and fractional differential equations, and the generalized Wright function is convergent in the whole complex plane. In the special case when δ = γ, the generalized Wright function reduces to the Wright function $W_{\alpha ,\beta } \left( z \right)$ (with two parameters α and β) which can be expressed as

$$W_{\alpha ,\beta } (z) = \sum\limits_{n = 0}^{ + \infty } {\frac{{z^{n} }}{n!\Gamma (\alpha n + \beta )}} .$$

(3)

Furthermore, the Wright-related functions cited above can also be connected to other special functions, such as Bessel and modified Bessel functions, the Airy function, and the confluent hypergeometric functions (see Table 2 ).

Table 2 Some special functions connected with the Wright function

In addition, according to Eq. (1), and by setting the appropriate parameters α, β, δ and γ, many special cases can be distinguished, for instance, the case $\alpha = - v{\kern 1pt} {\kern 1pt} ,{\kern 1pt} {\kern 1pt} {\kern 1pt} \beta = 1 - \nu {\kern 1pt} {\kern 1pt}$ and the case $\alpha = - v{\kern 1pt} {\kern 1pt} ,{\kern 1pt} {\kern 1pt} {\kern 1pt} \beta = 0{\kern 1pt} {\kern 1pt}$ will lead to the Mainardi function $M_{\nu } \left( z \right)$ and the auxiliary function $F_{\nu } \left( z \right)$, respectively. In the following, we consider a beam model at initial plane z = 0 with a Wright-based function of the form

$$E\left( {x_{0} ,y_{0} ,z_{0} = 0} \right) = E_{0} W_{\alpha ,\beta } \left( { - \frac{{x_{0} }}{{\omega_{0} }}} \right)\exp \left( {\frac{{a_{0} x_{0} }}{{\omega_{0} }}} \right)W_{\alpha ,\beta } \left( { - \frac{{y_{0} }}{{\omega_{0} }}} \right)\exp \left( {\frac{{a_{0} y_{0} }}{{\omega_{0} }}} \right),$$

(4)

where $\left( {x_{0,} y_{0} } \right)$ are the Cartesian coordinates at the initial plane, W_{α, β} (.) is the Wright function, ω₀ is the waist width of the Gaussian part, a₀ is the decay parameter that is associated with finite energy, and E₀ is a normalization factor related to the beam power and is taken to be unity for the sake of simplicity. The field of Eq. (4) is referred to as FWB.

The Wright functions are introduced in details in Refs (Mainardi 1995; Povstenko 2021) as solutions to the diffusion-wave equation.

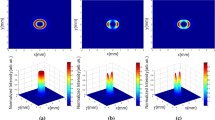
Figure 1 shows the intensity distribution of the FWB at the initial plane z = 0 for different values of the parameter a₀ (a₀ = 0, 0.03, 0.1 and 0.3).

Fig. 1

Normalized intensity distribution of FWB in the initial plane for: a $a_{0} = 0$, b $a_{0} = 0.03$, c $a_{0} = 0.1$ and d $a_{0} = 0.3$, with $\omega_{0} = 0.8\,{\text{mm}}$, $\alpha = - 0.34$ and $\beta = 0.83$

From Fig. 1, one can see that when $a_{0}$ = 0 (i.e., the ideal Wright beam case), the intensity profile of the FWB is Airy-like except that the central region is dark. With increasing the value of the parameter $a_{0}$, the side lobes intensity decreases gradually, and finally, when $a_{0}$ is larger than 0.3, the side lobes completely disappear. Besides that, one can deduct from Eq. 4 that, the parameter ω₀ plays also the role of a scaling coordinate factor, i.e. the ω₀ will affect proportionally the width of FWB lobes.

2.2 Propagation formula of a FWB in a paraxial ABCD optical system

In this Section, we investigate the propagation of a FWB in a paraxial ABCD system limited by an annular rectangular aperture. A schematic illustration of the considered aperture is shown in Fig. 2, where $a_{i}$ and $b_{i}$ $\left( {i = x,y} \right)$ denote the out and in half-width in the x- and y- directions, respectively.

Fig. 2

Schematic representation of the rectangular annular aperture

Within the framework of the paraxial approximation, the propagation of a FWB through a paraxial ABCD optical system obeys the Huygens-Fresnel diffraction integral, which can be expressed as (Lü and Ma 2000)

$$\begin{gathered} E\left( {x,y,z} \right) = \frac{i}{\lambda B}\exp \left( { - ikz} \right)\iint_{s} E\left( {x_{0} ,y_{0} ,0} \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \exp \left\{ { - \frac{ik}{{2B}}\left[ {A\left( {x_{0}^{2} + y_{0}^{2} } \right) - 2\left( {xx_{0} + yy_{0} } \right) + D\left( {x^{2} + y^{2} } \right)} \right]} \right\}dx_{0} dy_{0} , \hfill \\ \end{gathered}$$

(5)

where $E\left( {x_{0} ,y_{0} ,0} \right)$ and $E(x,y,z)$ are the beams at the source plane (z = 0) and the receiver plane, respectively. $z$ is the propagation distance, $k = \frac{2\pi }{\lambda }$ is the wavenumber with $\lambda$ is the wavelength of radiation in a vacuum, A, B and $D$ are the matrix elements of the optical system, and S is the annular area schematized in Fig. 2.

Equation (5) can be rearranged as.

$$\begin{aligned} E\left( {x,y,z} \right) & = C_{0} \left( {x,y,z} \right)\int\limits_{{ - a_{x} }}^{{a_{x} }} {\int\limits_{{ - a_{y} }}^{{a_{y} }} {E\left( {x_{0} ,y_{0} ,0} \right)\exp \left\{ { - \frac{ik}{{2B}}\left[ {A\left( {x_{0}^{2} + y_{0}^{2} } \right) - 2\left( {xx_{0} + yy_{0} } \right)} \right]} \right\}dx_{0} dy_{0} } } \\ & \quad - C_{0} \left( {x,y,z} \right)\int\limits_{{ - b_{x} }}^{{b_{x} }} {\int\limits_{{ - b_{y} }}^{{b_{y} }} {E\left( {x_{0} ,y_{0} ,0} \right)\exp \left\{ { - \frac{ik}{{2B}}\left[ {A\left( {x_{0}^{2} + y_{0}^{2} } \right) - 2\left( {xx_{0} + yy_{0} } \right)} \right]} \right\}dx_{0} dy_{0} } ,} \\ \end{aligned}$$

(6)

where

$$C_{0} \left( {x,y,z} \right) = \frac{i}{\lambda B}\exp \left( { - ikz} \right)\exp \left[ { - \frac{ikD}{{2B}}\left( {x^{2} + y^{2} } \right)} \right].$$

(7)

By introducing the hard aperture functions

$$A_{{p_{1} }} \left( {x_{0} ,y_{0} } \right) = \left\{ {\begin{array}{*{20}c} 1 & {\left| {x_{0} } \right| \le a_{x} ,\,\left| {y_{0} } \right| \le a_{y} } \\ 0 & {\left| {x_{0} } \right| > a_{x} ,\,\left| {y_{0} } \right| > a_{y} } \\ \end{array} } \right.,$$

(8a)

and.

$$A_{{p_{2} }} \left( {x_{0} ,y_{0} } \right) = \left\{ {\begin{array}{*{20}c} 1 & {\left| {x_{0} } \right| \le b_{x} ,\,\left| {y_{0} } \right| \le b_{y} } \\ 0 & {\left| {x_{0} } \right| > b_{x} ,\,\left| {y_{0} } \right| > b_{y} } \\ \end{array} } \right.,$$

(8b)

Equation (6) can be written as

$$\begin{aligned} E\left( {x,y,z} \right) & = C_{0} \left( {x,y,z} \right)\int\limits_{ - \infty }^{ + \infty } {\int\limits_{ - \infty }^{ + \infty } {A_{{p_{1} }} \left( {x_{0} ,y_{0} } \right)E\left( {x_{0} ,y_{0} ,z = 0} \right)\exp \left\{ { - \frac{ik}{{2B}}\left[ {A\left( {x_{0}^{2} + y_{0}^{2} } \right) - 2\left( {xx_{0} + yy_{0} } \right)} \right]} \right\}dx_{0} dy_{0} } } \\ & \quad - C_{0} \left( {x,y,z} \right)\int\limits_{ - \infty }^{ + \infty } {\int\limits_{ - \infty }^{ + \infty } {A_{{p_{2} }} \left( {x_{0} ,y_{0} } \right)E\left( {x_{0} ,y_{0} ,z = 0} \right)\exp \left\{ { - \frac{ik}{{2B}}\left[ {A\left( {x_{0}^{2} + y_{0}^{2} } \right) - 2\left( {xx_{0} + yy_{0} } \right)} \right]} \right\}dx_{0} dy_{0} } } . \\ \end{aligned}$$

(9)

As is known, the hard aperture functions $A_{{p_{1} }} \left( {x_{0} ,y_{0} } \right)$ and $A_{{p_{2} }} \left( {x_{0} ,y_{0} } \right)$ can be approximately expanded, respectively, into a finite sum of complex Gaussian functions (Wen and Breazeale 1998) as.

$$A_{{p_{1} }} \left( {x_{0} ,y_{0} } \right) = \sum\limits_{{h_{1} = 1}}^{N} {A_{{h_{1} }} \exp \left( { - \frac{{B_{{h_{1} }} x_{0}^{2} }}{{a_{{x_{0} }}^{2} }}} \right)} \sum\limits_{{g_{1} = 1}}^{N} {A_{{g_{1} }} \exp \left( { - \frac{{B_{{g_{1} }} y_{0}^{2} }}{{a_{{y_{0} }}^{2} }}} \right)} ,$$

(10)

and.

$$A_{{p_{2} }} \left( {x_{0} ,y_{0} } \right) = \sum\limits_{{h_{2} = 1}}^{N} {A_{{h_{2} }} \exp \left( { - \frac{{B_{{h_{2} }} x_{0}^{2} }}{{b_{{x_{0} }}^{2} }}} \right)} \sum\limits_{{g_{2} = 1}}^{N} {A_{{g_{2} }} \exp \left( { - \frac{{B_{{g_{2} }} y_{0}^{2} }}{{b_{{y_{0} }}^{2} }}} \right)} .$$

(11)

$A_{{h_{i} ,g_{i} }}$ and $B_{{h_{i} ,g_{i} }}$$\left( {i = 1,2} \right)$ denote the expansion and Gaussian coefficients, respectively, which could be obtained by optimization-computation directly (Wen and Breazeale 1998).

By inserting Eqs. (10) and (11) into Eq. (9), we obtain

$$\begin{gathered} E\left( {x,y,z} \right) = C_{0} \left( {x,y,z} \right)\int\limits_{ - \infty }^{ + \infty } {\int\limits_{ - \infty }^{ + \infty } {W_{\alpha ,\beta } \left( { - \frac{{x_{0} }}{{\omega_{0} }}} \right)\exp \left( {\frac{{a_{0} x_{0} }}{{\omega_{0} }}} \right)W_{\alpha ,\beta } \left( { - \frac{{y_{0} }}{{\omega_{0} }}} \right)\exp \left( {\frac{{a_{0} y_{0} }}{{\omega_{0} }}} \right)} } \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \sum\limits_{{h_{1} = 1}}^{N} {A_{{h_{1} }} \exp \left( { - \frac{{B_{{h_{1} }} x_{0}^{2} }}{{a_{x}^{2} }}} \right)} \sum\limits_{{g_{1} = 1}}^{N} {A_{{g_{1} }} \exp \left( { - \frac{{B_{{g_{1} }} y_{0}^{2} }}{{a_{y}^{2} }}} \right)} \exp \left\{ { - \frac{ik}{{2B}}\left[ {A\left( {x_{0}^{2} + y_{0}^{2} } \right) - 2\left( {xx_{0} + yy_{0} } \right)} \right]} \right\}dx_{0} dy_{0} \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, - C_{0} \left( {x,y,z} \right)\int\limits_{ - \infty }^{ + \infty } {\int\limits_{ - \infty }^{ + \infty } {W_{\alpha ,\beta } \left( { - \frac{{x_{0} }}{{\omega_{0} }}} \right)\exp \left( {\frac{{a_{0} x_{0} }}{{\omega_{0} }}} \right)W_{\alpha ,\beta } \left( { - \frac{{y_{0} }}{{\omega_{0} }}} \right)\exp \left( {\frac{{a_{0} y_{0} }}{{\omega_{0} }}} \right)} } \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \sum\limits_{{h_{2} = 1}}^{N} {A_{{h_{2} }} \exp \left( { - \frac{{B_{{h_{2} }} x_{0}^{2} }}{{b_{x}^{2} }}} \right)} \sum\limits_{{g_{2} = 1}}^{N} {A_{{g_{2} }} \exp \left( { - \frac{{B_{{g_{2} }} y_{0}^{2} }}{{b_{y}^{2} }}} \right)} \exp \left\{ { - \frac{ik}{{2B}}\left[ {A\left( {x_{0}^{2} + y_{0}^{2} } \right) - 2\left( {xx_{0} + yy_{0} } \right)} \right]} \right\}dx_{0} dy_{0} . \hfill \\ \end{gathered}$$

(12)

Equation (12) can be rearranged by using the variable separation method to give

$$\begin{gathered} E\left( {x,y,z} \right) = C_{0} \left( {x,y,z} \right)\sum\limits_{{n_{1} = 0}}^{ + \infty } {\frac{{\left( { - 1} \right)^{{n_{1} }} }}{{n_{1} !\omega_{0}^{{n_{1} }} a_{\alpha ,\beta } \left( {n_{1} } \right)}}\sum\limits_{{h_{1} = 1}}^{N} {A_{{h_{1} }} } } \int\limits_{ - \infty }^{ + \infty } {x_{0}^{{n_{1} }} \exp \left( { - u_{{h_{1} }} x_{0}^{2} + 2v_{x} x_{0} } \right)dx_{0} } \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \sum\limits_{{m_{1} = 0}}^{ + \infty } {\frac{{\left( { - 1} \right)^{{m_{1} }} }}{{m_{1} !\omega_{0}^{{m_{1} }} a_{\alpha ,\beta } \left( {m_{1} } \right)}}\sum\limits_{{g_{1} = 1}}^{N} {A_{{g_{1} }} } } \int\limits_{ - \infty }^{ + \infty } {y_{0}^{{m_{1} }} \exp \left( { - u_{{g_{1} }} x_{0}^{2} + 2v_{y} y_{0} } \right)dy_{0} } \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, - C_{0} \left( {x,y,z} \right)\sum\limits_{{n_{2} = 0}}^{ + \infty } {\frac{{\left( { - 1} \right)^{{n_{2} }} }}{{n_{2} !\omega_{0}^{{n_{2} }} a_{\alpha ,\beta } \left( {n_{2} } \right)}}\sum\limits_{{h_{2} = 1}}^{N} {A_{{h_{2} }} } } \int\limits_{ - \infty }^{ + \infty } {x_{0}^{{n_{2} }} \exp \left( { - u_{{h_{2} }} x_{0}^{2} + 2v_{x} x_{0} } \right)dx_{0} } \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \sum\limits_{{m_{2} = 0}}^{ + \infty } {\frac{{\left( { - 1} \right)^{{m_{2} }} }}{{m_{2} !\omega_{0}^{{m_{2} }} a_{\alpha ,\beta } \left( {m_{2} } \right)}}\sum\limits_{{g_{2} = 1}}^{N} {A_{{g_{2} }} } } \int\limits_{ - \infty }^{ + \infty } {y_{0}^{{m_{2} }} \exp \left( { - u_{{g_{2} }} x_{0}^{2} + 2v_{y} y_{0} } \right)dy_{0} } , \hfill \\ \end{gathered}$$

(13)

where

$$u_{{h_{1} }} = \frac{{B_{{h_{1} }} }}{{a_{x}^{2} }} + \frac{ikA}{{2B}},$$

(13a)

$$u_{{g_{1} }} = \frac{{B_{{g_{1} }} }}{{a_{y}^{2} }} + \frac{ikA}{{2B}},$$

(13b)

$$u_{{h_{2} }} = \frac{{B_{{h_{2} }} }}{{b_{x}^{2} }} + \frac{ikA}{{2B}},$$

(13c)

$$u_{{g_{2} }} = \frac{{B_{{g_{2} }} }}{{b_{y}^{2} }} + \frac{ikA}{{2B}},$$

(13d)

$$v_{x} = \frac{{a_{0} }}{{2\omega_{0} }} + \frac{ikx}{{2B}},$$

(13e)

and

$$v_{y} = \frac{{a_{0} }}{{2\omega_{0} }} + \frac{iky}{{2B}}.$$

(13f)

Recalling the following integral formula (Belafhal et al. 2020).

$$\int\limits_{ - \infty }^{ + \infty } {t^{n} } \exp \left( { - pt^{2} + 2qt} \right)dt = \exp \left( {\frac{{q^{2} }}{p}} \right)\sqrt {\frac{\pi }{p}} \left( {\frac{1}{2i\sqrt p }} \right)^{n} ,H_{n} \left( {\frac{iq}{{\sqrt p }}} \right),$$

(14)

with $H_{n} \left( . \right)$ is the Hermite polynomial of the order n, and after tedious but straight forward integral calculation, Eq. (13) yields

$$\begin{gathered} E\left( {x,y,z} \right) = \pi C_{0} \left( {x,y,z} \right)\sum\limits_{{n_{1} = 0}}^{ + \infty } {\frac{{\left( { - 1} \right)^{{n_{1} }} }}{{n_{1} !\left( {2i\omega_{0} } \right)^{{n_{1} }} a_{\alpha ,\beta } \left( {n_{1} } \right)}}\sum\limits_{{h_{1} = 1}}^{N} {A_{{h_{1} }} } } \frac{{e^{{{{v_{x}^{2} } \mathord{\left/ {\vphantom {{v_{x}^{2} } {u_{{h_{1} }} }}} \right. \kern-\nulldelimiterspace} {u_{{h_{1} }} }}}} }}{{\left( {\sqrt {u_{{h_{1} }} } } \right)^{{n_{1} + 1}} }}H_{{n_{1} }} \left( {\frac{{iv_{x} }}{{\sqrt {u_{{h_{1} }} } }}} \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \sum\limits_{{m_{1} = 0}}^{ + \infty } {\frac{{\left( { - 1} \right)^{{m_{1} }} }}{{m_{1} !\left( {2i\omega_{0} } \right)^{{m_{1} }} a_{\alpha ,\beta } \left( {m_{1} } \right)}}\sum\limits_{{g_{1} = 1}}^{N} {A_{{g_{1} }} } } \frac{{e^{{{{v_{y}^{2} } \mathord{\left/ {\vphantom {{v_{y}^{2} } {u_{{g_{1} }} }}} \right. \kern-\nulldelimiterspace} {u_{{g_{1} }} }}}} }}{{\left( {\sqrt {u_{{g_{1} }} } } \right)^{{m_{1} + 1}} }}H_{{m_{1} }} \left( {\frac{{iv_{y} }}{{\sqrt {u_{{g_{1} }} } }}} \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, - \pi C_{0} \left( {x,y,z} \right)\sum\limits_{{n_{2} = 0}}^{ + \infty } {\frac{{\left( { - 1} \right)^{{n_{2} }} }}{{n_{2} !\left( {2i\omega_{0} } \right)^{{n_{2} }} a_{\alpha ,\beta } \left( {n_{2} } \right)}}\sum\limits_{{h_{2} = 1}}^{N} {A_{{h_{2} }} } } \frac{{e^{{{{v_{x}^{2} } \mathord{\left/ {\vphantom {{v_{x}^{2} } {u_{h2} }}} \right. \kern-\nulldelimiterspace} {u_{h2} }}}} }}{{\left( {\sqrt {u_{{h_{2} }} } } \right)^{{n_{2} + 1}} }}H_{{n_{2} }} \left( {\frac{{iv_{x} }}{{\sqrt {u_{{h_{2} }} } }}} \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \sum\limits_{{m_{2} = 0}}^{ + \infty } {\frac{{\left( { - 1} \right)^{{m_{2} }} }}{{m_{2} !\left( {2i\omega_{0} } \right)^{{m_{2} }} a_{\alpha ,\beta } \left( {m_{2} } \right)}}\sum\limits_{{g_{2} = 1}}^{N} {A_{{g_{2} }} \frac{{e^{{{{v_{y}^{2} } \mathord{\left/ {\vphantom {{v_{y}^{2} } {u_{{g_{2} }} }}} \right. \kern-\nulldelimiterspace} {u_{{g_{2} }} }}}} }}{{\left( {\sqrt {u_{{g_{2} }} } } \right)^{{m_{2} + 1}} }}H_{{m_{2} }} \left( {\frac{{iv_{y} }}{{\sqrt {u_{{g_{2} }} } }}} \right)} } . \hfill \\ \end{gathered}$$

(15)

Equation (15) is the approximate analytical expression of a FWB propagating in a paraxial ABCD optical system limited by an annular aperture. This formula indicates that the output beam is a combination of decentred Hermite-Gaussian modes (with complex arguments), and depends on the initial beam parameters $\left( {a_{0} ,\omega_{0} } \right)$ and the optical system characteristics. By setting the appropriate values of α and β in Eq. (15), one may deduce the propagation equation for the auxiliary Wright beams, Mainardi beams, Airy beams and Hypergeometric beams.

2.3 Free space propagation

For the free space propagation, the ABCD matrix of the optical system is given as.

$${\kern 1pt} {\kern 1pt} \left( {\begin{array}{*{20}c} A & B \\ C & D \\ \end{array} } \right){\kern 1pt} {\kern 1pt} = {\kern 1pt} {\kern 1pt} \left( {\begin{array}{*{20}c} 1 & z \\ 0 & 1 \\ \end{array} } \right).$$

(16)

By substituting from Eq. (16) into Eq. (15), one can directly obtain the propagation formula of a FWB in free space as

$$\begin{gathered} E\left( {x,y,z} \right) = \pi C_{0} \left( {x,y,z} \right)\sum\limits_{{n_{1} = 0}}^{ + \infty } {\frac{{\left( { - 1} \right)^{{n_{1} }} }}{{n_{1} !\left( {2i\omega_{0} } \right)^{{n_{1} }} a_{\alpha ,\beta } \left( {n_{1} } \right)}}\sum\limits_{{h_{1} = 1}}^{N} {A_{{h_{1} }} } } \frac{{e^{{{{v_{x}^{2} } \mathord{\left/ {\vphantom {{v_{x}^{2} } {u_{{h_{1} }} }}} \right. \kern-\nulldelimiterspace} {u_{{h_{1} }} }}}} }}{{\left( {\sqrt {u_{{h_{1} }} } } \right)^{{n_{1} + 1}} }}H_{{n_{1} }} \left( {\frac{{iv_{x} }}{{\sqrt {u_{{h_{1} }} } }}} \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \sum\limits_{{m_{1} = 0}}^{ + \infty } {\frac{{\left( { - 1} \right)^{{m_{1} }} }}{{m_{1} !\left( {2i\omega_{0} } \right)^{{m_{1} }} a_{\alpha ,\beta } \left( {m_{1} } \right)}}\sum\limits_{{g_{1} = 1}}^{N} {A_{{g_{1} }} } } \frac{{e^{{{{v_{y}^{2} } \mathord{\left/ {\vphantom {{v_{y}^{2} } {u_{{g_{1} }} }}} \right. \kern-\nulldelimiterspace} {u_{{g_{1} }} }}}} }}{{\left( {\sqrt {u_{{g_{1} }} } } \right)^{{m_{1} + 1}} }}H_{{m_{1} }} \left( {\frac{{iv_{y} }}{{\sqrt {u_{{g_{1} }} } }}} \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, - \pi C_{0} \left( {x,y,z} \right)\sum\limits_{{n_{2} = 0}}^{ + \infty } {\frac{{\left( { - 1} \right)^{{n_{2} }} }}{{n_{2} !\left( {2i\omega_{0} } \right)^{{n_{2} }} a_{\alpha ,\beta } \left( {n_{2} } \right)}}\sum\limits_{{h_{2} = 1}}^{N} {A_{{h_{2} }} } } \frac{{e^{{{{v_{x}^{2} } \mathord{\left/ {\vphantom {{v_{x}^{2} } {u_{h2} }}} \right. \kern-\nulldelimiterspace} {u_{h2} }}}} }}{{\left( {\sqrt {u_{{h_{2} }} } } \right)^{{n_{2} + 1}} }}H_{{n_{2} }} \left( {\frac{{iv_{x} }}{{\sqrt {u_{{h_{2} }} } }}} \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \sum\limits_{{m_{2} = 0}}^{ + \infty } {\frac{{\left( { - 1} \right)^{{m_{2} }} }}{{m_{2} !\left( {2i\omega_{0} } \right)^{{m_{2} }} a_{\alpha ,\beta } \left( {m_{2} } \right)}}\sum\limits_{{g_{2} = 1}}^{N} {A_{{g_{2} }} \frac{{e^{{{{v_{y}^{2} } \mathord{\left/ {\vphantom {{v_{y}^{2} } {u_{{g_{2} }} }}} \right. \kern-\nulldelimiterspace} {u_{{g_{2} }} }}}} }}{{\left( {\sqrt {u_{{g_{2} }} } } \right)^{{m_{2} + 1}} }}H_{{m_{2} }} \left( {\frac{{iv_{y} }}{{\sqrt {u_{{g_{2} }} } }}} \right)} } , \hfill \\ \end{gathered}$$

(17)

where

$$C_{0} \left( {x,y,z} \right) = \frac{i}{\lambda z}\exp \left( { - ikz} \right)\exp \left[ { - \frac{ik}{{2z}}\left( {x^{2} + y^{2} } \right)} \right] ,$$

(17a)

$$u_{{h_{1} }} = \frac{{B_{{h_{1} }} }}{{a_{x}^{2} }} + \frac{ik}{{2z}} ,$$

(17b)

$$u_{{g_{1} }} = \frac{{B_{{g_{1} }} }}{{a_{y}^{2} }} + \frac{ik}{{2z}} ,$$

(17c)

$$u_{{h_{2} }} = \frac{{B_{{h_{2} }} }}{{b_{x}^{2} }} + \frac{ik}{{2z}} ,$$

(17d)

$$u_{{g_{2} }} = \frac{{B_{{g_{2} }} }}{{b_{y}^{2} }} + \frac{ik}{{2z}} ,$$

(17e)

$$v_{x} = \frac{{a_{0} }}{{2\omega_{0} }} + \frac{ikx}{{2z}} ,$$

(17f)

and

$$v_{y} = \frac{{a_{0} }}{{2\omega_{0} }} + \frac{iky}{{2z}}.$$

(17g)

2.4 Propagation in a FRFT system

The fractional Fourier transformation can be implemented optically by using Lohmann I, Lohman II systems, or a quadratic graded-index medium (Namias 1993; Mendolvic and Ozaktas 1993; Ozaktas and Mendolvic 1993; Lohmann et al. 1998). The Lohmann systems are equivalent, so we will consider hereafter the Lohmann I system (see Fig. 3).

Fig. 3

Schematic of Lohmann I setup

In Lohmann I setup, the focal length of the lens is $\frac{f}{\sin \left( \phi \right)}$, and the distance between the initial/receiver plane and the lens is $d = f{\kern 1pt} tg\left( {\phi /2} \right)$, where f is the standard focal length. The transfer matrix of the FRFT system is given by.

$$\left( \begin{gathered} A\,\,\,\,\,\,\,\,\,\,B \hfill \\ C\,\,\,\,\,\,\,\,\,\,D \hfill \\ \end{gathered} \right) = \left( \begin{gathered} \cos \phi \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,f\,\sin \phi \hfill \\ {{ - \sin \phi } \mathord{\left/ {\vphantom {{ - \sin \phi } f}} \right. \kern-\nulldelimiterspace} f}\,\,\,\,\,\,\,\,\cos \phi \hfill \\ \end{gathered} \right).$$

(18)

Conventionally, we put ${\kern 1pt} {\kern 1pt} \varphi = \,l\frac{\pi }{2}$, where l is called the order (or power) of the FRFT system.

It is obvious to note that if l takes odd values (i.e., l = 2 m + 1, m = 0, 1, 2…), the FRFT system will reduce to the standard Fourier transform system.

By substituting from Eq. (18) into Eq. (15), one can obtain the field expression of a FWB in apertured FRFT system of the form

$$\begin{gathered} E\left( {x,y,z} \right) = \pi C_{0} \left( {x,y,z} \right)\sum\limits_{{n_{1} = 0}}^{ + \infty } {\frac{{\left( { - 1} \right)^{{n_{1} }} }}{{n_{1} !\left( {2i\omega_{0} } \right)^{{n_{1} }} a_{\alpha ,\beta } \left( {n_{1} } \right)}}\sum\limits_{{h_{1} = 1}}^{N} {A_{{h_{1} }} } } \frac{{e^{{{{v_{x}^{2} } \mathord{\left/ {\vphantom {{v_{x}^{2} } {u_{{h_{1} }} }}} \right. \kern-\nulldelimiterspace} {u_{{h_{1} }} }}}} }}{{\left( {\sqrt {u_{{h_{1} }} } } \right)^{{n_{1} + 1}} }}H_{{n_{1} }} \left( {\frac{{iv_{x} }}{{\sqrt {u_{{h_{1} }} } }}} \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \sum\limits_{{m_{1} = 0}}^{ + \infty } {\frac{{\left( { - 1} \right)^{{m_{1} }} }}{{m_{1} !\left( {2i\omega_{0} } \right)^{{m_{1} }} a_{\alpha ,\beta } \left( {m_{1} } \right)}}\sum\limits_{{g_{1} = 1}}^{N} {A_{{g_{1} }} } } \frac{{e^{{{{v_{y}^{2} } \mathord{\left/ {\vphantom {{v_{y}^{2} } {u_{{g_{1} }} }}} \right. \kern-\nulldelimiterspace} {u_{{g_{1} }} }}}} }}{{\left( {\sqrt {u_{{g_{1} }} } } \right)^{{m_{1} + 1}} }}H_{{m_{1} }} \left( {\frac{{iv_{y} }}{{\sqrt {u_{{g_{1} }} } }}} \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, - \pi C_{0} \left( {x,y,z} \right)\sum\limits_{{n_{2} = 0}}^{ + \infty } {\frac{{\left( { - 1} \right)^{{n_{2} }} }}{{n_{2} !\left( {2i\omega_{0} } \right)^{{n_{2} }} a_{\alpha ,\beta } \left( {n_{2} } \right)}}\sum\limits_{{h_{2} = 1}}^{N} {A_{{h_{2} }} } } \frac{{e^{{{{v_{x}^{2} } \mathord{\left/ {\vphantom {{v_{x}^{2} } {u_{h2} }}} \right. \kern-\nulldelimiterspace} {u_{h2} }}}} }}{{\left( {\sqrt {u_{{h_{2} }} } } \right)^{{n_{2} + 1}} }}H_{{n_{2} }} \left( {\frac{{iv_{x} }}{{\sqrt {u_{{h_{2} }} } }}} \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \sum\limits_{{m_{2} = 0}}^{ + \infty } {\frac{{\left( { - 1} \right)^{{m_{2} }} }}{{m_{2} !\left( {2i\omega_{0} } \right)^{{m_{2} }} a_{\alpha ,\beta } \left( {m_{2} } \right)}}\sum\limits_{{g_{2} = 1}}^{N} {A_{{g_{2} }} \frac{{e^{{{{v_{y}^{2} } \mathord{\left/ {\vphantom {{v_{y}^{2} } {u_{{g_{2} }} }}} \right. \kern-\nulldelimiterspace} {u_{{g_{2} }} }}}} }}{{\left( {\sqrt {u_{{g_{2} }} } } \right)^{{m_{2} + 1}} }}H_{{m_{2} }} \left( {\frac{{iv_{y} }}{{\sqrt {u_{{g_{2} }} } }}} \right)} } , \hfill \\ \end{gathered}$$

(19)

where

$$C_{0} \left( {x,y,z} \right) = \frac{i}{\lambda f\sin \varphi }\exp \left( { - ikz} \right)\exp \left[ { - \frac{ik\cos \varphi }{{2f\sin \varphi }}\left( {x^{2} + y^{2} } \right)} \right],$$

(19a)

$$u_{{h_{1} }} = \frac{{B_{{h_{1} }} }}{{a_{x}^{2} }} + \frac{ik\cos \phi }{{2f\sin \phi }} ,$$

(19b)

$$u_{{g_{1} }} = \frac{{B_{{g_{1} }} }}{{a_{y}^{2} }} + \frac{ik\cos \phi }{{2f\sin \phi }} ,$$

(19c)

$$u_{{h_{2} }} = \frac{{B_{{h_{2} }} }}{{b_{x}^{2} }} + \frac{ik\cos \phi }{{2f\sin \phi }} ,$$

(19d)

$$u_{{g_{2} }} = \frac{{B_{{g_{2} }} }}{{b_{y}^{2} }} + \frac{ik\cos \phi }{{2f\sin \phi }} ,$$

(19e)

$$v_{x} = \frac{{a_{0} }}{{2\omega_{0} }} + \frac{ikx}{{2f\sin \phi }} ,$$

(19f)

and.

$$v_{y} = \frac{{a_{0} }}{{2\omega_{0} }} + \frac{iky}{{2f\sin \phi }}..$$

(19g)

3 Numerical results and discussion

To illustrate the paraxial propagation of a FWB, we present in Figs. 4, 5, 6 and 7 some typical numerical calculations of the intensity evolutions in free space and FRFT system as well based on the formulas derived above. For convenience, we assume that the annular aperture has a squared form, i.e., $a_{x} = a_{y} = a$ and $b_{x} = b_{y} = b$. In the following simulations, the calculation parameters (otherwise it is indicated) are set as $\lambda = 632.8\,{\text{nm}}$, $\omega_{0} = 0.8\,{\text{mm}}$, $a_{0} = 0.01$, $\alpha = - 0.34$ and $\beta = 0.84$.

To study the propagation properties of a FWB in free space, we depicted in Fig. 4 the intensity distribution of the beam at different propagation distances for $b = 0.5\,{\text{mm}}$ and $a = 10\,{\text{mm}}$.

Fig. 4

Normalized intensity distribution of FWB passing through a square annular aperture (with a = 10 mm and b = 0.5 mm) in free space at several propagation distances $z$: a$z = 200\,{\text{mm}}$, b$z = 600\,{\text{mm}}$, c $z = 1000\,{\text{mm}}$ and d$z = 2500\,{\text{mm}}$

As can be seen from Fig. 4, the profile of the beam is almost Airy-like; it consists of a central lobe and two side lobes along the x- and y-axis. The beam profile keeps invariant upon propagation in free space. However, one can note that the main lobe is slightly deformed as the propagation distance increases from $z = 600\,{\text{mm}}$.

Figure 5 illustrates the contour graph of the intensity distribution for a FWB propagating in free space for different values of the internal dimension $b$ and fixed value of the external dimension ($a = 10\,{\text{mm}}$) at the plane $z = 200\,{\text{mm}}$.

Fig. 5

Normalized intensity distribution of FWB passing through a square annular aperture (with $a = 10\,{\text{mm}}$) in free space at z = 200 mm for different values of $b$: a $b = 0.01$, b $b = 3$, c $b = 4$ d $b = 6$, e$b = 8$ and f $b = 9$

It is seen from the figure that, for a very small value of the internal size $b$ the beam keeps its initial shape unchanged. As the value $b$ is increased, the central lobe disappears in the first instance, and afterward, the secondary lobes close to the beam centre are gradually suppressed, and the energy repartition of the beam is modified. Further, in Fig. 6, we depicted the intensity distribution of the FWB in free space with different external size values and a fixed value of b ($b = 3\,{\text{mm}}$). The other parameters are the same as in Fig. 5. From Fig. 6 one can see that when the a value is small the FWB profile blurs, and by increasing gradually this parameter the FWB retrieves step by step its initial shape. By comparing Figs. 5 and 6, we observe that the effects of the variation of the internal and external dimensions on the evolution process of the intensity distribution in free space are opposite. It follows from the results obtained that the number of the side lobes, the presence or absence of the central lobe can be adjusted by selecting the appropriate dimensions (a and b) of the aperture.

Fig. 6

Normalized intensity distribution of FWB passing through a square annular aperture (with b = 3 mm) in free space for different values of $a$: a $a = 5$, b $a = 6$, c $a = 8$ and d $a = 10$

To study the evolution of the properties of a FWB in FRFT versus the fractional-order $l$, we have performed numerically the intensity distribution for different values of a and b. Through the numerical simulations, we deduced that the variation of the intensity distribution at the output plane versus the fractional-order l is periodic, and the value of the period is equal to 2. In the following, l is taken in the range between 0.1 and 1.9.

Figure 7 shows the intensity diagrams for a FWB at the FRFT plane for a = 10 mm, b = 0.01 mm, and f = 1000 mm. It is seen from the plots of Fig. 7 that: in the first half of the period, i.e. $0 < l \le 1$, the profile of the beam is invariant, and the beam energy is gradually focused towards the center as l is increased. It is found that at l = 1, the beam spot size reaches its minimum value. In the second half period (i.e., $1 < l \le 2$), the intensity distribution evolves in the opposite process of the one obtained in the first half period.

Fig. 7

Normalized intensity distribution of FWB passing through a square annular aperture (with a = 10 mm and b = 0.01 mm) in FRFT system with different fractional orders $l$: a $l = 0.1$, b $l = 0.3$, c $l = 0.5$, d $l = 1.5$, e $l = 1.7$ and f $l = 1.9$

At the plane l = 2 the beam retrieves its initial characteristics. One can also note that the localization of the beam spot change when l passes from the first half period to the second half period. The beam spot is in the third quadrant in the range $0 < l \le 1$, and it is located in the first quadrant when $1 < l \le 2$. The changing in the direction of the lobes can be explained physically by the changing of the sign of the terms A, B, C, and D (i.e. the quantities $\cos \phi \,$ and $\sin \phi$ in Eq. 18) with the variation of the power l of the FRFT. It is worth noting that, by setting $\alpha = - 1/3$ and $\beta = 1 + \alpha$, and multiplying the argument of the Wright function by a factor of $\sqrt[3]{3}$ Eq. (17), we readily find the results illustrated in Ref. (Ez-Zariy et al. 2014b).

We present in Fig. 8 the normalized intensity distribution of finite-Airy beam for different values $b$ by using the formula Eq. (17).

Fig. 8

Normalized intensity distribution of Finite-Airy passing through a square annular aperture (a = 10 mm) in free space for different values of $b$ a$b = 0.01$, b$b = 2$

As expected, we obtain the same result as that of Ref. (Ez-Zariy et al. 2014b). Hence, the aforementioned study becomes a special case of the current work. Note that, in our numerical simulations, we have evaluated the truncation error as equal to 2%.

4 Conclusion

In summary, we have introduced and investigated the paraxial propagation of the FWB. It is shown that the FWB is a general model which can be reduced by choosing specific values of the beam parameters to many known beams including Mainardi beam and Airy beams. The analytical expression of a FWB propagating through a rectangular aperture ABCD optical system with a hard annular aperture is derived based on the extended Huygens-Fresnel integral and the expansion of the aperture function into Gaussian functions. Numerical examples are given to illustrate the propagation properties of the beam in free space and through a FRFT system, the effect of the hard annular aperture characteristics has been analyzed, too. It is found that the FWB profile remains invariant but with a deformation of the main lobe, as it propagates from the source plan to another propagation distance. The results reveal that in the initial plane the profile of the FWB is an Airy-like beam but with a dark central spot. The number of the side lobes, the appearance or disappearance of the central lobe can be adjusted by selecting the appropriate dimensions aperture. The evolution of the intensity distribution at the FRFT plane is periodical versus the power of FRFT system and the period is equal to 2. Furthermore, the orientation and the size of the FWB lobes can be controlled by the power of the FRFT.

Appendix A

In the case of the Finite-Wright beam, we give some steps of the equation transformations from the propagation equation to the differential equation verified by the Wright function. In this appendix, we begun from the classical diffusion equation which is a time-fractional diffusion-wave equation.

We know that the time-fractional diffusion-wave equation is defined as (Mainardi 1995)

$$\frac{{\partial^{2\beta } u\left( {x,t} \right)}}{{\partial t^{2\beta } }} = D\frac{{\partial^{2} u\left( {x,t} \right)}}{{\partial x^{2} }},\,\,\,\,\,\,\,\,0 < \beta \le 1,\,\,\left| D \right| > 0,$$

(A-1)

where x and t are the space–time variables, and $u = u\left( {x,t} \right)$ is the wave function corresponding to the field, which is assumed to be a causal function of time, i.e., vanishing for $t < 0.$

As particular case, for $\beta = {1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}$, Eq. (A-1) reduces to the classical diffusion equation and it is introduced as

$$\frac{{\partial u\left( {x,t} \right)}}{\partial t} = D\frac{{\partial^{2} u\left( {x,t} \right)}}{{\partial x^{2} }}.$$

(A-2)

According to the main result of Ref. (Lipnevich and Luchko 2010), we find that the solution of Eq. A-2) can be given in terms of the Wright function and it is written as follows:

$u(x,t) = C_{1} t^{\gamma } W\left( { - \frac{1}{2},\,1 + \gamma ;\,\, - xt^{{ - {1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} } \right),$ with $C_{1}$ is arbitrary constant and $W\left( {\rho ,\alpha ;z} \right)$ is the Wright function. It is easy to show that Eq. (A-2) can be written, with $D = \frac{i\hbar }{{2m}},$ in the form: $\frac{{\partial u\left( {x,t} \right)}}{\partial t} = \frac{i\hbar }{{2m}}\frac{{\partial^{2} u\left( {x,t} \right)}}{{\partial x^{2} }}$. So, the Wright function is a solution of the Schrödinger equation: $i\hbar \frac{{\partial u\left( {x,t} \right)}}{\partial t} + \frac{{\hbar^{2} }}{2m}.\frac{{\partial^{2} u\left( {x,t} \right)}}{{\partial x^{2} }} = V(x).u\left( {x,t} \right)$ in the case of $V(x) = 0$. Finally, the Wright function evolves according to the potential-free Schrödinger equation and its evolution can be solved asymptotically by the use of an alternative efficient approach given by the classical Wentzel–Kramers–Brillouin method.

Note that the creation of the Finite-Wright beam is based on some theories developed early that show that the Wright function is a solution to the fractional wave-scattering equation, which corresponds, in the limiting case, to the Helmholtz equation.