1 Introduction

The vibration of a dynamical system motion can be reduced applying active controller or passive controller. The study of two-degree-of-freedom systems has not received much attention. The response of a two-degree-of-freedom system with quadratic coupling under a modulated amplitude sinusoidal excitation is investigated for different system motion [1, 2]. Nonlinear dynamics has many technical applications [2–4]. The stability properties, bifurcations, jump phenomena in systems, such as off-shore structures, railroad wheel sets, and aircraft are appeared in Refs. [5–10].

Kim et al. [11] investigated the resonances that arise from the synergistic effects of multi-frequency parametric excitation and single-frequency external excitation in a single-degree-of-freedom plate with a cubic nonlinearity, subjected to combine parametric and external excitations. Roy and Chatterjee [12] studied small vibrations of cantilever beams contacting a rigid surface, where the non-contacting length varies dynamically. It was indicated that the phase relationships of the external and each parametric excitation source have significant effect on the resulting response amplitude. El-Badawy and Nayfeh [13] used two simple control laws based on linear velocity and cubic velocity feedback to suppress the high-amplitude vibrations of a structural dynamic model of the twin-tail assembly of an F-15 fighter when subjected to primary resonance excitations. Eissa and Amer [14] controlled the vibration of a second-order system simulating the first mode of a cantilever beam subjected to primary and sub-harmonic resonance using cubic velocity feedback.

EL-Bassiouny [15] made an investigation into the control of the vibration of the crankshaft in internal-combustion engines subjected to both external and parametric excitations via an absorber having both quadratic and cubic stiffness nonlinearities. Belhaq and Houssni [16] investigated the control of chaos of the one-degree-of-freedom system with both quadratic and cubic nonlinearities subjected to combine parametric and external excitations. Several control methods leading to suppression of chaos have been presented. Sorokin and Ershov [17] applied active control to the resonant vibrations of a rectangular sandwich plate performed by the parametric stiffness modulation. Pai et al. [18] designed new nonlinear vibration absorbers using higher order internal resonances and saturation phenomena to suppress the steady-state vibrations of a linear cantilevered skew aluminum plate subjected to single external force. Higher order internal resonances are introduced using quadratic, cubic, and/or quartic terms to couple the controller with the plate. The displacement and velocity feedback signals are considered. Yaman and Sen [19] studied the problem of suppressing the vibrations of a nonlinear system with a cantilever beam of varying orientation subjected to parametric and direct excitation. They applied the cubic velocity feedback to the system to reduce the amplitudes of the system.

Lei et al. [20] applied an active control technique to coordinate a kind of two parametrically excited chaotic system. Oueini and Nayfeh [21] modeled the dynamics of the first mode of a cantilever beam with a second-order nonlinear ordinary differential equation subjected to a principal parametric excitation, and a control law based on cubic velocity feedback is introduced. Pai and Schulz [22] studied the control of the first mode vibration of a stainless steel beam through negative velocity feedback to the dynamic system. Amer and El-Sayed [23] investigated the nonlinear dynamics of a two-degree-of-freedom vibration system with nonlinear absorber when subjected to multi-external forces at primary and internal resonance with ratio 1:3. They reported that the steady-state amplitude of the main system is reduced to 2.5 % of its maximum value. Eissa et al. [24] and Amer et al. [25, 26] studied the nonlinear dynamics of two-degree-of-freedom vibrating system using multiple time scale perturbation up to the second-order approximation. They reported that how the active vibration control is effective at different resonance cases of the system.

In the present paper, second-order nonlinear differential equations of a dynamical system having quadratic coupling, quadratic, and cubic nonlinearities subjected to multi-parametric excitation forces is considered and solved. The method of multiple time scale perturbation [27] is applied to solve the nonlinear differential equations describing the controlled system up to second-order approximations. In this system, we added active controller via negative linear velocity feedback to the system. The behavior of the system is studied applying Runge–Kutta fourth-order method. The stability of the system is investigated applying both frequency response equations and phase-plane method. The resonance cases and effects of different parameters of the system are studied numerically. A comparison is made with the available published work.

2 Mathematical investigation

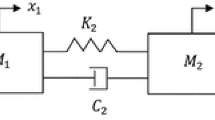
In this paper, we consider a nonlinear dynamical system subjected to multi-parametric excitations. The system can be written as

$$\begin{aligned}&\ddot{x}_1 +\omega _1^2 x_1 +2\varepsilon \mu _1 \dot{x}_1 +\varepsilon \alpha _1 x_1^3 +\varepsilon \alpha _2 x_1^2 +\varepsilon \alpha _3 x_1 x_2\nonumber \\&\quad =\varepsilon x_1 \sum _{j=1}^\mathrm{N} {F_j \cos j\Omega t} +R_1 ,\end{aligned}$$
(1)
$$\begin{aligned}&\ddot{x}_2 +\omega _2^2 x_2 +2\varepsilon \mu _2 \dot{x}_2 +\varepsilon \beta _1 x_2^3 +\varepsilon \beta _2 x_2^2\nonumber \\&\quad =\varepsilon x_2 \sum _{j=1}^\mathrm{N} {P_j \cos j\Omega t} +R_2 , \end{aligned}$$
(2)

where \(R_1 =-\varepsilon G_1 \dot{x}_1 \) and \(R_2 =-\varepsilon G_2 \dot{x}_2 \) are the control forces which added to the modes of the system.

Approximate solutions of nonlinear Eqs. (1) and (2) are obtained applying the multiple scales method, assuming \(x_{1}\) and \(x_{2}\) in the form

$$\begin{aligned} x_1 (\varepsilon ;t)&= x_{10} (T_0 ,T_1 )+\varepsilon x_{11} (T_0 ,T_1 )+O(\varepsilon ^{2}),\end{aligned}$$
(3)
$$\begin{aligned} x_2 (\varepsilon ;t)&= x_{20} (T_0 ,T_1 )+\varepsilon x_{21} (T_0 ,T_1 )+O(\varepsilon ^{2}), \end{aligned}$$
(4)

where \(T_{n}=\varepsilon ^{n}t\quad (n=0,1)\).

The derivatives will be in the form

$$\begin{aligned}&\frac{\mathrm{d}}{\mathrm{d}t}=D_0 +\varepsilon D_1 + \cdots ,\end{aligned}$$
(5)
$$\begin{aligned}&\frac{\mathrm{d}^{2}}{\mathrm{d}t^{2}}=D_0^2 +2\varepsilon D_0 D_1 +\cdots , \end{aligned}$$
(6)

where \(D_n =\frac{\partial }{\partial {T}_n },\quad n = 0, 1, 2\)

Substituting from Eqs. (3) and (4) into Eqs. (1) and (2) and equating the same power of \(\varepsilon \), we have

$$\begin{aligned}&\varepsilon ^{0}{:}\,(D_0^2 \!+\!\omega _1^2 )x_{10} \!=\!0,\end{aligned}$$
(7)
$$\begin{aligned}&(D_0^2 \!+\!\omega _2^2 )x_{20} \!=\!0, \end{aligned}$$
(8)
$$\begin{aligned}&\varepsilon ^{1}{:}\, (D_{0}^{2}\!+\!\omega _{1}^{2})x_{11}\!=\!-2D_{0}D_{1}x_{10}\!-\!2 \mu _{1}D_{0}x_{10}\!-\!\alpha _{1}x_{10}^{3}\nonumber \\&-\,\alpha _{2}x_{10}^{2}\!-\!\alpha _{3}x_{10}x_{20}\!+\!x_{10} \nonumber \\&\times \,\sum _{j=1}^\mathrm{N} {F_j \cos j\Omega t} \!-\!G_1 (D_0 x_{10} ),\end{aligned}$$
(9)
$$\begin{aligned}&(D_0^2 \!+\!\omega _2^2 )x_{21} \!=\!-2D_0 D_1 x_{20} \!-\!2\mu _2 D_0 x_{20}\!-\!\beta _1 x_{20}^3\nonumber \\&-\,\beta _2 x_{20}^2\!+\! x_{20}\sum _{j=1}^\mathrm{N} {P_j \cos j\Omega t} \nonumber \\&-\,G_2 (D_0 x_{20} ). \end{aligned}$$
(10)

The solutions of Eqs. (7) and (8) can be written in the form

$$\begin{aligned} x_{10} (T_0 , T_1 )&= A_{10} (T_1 )\exp (i\omega _1 T_0 )+\mathrm{cc} ,\end{aligned}$$
(11)
$$\begin{aligned} x_{20} (T_0 , T_1 )&= A_{20} (T_1 )\exp (i\omega _2 T_0 )+\mathrm{cc}, \end{aligned}$$
(12)

where \(A_{10}\) and \(A_{20}\) are complex functions in \(T_{1}\) and cc denotes the complex conjugate functions. Substituting Eqs. (11) and (12) into Eqs. (9) and (10), we get

$$\begin{aligned}&(D_0^2 \!+\!\omega _1^2 )x_{11} \!=\!-2 i\omega _{1}[(D_{1}A_{10})\exp (i\omega _{1}T_{0})]\nonumber \\&\quad -\,2{ i\omega }_{1} \mu _{1}[A_{10}\exp ({ i\omega }_{1}T_{0})]\!-\!\alpha _{1}[A_{10}^{3}\exp (3{ i\omega }_{1}T_{0})\nonumber \\&\quad +\,3A_{10}^2 \overline{A_{10} } \exp (i\omega _1 \mathrm{T}_0)]\!-\!\alpha _2 [A_{10}^2 \exp (2i\omega _1 \mathrm{T}_0 )\nonumber \\&\quad +\,A_{10} \overline{A_{10} } ]\!-\!\alpha _3 [A_{10} A_{20} \exp i(\omega _1\!+\!\omega _2 )\mathrm{T}_0\nonumber \\&\quad +\,A_{10} \overline{A_{20} } \exp i(\omega _1-\omega _2 )\mathrm{T}_0 ]\nonumber \\&\quad +\,\frac{A_{10} }{2}\sum _{j=1}^\mathrm{N} {F_j } \exp i(\omega _1 \!+\!j\Omega )\mathrm{T}_0\nonumber \\&\quad -\,i\omega _1 G_1 A_{10} \exp (i\omega _1 \mathrm{T}_0 )\!+\!\mathrm{cc},\quad \end{aligned}$$
(13)
$$\begin{aligned}&(D_0^2 \!+\!\omega _2^2 )x_{21} \!=\!-2i\omega _{2}[(D_{1}A_{20})\exp (i\omega _{2}T_{0})]\nonumber \\&\quad -\,2i\omega _{2}\mu _2[(A_{20})\exp (i\omega _{2}T_{0})]\!-\!\beta _{1}[A_{20}^{3}\exp (3 i\omega _{2}T_{0})\nonumber \\&\quad +\,3A_{20}^2 \overline{A_{20} } \exp (i\omega _2 \mathrm{T}_0 )]\!-\!\beta _2 [A_{20}^2 \exp (2i\omega _2 \mathrm{T}_0 )\nonumber \\&\quad +\,A_{20} \overline{A_{20} } ]\!+\!\frac{A_{20} }{2}\sum _{j=1}^\mathrm{N} {P_j } \exp i(\omega _2\!+\!j\Omega )\mathrm{T}_0\nonumber \\&\quad -\,i\omega _2 G_2 A_{20} \exp (i\omega _2 \mathrm{T}_0 )\!+\!\mathrm{cc}.\quad \end{aligned}$$
(14)

Eliminating the secular terms of Eqs. (13) and (14) to get bounded solutions, then the general solution of the resulting equations obtained as

$$\begin{aligned}&x_{11} \!=\!A_{11} \exp \left( {i\omega _1 \mathrm{T}_0 } \right) \!+\!\frac{\alpha _1 }{8\omega _1^2 }\left[ {A_{10}^3 \exp \left( {3i\omega _1 \mathrm{T}_0 } \right) } \right] \nonumber \\&\quad +\,\frac{\alpha _2 }{3\omega _1^2 }\left[ {A_{10}^2 \exp (2i\omega _1 \mathrm{T}_0 )} \right] \!-\!\frac{\alpha _3 }{(\omega _1^2 \!-\!(\omega _1 \!+\!\omega _2)^{2})}\nonumber \\&\quad \times \,\left[ {A_{10} A_{20} \exp i(\omega _1 \!+\!\omega _2 )\mathrm{T}_0} \right] \!-\!\frac{\alpha _3 }{(\omega _1^2 \!-\!(\omega _1 \!-\!\omega _2)^{2})}\nonumber \\&\quad \times \,\left[ {A_{10} \overline{A_{20} } \exp i(\omega _1 \!-\!\omega _2 )\mathrm{T}_0 } \right] \!+\!\frac{A_{10} }{2}\sum _{j=1}^3 \nonumber \\&\quad \times \,{F_j \frac{1}{(\omega _1^2 \!-\!(\omega _1 \!+\!j\Omega )^{2})}\left[ {\exp (i(\omega _1 \!+\!j\Omega )\mathrm{T}_0 )}\right] } \!+\! \mathrm{cc}, \nonumber \\\end{aligned}$$
(15)
$$\begin{aligned}&x_{21} \!=\!A_{21} \exp \left( {i\omega _2 \mathrm{T}_0 } \right) \!+\!\frac{\beta _1 }{8\omega _2^2 }\left[ {A_{20}^3\exp \left( {3i\omega _2 \mathrm{T}_0 } \right) } \right] \nonumber \\&\quad +\,\frac{\beta _2}{3\omega _2^2 }\left[ {A_{20}^2 \exp (2i\omega _2 \mathrm{T}_0 )}\right] \!+\!\frac{A_{20} }{2}\sum _{j=1}^3 \nonumber \\&\quad \times \,{P_j \frac{1}{(\omega _2^2 \!-\!(\omega _2 \!+\!j\Omega )^{2})}\left[ {\exp (i(\omega _2 \!+\!j\Omega )\mathrm{T}_0 )} \right] } \!+\!\mathrm{cc}. \nonumber \\ \end{aligned}$$
(16)

From Eqs. (3) and (4), the deduced resonance cases are:

  1. (i)

    Primary resonance: \(\Omega =\omega _{n}, \, n=1,2\)

  2. (ii)

    Sub-harmonic resonance: \(\Omega =2 \omega _{n}, \, n=1,2\)

  3. (iii)

    Super-harmonic resonance: \(\Omega =\frac{2}{3}\omega _{n} , \, n=1,2\)

  4. (iv)

    Internal resonance: \(\omega _{2}=r\omega _{1}, \, r~=~1,~2\)

  5. (iiv)

    Simultaneous resonance: Any combination of the above resonance cases is considered as simultaneous resonance.

3 Stability analysis

The stability of the controlled system is investigated at the worst resonance case (confirmed numerically), which is the simultaneous internal and super-harmonic resonance case where \(\omega _{2} \cong \omega _{1}, 3\Omega \cong 2\omega _{2}\).

Using the resonance conditions \(\omega _{2} \cong \omega _{1}+\varepsilon \sigma _{1}\) and \(3\Omega \cong 2 \omega _{2}+\varepsilon \sigma _{2}\), then \(3 \Omega =2 \omega _{1}+2\varepsilon \sigma _{1} + \varepsilon \sigma _{2}\), where \(\sigma _{1}\) and \(\sigma _{2}\) are called the detuning parameters. Eliminating the secular terms from the first approximations of Eqs. (13) and (14), we get the following:

$$\begin{aligned}&-2i\omega _1 D_1 A_{10} \!-\!2i\omega _1 \mu _1 A_{10} \!-\!3\alpha _1 A_{10}^2 \overline{A_{10} } \nonumber \\&\quad -\,i\omega _1 G_1 A_{10}\!+\!\frac{\overline{A_{10} } F_3 }{2}\exp i(2\sigma _1 \!+\!\sigma _2 )\mathrm{T}_1 \!=\! 0, \end{aligned}$$
(17)
$$\begin{aligned}&-2i\omega _2 D_1 A_{20} \!-\!2i\omega _2 \mu _2 A_{20} \!-\!3\beta _1 A_{20}^2 \overline{A_{20} } \nonumber \\&\quad -\,i\omega _2 G_2 A_{20}\!+\!\frac{\overline{A_{20} } P_3 }{2}\exp (i\sigma _2 \mathrm{T}_1 )\!=\!0. \end{aligned}$$
(18)

Using the polar form

$$\begin{aligned} A_{n0} =\frac{1}{2}a_n \exp (i\gamma _n ), \quad n=1,2, \end{aligned}$$
(19)

where \(a_{1,2}\) and \(\gamma _{1,2}\) are the steady-state amplitudes and phases of the motions, respectively. Substituting from Eq. (19) into Eqs. (17) and (18) and equating imaginary and real parts, we obtain

$$\begin{aligned}&{a}'_1 =-\mu _1 a_1 -\frac{1}{2}G_1 a_1 +\frac{F_3 }{4\omega _1 }a_1 \sin \theta _1 , \end{aligned}$$
(20)
$$\begin{aligned}&\frac{1}{2}a_1 \theta _1 ^{\prime }=-\frac{3\alpha _1 }{8\omega _1 }a_1^3 +\frac{F_3 }{4\omega _1 }a_1 \cos \theta _1 , \end{aligned}$$
(21)
$$\begin{aligned}&{a}'_2 =-\mu _2 a_2 -\frac{1}{2}G_2 a_2 +\frac{P_3 }{4\omega _2 }a_2 \sin \theta _2 , \end{aligned}$$
(22)
$$\begin{aligned}&\frac{1}{2}a_2 \theta _2 ^{\prime }=\frac{1}{2}a_2 \sigma _2 -\frac{3\beta _1 }{8\omega _2 }a_2^3 +\frac{P_3 }{4\omega _2 }a_2 \cos \theta _2 , \end{aligned}$$
(23)

where \(\theta _{1}=\sigma _{1}T_{1}+ \sigma _{2}T_{1}-2\gamma _{1}\) and \(\theta _{2}=\sigma _{2}T_{1}-2 \gamma _{2}\), then \(\theta _{1}^{\prime }=\sigma _{1}+\sigma _{2}-2 \gamma _{1}^{\prime }\) and \(\theta _{2}^{\prime }=\sigma _{2}-2\gamma _{2}^{\prime }\).

For steady-state solutions, we put \(a^{\prime }_{n}=\gamma ^{\prime }_{n}=0,\,n=1,2\) and the periodic solution at the fixed points corresponding to Eqs. (20)–(23) is given by

$$\begin{aligned}&-\mu _1 -\frac{1}{2}G_1 +\frac{F_3 }{4\omega _1 }\sin \theta _1 =0\end{aligned}$$
(24)
$$\begin{aligned}&\frac{1}{2}(\sigma _1 +\sigma _2 )-\frac{3\alpha _1 }{8\omega _1 }a_1^2 +\frac{F_3 }{4\omega _1 }\cos \theta _1 =0 , \end{aligned}$$
(25)
$$\begin{aligned}&-\mu _2 -\frac{1}{2}G_2 +\frac{P_3 }{4\omega _2 }\sin \theta _2 =0\end{aligned}$$
(26)
$$\begin{aligned}&\frac{1}{2}\sigma _2 -\frac{3\beta _1 }{8\omega _2 }a_2^2 +\frac{P_3 }{4\omega _2 }\cos \theta _2 =0 , \end{aligned}$$
(27)

From Eqs. (24)–(27), we have the following cases: (i) \(a_{1}\ne 0, \; a_{2}=0\), (ii) \(a_{2}\ne 0, \; a _{1}=0\), and (iii) \(a_{1}\ne 0, \; a_{2}\ne 0\).

For the practical (general) case \((a_{1}\ne 0, \; a_{2}\ne ~0)\), Squaring Eqs. (24) and (25), then adding the squared results together, similar to Eqs. (26) and (27) gives the following frequency response equations:

$$\begin{aligned}&\sigma _1^2 \!+\!\left[ 2\sigma _2 \!-\!\frac{3\alpha _1 }{2\omega _1 }a_1^2 ]\sigma _1 \!+\![4\mu _1^2 \!+\!G_1^2 \!+\!4\mu _1 G_1 \!+\!\sigma _2^2\right. \nonumber \\&\quad \left. +\frac{9\alpha _1^2 }{16\omega _1^2 }a_1^4 \!-\!\frac{3\alpha _1 }{2\omega _1 }\sigma _2 a_1^2 \!-\!\frac{1}{4\omega _1^2 }F_3^2 \right] \!=\!0 , \end{aligned}$$
(28)
$$\begin{aligned}&\sigma _2^2 +\left[ -\frac{3\beta _1 }{2\omega _2 }a_2^2 ]\sigma _2 +[4\mu _2^2 +G_2^2 +4\mu _2 G_2\right. \nonumber \\&\quad \left. +\frac{9\beta _1^2 }{16\omega _2^2 }a_2^4 -\frac{1}{4\omega _2^2 }P_3^2 \right] =0. \end{aligned}$$
(29)

To determine the stability of the fixed point solutions of Eqs. (24)–(27), we introduce the following forms:

$$\begin{aligned} A_{n0} =\frac{1}{2}(p_n -iq_n )\exp (i\sigma _n T_1 ), \quad n = 1, 2, \end{aligned}$$
(30)

where \(p_{1,2}\), \(q_{1,2}\) are real coefficients. The linearized form of Eqs. (17) and (18) are written as the following:

$$\begin{aligned}&-2i\omega _1 {A}'_{10} -2i\omega _1 \mu _1 A_{10} -i\omega _1 G_1 A_{10}\nonumber \\&\quad +\frac{\overline{A_{10} } F_3 }{2}\exp i(2\sigma _1 +\sigma _2 )\mathrm{T}_1 =0, \end{aligned}$$
(31)
$$\begin{aligned}&-2i\omega _2 {A}'_{20} -2i\omega _2 \mu _2 A_{20} -i\omega _2 G_2 A_{20}\nonumber \\&\quad +\frac{\overline{A_{20} } P_3 }{2}\exp i(\sigma _2 )\mathrm{T}_1 =0. \end{aligned}$$
(32)

Substituting from Eq. (30) into Eqs. (31) and (32) and equating the imaginary and real parts of Eqs. (31) and (32), we have

$$\begin{aligned}&{p}'_1 +(\mu _1 +G_1 /2)p_1 +(\sigma _1 )q_1 =0 ,\end{aligned}$$
(33)
$$\begin{aligned}&{q}'_1 +(-\sigma _1 )p_1 +(\mu _1 +G_1 /2)q_1 =0 , \end{aligned}$$
(34)
$$\begin{aligned}&{p}'_2 +(\mu _2 +G_2 /2)p_2 +(\sigma _2 )q_2 =0, \end{aligned}$$
(35)
$$\begin{aligned}&{q}'_2 +(-\sigma _2 )p_2 +(\mu _2 +G_2 /2)q_2 =0. \end{aligned}$$
(36)

The stability of a particular fixed point with respect to a proportional to \(\exp (\lambda T_{1})\) is determined by zeros of the characteristic equation:

$$\begin{aligned}&\!\!\!&\!\!\!\left| {\begin{array}{llll} (\lambda +\mu _1 + \frac{1}{2}G_1 )&{}\quad (\sigma _1 )&{}\quad 0&{}\quad 0 \\ -(\sigma _1 )&{}\quad (\lambda +\mu _1 + \frac{1}{2}G_1 )&{}\quad 0&{}\quad 0 \\ 0&{}\quad 0&{}\quad (\lambda +\mu _2 + \frac{1}{2}G_2 )&{}\quad (\sigma _2 ) \\ 0&{}\quad 0&{}\quad -(\sigma _2 )&{}\quad (\lambda +\mu _2 + \frac{1}{2}G_2 ) \end{array}} \right| =0 .&\!\!\! \end{aligned}$$
(37)

To analyze the stability of the non-trivial solution, one uses Eq. (37) to obtain

$$\begin{aligned} \lambda ^{4}+r_1 \lambda ^{3}+r_2 \lambda ^{2}+r_3 \lambda +r_4 =0, \end{aligned}$$
(38)

where

$$\begin{aligned} r_1&= 2(\mu _1 \!+\!\mu _2 )\!+\!G_1 \!+\!G_2 ,\nonumber \\ r_2&= \mu _1^2 \!+\!\mu _2^2 \!+\!\sigma _1^2 \!+\!\sigma _2^2 \!+\!\mu _1 G_1 \!+\!\mu _2 G_2\!+\!4\mu _1 \mu _2\nonumber \\&+\,2\mu _1 G_2 \!+\!2\mu _2 G_1 \!+\!G_1 G_2\!+\!\frac{1}{4}(G_1^2 \!+\!G_2^2 ),\nonumber \\ r_3&= 2(\mu _1^2 \mu _2 \!+\!\mu _2^2 \mu _1 \!+\!\mu _2 \sigma _1^2 \!+\!\mu _1 \sigma _2^2 )\!+\!\mu _1^2 G_2 \nonumber \\&+\,\mu _2^2 G_1\!+\!\frac{1}{2}(\mu _2 G_1^2 \!+\!\mu _1 G_2^2 )\!+\!\frac{1}{4}(G_1^2 G_2 \nonumber \\&+\,G_2^2 G_1 )\!+\!2\mu _1 \mu _2 (G_1 \!+\!G_2 )\!+\!G_1 G_2 (\mu _1 \!+\!\mu _2 )\nonumber \\&+\,G_1 \sigma _2^2\!+\!G_2 \sigma _1^2 , \nonumber \\ r_4&= \mu _1^2 \mu _2^2 \!+\!\frac{1}{4}(\mu _1^2 G_2^2 \!+\!\mu _2^2 G_1^2 \!+\!\mu _2 G_1^2 G_2 \!+\!\mu _1 G_2^2 G_1 \nonumber \\&+\,G_1^2 \sigma _2^2\!+\!G_2^2 \sigma _1^2 )\!+\!\mu _1^2 \mu _2 G_2 \!+\!\mu _2^2 \mu _1 G_1 \nonumber \\&+\,\mu _1^2 \sigma _2^2 \!+\!\mu _2^2 \sigma _1^2 \!+\!\mu _1 G_1 \sigma _2^2 \!+\!\mu _2 G_2 \sigma _1^2 \nonumber \\&+\,\mu _1 \mu _2 G_1 G_2\!+\!(G_1^2 G_2^2 /16)\!+\!\sigma _1^2 \sigma _2^2 .\nonumber \\ \end{aligned}$$

According to the Routh–Hurwitz criterion, the initial equilibrium solution (\(p_{1},\,q_{1},\,p_{2},\,q_{2})=~(0,~0,~0,~0)\) is stable if the following conditions: \(r_{1}\!>\!0, r_{1}r_{2}\!-\!r_{3} >\!0,r_{3}(r_{1}r_{2}\!-\!r_{3})\!-\!r_{1}^{2}r_{4}\!>\!0\), and \(r_{4}>0\) are satisfied, while these conditions are not satisfied, the initial equilibrium solution is unstable and bifurcations may occur.

4 Results and discussion

The Rung–Kutta fourth-order method has been applied to determine the numerical solution of the given system. Figure 1 shows the non-resonant system behavior. It can be seen that the maximum steady-state amplitude of \(x_{1}\) and \(x_{2}\) are about 65 and 60 % of excitation forces \(F_{1}\) and \(P_{1}\), respectively, this case can be regarded as a basic case.

Fig. 1
figure 1

Non-resonant time response solution at selected values: \(\mu _1 =0.5, \mu _2 =0.6, \alpha _1 =2.4, \alpha _2 =3.5, \alpha _3 =1.0, \beta _1 =1.4, \beta _2 =3.5, \omega _1 =1.24, \omega _2 =1.52, \Omega =3.23, F_1 =P_1 =1.4, F_2 =P_2 =1.5,\) and \(F_3 =P_3 =2.25 \)

Full size image

4.1 Resonance cases

Table  1 shows the results of some of the worst resonance conditions. It describes the effect of the different worst resonance cases of the system before and after controller. The worst resonance case of the system is the simultaneous internal and super-harmonic resonance case where \(\omega _{2} \cong \omega _{1}\) and \(3\Omega \cong ~2\omega _{2}\). Figure 2 shows the worst resonance case behavior of the system without controller and with controller. We recognized that the amplitudes of the worst resonance of the system eliminate the vibration. In this table, we applied active control (negative linear velocity feedback) to all resonance cases and we found that the amplitudes of the system are eliminated. The effectiveness of the controller is determined from the relation (\(E_\mathrm{a}\) = steady-state amplitude of the system before controller/steady-state amplitude of the system after controller), as shown in Table 1.

Fig. 2
figure 2

Simultaneous internal and super-harmonic resonance case, \(\omega _2 \cong \omega _1 \) and \( 3\Omega \cong 2 \omega _{2}\). a System without controller. b System with negative linear velocity feedback controller

Full size image
Table 1 Summary of the worst resonance cases
Full size table

4.2 Effects of different parameters

The frequency response equations (28) and (29) are nonlinear algebraic equations of \(a_{1}\) against \(\sigma _{1}\) and \(a_{2}\) against \(\sigma _{2}\). These equations solved numerically as shown in Figs. 3 and 4. Some of these figures are bent to the right and the others bent to the left. This bending leads to multi-valued solutions and jump phenomenon. We discuss the practical case, \( a_{1} \ne 0\) and \(a_{2}\ne 0 \) as the following: Fig. 3, shows that the steady-state amplitude \(a_{1}\) of the system is monotonic increasing to the linear natural frequency of roll mode \(\omega _{1}\), the excitation coefficient of first mode \(F_{3}\) and decreasing in the nonlinear parameter \(\alpha _{1}\), the linear damping coefficient \(\mu _{1}\), and the gain \(G_{1}\). Also, for the nonlinear parameter \(\alpha _{1} >0\) the steady-state amplitude \(a_{1}\) is shifted to the right indicating hardening-type nonlinearity, but for \(\alpha _{1}<0\) the steady-state amplitude \(a_{1}\) is shifted to the left indicating softening-type nonlinearity. Furthermore, the steady-state amplitude \(a_{2}\) of the system is monotonic increasing to the linear natural frequency of pitch mode \(\omega _{2}\), the excitation coefficient of second mode \(P_{3}\) and decreasing in the nonlinear parameter \(\beta _{1}\), the linear damping coefficient \(\mu _{2}\), and the gain \(G_{2}\). Also, for the nonlinear parameter \(\beta _{1}>0\) the steady-state amplitude \(a_{2}\) is shifted to the right indicating hardening-type nonlinearity, but for \(\beta _{1}<0\) the steady-state amplitude \(a_{2}\) is shifted to the left indicating softening-type nonlinearity as appeared in Fig. 4.

Fig. 3
figure 3

Theoretical frequency response curves \(\mu _1 =0.5, \alpha _1 =2.4, \omega _1 =0.34, F_3 =2.25,\) and \(G_1 =1.4\)

Full size image
Fig. 4
figure 4

Theoretical frequency response curves \(\mu _2 =0.6, \beta _1 =1.4, \omega _2 =0.28, P_3 =2.25,\) and \(G_2 =1.75\)

Full size image

4.3 Vibration control

Figure 5 shows the effects of the gains on the modes of the system at the simultaneous internal and super-harmonic resonance case where \(\omega _{2} \cong \omega _{1}\) and \(3\Omega \cong \) \(2\omega _{2}\) We found that the amplitudes of the system are monotonic decreasing functions of the gains \(G_{1}\) and \(G_{2}\). The saturation occurs when \(G_{1}>0.9\) for the mode amplitude \(x_{1}\) and \(G_{1}>~0.15\) for the mode amplitude \(x_{2}\), \(G_{2}\ge 0.6\) for the mode amplitude \(x_{1}\) and \(G_{2}\ge 0.3\) for the mode amplitude \(x_{2}\). From all the results, we have a threshold value for the damping coefficients of the worst case of the system. The threshold value occurs at 0.65 for two amplitudes \(x_{1}\) and \(x_{2}\) as regarded in Fig. 6.

Fig. 5
figure 5

Effects of the gains

Full size image
Fig. 6
figure 6

Threshold values of damping coefficients at the worst resonance case of the system

Full size image

4.4 Comparison with available published work

In comparison with the previous work [14] controlled the vibration of a cantilever beam subjected to primary and sub-harmonic resonance using cubic velocity feedback. While in Ref. [21] introduced a control law based on cubic velocity feedback of the first mode of a cantilever beam with a second-order nonlinear ordinary differential equation subjected to a principal parametric excitation.

However, in this paper, the new is controlling the nonlinear two-degree-of-freedom model of a dynamical system, having quadratic coupling, quadratic, and cubic nonlinearities, subjected to multi-parametric excitation forces via a negative feedback velocity. This controller is the best one for the worst resonance case as it reduces the vibration dramatically. Multiple time scale perturbation method is useful to determine approximate solutions for the coupled differential equations describing the system up to second-order approximation.

5 Conclusions

The vibrations of a two–degree-of-freedom nonlinear differential equations having quadratic coupling, quadratic, and cubic nonlinearities, subjected to multi-parametric excitation forces controlled via a negative feedback velocity. Multiple time scale perturbation method is useful to determine approximate solutions for the coupled differential equations describing the system up to second-order approximation. The stability of the system is considered by both the frequency response equations and the phase-plane technique. The effects of the different parameters of the system are studied numerically. From the above study, the following may be concluded:

  1. 1.

    The worst resonance case of the system is the simultaneous internal and super-harmonic resonance case where \(\omega _{2}\cong \omega _{1}\) and \(3\Omega \cong 2\omega _{2}\).

  2. 2.

    Negative linear velocity feedback active controller is the best one for the worst resonance case as it reduces the vibration dramatically.

  3. 3.

    The effectiveness of the controller at the reported worst resonance case are about \(E_\mathrm{a}=100\) for the mode amplitude \(x_{1}\) and \(E_\mathrm{a}=200\) for the mode amplitude \(x_{2}\), respectively.

  4. 4.

    The steady-state amplitudes of both modes are monotonic increasing functions in the linear natural frequencies \(\omega _{1}\), \(\omega _{2}\), the excitation coefficient modes \(F_{3}\), \(P_{3}\), respectively, decreasing in the nonlinear parameters \(\alpha _{1}\), \(\beta _{1}\), the linear damping coefficients \(\mu _{1}\), \(\mu _{2}\), and the gains \(G_{1}\), \(G_{2}\).

  5. 5.

    Also, for the nonlinear parameters \(\alpha _{1}\), \(\beta _{1} >0\) steady-state amplitudes of both modes are shifted to the right indicating hardening-type nonlinearity, but for \(\alpha _{1}\), \(\beta _{1}< 0\) steady-state amplitudes of both modes are shifted to the left indicating softening-type nonlinearity.

  6. 6.

    The saturation occurs when \(G_{1}>0.9\) for the mode amplitude \(x_{1}\) and \(G_{1} > 0.15\) for the mode amplitude \(x_{2}\), \(G_{2} \ge 0.6\) for the mode amplitude \(x_{1}\) and \(G_{2} \ge 0.3\) for the mode amplitude \(x_{2}\).

  7. 7.

    The threshold value for the damping coefficients of the worst case of the system occurs at 0.65.