The Westcott convention converted in the extended version of the modified Høgdahl convention

Abstract

In k₀-NAA it is common practice to use the modified Høgdahl convention for the description of (n, γ) activation. This convention is based on the principle that the activation cross-section in the thermal neutron range is following a 1/v shape, which results in an activation rate is independent of the speed distribution of the neutron density. For some isotopes, this is not the case. We call these isotopes “non-1/v”. For these nuclides, the activation rate in k₀-NAA theory is described by the Westcott convention. Westcott uses a Maxwellian thermal neutron energy distribution comprised in a neutron temperature dependent “g”-factor. For the activation by epithermal neutrons a “s”-factor is defined. In this paper, the original Westcott convention is converted into an extension of the Høgdahl convention. The benefit is that Høgdahl’s flux parameters can be used. The epithermal flux description defined by Westcott is used to calculate extended Q_E’s with their appropriate effective resonance energies, E_r. Since Westcott flux descriptions are neutron temperature dependent, the Q_E’s and E_r’s are as well. All the data needed for the evaluation of concentrations for Lu, Eu and Yb based on their non-1/v nuclides using the standard Høgdahl flux parameters are presented.

Introduction

The use of so-called non-1/ nuclides in k₀-NAA [1] using the Westcott convention was always a little cumbersome and never fully adapted. Already in the beginning of the nineties of last century the Westcott convention [2] was introduced and converted in a modified version for use in the k₀-method [3]. Nevertheless, these nuclides were not routinely used in k₀-NAA. In an effort to make non-1/v nuclides easier to analyze, a simplified method was introduced at the k₀-Users Meeting in Budapest [4], g-factors were calculated [5] and even Q₀’s were described and calculated [6]. However, a straight forward derivation of Westcott’s formula into the commonly used Høgdahl convention was only possible based on Westcott’s 1970 revision of his paper [2], resulting in the “extended” Modified Høgdahl convention.

Theory

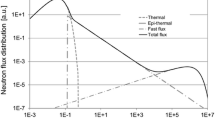
Westcott’s paper on “Effective Cross Section Values for Well-Moderated Thermal Reactor Spectra”, describes in the November 1970 version [2] how to handle nuclides that have a “non-1/v” activation cross-section in the thermal neutron energy region see Fig. 1. This paper is starting point to derive, in a straight forward way, the description in terms of the Høgdahl convention.

Fig. 1

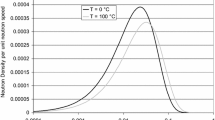
A Thermal and epithermal speed distribution of the neutron density according to Westcott and Høgdahl. B The relative activation cross section for the three most relevant non-1/v nuclides [7]

Without going into detail, Westcott described the thermal neutron density by one Maxwellian neutron density function and an epithermal 1/E neutron density function, limited at the lower energies using a cut-off function.

The reaction rate, R, the number of nuclei formed in a neutron flux (nv₀) per unit of time, is given by:

$$R = \mathop \int \limits_{0}^{\infty } n\left( v \right)\sigma \left( v \right)vdv = nv_{0} \hat{\sigma }$$

(1)

In the Westcott convention the effective cross section, $\hat{\sigma }$ is often used to describe the activation rate.

The effective cross section is defined by Westcott as:

$$\hat{\sigma } = \sigma_{0} \left( {g + rs} \right)$$

(2)

where $\sigma_{0}$ is the activation cross section at $v_{0}$(or 2200 m/s); g is called Westcott’s g-factor; s is called Westcott’s s-factor; s and g can be simply obtained by evaluating Eq. (1) using Westcott’s definition of the neutron spectrum, Eq. (3).

The speed distribution of the neutron density according to Westcott (Fig. 1A):

$$n\left( v \right) = \frac{4n}{\sqrt \pi }\left( {\left[ {1 - \frac{4r}{\sqrt \pi }\mathop \int \limits_{0}^{\infty } \frac{{v_{T} }}{{v^{2} }}\Delta dv} \right]\frac{{v^{2} }}{{v_{T}^{3} }}e^{{ - \frac{{v^{2} }}{{v_{T}^{2} }}}} + r\frac{{v_{T} }}{{v^{2} }}\Delta } \right)$$

(3)

where n is the total neutron density; v_T is the modal velocity of the Maxwellian distribution for temperature T,

$$v_{T} = v_{0} \sqrt {\frac{T}{{T_{0} }}} ,\quad {\text{with}}\;\;T_{0} = \, 293.6\,{\text{K}}$$

∆ is the epithermal neutron flux cut-off function; r is the epithermal index, if r = 0 the spectrum has a pure Maxwellian distribution.

Westcott described 4 different cut-off functions, see Fig. 2 and Table 1. Two of these functions exhibit a maximum at energies just above the cut-off energy in order to describe measurements of the neutron flux distribution from that time, he preferred actually only 2 and 4. The neutron temperature of the measured spectrum was around 60 °C, while the actual proposed application of his method was up too 600 °C, and higher. For k₀-NAA a temperature range between 0 and 100 °C will be sufficient.

Fig. 2

Cut-off functions as used by Westcott

Table 1 Cut-off functions and the related values for b and the equivalent cut-off energy μkT_n

The g-factor can be easily found by assuming r = 0, and combining Eqs. (1), (2) and (3), giving:

$$g = \frac{1}{{ v_{0} \sigma_{0} }} \mathop \int \limits_{0}^{\infty } \frac{4}{\sqrt \pi }\frac{{v^{2} }}{{v_{T}^{3} }}e^{{ - \frac{{v^{2} }}{{v_{T}^{2} }}}} \sigma \left( v \right)vdv$$

(4)

As can be seen, Westcott’s g-factor is only depending on the neutron temperature and activation cross section distribution. For most nuclides in the k₀-data base, g is very close to 1, see [1, 5] since the Høgdahl convention, is only valid for nuclides with a neutron (n,γ)-activation cross-section distribution close to 1/v in the thermal energy region. The most relevant non-1/v nuclides are Eu-151, Yb-168 and Lu-176, they exhibit extreme non-1/v behavior in the thermal energy region, see Fig. 1B.

In a similar way, one can derive Westcott’s s-factor from Eq. (3) by substituting,

$$b = \frac{4}{\sqrt \pi }\mathop \int \limits_{0}^{\infty } \left( {\frac{{v_{T} }}{{v^{2} }}} \right)\Delta dv$$

(5)

and

$$\varSigma = \mathop \int \limits_{0}^{\infty } \frac{\sigma \left( E \right)}{E}\Delta dE$$

(6)

we get

$$s = \sqrt {\frac{4T}{{\pi T_{0} }} } \frac{\sum }{{\sigma_{0 } }} - bg$$

(7)

By using this derivation for s, in Eq. (2) we get, identical with Westcott:

$$\hat{\sigma } = \sigma_{0} \left( {g + r\left( {\sqrt {\frac{4T}{{\pi T_{0} }}} \frac{\sum }{{\sigma_{0 } }} - bg} \right)} \right)$$

(8)

After separating the thermal and the epithermal part and some rearrangements this gives:

$$R \approx nv_{0} \hat{\sigma } = nv_{0} \sigma_{0} r\sqrt {\frac{4T}{{\pi T_{0} }}} \left( {\frac{1 - rb}{{r\sqrt {\frac{4T}{{\pi T_{0} }}} }}g + \frac{\sum }{{\sigma_{0 } }}} \right)$$

with

$$\phi_{epi} = nv_{0} r\sqrt {\frac{4T}{{\pi T_{0} }}} ,\quad \phi_{M} = nv_{0} \left( {1 - rb} \right),\quad Q_{E} = \frac{\sum }{{\sigma_{0 } }}\quad and\quad f_{M} = \phi_{M} /\phi_{epi}$$

this becomes

$$R \approx \sigma_{0} \phi_{epi} \left( {gf_{M} + Q_{E} } \right)$$

(9)

The result is a similar equation as used by the Høgdahl convention in which the epithermal and thermal part of the spectrum are split by the Cd cut-off energy, see Fig. 1. A 1 mm cadmium metal cover will absorb all energies lower than 0.55 eV. The Høgdahl convention is written as: $R \approx \sigma_{0} \phi_{epi} \left( {f + Q_{0} } \right)$. f is the thermal to epithermal flux ratio. In case of Westcott’s flux definition f is the Maxwellian thermal flux to epithermal flux ratio, or f_M. The resonance integral is now the integration of the whole epithermal flux described as ∆/E instead of the flux starting at the Cd-cut-off energy. Since for pure 1/v nuclides both Høgdahl and this extended Høgdahl convention should yield the same result (f + Q₀ = gf_M + Q_E) we can convert f into f_M, and since g = 1 (for 1/v) one can simply derive:

$$f - f_{M} = \mathop \int \limits_{0}^{\infty } \frac{{\Delta \sqrt {E_{0} } }}{{E^{{\frac{3}{2}}} }}dE - \mathop \int \limits_{{E_{Cd} }}^{\infty } \frac{{\sqrt {E_{0} } }}{{E^{{\frac{3}{2}}} }}dE$$

(10)

If we call $\varSigma$ the extended resonance integral, $\frac{\varSigma }{{\sigma_{0 } }}$ becomes the extended Q₀ or Q_E,i,T, which is now depending on neutron temperature and the type of cut-off function, $\Delta_{i}$.

Modified extended Høgdahl convention

The modified Høgdahl convention was derived to take into account a non-ideal epithermal neutron flux. The shape of the epithermal flux is described by 1/E whereas the real epithermal flux can be better described by 1/E^1+α. This makes Q₀ depending on α:

$$Q_{0} \left( \alpha \right) = \frac{1}{{\sigma_{0} }}\mathop \int \limits_{{E_{Cd} }}^{\infty } \frac{\sigma \left( E \right)}{{E^{1 + \alpha } }}dE$$

In a similar way the extended Q_E,Tn becomes

$$Q_{{{\text{E}},{\text{Tn}}}} \left( \alpha \right) = \frac{1}{{\sigma_{0} }}\mathop \int \limits_{0}^{\infty } \frac{\sigma \left( E \right)}{{E^{1 + \alpha } }}\Delta dE$$

(11)

The non-ideal epithermal flux will also affect the conversion from f to f_M, which will become:

$$f - f_{M} = \mathop \int \limits_{0}^{\infty } \frac{{\Delta \sqrt {E_{0} } }}{{E^{{\frac{3}{2} + \alpha}} }}dE - \mathop \int \limits_{{E_{Cd} }}^{\infty } \frac{{\sqrt {E_{0} } }}{{E^{{\frac{3}{2} + \alpha}} }}dE$$

(12)

We call this dQ, which is again depending on α, the cut-off function and the neutron temperature, $dQ_{{T_{N} }}$. Combined, this yields the modified extended Høgdahl convention:

$$R \approx \sigma_{0} \phi_{epi} \left( {g(T_{N} )\left( {f - dQ_{{T_{N} }} \left( \alpha \right)} \right) + Q_{{E,T_{N} }} \left( \alpha \right) } \right) .$$

(13)

Results

Modern nuclear cross section data libraries for (n,γ)-reactions as for instance ENDF/B-VII.1 [7] allow the calculation of the activation parameters, Q_E and g for k₀-NAA, by numerical integration using Eqs. (4) and (11). These calculations were done for extended Q_E,Tn(α)’s for Lu-176, Eu-151 and Yb-168 as a function of temperature in the range of 0–100 °C with an interval of 10, and α’s from − 0.1 to 0.3 with an interval of 0.01. And for all 4 cut-off functions as described by Westcott as well. In Fig. 3 the results are given as a function of α for these three nuclides at a neutron temperature of 20 °C and cut-off function Δ₄, dQ_E,Tn(α) is plotted as well.

Fig. 3

dQ and Q_E for Lu-176, Eu-151 and Yb-168 as a function of $\alpha$ for cut-off function Δ₄ and neutron temperature 20 °C, the thin lines are the fitted curves as explained in the text

Choice of cut-off function

Westcott’s preference was to use cut-off function 4 because the maximum in this function was a good representation of the expected epithermal flux measured at that time. From previous papers, he preferred function 2. The s-factors in his paper are actually only calculated for these two cut-off functions.

In this paper, we follow his preferences and compare the effect on the end result for the two functions. The calculations were done for flux ratio’s ranging from 10 to 1000, were for each f an appropriate α was chosen based on an empirical relation between f and α (α = 0.135 log(f) − 0.175). This relation is based on the reactor channel calibration data used for k₀-measurements by Simonits and DeCorte in the 70s and 80s [1].

The effect on the concentrations is of course maximal for the lowest f values, see Fig. 4. The largest effect is for Yb-168: 6% at 100 °C. For Eu-151 it is less than 2% and for Lu-176 it is less than 3%.

Fig. 4

Effect on the end result, as a function of f, if cut-off function 2 is used instead of 4 for a neutron temperature of A 0 °C and B 100 °C

The large effect for Yb-168 is caused by the position of maximum in cut-off function 4 which is moving up with neutron temperature and which is located around the strongest resonance peak, see Fig. 1B.

Concept of Q₀(α)

In the modified Høgdahl convention, Q₀(α) is analytically calculated by using an approximation formula which is based on the simplification that all resonances (the cross-section without 1/v-part) are assumed to be located in one single Dirac shaped resonance peak located at an effective resonance energy E_res, this leads to the following equation [6]:

$$Q_{0} \left( \alpha \right) = \frac{{Q_{0} - 0.429}}{{E_{res}^{\alpha } }} + \frac{0.429}{{(1 + 2\alpha ) 0.55^{\alpha } }}$$

(14)

By averaging the resonance energies from data tables the effective resonance energy is found. A drawback of this method is that the found energy is still depending on α. In case of non-1/v nuclides there is even a bigger problem because the lowest resonances are partly outside the epi-thermal spectrum, see Fig. 1.

Since we numerically integrated Q_E,i,T($\alpha$)’s and know their value as a function of α we can also fit this data using Eq. (14) and find an α independent resonance energy. This method was also used in [6] for the determination of resonance energies of all k₀-nuclides. See Fig. 3 for a graph of numerically integrated Q_E(α)’s for cut-off function 4 and a neutron temperature of 20 °C as a function of α and their best fit. The parameter needed to convert f into f_M, $dQ_{{{\text{i}},{\text{T}}}}$ can be treated in a similar but slightly different way, see Fig. 3, by using Eq. (15). There is no need to subtract the 1/v activation cross section above 0.55 eV because the value of dQ almost only determined by the energy region below 0.55 eV.

$$dQ_{i,Tn} \left( \alpha \right) = dQ_{i,Tn} \cdot E_{res,Tn,i}^{ - \alpha }$$

(15)

dQ_i,Tn and the extended Q_E,i,Tn’s for Eu-151, Lu-176, and Yb-168 with the related resonance energies are determined by fitting using Eqs. (14) and (15) for 11 neutron temperatures in the range of 0–100 °C, see Fig. 5 for ∆₄.

Fig. 5

A E_res and B Q_E versus temperature for cut-off function 4, for Eu-151, Yb-168 and Lu-176 and also for dQ (this work)

Temperature dependence

In Fig. 5E_res and Q_E are plotted for the three nuclides and dQ, for cut-off function 4.

As can be expected, both E_res and Q_E,i,Tn are depending on temperature. By fitting a second order polynomial (x = a + bT + cT², with T in °C) through the data points a very good regression quality r > 0.999 is obtained for both resonance energy and integral.

New data for Eu-151, Yb-168 and Lu-176

The parameters for the calculation of Q_E($\alpha ,T_{N}$), based on cut-off function 4 are presented in Table 2. The maximum deviation between numerically integrated values and fitted results in the temperature range between 0–100 °C and α’s between − 0.1 to 0.3 is less than 0.03 (absolute). The largest absolute differences are found for the extreme values of α’s (− 0.1 and 0.3) and highest temperature (100 °C). These differences are negligible considering the effect on concentrations and the uncertainty of the nuclear data, and similar to the deviations found when using traditionally averaged effective resonance energies. The data for the calculation of the appropriate g-factors [5] are given as well.

Table 2 All relevant temperature dependent parameters for the modified extended Westcott convention for Eu, Yb and Lu, based on the preferred cut-off function 4

Conclusion

Only based on Westcott’s neutron flux description the extended modified Høgdahl convention is presented, this new convention allows the use of the standard Høgdahl flux parameters. All nuclear data for the evaluation of concentrations using this new method for Lu, Eu and Yb based on their non-1/v nuclides are calculated and tabulated. Westcott described different cut-off functions of which we used only the one he preferred at that time. The concept of fitting resonance integrals and a spectrum correction term, as presented in this paper can also be used for different descriptions of cut-off functions and/or thermal neutron density distributions. Whereas the uncertainty in the analysis results greatly depends on the accuracy of the neutron temperature determination the effect of the choice of cut-off function is relatively small, but largest for Yb-168. The neutron temperature can be easily determined by iteration after irradiating and measuring an Au- and a Lu-comparator. The fact that this new approach is easy to implement, very straight forward to understand and will give exactly the same results as Westcott’s convention, will hopefully lead to a wider use, more experience and knowledge on the use of non-1/v nuclides for analytical purposes.