1 Introduction

Over the last few years, there has been a lot of interest in quantum analysis of atom-cavity coupled systems [1,2,3,4,5,6]. This is attributed to the generation of squeezed and entangled light when three-level atoms interact with cavity radiation, for example [7]. Squeezing, antibunching [8,9,10], and vacuum Rabi splitting [11,12,13] are all quantum properties observed when two-level atoms interact with radiation. The study of quantum well inside a microcavity has recently received a lot of attention because it is possible to reach the strong coupling regime in such systems. Besides understanding the basics of atom-radiance coupling in this research, it has interesting applications in optoelectronic devices [14, 15]. The spectrum of the exciton-cavity system contains two well-resolved peaks in the strong coupling regime (the coupling strength between the exciton and the photon is greater than the medium and cavity relaxation rates) [16, 17]. The strong coupling between the exciton and the photon raises the degeneracy and gives rise to the dressed state (cavity polaritons) [16, 18, 19]. Cavity polaritons are hybrid modes of photons and excitons. During mixing, the cavity photon and the semiconductor exciton exchange energy, resulting in Rabi splitting [20]. Because of their long-time coherence to regulate entanglement, cavity polaritons are proposed as a strong candidate in quantum information processing.

Moreover, the Rabi oscillation is experimentally realizable in a quantum well inside a microcavity [21]. As a result, numerous theoretical and experimental studies have been conducted. According to these findings, photon-exciton coupling causes vacuum Rabi splitting and Rabi oscillations in the semiconductor microcavity [22, 23]. In addition, research on normal mode oscillations in semiconductor microcavities shows that inhomogeneous broadening can significantly affect the energy exchange between photons and excitons [24].

Experiments and theories also showed that radiation from quantum wells in a microcavity exhibits squeezing [25,26,27,28]. Squeezing is characterized by the reduction of quantum fluctuation below the shot noise limit in one quadrature component of the field. Eyob et al [28] investigated the effect of squeezed light from a sub-threshold degenerate parametric oscillator in microcavity with semiconductor quantum well on the quantum properties of the cavity and output radiation of the fluorescent light in recent years. They demonstrated that the squeezed light from the parametric oscillation has a significant effect on the degree of squeezing of the output field.

The quantum statistical and quadrature squeezing properties of fluorescent light produced by a quantum well embedded in a microcavity are investigated in this paper. We consider a microcavity made of two Bragg reflecting mirrors separated by a wavelength-sized distance. Squeezed light from the reservoir is injected into a cavity through one of these mirrors. In the center of the microcavity, we placed a semiconductor quantum well. Because of the radiation in the microcavity, an electron from the filled valance band can be excited to the conduction band, resulting in the formation of a hole in the valance band.The exciton is formed as a result of the interaction of the hole and the electron. In the strong coupling regime, exciton-photon detuning reduces the intensity of the fluorescent light [29]. The effect of the squeezed vacuum reservoir and the initial cavity photons on the quantum properties of fluorescent light are presented here. The quantum Langevin equations for the cavity and exciton modes are determined using the reduced density operator. We investigate the intensity, intensity spectrum, quadrature squeezing, and second-order correlation function of the exciton mode using solutions to the quantum Langevin equation.

2 Model and the Quantum Langevin Equations

We investigate the non-classical properties of the excitonic mode emitted from a semiconductor quantum well in the microcavity in the linear excitation regime, where the density of the exciton is small enough to ignore exciton-exciton scattering, coupled to a one-mode squeezed vacuum reservoir. For low carrier density, the interaction between the semiconductor quantum well and the field in the microcavity can be described in the rotating wave and in the interaction picture by the Hamiltonian

$$\begin{aligned} \hat{H}_{I}=\Delta '\hat{b}^{\dagger }\hat{b}+ig(\hat{a}^{\dagger }\hat{b}-\hat{a}\hat{b}^{\dagger }), \end{aligned}$$
(1)

where \(\hat{a}\)(\(\hat{b}\)) are the annihilation operators for the photonic (excitonic) modes, g describes the coupling between the cavity and exciton fields, and \(\Delta '=\omega _{e}-\omega _{p}\) (with \(\omega _{e}\) (\(\omega _{p}\)) being the frequencies of the exciton (the photon) modes).

The master equation describing the interaction of the system, following the approach in [30], can be written as

$$\begin{aligned} \frac{d\hat{\rho }}{dt}= & {} -i[\Delta '\hat{b}^{\dagger }\hat{b},\hat{\rho }]+g[\hat{a}^{\dagger }\hat{b}-\hat{a}\hat{b}^{\dagger },\hat{\rho }] + \frac{1}{2}\kappa [N +1] (2\hat{a}\hat{\rho }\hat{a}^{\dagger }-\hat{a}^{\dagger }\hat{a}\hat{\rho }- \hat{\rho }\hat{a}^{\dagger }\hat{a})\nonumber \\{} & {} \!+ \frac{1}{2}\kappa N(2\hat{a}^{\dagger }\hat{\rho }\hat{a}\!-\hat{a}\hat{a}^{\dagger }\hat{\rho }\!- \hat{\rho }\hat{a}\hat{a}^{\dagger })\!+ \frac{\kappa }{2}M(2\hat{a}\hat{\rho }\hat{a}\!-\hat{a}^{2}\hat{\rho }\!-\hat{\rho }\hat{a}^{2}\!+2\hat{a}^{\dagger }\hat{\rho }\hat{a}^{\dagger }\!-\hat{a}^{\dagger 2}\hat{\rho }\!-\hat{\rho }\hat{a}^{\dagger 2}) \nonumber \\{} & {} + \frac{\gamma }{2}(2\hat{b}\hat{\rho }\hat{b}^{\dagger }-\hat{b}^{\dagger }\hat{b}\hat{\rho }-\hat{\rho }\hat{b}^{\dagger }\hat{b}), \end{aligned}$$
(2)

where \(\kappa \) is the rate of the cavity dissipation, \(\gamma \) is the spontaneous emission rate for exciton, \(N=sinh^{2}(r)\), and \(M=cosh(r) sinh(r)\) with r being the squeeze parameter. N and M represent the mean photon number and the phase correlation of the input squeezed light. The quantum Langevin equations that follow from this master equation are

$$\begin{aligned} \frac{d}{dt}\hat{a}= & {} -\frac{\kappa }{2}\hat{a}+g\hat{b}+\hat{F}_{c}(t), \end{aligned}$$
(3)
$$\begin{aligned} \frac{d}{dt}\hat{b}= & {} -(i\Delta '+\frac{\gamma }{2})\hat{b}-g\hat{a}+\hat{F}_{e}(t), \end{aligned}$$
(4)

in which \(\hat{F}_{c}(t)\) and \(\hat{F}_{e}(t)\) are the Langevin noise operators associated with the photonic and excitonic modes respectively. Since the photonic mode is damped by the squeezed vacuum reservoir, the Langevin noise operator for it has the following correlation properties:

$$\begin{aligned} \langle \hat{F}_{c}(t)\rangle= & {} \langle \hat{F}_{c}^{\dagger }(t)\rangle =0, \end{aligned}$$
(5)
$$\begin{aligned} \langle \hat{F}_{c}(t)\hat{F}_{c}(t')\rangle= & {} \langle \hat{F}_{c}^{\dagger }(t)\hat{F}_{c}^{\dagger }(t')\rangle =\kappa M\delta (t-t'), \end{aligned}$$
(6)
$$\begin{aligned} \langle \hat{F}_{c}(t)\hat{F}_{c}^{\dagger }(t')\rangle= & {} \kappa (N+1)\delta (t-t'),\end{aligned}$$
(7)
$$\begin{aligned} \langle \hat{F}_{c}^{\dagger }(t)\hat{F}_{c}(t')\rangle= & {} \kappa N\delta (t-t'), \end{aligned}$$
(8)

and the corresponding non-zero correlation property for the exciton Langevin noise operator is

$$\begin{aligned} \langle \hat{F}_{e}(t)\hat{F}_{e}^{\dagger }(t')\rangle =\gamma \delta (t-t'). \end{aligned}$$
(9)

We consider the case in which the cavity and the exciton frequencies are equal. The expressions in (3) and (4) can then be written in a single equation as

$$\begin{aligned} \frac{d}{dt}\hat{Y}(t)=-\frac{1}{2}m\hat{Y}(t) + \hat{F}(t), \end{aligned}$$
(10)

where

$$\begin{aligned} \hat{Y}(t)= & {} \begin{bmatrix} \hat{a}(t) \\ \hat{b}(t) \end{bmatrix} , \end{aligned}$$
(11)
$$\begin{aligned} m= & {} \begin{bmatrix} \kappa &{} -2g \\ 2g &{} \gamma \end{bmatrix} , \end{aligned}$$
(12)
$$\begin{aligned} \hat{F}(t)= & {} \begin{bmatrix} \hat{F}_{c}(t) \\ \hat{F}_{e}(t) \end{bmatrix} . \end{aligned}$$
(13)

We next seek to obtain the eigenvalues and eigenstates of the matrix m. To this end, the eigenvalue equation for the matrix m can also be written as

$$\begin{aligned} (m-\lambda I)X=0, \end{aligned}$$
(14)

where \(\hat{I}\) is the identity matrix, \(\lambda \) is the eigenvalue of the matrix m, and X is the eigenvector of the matrix m. This equation will have a non-trivial solution provided that the determinant of this equation is zero. It then leads to

$$\begin{aligned} \lambda _{1}= & {} \frac{\gamma _{+}}{2}+\frac{\Delta }{2},\end{aligned}$$
(15)
$$\begin{aligned} \lambda _{2}= & {} \frac{\gamma _{+}}{2}-\frac{\Delta }{2}, \end{aligned}$$
(16)

in which

$$\begin{aligned} \gamma _{\pm }=\kappa \pm \gamma \end{aligned}$$
(17)

and

$$\begin{aligned} \Delta =\sqrt{(\kappa -\gamma )^{2}-16g^{2}}. \end{aligned}$$
(18)

The corresponding eigenstates for the eigenvalues \(\lambda _{1}\) and \(\lambda _{2}\) are respectively given by

$$\begin{aligned} Y_{1}=\frac{1}{\sqrt{4g^{2}+(\frac{\gamma _{-}}{2}-\frac{\Delta }{2}})^{2}} \begin{bmatrix} 2g \\ \frac{\gamma _{-}}{2}-\frac{\Delta }{2} \end{bmatrix} \end{aligned}$$
(19)

and

$$\begin{aligned} Y_{2}=\frac{1}{\sqrt{4g^{2}+(\frac{\gamma _{-}}{2}+\frac{\Delta }{2}})^{2}} \begin{bmatrix} 2g \\ \frac{\gamma _{-}}{2}+\frac{\Delta }{2} \end{bmatrix} . \end{aligned}$$
(20)

Let us introduce a matrix V such that

$$\begin{aligned} V=(Y_{1},Y_{2}). \end{aligned}$$
(21)

It then follows that

$$\begin{aligned} V= \begin{bmatrix} \frac{2g}{\sqrt{4g^{2}+(\frac{\gamma _{-}}{2}-\frac{\Delta }{2}})^{2}} &{} \frac{2g}{\sqrt{4g^{2}+(\frac{\gamma _{-}}{2}+\frac{\Delta }{2}})^{2}} \\ \frac{\frac{\gamma _{-}}{2}-\frac{\Delta }{2}}{\sqrt{4g^{2}+(\frac{\gamma _{-}}{2}-\frac{\Delta }{2}})^{2}} &{} \frac{\frac{\gamma _{-}}{2}+\frac{\Delta }{2}}{\sqrt{4g^{2}+(\frac{\gamma _{-}}{2}+\frac{\Delta }{2}})^{2}} \end{bmatrix} \end{aligned}$$
(22)

and the inverse of this matrix is

$$\begin{aligned} V^{-1}=\frac{\sqrt{4g^{2}+(\frac{\gamma _{-}}{2}-\frac{\Delta }{2}})^{2}\sqrt{4g^{2}+(\frac{\gamma _{-}}{2}+\frac{\Delta }{2}})^{2}}{2g\Delta } \begin{bmatrix} \frac{\frac{\gamma _{-}}{2}+\frac{\Delta }{2}}{\sqrt{4g^{2}+(\frac{\gamma _{-}}{2}+\frac{\Delta }{2}})^{2}} &{} \frac{-2g}{\sqrt{4g^{2}+(\frac{\gamma _{-}}{2}+\frac{\Delta }{2}})^{2}} \\ \frac{-(\frac{\gamma _{-}}{2}-\frac{\Delta }{2})}{\sqrt{4g^{2}+(\frac{\gamma _{-}}{2}-\frac{\Delta }{2}})^{2}} &{} \frac{2g}{\sqrt{4g^{2}+(\frac{\gamma _{-}}{2}-\frac{\Delta }{2}})^{2}} \end{bmatrix}. \end{aligned}$$
(23)

One can put the expression in (10) using the relation \(VV^{-1}=1\) in the form

$$\begin{aligned} \frac{d}{dt}V^{-1}\hat{Y}(t)=-\frac{1}{2}V^{-1}VV^{-1}mVV^{-1}\hat{Y}(t) + V^{-1}\hat{F}(t). \end{aligned}$$
(24)

Or

$$\begin{aligned} \frac{d}{dt}V^{-1}\hat{Y}(t)=-\frac{1}{2}NV^{-1}\hat{Y}(t) + V^{-1}\hat{F}(t), \end{aligned}$$
(25)

where

$$\begin{aligned} N= \begin{bmatrix} \frac{\gamma _+}{2}+\frac{\Delta }{2} &{} 0 \\ 0 &{} \frac{\gamma _+}{2}-\frac{\Delta }{2} \end{bmatrix} . \end{aligned}$$
(26)

Now applying the solution of (25) along with (26), one can find

$$\begin{aligned} \hat{a}(t)=A(t)\hat{a}(0) +B(t)\hat{b}(0) +\int _{0}^{t}A(t-t')\hat{F}_{c}(t')dt' + \int _{0}^{t}B(t-t')\hat{F}_{e}(t'))dt', \end{aligned}$$
(27)
$$\begin{aligned} \hat{b}(t)=-B(t)\hat{a}(0) +D(t)\hat{b}(0) -\int _{0}^{t}B(t-t')\hat{F}_{c}(t')dt'+\int _{0}^{t}D(t-t')\hat{F}_{e}(t')dt', \end{aligned}$$
(28)

in which

$$\begin{aligned} A(t)= & {} \bigg ( cos(gt)+\frac{\gamma -\kappa }{4g}sin(gt)\bigg )e^{\gamma _+ t/4}, \end{aligned}$$
(29)
$$\begin{aligned} B(t)= & {} sin(gt)e^{-\gamma _+ t/4}, \end{aligned}$$
(30)
$$\begin{aligned} D(t)= & {} [cos(gt) + \frac{\kappa -\gamma }{4g}sin(gt)]e^{-\gamma _+t/4}. \end{aligned}$$
(31)

We study the dynamics of the exciton mode using the basic equation described in (28).

3 Intensity of the Exciton Mode

The mean photon number of the excitonic mode is expressible, applying (28), as

$$\begin{aligned} \langle \hat{b}^{\dagger }\hat{b}\rangle= & {} B^{2}(t)\langle \hat{a}^{\dagger }(0)\hat{a}(0)\rangle -B(t)D(t)\langle \hat{a}^{\dagger }(0)\hat{b}(0)\rangle + B(t)\int _{0}^{t}B(t-t')\langle \hat{a}^{\dagger }(0)\hat{F}_{c}(t')\rangle dt' \nonumber \\{} & {} - B(t)\int _{0}^{t}D(t-t')\langle \hat{a}^{\dagger }(0)\hat{F}_{e}(t')\rangle dt'-B(t)D(t)\langle \hat{b}^{\dagger }(0)\hat{a}(0)\rangle + D^{2}(t)\langle \hat{b}^{\dagger }(0)\hat{b}(0)\rangle \nonumber \\{} & {} - D(t)\int _{0}^{t}B(t-t')\langle \hat{b}^{\dagger }(0)\hat{F}_{c}(t')\rangle dt' +D(t)\int _{0}^{t}D(t-t')\langle \hat{b}^{\dagger }(0)\hat{F}_{e}(t')\rangle dt' \nonumber \\{} & {} + B(t)\int _{0}^{t}B(t-t'')\langle \hat{F}_{c}(t'')\hat{a}(0)\rangle dt'' - D(t)\int _{0}^{t}B(t-t'')\langle \hat{F}_{c}^{\dagger }(t'')\hat{b}(0)\rangle dt'' \nonumber \\{} & {} + \int _{0}^{t}\int _{0}^{t}B(t-t')B(t-t'')\langle \hat{F}_{c}^{\dagger }(t'')\hat{F}_{c}(t')\rangle dt' dt'' \nonumber \\{} & {} -\int _{0}^{t}\int _{0}^{t}B(t-t'')D(t-t')\langle \hat{F}_{c}^{\dagger }(t'')\hat{F}_{e}(t')\rangle dt' dt'' \nonumber \\{} & {} - B(t)\int _{0}^{t}D(t-t'')\langle \hat{F}_{e}^{\dagger }(t'')\hat{a}(0)\rangle dt'' + D(t)\int _{0}^{t}D(t-t'')\langle \hat{F}_{e}^{\dagger }(t'')\hat{b}(0)\rangle dt''\nonumber \\{} & {} - \int _{0}^{t}\int _{0}^{t}D(t-t'')B(t-t')\langle \hat{F}_{e}^{\dagger }(t'')\hat{F}_{c}(t')\rangle dt' dt'' \nonumber \\{} & {} + \int _{0}^{t}\int _{0}^{t}D(t-t'')D(t-t')\langle \hat{F}_{e}^{\dagger }(t'')\hat{F}_{e}(t')\rangle dt' dt'' \end{aligned}$$
(32)
Fig. 1
figure 1

Intensity \(\langle \hat{b}^{\dagger }\hat{b}\rangle \) (34) of the fluorescent light vs \(\gamma t\) for \(g/\gamma =4\), \(\gamma =\kappa \), \(N=1\), \(\bar{n}_{e}=1\), and for different values of \(\bar{n}_{c}\)

Full size image
Fig. 2
figure 2

Intensity \(\langle \hat{b}^{\dagger }\hat{b}\rangle \) (34) of the fluorescent light vs \(\gamma t\) for \(g/\gamma =4\), \(\gamma =\kappa \), \(\bar{n}_{c}=2\), \(\bar{n}_{e}=1\), and for different values of N

Full size image

Because the exciton and the cavity modes at the initial time are uncorrelated with the noise operators; and writing the coupled cavity-exciton initial state as \(|\Psi \rangle =|n,n_{e}\rangle \) for the cavity and exciton modes initially assumed to be respectively in a number state \(|n\rangle \) and \(|n_{e}\rangle \), the intensity of the exciton mode can be written with the aid of (8) as

$$\begin{aligned} \langle \hat{b}^{\dagger }\hat{b}\rangle= & {} B^{2}(t)\bar{n}_{c}+D^{2}(t)\bar{n}_{e} + \int _{0}^{t}\int _{0}^{t} B(t-t')B(t-t'')\kappa N \delta (t'-t'') dt' dt'', \end{aligned}$$
(33)

in which \(\bar{n}_{c}\) (\(\bar{n}_{e}\)) are the initial mean photon number of the cavity (exciton) modes. Now using (30) and (31) and on performing the integration, we get

$$\begin{aligned} \langle \hat{b}^{\dagger }\hat{b}\rangle= & {} sin^{2}(gt)e^{-\gamma _+ t/2}\bar{n}_{c}+ [cos(gt) + \frac{\kappa -\gamma }{4g}sin(gt)]^{2}e^{-\gamma _+t/2}\bar{n}_{e}\\{} & {} + \frac{\kappa Ne^{-\gamma _{+}t/2}}{16\gamma _{+}g^{2}+\gamma _{+}^{3}}\bigg [ 16g^{2}(e^{\gamma _{+}t/2}-1)+ \gamma _{+}^{2}(cos(2gt)-1)-4g\gamma _{+}sin(2gt)\bigg ].\nonumber \end{aligned}$$
(34)

We note from this expression that the intensity of the fluorescent light does not depend on the parameter M, which characterizes the phase correlation of the injected squeezed light. The steady-state solution of (34), using (17), is given by

$$\begin{aligned} \langle \hat{b}^{\dagger }\hat{b}\rangle _{ss}=\frac{16g^{2}\kappa N}{(\kappa +\gamma )(16g^{2}+(\kappa +\gamma )^{2})}. \end{aligned}$$
(35)

It is easy to see from this expression that the exciton relaxation rate leads to a decrease in the mean intensity of the fluorescent light. Moreover, Figures 1, 2, and 3 show the intensity of the exciton mode as a function of time to investigate how the intensity of the exciton mode depends on the initial cavity photon (\( \bar{n}_{c}\)), the mean photon number of the injected squeezed light (N), and the cavity-atom coupling strength (g), respectively when there is initially one exciton (\(\bar{n}_{e}=1\)) in the microcavity. All the three figures show that the intensity of the exciton mode oscillates with the Rabi frequency. The amplitude of these oscillations decrease with time due to cavity damping in all the three cases. These oscillations are caused by the exchange of energy between the photon and the exciton. Figure 1 also shows that the initial mean cavity photons influence the mean intensity of the exciton mode. It is clear that the initial mean cavity photon increases the mean intensity of the exciton mode. Moreover, we note that for \(\bar{n}_{c}>1\), the mean intensity of the exciton mode is above the curve \(\bar{n}_{c} =1\) and below the curve for \(\bar{n}_{c} <1\). Figure 2 depicts the relationship between the intensity of the exciton mode and the mean photon number of the injected squeezed light. This figure shows that the effect of the mean photon number of the injected squeezed light is less significant initially but becomes more significant as time passes. This is due to the fact that the number of photons coming in through the port mirror increases. In Fig. 3, we plot the intensity of the exciton mode as a function of time for various values of cavity-exciton coupling constant. In the weak coupling regime, the oscillatory nature of the intensity damps out due to the disappearance of excitation before the occurrence of the Rabi oscillation. However, in the strong coupling regime, an excitation is transferred back and forth between the photon and the exciton before it is lost in the environment. As a result, we see damped Rabi oscillations.

Fig. 3
figure 3

Intensity \(\langle \hat{b}^{\dagger }\hat{b}\rangle \) (34) of the fluorescent light vs \(\gamma t\) for \(\gamma =\kappa \), \(\bar{n}_{c}=2\), \(\bar{n}_{e}=1\), \(N=1\), and for different values of \(g/\gamma \)

Full size image

4 Intensity Spectrum

The intensity spectrum tells us how the intensity is spread over the frequency range. The intensity spectrum of the fluorescent light is expressible, at steady state, as

$$\begin{aligned} P(\omega )=\frac{1}{\pi }Re\int _{0}^{\infty } d\tau e^{i\omega '\tau }\frac{\langle \hat{b}^{\dagger }(t)\hat{b}(t+\tau )\rangle _{ss} }{\langle \hat{b}^{\dagger }(t)\hat{b}(t)\rangle _{ss}}, \end{aligned}$$
(36)

with \(\omega '=\omega -\omega _{o}\). We proceed to determine the two-time correlation function involved in this expression. To this end, one can write

$$\begin{aligned} \langle \hat{b}^{\dagger }(t)\hat{b}(t+\tau ) \rangle= & {} \langle [-B(t)\hat{a}^{\dagger }(0)+D(t)\hat{b}^{\dagger }(0)-\int _{0}^{t}B(t-t')\hat{F}_{c}^{\dagger }(t')dt'\nonumber \\{} & {} + \int _{0}^{t}D(t-t')\hat{F}_{e}^{\dagger }(t')dt']\times [-B(t+\tau )\hat{a}(0)+D(t+\tau )\hat{b}(0)\\{} & {} -\int _{0}^{t+\tau }B(t+\tau -t'')\hat{F}_{c}(t'')dt'' +\int _{0}^{t+\tau }D(t+\tau -t'')\hat{F}_{e}(t'')dt'']\rangle ,\nonumber \end{aligned}$$
(37)

from which follows

$$\begin{aligned} \langle \hat{b}^{\dagger }(t)\hat{b}(t+\tau ) \rangle= & {} e^{-\gamma _{+}t/2}e^{-\gamma _{+}\tau /4}\bigg [sin(gt)sin(g(t+\tau ))\bar{n}_{c}\nonumber \\{} & {} \!+\! \bigg (cos(gt)\!+\!\frac{\kappa -\gamma }{4g}sin(gt)\bigg )\bigg (cos(g(t\!+\!\tau ))+\frac{\kappa -\gamma }{4g}sin(g(t\!+\!\tau ))\bigg )\bar{n}_{e}\bigg ]\nonumber \\{} & {} + \frac{\kappa N e^{-\gamma _{+}t/2}e^{-\gamma _{+}\tau /4}}{\gamma _{+}^{3}+16g^{2}\gamma _{+}}\bigg [16g^{2}(e^{\gamma _{+}t/2}-1)cos(g\tau ) -\gamma _{+}^{2}cos(g\tau )\\{} & {} +\gamma _{+}^{2}cos(g(2t+\tau )) + 4g\gamma _{+}e^{\gamma _{+}t/2}sin(g\tau )-4g\gamma _{+}sin(g(2t+\tau ))\bigg ].\nonumber \end{aligned}$$
(38)

The steady-state solutions of this equation is given by

$$\begin{aligned} \langle \hat{b}^{\dagger }(t)\hat{b}(t+\tau )\rangle _{ss} = \frac{\kappa N e^{-\gamma _{+}\tau /4}}{\gamma _{+}^{3}+16g^{2}\gamma _{+}}[16g^{2}cos(g\tau )+4g\gamma _{+}sin(g\tau )] \end{aligned}$$
(39)

and on introducing (35) and (39) into (36), we get

$$\begin{aligned} P(\omega )=\frac{1}{\pi }Re\int _{0}^{\infty }d\tau e^{-(\frac{\gamma _{+}}{4}-i\omega ')\tau }\bigg (cos(g\tau )+\frac{\gamma _{+}}{4g}sin(g\tau )\bigg ). \end{aligned}$$
(40)

Now on carrying out the integration, we find

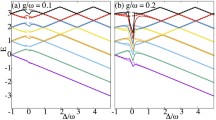
$$\begin{aligned} P(\omega )= \frac{\gamma _{+}}{\pi }\frac{8(\gamma _{+}^{2}+16g^{2})}{(16g^{2}+\gamma _{+}^{2}-32g\omega '+16\omega ^{'2})(16g^{2}+\gamma _{+}^{2}+32g\omega '+16\omega ^{'2})}. \end{aligned}$$
(41)

It is clear from (41) that the intensity spectrum of the fluorescent light does not depend on the initial mean cavity photon and the mean photon number of the input squeezed light. In Fig. 4, we plot the intensity spectrum as a function of \(g/\gamma \) and \((\omega -\omega _o)/\gamma \). It is not hard to see that the intensity spectrum has a single peak in the weak-coupling regime \((g/\gamma \ll 1)\). In the strong coupling regime \((g/\gamma \gg 1)\), however, the intensity spectrum has two symmetrical peaks at \(\omega -\omega _o=\pm g\) around \(\omega =\omega _o\). This division could be explained from the standpoint of the dressed state. Because the microcavity initially contains only one exciton, there are two bare states; \(|0,1\rangle \) a state with no exciton and one photon and \(|1,0\rangle \) a state for which the photon is absorbed to create an exciton. The strong coupling regime lifts the degeneracy of the two bare states results in the dressed states (cavity polaritons). Because the microcavity is coupled to the external reservoir, the photon and the exciton decay lead to the polaritons’ decay. The polaritons decay produces two peaks in the intensity spectrum [29].

Fig. 4
figure 4

Intensity spectrum \(\gamma P(\omega )\) (41) of the fluorescent light vs \(g/\gamma \) and \(\omega /\gamma \) for \(\kappa /\gamma =0.8\)

Full size image
Fig. 5
figure 5

Second-order correlation function \(g^{2}(\tau )\) (44) of the fluorescent light vs \(\gamma t\) for \(g/\gamma =5\) and for different value of the squeeze parameter (r)

Full size image

5 Second-order Correlation Function

The second-order correlation function, which represents the joint probability of detecting a photon at time \(t +\tau \) and another one at an earlier time t, is expressible as

$$\begin{aligned} g^{2}(\tau )=\frac{\langle \hat{b}^{\dagger }(t)\hat{b}^{\dagger }(t+\tau )\hat{b}(t+\tau )\hat{b}(t)\rangle _{ss}}{\langle \hat{b}^{\dagger }(t)\hat{b}(t)\rangle _{ss}^{2}}. \end{aligned}$$
(42)

Because \(\hat{b}(t)\) is a Gaussian variable and applying the Gaussian properties of the noise operators [31], the second-order correlation function can be written as

$$\begin{aligned} g^{2}(\tau )=1+ \frac{|\langle \hat{b}^{\dagger }(t)\hat{b}^{\dagger }(t+\tau )\rangle _{ss}|^{2}+|\langle \hat{b}^{\dagger }(t)\hat{b}(t+\tau )\rangle _{ss}|^{2}}{\langle \hat{b}^{\dagger }(t)\hat{b}(t)\rangle _{ss}^{2}}. \end{aligned}$$
(43)

To obtain an analytical expression for the second-order correlation function, we first need to fix the two-time correlation functions that appear in this expression. To this end, making use of (28) together with the properties of the noise operators, we get

$$\begin{aligned} g^{2}(\tau )=1+e^{-\gamma _{+}\tau /2}\bigg (cos(g\tau )+\frac{\gamma _{+}}{4g}sin(g\tau )\bigg )^{2}(1+\frac{|M|^{2}}{|N|^{2}}). \end{aligned}$$
(44)

The dynamical nature of the second-order correlation function is dependent on the input squeezed light, as shown by (44). Moreover, one can note from this expression that \(g^{2}(0)\) is always greater than 1. This implies that the fluorescent light is bunched. This could be explained on the basis of the squeezed photon that can enter the cavity. These photons can excite an exciton to a higher occupation number, resulting in the emission of two or more photons. In other words, even though we start with a single exciton in the quantum well, there is a finite probability of getting two or more excitons in the cavity, which causes photon bunching in fluorescent light.

In Fig. 5, we plot the second-order correlation function of the fluorescent light versus the time delay. The second-order correlation function oscillates with the Rabi frequency. We see from this figure that the input squeezed light has an effect on the amplitude of oscillation of the second-order correlation function. The amplitude of oscillation of the second-order correlation function decreases as the squeeze parameter increases. Moreover, it is shown in Fig. 6 that the oscillatory behavior of the second-order correlation function decreases in the weak coupling regime. This is due to the transfer of cavity photons to an exciton, which will not be converted back to cavity photons.

Fig. 6
figure 6

Second-order correlation function \(g^{2}(\tau )\) (48) of the fluorescent light vs \(\gamma \tau \) for \(r=0.1\), \(\kappa =\gamma \) and for different value of \(g/\gamma \)

Full size image
Fig. 7
figure 7

Quadrature variance \((\Delta b_-^2)\) (48) of the fluorescent light vs \(\gamma t\) for \(g/\gamma =4\) and for different value of the squeeze parameter (r)

Full size image

6 Quadrature Variance

To investigate the squeezing properties of the exciton mode, we introduce two Hermitian quadrature operators defined by \(\hat{b}_{+}=\hat{b}+ \hat{b}^{\dagger }\) and \(\hat{b}_{-}=i(\hat{b}^{\dagger }-\hat{b})\). It is possible to verify from (28) that \(\langle \hat{b}\rangle =0\). Thus the variances of these two quadrature operators are expressible as

$$\begin{aligned} (\Delta b_{\pm })^{2}=1+2\langle \hat{b}^{\dagger }\hat{b}\rangle \pm (\langle \hat{b}^{\dagger 2}\rangle +\langle \hat{b}^{2}\rangle ). \end{aligned}$$
(45)

The two quadrature operators for the exciton mode obey the commutation relation

$$\begin{aligned}{}[\hat{b}_{+},\hat{b}_{-}]=2i. \end{aligned}$$
(46)

In view of this result, the fluorescent light is said to be in a squeezed state if either \((\Delta b_{+})^{2}<1\) or \((\Delta b_{-})^{2}<1\). Applying (28), one can easily establish that

$$\begin{aligned} \langle \hat{b}^{2}\rangle= & {} \langle \hat{b}^{\dagger 2}\rangle =\frac{\kappa Me^{-\gamma _{+}t/2}}{16g^{2}\gamma _{+}+\gamma _{+}^{3}}[16g^{2}(e^{\gamma _{+}t/2}-1)-\gamma _{+}^{2}+\gamma _{+}^{2}cos(2gt)\nonumber \\{} & {} -4g\gamma _{+}sin(2gt)] \end{aligned}$$
(47)

Now substitution of (34) and (47) into (45) yields

$$\begin{aligned} (\Delta b_{\pm })^{2}= & {} 1+\frac{2\kappa e^{-\gamma _{+}t/2}}{16g^{2}\gamma _{+}+\gamma _{+}^{3}}[16g^{2}(e^{\gamma _{+}t/2}-1)-\gamma _{+}^{2}+\gamma _{+}^{2}cos(2gt)\nonumber \\{} & {} -4g\gamma _{+}sin(2gt)](N\pm M) + sin^{2}(gt)e^{-\gamma _{+}t/2}\bar{n}_{c}+[cos(gt)\nonumber \\ {}{} & {} +\frac{\kappa -\gamma }{4g}sin(gt)]^{2}e^{-\gamma _{+}t/2}. \end{aligned}$$
(48)

In Figs. 7–9, we plot quadrature variance as a function of time to investigate how the squeezing varies with the squeeze parameter, the cavity-exciton coupling strength, and the initial mean cavity photons. In all the three cases, the exciton mode does not exhibit squeezing at first. However, for the longer time, the variance of the quadrature operator for the exciton mode is less than one (the shot-noise limit), indicating that the exciton mode is squeezed. It is not difficult to see from these figures that the degree of squeezing increases with time. Furthermore, as shown in Fig. 7, the quadrature variance exhibits oscillatory behavior for a longer value of time, and the squeezed input has the effect of increasing the quadrature squeezing of the fluorescent light. To see how the cavity-exciton coupling strength affects the degree of squeezing, we plot the quadrature variance as a function of time for different values of the cavity-exciton coupling strength, as indicated in Fig. 8. This figure shows that the amount of squeezing increases as the coupling between the exciton and cavity modes increases. Because there is no interaction between the cavity and the exciton modes, the squeezing disappears for uncoupled cavity and exciton modes.

Fig. 8
figure 8

Quadrature variance \((\Delta b_-^2)\) (48) of the fluorescent light vs \(\gamma t\) for \(r=1\), \(\kappa =\gamma \) and for different value of \(g/\gamma \)

Full size image
Fig. 9
figure 9

Quadrature variance \((\Delta b_-^2)\) (48) of the fluorescent light vs \(\gamma t\) for \(r=1\), \(\kappa =\gamma \) and for \(\bar{n}_{c}=2\) (solid curve), \(\bar{n}_{c}=3\) (dashed curve), and \(\bar{n}_{c}=4\) (dotted curve)

Full size image

As can be seen in Fig. 9, the quadrature variance of the fluorescent light depends on the initial mean cavity photons. We observe that the quadrature variance of the fluorescent light oscillates with an amplitude that gradually decreases over time. Although the initial cavity photons have a negative impact on the amount of squeezing at the initial moment, the effect fades after a sufficiently long time.

7 Conclusion

In the low excitation regime, a quantum well in a microcavity containing photons initially and coupled to a single-mode squeezed vacuum reservoir is studied using the solutions of the quantum Langevin equations. The input squeezed light improves the intensity and quadrature squeezing of the fluorescent light. Due to the two energies of the dressed sates, the intensity spectrum exhibits two peaks in the strong coupling regime. Photon antibunching is observed in atom cavity QED, but photon bunching is observed in semiconductor cavity QED. Furthermore, the initial cavity photons significantly increase the intensity of the fluorescent light at the beginning, and the effect gradually diminishes as time goes on. However, the initial cavity photons significantly reduce quadrature squeezing at the initial moment, and this effect vanishes after a sufficiently long time.

Furthermore, the squeezed vacuum reservoir has no effect on the intensity spectrum of the fluorescent light. The second order correlation function oscillates in the strong coupling regime. The amplitude of this oscillation decreases with the input squeezed light.