1 Introduction

Bonded joints are employed in various engineering fields as an efficient process to join components (Adams and Wake 1984). Among the various techniques available for the strength prediction of bonded joints, one may find stress approaches which compare maximum stresses with material strength or energy approaches which rely on linear elastic fracture mechanics and assume the existence of an initial flaw (Da Silva and Campilho 2012). To predict accurately crack initiation within the bonded area, other approaches are needed.

Based on damage mechanics, cohesive zone models (CZM) describe the traction separation behavior of interfaces using a softening law which can be distinguished by three parts: (1) the increase of the traction until a peak value, which may correspond to an elastic undamaged behavior, (2) the decrease of the traction as a consequence of the development of interfacial damage which induces the loss of the interfacial stiffness of the cohesive element and (3) the apparition of traction-free surfaces when the traction attains the zero value. Following the first papers of (Barenblatt 1959) and (Dugdale 1960), CZM (Tvergaard and Hutchinson 1992; Xu and Needleman 1994) have been extensively used in the literature to analyze the fracture of interfaces in various material systems including delamination in composite laminates (Allix and Ladeveze 1992; Corigliano 1993; Schellekens and Borst 1993; Allix and Corigliano 1996; Turon et al. 2010) and bonded joints (Liljedahl et al. 2006; Gustafson and Waas 2009; Campilho et al. 2012).

Linear elastic fracture mechanics analyzes crack growth in brittle materials but fails to predict crack initiation. For this purpose, finite fracture mechanics is convenient as it allows to consider finite crack increment (Hashin 1996; Nairn 2000). As shown by Leguillon (2002), combining stress and energy conditions provides the applied loading and the crack increment size at crack initiation. This coupled criterion (CC) has recently been demonstrated successful to solve various problems enclosing crack interaction with an interface (Mantič 2009; Leguillon and Martin 2012; García et al. 2014, 2015), edge cracking (Martin et al. 2010; Leguillon et al. 2015), notched strength (Carpinteri et al. 2008; Hebel et al. 2010; Martin et al. 2012; Sapora et al. 2013) and bond strength (Weißgraeber and Becker 2013; Hell et al. 2014; Carrère et al. 2015).

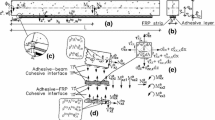
The aim of this paper is to compare the ability of these two approaches to predict the initiation of interfacial debonding. The selected geometry is a bimaterial specimen submitted to a four-point bending test (Fig. 1a). It is assumed that edge debonding (e.g debonding failure starts from the ends of the sample as illustrated in Fig. 1b) is the dominant failure mechanism which results from the concentration of interfacial stresses. This testing geometry may be used to characterize adhesive joints (Cotton et al. 2001; Leguillon et al. 2003) and metal-ceramic bonds (Lenz et al. 1995) or to analyze the debonding of stiffeners for aeronautic components (Bertolini et al. 2009) and of reinforcing plates for civil engineering structures (Lorenzis and Zavarise 2009; Cornetti et al. 2015). This paper is the main part of a study on edge debonding which has been completed by other contributions concerning the comparison of various shapes of the cohesive law (Vandellos et al. 2014), the use of a strain energy criterion (Martin and Leguillon 2015) and the analysis of substrate cracking in the vicinity of the edge (Martin et al. 2014). The paper is organized as follows: the CC is outlined in Sect. 2, Section 3 describes briefly the CZM and a comparison between both methods with an experimental result is presented in Sect. 4.

The geometrical parameters reported in Fig. 1 are fixed to be \(\ell =20\,\hbox {mm}\), \(L_1 =25\,\hbox {mm}\) and \(L_2 =40\,\hbox {mm}\). The substrates have the same thickness \(h=\hbox {2 mm}\) and the same elastic properties (Young’s modulus \(E_s =400\,\hbox {GPa}\) and Poisson’s ratio \(\nu _s =0.2)\). The thickness of the bonding interlayer is first neglected. The sample is submitted to a monotonic increasing displacement d.

Fig. 1
figure 1

The bimaterial specimen: a geometry of the four-point bending test, b initiation of edge debonding upon loading

Full size image

2 Coupled criterion

Within the framework of finite fracture mechanics (Hashin 1996), crack initiation in the vicinity of a stress concentration point is the formation of a crack with a finite area \(\Delta A\). The incremental energy criterion is defined by

$$\begin{aligned} -\Delta W\ge G^{c}\Delta A, \end{aligned}$$
(1)

where \(-\Delta W=W\left( 0 \right) -W(A)\) denotes the decrease of the potential energy and \(G^{c}\Delta A\) (where \(G^{c}\) is the fracture toughness) is the energy dissipated during the crack onset. For a 2D analysis the crack area is given by \(\Delta A=ba\) where a is the crack increment length and b is the sample width. The energy condition can also be written as

$$\begin{aligned} G^{inc}(a)\ge G^{c}, \end{aligned}$$
(2)

which introduces the incremental energy release rate defined by \(G^{inc}(a)=-\frac{1}{b}\frac{\Delta W}{a}\). This parameter must be distinguished from the differential energy release rate \(G(a)=-\frac{1}{b}\frac{dW}{da}\) which enters the formulation of the classical Griffith criterion. As established by Martin and Leguillon (2004), the relationship between G(a) and \(G^{inc}(a)\) is

$$\begin{aligned} G^{inc}\left( a \right) =\frac{1}{a}\int _0^a {G\left( \ell \right) } d\ell . \end{aligned}$$
(3)

To determine the crack size at initiation, an additional condition is needed. Leguillon (2002) proposed to use a stress condition which states that the opening normal stress \(\sigma _{op} \) along the expected crack surface S must exceed the tensile strength \(\sigma ^{c}\) with

$$\begin{aligned} \sigma _{op} \left( M \right) \ge \sigma ^{c},\quad \forall M\in S. \end{aligned}$$
(4)

Relations (2) and (4) define the coupled criterion for crack initiation. As shown by Leguillon (2002), \(G^{inc}(a)\) is usually an increasing function of the crack length. Fulfilling the inequality (2) implies a crack jump at onset and defines a lower bound of admissible crack lengths. This lower bound decreases with the applied load. Similarly, the stress \(\sigma _{op} \) is a decreasing function of the potential crack length and the second inequality (4) provides an upper bound of admissible crack lengths. This upper bound increases with the applied load. Combining (2) and (4) supplies a unique crack length and the lowest loading at crack onset. This approach naturally introduces a characteristic fracture length \(E_s \frac{G^c }{\left( {\sigma ^c } \right) ^{2}}\) to derive the crack length at initiation. The next section formulates the coupled criterion with the help of a two-dimensional analysis of the edge debonding applied to the selected bimaterial specimen.

2.1 Formulation

Although the normal opening stress is prevailing in the vicinity of the edge, a stress analysis reveals the presence of shear along the interface. Following the proposal of García and Leguillon (2012), the stress condition is written

$$\begin{aligned}&\sqrt{\left( {\frac{\sigma _{yy} \left( {x,y=0} \right) }{\sigma _i^c }} \right) ^{2}+\left( {\frac{\sigma _{xy} \left( {x,y=0} \right) }{\tau _i^c }} \right) ^{2}}\ge 1,\nonumber \\&\quad \forall x\le a, \end{aligned}$$
(5)

where \(\sigma _{yy} \) and \(\sigma _{xy} \) are respectively the interfacial opening and shear stresses, \(\sigma _i^c \) and \(\tau _i^c \) are respectively the interfacial tensile and shear strengths. The coordinates (x, y) are indicated in Fig. 2. To take into account the influence of mode mix on the dissipated energy, the energy condition is written

$$\begin{aligned} G_i^{inc} \left( a \right) \ge \frac{1}{a}\int _0^a {G_i^c \left( {\psi \left( x \right) } \right) dx} , \end{aligned}$$
(6)

where the index i holds for interface. The mode mix \(\psi \left( x \right) \) is obtained with the help of the stress state prior to the crack onset (García and Leguillon 2012) with

$$\begin{aligned} \psi \left( x \right) =\tan ^{-1}\left( {\frac{\sigma _{xy} \left( x \right) }{\sigma _{yy} \left( x \right) }} \right) . \end{aligned}$$
(7)

The main difficulty lies in the definition of \(G_i^c \left( \psi \right) \) which relies on empirical laws which are difficult to identify (Banks-Sills 2015). In order to facilitate the comparison between CC and CZM, the influence of mode mix is not considered by assuming that \(\tau _i^c =\sigma _i^c \) and that \(G_i^c \left( \psi \right) =G_i^c \). The stress condition (5) can be expressed as

$$\begin{aligned} \sigma _{eq} \left( x \right) =\sqrt{\sigma _{yy}^2 \left( x \right) +\sigma _{xy}^2 \left( x \right) }\ge \sigma _i^c ,\quad \forall x\le a. \end{aligned}$$
(8)

The equivalent stress \(\sigma _{eq} \) is linearly related to the applied displacement d with \(\sigma _{eq} \left( x \right) =E_i k_{eq} \left( x \right) \left( {\frac{d}{h}} \right) \) which introduces a dimensionless coefficient \(k_{eq} \left( x \right) \) and the plane strain modulus \(E_i =\frac{E_s }{1-\nu _s ^{2}}\). The incremental energy release rate which depends on the square of the loading can be written as

$$\begin{aligned} G_i^{inc} \left( a \right) =E_i h\bar{{A}}_i \left( a \right) \left( {\frac{d}{h}} \right) ^{2}, \end{aligned}$$
(9)

where \(\bar{{A}}_i \left( a \right) \)is a dimensionless coefficient and h is the thickness of the substrate as shown in Fig. 1.

Fig. 2
figure 2

Geometry of the two-dimensional model used to obtain the numerical results

Full size image

Coefficients \(\bar{{A}}_i \left( a \right) \) and \(k_{eq} \left( x \right) \) are estimated with the help of an elastic finite element analysis. A two-dimensional model is used to perform elastic calculations assuming a plane strain state. Only one half of the sample is meshed and the boundary conditions are prescribed displacements as illustrated in Fig. 2. The mesh is highly refined near the edge with an element size \(L_{mesh} \) smaller than 0.08h over an area larger than \(50L_{mesh}\) by \(50L_{mesh} \). Interfacial nodes are unbuttoned to introduce a crack increment of length a and \(\bar{{A}}_i \left( a \right) \) is obtained by \(\bar{{A}}_i \left( a \right) =\frac{\Delta W}{aE_i h\left( {\frac{d}{h}} \right) ^{2}}\) where \(\Delta W=W\left( {a=0} \right) -W\left( a \right) \) is the change in elastic energy induced by crack initiation. Numerical results confirm that \(k_{eq} \left( x \right) \) is a decreasing function but also reveal that \(\bar{{A}}_i \left( a \right) \)is not a monotonic function (Fig. 3) and exhibits a maximum value for \(a=a^{\max }\). A similar non-monotonic behavior is also reported for different geometries involved in the analysis of free edge delamination (Martin et al. 2010) or transverse cracking (García et al. 2014) in composite laminates or debonding at the fiber/matrix interface in composite materials (Martin et al. 2008). A more complex behavior showing the presence of a local maximum followed by a local minimum is demonstrated for the single lap joint geometry (Hell et al. 2014; Carrère et al. 2015).

Fig. 3
figure 3

Dimensionless incremental \(\left( {\bar{{A}}_i \left( a \right) } \right) \) and differential \(\left( {A_i \left( a \right) } \right) \) energy release rates versus the dimensionless crack length. The incremental energy release rate is maximum for \(a=a^{\max }=0.09h\) and the property \(\bar{{A}}_i \left( {a^{\max }} \right) =A_i \left( {a^{\max }} \right) \) is observed and can be proved (Martin and Leguillon 2004)

Full size image

As a consequence of the existence of this maximum and for a monotically increasing displacement, the energy condition (2) will be first met for \(a=a^{\max }\) if

$$\begin{aligned} G_i^{inc} (a^{\max })=E_i h\bar{{A}}_i \left( {a^{\max }} \right) \left( {\frac{d}{h}} \right) ^{2}=G_i^c . \end{aligned}$$
(10)

In order to fulfill the coupled criterion, the stress condition (8) must also be satisfied with

$$\begin{aligned} \sigma _{eq} \left( x \right) =E_i k_{eq} \left( x \right) \left( {\frac{d}{h}} \right) \ge \sigma _i^c ,\quad \forall x\le a^{\max }. \end{aligned}$$
(11)

Combining (10) and (11) (and reminding that \(k_{eq} \left( x \right) \) is a decreasing function) leads to the condition

$$\begin{aligned} E_i \frac{G_i^c }{\left( {\sigma _i^c } \right) ^{2}}\ge \frac{\bar{{A}}_i \left( {a^{\max }} \right) }{\left[ {k_{eq} \left( {a^{\max }} \right) } \right] ^{2}}h, \end{aligned}$$
(12)

which involves the structural length \(L_i^{\max } =\frac{\bar{{A}}_i \left( {a^{\max }} \right) }{\left[ {k_{eq} \left( {a^{\max }} \right) } \right] ^{2}}h\) and the length \(L_i^c =E_i \frac{G_i^c }{\left( {\sigma _i^c } \right) ^{2}}\) which is a characteristic fracture length of the interface. If the characteristic length is large enough to satisfy (12), the energy condition is governing since the stress condition holds true. In this case, the crack length at initiation is \(a^{*}=a^{\max }\) and the corresponding applied displacement is given by \(d^{*}=h\sqrt{\frac{G_i^c }{E_i h{\bar{{A}}}_i \left( {a^{\max }} \right) }}\) which only depends on \(G_i^c \). If condition (11) is not met, the applied displacement must be increased and the energy condition \(E_i h\bar{{A}}_i \left( a \right) \left( {\frac{d}{h}} \right) ^{2}=G_i^c \) will be satisfied for \(a<a^{\max }\). Combining with the stress condition \(E_i k_{eq} \left( x \right) \left( {\frac{d}{h}} \right) =\sigma _i^c \) leads to the estimation of the crack length at initiation \(a^{*}\) with

$$\begin{aligned} \frac{\bar{{A}}_i \left( {a^{*}} \right) }{k_{eq} \left( {a^{*}} \right) ^{2}}=\frac{L_i^c }{h}. \end{aligned}$$
(13)

Relation (13) can also be written \(\frac{\sqrt{\bar{{A}}_i \left( {a^{*}} \right) }}{k_{eq} \left( {a^{*}} \right) }=\sqrt{\frac{L_i^c }{h}}\) where \(\sqrt{\frac{L_i^c }{h}}\) is a dimensionless number similar to the brittleness number introduced by Mantič (2009) as a generalization of Carpinteri’s brittleness number (Carpinteri 1982) to interfacial cracks. Solving (13) is always possible as its left hand side is an increasing function of \(a^{*}\) vanishing for \(a^{*}=0\). Then the displacement at initiation is given by \(d^{*}=h\sqrt{\frac{G_i^c }{E_i h\bar{{A}}_i \left( {a^{*}} \right) }}=h\frac{\sigma _i^c }{E_i k_{eq} \left( {a^{*}} \right) }\) which now depends on \(G_i^c \) and \(\sigma _i^c \). The solution provided by the CC can be summarized in

$$\begin{aligned} \left\{ {\begin{array}{l} L_i^c <L_i^{\max } ,a^{*}<a^{\max }\hbox { with }\frac{\bar{{A}}_i \left( {a^{*}} \right) }{k_{eq} \left( {a^{*}} \right) ^{2}}=\frac{L_i^c }{h},\\ d^{*}=h\sqrt{\frac{G_i^c }{E_i h\bar{{A}}_i \left( {a^{*}} \right) }} \\ L_i^c \ge L_i^{\max } ,a^{*}=a^{\max },d^{*}=h\sqrt{\frac{G_i^c }{E_i h\bar{{A}}_i \left( {a^{\max }} \right) }} \\ \end{array}} \right. \end{aligned}$$
(14)

It is to be noted that \(a^{\max }\) and \(L_i^{\max } \) are structural values which depend on the geometry. For \(h=2\hbox {mm}\), it is found that \({a^{\max }}/h=0.09\) and \({L_i^{\max } }/h=0.87\). Numerical results (obtained for \(0.5\,\hbox {mm}\le h\le 2\,\hbox {mm})\) reveal that these values are weakly dependent on h. Relation (14) is illustrated in Fig. 4a which plots the crack length at initiation versus the ratio \(\frac{L_i^c }{h}\). Figure 4b plots the predicted applied displacement at initiation of debonding versus \(\frac{L_i^c }{h}\) for two values of the interfacial strength. As expected this critical displacement increases with \(L_i^c \) for a given value of \(\sigma _i^c \) (or equivalently with \(G_i^c \) for a given value of \(\sigma _i^c )\).

Fig. 4
figure 4

Predictions provided by the coupled criterion: a crack length at initiation of debonding versus the characteristic fracture length of the interface, b applied displacement at initiation of debonding versus the characteristic fracture length of the interface for two values of the interfacial strength; the values provided by the asymptotic analysis (ASY) are also plotted with dotted lines and are explained in the next section

Full size image

2.2 Comparison with the asymptotic approach

It is interesting to compare the presented approach which relies on direct finite element computations with the asymptotic expansion procedure proposed by Leguillon and Sanchez-Palencia (1987). The theory of singularity allows expressing the tensile stress \(\sigma _{yy} \) along the interface in the vicinity of the edge by

$$\begin{aligned} \sigma _{yy} \left( x \right) =E_i k_0 \left( {\frac{x}{h}} \right) ^{\lambda -1}\left( {\frac{d}{h}} \right) , \end{aligned}$$
(15)

where the singularity exponent is \(\lambda =0.545\) (Leguillon et al. 2003). The dimensionless coefficient \(k_0 \) is related to the generalized stress intensity factor and can be computed using a path independent integral (Leguillon and Sanchez-Palencia 1992).

The incremental energy release rate is given by

$$\begin{aligned} G_i^{inc} \left( a \right) =E_i hA_0 \left( {\frac{a}{h}} \right) ^{2\lambda -1}\left( {\frac{d}{h}} \right) ^{2}. \end{aligned}$$
(16)

Determining the scaling coefficient \(A_0 \) requires matching asymptotic expansions and the use of a path independent integral (Leguillon and Sanchez-Palencia 1992). The coefficients \(k_0 \) and \(A_0 \) do not depend on the thickness h. Combining (15) and (16) with the stress and energy conditions required by the CC takes advantage to provide analytical expressions for crack length and displacement at crack initiation by

$$\begin{aligned} \left\{ {\begin{array}{l} a^{*}=\left( {\frac{k_0 ^{2}}{A_0 }} \right) L_i^c \\ d^{*}=h\left( {\frac{G_i^c }{E_i A_0 h}} \right) ^{1-\lambda }\left( {\frac{\sigma _i^c }{E_i k_0 }} \right) ^{2\lambda -1} \\ \end{array}} \right. \end{aligned}$$
(17)

As expected from the asymptotic assumption, Fig. 4a reveals that equations (14) and (17) only match for small values of the characteristic length such that \(L_i^c <0.05h\), i.e. \(L_i^c \) must be small compared to the characteristic dimension of the structure. This condition thus implies small initiation lengths for which the asymptotic analysis is valid. In this case, the initiation length is proportional to \(L_i^c \) as shown by (17). Figure 4b indicates that using (17) for larger values of \(L_i^c \) (\(L_i^c \ge 0.1h)\) leads to underestimate the load at initiation. In this case, the approximation \(a^{*}=a^{\max }\) in (14) produces a reliable estimate of \(d^{*}\) as a consequence of the slow variation of \(\bar{{A}}_i \left( a \right) \) with a.

2.3 Propagation of the interfacial crack

Even if we focus on the initiation of the interfacial crack, the analysis of its subsequent propagation is worthwhile for comparison purpose. The usual Griffith criterion requires

$$\begin{aligned} G_i (a)=-\frac{1}{b}\frac{dW}{da}\ge G_i^c \quad \hbox { and }\;a\ge a^{*}. \end{aligned}$$
(18)

The differential energy release rate \(G_i (a)\) is expressed as

$$\begin{aligned} G_i (a)=E_i hA_i \left( a \right) \left( {\frac{d}{h}} \right) ^{2}, \end{aligned}$$
(19)

where \(A_i \left( a \right) \) is a dimensionless coefficient. As a consequence of (3), the relationship between the dimensionless coefficients \(\bar{{A}}_i \left( a \right) \) and \(A_i \left( a \right) \) is given by

$$\begin{aligned} \bar{{A}}_i \left( a \right) =\frac{1}{a}\int _0^a {A_i \left( \ell \right) d\ell } . \end{aligned}$$
(20)

Reminding that the incremental energy release rate is maximum for \(a=a^{\max }\) allows writing

$$\begin{aligned} \frac{d\bar{{A}}_i }{da}\left( {a=a^{\max }} \right) =0. \end{aligned}$$
(21)

As shown by (Martin and Leguillon 2004), combining (20) and (21) leads to

$$\begin{aligned} \bar{{A}}_i \left( {a^{\max }} \right) =A_i \left( {a^{\max }} \right) . \end{aligned}$$
(22)

Figure 3 plots the evolution of \(A_i \) and \(\bar{{A}}_i \) versus the crack length, it illustrates this last relation and also discloses that

$$\begin{aligned} A_i \left( a \right) >\bar{{A}}_i \left( a \right) \hbox { for }a<a^{\max }. \end{aligned}$$
(23)

Once \(G_i (a)\) is estimated, applying the Griffith criterion provides the crack length \(a\left( d \right) \) versus the applied displacement as depicted by Fig. 5a for \(\sigma _i^c =500\hbox { MPa}\). This plot clearly reveals a crack jump at initiation of debonding. For high values of the characteristic length such that \(L_i^c \ge L_i^{\max } \), the nucleation length is \(a^{*}=a^{\max }\) with \(G^{inc}\left( {a^{*}} \right) =G_i \left( {a^{*}} \right) =G_i^c \) which shows that \(a^{*}\) is an arrest length. This is the case in Fig. 5a for \(G_i^c =2500\hbox { Jm}^{{-2}}\) which leads to \(L_i^c =2.08h\ge L_i^{\max }\). Reducing \(L_i^c \) with \(L_i^c <L_i^{\max }\) implies \(a^{*}<a^{\max }\) and \(G_i \left( {a^{*}} \right) >G^{inc}\left( {a^{*}} \right) =G_i^c \) as a consequence of (23). The nucleated crack is thus unstable and reaches the length \(a_1 \) defined by \(G_i \left( {a_1 } \right) =G_i^c \) with \(a_1 >a^{\max }\) as it is observed in Fig. 5a for \(G_i^c =100,500,1000\hbox { Jm}^{{-2}}\). Evaluating the compliance \(C\left( a \right) \) of the specimen versus the interfacial crack length supplies the applied force \(F=\frac{d}{C\left( {a\left( d \right) }\right) }\) as plotted in Fig. 5b. This figure identifies the applied load at initiation of debonding and shows the corresponding decrease of the load which is followed by a stable crack propagation.

Fig. 5
figure 5

Predictions provided by the coupled criterion: a crack length versus the applied displacement for \(\sigma _i^c =500\,\hbox {MPa}\) and various values of \(G_i^c \), b load-displacement curve for \(G_i^c =100\hbox { Jm}^{\hbox {-2}}\) and various values of \(\sigma _i^c \)

Full size image

3 Cohesive zone model

A cohesive zone model is defined by surface constitutive relations which describe the evolution of tractions \(\left[ T \right] \) generated across the faces of a process zone as a function of the relative opening \(\left[ u \right] \). Among the various two-dimensional shapes of the model which have been proposed (Alfano 2006), we select a bilinear law (Alfano and Crisfield 2001) which writes

$$\begin{aligned} \left\{ {\begin{array}{l} T_n =\left( {1-\beta } \right) \frac{u_n }{\delta _{0n} }\sigma _i^c \\ T_t =\left( {1-\beta } \right) \frac{u_t }{\delta _{0t} } \tau _i^c \\ \end{array}} \right. , \end{aligned}$$
(24)

where \(\left[ T \right] =\left( {T_n ,T_t } \right) \) are respectively the normal and tangential interfacial tractions and \(\left[ u \right] =\left( {u_n ,u_t } \right) \) are respectively the normal and tangential relative displacements along the interface. The traction-separation law is linear and reversible prior to the peak traction. During the softening phase, the damage variable is \(\beta =\frac{\kappa }{\eta \left( {1+\kappa } \right) }\)with \(\eta =\left( {1-\frac{\delta _{0n} }{\delta _n^c }} \right) =\left( {1-\frac{\delta _{0t} }{\delta _t^c }} \right) \) and \(\kappa =\sqrt{\left( {\frac{u_n }{\delta _{0t} }} \right) ^{2}+\left( {\frac{u_t }{\delta _{0n} }} \right) ^{2}}-1\). No attempt was made here to distinguish mode I from mode II by setting \(\sigma _i^c =\tau _i^c \), \(\delta _{0n}= \delta _{0t}=\delta _0 \) and \(\delta _n^c =\delta _t^c =\delta ^{c}\). This specific choice implies that i) the initiation of interfacial damage is driven by a stress criterion similar to condition (8), ii) the propagation of damage depends on the interfacial fracture energy \(G_i^c =\frac{1}{2}\sigma _i^c \delta ^{c}\)whatever the mode mix. The CZM is thus defined with the help of the three parameters \(\left( {\delta _0 ,\delta ^{c},\sigma _i^c } \right) \) as plotted in Fig. 6.

Fig. 6
figure 6

Traction-separation law of the bilinear cohesive zone model

Full size image

A small value of the separation threshold \(\frac{\delta _0}{h}=5.10^{-6}\) is chosen. With such a choice, the overall rigidity of the model and the computational convergence are not altered. Practical use of a CZM requires a mesh fine enough to obtain an accurate resolution of the cohesive length. This condition can be expressed with the help of an upper bound of the mesh size defined by \(L_{mesh} \le \frac{1}{3}L_{czm}\) with \(L_{czm} \approx \frac{\pi }{2}L_i^c \) (Acary and Monerie 2006). Another requirement states that the critical displacement \(\delta ^{c}\) must be smaller than the characteristic dimension of the structure which can be formulated as \(\delta ^{c}<\frac{h}{10}\). Reminding that \(G_i^c =\frac{1}{2}\sigma _i^c \delta ^{c}\), this condition establishes an upper bound of \(L_i^c \) for a given value of the interfacial strength. Finally, these two conditions lead to

$$\begin{aligned} \frac{6}{\pi }\frac{L_{mesh} }{h}<\frac{L_i^c }{h}<\frac{1}{20}\frac{E_i }{\sigma _i^c }. \end{aligned}$$
(25)

To analyze the mechanical behavior of the bimaterial specimen (Fig.  1), cohesive zone elements are inserted at the interface between the two substrates. The parameters of the CZM are selected in order to satisfy condition (25). An implicit quasi-static finite element code (Burlet and Cailletaud 1991) is used to perform the analysis. A large number of loading increments is imposed to capture accurately the applied loading at crack onset. The crack length is recorded by tracking the interfacial elements for which the damage variable reaches the value \(\beta =1\). Figure 7 plots some predictions provided by the bilinear CZM. A peak force (Fig. 7b) is recorded on the load displacement curve which indicates initiation of edge debonding which is followed by crack propagation (Fig. 7a). The softening part observed on the load displacement response results from the development of the cohesive zone (or interfacial damage also called process zone) prior to crack initiation.

Fig. 7
figure 7

Predictions provided by the bilinear CZM : a crack length and b load-displacement curve versus the applied displacement for \(\sigma _i^c =50\hbox { MPa}\) and various values of \(G_i^c \)

Full size image

4 Results and discussion

A series of finite element computations was performed to compare the applied displacement for the onset of interfacial cracking as provided by the CC and CZM. Figure 8 reveals that a good agreement is only obtained with the smallest values of the fracture length such that \(\frac{L_i^c }{h}<1\). This result was already observed by previous authors (Henninger et al. 2007; Murer and Leguillon 2010) showing a perfect agreement between the coupled criterion and the Dugdale cohesive zone model with a rectangular shape for predicting crack initiation at a V notch : these comparisons were carried out within an asymptotic framework which assumes small crack increments and thus small fracture lengths.

Fig. 8
figure 8

Comparison between CC (solid lines) and CZM (dotted lines): prediction of applied displacement at initiation of debonding versus the characteristic fracture length of the interface for different values of the interfacial strength

Full size image

In order to analyze these results, force and crack length are now examined as a function of the applied displacement. Figure 9a shows that the response of the CZM is very close to the response provided by the finite fracture mechanics approach for \(\frac{L_i^c }{h}=1.042\). In this case, CZM produces a small cohesive length and can match the brittle response of the CC. Increasing the fracture length with \(\frac{L_i^c }{h}=10.42\) extends the cohesive zone and induces a large amount of softening which postpones crack onset (Fig. 9b). In this case, the damageable response of the CZM is enhanced and moves away from the brittle response of the CC. This draws the conclusion that CZM cannot reproduce a brittle interfacial behavior with a large fracture length.

Previous authors (Acary and Monerie 2006) have pointed out that the shape of the cohesive law influences the crack initiation. This is confirmed by a previous work (Vandellos et al. 2014) comparing different shapes of CZM defined within the framework proposed by (Vandellos et al. 2013). Those results indicate that the trapezoidal CZM provides results closer to those obtained with the CC when compared with the bilinear shape. This result is directly related to the observation that the cohesive length of the trapezoidal shape is smaller than the bilinear one.

Fig. 9
figure 9

Comparison between the load-displacement and crack-displacement response predicted by CC and bilinear CZM: a \(\sigma _i^c =100\hbox { MPa}\), \(G_i^c =50\hbox { Jm}^{{-2}}\), b \(\sigma _i^c =100\hbox { MPa}\), \(G_i^c =500\hbox { Jm}^{{-2}}\)

Full size image

Both approaches (CC and CZM) are now used to analyze an experimental response. Our aim here is to illustrate the difference between CC and CZM and also to show that they can be complementary. For this purpose, a testing result demonstrating interfacial damage is selected. The specimen (with the geometry already given in section  1) consists in two silicon carbide substrates bonded by a porous interlayer with the help of a sintering process (Jacques et al. 2014). The elastic properties of the substrate are \(E_s =420\) GPa and \(\nu _s =0.14\). The thickness of the interlayer is \(e = 0.3\) mm and the width of the sample is \(b = 4\) mm. A contacting transducer was used to estimate the specimen deflection at mid span. Depending on the bond strength (controlled by the sintering conditions as shown by Jacques (2012)), experimental results have evidenced substrate cracking or edge debonding. Substrate cracking can be easily analyzed with CC (Martin et al. 2014) and we focus on the last case which exhibits the typical mechanical response plotted in Fig. 11. This response reveals a non-linear part which results from the damage of the weak interlayer followed by a plateau which corresponds to the interfacial crack propagation (Jacques 2012). According to the previous results, it is clear that the CC is not able to reproduce the observed softening but it is used to provide a first estimate of the interfacial parameters needed by the CZM.

The finite fracture mechanics approach is first undertaken. The two-dimensional model (Fig. 10) now includes the interlayer whose thickness cannot be considered as negligible when compared to the substrates one. Following the guidelines recommended by previous authors (Moradi et al. 2013b), at least twenty elements are inserted within the interlayer thickness. It is also assumed that edge debonding occurs at the upper edge of the substrate/interlayer interface where the stress singularity is maximum (Moradi et al. 2013a).

Fig. 10
figure 10

Geometry of the two-dimensional model with an interlayer; edge debonding is introduced at the upper edge of the substrate/interlayer interface

Full size image
Fig. 11
figure 11

Comparison between the experimental load-span response of a bonded specimen with a the CC prediction, b the bilinear CZM prediction

Full size image

The comparison of the initial slope of the experimental response with the finite element results provides an estimate of the modulus of the porous interlayer (\(E_{layer} =2\hbox { GPa})\) and it is assumed that \(\nu _{layer} =0.2\). As depicted in Fig. 11, the experimental response exhibits a plateau corresponding to the interfacial propagation. This stable step allows one to estimate the interfacial fracture energy with the help of relation (18) and leads to \(G_i^c =28\hbox { Jm}^{\hbox {-2}}\). The value of the structural length \(L_i^{\max }\) is found to be \(L_i^{\max } =28.13\,\hbox {h}\). Satisfying (12) thus implies that the interfacial strength \(\sigma _i^c \) must be lower than \(\sigma _i^*=\hbox {14.7 MPa}\) to obtain a dominant energy condition. Assuming a weak enough interlayer, this condition leads to the prediction plotted in Fig.  11a which does not depend on \(\sigma _i^c \). Increasing the estimation of \(\sigma _i^c \) over \(\sigma _i^*\) will increase the applied displacement and load at initiation of debonding as already displayed in Fig. 5b and will move away the prediction from the experimental response. It is clear that the CC hypothesizes a finite crack increment at initiation and cannot reproduce the non-linearity observed on the experimental response. Nevertheless, the FFM approach easily provides a first estimate of the interfacial fracture properties.

The bilinear CZM is now used. In this case, the interlayer is not meshed but introduced by relating the parameter \(\delta _{0} \) to the interlayer modulus with \(\delta _{0} =e\frac{\sigma _i^c}{E_{layer} }\). The interfacial properties obtained with the help of the CC are first used and the results are plotted in Fig. 11b. The prediction based on \(G_i^c =28\hbox { Jm}^{{-2}}\) and \(\sigma _i^c =\hbox {14 MPa}\) is not close to the response provided by the CC. According to the results of the comparison between CZM and CC presented in Fig. 8, this was expected because the value of \(\frac{L_i^c }{h}=26.9\) is significantly larger than unity. The plateau value is also higher. Reducing \(\sigma _i^c \) to a value of 10 MPa decreases the non-linearity threshold. This is clearly different from the CC prediction which is independent from \(\sigma _i^c \) for \(\sigma _i^c \le \sigma _i^*= \hbox {14.7 MPa}\). Finally a good agreement with the experimental response is found for \(G_i^c =25\hbox { Jm}^{-\hbox {2}}\) and \(\sigma _i^c =5.5\hbox { MPa}\).

4.1 Conclusion

Initiation of edge debonding within a bimaterial specimen submitted to four point bending was predicted both using FFM and CZM. The first approach relies on the CC which combines a stress and an energy conditions. The CZM uses a bilinear interface law. The comparison between these approaches reveal their advantages and drawbacks : i) CC is limited to a brittle behavior (whatever the fracture length), ii) CZM only produces a linear response for small values of the fracture length and shifts to a non linear response for large values of the fracture length. A good agreement between the two methods is only obtained for small values of the fracture lengths such that \(\frac{L_i^c }{h}<1\). Use of CZM with largest fracture lengths extends the cohesive zone and thus introduces softening which postpones crack initiation compared to the CC. Finally, the analysis of a specimen bonded with a damageable interlayer shows that the CC can be helpful to provide a first set of values to identify the parameters of the CZM. Further work should include the influence of mode mix on the initiation of edge debonding which was not included in the present analysis. However, this influence is expected to be weak as a consequence of the prevailing opening mode at least for moderate values of the ratio \(\frac{L_i^c }{h}\) which will lead to short crack lengths at initiation.