In considering various characteristics of the functional and operational properties of polymeric textile materials, it can be noted that the maximum information about their functional properties can be found using deformation and energy components [1,2,3].

As is known, for the study of operational characteristics, it is easiest to use mechanical work on deformation of polymer textile materials, which can be determined graphically from the stress-strain curve of these materials. At the same time, taking into account the specific operating conditions of polymeric textile materials, it can be noted that only the initial zone of the stress-strain curve is important for them, where there is still no significant destruction of the materials [4,5,6].

The most objective picture of the functional behavior of a polymer textile material during its operation can be obtained by measuring the deformation characteristics in the initial deformation zone. By “deformation characteristics”, we refer to the elastic and plastic components of the work on deformation of the material, as well as the corresponding components of the total deformation [7,8,9,10].

The determination of these components of the work on the deformation of the polymer textile material and the total deformation is carried out either graphically from the stress-strain curve, or by numerical prediction using the creep parameters of the corresponding mathematical model.

The development of computer algorithms and computer programs for calculating the elastic and plastic components of the operational deformation processes of polymer textile materials allows them to be used both at the stage of design of new materials and at the stage of organizing their production [11,12,13,14].

Consider the process of deformation of polymeric textile materials with a certain fixed strain rate \( \dot{\varepsilon} \) in the initial zone of the stress-strain diagram (Fig. 1).

Fig. 1
figure 1

Experimental diagram of stretching of a polymeric textile material made of polypropylene with a surface density of 160 g/m2 at a strain rate of 0.0166 s–1.

Full size image

Stress-strain curves of polymeric textile materials can be obtained by predicting the process of their stretching according to the following law:

$$ {\upvarepsilon}_t=\dot{\varepsilon}\cdot t+{\upvarepsilon}_0 $$
(1)

where ε0 is the initial deformation, which is most often taken to equal zero: ε0 = 0.

Graphically, as shown in Fig. 1, from the stress-strain curve of a polymeric textile material, one can determine the values of the initial E0 and final E∞ relaxation modulus [15,16,17].

We take into account that

$$ {E}_{\upvarepsilon t}=\frac{F^{-1}\cdot {P}_t}{\upvarepsilon t}=\frac{F^{-1}\cdot {P}_t}{\upvarepsilon_0+\dot{\varepsilon}\ t}, $$
(2)

or, for ε0 = 0,

$$ {E}_{\upvarepsilon t}=\frac{F^{-1}\cdot {P}_t}{\dot{\varepsilon}t}. $$
(3)

It can be noted that the numerical value of the initial relaxation modulus E0 is equal to the upper asymptote to the curve of the relaxation modulus Eεt for t → 0:

$$ {E}_0{=}_{t\to 0}^{\lim {E}_{\upvarepsilon t}}. $$
(4)

Similarly, the numerical value of the end relaxation modulus E∞ is equal to the lower asymptote to the curve of the relaxation modulus Eεt for t → ∞:

$$ {E}_{\infty }{=}_{t\to \infty}^{\lim {E}_{\upvarepsilon t}}, $$
(5)

That is, the numerical values of the initial and final modulus of elasticity of the polymeric textile material can be obtained graphically from the stress-strain curve; these are the corresponding values of the tangents of the angles at which the tangents are inclined, drawn to the experimental stress-strain curve for its initial and end points [18,19,20]:

$$ {E}_0=\mathrm{tg}\upalpha \cdot A, $$
(6)
$$ {E}_{\infty }=\mathrm{tg}\upbeta \cdot A, $$
(7)

where A = 1 GPa is a normalizing constant.

Consider in detail the stretching process, the diagram of which is shown in Fig. 1.

Here, \( {\upvarepsilon}_t=\dot{\upvarepsilon}\cdot t \) and Pt are the coordinates of some point on the stress-strain curve.

For the general case, when \( \dot{\upvarepsilon}\ne \mathrm{cons}\;t \), and for the particular case, when \( \dot{\upvarepsilon}\ne \mathrm{cons}\;t \), the total deformation of the polymer textile material can be decomposed into elastic (εt0) and viscoplastic (εtt) components according to the following formulas [21,22,23]:

$$ {\upvarepsilon}_{t0}={E}_0^{-1}\cdot {F}^{-1}\cdot {P}_t, $$
(8)
$$ {\upvarepsilon}_{tt}={\upvarepsilon}_t+{\upvarepsilon}_{t0}. $$
(9)

The values of the elastic and viscoplastic deformation components of a polymeric textile material made of polypropylene with a surface density of 160 g/m2 according to the stress-strain curve with a strain rate \( \dot{\upvarepsilon} \) = 0.0166c−1 are given below:

εt, %

εt0, %

εtt = εt − εt0, %

4.0

3.8

0.2

8.0

6.6

1.4

12.0

8.6

3.4

16.0

10.0

6.0

20.0

11.3

8.7

Figures 2 and 3 show graphical illustrations of the decomposition of the complete deformation of a polymeric textile material made of polypropylene with a surface density of 160 g/m2 into the elastic and viscoplastic components [24,25,26].

Fig. 2
figure 2

Isolation of the elastic (εt0, at0) and viscoplastic (εtt, att) components from the total deformation εt and from the total work at of a polypropylene textile material with a surface density of 160 g/m2 according to the stress-strain curve with a strain rate of 0.0166 s–1.

Full size image
Fig. 3
figure 3

A variant of decomposition of the total deformation of a polymeric textile material made of polypropylene with a surface density of 160 g/m2 into the elastic and viscoplastic components using a stress-strain curve diagram with a strain rate of 0.0166 s–1.

Full size image

The proportions of the elastic and viscoplastic components of the total deformation of the polymer textile material can be calculated using the following formulas [25,26,27,28]:

$$ {\upvarepsilon}_{t0}\cdot {\upvarepsilon}_t^{-1}={E}_0^{-1}{F}^{-1}\cdot {P}_t{\upvarepsilon}_t^{-1}, $$
(10)
$$ {\upvarepsilon}_{tt}\cdot {\upvarepsilon}_t^{-1}=\left({\upvarepsilon}_{tt}-{\upvarepsilon}_{t0}\right){\upvarepsilon}_t^{-1}. $$
(11)

Thus, the total deformation εt of polymer textile materials can be divided into the elastic εt0 and viscoplastic εtt components using the experimental stress-strain curve (1).

Consider the separation from the deformation work at of its elastic and viscoplastic at0 components [29,30,31].

Selecting a point (εt, σt = F–1·Pt) (Fig. 1) on the stress-strain curve of a polymeric textile material, we can calculate the deformation work at by the formula

$$ {a}_t=\underset{0}{\overset{\upvarepsilon_t}{\int }}{\upsigma}_t\cdot d\upvarepsilon =\underset{0}{\overset{\upvarepsilon_t}{\int }}{F}^{-1}\cdot {P}_t\cdot d\upvarepsilon . $$
(12)

Then its elastic component will look like:

$$ {a}_{t0}=0.5\cdot {E}_0^{-1}\cdot {\upsigma}_t^2=0.5\cdot {E}_0^{-1}\cdot {F}^{-2}\cdot {P}_t^2, $$
(13)

and the formula for finding the fraction of the elastic component of the deformation work (at0/at) can be written, taking into account formulas (12) and (13), as follows [31, 32]:

$$ \frac{a_{t0}}{a_t}=\frac{P_t^2}{2\cdot {E}_0F\cdot \underset{0}{\overset{\upvarepsilon_t}{\int }}{P}_t\cdot d\upvarepsilon}. $$
(14)

The expression for the viscoplastic component of the deformation work has the form

$$ {a}_{tt}={a}_t-{a}_{t0}, $$
(15)

and its fraction can be calculated by the formula

$$ \frac{a_{tt}}{a_t}=1-\frac{a_t0}{a_t}, $$
(16)

which follows from formulas (15) and (16).

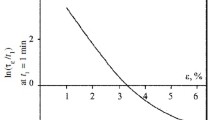
A sample calculation of the fraction of elastic at0/at and viscoplastic att/at components of the work on deformation of a polymeric textile material made of polypropylene with a surface density of 160 g/m2, for a temperature of T = 40 C, is shown in Fig. 4. The strain rate here was 0.0166 s–1.

Fig. 4
figure 4

Graphical representation of the fraction of elastic at0/at and viscoplastic att/at components of the work on deformation of polymeric textile material made of polypropylene with a surface density of 160 g/m2, the strain rate is 0.0166 s–1.

Full size image

From the graph (Fig. 4) we see that the fraction of att in the work on deformation increases, and the fraction of at0 decreases with increasing deformation ε. From this it can be concluded that the polymer textile material requires some energy to recover.

Then, on the basis of the law of conservation of energy, the specified additional energy can be transferred to the polymer textile material only by the external environment, and the greater the amount of deformation, the more external energy will need to be transferred.

All of the above indicates an increase in the significance of the viscoplastic factor with a decrease in deformation.

The indicated methods of separation of deformation and work on deformation of materials into components contribute to the assessment of the elastic, viscoelastic and plastic properties of these materials during technological sampling.

For the manufacture of products from polymeric textile materials that need to quickly restore their original shape (for example, business clothing), it is recommended to use materials in which the elastic component of deformation prevails.

At the same time, if it is necessary to manufacture products from polymeric textile materials with plastic functionality (for example, construction workwear), it is necessary to use materials with a predominance of the viscoplastic component of deformation as these materials have the ability to absorb harmful mechanical influences.

This work was financed under the grant of the President of the Russian Federation MK-1210.2020.8 and within the framework of the state assignment of the Ministry of Science and Higher Education of the Russian Federation, Project FSEZ-2020-0005.