1 Introduction

It is well established that structures do not remain elastic under extreme ground motion. Non-linear behavior therefore constitutes the key issue in seismic design and assessment of structures. However, to avoid the use of more elaborate analysis, structural engineering approaches are usually based on simplified static methods to determine seismic action. In these simplified methods, compared to linear behavior, seismic action is reduced according to the deformation capacity and the energy dissipation capacity of the structure as it undergoes large inelastic deformations. The majority of the building codes around the world are based on this design philosophy.

Seismic assessment using any method but non-linear time history analysis therefore requires a reliable estimation of the seismic displacement demand. Since Veletsos and Newmark (1960), it has been widely accepted that the displacements of elastic and inelastic systems are approximately the same (Equal displacement principle). This empirical principle was confirmed by numerous numerical and experimental investigations (e.g. Lestuzzi and Badoux 2003), except for low period structures, for which inelastic displacements are rather higher than elastic displacements. Since then, many authors tried to model inelastic displacements using linear approaches in order to allow earthquake engineers to easily perform these computations (e.g. Fajfar 1999). It should be emphasized that, until non-linear time history analysis becomes a standard procedure, there is a need for such simplified methods to estimate inelastic seismic demand.

The plateau range of the design spectra is of particular significance for seismic design and assessment. For instance, most of the buildings in Europe are lower than 5-story structures, and therefore have a natural period lower than 1 s. As a consequence, the natural period of a large part of these structures is located on the plateau of the design spectra, i.e. out of the assumed standard range of application of the equal displacement rule.

Moreover, contrary to design procedures, assessment procedures may lead to account for high strength reduction factors, due to several reasons. First, the shear strength of existing structures, especially unreinforced masonry structures, may be very low despite a creditable displacement capacity; these two parameters being not necessary linearly related. This has been shown in laboratory tests (e.g. ElGawady et al. 2005; Tomazevic and Weiss 2010). Second, some codes allow relatively high strength reduction factors, e.g. 3–4 in Germany for unreinforced masonry structures. In Switzerland, the minimum permitted compliance factor (ratio of the capacity of the structure over the demand in the current codes) is 0.25. Weak buildings therefore have to be assessed to high demands compared to their capacity, thus leading to computing the demand for high strength reduction factors (up to 8) nevertheless resulting in compliant values. Even if these computations are theoretical, they are of practical need for the engineers.

Current linearization methods can be grouped into one of two categories: (1) methods based on \(\hbox {R}-\mu -\hbox {T}\) relationships and (2) equivalent damping approaches (Lin and Miranda 2009). While the latter were developed and included in the design code in the US (Kowalsky 1994; ATC 2005), the former has been more inspiring in Europe (Fajfar 1999; CEN 2004). However, it has been shown that the simplification of the N2 method (Fajfar 1999) in EC8 led to an underestimation of the demand in some cases (Norda and Butenweg 2011). Therefore, there is a need for a simple method of estimation of the demand that should be at least conservative for structures on the plateau of the design spectra.

In this paper, different linearization methods are evaluated with respect to an inelastic model and compared to a new simple \(\hbox {R}-\mu -\hbox {T}\) relationship based on graphical assumptions.

2 Approaches for predicting the non-linear displacement

2.1 Current available approaches

2.1.1 R-\(\mu \)-T relationships

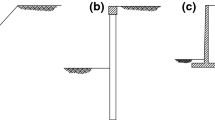
Veletsos and Newmark (1960) first found out that for large to medium periods, the displacements of elastic and inelastic systems were approximately the same. This empirical statement known as equal displacement principle or equal displacement rule (EDR) is nowadays widely used for seismic design purposes, e.g. in Eurocode 8 (CEN 2004). The basic assumption of the EDR is to model an inelastic system using the equivalent elastic system with the same period and damping coefficient. As illustrated in Fig. 1a, the equal displacement rule states that inelastic peak displacements (\({\mathrm{y}_\mathrm{p}}\)) are approximately equal to elastic peak displacements (\(\hbox {y}_{\mathrm{el}}\)) whatever the selected yield strength (\(\hbox {F}_{\mathrm{y}}\,=\,\hbox {F}_{\mathrm{el}}/\hbox {R}\) or yield displacement \(\hbox {y}_{\mathrm{y}}=\hbox {y}_{\mathrm{el}}/\hbox {R}\)) of the structure. Note that when assuming that the stiffness is independent of strength, the equal displacement rule leads to a strength reduction factor (R) equal to the global displacement ductility.

Fig. 1
figure 1

a Schematic description of the equal displacement rule. b ADRS representation of the non-linear displacement demand according to EC8 (adapted from CEN 2004)

Full size image

Numerous studies of the so-called \(\hbox {R}-\mu -\hbox {T}\) relationships propose adjustments to this approximate prediction by giving the strength reduction factor R as a function of displacement ductility \(\mu \) and possibly period T, as reviewed by Miranda and Bertero (1994), Miranda and Ruiz-Garcia (2002) or Chopra and Chintanapakdee (2004).

On the plateau period range of the design spectra, Riddell et al. (1989) and later Vidic et al. (1994) first proposed the linear variation of the strength reduction factor as a function of the period for a constant displacement ductility demand, later used in EC8:

$$\begin{aligned} R\,=\,1+(\mu -1)\frac{T_0}{T} \end{aligned}$$
(1)

In this assumption, the strength reduction factor varies between 1 for a zero period and the value of the displacement ductility demand for the period \(\hbox {T}_{0}\) being close to the end of the plateau \(\hbox {T}_{\mathrm{C}}\) (0.25–0.4 s for moderate to high hazard countries). Based on Eq. 1, the peak response \(\hbox {y}_{\mathrm{N2}}\), implemented in a more general seismic analysis method, the N2 method (Fajfar 1999) is computed as follows:

$$\begin{aligned} \begin{array}{l} T_0\,=\,0.65\times \mu ^{0.3}\times T_c \le T_c \\ \left\{ {{\begin{array}{l@{\quad }l} {\forall T<T_0 }&{} \quad {y_{N2} =y_{el} } \\ {\forall T\ge T_0 }&{} \quad {y_{N2}\,=\,\frac{y_{el} }{R}\left( {1+(R-1) \frac{T_0 }{T}} \right) } \\ \end{array} }} \right. \\ \end{array} \end{aligned}$$
(2)

In EC8 (CEN 2004), a simplified version of the N2 method is proposed for seismic assessment using pushover analysis (Fig. 1b). The determination of the displacement demand (target displacement) is performed according to the equal displacement rule for medium and long period structures and according to the N2 method for short period structures. While assuming that \(\hbox {T}_{0}\,=\,\hbox {T}_{\mathrm{c}}\) for the sake of simplification as proposed by Fajfar (1999). In this paper, both versions (i.e. original and EC8) of the procedure are investigated.

Miranda (2001) showed using statistical analysis that the strength reduction factors were approximately equal to the displacement ductility (equal displacement rule) from approximately 0.2 s for a ductility of 1.5 and 1.2 s for a ductility of 6. All methods based on the \(\hbox {R}-\mu -\hbox {T}\) relationships tend to use the equal displacement rule for periods greater than 0.25–0.5 s. For lower period values, some researchers suggested using the so-called equal energy approximation (EEA). Miranda (2006) considers this “rule” as a “myth”, showing there is no statistical correlation between its results and non-linear displacement values. Ye and Otani (1999) further developed the equal energy concept and conclude that it cannot be applied to short period systems. However, it is still used by some researchers and engineers especially to interpret experimental tests in terms of ductility (e.g. Lu and Kasa 2008). This modification of the strength reduction factor using the equal energy rule leads to a response \(\hbox {y}_{\mathrm{EEA}}\) that can be calculated using:

$$\begin{aligned} y_{EEA}\,=\,\frac{R^2+1}{2R}y_{EDR} \end{aligned}$$
(3)

Even if the equal displacement rule has been shown to be accurate on average through statistical analysis in the intermediate to long period range, the resultant displacement values may not be necessary conservative due to the associated variability, as stated by Miranda and Bertero (1994). However, corrections on the displacement demand using the strength reduction factors can only change the average value, i.e. the bias, but do not change the variability, inherent in the inelastic behavior. The only way to handle this issue with \(\hbox {R}-\mu -\hbox {T}\) relationships is to adopt conservative values for design purposes.

2.1.2 Equivalent period and damping ratios

In the second category, in order to consider inelastic systems as equivalent linear systems, as first proposed by Jacobsen (1930), Rosenblueth and Herrera (1964) suggested using the secant stiffness instead of the linear or initial one. Iwan and Gates (1979); Miranda and Ruiz-Garcia (2002); Sullivan et al. (2004); Priestley (2006); Miranda (2006) or Lin and Miranda (2009) already extensively reviewed these methods. The period \(\hbox {T}_{\mathrm{e}}\) corresponding to the secant stiffness assuming a hardening r, defined as the ratio between initial and post-yield stiffness, is given by the equation:

$$\begin{aligned} T_e=T\sqrt{\frac{\mu }{1-r+r\mu }} \end{aligned}$$
(4)

Or more simply, assuming r = 0 which corresponds to the pure elasto-plastic model:

$$\begin{aligned} T_e=T\sqrt{\mu } \end{aligned}$$
(5)

These researchers assume that the best elastic system to represent an inelastic one has a larger period and a higher damping ratio than is obtained using formulae based on the energy dissipated during cycles. Several researchers (e.g. Gülkan and Sozen 1964; Iwan and Gates 1979) argued that the equivalent damping ratio \(\xi _{\mathrm{e}}\) given by Rosenblueth and Herrera (1964), based on the harmonic response, was overestimated (Miranda and Ruiz-Garcia 2002) and suggested several modifications based on theoretical considerations (Chopra and Goel 1999; Calvi 1999; Levy et al. 2006) or by fitting the displacement obtained by inelastic SDOF models loaded by recorded strong motions (Dwairi et al. 2007). Dwairi et al. (2007), which gives very close damping values to the formulation of Calvi (1999), is one of the most recent. They propose several relationships for the equivalent damping ratio depending on parameters of the hysteretic model. The equation related to the Small Takeda model (r \(=\) 0, \(\alpha =0.5, \upbeta \,=\,0\)) has been selected for this study:

$$\begin{aligned}&\xi _e=\xi +C \frac{\mu -1}{\pi \mu }\nonumber \\&\hbox {with} \left\{ {{\begin{array}{l@{\quad }l} {\forall T_e>1 }&{}\quad C=0.5\\ {\forall T_e\le 1 }&{}\quad C=0.5+0.4\cdot (1-T_e) \end{array} }} \right. \end{aligned}$$
(6)

Recently, this method has been used by Colombi et al. (2008) to assess the vulnerability of Italian buildings, following the work of Borzi et al. (2008). They chose this model for masonry buildings and the Large Takeda model (r = 0, \(\alpha \,=\,0, \upbeta \,=\,1\)) for RC buildings.

These linearization methods are now widely used for design purposes (ATC 2005) as well as for large-scale seismic vulnerability assessment (FEMA 1999; Calvi 1999; Borzi et al. 2008; Colombi et al. 2008). This approach, slightly more complicated than the initial stiffness methods, is aimed at better describing the physics of structural damage, even if its theoretical basis remains arbitrary (Miranda 2006).

Finally, other authors suggest intermediate values of equivalent periods and damping ratios using empirical relationships. Iwan (1980) computed fully empirical relations for both equivalent periods and damping ratios using few recorded signals and found relationships that outperform the previous described techniques. Lin and Miranda (2008) followed the same approach but used more extensive data and computed these parameters as a function of the strength reduction factor R in order to avoid iterations. Their results are the following:

$$\begin{aligned} T_e&= T\cdot \left( 1+\frac{0.026}{T^{0.87}}\cdot (R^{1.8}-1)\right) \end{aligned}$$
(7)
$$\begin{aligned} \xi _e&= \xi +\frac{0.016}{T^{0.84}}\cdot (R-1) \end{aligned}$$
(8)

Bringing more knowledge into the models (more parameters, more degrees of freedom in the regression equation), these more complicated linearization methods therefore aim to decrease the errors in the computation of the maximum displacement, especially at low periods where the equal displacement rule is not relevant anymore. They are successful in this task as shown by Lin and Miranda (2009).

2.2 New proposed R-\(\mu \)-T simplified relationships

It is obvious that linear methods will never be able to reproduce the complexity of non-linear computations. Moreover, the objective of these linearization methods for the engineer is to be able to practically estimate the displacement demand that the studied structure may experience for a given hazard level. In most of the cases, the engineer prefers having (slightly) conservative values in order to be on the safe side. For more advanced applications, non-linear computations would nowadays be employed. Therefore, simple, slightly conservative and graphically obvious ways of estimating the non-linear displacement demand for low period buildings on the plateau of the design spectra are proposed here. They should only depend on the natural frequency of the structure and therefore be independent of the strength reduction factor.

Observing the non-linear displacement demand according to EC8 (Fig. 2a), in the period range for the plateau of the corresponding design spectrum (between \(\hbox {T}_{\mathrm{B}}\) and \(\hbox {T}_{\mathrm{C}})\), the displacement demand tends quickly to a linear variation for increasing strength reduction factors (R). This linear variation is the prolongation of the Equal Displacement Rule that is valid for the period range after the plateau and constitutes Proposition 1 (Fig. 2b). Proposition 2 keeps the linear variation but with an initial value of one third of the spectral displacement at the end of the plateau.

Fig. 2
figure 2

a Spectral displacement representation for increasing strength reduction factors (R) of the non-linear displacement demand according to EC8. b The two propositions for the non-linear displacement demand in the period range for the plateau

Full size image

Proposition 1

Proposition 1 is the linear extension of the [\(\hbox {T}_{\mathrm{C}}\;\hbox {T}_{\mathrm{D}}\)] interval of the design spectra, i.e. the constant pseudo-velocity interval. This corresponds to the EC8 formula with an infinite strength reduction factor (Eq. 2). With \(\hbox {S}_{\mathrm{ap}}\) the spectral acceleration value of the plateau, the proposed spectral inelastic displacement \(S_{d,inel}^{prop1}\) in this period range corresponds to the following equation:

$$\begin{aligned} {\forall T \in [T_B,T_C],S_{d,inel}^{prop1}(T)}=S_{ap}\left( \frac{T_C}{2\pi }\right) ^2\frac{T}{T_C} \end{aligned}$$
(9)

Since in this period range (the plateau), the elastic displacement response spectra is: \(\forall T \in [T_B,T_C],S_{d,el}^{code}(T)=S_{ap}\left( \frac{T}{2\pi }\right) ^2\), the corresponding ductility demand can be simply written\(:\)

$$\begin{aligned} {\forall T \in [T_B,T_C],\mu }=\frac{S_{d,inel}^{prop1}(T)}{S_{d,el}^{code}(T)}=\frac{T_C}{T} \end{aligned}$$
(10)

With the ductility tending to 1 (equal displacement rule) when T approaches the corner of the plateau.

Proposition 2

In case the conservative Proposition 1 is not conservative enough for moderate to large non-linearity levels, a further linear extension of the spectrum is proposed (Proposition 2) with an ordinate at the origin being a fraction \(\alpha \) of the displacement demand in \(\hbox {T}_{\mathrm{C}}\). In this case, the displacement demand is written as follows\(:\)

$$\begin{aligned} {\forall T} \in [T_B,T_C],S_{d,inel}^{prop2}(T)=S_{ap}\left( \frac{T_C}{2\pi }\right) ^2 \left( \frac{T}{T_C}(1-\alpha )+\alpha \right) \end{aligned}$$
(11)

and the corresponding ductility demand is as follows:

$$\begin{aligned} {\forall T} \in [T_B,T_C],\mu =\frac{S_{d,inel}^{prop2}(T)}{S_{d,el}^{code}(T)}=\left( \frac{T_C}{T} \right) ^2 \left( \frac{T}{T_C}(1-\alpha )\!+\!\alpha \right) =\frac{T_C}{T}(1-\alpha )\!+\!\left( \frac{T_C}{T}\right) ^2 \alpha \nonumber \\ \end{aligned}$$
(12)

In the following, \(\alpha \) is chosen as \(\alpha =1/3\) for graphical reasons explained hereafter.

2.2.1 Graphical estimation of displacement demand prediction

The proposed simplified displacement demand predictions can be easily graphically approximated. This constitutes a significant advantage for practical applications. Starting from the spectral displacement related to the natural frequency of the structures and corresponding to the EDR, the increase of the displacement demand predictions may be approximated by a portion of the spectral displacement difference between the natural frequency of the structure and the plateau corner period (\(\hbox {T}_{\mathrm{C}})\) of the design response spectrum. As illustrated in Fig. 3, in the standard ADRS format, proposition 1 corresponds to an additional one third of the spectral displacement difference and proposition 2 corresponds to an additional one half.

Fig. 3
figure 3

Graphical estimation of the two proposed displacement prediction in the ADRS format

Full size image

Such a graphical estimation allows the displacement demand prediction to be performed with less than 5 % error compared to the exact values of the propositions.

Note that since proposition 1 constitutes the upper bound of the original EC8 displacement demand prediction, the corresponding graphical estimation (an additional one third of the spectral displacement difference) may be used for a quick check of computed displacement demand in a practical case using the current EC8 method.

3 Methodology

Figure 4 illustrates the methodology used in this study. It consists in the computation of the non-linear responses of SDOF systems subjected to earthquake records and of the assessment of the difference between the obtained peak displacement demands and those predicted by selected approaches. For the non-linear time-history analyses, structural behavior is described by several widely used hysteretic models such as Takeda or Gamma models (Lestuzzi and Badoux 2003). Four sets of 12 records selected from the European Strong Motion Database (Ambraseys et al. 2002), slightly modified to match design response spectra for different ground types, are developed to evaluate the different methods.

Fig. 4
figure 4

Schematic description of the methodology used

Full size image

3.1 Ground motions

As the objective is to propose a practical but relevant method to estimate inelastic displacements, and not a physical theory aiming at explaining the phenomena, the validation is made using ground motions matching design spectra. Though these ground motions do not represent a realistic hazard, they facilitate the understanding of key parameters influencing the results.

3.1.1 ESMD database of recorded ground motions

Non-linear time-history analysis may be carried out using both recorded earthquakes or artificially generated earthquakes (e.g. Schwab and Lestuzzi 2007). In the past, artificial ground motions were preferred for earthquake engineering purposes since they could be easily generated to match an elastic design spectrum and therefore be used in the frame of design codes. This approach is criticized in the literature because generated ground motions may not be realistic and do not cover the variability of actual ground motions (e.g. Lestuzzi et al. 2004). Nowadays, the amount of records of strong earthquake is exponentially increasing so that real accelerograms with given characteristics, such as their similarity with design spectra, can be selected (e.g. Iervolino et al. 2011).

Schwab and Lestuzzi (2007) selected 164 recorded ground acceleration time histories from the European Strong Motion Database (Ambraseys et al. 2002). The selection of the records in this initial database is based on structural engineering considerations rather than seismological ones. As a consequence, earthquakes with different focal mechanisms are incorporated into the dataset. The main objective is to perform a statistical study of the non-linear response of structures undergoing any earthquake record.

In order to consider earthquakes that may produce significant non-linearities in structural behavior, only records with a magnitude larger than 5 were considered for this selection. The magnitudes range from 5.0 to 7.6, the epicentral distances range from 2 to 195 km and the peak ground accelerations (PGA) range from 0.61 to \(7.85\,\hbox {m}/\hbox {s}^{2}\).

This selection was already used in other research projects in the field of seismic non-linear behavior (Lestuzzi et al. 2004 and 2007).

3.1.2 Selection of sets of 12 records

Out of this preliminary selection, sets of 12 records best matching different design response spectra were extracted. For the selection of these sets, four design response spectra from EC8 are considered. These EC8 spectra are of type 1, for the usual viscous damping ratio of 5 % and for ground types A, B, C and D. They are scaled for a peak ground acceleration of 1 \(\hbox {m}/\hbox {s}^{2}\) corresponding to the design response spectra of the Swiss seismic zone 2 (SIA 2003).

The selection is performed for the best match to the considered response spectrum (target spectrum) through ranking the 164 records of the initial database by the difference between their response spectrum and the target spectrum. The best twelve records form the set related to the considered target response spectrum. As an example, the response spectrum of the twelve records selected for the best fit to the design spectrum of ground type C are plotted separately in Fig. 5. Finally, a total of 33 records are selected for the four sets of 12 records each; with several records belonging to two or more sets. The main characteristics of the 33 selected records and their corresponding sets are listed in the Table 1.

Fig. 5
figure 5

Response spectrum of the twelve records selected for the best fit to the design spectrum of ground type C

Full size image
Table 1 Main characteristics of the 33 selected records and their distribution in the four different sets of twelve records each (as = aftershock)
Full size table

3.1.3 Modification of records for matching target spectra

After selection into the initial database, the 33 records are modified with the non-stationary spectral matching technique of Abrahamson (1992) in order to match individually the related design spectrum. Spectral matching for a given design spectrum is not always regarded as relevant, since design spectra are envelopes of possible earthquake spectra. The reason to perform a selection and matching of the records to the design spectra is to show the consequences of the choice of a linearization method in terms of spectra, i.e. for engineering purposes. In addition, it allows the removal of the variability due to ground motion in order to evaluate that due to the estimation of the response only. Moreover, the corner period \(\hbox {T}_{\mathrm{c}}\) is often used as a parameter to compute the reduction factors (e.g. Vidic et al. 1994). Spectral matching is therefore a way to have sets of ground motion time histories with a well-defined \(\hbox {T}_{\mathrm{c}}\).

The matching process is performed for the period range from the beginning of the plateau of the considered target spectrum and for a maximum of ten iterations. Statistical characteristics of the response spectra for the sets of the twelve records before and after modification for matching target spectrum show the efficiency of the technique of Abrahamson. As an example, response spectrum average and mean values plus and minus one standard deviation are plotted in Fig. 6 for ground type C. The figure clearly shows that the selection performed on the twelve records for each set already leads to a good match with respect to mean values. The modification using the technique of Abrahamson then always produces an improvement of the match and is associated to a significant reduction of the variability.

Fig. 6
figure 6

Statistical characteristics of the response spectra for the set of the twelve records selected for the best fit to the design spectrum of soils class C before (left) and after (right) modification for matching target spectrum

Full size image

3.2 Hysteretic models

The non-linear SDOF system is defined by its initial natural frequency or period T, its strength reduction factor R and the hysteretic model according to which the structure behaves in the non-linear range. Several hysteretic models were used to compute the non-linear responses: the modified Takeda-model, the Q-model (Saiidi and Sozen 1981) and the Gamma model (Lestuzzi and Badoux 2003). However, as already reported in other studies (e.g. Lestuzzi et al. 2007) the results are similar and lead to the same conclusions. Therefore, only the results related to the modified Takeda-model are presented in the following. The Takeda-model was initially proposed in an original version by Takeda et al. (1970). The modified Takeda-model was developed independently by Otani (1974) and Litton (1975). It was later adapted by many researchers. The version used here is the one of Allahabadi and Powell (1988). The related force-displacement relationship is plotted in Fig. 7. The modified Takeda-model provides a much better simulation of the behavior of materials such as reinforced concrete than the classical elasto-plastic model.

Fig. 7
figure 7

Force-displacement relationship defining the modified Takeda hysteretic model

Full size image

The force-displacement relationship of the modified Takeda-model is specified through five parameters: the initial stiffness, the yield displacement, the post-yield stiffness, a parameter relating the stiffness degradation (\(\alpha \)) and a parameter (\(\upbeta \)) specifying the target for the reloading curve. Standard values of the parameters corresponding to the widely used “small Takeda model” (\(\alpha \,=\,0.4\) and \(\upbeta \,=\,0.0\)) are used in all analyses (Lestuzzi et al. 2007). Note that a low \(\alpha \) value improves the rate of convergence of computations. Values of 0, 5 and 10 % have been tested for the hardening coefficient r (post-yield stiffness).

3.3 Processing

For each target response spectrum, the following computations are made for each strength reduction factor R, each hardening coefficient r and each selected ground motion:

  • The elastic peak displacement \(\hbox {y}_{\mathrm{el}}\) is obtained calculating the linear response of a SDOF of period T and damping ratio \(\xi \). It corresponds to the inelastic displacement according to the equal displacement rule.

  • The yield displacement associated with each computation, calculated as follows:

    $$\begin{aligned} y_y=\frac{y_{el}}{R} \end{aligned}$$
    (13)

  • The corresponding non-linear maximum displacement value \(\hbox {y}_{\mathrm{nl}}\) using the hysteretic model.

  • The displacement ductility is then computed based on the inelastic model, for a given strength reduction factor R:

    $$\begin{aligned} \mu =R \frac{y_{nl}}{y_{el}} \end{aligned}$$
    (14)

  • The displacement demand predictions according to the N2 method (Vidic et al. 1994), the version implemented in the EC8 (CEN 2004) and equal energy approximation are obtained following Eq. 2 and 3. It can be noticed that \(\mu \) is needed for the original Vidic et al. (1994) computation and \(\hbox {T}_{\mathrm{c}}\) is known since the response spectra is assumed.

  • The estimation of the linearized response as proposed by Dwairi et al. (2007) by computing the response of the SDOF for an equivalent period and damping using Eqs. 5 and 6 and the ductility.

  • Finally, the linearized response following Lin and Miranda (2008), which is estimated by computing the linear response of the SDOF for an equivalent period and damping following Eqs. 7 and 8.

  • Finally, the proposed estimates are computed using Eqs. 9 and 11.

The chosen representation of these results is the period-peak displacement plane, commonly used for displacement response spectra. Compared to the representation of the standard error with respect to a reference inelastic peak displacement, this representation shows better if the trend of the different linearized methods is correct and if their variability is coherent with the non-linear computations. For engineering purposes, the accuracy of the method is not critical but it is important not to underestimate the displacement demand that can be observed in this representation.

Moreover, this representation recalls that the spectral displacement in the plateau region is a function of the square of the period, such that a small uncertainty in the period estimation leads to large errors in the displacement demand estimate. Michel et al. (2010), computed the standard deviation of period height-relationships for RC shear wall buildings in France from ambient vibration measurements and found 0.08 s, i.e. up to 50 % of the period value on the plateau. Even numerical models, based on simplified assumptions, cannot predict the period with an excellent accuracy.

The displacement demand predicted by the different approaches is compared to the statistical characteristics of the peak displacements computed according to hysteretic model for various periods and strength reduction factors. This study is focused on displacement demand prediction in the short period range corresponding to the plateau of the related design response spectrum. Therefore, the methodology detailed above has been applied for periods T between 0.1 and 1 s and strength reduction factors R of 1.5, 2, 4 and 6. The initial damping ratio \(\xi \) was set to 5 %. A constant value for strength reduction factor R is used instead of a constant displacement ductility \(\mu \) to ensure the same non-linearity level for each ground motion. The goal of the study is to determine in which cases the different methods avoid an underestimation of the peak displacement demand.

4 Results

4.1 Performance of the classical methods

First the simplest methods are evaluated with respect to the “true” inelastic response. Fig. 8 (left part) compares this inelastic response with the linear response (equal displacement rule), the equal energy approximation and the N2 method as implemented in the EC8 (CEN 2004) for ground type B. The variability of the non-linear response is well demonstrated by the black area in Fig. 8. The simple linearization methods such as that used in EC8, on the contrary, just propagate the residual uncertainty from the spectral matching method. These methods cannot reproduce this variability so they have to choose between representing the inelastic response on average (best-estimate), for instance for risk assessment, or in a conservative way, for engineering purposes.

Fig. 8
figure 8

Displacement demand for increasing reduction factor R (ground type B, hardening coefficient r = 5 %). Comparison between elastic demand, inelastic demand (Takeda hysteretic model) and on the left: equal energy “rule” and EC8 prediction; on the right: Vidic et al. (1994); Dwairi et al. (2007) and Lin and Miranda (2008) predictions

Full size image

For low strength reduction factors (\(\hbox {R}\le 2\)), these methods provide reasonable displacements on average in the investigated period range for all ground types, even the equal displacement rule. The non-linear phenomena are limited so that the variability of the non-linear response is not critical. EC8 is slightly conservative, especially for ground type D (not displayed). The equal energy approximation gives relevant values only below 0.5 s. For intermediate strength reduction factors (R = 4), the inelastic response deviates significantly from the elastic response. At low periods (below 0.4 s), the increased inelastic displacement can be clearly seen. The equal energy approximation provides relevant values on a narrow period range only. The EC8 approach underestimates the increase in displacement at low periods, which leads to non-conservative values of inelastic displacement for periods lower than 0.3 s, i.e. for RC shear wall buildings lower than 3-stories high according to the EC8 (CEN 2004) frequency/height relationship. The inelastic displacement even exceeds the upper bound of the EC8 (infinite R – linear trend) even for R = 4 for several soil classes. It is therefore already obvious that proposition 1 is not conservative enough for moderate to large non-linearity levels. Finally, at large strength reduction factors (R = 6), these phenomena are amplified, with the equal energy approximation being simply irrelevant and EC8 strongly underestimating the displacement at low periods.

The more advanced methods of Vidic et al. (1994); Dwairi et al. (2007) and Lin and Miranda (2008), representative respectively of methods using the initial stiffness, the secant stiffness and the intermediate stiffness are compared in Fig. 8 (right part). These methods perform particularly well on average at low strength reduction factors, even reproducing partly the variability of the response. This is not valid anymore looking at higher reduction factors. The Dwairi et al. (2007) values, in particular, strongly overestimate the response at low periods, whereas the Lin and Miranda (2008) relationship may underestimate the response in some cases for very low periods. Figure 9 investigates the effect of the hardening coefficient r. No hardening (pure plastic behavior) is not realistic and leads to large displacements at low periods that are not reproduced by the different methods. More realistic values of hardening lead to a better match with the different models. This means that comparisons with zero hardening (e.g. pure elasto-plastic models) should be avoided. Otherwise, the impact of this parameter on the results is limited.

Fig. 9
figure 9

Displacement demand for increasing hardening coefficient r (ground type B and reduction factor R = 4). Comparison between elastic demand, inelastic demand (Takeda hysteretic model), Vidic et al. (1994); Dwairi et al. (2007) and Lin and Miranda (2008) predictions

Full size image

The results are slightly affected by the used hysteretic model but same trends are obtained. It can be noticed, however, that only ductile behavior is considered here. Non-ductile behavior may change the conclusions. An important conclusion here is that reproducing the non-linear response of structures using linear SDOF systems is not an easy task, even by adding more and more parameters to the relationships. Moreover, extending the study to MDOF systems would introduce even more variability (e.g. Erduran and Kunnath 2010).

The approach of choosing intermediate periods between the initial and secant stiffness (Lin and Miranda 2008) is doubtless the most relevant from a physical point of view, but these results show that the improvements it provides are not critical. Considering the fast development of non-linear modeling, linearized models have only a future for first order design and assessment purposes. Therefore, instead of looking for more complex – but still linear - models, we propose simplified, conservative estimates for design code purposes.

4.2 Performance of the proposed method

Figure 10 shows the comparison of the peak displacement predicted by the original EC8 procedure, both propositions, and the inelastic response related to the Takeda hysteretic model for various periods, strength reduction factors and for ground types A, B and C.

Fig. 10
figure 10

Displacement demand for increasing reduction factor R and the ground types A, B and C. Comparison between elastic demand, inelastic demand (Takeda hysteretic model), the two propositions in this study and EC8 prediction

Full size image

Compared to EC8 displacement demands that are not on the safe side, proposition 1 leads to better results, in the engineering sense, for moderate strength reduction factors up to R = 4. Proposition 2 may be applied for higher strength reduction factors. However, for strength reduction factors higher than R = 6, displacement demand increases significantly and the equal displacement rule is no longer valid, even for intermediate and large periods.

The same trends are related to the different ground types. However, the displacement demand predictions corresponding to ground type A are less accurate than for the other ground types. The results show that for this type of design spectra, with short corner periods of the plateau, even the displacement demand predictions according to proposition 2 underestimate the results for high strength reduction factors.

5 Summary and conclusions

The comparison of several methods (equal displacement rule – EDR –, equal energy approximation, a secant stiffness method and two empirical equivalent period and damping methods) was performed in order to evaluate their efficiency, more specifically in the period range of the plateau of the design spectra. The study focused on the reliability of the methods’ seismic displacement demand prediction with respect to their complexity when compared to the statistical characteristics of the response of the modified Takeda hysteretic model. In order to load this non-linear model, recorded ground motions were selected and slightly modified to match design spectra of different ground types in order to study the effect of the structural response, irrespective of the ground motion variability.

As shown by previous similar studies, knowledge invested in a more complex – but still linear – method can improve the accuracy of the predictions, especially for intermediate to large periods. However, this investment may not be justified for all applications such as preliminary structural design or assessment. The results show that detailed computations lead to an incorrect understanding of accuracy, since other uncertainties, such as that of the fundamental period, limit the precision of the demand estimation in any case. For low periods, none of the examined methods perform satisfactorily in all cases. Particularly, the original procedure proposed in EC8 systematically underestimates the demand in this period range. The equal energy “rule”, still often proposed for this period range, diverges dramatically. Consequently, the linear approximation seems not to be justified for the lowest periods, corresponding to low-rise buildings.

Therefore, following the “principle of consistent crudeness” (Elms 1985) two new simple, but conservative, displacement demand estimation methods are proposed. The peak displacement predicted by the original EC8 procedure and both propositions were compared the inelastic response of to the modified Takeda hysteretic model for various periods and strength reduction factors. Both propositions may be graphically approximated which is a significant advantage for practical application. The results show that, except for low strength reduction factors up to R = 2, the propositions prevent the underestimation of the displacement demand that was observed with original EC8 procedure. The propositions may be further improved for a better prediction of displacement demand. However, such an improvement would be related with a more sophisticated expression, which may not be justified for practical applications. The reported investigations are focused on SDOF and on hysteretic models featuring ductile structural seismic behavior. These options are related to the objectives of the study. Even if some slight differences arise with MDOF (e.g. Schwab and Lestuzzi 2007), the crucial characteristics of the seismic response are captured with SDOF. Compared to ductile structural behavior, limited hysteretic energy dissipation behavior still increases the non-linear demand (Lestuzzi et al. 2007) and further investigations would be necessary in order to propose adequate displacement demand predictions for such cases.

The significance of the obtained results should be distinguished between new and existing structures. The impact is relatively limited for the design of new structures. For conventional design, current procedures are reliable because only relatively small values of the strength reduction factor are allowed. The usual construction codes practice of considering the equal displacement rule for the whole period range is even validated by the results. For ductile design, such as capacity design, restricted modifications may be involved for the low period range only. By contrast, for the seismic assessment of existing structures, such as unreinforced masonry low-rise buildings, the current procedure of EC8 should be modified in order to provide accurate predictions of the displacement demand in the domain of the response spectrum plateau. Current procedure is reliable only for small values of the strength reduction factors (\(\hbox {R}\le 2\)). For higher values of strength reduction factors, the two propositions developed in this study lead to significantly more relevant displacement demand prediction. Consequently, it is suggested to replace the current EC8 procedure by the proposition 1 for strength reduction factors between 2 and 4 and by proposition 2 for higher strength reduction factors.