Introduction

For non-swelling clay, the effect of compaction on the saturated hydraulic conductivity, k sat, has been thoroughly investigated. Terzaghi (1922) found that k sat depends on porosity n, and also on the preparation and compaction modes. Mitchell et al. (1965) have shown that specimens compacted wet of optimum may have k sat values 100–1,000 times lower than specimens compacted dry of optimum. These authors defined two porosities. The porosity of the clay matrix (primary porosity) corresponds to the fine structure at the micron scale of solid particles. The porosity between artificially formed clay clods (secondary porosity) corresponds to a macrostructure resulting from remolding (excavation, transport, handling) or weathering processes (wetting–drying, freeze-thaw). These two porosities can be quantified using techniques such as the mercury intrusion test or scanning electron microscopy (e.g., Delage et al. 1982). The k sat value of compacted non-swelling clay was found to depend mainly on the secondary porosity. Swelling clays (e.g., bentonite) behave differently and can achieve fairly low k sat values even when compacted dry of optimum (e.g., Chapuis 1981, 2002; Chapuis et al. 1992; Haug and Wong 1992).

In practice, compacted clay layers are controlled using indirect methods (e.g., dry density and water content) and direct methods (e.g., field permeability tests). According to experience, many field tests are done to assess the compaction condition whereas only a few permeability tests are performed. Being able to predict the k sat value at each location at which a compaction test was performed would provide a large data bank of predicted values to be used to assess the overall performance of the liner or cover. This paper proposes a predictive model for k sat and a simple graphical method to predict k sat from the local field values of dry density and water content just after compaction.

Known parameters influencing k sat

Call-for-tender documents for clay liners and covers specify clay compaction conditions. They usually include a minimum dry density (e.g., 95% of the standard Proctor or 90% of the modified Proctor), a minimum water content (e.g., the optimum value) and sometimes a minimum degree of saturation (e.g., 90%) which is known to be important to achieve a low k sat. In a standard or modified Proctor test, the wet side of the compaction curve corresponds to a degree of saturation, S r, of about 90% for clay (Fig. 1). Discontinuous tiny air bubbles, representing typically about 10% of the void space, are trapped in the compacted clay. They cannot be evacuated during compaction because the clay becomes impervious to air (e.g., Fredlund and Morgenstern 1977). In turn, this indicates an excellent interlocking of the clods and a low k sat. This near-zero air-permeability of well-compacted clay was used in some projects as a means to control compaction conditions in the field (e.g., Langfelder et al. 1968; Leflaive and Schaeffner 1980).

Fig. 1
figure 1

Examples of typical compaction curves for clay

Full size image

Other criteria are related to the liner total thickness, thickness of each layer (usually between 15 and 30 cm), compaction method (e.g., a sheep foot roller with a minimum length of 20 cm for the steel feet) and sometimes a maximum clod size. Either the standard or the modified Proctor test may be used as reference since the two optimum dry densities and the two optimum water contents are well correlated according to Chapuis (2002) who gathered data published by Sherwood (1975), Blotz et al. (1998) and Benson et al. (1999).

A good interlocking, minimizing the secondary porosity, is more easily achieved when the clay deforms without fissuring. This means that the molding water content should be higher than the clay plastic limit. Arquié (1964) and others (e.g., Leroueil et al. 1992) noted that the clay plastic limit is often close to the optimum water content of the standard Proctor. However, Chapuis (2002) found that the correspondence is very approximate, using data from Daniel (1984, 1985), Boynton and Daniel (1985), Day and Daniel (1985), Mundell and Bailey (1985), Stewart and Nolan (1987), Daniel and Benson (1990), Elsbury et al. (1990), Leroueil et al. (1992), Blotz et al. (1998), Benson et al. (1999) and personal data. The correlation between the optimum water content of the standard Proctor and the liquid limit is equally poor, as shown by Granger (1969), Popovic and Sarac (1980), Benson et al. (1999) and Chapuis (2002). Since these relationships are approximate, it is preferable, in call-for-tender documents, to specify the minimum water content in the field as the plastic limit, instead of the optimum of the standard Proctor, when the soil to be compacted has no swelling properties.

Other considerations for compacted clay relate to the ability of field equipment to achieve the desired compaction in order to obtain a low k sat value. First, the clay plastic limit may be used as a reference for its undrained shear strength, c u, that is close to 140 kPa (Biarez 1972). According to plasticity theory for isotropic materials, its surface bearing capacity is equal to (π + 2)c u = 700 kPa. Thus, it is difficult to knead and compact the clay at this water content. This is why a sheep foot roller is frequently used. For a dam core, the water content must be low enough to minimize settlements during construction and after reservoir filling. For liners, mechanical properties are usually less critical but the clay must be able to deform without fissuring. The water content can be fairly high as long as it allows the circulation of compaction equipment. This may be viewed as either a penetration resistance (e.g., Faure and Da Mata 1994) or a bearing capacity problem that is usually solved when the clay’s undrained shear strength, c u, is higher than 30 kPa. According to Leroueil et al. (1992), this is achieved when the ratio (w - w opt)/I P is lower than 0.3, where w opt is the standard Proctor optimum water content and I P is the plasticity index.

Finally, a non-swelling compacted clay must not dry or freeze before being in use, otherwise its k sat value could increase by a factor of 100–1,000 and other properties may be affected (Chamberlain and Gow 1979; Zimmie 1992; Benson and Othman 1993; Othman et al. 1995; Eigenbrod 1996).

Relationship for k sat of compacted clay

Considering the large number of parameters influencing k sat, several approaches can be taken to try to predict it. For predicting the performance of liners, it is important to use k sat which is the maximum value of k (all voids are filled with water) that can be reached for a specimen. Among several predictive methods, Kodikara and Rahman (2002) tried to correlate the moisture contents for the minimum re-saturated k value and the maximum wet unit weight. Benson et al. (1994) defined several trends after having performed a multivariate regression analysis of laboratory determinations of k conducted on a wide variety of clayey soils from the liners of 67 landfills.

In this paper, the authors use a physically based model relating k sat to the secondary porosity of re-saturated compacted clay (Chapuis 2002). At the end of compaction, all water is assumed to be held within the clods and none between the clods, the secondary porosity being filled with air only. Thus, the air volumetric fraction, or secondary porosity of the compacted clay equals n c(1 - S rc) where n c is the total porosity after compaction, and S rc is the degree of saturation after compaction (Fig. 2). When the compacted clay specimen is prepared for permeability testing, its degree of saturation increases from S rc to S r (usually close to 100% when back-pressuring is used). According to several authors, the size of laboratory compacted specimens (about 100 or 150 mm in diameter) is usually large enough to avoid the problem of scale effect that can appear with smaller specimens (e.g., Cazaux and Didier 2002).

Fig. 2
figure 2

Schematic illustration of secondary porosity in compacted non-swelling clay

Full size image

The fluid movement in an ideal single fracture yields the Poiseuille flow frequently quoted as the “cubic law.” In real saturated fractured materials, a continuum formulation can be used in which the k sat value may be correlated to the cube of the water filled secondary porosity. The latter is equal to the void volume fraction after compaction, n c, minus the volume of bound water, n c S rc. Thus,

$$ k_{{{\text{sat}}}} = {\text{function}}\;{\text{of}}\;n^{3}_{{\text{c}}} (1 - S_{{{\text{rc}}}} )^{3} . $$
(1)

Laboratory data for several compacted clays in Quebec projects (Fig. 3) confirm Eq. 1. Results for other compacted clays (Lambe 1958; Mitchell et al. 1965) provided similar results (Chapuis 2002), the k sat value being more generally represented by

$$ k_{{{\text{sat}}}} = an^{b}_{{\text{c}}} (1 - S_{{{\text{rc}}}} )^{b} $$
(2)

where a and b are factors derived by curve-fitting. Equation 2 predicts k sat=0 for a specimen that is fully saturated after compaction, which is unrealistic. This is why a minimum value of 10−8 cm/s can be considered when using Eq. 2.

Fig. 3
figure 3

Permeability test results for re-saturated compacted non-swelling clay

Full size image

In Fig. 3, the bisecting line represents the case of Eq. 2 where a=10−2 cm/s and b=3. For other non-swelling clays, the specific values of a and b can be determined experimentally.

Graphical presentation of compaction data

Compaction formulae relate the dry density ρ d and the degree of saturation S r to the densities of solids, ρ s, and water ρ w, and the void ratio, e, by

$$ \rho _{{\text{d}}} /\rho _{{\text{w}}} = G_{{\text{s}}} /(1 + e) $$
(3)
$$ S_{{\text{r}}} = w(\rho _{{\text{d}}} /\rho _{{\text{w}}} )G_{{\text{s}}} /(G_{{\text{s}}} - (\rho _{{\text{d}}} /\rho _{{\text{w}}} )) $$
(4)

where G s = ρ s/ρ w is the specific gravity of solid.

The compaction test results are conventionally plotted in a graph (x = w; y = ρ d/ρ w = γ d/γ w). Then, Eq. 4 becomes

$$ S_{{\text{r}}} = xyG_{{\text{s}}} /(G_{{\text{s}}} - y) $$
(5)

that can be rearranged as

$$ y = \frac{{S_{{\text{r}}} G_{{\text{s}}} }} {{xG_{{\text{s}}} + S_{{\text{r}}} }} $$
(6)

indicating that the curves of constant S r values are hyperbolas in the frame (x, y). Instead of using the conventional graph, this paper proposes to plot the data as X = w and Y = ρ w/ρ d = y −1. Thus Eq. 4 becomes

$$ Y = \frac{{XG_{{\text{s}}} + S_{{\text{r}}} }} {{S_{{\text{r}}} G_{{\text{s}}} }} = \frac{X} {{S_{{\text{r}}} }} + \frac{1} {{G_{{\text{s}}} }} = \frac{{1 + e}} {{G_{{\text{s}}} }} $$
(7)

which means that the curves of constant S r values become straight lines in the frame (X, Y). All constant S r straight lines intersect at (X=w=0, Y = ρ w/ρ d = 1/G s).

Curves of equal k sat value

The equation of equal k sat curve for the proposed model of Eq. 2 can be established as follows. In the usual compaction graph with the coordinates (x, y), as previously defined, S r is defined by Eq. 4 whereas the porosity n is

$$ n = (G_{{\text{s}}} - y)/G_{{\text{s}}} . $$
(8)

Substituting Eqs. 5 and 8 into Eq. 2 yields

$$ k_{{{\text{sat}}}} ({\text{cm}}/{\text{s}}) = a[(G_{{\text{s}}} - y - xyG_{{\text{s}}} )/G_{{\text{s}}} ]^{b} $$
(9)

which gives the equation of equal k sat curve as

$$ y = G_{{\text{s}}} (1 - \alpha )/(1 + xG_{{\text{s}}} ) $$
(10)

in which the constant α is defined as

$$ \alpha = (k_{{{\text{sat}}}} /a)^{{1/b}} $$
(11)

where k sat and a have the same unit, e.g., cm/s.

Thus in the usual graph, the curves of constant k sat value are hyperbolas according to Eq. 10, and the curves of constant S r value are also hyperbolas. In the proposed reference plane (X, Y), it has already been shown that the curves of constant S r values are straight lines. Similarly, the curves of equal k sat value become straight lines defined from Eq. 10 as

$$ Y = (1 + XG_{{\text{s}}} )/G_{{\text{s}}} (1 - \alpha ) $$
(12)

that appear in Fig. 4, in which the linear properties facilitate any reading or interpolation. Note that the mean values a=10−2 cm/s and b=3 of Fig. 3 have been used to draw Fig. 4.

Fig. 4
figure 4

Proposed plotting for curves of equal degree of saturation (fine solid lines) and equal values of saturated hydraulic conductivity after compaction and re-saturation (bold broken lines)

Full size image

Assessing freeze-thaw effects

Damage induced by freeze-thaw cycles can be assessed using the previous dual porosity model, considering that the damage is mainly an increased secondary porosity. The total porosity and degree of saturation after compaction are noted n c and S rc, whereas they are noted n f and S rf after freeze-thaw cycles. If the compacted clay was never frozen and is tested at S r≤100%, its water filled secondary porosity is n c(S r – S rc) and thus, according to the model, its hydraulic conductivity, k, not necessarily saturated, is

$$ k_{{\text{c}}} ({\text{cm}}/{\text{s}}) = an^{b}_{{\text{c}}} (S_{{\text{r}}} - S_{{{\text{rc}}}} )^{b} . $$
(13)

The compacted clay, after freeze-thaw cycles, is tested for permeability at S r≤100%. The water filled secondary porosity is now the difference between the water volume during testing, n f S r, and the volume of bound water, n c S rc, and thus the hydraulic conductivity k f is given as

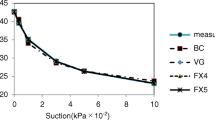
$$ k_{{\text{f}}} ({\text{cm}}/{\text{s}}) = a(n_{{\text{f}}} {\text{S}}_{{\text{rf}}} - n_{{\text{c}}} S_{{{\text{rc}}}} )^{b} . $$
(14)

The adequacy of Eq. 14 to represent the k f value of frost-damaged compacted clay was assessed with the detailed data provided by Aubertin and Dagenais (2000) for four clay specimens. The specimens were tested in rigid-wall permeameters after compaction and then after 5, 10, 15 and 20 freeze-thaw cycles (no external effective stress was applied during these cycles). The degree of saturation for each permeability test was evaluated using the mass and volume method (Chapuis et al. 1989; Chapuis 2004). When plotted versus the right member of Eq. 14, the test data (Fig. 5) indicate that the proposed model can correctly assess the impact of frost damage on k sat.

Fig. 5
figure 5

Assessing the effects of freeze-thaw and drying–wetting cycles

Full size image

Discussion and conclusion

Laboratory compaction data are usually plotted as y = ρ d/ρ w = γ d/γ w versus x = w. In the (x, y) frame, the curves of equal degree of saturation S r are hyperbolas. It is proposed here to plot the data as Y = (ρ d/ρ w)−1 versus X = w. In the new (X, Y) frame, the curves of equal S r are straight lines passing through the point X=0, Y = ρ w/ρ d = 1/G s. This new frame proves useful when assessing the saturated hydraulic conductivity, k sat, of compacted non-swelling clays. Based on laboratory test data, a model is proposed to predict k sat as a function of the compacted clay secondary porosity. The curves of equal predicted values of k sat appear as straight lines in the new (X, Y) frame.

Once laboratory permeability tests have been performed on the compacted clay to determine the values of its a and b parameters in the model, the proposed plotting frame and the linearity of the predictive equations can be used to assess rapidly, in the field, the k sat value at each location where the compaction is assessed. This, in turn, can help to assess the overall performance of a clay liner or cover, which is a statistically complicated problem, highly dependent on extreme k values (e.g., Chapuis 1990, 2002; Benson et al. 1999; Guyonnet et al. 2003), which means that minor defects have a large influence on the overall performance.