Motion Around Out-of-Plane Equilibrium Points in the Frame of Restricted Six-Body Problem Under Radiation Pressure

Abstract

The goal of the present manuscript is to study the existence of out-of-plane equilibrium points and their stability in the photogravitational restricted six-body problem. It is found that there exist two out-of-plane equilibria under the restrictions $-\frac{4\mu }{1-4\mu }<q<0$ and $0< \mu < 0.25$, where q and $\mu$ are the radiation factor and mass parameter, respectively. It is also observed that the out-of-plane equilibria are linearly unstable in the above mentioned interval. With the help of Poincaré section, we have examined the orbits such as consistent, periodic, quasi-periodic, disordered, or stochastic.

1 Introduction

The nonlinear models in the field of Celestial Mechanics and Space Dynamics, the N-body problem has a very significant contribution and therefore have gained considerable attention of scholars working in this field. There are various solicitations in the playing field of galactic dynamics and motion of planetary objects. An example of such model is a well-known restricted problem of N bodies. Many researchers studied different aspects of it such as:

Ragos and Zagouras [17] have discussed periodic solutions about the out-of-plane equilibrium points in the photogravitational restricted three-body problem. Douskos and Markellos [4] have studied out-of-plane equilibrium points in the restricted three-body problem with oblateness. Das et al. [3] have studied out-of-plane equilibrium points in photogravitational restricted three-body problem. Douskos [5] has discussed collinear equilibrium points of Hill’s problem with radiation and oblateness and their fractal basin of attraction. Idrisi and Taqvi [11] have studied restricted three-body problem when one of the primaries is an ellipsoid. Singh and Umar [18] have worked on out-of-plane equilibrium points in the elliptic restricted three-body problem with radiating and oblate primaries. Ullah et al. [22] have introduced a cyclic kite configuration. Idrisi [12] has shown existence and stability of the libration points in CR3BP when the smaller primary is an oblate spheroid.

Abouelmagd and Mostafa [1] have studied out-of-plane equilibrium points, locations and the forbidden movement regions in the restricted three-body problem with variable mass. Singh and Amuda [21] have worked on out-of-plane equilibrium points in the photogravitational CR3BP with oblateness and Poynting Robertson drag. Singh and Vincent [19] have worked on out-of-plane equilibrium points in the photogravitational restricted four-body problem. Singh and Vincent [20] have worked on out-of-plane equilibrium points in the photogravitational restricted four-body problem with oblateness. Idrisi and Ullah [8] have worked on non-collinear libration points in ER3BP with albedo effect and oblateness. Narayan et al. [16] have studied the effect of oblateness on existence and stability of out-of-plane equilibrium points in spatial elliptic restricted three-body problem. Abouelmagd et al. [2] have discussed planar five-body problem in a framework of heterogeneous and mass variation effects.

Idrisi and Ullah [7, 10, 13] have studied non-collinear libration points under the combined effects of radiation pressure and Stokes drag. Idrisi and Ullah [7, 10, 13] have introduced central-body square configuration of restricted six-body problem and studied the libration points and their stability in linear sense. Idrisi and Ullah [7, 10, 13] have shown albedo effects on libration points in the elliptic restricted three-body problem. Idrisi et al. [6] have studied elliptic restricted synchronous three-body problem with a mass dipole model. Kumar et al. [14, 15] have studied the unpredictable basin boundaries in restricted six-body problem with square configuration. Kumar et al. [14, 15] have studied capricious basin of attraction in photogravitational magnetic binary problem. Idrisi et al. [9] have discussed the effect of perturbations in coriolis and centrifugal forces on libration points in the restricted six-body problem. Ullah et al. [23] have studied Sitnikov five-body problem with combined effects of radiation pressure and oblateness.

In the present manuscript, we have studied the out-of-plane equilibria in restricted six-body problem and we have organized the present work as follows: The formulation of the problem and equations of motion are explained in Sect. 2. The out-of-plane equilibrium points are derived in Sect. 3. In Sect. 4, linear stability of out-of-plane equilibrium points is found numerically and discussed graphically. In Sect. 5, the Poincare sections are discussed graphically. In Sect. 6, the families of periodic orbits are discussed graphically. In the last Sect. 7, conclusions and discussion of the problem are drawn.

2 Equations of Motion

In the central-body square configuration of restricted six-body problem it is assumed that the four less massive primaries of equal masses form a square such that two primaries are always on x-axis, two on y-axis and a more massive primary which is a source of radiation is placed at the center of mass of the system (Fig. 1). The less massive primaries are moving in a circular orbit of radius a with angular velocity $\omega$ around the center of mass of the system. The only force considered on the primaries is Newtonian gravitational force. If l is the side of square then by geometry it is easy to show that $l = \surd 2a$. By fixing the units, i.e., $a = 1$ and $G = 1$ the gravitational potential on infinitesimal mass $m'$ per unit mass in the restricted six-body problem in synodic coordinate system (x, y, z) is given by,

$$\begin{aligned} -V=\frac{n^{2}}{2}\left( {x^{2}+y^{2}} \right) +\frac{q(1-4\mu )}{r_{0} }+\sum \limits _{i=1}^4 {\frac{\mu }{r_{i} }} , \end{aligned}$$

(1)

where q is the radiation factor and n is the mean-motion of the primaries and defined as $n^{2} = 1 - c\mu , \mu = m / M$ is the mass parameter ($0< \mu < 0.25), M$ is total of mass of the system taken as unity and $c = (15\surd 2 - 4) / 4\surd 2, r_{0}^{2} = x^{2} + y^{2}+z^{2}$, $r_{1}^{2} = (x - 1)^{2} + y^{2} +z^{2}, r_{2}^{2} = x^{2} + (y - 1)^{2}+z^{2}, r_{3}^{2} = (x + 1)^{2} + y^{2} +z^{2}, r_{4}^{2} = x^{2} + (y + 1)^{2} +z^{2}$ (Idrisi and Ullah, [7, 10, 13]).

The Lagrangian of the restricted six-body problem in the rotating frame ($\xi , \eta , \zeta )$ is given by

$$\begin{aligned} L\left( {\xi ,\eta ,\zeta ,\dot{{\xi }},\dot{{\eta }},\dot{{\zeta }}} \right) =T-V=\frac{1}{2}\left( {\dot{{\xi }}^{2}+\dot{{\eta }}^{2}+\dot{{\zeta }}^{2}} \right) +\frac{n^{2}}{2}\left( {\xi ^{2}+\eta ^{2}} \right) +\frac{q(1-4\mu )}{r_{0} }+\sum \limits _{i=1}^4 {\frac{\mu }{r_{i} }} , \end{aligned}$$

(2)

where T and V are the kinetic and potential energies of infinitesimal mass $m'$per unit mass, respectively.

As

$$\begin{aligned} \xi = x \cos \omega + y \sin \omega , \eta = x \sin \omega -y \cos \omega , \zeta = z \end{aligned}$$

(3)

$\omega =nt$ is the angular velocity of the system.

Using (1) and (3) in Eq. (2), we have

$$\begin{aligned} L\left( {x,y,z,\dot{{x}},\dot{{y}},\dot{{z}}} \right) =\frac{1}{2}\left[ {\left( {\dot{{x}}^{2}+\dot{{y}}^{2}+\dot{{z}}^{2}} \right) +n^{2}\left( {x^{2}+y^{2}} \right) +2n\left( {x\dot{{y}}-y\dot{{x}}} \right) } \right] +\frac{1-4\mu }{r_{0} }+\sum \limits _{i=1}^4 {\frac{\mu }{r_{i} }} . \end{aligned}$$

(4)

Let $p_{i}$ be the corresponding momenta of $q_{i}$, therefore using the definition of momentum $p_{i} =\frac{\partial L}{\partial \dot{{q}}_{i} },i=\,1,\,2,\,3,$ we have

$$\begin{aligned} p_{x} =\dot{{x}}-ny,\,p_{y} =\dot{{y}}+nx,\,p_{z} =\dot{{z}}, \end{aligned}$$

(5)

where $p_{1} = p_{x}$, $p_{2} = p_{y}$, $p_{3} = p_{z}$, $q_{1} =x$, $q_{2} = y$, $q_{3} = z$.

The Hamiltonian function is defined as

$$\begin{aligned} \hbox {H}(q_{1} ,q_{2} ,q_{3} ,p_{1} ,p_{2} ,p_{3} )=\sum \limits _{i=1}^3 {p_{1} \dot{{q}}_{i} } -L. \end{aligned}$$

(6)

Using (4) and (5) in (6), the Hamiltonian of restricted six-body problem takes the form

$$\begin{aligned} H(x,y,z,p_{x} ,p_{y} ,p_{z} )=\frac{1}{2}\left[ {\left( {p_{x}^{2} +p_{y}^{2} +p_{z}^{2} } \right) -2n\left( {xp_{y} -yp_{x} } \right) } \right] -\left[ {\frac{q(1-4\mu )}{r_{0} }+\sum \limits _{i=1}^4 {\frac{\mu }{r_{i} }} } \right] . \end{aligned}$$

(7)

The Hamiltonian equations of motion of infinitesimal mass $m'$ in the synodic system (x, y, z) are given by

$$\begin{aligned} \left. {\begin{array}{l} \dot{{x}}=\frac{\partial H}{\partial p_{x} }=p_{x} +ny,\,\,\dot{{y}}=\frac{\partial H}{\partial p_{y} }=p_{y} -nx,\,\,\dot{{z}}=\frac{\partial H}{\partial p_{z} }=p_{z} , \\ \dot{{p}}_{x} =-\frac{\partial H}{\partial x}=\dot{{y}}+n^{2}x-\left[ {\frac{q(1-4\mu )}{r_{0}^{3}}x+\frac{\mu }{r_{1}^{3} }(x-1)+\frac{\mu }{r_{2}^{3} }x+\frac{\mu }{r_{3}^{3} }\left( {x+1} \right) +\frac{\mu }{r_{4}^{3} }x} \right] , \\ \dot{{p}}_{y} =-\frac{\partial H}{\partial y}=-\dot{{x}}+n^{2}y-\left[ {\frac{q(1-4\mu )}{r_{0}^{3}}y+\frac{\mu }{r_{1}^{3} }y+\frac{\mu }{r_{2}^{3} }\left( {y-1} \right) +\frac{\mu }{r_{3}^{3} }y+\frac{\mu }{r_{4}^{3} }\left( {y+1} \right) } \right] , \\ \dot{{p}}_{z} =-\frac{\partial H}{\partial z}=-z\left[ {\frac{q(1-4\mu )}{r_{0}^{3}}+\frac{\mu }{r_{1}^{3} }+\frac{\mu }{r_{2}^{3} }+\frac{\mu }{r_{3}^{3} }+\frac{\mu }{r_{4}^{3} }} \right] .\\ \end{array}} \right\} \end{aligned}$$

(8)

Now, the Jacobi integral can be defined as

$$\begin{aligned} v^{2}=\dot{{p}}_{x}^{2} +\dot{{p}}_{y}^{2} +\dot{{p}}_{z}^{2} =-2V+C \end{aligned}$$

(9)

As the velocity vof infinitesimal mass $m'$ tends to zero, Eq. (9) gives

$$\begin{aligned} C = 2V \end{aligned}$$

(10)

where C is known as Jacobi constant and Eq. (10) is used to study zero velocity curves, Poincare surface of sections and the stability of motion of infinitesimal mass around the libration points.

Fig. 1

Configuration of the problem

3 Out-of-Plane Equilibrium Points

The equilibrium points can be found by setting all velocity and acceleration components of infinitesimal mass $m'$ to zero in Eq. (8), we have

$$\begin{aligned} n^{2}x-\left[ {\frac{q(1-4\mu )}{r_{0}^{3}}x+\frac{\mu }{r_{1}^{3} }(x-1)+\frac{\mu }{r_{2}^{3} }x+\frac{\mu }{r_{3}^{3} }\left( {x+1} \right) +\frac{\mu }{r_{4}^{3} }x} \right]= & {} 0,\end{aligned}$$

(11)

$$\begin{aligned} n^{2}y-\left[ {\frac{q(1-4\mu )}{r_{0}^{3}}y+\frac{\mu }{r_{1}^{3} }y+\frac{\mu }{r_{2}^{3} }\left( {y-1} \right) +\frac{\mu }{r_{3}^{3} }y+\frac{\mu }{r_{4}^{3} }\left( {y+1} \right) } \right]= & {} 0 \end{aligned}$$

(12)

$$\begin{aligned} -z\,\left[ {\frac{q(1-4\mu )}{r_{0}^{3}}+\frac{\mu }{r_{1}^{3} }+\frac{\mu }{r_{2}^{3} }+\frac{\mu }{r_{3}^{3} }+\frac{\mu }{r_{4}^{3} }} \right]= & {} 0\,. \end{aligned}$$

(13)

As we are interested in out-of-plane equilibria, i.e., $z \ne 0$, therefore from Eq. (13), we have

$$\begin{aligned} \frac{q(1-4\mu )}{r_{0}^{3}}+\frac{\mu }{r_{1}^{3} }+\frac{\mu }{r_{2}^{3} }+\frac{\mu }{r_{3}^{3} }+\frac{\mu }{r_{4}^{3} }=0\,. \end{aligned}$$

(14)

Using (14) in (11) and (12), we get

$$\begin{aligned} n^{2}x+\frac{\mu }{r_{1}^{3} }-\frac{\mu }{r_{3}^{3} }=0 \end{aligned}$$

(15)

and

$$\begin{aligned} n^{2}y+\frac{\mu }{r_{2}^{3} }-\frac{\mu }{r_{4}^{3} }=0. \end{aligned}$$

(16)

$r_{1} =r_{3} \Rightarrow x=0$ and $r_{2} =r_{4} \Rightarrow y=0$ are the solutions of Eqs. (15) and (16), respectively. Now, substitute $x = 0$ and $y =$ 0 in Eq. (14), we get

$$\begin{aligned} \frac{q(1-4\mu )}{r_{00}^{3} }+\frac{4\mu }{r_{10}^{3} }=0\,, \end{aligned}$$

(17)

where $r_{00} = z_{0}, r_{10} = (z_{0}^{2} + 1)^{1/2}$ and the subscript ‘0’ is used to denote the equilibrium values.

From Eq. (17), we have that

$$\begin{aligned} \frac{r_{00} }{r_{10} }=\left[ {-q\frac{1-4\mu }{4\mu }} \right] ^{1/3}\equiv \Lambda . \end{aligned}$$

(18)

For the existence of any real solution of Eq. (18), one of the following conditions is necessary to hold, i.e., $q < 0$ or $\frac{1-4\mu }{4\mu }<0.$ The second condition does not hold as it contradicts the assumption $\mu < 0.25$. Thus, $q < 0$ (radiation pressure force exceeds its gravitational attraction) holds good to obtain real solutions of Eq. (18).

Thus, solving Eq. (18) we have two real solutions given below

$$\begin{aligned} z_{0} =\pm \sqrt{\frac{\Lambda ^{2}}{1-\Lambda ^{2}}} . \end{aligned}$$

(19)

It is seen that there exists only two equilibrium points on z-axis (we denote these two equilibria as $E_{1,2}^{z} )$. For the existence of equilibria $E_{1,2}^{z} , \Lambda$ must lie in the interval (0, 1) which is possible if and only if $-\frac{4\mu }{1-4\mu }<q<0$. For different values of mass parameter $\mu$, we have different intervals of radiation factor q as shown in Table 1. The numerical positions of equilibrium points corresponding to $\mu = 0.01$ are given in Table 2, and graphically shown in Figs. 2 and 3. We observed that as q approaches to zero, the equilibrium point $E_{1}^{z}$ moves downward while $E_{2}^{z}$moves upward along z-axis and both approaches to center of mass of the system.

Table 1 Interval of q for different values of $\mu$

Table 2 Positions of $E_{1,2}^{z} (0,0, \pm z)$

Fig. 2

q versus $E_{1,2}^{z}$for $\mu = 0.01$

4 Stability of Out-of-Plane Equilibrium Points

To study the linear stability of the equilibrium points $E_{1,2}^{z} (0, 0, \pm z_{0})$, we displace the infinitesimal mass to the point ($\xi ,\eta , z_{0} + \zeta )$, where $\xi , \eta$ and $\zeta$ are the corresponding perturbations along the axes Ox, Oy and Oz, respectively. So, linearizing the system (1) we obtain the variational equations of motion as

$$\begin{aligned} \left. {\begin{array}{l} \ddot{{\xi }}-2n\,\dot{{\eta }}=\xi {\mathop {V}\limits ^{\circ }}_{xx} \,+\,\eta \,{\mathop {V}\limits ^{\circ }}_{xy} +\zeta {\mathop {V}\limits ^{\circ }}_{xz} \, \\ \ddot{{\eta }}+2n\dot{{\xi }}=\xi {\mathop {V}\limits ^{\circ }}_{yx} \,+\,\eta \,{\mathop {V}\limits ^{\circ }}_{yy} +\zeta {\mathop {V}\limits ^{\circ }}_{yz} \, \\ \ddot{{\zeta }}=\xi {\mathop {V}\limits ^{\circ }}_{zx} \,+\,\eta \,{\mathop {V}\limits ^{\circ }}_{zy} +\zeta {\mathop {V}\limits ^{\circ }}_{zz}\\ \end{array}} \right\} \end{aligned}$$

(20)

where ‘o’ indicates that the partial derivatives are to be calculated at the equilibrium points under consideration.

The partial derivatives in system of Eq. (20) are defined as follows:

$$\begin{aligned}&{\mathop {V}\limits ^{\circ }}_{xy} \,={\mathop {V}\limits ^{\circ }}_{yx} ={\mathop {V}\limits ^{\circ }}_{yz} = {\mathop {V}\limits ^{\circ }}_{zy} ={\mathop {V}\limits ^{\circ }}_{xz} ={\mathop {V}\limits ^{\circ }}_{zx} =0,\\&{\mathop {V}\limits ^{\circ }}_{xx} \,={\mathop {V}\limits ^{\circ }}_{yy} =n^{2}-\frac{q(1-4\mu )}{r_{00}^{3} }-\frac{4\mu }{r_{10}^{3} }-\frac{6\mu }{r_{10}^{5} }, \\&{\mathop {V}\limits ^{\circ }}_{zz} =-\frac{q(1-4\mu )}{r_{00}^{3} }-\frac{4\mu }{r_{10}^{3} }-3z_{0}^{2} \left[ {\frac{q(1-4\mu )}{r_{00}^{5} }+\frac{4\mu }{r_{10}^{5} }} \right] . \end{aligned}$$

The characteristic equation corresponding to the system of Eq. (20) is given by

$$\begin{aligned} f(\lambda )=\lambda ^{6}+\alpha \,\lambda ^{4}+\,\beta \lambda ^{2}+\gamma =0,\, \end{aligned}$$

(21)

is a sixth degree equation in $\lambda$, where

$$\begin{aligned} \alpha= & {} 4n^{2}-{\mathop {V}\limits ^{\circ }}_{xx} \,-{\mathop {V}\limits ^{\circ }}_{yy} -{\mathop {V}\limits ^{\circ }}_{zz}, \\ \beta= & {} {\mathop {V}\limits ^{\circ }}_{xx} \,{\mathop {V}\limits ^{\circ }}_{yy} +{\mathop {V}\limits ^{\circ }}_{yy} \,{\mathop {V}\limits ^{\circ }}_{zz} +{\mathop {V}\limits ^{\circ }}_{zz} \,{\mathop {V}\limits ^{\circ }}_{xx} -4{\mathop {V}\limits ^{\circ }}_{zz} , \\ \gamma= & {} -{\mathop {V}\limits ^{\circ }}_{xx} \,{\mathop {V}\limits ^{\circ }}_{yy} {\mathop {V}\limits ^{\circ }}_{zz}. \end{aligned}$$

The roots $\lambda _{i} (i = 1, 2, 3, 4, 5, 6)$ of the characteristic Eq. (21) determine the stability or instability of the respective equilibrium points. An equilibrium point is said to be stable if the characteristic equation has six imaginary roots or complex roots with non-positive real parts.

On solving the characteristic Eq. (21) for different values of $\mu$, we found that two roots are real and four are pure imaginary which lead to the instability of the equilibrium points. The real roots of the characteristic Eq. (21) are also shown in Fig. 3. Thus, the equilibrium points obtained in the out-of-plane circular restricted six-body problem are unstable in linear sense for all values of mass parameter, $i.e., 0< \mu <0.25$. The roots of the characteristic Eq. (21) corresponding to $\mu = 0.01$ are given in Table 3.

Table 3 Stability of $E_{1,2}^{z}$

Fig. 3

Real roots of characteristic equation

5 Poincaré Section

In the field of Celestial Mechanics and Space Dynamics, a Poincaré section is the intersection of a point in which the system returns to after a certain number of function iteration in the set of all possible configurations of a system. A state-space of a continuous dynamical system with a certain lower-dimensional subspace is called the Poincaré section. The Poincaré section and families of periodic orbits are obtained from the given equation

$$\begin{aligned} \frac{d^{2}z}{dt^{2}}=-z\left\{ {\frac{\left( {1-4\mu } \right) q}{z^{3}}+\frac{4\mu }{\left( {z^{2}+1} \right) ^{3/2}}} \right\} \end{aligned}$$

(22)

Since the out-of-plane equilibrium points are lying on z-axis only. Therefore, on substituting $x = 0$ and $y = 0$ in Eq. (8), we get Eq. (22).

In this section, we have considered the motion of infinitesimal mass $m'$ under the combined effects of mass parameter $\mu$ and radiation factor q as consistent, periodic, quasi-periodic, disordered, or stochastic by using Poincaré section. We have plotted the Poincaré section by sequentially increasing the values of mass parameter $\mu$ and radiation factor q. We performed many tests by fixing mass parameter $\mu$ and increasing radiation factor q successively. Our tests and explanations on behalf of Poincaré section on the motion of infinitesimal mass $m'$ (Fig. 4) are given as follows:

(i) For $\mu = 0.01$ and $q = -0.0006567$, the Poincaré sections are plotted in Fig.4a and the following points have been observed:

• The regions of motion of infinitesimal mass $m'$ are valid for $z\in \left( {\hbox {0,\, 5}} \right)$ and $\dot{{z}}\in \left[ {-\,0.241,\,\,0.241} \right]$.

• The plotted Poincaré sections are symmetric about the oscillatory motion ($\dot{{z}})$.

• All the curves are invariant in the graph of Poincaré sections. These invariant curves represent quasi-periodic motion of infinitesimal mass $m'$.

Increasing the radiation factor $q = - 0.0000267$ for the same data taken in figure 4a, it is seen that quasi-periodic motion occurs in the graph of Poincaré sections. It is also seen that the region of oscillation increases as we increase radiation pressure (Fig.4b).

(ii) For $\mu = 0.10$ and $q = - 0.156667$, the Poincaré sections are plotted in Fig. 4c.

All the curves are invariant in the graph of Poincaré sections for the values of $z\,\,\left( {0\,\,\le \,\,z\,\,\le \,\,5} \right)$. These invariant curves represent quasi-periodic motion of infinitesimal mass $m'$.

Increasing the radiation factor $q = - 0.096667$ for the same data taken in figure 4c, it is seen that quasi-periodic motion occurs in the graph of Poincaré sections. It is also seen that the region of oscillation increases as we increase radiation pressure (Fig. 4d).

(ii) For $\mu =0.20$and $q = - 0.399$, the Poincaré sections are plotted in Fig. 4e.

For the values of $z\,\,\left( {0\,\,\le \,\,z\,\,\le \,\,5} \right)$, all the curves are invariant in the graph of Poincaré sections. All these invariant curves represent quasi-periodic motion of infinitesimal mass $m'$.

By increasing the radiation factor $q = -0.11$ for the same data taken in figure 4e. Same observations are seen in the graph of Poincaré sections i.e. quasi-periodic motion occurs. It is also seen that as we increase radiation pressure, the region of oscillation increases (Fig. 4f).

Fig. 4

Poincaré sections for different values of q and $\mu$; z versus $\dot{{z}}$

6 Families of Periodic Orbits

The periodic orbits play a vital role in the deterministic dynamical system. In the study of dynamical system, an orbit is a group of points connected by the evolution function of the dynamical system and it corresponds to a special type of solution for a dynamical system, namely one which repeats itself in time.

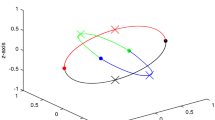
In this section, we have investigated four families of periodic orbits around the infinitesimal mass ${m}'$ for different values of radiation factor q and mass parameter $\mu$ in the frame of restricted six-body problem. Periodic orbits provide machinery which allows us to use the knowledge by the individual solutions, such as locations, stabilities and periods to make predictions about the statistics as Entropies, Lyapunov exponents etc. In this section, the dynamical system are smooth n-dimensional maps which is of the form $f\left( {x_{i} } \right) =x_{i+1}$, where $x_{i}$ is an n-dimensional vector in n-dimensional phase space. In our study, we have plotted some periodic orbits by hit and trial method using the software Mathematica-7.

We have calculated periods of periodic orbits around the infinitesimal mass $m'$ under the combined effects of mass parameter $\mu$ and radiation factor q. For the initial position $z=0.6$ and initial velocity $\dot{z}=0.001$, we did numerous tests by setting mass parameter $\mu$ and varying radiation factor q sequentially. The numerical location and periods of periodic orbits of the infinitesimal mass $m'$ for different values of mass parameter $\mu$ and radiation factor q are given in Table 4. Graphically, families of periodic orbits under the combined effects of mass parameter $\mu$ and radiation factor q are displayed from Figs. 5, 6, 7 and 8 and the following points have been observed:

• (i) For $\mu = 0.01$ and different values of q, the families of periodic orbits around the infinitesimal mass ${m}'$ are purely periodic, Fig. 5. Also, it is seen that as the radiation factor q increases, the period of periodic orbits decreases.

• (ii) For $\mu = 0.05, 0.10$ and 0.15, the families of periodic orbits around the infinitesimal mass $m'$ are plotted in Figs. 6, 7 and 8 and it is observed that the orbits are purely periodic and period of periodic orbits decreases as radiation factor q increases.

Table 4 Periods of periodic orbits of $m'$

Fig. 5

Families of periodic orbits around $m'; q=-0.0006567\,(\mathrm{black}), q=-0.0004567\,(\mathrm{red}), q=-0.0002567\,(\mathrm{green}), q=-0.0000567\,(\mathrm{magenta} )$ for $\mu =0.01$; z versus $\dot{{z}}$

Fig. 6

Families of periodic orbits around $m'; q=-0.050\,\,(\mathrm{black}), q=-0.047\,(\mathrm{red}), q=-0.044\,(\mathrm{green}), q=-0.041\,(\mathrm{magenta})$ for $\mu =0.05$; z versus $\dot{{z}}$

Fig. 7

Families of periodic orbits around $m'; q=-0.156667\,(\mathrm{black}), q=-0.136667\,(\mathrm{red}), q=-0.116667\,(\mathrm{green}), q=-0.096667\,(\mathrm{magenta})$ for $\mu =0.10$; z versus $\dot{{z}}$

Fig. 8

Families of periodic orbits around $m'; q=-0.50\,(\mathrm{black}), q=-0.47\,(\mathrm{red}), q=-0.44\,(\mathrm{green}), q=-0.41\,(\mathrm{magenta})$ for $\mu =0.15$; z versus $\dot{{z}}$

7 Conclusion

In the present manuscript, we study the existence of out-of-plane equilibrium points and their stability in the photogravitational restricted six-body problem. We observed that there exist two out-of-plane equilibria $E_{1,2}^{z}$ in the open interval $q\in \left( {-\frac{4\mu }{1-4\mu },\,\,0} \right)$, where q is the radiation factor parameter and $0< \mu <0.25$ is the mass parameter. We further observed that as q approaches to zero, the equilibrium point $E_{1}^{z}$ moves downward while $E_{2}^{z}$ moves upward along z-axis and both approaches to center of mass of the system. In order to check the linear stability of out-of-plane equilibria we have solved the characteristic Eq. (21) for different values of $\mu$ and found that two roots are real and four are pure imaginary which lead to the instability of the equilibrium points. Also, we have seen periodicity of the motion of infinitesimal mass $m'$ by using Poincaré section for different values of mass parameter $\mu$ and radiation factor q. For the fixed value of mass parameter $\mu$ and increased value of radiation factor q, it is observed that the motion of infinitesimal mass $m'$ reveal to irregular. It is also observed that region of oscillation increases as we increase radiation factor q (Fig. 4). For $\mu = 0.01, 0.05, 0.10$ and 0.15, the families of periodic orbits around the infinitesimal mass $m'$ are plotted in Figs. 5, 6, 7 and 8 and it is observed that the orbits are purely periodic and period of periodic orbits decreases as radiation factor q increase.