1 Introduction

The flow through a stretching surface grabs the attention of researchers due to its huge number of applications in the extrusion of plastic sheets, aerodynamics, production of paper, condensation process of boundary layer liquid film and glass blowing. Thomas and Yang [1] presented numerous applications for stretching wedges, sheets, nano-bio-polymers and in differential geometrical aspects. Stasiak et al. [2] addressed in detail the effects of stretching on the physical features for bio-polymer cylindrical coatings. Bachok and Ishak [3] investigated the heat flux with heat transfer through a stretching cylinder. Stretching or contracting has a significant importance which is critical for the accomplishment of polymer products from a macroscopic to a nanoscale level. The boundary layer flow on a moving stretching surface was first considered by Sakiadis [4]. Further, this concept of stretching surface was extended by Crane [5]. In his paper, he studied stretching sheet with linearly varying surface speed for steady two-dimensional flow. Moreover, the work of Crane [5] was extended by Datta et al. [6] and Chen and Char [7]. They have studied the heat and mass transfer analysis of different physical situations. Bhatti et al. [8] studied numerically the fluid flow through a porous shrinking sheet using successive linearisation method and Chebyshev spectral collocation method. Bhatti et al. [9] again analysed the effects magnetohydrodynamics over a permeable shrinking/stretching sheet through a porous medium with heat transfer.

During the past two decades, an important development in thermal engineering and material science has been that of nanofluids. Nanofluids contain very small nanoparticles (d ≤ 50 mm) in base fluids, i.e. water, ethylene glycol, oil, whereas nanoparticle is made up of oxides and metals such as nitride ceramics (A1N, SiN), semiconductors (SiC) and metals (Ag, Cu, Au). The suspension of nanoparticles in a base fluid can help to enhance markedly the thermal conductivity of the fluid, heat transfer proficiency and fluid flow characteristics, etc. This has implications in a treatment of cancer during thermal therapy, aerospace, power generation, micromanufacturing and various medical applications [10–12].

Bio-convection is associated with a macroscopic motion of convecting fluid influenced by density gradients due to hydrodynamic propulsion such as swimming of motile microorganism. The suspension of microorganism, i.e. bacteria and algae into a base fluid, i.e. water produces the process of bio-convection. This process is directly oriented swimming and typically through a naturally or imposed present stimulus such as magnetic field, gravity, chemical concentration (oxygen) and light. The density of a microorganism in inclined is higher as compared to a free stream fluid. Hence, an unstable density profile has been observed with a consecutive upending of a fluid against gravity [13]. To survive and remain active for most of the microorganism, the base fluid is considered as water and it is supposed to be that the addition of nanoparticles will remain secure and do not aggregate for few weeks [14]. Due to dilute suspension, bio-convection takes place since nanoparticle will enhance the suspension’s viscosity and viscosity will govern the bio-convection instability [15]. Xu and Pop [16] studied the mixed convection nanofluid flow having gyrotactic microorganism through a stretching surface. Aziz et al. [17] examined the natural bio-convection nanofluid boundary layer flow and found that the bio-convection parameters create an effect on the motile microorganism, heat and mass transfer. Bio-convection plays a significant role in bio-microsystems for the propagation of mass augmentation and different microfluidics devices, i.e. bacteria powered micromixers [18]. Furthermore, nanofluids with bio-convection can be found in the synthesis of drug delivery systems (pharmacological agents) [19, 20]. Microorganism is employed to enhance different desirable medical features, i.e. biocompatibility, DNA (gene therapy), bioavailability, protein deliverability and encapsulation, whereas it is also helpful to improve a biodegradable nanomaterials. Nowadays, the manufacturer of bio-nano-polymers introduced various kinds of drugs that can achieve control release which is very helpful to enhance the influence of therapeutic in patients. For example, polylactic acid, polycaprolactone, chitosan–gelatin, poly alkyl cyanoacrylate, poly (lactic-co-glycolic acid) and polyhydroxylalkanoates.

Heat transfer with thermal radiation plays a significant influence in the establishment of high temperature. The different technological process performs at high temperature, and an excellent knowledge of radiative heat transfer plays a worthy role to manipulate the apparatus. In different applications depending on geometry as well as surface properties, the transfer of radiation is similar to a convective heat transfer. In the study of radiative heat transfer fluid flow, a few complexities arise. Firstly, when the radiative heat transfer arises, the radiation diffused in the periphery of a system as well as at system restrictions. Hence, the prophecy of a fluid amalgamation is a complicated chore. Secondly, the thermal radiation term that arises in the energy equation makes the equation nonlinear. Thirdly, the coefficient of amalgamation of alluring fluids markedly depends on wavelength. Due to such kinds of complexities, the influence of energy on convecting fluid flow has been analysed with rationally simplified models. For instance, the radiation effect on heat transfer in electrically conducting fluid at a stretching surface with uniform free stream was examined by Eldahab and Aziz [21]. Cortell [22] studied the effects of viscous dissipation and work done by deformation on MHD flow and heat transfer of a viscoelastic fluid over a stretching sheet and also he [23] also observed the radiation effect on thermal boundary layer. Few more pertinent studies can be found from Refs. [24–26].

On the other hand, heat and mass transfer under the effects of chemical reaction has significant importance in many practical applications in science and engineering such as chemical engineering process, transfer of energy in a wet cooling tower and evaporation at the surface of water body. Anjalidevi and Kandasamy [27] studied the simultaneous effects of chemical reaction with and mass transfer through the semi-infinite horizontal plate. Hayat et al. [28] addressed the heat and mass transfer with combine effects of radiation and chemical reaction on three-dimensional boundary layer flow of a second-grade nanofluids through a stretching surface. Abel et al. [29] examined the effects of variable thermal conductivity and buoyancy on magnetohydrodynamics boundary layer flow of a power-law fluid in the presence of heat source through a stretching sheet. Various authors [30–32] have examined different problems by considering nanofluid, thermal radiation, chemical reaction, magnetic field and viscous dissipation in various geometrical aspects.

The study of applied magnetic field also plays a prominent role in heat and mass transfer, controlling momentum in boundary layer nanofluid flow through a shrinking/stretching surface. Furthermore, magnetohydrodynamics (MHD) is applicable in various engineering applications such as cooling of the nuclear reactor, electromagnetic casting and plasma confinement. Moreover, in microfluidics problems, magnetohydrodynamics is helpful to create non-pulsating and continuous flow in the design of a complex microchannel. In biomedical engineering, magnetohydrodynamics is applicable for magnetic drug targeting in cancer diseases. Ishak et al. [33] investigated MHD effects on flow and heat transfer over a stretching cylinder. The slip condition plays an important role in shear skin and effect of hysteresis. It also arises in hydrophobic surfaces, particularly in, microfluidics. The effect of partial slip with MHD and chemically solute transfer over a stretching cylinder is studied by Mukhopadyay [34]. The unsteady laminar flow of a viscous incompressible and electrically conducting fluid over a continuously stretching surface in the presence of magnetic field was investigated by Ishak [35]. Pop and Na [36] considered a note on MHD flow over a stretching permeable surface. Khan et al. [37] studied the viscoelastic MHD flow heat and mass transfer over a porous stretching sheet with the dissipation of energy and stress work.

The study of flow through a porous medium is also very important in engineering and industrial process. A porous media is a material having pores, i.e. oxides and metals, whereas these pores are covered with a fluid. Such type of flow has two main benefits, as its area of dissipation is higher as compared to conventional fins that improve the heat convection. Moreover, the regular motion of a fluid along the whole individual beads blends the fluid effectively [38]. According to some recent studies, it is found that nanofluid flow through a porous media reveals improved thermal properties, i.e. convective heat transfer coefficients as compared with a base material and higher thermal conductivity [39]. Therefore, a porous media having nanoparticles plays a vital role in the enhancement of heat transfer and this mechanism is very important from an application and theoretical point of view. Such kinds of flow can be observed infiltration, oil flow, heat exchangers, drying process and geothermal systems [40]. Particularly, nanofluid flow through a porous media has many in thermal engineering such as favourable to improve the thermal properties of lubricants and oil [41] electronic cooling equipment and vehicle cooling.

Motivated from the above discussion, the aim of present study is to present a mathematical model of MHD viscous nanofluid having gyrotactic microorganism under the effects of thermal radiation and chemical reaction through a porous stretching cylinder. Simultaneous effects thermal slip and velocity slip are also taken into account in the presence of heat source/sink. For an excellent fabrication of bio-nano-materials, physio-mathematical and numerical simulation has played a significant role. This is a strong motivation for the present study. Numerical computation has been employed using shooting method to obtain the solution for the governing nonlinear highly coupled differential equations. The effects of all the governing parameters are discussed for velocity, temperature, nanoparticle concentration and motile microorganism density profile. Moreover, the expression for skin friction coefficient, Nusselt number, Sherwood number and motile microorganism density number is presented with the help of tables. The numerical comparison is also presented with the existing published results. This paper is organised in the following way; after the introduction and background in Sect. 1. Section 2 describes the mathematical formulation, while Sect. 4 deals with the solution methodology. Finally, Sect. 5 is devoted numerical and graphical results of all the physical parameters.

2 Mathematical formulation

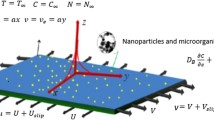
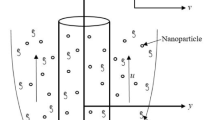
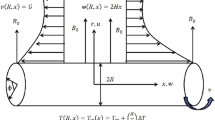
Let us assume the unsteady forced nanofluid bio-convection flow that comprises of gyrotactic microorganism and nanoparticles through an infinite stretching cylinder. The diameter of a cylinder is considered a time function with unsteady radius, i.e.

$$\tilde{a} = a_{0} \sqrt {1 - \bar{\beta }\bar{t}} ,$$
(1)

where \(\bar{\beta }\) is the contraction expansion strength, \(\bar{t}\) is the time, and a 0 (>0) is a constant. The nanoparticles fraction on the atmosphere is supposed to follow the passively control modelled presented by Bashir et al. [44], whereas the temperature distribution and nanoparticles concentration on an atmosphere are considered as \(\bar{C}_{\infty }\) and \(\bar{T}_{\infty }\), respectively. It is important to note that microorganisms can only live in water. For this purpose, we have considered the base fluid as a water (Fig. 1).

Fig. 1
figure 1

Geometry of the problem

Full size image

Using these assumptions and following Bashir et al. [44], the governing equations of continuity conservation of mass, thermal energy, nanoparticle concentration and microorganism concentration can be written as [44]

$$\nabla \cdot {\bar{\text{v}}} = 0,$$
(2)
$$\frac{{\partial {\bar{\text{v}}}}}{{\partial \bar{t}}} + \left( {{\bar{\text{v}}} \cdot \nabla } \right){\bar{\text{v}}} = - \frac{1}{\rho }\nabla \bar{P} + \nu \nabla^{2} {\bar{\text{v}}} - \sigma B_{0}^{2} {\bar{\text{v}}} - \frac{\mu }{{\rho \bar{k}_{p} }}{\bar{\text{v}}} - \frac{{c_{F} }}{{\bar{k}_{p}^{1/2} }}{\bar{\text{v}}}^{2} ,$$
(3)
$$\frac{{\partial \bar{T}}}{{\partial \bar{t}}} + {\bar{\text{v}}} \cdot \nabla \bar{T} = \bar{\alpha }_{m} \nabla^{2} \bar{T} + \tau \left[ {D_{\text{B}} \nabla \bar{T} \cdot \nabla \bar{C} + \frac{{D_{{\bar{T}}} }}{{\bar{T}_{\infty } }}\nabla \bar{T} \cdot \nabla \bar{T}} \right] - \frac{1}{{\left( {\rho c} \right)_{\text{p}} }}\frac{{\partial \bar{Q}_{R} }}{{\partial \bar{r}}} + \frac{{Q_{0} \left( {\bar{T} - \bar{T}_{\infty } } \right)}}{{\left( {\rho c} \right)_{\text{p}} }},$$
(4)
$$\frac{{\partial \bar{C}}}{{\partial \bar{t}}} + {\bar{\text{v}}} \cdot \nabla \bar{C} = D_{\text{B}} \nabla^{2} \bar{C} + \frac{{\tau D_{{\bar{T}}} }}{{\bar{T}_{\infty } }}\nabla^{2} \bar{T} - k_{1} \left( {\bar{C}_{w} - \bar{C}_{\infty } } \right),$$
(5)
$$\frac{{\partial \bar{n}}}{{\partial \bar{t}}} + \nabla \cdot \bar{J} = 0.$$
(6)

The nonlinear radiative heat flux can be written as

$$\bar{Q}_{r} = - \frac{{4\bar{\sigma }}}{{3k^{\prime } }} \frac{{\partial \bar{T}^{4} }}{{\partial \bar{r}}} = - \frac{{16\bar{\sigma }\bar{T}^{3} }}{{3k^{\prime } }}\frac{{\partial \bar{T}}}{{\partial \bar{r}}}.$$
(7)

where \({\bar{\text{v}}} = \left[ {\bar{u},\bar{v},\bar{w}} \right]\) is the velocity components, \(\bar{P}\) is the pressure, \(\bar{C}\) is the nanoparticle volume fraction, \(\bar{n}\) is the density of motile microorganisms, ν is a kinematic viscosity of a suspension of microorganism and nanofluid, ρ is the density, \(\bar{\alpha }_{m}\) is the thermal conductivity, \(\tau = \left( {\rho c} \right)_{\text{p}} /\left( {\rho c} \right)_{\text{f}}\) is parameter in which (ρc)f and (ρc)p is the heat capacity of the fluid and nanoparticle, respectively, σ is the electrical conductivity, D B is a Brownian diffusion coefficient, \(D_{{\bar{T}}}\) is a thermophoretic diffusion coefficient, J is a heat flux of microorganisms due to a convection of fluid, diffusion and self-propelled swimming are described as \(J = \bar{n}{\bar{\text{v}}} + \bar{n}{\hat{\bar{\text{v}}}} - D_{{\bar{n}}} \nabla n\), where \({\hat{\bar{\text{v}}}} = \left\{ {\frac{{\bar{b}W_{c} }}{{\bar{C}_{\infty } }} } \right\}\nabla \bar{C}\) is a velocity vector associated with swimming of cell in nanofluids, \(D_{{\bar{n}}}\) is a microorganism diffusivity, \(\bar{b}\) is a chemotaxis constant, and W c is maximum cell swimming speed. We have considered cylindrical coordinates \(\left( {\bar{r},\bar{z}} \right)\) in radial and axial directions. Taking the approximation of boundary layer flow under the assumptions of axisymmetric flow, neglecting the velocity components of azimuthal and order of a magnitude analysis, the governing equations reduce to the following form [44]

$$\frac{{\partial \bar{u}}}{{\partial \bar{r}}} + \frac{{\partial \bar{w}}}{{\partial \bar{z}}} + \frac{{\bar{u}}}{{\bar{r}}} = 0,$$
(8)
$$\frac{{\partial \bar{u}}}{{\partial \bar{t}}} + \bar{u}\frac{{\partial \bar{u}}}{{\partial \bar{r}}} + \bar{w}\frac{{\partial \bar{u}}}{{\partial \bar{z}}} = - \frac{1}{\rho }\frac{{\partial \bar{P}}}{{\partial \bar{r}}} + \nu \left[ {\frac{{\partial^{2} \bar{u}}}{{\partial \bar{r}^{2} }} + \frac{{\partial^{2} \bar{u}}}{{\partial \bar{z}^{2} }} + \frac{1}{{\bar{r}}}\frac{{\partial \bar{u}}}{{\partial \bar{r}}} - \frac{{\bar{u}}}{{\bar{r}^{2} }}} \right],$$
(9)
$$\frac{{\partial \bar{w}}}{{\partial \bar{t}}} + \bar{u}\frac{{\partial \bar{w}}}{{\partial \bar{r}}} + \bar{w}\frac{{\partial \bar{w}}}{{\partial \bar{z}}} = - \frac{1}{\rho }\frac{{\partial \bar{P}}}{{\partial \bar{z}}} + \nu \left[ {\frac{{\partial^{2} \bar{w}}}{{\partial \bar{r}^{2} }} + \frac{{\partial^{2} \bar{w}}}{{\partial \bar{z}^{2} }} + \frac{1}{{\bar{r}}}\frac{{\partial \bar{w}}}{{\partial \bar{r}}}} \right] - \sigma B_{0}^{2} \bar{w} - \frac{\mu }{{\rho \bar{k}_{p} }}\bar{w} - \frac{{c_{F} }}{{\bar{k}_{p}^{1/2} }}\bar{w}^{2} ,$$
(10)
$$\begin{aligned} \frac{{\partial \bar{T}}}{{\partial \bar{t}}} + \bar{u}\frac{{\partial \bar{T}}}{{\partial \bar{r}}} + \bar{w}\frac{{\partial \bar{T}}}{{\partial \bar{z}}} &= \bar{\alpha }_{m} \left[ {\frac{{\partial^{2} \bar{T}}}{{\partial \bar{r}^{2} }} + \frac{{\partial^{2} \bar{T}}}{{\partial \bar{z}^{2} }} + \frac{1}{{\bar{r}}}\frac{{\partial \bar{T}}}{{\partial \bar{r}}}} \right] + \tau D_{B} \left[ {\frac{{\partial \bar{T}}}{{\partial \bar{r}}}\frac{{\partial \bar{C}}}{{\partial \bar{r}}} + \frac{{\partial \bar{T}}}{{\partial \bar{z}}}\frac{{\partial \bar{C}}}{{\partial \bar{z}}}} \right] \\ &\quad + \,\frac{{\tau D_{{\bar{T}}} }}{{\bar{T}_{\infty } }}\left[ {\left( {\frac{{\partial \bar{T}}}{{\partial \bar{r}}}} \right)^{2} + \left( {\frac{{\partial \bar{T}}}{{\partial \bar{z}}}} \right)^{2} } \right] + \frac{{16\bar{\sigma }\bar{T}^{3} }}{{3k^{\prime } }}\frac{{\partial^{2} \bar{T}}}{{\partial \bar{r}^{2} }} + \frac{{Q_{0} \left( {\bar{T} - \bar{T}_{\infty } } \right)}}{{\left( {\rho c} \right)_{p} }}, \\ \end{aligned}$$
(11)
$$\frac{{\partial \bar{C}}}{{\partial \bar{t}}} + \bar{u}\frac{{\partial \bar{C}}}{{\partial \bar{r}}} + \bar{w}\frac{{\partial \bar{C}}}{{\partial \bar{z}}} = D_{\text{B}} \left[ {\frac{{\partial^{2} \bar{C}}}{{\partial \bar{r}^{2} }} + \frac{{\partial^{2} \bar{C}}}{{\partial \bar{z}^{2} }} + \frac{1}{{\bar{r}}}\frac{{\partial \bar{C}}}{{\partial \bar{r}}}} \right] + \frac{{D_{{\bar{T}}} }}{{\bar{T}_{\infty } }}\left[ {\frac{{\partial^{2} \bar{T}}}{{\partial \bar{r}^{2} }} + \frac{{\partial^{2} \bar{T}}}{{\partial \bar{z}^{2} }} + \frac{1}{{\bar{r}}}\frac{{\partial \bar{w}}}{{\partial \bar{r}}}} \right] - k_{1} \left( {\bar{C}_{w} - \bar{C}_{\infty } } \right),$$
(12)
$$\frac{{\partial \bar{n}}}{{\partial \bar{t}}} + \bar{u}\frac{{\partial \bar{n}}}{{\partial \bar{r}}} + \bar{w}\frac{{\partial \bar{n}}}{{\partial \bar{z}}} = \frac{{\bar{b}W_{c} }}{{\bar{C}_{\infty } }}\left[ {\frac{\partial }{{\partial \bar{r}}}\left\{ {\bar{n}\frac{{\partial \bar{C}}}{{\partial \bar{r}}}} \right\} + \frac{\partial }{{\partial \bar{z}}}\left\{ {\bar{n}\frac{{\partial \bar{C}}}{{\partial \bar{z}}}} \right\}} \right] + D_{{\bar{n}}} \left[ {\frac{{\partial^{2} \bar{n}}}{{\partial \bar{r}^{2} }} + \frac{{\partial^{2} \bar{n}}}{{\partial \bar{z}^{2} }} + \frac{1}{{\bar{r}}}\frac{{\partial \bar{n}}}{{\partial \bar{r}}}} \right].$$
(13)

Their respective boundary conditions become:

$$\left( {\bar{u}\left( {a\left( {\bar{t}} \right),\bar{z},\bar{t}} \right),\bar{w}\left( {a\left( {\bar{t}} \right),\bar{z},\bar{t}} \right)} \right) = \left( {0,\frac{{4\nu \bar{z}}}{{1 - \beta \bar{t}}} + N_{1} \nu \frac{{\partial \bar{w}}}{{\partial \bar{r}}}} \right)\quad {\text{at}}\quad \bar{r} = a\left( {\bar{t}} \right),$$
(14)
$$\bar{T} = \bar{T}_{\infty } + \bar{D}_{1} \frac{{\partial \bar{T}}}{{\partial \bar{r}}},\quad D_{B} \frac{{\partial \bar{C}}}{{\partial \bar{r}}} + \frac{{D_{{\bar{T}}} }}{{\bar{T}_{\infty } }}\frac{{\partial \bar{T}}}{{\partial \bar{r}}} = 0,\quad \bar{n} = \bar{n}_{w} \quad {\text{at}}\quad \bar{r} = a\left( {\bar{t}} \right).$$
(15)
$$\tilde{w} = 0,\quad \bar{n} = 0,\quad \bar{T} = \bar{T}_{\infty } ,\quad \bar{C} = \bar{C}_{\infty } \quad {\text{at}}\quad \bar{r} \to \infty .$$
(16)

In the above equations, \(\bar{T}_{w}\) is the surface temperature, N 1 is the velocity slip parameter, \(\bar{n}_{w}\) is the surface density of motile-organism, \(\bar{k}_{p}\) Permeability of porous medium, c F Forchheimer coefficient, Q 0 represents the heat source when Q 0 > 0 and the heat sink Q 0 < 0 and \(\bar{D}_{1}\) is the thermal slip factor. Defining the following similarity transformation and dimensionless variables [44]

$$\xi = \frac{{\bar{r}^{2} }}{{a_{0}^{2} \left( {1 - \bar{\beta }\bar{t} } \right)}},\quad \bar{u} = - \frac{1}{{a_{0} \sqrt \xi }}\frac{2\nu F\left( \xi \right)}{{\sqrt {1 - \bar{\beta }\bar{t}} }},\quad \bar{w} = \frac{{4\nu \bar{z}}}{{a_{0}^{2} \left( {1 - \bar{\beta }\bar{t}} \right)}}F^{\prime } \left( \xi \right),\quad \theta \left( \xi \right) = \frac{{\bar{T} - \bar{T}_{\infty } }}{{\bar{T}_{w} - \bar{T}_{\infty } }},\quad \phi = \frac{{\bar{C} - \bar{C}_{\infty } }}{{\bar{C}_{\infty } }},\quad \varPhi = \frac{{\bar{n}}}{{\bar{n}_{w} }}.$$
(17)

and using Eq. (8) into Eqs. (1)–(7), we get

$$\xi F^{\prime \prime \prime } + \left( {1 + F} \right)F^{\prime \prime } - F^{\prime 2} - S\left( {\xi F^{\prime \prime } + F^{\prime } } \right) - MF^{\prime } - k_{\text{f}} F^{\prime 2} - kF^{\prime } = 0,$$
(18)
$$\xi \theta^{\prime \prime } + \theta^{\prime } + \frac{4\xi }{3}R_{d} \theta^{\prime \prime } + \frac{2}{3}R_{d} \theta^{\prime } + PrF\theta^{\prime } - PrS\xi \theta^{\prime } + \xi \left\{ {N_{\text{b}} \phi^{\prime } \theta^{\prime } + N_{\text{t}} \theta^{\prime 2} } \right\} + H_{\text{S}} = 0,$$
(19)
$$N_{\text{b}} \xi \phi^{\prime \prime } + N_{\text{b}} \phi^{\prime } + ScN_{\text{b}} \left\{ {F\phi^{\prime } - S\xi \phi^{\prime } } \right\} + N_{\text{t}} \left\{ {\xi \theta^{\prime \prime } + \theta^{\prime } } \right\} - \gamma \phi = 0,$$
(20)
$$\xi \varPhi^{\prime \prime } + \varPhi^{\prime } + Sb\left\{ {F\varPhi^{\prime } - S\xi \varPhi^{\prime } } \right\} - P_{e} \left\{ {\xi \varPhi \phi^{\prime \prime } + \frac{1}{2}\varPhi \phi^{\prime } + \xi \phi^{\prime } \varPhi^{\prime } } \right\} = 0.$$
(21)

Their corresponding boundary conditions are

$$F\left( 1 \right) = F_{w} ,F^{\prime } \left( 1 \right) = 1 + \beta F^{\prime \prime } \left( 1 \right),\quad F^{\prime } \left( \infty \right) = 0,$$
(22)
$$\theta \left( 1 \right) = 1 + \beta_{\text{T}} \theta^{\prime } \left( 1 \right),\quad \theta \left( \infty \right) = 0,$$
(23)
$$N_{\text{b}} \phi^{\prime } \left( 1 \right) + N_{\text{t}} \theta^{\prime } \left( 1 \right) = 0,\quad \phi \left( \infty \right) = 0,$$
(24)
$$\varPhi \left( 1 \right) = 1,\quad \varPhi \left( \infty \right) = 0.$$
(25)

where

$$S = \frac{{\bar{\beta }a_{0}^{2} }}{4\nu },\quad N_{\text{b}} = \frac{{\tau D_{B} \bar{C}_{\infty } }}{{\bar{\alpha }_{m} }},\quad N_{\text{t}} = \frac{{\tau D_{{\bar{T}}} \left[ {\bar{T}_{w} - \bar{T}_{\infty } } \right]}}{{\bar{\alpha }_{m} }},\quad Sc = \frac{\nu }{{D_{B} }},\quad Sb = \frac{\nu }{{D_{{\bar{n}}} }},\quad Pe = \frac{{\bar{b}W_{c} }}{{D_{{\bar{n}}} }},\quad \beta = \frac{{2\left[ {N_{1} } \right]_{0} \nu }}{{a_{0} }},\quad \beta_{\text{T}} = \frac{{2\left[ {\bar{D}_{1} } \right]_{0} \nu }}{{a_{0} }},\quad R_{d} = \frac{{4\bar{\sigma }\bar{T}^{3} }}{{k^{\prime } \kappa }},\quad Pr = \frac{\nu }{{\bar{\alpha }_{m} }},\quad M = \frac{{\sigma B_{0}^{2} }}{4\nu \rho }\left[ {a_{0}^{2} \left( {1 - \bar{\beta }\bar{t}} \right)} \right],\quad k = \frac{\mu }{{\rho \nu \bar{k}_{p} }}\left[ {a_{0}^{2} \left( {1 - \bar{\beta }\bar{t}} \right)} \right],\quad k_{f} = \frac{\mu z}{{\rho \bar{k}_{p}^{1/2} }}.$$
(26)

In the above equations, S is the unsteady parameter, Pr is the Prandtl number, N b Brownian motion coefficient, N t thermophoresis coefficient, Sc is the Schmidt number, Sb bio-convection Schmidt number, Pe is the Peclet number, β is the velocity slip, k f is Forchheimer number, β T is the thermal slip, M is the magnetic parameter, R d is a thermal radiation parameter, γ is the chemical reaction parameter, H S is dimensionless heat source/sink parameter.

3 Physical quantities of interest

The physical quantities of interest such as skin friction coefficient, local Nusselt number, Sherwood number, local density number of motile microorganisms are defined as [44]

$$C_{{F\bar{x}}} = \frac{{\tau_{w} }}{{\rho \bar{w}_{w}^{2} /2}},\quad Nu_{{\bar{x}}} = \frac{{\bar{a}\left( {\bar{t}} \right)q_{w} }}{{2k\left( {\bar{T} - \bar{T}_{\infty } } \right)}},\quad Sh_{{\bar{x}}} = \frac{{\bar{a}\left( {\bar{t}} \right)q_{\text{M}} }}{{2D_{B} \left( {\bar{C} - \bar{C}_{\infty } } \right)}},\quad N_{{n\bar{x}}} = \frac{{\bar{a}\left( {\bar{t}} \right)q_{N} }}{{2k\bar{n}_{w} }}.$$
(27)

where τ w , q w , q M and q N are the shear stress, surface heat flux, surface mass flux and the motile surface microorganism flux which are defined as

$$\tau_{w} = \mu \left. {\frac{{\partial \bar{w}}}{{\partial \bar{r}}}} \right|_{{\bar{r} = a\left( {\bar{t}} \right)}} ,\quad q_{w} = - \kappa \left. {\frac{{\partial \bar{T}}}{{\partial \bar{r}}}} \right|_{{\bar{r} = a\left( {\bar{t}} \right)}} ,\quad q_{\text{M}} = - D_{B} \left. {\frac{{\partial \bar{C}}}{{\partial \bar{r}}}} \right|_{{\bar{r} = a\left( {\bar{t}} \right)}} ,\quad q_{N} = \left. {\frac{{\partial \bar{n}}}{{\partial \bar{r}}}} \right|_{{\bar{r} = a\left( {\bar{t}} \right)}} .$$
(28)

Using Eq. (17) and Eq. (27), we have

$$C_{{F\bar{x}}} = F^{\prime \prime } \left( 1 \right),\quad Nu_{{\bar{x}}} = - \left( {1 + \frac{4}{3}R_{d} } \right)\theta^{\prime } \left( 1 \right),\quad Sh_{{\bar{x}}} = - \phi^{\prime } \left( 1 \right),\quad N_{{n\bar{x}}} = - \varPhi^{\prime } \left( 1 \right).$$
(29)

4 Method of solution: shooting method

The exact solution for Eqs. (18) to (21) is not possible, for this purpose, we have employed shooting method to obtain the solution. Initially, all the equations are reduced to first-order differential equations and then shooting method is applied with a suitable initial guess. Equations (18) to (21) are reduced to the following form

$$F^{\prime } = u_{1} ,u_{1}^{\prime } = u_{2} ,u_{2}^{\prime } = - \frac{1}{\xi }\left[ {\left( {1 + F} \right)u_{2} - u_{1}^{2} - S\left( {\xi u_{2} + u_{1} } \right) - Mu_{1} - k_{\text{f}} u_{1}^{2} - ku_{1} } \right],$$
(30)
$$\theta^{\prime } = v_{1} ,v_{1}^{\prime } = \frac{ - 1}{{ \xi \left( {1 + \frac{4}{3}R_{d} } \right)}}\left[ {v_{1} + \frac{2}{3}R_{d} v_{1} + PrFv_{1} - PrS\xi v_{1} + \xi \left( {N_{\text{b}} v_{1} w_{1} + N_{\text{t}} v_{1}^{2} } \right) + H_{\text{S}} } \right],$$
(31)
$$\phi^{\prime } = w_{1} ,w_{1}^{\prime } = - \frac{1}{{\xi N_{\text{b}} }}\left[ {N_{\text{b}} w_{1} + ScN_{\text{b}} \left( {Fw_{1} - S\xi w_{1} } \right) + N_{\text{t}} \left( {\xi v_{1}^{\prime } + v_{1} } \right) - \gamma \phi } \right],$$
(32)
$$\varPhi^{\prime } = r_{1} ,r_{1}^{\prime } = - \frac{1}{\xi }\left[ {r_{1} + Sb\left( {Fr_{1} - S\xi r_{1} } \right) - Pe\left( {\xi \varPhi w_{1}^{\prime } + \frac{\varPhi }{2}w_{1} + \xi w_{1} r_{1} } \right)} \right],$$
(33)

and their corresponding boundary conditions reduces to the following form

$$F\left( 1 \right) = F_{w} ,u_{1} \left( 1 \right) = 1 + \beta u_{2} \left( 1 \right),\quad u_{2} \left( 1 \right) = s_{1} .$$
(34)
$$\theta \left( 1 \right) = 1 + \beta_{\text{T}} v_{1} \left( 1 \right),\quad v_{1} \left( 1 \right) = s_{2} .$$
(35)
$$N_{\text{b}} w_{1} \left( 1 \right) + N_{\text{t}} v_{1} \left( 1 \right) = 0,\quad \phi \left( 1 \right) = s_{3} .$$
(36)
$$\varPhi \left( 1 \right) = 0,\quad w_{1} \left( 1 \right) = s_{4} .$$
(37)

where s 1, s 2, s 3 and s 4 are the initial guess. “Matlab” is used for numerical computations of shooting technique with error less than 10−4, while the step is taken \(\Delta \zeta = 1 \times 10^{ - 4}\).

5 Numerical results and discussion

This section deals with graphical and numerical results of all the governing parameters arises in the governing flow problem. Particularly, we discussed the effects of Forchheimer number k f, porosity parameter k, magnetic parameter M, Brownian motion parameter N b, thermophoresis parameter N t, chemical reaction parameter γ, unsteady parameter S, Schmidt number Sc, Peclet number Pe, heat source/sink parameter H S, radiation parameter R d , velocity slip β, thermal slip β T, Prandtl number Pr and bio-convection Schmidt number Sb on velocity F′, temperature θ, nanoparticle concentration ϕ and motile microorganism density Φ profile. Table 1 shows the numerical comparison with the exiting published results [42–44] as a special case of our study by taking M = γ = R d  = k f = k = H S = 0. The numerical comparison shows that the present results are in excellent agreement which also confirms the validity of the present methodology. All the numerical computations have been carried out for the following parametric values: k = 1, N b = 0.1, N t = 0.1, γ = 1, Sb = 10, M = 2, Pe = 0.1, Sc = 10, β = 0.1, β T = 0.1, k f = 3, H S = 0.1, S = 0.1, Pr = 6.8. Numerical values of local Nusselt number, skin friction coefficient, local Sherwood number and motile microorganism of local density are presented in Table 2 against all the emerging parameters.

Table 1 Numerical comparison for skin friction coefficient and local Nusselt number by taking \(M = \gamma = R_{d} = k_{\text{f}} = k = H_{\text{S}} = 0\)
Full size table
Table 2 Numerical values of Nusselt number, Sherwood number, skin friction coefficient and motile microorganism of local density number
Full size table

Figures 2a, b and 3a, b are sketched for velocity against all the involved parameters. It depicts from Fig. 2a the greater influence of magnetic field causes a reduction in the velocity profile. Physically, when an external magnetic field is applied to any electrically conducting fluid, then a repulsive force originated known as “Lorentz force” which tends to oppose the flow markedly. It can also be seen from this figure that unsteady parameter is favourable to enhance the velocity of the fluid. It is worth mentioning here that positive values of S > 0 are associated with accelerating flow and negative values of S < 0 are associated with decelerating flow across the surface of the stretching cylinder. Moreover, for S = 0 corresponds to steady state problem, while S ≠ 0 correspond to unsteady. It can be observed from Fig. 2b that Forchheimer parameter also opposes the flow substantially, while the variation in velocity becomes smaller as the effects of magnetic field increase. Furthermore, we can also notice from Fig. 3a that porosity parameter causes a significant reduction in the velocity profile. The effects of porosity are modelled as −kF′ − k f F′2 [see Eq. (18)] which also contains a strong nonlinear term F′2. With progressively larger porosity, the matrix resistance produced due to solid fibres towards a porous medium is energised and hence the porous drag force increases, resulting that fluid decelerate very significantly. Figure 3b shows the variation of velocity against slip parameter β. It depicts from this figure that an increment in slip parameter β causes a markedly decline the velocity of the fluid. Physically, when the slip effect ensues, the drag force on the stretching wall is partially transferred to the fluid and hence the velocity of the fluid depleted. Moreover, with an increment in a hydrodynamic slip parameter tends to reduce the magnitude of a wall shear stress.

Fig. 2
figure 2

Effect of magnetic parameter M and unsteady parameter S, Forchheimer number k f and magnetic parameter M on velocity profile

Full size image
Fig. 3
figure 3

Effect of unsteady parameter S and porosity parameter k and slip parameter β on velocity profile

Full size image

Figures 4a, b, 5 and 6 are sketched for temperature profile against unsteady parameter S, radiation parameter R d , Prandtl number Pr, heat source/sink parameter H S, thermophoresis parameter N t and thermal slip parameter β T. It depicts from Fig. 4a that for higher values of unsteady parameter S, the temperature profile rises very significantly. From Fig. 4b we can see that higher values of radiation parameter R d enhance the temperature profile and its boundary layer thickness. Physically, for higher values of radiation parameter R d gives more heat to the governing fluid that reveals an increment in its boundary layer thickness and temperature profile. Furthermore, we can also see that higher values of Prandtl number Pr cause a significant reduction in temperature profile and its relevant boundary layer thickness. Large values of Prandtl number Pr are associated with weaker thermal diffusivity. Those fluids that contain weaker thermal diffusivity have a lower temperature. Such kind of weaker thermal diffusivity reveals a decrement in the temperature profile and its boundary layer thickness. It depicts from Fig. 5a that thermophoresis parameter also enhances the temperature profile markedly. An enhancement in thermophoresis parameter N t produced thermophoresis forces which allow moving the nanoparticles from hotter area to colder region and as a result, it tends to rise the thermal boundary layer thickness and temperature profile. It can be observed from Fig. 5b that heat source/sink parameter H S enhances the temperature profile and its corresponding boundary layer thickness. It can be scrutinised from Fig. 6 that an increment in thermal slip parameter β T causes a compelling reduction in the temperature profile. Physically, when the thermal slip parameter increases, then the movement of the fluid within a boundary layer will not be very sensitive due to the heating influence of cylindrical surface. A reduction in a temperature (thermal energy) profile will be transferred from a hot cylinder to the fluid, and as a result a reduction in the temperature profile has been observed, i.e. a decrement in a thermal boundary layer thickness (thinning and cooling of thermal boundary layer).

Fig. 4
figure 4

Effect of unsteady parameter S, radiation parameter R d and Prandtl number Pr on temperature profile

Full size image
Fig. 5
figure 5

Effect of thermophoresis parameter N t and heat source/sink parameter H S on temperature profile

Full size image
Fig. 6
figure 6

Effect of thermal slip β T on temperature profile

Full size image

Figures 7a, b and 8 show the effects of unsteady parameter S, Brownian motion parameter N b, thermophoresis parameter N t, chemical reaction parameter γ and Schmidt number Sc on nanoparticle concentration profile. It depicts from Fig. 7a that when the unsteady parameter S increases then the nanoparticle concentration profile and its boundary layer thickness increases. It can be examined from Fig. 7b that for large values of Brownian motion parameter N b, nanoparticle concentration profile decreases substantially. The presence of nanoparticles in the nanofluid, the Brownian motion takes place with the rise of Brownian motion parameter N b and as a result the nanoparticle concentration profile and its corresponding boundary layer thickness diminish. Furthermore, we can also see that the greater influence of thermophoresis parameter N t also enhances the boundary layer thickness and nanoparticle concentration profile. From Fig. 8 we can see that concentration profile increases significantly for large values of chemical reaction parameter γ. Moreover, we can also notice that Schmidt number Sc also enhances strongly the nanoparticle concentration profile and its boundary layer thickness. Figure 9a, b is sketched for motile microorganism density against bio-convection Schmidt number Sb, unsteady parameter S and Peclet number Pe. It depicts from Fig. 9a that bio-convection Schmidt number \(S_{b} \left( { = \frac{\nu }{{D_{{\bar{n}}} }}} \right)\) tends to diminish the microorganism density profile. This parameter is solely arises in the microorganism density profile [see Eq. (21)] and is defined as the ratio of a momentum diffusivity and microorganism diffusivity. An increment in bio-convection Schmidt number causes a diffusivity difference to rise and a rate of momentum diffusion controls the microorganism diffusion rate and as a result a reduction in the microorganism density profile has been observed (see Fig. 9a). It can also be seen in Fig. 9a that for S > 0 (accelerating flow) the microorganism density profile is higher as compared to the decelerating flow S < 0. It can be notice from Fig. 9b that microorganism density profile is strongly increased for large values of Peclet number Pe. \(P_{e} \left( { = \frac{{\bar{b}W_{\text{c}} }}{{D_{{\bar{n}}} }}} \right)\) is directly proportional to W c (maximum speed of cell swimming) and \(\bar{b}\) (chemotaxis constant), whereas it is inversely proportional to diffusivity of microorganism \(D_{{\bar{n}}}\). Hence large values of Peclet number will reduce the diffusivity of microorganism and/or microorganism speed will be diminished. As a result, the microorganism density profile and its associated boundary layer thickness increase as shown in Fig. 9b. The present study reveals various interesting behaviour that warrant further study on nanofluid having gyrotactic microorganism. Figures 10, 11, 12 and 13 are plotted for skin friction coefficient, motile microorganism density number, Nusselt number and Sherwood number versus magnetic parameter for multiple values of suction/injection parameter F w .

Fig. 7
figure 7

Effect of unsteady parameter S, thermophoresis parameter N t and Brownian motion parameter N b on concentration profile

Full size image
Fig. 8
figure 8

Effect of chemical reaction parameter γ and Schmidt number Sc on concentration profile

Full size image
Fig. 9
figure 9

Effect of Peclet number Pe, bio-convection Schmidt number Sb and unsteady parameter S on motile microorganism density profiles

Full size image
Fig. 10
figure 10

Skin friction coefficient versus magnetic parameter M

Full size image
Fig. 11
figure 11

Nusselt number versus magnetic parameter M

Full size image
Fig. 12
figure 12

Sherwood number versus magnetic parameter M

Full size image
Fig. 13
figure 13

Density number (motile microorganism) versus magnetic parameter M

Full size image

6 Conclusion

In this article, three-dimensional MHD nanofluid boundary layer flow having gyrotactic microorganism through a porous stretching cylinder has been examined. The effects of thermal radiation, chemical reaction, velocity slip and thermal slip are also taken into account. Similarity transformation variables are used to model the governing equations. The resulting highly nonlinear coupled partial differential equations are solved numerically with the help of shooting method. The influence of all the emerging parameters is discussed with the help of graphs and tables. Comparison with the existing published results is also presented as a special case of our study. The major points for the present study are summarised below:

  1. 1.

    Magnetic and porosity effects cause a reduction in the velocity profile.

  2. 2.

    The influence of slip parameter shows a significant increase in the velocity profile.

  3. 3.

    Radiation parameter tends to rise the temperature profile and its boundary layer thickness, while Prandtl number and thermal slip show opposite behaviour.

  4. 4.

    Brownian motion thermophoresis parameter shows converse behaviour on concentration profile.

  5. 5.

    Chemical reaction parameter and Schmidt number markedly enhance the concentration profile.

  6. 6.

    Heat source/sink parameter tends to rise the temperature profile significantly.

  7. 7.

    Peclet number enhances the motile microorganism density profile, while bio-convection Schmidt number depicts opposite behaviour.

The present study reveals various interesting behaviour that warrants further study on nanofluid having gyrotactic microorganism.