The effect of orientation of square perforations on the heat transfer enhancement from a fin subjected to natural convection

Abstract.

The heat transfer dissipation from a horizontal rectangular fin embedded with square perforations of two orientations under natural convection is numerically investigated. The parameters considered in this investigation are the geometrical dimensions and the orientations of the perforation. A comparison between heat dissipation of the fin with variable orientations of the perforations is presented. It is found that the heat dissipation enhancement from a fin with square perforation parallel to the fin base was more than that of fin with inclined square perforation. The problem of this study was numerically solved using the variational approach finite element technique.

A:

cross sectional area of the fin

A_c :

cross sectional area of the perforation

A_f :

heat transfer surface area of the non-perforated (solid) fin

A_fp :

heat transfer surface area of the perforated fin

b:

perforation dimension

g:

acceleration due to gravity

h:

heat transfer coefficient of the non-perforated surface

k:

thermal conductivity of fin material

Kair:

thermal conductivity of air

L:

fin length

L_c :

characteristic length

N:

Number of perforations

N_c :

total number of perforations

Nu:

average Nusselt number

Nu_c :

average Nusselt number of the inner perforation surface

OA:

open area of the perforated surface

P_f :

perimeter of the fin

Pr:

Prandtl number

Q:

heat transfer rate

Q_f :

heat dissipation rate of the solid fin

Q_fp :

heat dissipation rate of the perforated fin

Ra:

Rayleigh number

Ra_c :

Rayleigh number of the perforation inner lining surface

ROA:

Ratio of open area

RQF:

Ratio of heat dissipation rate of perforated fin to that of non-perforated one

S_x :

longitudinal perforation spacing

S_y :

lateral perforation spacing

T:

temperature

t:

fin thickness

V:

volume of the perforated fin

W:

fin width

x:

longitudinal direction or coordinate

y:

transverse (lateral) direction or coordinate

fp:

perforated fin

pc:

perforation inner surface (within the perforation)

ps:

remaining solid portion of the perforated fin

t:

fin tip

x:

in or along the x-direction

y:

in or along the y-direction

u:

upper surface of fin

l:

lower surface of fin

max:

maximum

∞:

Ambient

b:

fin base

m:

mean (average)

β:

coefficient of thermal expansion

μ:

dynamic viscosity

υ:

kinematic viscosity

1 Introduction

The thermal systems must be designed and sized to generate, transmit, or dissipate the appropriate amount of unwarranted heat with the required demand. The successful and safe operation of thermal units depends on various requirements including cooling and/or heating of certain component parts or partition walls of such systems. The cooling of these parts can be accomplished by removing heat continuously with adequate rate from them. In electric and electronic systems, the generated heat may cause burning or overheating problems that lead to system failure and costly damage. In most cases, poorly designed thermal systems are associated with overheated surfaces that are unable to transmit the right amount of undesired heat. The fin industry has been engaged with continuous search to reduce the fin size, weight and cost. The reduction in fin size and cost is achieved by the enhancement of heat transfer carried out by the fins. This enhancement can be accomplished by different method such as [1, 2]: increasing the ratio of the heat transfer surface area of the fin to its volume, manufacturing fins from materials having high thermal conductivity, and increasing the heat transfer coefficient between the fin and its surroundings.

Several studies have been conducted to find the optimum shape of fins (rectangular, triangular, pin, wavy, serrated, and slotted). Some of these studies are based on splitting a certain dimension of the fin in an optimal way provided that the total volume of the fin material is fixed. Others have introduced some shape modifications by cutting some material from the fin to make cavities, holes, slots, grooves, or perforations through the fin body in order to increase the effective heat transfer surface area and/or the heat transfer coefficient [2, 3, 4].

Fins as heat transfer enhancement devices have been quite common. As the extended surface technology continues to grow, new design ideas emerge including fins made of an isotropic composites, porous media, and interrupted plates [5, 6, 7]. Due the high demand for lightweight, compact, and economical fins, the optimization of fin size is of great importance. Therefore, fins must be designed to achieve maximum heat removal with minimum material expenditure taking into account, however, the ease of manufacturing of the fin shape.

One popular heat transfer augmentation technique involves the use of rough surfaces of different configurations. The surface roughness aims at promoting surface turbulence that is intended mainly to increase the heat transfer coefficient rather than the surface area. It was reported that non-flat surfaces have natural convection coefficients that are 50% to 100% more than those of flat surfaces [6]. Several other researchers reported a similar trend for interrupted (perforated) fins attributing the improvement to the restarting of the thermal boundary layer after each interruption indicating that the increase in convection coefficient is even more than enough to offsets lost area, if any [6, 7, 8].

Perforated plates (fins) represent an example of surface interruption [6, 9] and are widely used in different heat exchanger, film cooling, and solar collector applications [2]. Despite the fact that correlations for the convection coefficient within cavities and over the surfaces of non-perforated plates are readily available [5, 6], literature search indicated a lack of such relations for the perforated surfaces under natural convection. Consequently, surface coefficients were estimated based on the concepts of augmentation ratio [6] and open area of the perforated surface. The enhancement of heat transfer of the perforated fins was studied for some cases of perforation (holes, square perforations, triangular perforations and slots) [11]. It was found that in some cases, perforated fins enhancing heat transfer up to 200% of that of the solid one.

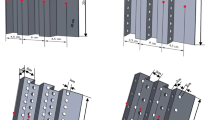
This study aims mainly at examining the extent of heat transfer enhancement from a horizontal rectangular fin under natural convection conditions as a result of introducing surface modifications (interruptions) to the fin. The modifications in this work are Vertical Square perforations made through the fin thickness with two orientations. The study investigates the influence of square perforation orientation on heat transfer dissipation. The heat dissipation of the fin with parallel perforation (Fig. 1) is compared with that of the fin with inclined perforation (Fig. 2). The study eventually attempts to make the best use of the material and size of a given fin, which involves some sort of optimization.

Fig. 1.

Fin with parallel square perforation

Fig. 2.

Fin with inclined square perforation

The overall objective of this study was to evaluate the potential of heat transfer enhancement when body perforations of square cross section are introduced to a horizontal rectangular plate (fin) under natural convection conditions. The specific objectives of the work may be summarized as follows:

• 1. Determine the values and/or ranges of parameters that would result in maximum heat dissipation of the fins with parallel and inclined square perforations.

• 2. Compare the maximum heat dissipation of the fins with parallel and inclined square perforations.

2 Analysis

Consider the fin of length (L), width (W) and thickness (t) with parallel and inclined square perforation (b × b) shown in Fig. 1 and 2, respectively. In order to simplify the following analysis, the heat flow is assumed to be steady, one-dimensional heat conduction; with homogeneous and isotropic fin material, a constant thermal properties, no heat sources/sinks in the fin body, uniform base and ambient temperatures, side area of the fin is much smaller than its surface area (i.e. the fin width is very larger compared to its thickness), uniform heat transfer coefficient over the whole fin solid surface (perforated or solid), uniform heat transfer coefficient within the perforation; and negligible radiation effects.

Also, for the middle symmetric part and for any other symmetric parts, the heat transfers cross:

• i) The remaining of peripheral surface area of perforated surface of the fin with heat transfer coefficient of (h_ps).

• ii) The perforation inner lining surface with heat transfer coefficient of (h_pc).

• iii) The fin tip surface with heat transfer coefficient of (h_t). (Note: h_ps ≠ h_pc ≠ h_t). While, the other surfaces are adiabatic (surface of symmetry), so there is no heat transfer cross them.

Based on these assumptions, the energy equation of the fin along with the boundary conditions may be written according to the formulation adopted in [10] as

$${\hbox{d} \over {\hbox{dx}}}\left( {\hbox{k}{{\hbox{dT}} \over {\hbox{dx}}}} \right) = {0}$$

(1)

The associated boundary conditions are

• 1. At the base surface (x = 0): T = T_b

• 2. At the perforated surfaces.

  • i. At the remaining peripheral fin surfaces

    $$\hbox{k}{{\hbox{dT}} \over {\hbox{dx}}} + \hbox{h}_{\hbox{ps}} (\hbox{T} - \hbox{T}_\infty) = 0$$

    (2)

  • ii. At the perforation inner lining surface

    $$\hbox{k}{{\hbox{dT}} \over {\hbox{dx}}} + \hbox{h}_{\hbox{pc}} (\hbox{T} - \hbox{T}_\infty) = 0$$

    (3)

• 3. At the fin tip surface (x = L)

  $$\hbox{k}{{\hbox{dT}} \over {\hbox{dx}}} + \hbox{h}_{\rm t} (\hbox{T}_{\rm t} - \hbox{T}_\infty) = 0$$

  (4)

The governing equations are solved numerically utilizing the one dimension, variational approach, finite-element technique, [10]. The corresponding variational statement of the elements not adjacent to the perforations has the following form:

$$ {\rm I}_{\rm n} = {1 \over 2}\mathop{\int\!\!\!\int\!\!\!\int}\limits_{\kern-5.5pt {\rm V}} {\hbox{k}\left({\hbox{dT} \over \hbox{dx}} \right)^2 \hbox{dV}} + {1 \over 2}\int\!\!\!\int\limits_{{\rm A}_{{\rm ps}}} {\hbox{h}_{{\rm ps}} (\hbox{T} - \hbox{T}_\infty)^2 \hbox{dA}_{{\rm ps}}} $$

(5)

The corresponding variational statement of the elements adjacent to the perforations has the following form:

$$ \hbox{I}_{\rm n} = {1 \over 2}\mathop{\int\!\!\!\int\!\!\!\int}\limits_{\kern-5.5pt {\rm V}} {\hbox{k}\left({\hbox{dT} \over \hbox{dx}} \right)^2 \hbox{dV}} + {1 \over 2}\int\!\!\!\int\limits_{{\rm A}_{{\rm ps}} } {\hbox{h}_{{\rm ps}} (\hbox{T} - \hbox{T}_\infty)^2 \hbox{dA}_{{\rm ps}} } + {1 \over 2}\int\!\!\!\int\limits_{{\rm A}_{{\rm pc}} } {\hbox{h}_{{\rm pc}} (\hbox{T} - \hbox{T}_\infty)^2 \hbox{dA}_{\hbox{pc}}} $$

(6)

The corresponding variational statement of the tip element has the following form:

$$ \hbox{I}_{\rm n} = {1 \over 2}\mathop{\int\!\!\!\int\!\!\!\int}\limits_{\kern-5.5pt {\rm V}} {\hbox{k}\left({\hbox{dT} \over \hbox{dx}}\right)^2 \hbox{dV}} + {1 \over 2}\int\!\!\!\int\limits_{{\rm A}_{{\rm ps}}} {\hbox{h}_{{\rm ps}} (\hbox{T} - \hbox{T}_\infty)^2 \hbox{dA}_{\hbox{ps}}} + \int\!\!\!\int\limits_{{\rm A}_{\rm t}} {\hbox{h}_{\rm t} (\hbox{T}_{\rm t} - \hbox{T}_\infty) \hbox{T}\, \hbox{dA}_{\rm t}} $$

(7)

The variational statements for solving the heat transfer problem of the perforated fin may be best clarified by reference to Figures 1 to 4. Figures 3 and 4 show the symmetry parts considered for analysis (shown hatched). It is established that everything else being the same, heat dissipation from a fin, solid or perforated, depends on fin surface area and heat transfer coefficient. For the solid fin, both aspects are established. The average value of h is that for a single horizontal plate in natural convection and may be given by

$$\hbox{h} = \hbox{Nu k}_{{\rm air}} /\hbox{L}_{\rm c} $$

(8)

Fig. 3.

The fin with inclined square perforations along with the symmetrical part (hatched part) of the perforated fin

Fig. 4.

The fin with parallel square perforations along with the symmetrical part (hatched part) of the perforated fin

The average Nusselt number, Nu, is given by [11]

$$\hbox{Nu} = (\hbox{Nu}_{\rm u} + \hbox{Nu}_{\rm 1} )/2$$

(9)

$$ \hbox{Nu}_{\rm u} = \left[(1.4/\ln (1 + 1.4/(0.43\,\hbox{Ra}^{0.25})))^{10} + (0.14\,\hbox{Ra}^{0.333} )^{10} \right]^{0.1} $$

(10)

And

$$\hbox{Nu}_{\rm I} = 0.527\, \hbox{Ra}^{0.2} /(1 + (1.9/\hbox{Pr})^{0.9} )^{2/9}$$

(11)

The surface area of the uniform longitudinal rectangular perforated fin can be expressed as:

$$ \eqalign{{\rm A}_{{\rm fp}} & = \hbox{A}_{{\rm ps}} + \hbox{A}_{\rm t} + \hbox{N}_{\rm c} \cdot \hbox{A}_{{\rm pc}} \cr & = (2\hbox{W} \cdot \hbox{L} - 2\hbox{N}_{\rm c} \cdot \hbox{A}_{\rm c} ) + (\hbox{W} \cdot \hbox{t}) + (\hbox{N}_{\rm c} \cdot \hbox{A}_{{\rm pc}} ) \cr & = \hbox{A}_{\rm f} + \hbox{N}_{\rm c} (\hbox{A}_{{\rm pc}} - 2\hbox{A}_{\rm c} ) = \hbox{A}_{\rm f} + \hbox{N}_{\rm x} \cdot \hbox{N}_{\rm y} (\hbox{A}_{{\rm pc}} - 2\hbox{A}_{\rm c})} $$

(12)

Where (A_c) is the perforation opening area and (A_pc) is the perforation inner lining surface area. (A_ps) is the remaining of peripheral fin surface area and (A_t) is the fin tip surface area. For the perforated fin there are three distinct heat transfer coefficients that are discussed below.

Heat transfer coefficient of the solid portion of the perforated surfaces, h_ps

_ps Literature search revealed that there is lack of correlations for the perforated surface under natural convection h_ps. Consequently, it was decided in this study to adapt relation depends on linear approximate estimation. Studies of heat transfer reported that h_ps was a function of the open area ratio of the perforated surface [6, 8]. The open area ratio, ROA, for a perforated surface is defined as

$$\hbox{ROA} = \hbox{OA}/\hbox{OA}_{{\rm max}}$$

(13)

Equation (13) shows that ROA ranges between zero (for the non-perforated) and one (for maximum perforation effect). Based on averaging literature estimates of increases in heat transfer coefficient due to perforations (50 to 100%, – the mean value is 75% which adopted in this study-) and assuming a linear relationship between ROA and heat transfer coefficient, the following expression is introduced to estimate h_ps in terms of ROA and h:

$$\hbox{h}_{{\rm ps}} = (1 + 0.75\, \hbox{ROA})\hbox{h}$$

(14)

The expression in the brackets is called the heat transfer augmentation ratio, Rhs, of the interrupted surface. Notice that the value of h_ps ranges between h and 1.75 h.

Heat transfer coefficient within the perforation, h_pc

_pc Correlations for heat transfer coefficient in natural convection for square perforations are available. With uniform wall temperature, the Nusselt number, Nu_c, is as follows [5]:

$$\hbox{Nu}_{\rm c} = \left[ \left( {{{\hbox{Ra}_{\rm c} } \over {14.225}}} \right)^{- 1.5} + (0.62\hbox{Ra}_{\rm c} ^{0.25})^{-1.5} \right]^{1/ - 1.5} $$

(15)

$$\hbox{Ra}_{\rm c} = \hbox{g} * \beta * (\hbox{T}_{\rm m} - \hbox{T}_\infty) * \hbox{L}_{\rm c} ^{4} /(\hbox{t} * {\nu} * \mu)$$

(16)

Where Ra_c < 10⁴

$$\hbox{L}_{\rm c} = 2 * \hbox{A}_{\rm c} /\hbox{P}_{\rm c} $$

(17)

The average film heat transfer coefficient of the perforation surface is, then, calculated from the expression

$$\hbox{h}_{{\rm pc}} = \hbox{Nu}_{\rm c} * \hbox{k}_{{\rm air}} /\hbox{L}_{\rm c} $$

(18)

Equations 13 through 16 indicate that the average film heat transfer coefficient of the perforation surface in the perforated fin is a function of the perforation geometry and the fin thickness, and the properties of the working fluid within the perforation.

Heat transfer coefficient at fin tip, h_t

_t For the fin considered in this study, both perforated and non-perforated, the fin tip is a vertical surface for which heat transfer coefficient is given by

$$\hbox{h}_{\rm t} = \hbox{Nu}_{\rm t} \, \hbox{k}_{{\rm air}} /\hbox{t}$$

(19)

Nusselt number, Nu_t, is given by [5]

$$\hbox{Nu}_{\rm t} = 0.5\left( {\left( {{2.8 \over \hbox{ln}\left( {1 + {2.8 \over 0.515 * \hbox{Ra}^{.25}}} \right)}} \right)^{6} + \left({.103} * \hbox{Ra}^{0.333} \right)^{6} } \right)^{(1/6)} $$

(20)

Ratio of heat dissipation rate (RQF)

The ratio of heat dissipation rate RQF from the perforated fin to that of the corresponding solid fin can be expressed as:

$$\hbox{ROF} = \hbox{Q}_{{\rm fp}} /\hbox{Q}_{\rm f} $$

(21)

Where Q_fp: is the heat dissipation rate of the perforated fin which was computed using the finite element technique, and Qf is the heat dissipation rate of the solid fin which was computed using the following analytical equation [1]

$$ {{\rm{Q}}_{{\rm{f}}} = {\rm{k}} \cdot {\rm{A}} \cdot {\rm{m}}({\rm{T}}_{{\rm{b}}} - {\rm{T}}_{\infty } ){{\sin \;h({\rm{m}} \cdot {\rm{L}}) + ({\rm{h}}_{{\rm{t}}} /({\rm{m}} \cdot {\rm{k}}))\cos \;h({\rm{m}} \cdot {\rm{L}})} \over {\cos \;h({\rm{m}} \cdot {\rm{L}}) + ({\rm{h}}_{{\rm{t}}} /({\rm{m}} \cdot {\rm{k}}))\sin \;h({\rm{m}} \cdot {\rm{L}})}}} $$

(22)

Where m is defined as

$$ \hbox{m} = \sqrt {\hbox{h} \cdot \hbox{P}_{\rm f} \over \hbox{k} \cdot \hbox{A}} $$

(23)

3 Results and discussion

The ratio of heat dissipation rate RQF, is studied in terms of perforation parameter (b) with S_y =1 mm, S_x = 1 mm, L = 50 mm and T_b =100 °C for different values of fin thickness and thermal conductivity. The results are shown in Figure 5 and 6, which show that thicker fins produced larger heat transfer enhancement at any (b). The variation of RQF with (b) at various (t) showed a consistent trend of increasing to a maximum value followed by a decrease. The net effects of changing area and heat transfer coefficients due to perforations may explain this trend. The increase of RQF in the first part of the curves is due to the fact that A_fp, h_ps and h_pc are increased with (b) which lead to increase the value of RQF. While, in second part the A_fp tends to decrease with (b) but h_ps and h_pc are increased, the net result is a decrease in RQF value. The maximum value of RQF is occurs at some value of perforation dimension symbolized as (b_o). The effect of lateral spacing (S_y) at RQF was studied in terms of (b_o), Sx = 1 mm, L = 50 mm and T_b =100 °C for different values of fin thickness. The results are shown in Figures 7 and 8. The variation of RQF with S_y at various t showed a consistent trend of increasing to a maximum value followed by a decrease. The maximum value of RQF is occurs at some value of lateral perforation spacing symbolized as (S_yo). To compare the performance of the two studied square perforations (parallel and inclined), the maximum RQF at (bo) and (S_yo) was plotted as a function of fin thickness in figure 9. This figure shows that performance of inclined perforation is better for small fin thickness and it is better for parallel perforation for large fin thickness. The converting point depends on the fin thermal conductivity. The fin thickness at which the converting occurs decreases as the fin thermal conductivity increases. It is worth noted that the above results cannot be generalized for other fin configurations, but they can be extended for different fin parameters such as T_b = 50, 150, and 200 °C and L = 30, 40, 60, and 70 mm. Actually, this extensions were conducted and the results were similar to case of L = 50 mm and T_b = 100 °C.Thus, these results did not reported here for seek of brevity.

Fig. 5.

RQF as a function of the perforation dimension at different fin thickness for thermal conductivity of 50 W/m. °C. I) Parallel Square. II) Inclined square

Fig. 6.

RQF as a function of the perforation dimension at different fin thickness for thermal conductivity of 300W/m. °C. I) Parallel square. II) Inclined square.

Fig. 7.

RQF as a function of the perforation lateral spacing at different fin thickness for thermal conductivity of 50 W/m.°C. I) Parallel square. II) Inclined square.

Fig. 8.

RQF as a function of the perforation lateral spacing at different fin thickness for thermal conductivity of 300 W/m.°C. I) Parallel square. II) Inclined square.

Fig. 9.

RQF as a function of the fin thickness for thermal conductivity. I) 50 W/m.°C. II) 300 W/m.°C

4 Conclusions

From this study the following conclusion may be stated:

• 1 Introducing square perforations to fin body increases surface area and heat dissipation for a given range of the perforation dimension.

• 2 for certain values of perforation dimension, the perforated fin can enhance heat transfer. The magnitude of enhancement proportional to the fin thickness.

• 3 The gain in heat dissipation rate for the perforated fin is a strong function of both, the perforation dimension and lateral spacing. This function attains a maximum value at given perforation dimension and spacing, which is may called the optimum perforation dimension (bo), and optimum lateral spacing ( S_yo), respectively.

• 4 The square perforation of inclined type is preferable for low fin thickness and thermal conductivity.

• 5 The square perforation of parallel type is preferable for high fin thickness and thermal conductivity.