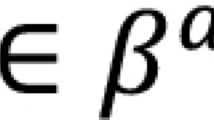Environmental options and technological innovation: an evolutionary game model

Abstract

This paper analyzes the effects on economic agents’ behavior of an innovative environmental protection mechanism that the public administration of a tourist region may adopt to attract visitors while protecting the environment. On the one hand, the public administration sells to the tourists an environmental call option that gives them the possibility of being (partially or totally) reimbursed if the environmental quality in the region turns out to be unsatisfactory. On the other hand, it offers the firms that adopt an innovative, non-polluting technology an environmental put option that allows them to get a reimbursement for the additional costs imposed by the new technology if the environmental quality is sufficiently good. The aim of the paper is to study the dynamics that arise with this financial mechanism from the interaction between the economic agents and the public administration in an evolutionary game context. The evolution of visitors’ and firms’ behavior is modeled in the paper using the so-called replicator dynamics, according to which a given choice spreads across the population as long as its expected payoff is greater than the average payoff. From the model it emerges that such dynamics may lead either to a welfare-improving attractive Nash equilibrium, in which all firms adopt the environmental-friendly technology, or to a Pareto-dominated equilibrium with no technological innovation and no tourism. As shown in the paper, the attraction basin of the virtuous equilibrium will be maximum if total reimbursement is offered by the public administration to the visitors, and will be minimum if a simple entrance ticket is imposed on the tourists with no chance of reimbursement.

1 Introduction

Environmental problems deriving from economic activity and the suitable policy measures to reduce them have been the object of a heated debate among economists in the last decades. Among the many proposals set forth to decrease pollution and/or to protect the environment, much attention has been devoted in the literature to the introduction of specific financial instruments that can integrate the traditional operating of the public sector by providing market incentives to achieve environmental objectives.

One of the most relevant examples of financial assets that can be issued in accordance with environmental purposes is constituted by the so-called Environmental Bond (EB), introduced by Perrings (1987, 1989).¹ The EB is a mandatory deposit paid to the public administration by any agent whose activity may damage the environment. The deposit is (totally or partially) refundable if the holder of the bond can prove to the regulation authority that he avoided the expected environmental damage of his activity. The EB represents, therefore, an incentive-based instrument of environmental risk control (Costanza and Perrings 1990) and can be conceived as a generalization of the deposit-refund systems that have been applied in different contexts characterized by environmental risk, such as compulsory deposits on waste lubricant oil, junked cars, beverage containers, dangerous substances contained in materials or products, and so on (cf. Bohm 1981; Huppes 1988).²

The EB shares some common features with other policy instruments, such as marketable permits, environmental taxes and subsidies. For instance, as some authors have pointed out (Torsello and Vercelli 1998), the EB can be considered symmetrical to tradeable permits. In the latter case, the regulatory authority establishes the total quantity of the permits, leaving their price to be determined by decentralized market decisions; while in the case of an EB system, the authority fixes the price of the EB, or risk premium, for the possible damages caused to the environment, leaving the market free to determine the quantity of EB.

Moreover, the EB can be regarded as the joint implementation of an environmental tax (the price of the EB) and a potential subsidy (the refund), but it is often considered politically more attractive than these two alternative fiscal measures taken separately. In an EB system, in fact, subsidies (refunds) are self-financed by taxes (deposits); therefore—differently from environmental subsidies—the EB does not imply any worsening of the public budget. Moreover, the prospective of a refund often makes the EB more acceptable to public opinion than the environmental taxes, since in the EB the punishment is proportional to the damage effectively produced and the refund is received only by the agents who can prove deserving.

The idea originally set forth by Perrings has been subsequently further developed by Horesh (2000, 2002a, b), who proposed a slightly different kind of EB that are auctioned by the public administration (PA) on the open market, but, unlike ordinary bonds, can be redeemed at the face value only if a specified environmental objective has been achieved. They do not bear any interest, and what yield investors can gain depends on the difference between the auctioned price and the face value in the case of redemption. Economic agents involved in the environmental objective (either polluters or not), once in possession of the bonds, have a strong interest to operate in such a way that the objective itself is quickly achieved, so as to cash in the expected gains as soon as possible.

In our paper, we follow a rather different path, proposing two financial activities, issued by the PA of a tourist region (R), which work like contracts between the PA and, respectively, visitors and firms operating in R, and can be regarded as (cash-or-nothing) environmental call (EC) and environmental put (EP) options. More specifically, the context we analyze has the following features.

An individual who desires to spend a period of time in the region R has to purchase the environmental call (EC) sold by the PA at a given price $\widetilde{p}$. This implies a cost for the visitor in the case of a satisfactory environmental quality, that is, when a properly defined environmental quality index Q is above a given threshold level $\overline{Q}$ fixed by the PA (the value of Q being evaluated by an independent authority), but offers the visitor the possibility of a reimbursement in the case of low environmental quality (namely, when $Q< \overline{Q}$). Consequently, buying the EC represents a self-insurance device that allows the visitor protection from environmental degradation. Thus, potential visitors have to choose between the following strategies:

(V ₁):

visit the region R (and consequently buy the EC)

(V ₂):

not to visit the region.

Analogously, the PA offers to a potentially polluting firm operating in the region R the choice between subscribing or not the environmental put option (EP) issued by the PA. This financial activity is a contract, which binds the firm to adopt a new environmental-friendly technology, thus bearing a supplementary cost given by the difference between the cost of the new, non polluting technology ( c _NP ) and that of the old, polluting technology (c _P ), and implies a financial aid for the firm only if the environmental quality index Q results higher than the threshold level $\overline{Q}$.

Therefore, potentially polluting firms have to choose between the following strategies:

(F ₁):

adopting the new environmental-friendly technology (and subscribing the EP)

(F ₂):

carrying on its activity with the polluting technology in the region R.

We will assume the value of Q to depend on the number of firms choosing the environment-preserving technology, i.e. subscribing the EP.

Hence, if $Q<\overline{Q}$, the visitors choosing V ₁ receive a reimbursement for the low environmental quality experienced during the period spent in R, while the firms choosing F ₁ do not receive any financial aid. If, on the contrary, $Q\geq \overline{Q}$, the visitors choosing V ₁ bear a cost but can enjoy high environmental quality in region R, while the firms choosing F ₁ receive financial support for their investments aimed at protecting the environment. In this way, the PA can achieve the goal of improving the environmental quality at a relatively low cost. As a matter of fact, both visitors and firms have an incentive to protect the environment, the former in order to enjoy a better environmental quality in region R, the latter in order to get financial aid. Therefore, the costs born by the PA to finance the firms that subscribe the EP can be compensated by the revenues the PA cashes in from selling the EC to the visitors.

The PA determines prices and reimbursements taking into account, among other things, the number of visitors and firms aiming to subscribe the financial activities, as well as the cost of the environment-preserving technological innovation.

The financial activities proposed here resemble, under certain aspects, the deposit-refund system implicit in the EB, but differ from it in other respects. In the EB, the burden of the proof falls on the holder, which is often considered to be an attractive feature of the EB. However, this does not eliminate the monitoring costs for the regulatory authority that has to verify the evidence brought forward by the EB holders that their negative externalities were actually lower than expected. On the contrary, the regulatory authority may find it difficult and expensive to attribute the responsibility for a certain damage to a potential polluter (due to asymmetric information, scientific uncertainty, non-point sources and so on). In the present case, instead, the PA should only monitor the overall level of the chosen indicator Q (through an independent environmental authority, as proposed above), which might possibly reduce the monitoring costs of the system, while the agents do not have to suffer the burden of the proof that the environmental damage was lower than expected.

Moreover, the present proposal extends the application of the deposit-refund system typical of the EB from the set of potential polluters to the set of the visitors who would benefit from avoiding pollution. As a consequence, the mechanism described above generates a strong interdependency between the firms’ and the visitors’ payoffs. The aim of the paper is to study the dynamics that arises in this context from the interaction between economic agents (firms and visitors) and the PA.

For this purpose, the choice process of firms and visitors is represented by a two-population evolutionary game, where the population of firms strategically interacts with that of visitors. The evolution of visitors’ and firms’ behavior is modelled using the so-called replicator dynamics (e.g., see Weibull 1995), according to which a given choice spreads among the population as long as its expected payoff is greater than the average payoff. As it emerges from the model, such dynamics may lead to a welfare-improving attractive Nash equilibrium, in which all firms adopt the environmental-friendly technology and all potential visitors choose to visit region R. The attraction basin of this equilibrium expands as the reimbursement due to the visitors increases.

The paper has the following structure. Section 2 introduces the model and Section 3 provides the basic mathematical results. Section 4 concludes.

2 The model

Let us assume that, at each period of time t, potential visitors and firms play a one-shot population game (i.e. all agents play the game simultaneously). Each firm has to choose ex-ante whether to buy the EP and to adopt the new environmental-friendly technology (strategy F ₁) or to keep on using the old polluting technology (strategy F ₂). Similarly, each potential visitor has to choose ex-ante whether to buy the EC and to visit the region R (strategy V ₁) or to go on holiday somewhere else (strategy V ₂). Only the firms (potential visitors) that adopt the new technology (who decide to visit region) can buy the EP (EC). We assume that the potential visitors know ex-ante the criterion (specified below) that is used by the PA to fix the price of the EC; therefore, they also know in advance the maximum price that they might have to pay to visit the region. At the end of the time period t, the PA reimburses firms and visitors who bought the EP and the EC, respectively, on the basis of the data on the environmental quality in region R that are released by an independent environmental agency.

We assume the two populations to be constant over the time and normalize to 1 the number of both potential visitors and firms. Let the variable x(t) denote the share of firms choosing F ₁ at time t, 0 ≤ x(t) ≤ 1. Analogously, let y(t) denote the share of potential visitors adopting choice V ₁ at time t, 0 ≤ y(t) ≤ 1 and let E(Q) be their expected benefit from the environmental quality (measured by index Q) that they can enjoy during the time spent in region R.

For the sake of simplicity, we will assume index Q to be a discrete variable that can take two possible values: Q ^H (“high environmental quality”) and Q ^L (“low environmental quality”). The visitors of region R will thus be reimbursed if Q = Q ^L, while they will get no reimbursement if Q = Q ^H. The opposite applies to the firms that buy the EP.

Let us indicate with $\widetilde{p}(x,y)$ the price (fixed by the PA) of the EC bought by visitors choosing V ₁ (assumed to depend on the proportion of individuals choosing V ₁ and of firms choosing F ₁); and with $\widetilde{r}_{V}(x,y)=\alpha \widetilde{p}(x,y)$ the reimbursement due by the PA to these visitors when Q = Q ^L, where α is a parameter satisfying the condition 0 ≤ α ≤ 1 (α = 1 means that the amount $\widetilde{p}$ is totally reimbursed, whereas, if α = 0, visitors are not reimbursed at all).

We assume that, when the environmental goal is attained (i.e. Q = Q ^H), the payoff of a visitor buying the call option is:

$$ \beta Q^{H}-\widetilde{p} $$

where β is a strictily positive parameter. In case the goal is not attained (i.e. Q = Q ^L), the payoff is:

$$ \beta Q^{L}-\widetilde{p}+\alpha \cdot \widetilde{p}=\beta Q^{L}-\widetilde{p }(1-\alpha ) $$

Denoting by θ(x) the probability that Q = Q ^L (assumed to depend negatively on the proportion of firms adopting the environment-friendly technology), the expected payoff of strategy V ₁ is, therefore, given by:

$$\begin{array}{rll} EV_{1}(x,y) &=&\beta \left[ Q^{L}\cdot \theta (x)+Q^{H}\cdot (1-\theta (x)) \right] -\widetilde{p}(x,y)+\alpha \cdot \widetilde{p}(x,y)\cdot \theta (x) \\[6pt] &=&\beta \left[ Q^{L}\cdot \theta (x)+Q^{H}\cdot (1-\theta (x))\right] - \widetilde{p}(x,y)\left[1-\alpha \cdot \theta (x)\right] \end{array}$$

For the sake of simplicity, we assume:

$$ \widetilde{p}(x,y)=\gamma +\delta y+\varepsilon x $$

(1)

where γ, ε > 0, $\delta \gtreqless 0$ and γ + δ > 0.³

Notice that $\widetilde{p}(x,y)$ is an increasing function of the number x of non-polluting firms. As a matter of fact, the entries obtained by the PA from the visitors through the call options EC can contribute to finance the firms that adopt the innovative technology. Therefore, if x increases, the PA tends to increase the price of the EC to finance the larger amount of the potential reimbursements due to the non-polluting firms. Stated differently, the price paid by the visitors increases as technological progress spreads among the firms of the region, thus improving its environmental quality Q.

The price of the EC, moreover, may be positively or negatively correlated to the number of visitors y, according to the sign of δ. On the one hand, an increase in the number of visitors raises the demand of call options, which induces the PA to increase their price (δ > 0). On the other hand, an increase in the number of visitors tends to enhance the entries available to the PA; therefore, the latter may have an incentive to reduce the price of the call option to attract an even higher number of potential tourists (δ < 0). The sign of δ, therefore, is a priori ambiguous and depends on which one of these two opposite mechanisms will tend to prevail.⁴

Finally, we assume:

$$ \theta (x)=1-x $$

This is equivalent to saying that if all firms adopt strategy F ₁ and invest in the non-polluting technology (x = 1), the environmental quality index Q will certainly be equal to Q ^H (i.e., θ = 0) and the visitors will not be entitled to any reimbursement; whereas such an index will certainly be equal to Q ^L (i.e., θ = 1, and visitors have to be reimbursed) if all firms choose strategy F ₂ (x = 0).

Without loss of generality, we can normalise to zero the payoff of individuals choosing V ₂ (i.e. who decide not to visit the region):

$$ EV_{2}(x,y)=0 $$

In order to reduce the number of parameters of the model and without any loss of generality, we can also normalize to zero the value of Q ^L, i.e. Q ^L = 0. This is equivalent to assuming that the payoff of the visitors who experience a low environmental quality in the region is equal to that of the agents who decided not to visit the region.

Under the assumptions above, the expected payoff of strategy V ₁ becomes:

$$ EV_{1}(x,y)=\beta ^{\prime}x-(\gamma +\delta y+\varepsilon x)\left[ 1-\alpha (1-x)\right] $$

where β′ = βQ ^H.

Turning now to the firm’s decision process, if the environmental goal is missed (Q = Q ^L), the profits of a firm subscribing the put option are:

$$ R(y)-\overline{T}-c_{NP} $$

where:

R(y) are the firm’s revenues, which are an increasing function of the number y of visitors (and are independent of the adopted technology that is assumed to affect only the production costs);

$\overline{T}$ is a lump-sum tax, fixed by the PA, that each firm has to pay to the PA (regardless of the adopted technology) to cover the administrative costs of the mechanism described above as well as for the other services provided by the PA to all firms (e.g. infrastructures, consultancy, etc...);

c _NP  > 0 is a parameter representing the cost of the non-polluting technology plus the cost of the put option sold by the PA.⁵

In case the goal is achieved (Q = Q ^H), the profits are given by:

$$ R(y)-\overline{T}-c_{NP}+\widetilde{r}_{F}(x,y) $$

where $\widetilde{r}_{F}(x,y)$ is the financial aid received by a firm choosing F ₁ in case Q = Q ^H.

Therefore, the expected profits EF ₁ of the firms choosing strategy F ₁ are:

$$ EF_{1}(x,y)=R(y)-\overline{T}-c_{NP}+\widetilde{r}_{F}(x,y)\cdot (1-\theta (x)) $$

where 1 − θ(x) = x is the probability that Q = Q ^H.

If, instead, the firm keeps on using the polluting technology (strategy F ₂), its profits are given by:

$$ EF_{2}(x,y)=R(y)-\overline{T}-c_{P} $$

where c _P is the cost of the traditional (polluting) technology and it is: c _NP  > c _P  > 0.

We assume:

$$ \widetilde{r}_{F}(x,y)=\lambda +\mu y+\nu x $$

where λ, μ > 0 and $\nu \gtreqqless 0$ are parameters fixed by the PA.

Notice that the financial aid received by a firm ($\widetilde{r}_{F}(x,y)$) is positively related to the number y of visitors choosing strategy V ₁ . In other words, as pointed out above, the PA uses the entries deriving from the visitors’ subscription of the EC to finance the firms’ adoption of new, low-impact technologies. Moreover, the financial aid may be positively or negatively related to the share of “clean” firms x. In fact, on the one hand, an increase in x improves the environmental quality of R; this tends to lower the likelihood that the PA will have to reimburse the visitors, thus setting free more financial resources that the PA can use to subsidy the non-polluting firms. On the other hand, an increase in x implies that more firms will be entitled to the financial aid, thus reducing the reimbursement level at disposal for each single firm.

Notice that, in the context described above, the expected budget B(x, y) of the PA is given by:

$$ B(x,y)=\widetilde{p}(x,y)\cdot y\left[ 1-\alpha \cdot \theta (x)\right] + \overline{T}-\widetilde{r}_{F}(x,y)\cdot x(1-\theta (x)) $$

In our analysis, we assume that at every time t the PA sets the taxation level $\overline{T}$ such that the balanced budget condition B(x, y) = 0 is satisfied.⁶

The process of adopting strategies is modelled by the so called replicator dynamics (see, e.g., Weibull 1995), according to which the strategies with expected payoffs greater than the average payoff spread within the populations at the expense of the alternative strategies:

$$ \begin{array}{rll} \overset{\cdot }{x} &=&x\left( EF_{1}-\overline{EF}\right)\\ \overset{\cdot }{y} &=&y\left( EV_{1}-\overline{EV}\right) \end{array} $$

(2)

where

$$ \begin{array}{rll} \overline{EF} &=&x\cdot EF_{1}+(1-x)\cdot EF_{2} \\ \overline{EV} &=&y\cdot EV_{1}+(1-y)\cdot EV_{2} \end{array} $$

are the average payoffs of the populations of firms and visitors, respectively.

We assume that, at the beginning of any period t, economic agents do not know ex ante the values of x and y that will prevail over the period. However, they can learn these values ex post—namely, at the end of period t (the length of which in a continuous dynamic system is reduced to zero)—when the values of x and y become common knowledge to the agents.⁷ Given the bounded rationality hypothesis underlying the evolutionary game presented in the paper, we assume that, at any time t, agents expect that x and y will remain unchanged in the following period and thus use the current values of x and y to form their expectations on the relative performance of the available strategies in the next period. Notice that this assumption implies that, at the limit, the agents’ expectations will be self-fulfilling, since x and y will vary slower and slower as they approach their equilibrium values (i.e. the fixed points).

The replication equations system 2 can be written as follows:

$$ \overset{\cdot }{x} = x(1-x)\left( EF_{1}-EF_{2}\right) =x(1-x)F(x,y) $$

(3)

$$ \overset{\cdot }{y} = y(1-y)\left( EV_{1}-EV_{2}\right) =y(1-y)G(x,y) $$

where:

$$ \begin{array}{l} F(x,y)=-(c_{NP}-c_{P})+\lambda x+\mu xy+\nu x^{2} \\ \\ G(x,y)=-\gamma (1-\alpha )+\left[ \beta ^{\prime}-\alpha \gamma -\varepsilon (1-\alpha )\right] x-\delta (1-\alpha )y-\alpha \delta xy-\alpha \varepsilon x^{2} \end{array} $$

(4)

We assume the parameters to satisfy the following conditions:

$$ \begin{array}{l} C_{1})\text{ \ }0\leq \alpha \leq 1 \\ C_{2})\text{ \ }c_{NP}>c_{P}>0 \\ C_{3})\text{ \ }\beta ^{\prime},\gamma ,\varepsilon >0\text{; }\delta \lesseqqgtr 0\text{; }\gamma +\delta >0 \\ C_{4})\text{ \ }\lambda ,\mu >0\text{; }\nu \lesseqqgtr 0 \\ C_{5})\ \lambda +\mu y+\nu >c_{NP}-c_{P}\ \forall \text{ }y\in \left[ 0,1 \right] \\ C_{6})\ \beta ^{\prime}>\gamma +\delta y+\varepsilon \text{ }\ \forall \text{ }y\in \left[ 0,1\right] \end{array} $$

(5)

We have already discussed above conditions C ₁)–C ₄). As to condition C ₅), this means that, no matter the number of visitors y, non-polluting industries will certainly be financed if their share is sufficiently high (x ∼ 1) (since, in that case, $\overset{\cdot }{x}>0$ ∀ $y\in \left[ 0,1\right]$ and consequently θ = 0). Analogously, condition C ₆) implies that, no matter the number of visitors, if the share of non-polluting industries is sufficiently high ( x ∼ 1), the strategy V ₁ turns out to be the more remunerative one (i.e. EV ₁(x, y) > EV ₂(x, y)). Therefore, at the end of the holidays, the tourists will be satisfied with their choice of coming to visit region R.

3 Analysis of the model

Let us consider the dynamic system 3 the parameters of which satisfy Eq. 5. System 3 is defined in [0, 1]², that is, in the unit square S:

$$ S=\left\{ (x,y):0\leq x\leq 1,0\leq y\leq 1\right\} . $$

All sides of this square are invariant, that is, if the pair (x, y) initially lies on one side, then the whole correspondent trajectory also lies on that side. The following Proposition holds ∀ α ≠ 1.⁸

Proposition 1

System 3 has six equilibria on the boundary of [0, 1]² , i.e. the four vertices plus two points P ₁ = (x ₁, 1) and P ₂ = (x ₂, 0), with 0 < x ₁ < x ₂ < 1. The two vertices (0, 0) and (1, 1) are attractors, while (0, 1) and (1, 0) are saddles. Moreover, P ₁ is a saddle or a repellor if, respectively, G(x ₁, 1) is > 0 or < 0; whereas P ₂ is a saddle or a repellor if, respectively, G(x ₂, 0) is < 0 or > 0.

Proof

Writing the Jacobian matrix J ⁹ at the vertices of [0, 1]², it is easy to check that from conditions 5 it follows: $\det J(0,0)>0$, $\det J(1,1)>0$, $\det J(1,0)<0$, $\det J(0,1)<0$; besides trace J(0,0) < 0, trace J(1, 1) < 0. This proves the statements about the vertices.

Moreover it is easily computed that the intersections of F(x, y) = 0 and G(x, y) = 0 with $\left[ 0,1\right] ^{2}$ are, respectively, the graphs of the functions

$$ y=f(x)=\frac{1}{\mu }\left( \frac{c_{NP}-c_{P}}{x}-\lambda -\nu x\right) $$

(6)

and

$$ y=g(x)=\frac{1}{\delta }\left( \frac{-\gamma \left( 1-\alpha \right) +\left( \beta ^{\prime}-\alpha \gamma -\varepsilon \left( 1-\alpha \right) \right) x-\alpha \varepsilon x^{2}}{1-\alpha +\alpha x}\right) $$

(7)

It follows from conditions 5 that

$$ \underset{x\rightarrow 0+}{\lim }f(x)=+\infty \text{, \ }f(1)<0 $$

and that f(x) either has no extreme (if ν > 0) or has a maximum at some $\overline{x}<0$ and a minimum at some $\overline{\overline{x}}>0$ (if ν < 0). Hence the intersection of y = f(x) with $\left[ 0,1\right] ^{2}$ is the graph of a decreasing function defined in $\left[ x_{1},x_{2}\right]$, 0 < x ₁ < x ₂ < 1, with f(x ₁) = 1 and f(x ₂) = 0.

Analogously, one can check that

$$ \begin{array}{l} g(0)<0\text{, }g(1)>1\text{ \ if }\delta >0 \\ g(0)>1\text{, }g(1)<0\text{ \ if }\delta <0\text{, }\gamma +\delta >0 \end{array} $$

and that g(x) has a minimum (maximum) at some $\widetilde{x}<0$ and a maximum (minimum) at some $\widetilde{\widetilde{x}}>0$ if δ > 0 ( δ < 0). This implies that: if δ > 0, y = g(x) is an increasing function defined in an interval $\left[ x_{3},x_{4}\right]$, 0 < x ₃ < x ₄ < 1, with g(x ₃) = 0 and g(x ₄) = 1; if δ < 0 and γ + δ > 0, y = g(x) is a decreasing function defined in an interval $\left[ x_{5},x_{6}\right]$, 0 < x ₅ < x ₆ < 1, with g(x ₅) = 1 and g(x ₆) = 0.

Hence the other statements of the Proposition follow in a straightforward way. □

Notice that, in the four vertices of the square, only one strategy is played by firms and potential visitors. In particular, in the attractor (1, 1), all firms adopt the non-polluting technology and all potential tourists choose to visit the region, as they are attracted by its high environmental quality deriving from the widespread adoption in the region of new, environmental-friendly technologies. The opposite holds in the attractor (0, 0): all firms keep on using the traditional technology causing high pollution in the region. Therefore, none of the potential tourists decides to come to visit R. In (0, 1), all firms are polluting; nevertheless, all potential visitors choose to spend their holidays in the region R. In this case, therefore, the visitors are attracted by the reimbursement received rather than by the environmental quality of R. This fixed point might describe the case of some popular tourist destinations where -despite the low environmental quality (e.g. polluted sea and crowded beaches)– tourists are mainly attracted by the low costs of the area (which is equivalent to getting a reimbursement that lowers the holiday costs in the present case). Notice, however, that this fixed point is non attractive, and so it is not a Nash equilibrium of the model. Mutatis mutandis, the same reasoning (and dynamic features) obviously applies to the saddle point (1, 0): although the quality of the environment of region R is extremely high (all firms being non-polluting), potential visitors care more for the holiday costs than for the environmental quality of R. Therefore, in this case, they do not come to the region, since there is no chance of reimbursement.

Beyond the vertices of the unit square S, system 3 can have two more possible equilibria on the boundaries, P ₁ = (x ₁, 1) and P ₂ = (x ₂, 0), in which heterogeneous strategies are played by the firms (some firms adopt the new environmental-friendly technology, others keep on using the old technology), while a unique strategy is played by the population of individuals (they all choose either to visit or not to visit the region, respectively). None of these two equilibria, however, is an attractor, being either sources or saddles.

In addition to the boundary equilibria described in Proposition 1, system 3 can have up to three equilibria in the interior of S. The next Proposition and the following Remark provide a complete characterization of these internal equilibria.

Proposition 2

The internal equilibria of system 3 , i.e. the equilibria lying in the open square $(0,1)^{2}=\left\{ 0<x<1,0<y<1\right\}$ , can be 0, 1, 2 or 3. More precisely:

• if δ > 0, there is at most one internal equilibrium, which, in case it exists, is a saddle;

• if δ < 0, the number of internal equilibria (counted by their multiplicity) is even if G(x ₁, 1)·G(x ₂, 0) > 0 , odd if G(x ₁, 1)·G(x ₂, 0) < 0;

• no internal equilibrium is attracting: in particular, there exist at most one internal saddle and at most two internal repellors.

Proof

As the two hyperbolas F(x, y) = 0 and G(x, y) = 0 have a common asymptotic direction, that of the y-axis, their intersections in the real Euclidean plane are at most three: in fact, the equation f(x) = g(x), x > 0, is easily transformed into an equation P(x) = 0, where P(x) is a third degree polynomial. Actually, the numerical examples below show that all the possible cases (0, 1, 2 or 3 internal equilibria) are feasible for suitable values of the parameters satisfying Eq. 5. Then we analyze the stability of a possible internal equilibrium. So, let $Q=\left( x^{\ast },y^{\ast }\right)$ be an internal equilibrium and denote by J(Q) its Jacobian matrix. Recall (see the Proof of Proposition 1) that $\left\{ F=0\right\} \cap \left[ 0,1\right] ^{2}$ is given by the graph of a decreasing function y = f(x), while $\left\{ G=0\right\} \cap \left[ 0,1 \right] ^{2}$ is given by the graph of a function y = g(x), increasing when δ > 0 and decreasing when δ < 0, both taking values y ∈ [0, 1] . Recalling conditions 5, it is easily checked that $\det J(Q)<0$ if δ > 0; while, if δ < 0

$$ \det J(Q)\gtrless 0\text{ \ iff \ }\left\vert f{^\prime }\left( x^{\ast }\right) \right\vert \gtrless \left\vert g^{\prime }\left( x^{\ast }\right) \right\vert $$

Moreover, when δ < 0, being y = f(x) decreasing in $\left[ 0,1\right] ^{2}$, it follows that $\frac{\partial F}{\partial x}$, $\frac{\partial G}{ \partial y}$ and thus trace(J(Q)) are positive (in fact $\frac{\partial F}{ \partial x}$ must have the same sign of $\frac{\partial F}{\partial y}$, which is positive since μ > 0, while $\frac{\partial G}{\partial y}$ has the sign of − δ). Therefore Q is either a saddle or a repellor.

In particular, suppose that δ < 0 and three internal equilibria exist, say $Q_{1}=\left( x_{1}^{\ast },y_{1}^{\ast }\right)$, $Q_{2}=\left( x_{2}^{\ast },y_{2}^{\ast }\right)$, $Q_{3}=\left( x_{3}^{\ast },y_{3}^{\ast }\right)$, $x_{1}^{\ast }<x_{2}^{\ast }<x_{3}^{\ast }$. Then it is easily observed that $\left\vert f^{\prime }\left( x_{i}^{\ast }\right) \right\vert >\left\vert g^{\prime }\left( x_{i}^{\ast }\right) \right\vert$ when i = 1,3, whereas $\left\vert f^{\prime }\left( x_{2}^{\ast }\right) \right\vert <\left\vert g^{\prime }\left( x_{2}^{\ast }\right) \right\vert$. Hence Q ₁ and Q ₃ are repellors, while Q ₂ is a saddle. □

Remark 3

It follows from the arguments developed in the Proof of the above Proposition that two consecutive non-degenerate¹⁰ equilibria lying on $\left\{ F=0\right\} \cap \left[ 0,1\right] ^{2}$ are one saddle and one repellor.

The Propositions examined so far analyze the existence and stability of the boundary equilibria and of the (possible) internal equilibria of system 3. The phase portrait of system 3 can be fully described by combining the results of the previous Propositions with the following one that shows that there cannot exist any limit cycle inside the square S.

Proposition 4

System 3 admits no limit cycle in (0, 1)².

Proof

Due to the Index Theorem (see, for example, Guckenheimer and Holmes 1983) and the results of Proposition 3, a possible limit cycle in (0, 1)² must surround some repellor (precisely, either one repellor or two repellors and one saddle). Because of Proposition 3, this implies δ < 0. Hence, let $Q=\left( x^{\ast },y^{\ast }\right)$ be an internal repellor. It is easily checked that either $P_{1}=\left( x_{1},1\right)$ or $P_{2}=\left( x_{2},0\right)$ is such that no other equilibrium exists in the strip $\left[ x_{1},x^{\ast }\right] \times \left[ 0,1\right]$ (or $\left[ x^{\ast },x_{2}\right] \times \left[ 0,1\right]$ ) and, correspondingly, P ₁, or P ₂, is a saddle.

Assume this is true for P ₁ (mutatis mutandis the same applies to P ₂) and consider the triangoloid $T = \left\{ x_{1}\leq x\leq x^{\ast }\text{, }g(x)\leq y\leq f(x)\right\}$, with sides $L_{1}=\left\{ x=x_{1}, g(x_{1})\leq y\leq 1\right\}$, $L_{2}=\left\{ x_{1}\leq x\leq x^{\ast }\text{, }y=g(x)\right\}$, $L_{3}=\left\{ x_{1}\leq x\leq x^{\ast }\text{, }y=f(x)\right\}$.

Then it is easily observed that the vector field points outward T along L ₁ ∪ L ₂ ∪ L ₃ and there must exist a separatrix in T between the trajectories crossing L ₁ ∪ L ₂ and those crossing L ₃. It follows that such a separatrix must be a trajectory joining P ₁ and Q, which can be represented by the graph of some decreasing function y = l(x), $x_{1}\leq x\leq x^{\ast }$. Thus Q cannot be surrounded by a limit cycle. The same argument holds if P ₁ is replaced by P ₂. □

Since all the additional (boundary and internal) equilibria beyond the vertices of the square are unstable equilibria and no limit cycle exists inside the square, from the Propositions examined so far it follows that “almost every” trajectory of system 3 (i.e. excluded those belonging to a zero-measure subset of the square [0, 1] ²) approaches one of the attracting vertices of the square.¹¹ Therefore, no matter the initial conditions, the dynamics of the system will almost always lead to one of the two attractors of the square.

In what follows, with the help of numerical simulations, we provide a few examples of some possible dynamics that can emerge in the model with 0, 1, 2 or 3 internal equilibria.¹²

Example 5

Consider the following set of parameters: c _NP  − c _P  = 36 + ρ, λ = 60, μ = 84 + σ, ν = 0, α = 0.6, β′ = 191, γ = 90.9, ε = 100, δ = − 32. Then straightforward computations allow to check that:

• 1. when ρ = 0, σ = 0, the system 3 corresponding to the above parameters has three internal equilibria;

• 2. when ρ = 0.225, σ = 0.9, the system 3 corresponding to the above parameters has two internal equilibria;

• 3. when ρ = − 1, σ = − 4, the system 3 corresponding to the above parameters has one internal equilibrium;

• 4. when ρ = 1, σ = 4, the system 3 corresponding to the above parameters has no internal equilibrium.

Figures 1, 2, 3, and 4 illustrate, through simulations, the phase portraits of the system in the above cases.

Fig. 1

Three internal equilibria. Parameter values: c _NP  − c _P  = 36, λ = 60, μ = 84, ν = 0, α = 0.6, β′ = 191, γ = 90.9, ε = 100,δ = − 32

Fig. 2

Two internal equilibria. Parameter values: c _NP  − c _P  = 36.225, λ = 60, μ = 84.9, ν = 0, α = 0.6, β′ = 191, γ = 90.9, ε = 100, δ = − 32

Fig. 3

One internal equilibrium. Parameter values: c _NP  − c _P  = 35, λ = 60, μ = 80, ν = 0, α = 0.6, β′ = 191, γ = 90.9, ε = 100, δ = − 32

Fig. 4

No internal equilibria. Parameter values: c _NP  − c _P  = 37, λ = 60, μ = 88, ν = 0, α = 0.6, β′ = 191, γ = 90.9, ε = 100, δ = − 32

Attractors, repellors and saddle points are represented in the figures by full circles, empty circles and full squares, respectively. The attraction basins of (0, 0) and (1, 1) are separated in the figures by the bold line that connects the two boundary equilibria (x ₁, 1) and (x ₂, 0). The following Proposition provides a complete description of the separatrix.

Proposition 6

The separatrix between the attracting basins of (0, 0) and (1, 1) is a curve Γ joining the boundary equilibria P ₁ = (x ₁, 1) and P ₂ = (x ₂, 0). Γ can be represented as the graph of a decreasing function $x=h\left( y\right)$ , h(0) = x ₂ , h(1) = x ₁.

Proof

As system 3 has no attractor or limit cycle in (0, 1)², the only attractors are the vertices (0,0) and (1,1). The separatrix, Γ, between their attracting basins must be a union of stable manifolds of some saddles. Since the stable manifolds of (0,1) and (1,0) lie on the boundary of the square, Γ is the union of stable manifolds of saddles lying on $\left\{ F=0\right\} \cap \left[ 0,1\right] ^{2}$, i.e. along the graph of the decreasing function y = f(x), f(x ₁) = 1 , f(x ₂) = 0. Moreover, we have seen from Remark 4 that saddles and repellors alternate along y = f(x). Therefore, along Γ, each saddle is connected to a repellor by an arc representing the graph of some decreasing function and thus the whole Γ can be regarded as the graph of a decreasing function, which, for later convenience, we represent as x = h(y), with h(0) = x ₂ and h(1) = x ₁. □

Consider, for instance, Fig. 1 that describes the dynamic regimes that may emerge in the model when the highest possible number of internal equilibria occurs. As the arrows in the figures show, the dynamics are path dependent. As a matter of fact, if the initial levels of firms and tourists that buy the options are sufficiently high (i.e. x and y are above the separatrix), then all the other agents will tend to imitate their behavior and the system will eventually converge towards (1, 1). If, on the contrary, the initial values of x and y are sufficiently low (i.e. below the threshold level given by the separatrix), then the opposite strategies F ₂ and V ₂ will tend to spread among the populations of firms and potential visitors and the system will converge towards (0, 0). Although the morphology of the attraction basins may differ from one case to the other, similar “threshold effects” emerge also in the other cases, regardless of the number (from zero to three) and stability features (saddles or repellors) of the internal equilibria. As the arrows show, in all possible cases the system will eventually converge towards one of these two attractors depending on whether the initial values of x and y lie above or below the separatrix.

Let us now compare the expected payoffs of the agents in the attracting vertices.

Proposition 7

Under the assumptions C ₁ –C ₆ , the equilibrium (1, 1) Pareto-dominates the other attracting equilibrium (0, 0) of system 3 ; i.e. EV ₁(1, 1) > EV ₂(0, 0) and EF ₁(1, 1) > EF ₂(0, 0).

Proof

Notice that the expected payoffs evaluated in (1, 1) and (0, 0) are, respectively:

$$\begin{array}{rll} EV_{1}(1,1) &=&\beta ^{\prime}-(\gamma +\delta +\varepsilon ) \\ EF_{1}(1,1) &=&R(1)-\overline{T}-c_{NP}+(\lambda +\mu +\nu ) \end{array}$$

and

$$\begin{array}{rll} EV_{2}(0,0) &=&0 \\ EF_{2}(0,0) &=&R(0)-\overline{T}-c_{P} \end{array}$$

where it is always EF ₁(1, 1) > EF ₂(0, 0) under assumption C ₅ and EV ₁(1, 1) > EV ₂(0, 0) under assumption C ₆. □

From the Proposition above, it follows that (1, 1) is a “virtuous equilibrium”, since the region achieves the highest possible levels of environmental quality and tourism, and all agents (visitors and firms) are better-off than in the alternative sink (0, 0) of system 3. The latter, on the contrary, may be interpreted as a “poverty trap”¹³ to which the system may converge, leading to a “vicious equilibrium” in which the region R is extremely polluted and unable to attract any tourist. To minimize this risk, therefore, the PA will try to fix the parameter values so as to maximize the attraction basin of (1, 1), thus increasing as much as possible the set of initial values of x and y that make the system converge to the virtuous equilibrium. The following Proposition describes one possible way in which the PA may achieve this goal.

Proposition 8

The basin of attraction of (1, 1) expands as α increases.

Proof

Consider two systems of type 3, say $\Sigma _{i}=\left( \overset{\cdot }{x}_{i},\overset{\cdot }{y}_{i}\right)$, i = 1, 2 , differing only for the value of the parameter α, i.e. 0 ≤ α ₁ < α ₂ < 1. Denote by Γ _i , x = h _i (y), the respective separatrices, clearly intersecting at the equilibria P ₁ = (x ₁, 1) and P ₂ = (x ₂, 0). Now take, for example, a point $P=\left( x_{0},y_{0}\right) \in \Gamma _{2}$ which is not an equilibrium for either system. Then at P $h_{2}^{\prime }\left( y_{0}\right) =\frac{ \overset{\cdot }{y}_{2}(P)}{\overset{\cdot }{x}_{2}(P)}<0$ implies that $\overset{\cdot }{y}_{2}$ and $\overset{\cdot }{x}_{2}$ have opposite signs. On the other hand, it follows from ε > 0 and γ + δ > 0 (condition C ₃) that $\frac{\partial \overset{\cdot }{y}}{\partial \alpha }>0$, so that $\overset{\cdot }{y}_{1}(P)<\overset{\cdot }{y}_{2}(P)$ , while $\overset{\cdot }{x}_{1}(P)=\overset{\cdot }{x}_{2}(P)$. Thus it is easily checked that the vector $\left( \overset{\cdot }{x}_{1}(P),\overset{ \cdot }{y}_{1}(P)\right)$ points, at P, to the left of Γ₂ , that is inside the attracting basin of $\left( 0,0\right)$ for system Σ₂. Now, let us suppose, by contradiction, that the statement of the Proposition is not true. Hence there should exist two arcs $\widetilde{\Gamma_{1}}\subseteq \Gamma _{1}$ and $\widetilde{\Gamma_{2}}\subseteq \Gamma _{2}$, having the same end-points, such that $\widetilde{\Gamma _{2}}$ lie at the right of $\widetilde{\Gamma_{1}}$ . In other words, there should exist y′ and y″, 0 ≤ y′ < y″ ≤ 1, such that $h_{1}(y^{\prime })=h_{2}(y^{\prime })$, $h_{1}(y^{\prime \prime })=h_{2}(y^{\prime \prime })$ and h ₁(y) < h ₂(y) for y′ < y < y″. Consider the loop constituted by $\widetilde{\Gamma _{1}}\cup \widetilde{\Gamma _{2}}$ and denote by $D=\left\{ h_{1}(y)<x<h_{2}(y),\text{ }y^{\prime }<y<y^{\prime \prime }\right\}$ its interior. Take the positive (i.e. defined for t ≥ 0) trajectory from a point P ∈ D in system Σ₁. As Γ₁ is invariant for Σ₁ and $\left( \overset{\cdot }{x}_{1},\overset{\cdot }{y}_{1}\right)$ along Γ₂ points inside D, such a trajectory cannot leave D. But this is a contradiction, since the trajectory of Σ₁ from P must tend to $\left( 1,1\right)$ as t→ + ∞. Therefore, Γ₂ lies entirely at the left of Γ₁ for 0 < y < 1 and thus the attracting basin of $\left( 1,1\right)$ is larger in system Σ₂ than in system Σ₁. □

Therefore, by increasing the reimbursement share α, the PA can enhance the attraction basin of the first best outcome. In other words, the higher the reimbursement share α, the lower the initial values of x and y that are needed to converge to (1, 1). If this is the case, it is sufficient that a lower initial number of firms (visitors) choose the financial instruments proposed by the PA (EP and EC, respectively) to convince all other firms (potential visitors) to imitate their choice and behave the same way.

The attraction basin of (1, 1) will obviously be maximum when α = 1 (i.e. the price of the call-option is totally reimbursed if the environmental goal is missed) and minimum when α = 0 (i.e. no reimbursement occurs). Notice that, in the latter case, the price paid by the tourists becomes simply a tourist tax, that is, an entrance ticket that tourists pay to have access to the region.

We can conclude that the financial mechanism proposed here (that allows visitors to be refunded in case of an unsatisfactory environmental quality) is more likely to lead the system towards the virtuous equilibrium (1, 1) than the traditional entrance ticket without any refund possibility. Moreover, by proposing total reimbursement in case of low environmental quality, the PA actually minimizes the probability of refunding the tourists, since this maximizes the attraction basin of the non-polluted equilibrium (1, 1).

Figure 5 shows the phase portrait of a system of type 3, where the parameters are the same as in Fig. 4, except α , which is set equal to 0.9.

Fig. 5

A larger basin of attraction for (1, 1). Same parameter values as in Fig. 4, except α = 0.9

Finally, the following Proposition describes the case α = 1.¹⁴

Proposition 9

Let α = 1 in system 3 . Then the side x = 0 of [ 0, 1]² is filled with (degenerate) equilibria. The other equilibria are: the attractor (1, 1), the saddles (1, 0) and P ₁ = (x ₁, 1), the repellor P ₂ = (x ₂, 0) (P ₁ and P ₂ denote, as above, the intersections of F = 0 with the boundary of [0, 1]²). There is no internal equilibrium. Moreover there exists a separatrix Γ, which can be represented by the graph of a decreasing function $x=h\left( y\right)$ , h(0) = x ₂ , h(1) = x ₁ , such that:

• if $P_{0}=\left( x_{0},y_{0}\right)$ satisfies 0 < y ₀ ≤ 1, $h\left( x_{0}\right) <x_{0}\leq 1$ , then the trajectory from P ₀ converges to $\left( 1,1\right)$ as t→ + ∞;

• if $P_{0}=\left( x_{0},y_{0}\right)$ satisfies 0 ≤ y ₀ ≤ 1, $0<x_{0}<h\left( y_{0}\right)$ , then the trajectory from P ₀ converges to a point $\left( 0,\overline{y}\right)$ ; furthermore, for any $\overline{ y}\in \left[ 0,1\right]$ there exists exactly one trajectory in $\left\{ 0\leq y\leq 1,0<x<h(y)\right\}$ converging to $\left( 0,\overline{y}\right) .$

As stated in the Proposition above, when α = 1 (total reimbursement), there exists a continuum of equilibria along the side x = 0 (rather than the only attracting equilibrium (0, 0)) and a unique trajectory leading to each of them (see Fig. 6). This implies that, if the initial values of x and y are sufficiently low (i.e. below the separatrix ), we can have any number of visitors at the equilibrium depending on the initial situation. In this case, we have minimum environmental quality (all firms being polluting) and maximum reimbursement. Therefore, it is not possible to predict a priori whether the tourists will be more attracted by the possibility of being totally reimbursed or more discouraged by the degradation of the environmental quality in region R. As a matter of fact, in this case, the expected payoff of strategy V ₁ (visit the region) equals zero and the potential visitors will be indifferent between coming or not coming to the region (i.e. EV ₁ = EV ₂).

Fig. 6

The side x = 0 of the square is filled with equilibria. Same parameter values as in Fig. 4, except α = 1

4 Conclusions

The present paper has suggested an innovative financial mechanism that the PA of a tourist region may adopt to attract visitors while protecting the environment. On the one hand, the PA sells to the tourists an environmental call option that gives them the possibility of getting a reimbursement if the environmental quality in the region turns out to be unsatisfactory (i.e. below a given threshold level). On the other hand, the PA offers the firms that adopt an innovative, non-polluting technology the possibility of getting a reimbursement to cover the additional costs imposed by the new technology if the environmental quality turns out to be sufficiently good (i.e. above the threshold level).

Since the two kinds of reimbursements (to visitors and firms) are linked to the same environmental index, they will tend to compensate each other. More precisely, if the environmental quality target is achieved, the entries that the PA gets from selling the call options to the visitors plus those possibly earned from selling the put options to the firms contribute to finance the financial aid given to the non-polluting firms. If, on the contrary, the environmental quality target is missed, the entries earned by the PA finance the reimbursements due to the visitors. The fund-raising mechanism proposed here, therefore, could be a useful instrument to promote and spread across the firms a technological shift from a polluting technology towards a more environmental-friendly one.

The mechanism described above extends to a two-population game the deposit-refund systems that have been applied in some specific contexts and that provide the basic idea underlying the environmental bonds proposed in the literature. Differently from these instruments, however, in the present case, the burden of the proof does not fall on the holder of the financial instrument, since the reimbursement is linked to the observed performance of an overall environmental quality index. This may have a twofold effect: on the one hand, it reduces the costs that a firm may encounter to prove ex-post that its activity did not actually damage the environment and, on the other hand, it generates a strong interdependency between the choices of the two populations (firms and visitors). The present mechanism, moreover, can reduce the risk of moral hazard behavior that may arise with the environmental bonds. The latter instrument, in fact, may induce the PA to overestimate the environmental degradation provoked by a single firm to avoid refunding it, whereas, in the present case, the reimbursement depends on the observed values of an environmental quality index measured by an independent external agency.

As shown in the paper, the system is characterized by a multiplicity of possible equilibria (up to six fixed points along the boundaries and up to three in the interior of the unit square). From the dynamics that emerge in the model, it turns out that only two of these possible equilibria are attractors (namely, the fixed points (1, 1) and (0, 0)) and that almost all trajectories will converge to them, since no limit cycle may occur in the interior of the unit square. In both attractors, all the agents of each population choose the same strategy. Both firms and tourists would be better-off at the “virtuous equilibrium” (1, 1) in which all firms adopt the non-polluting technology and all potential visitors come to visit the region. However, the trajectories deriving from the interaction between the two populations may also lead to an attracting poverty trap in which all firms are polluting and no tourist come to the region (0, 0).

Whether the system will converge to the first-best equilibrium or to the alternative attractor will depend on the initial share of firms (x) and potential tourists (y) that buy the environmental call and put options offered by the PA. If these shares are sufficiently high, then the system is likely to converge to the virtuous equilibrium (1, 1). Otherwise it may end up in a Pareto-dominated attracting equilibrium from which the PA may find it difficult to escape. The final outcome towards which the system will eventually converge is, therefore, strongly path-dependent for the existence of threshold effects and imitative behaviors that spread the most remunerative strategy across the agents within each population. The PA, however, can affect these threshold effects by modifying the reimbursement share due to the visitors in case of a low environmental quality in the region. If the PA aims at simultaneously achieving the maximum environmental quality and the maximum number of tourists, it should offer total reimbursement to the visitors, as this maximizes the attraction basin of the virtuous equilibrium (1, 1). If, on the contrary, the PA levies a simple entrance ticket on the tourists with no chance of being reimbursed, this minimizes the attraction basin of (1, 1), increasing the critical mass of x and y that are needed to escape the poverty trap (0, 0). Increasing the reimbursement share, therefore, might paradoxically lower the costs of the financial mechanism for the PA: if the system converges to (1, 1), no reimbursement will be paid by the PA to the tourists and the entries obtained from the call options can be used by the PA to finance the firms for their virtuous (non-polluting) behavior.

In our opinion, the present analysis could be extended in several directions in the future. In particular, it would be interesting to compare the present results with those emerging from an optimal control model in which the PA enters as a third player of the game that aims at maximizing its own objective function. Another important issue that would deserve further analysis concerns the optimal determination by the PA of the threshold level $\overline{Q}$ that determines the eligibility of firms and visitors to the reimbursement when the environmental quality Q can take on a continuum of values. However, further research will be needed to investigate these problems in the future.