Abstract
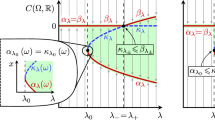
We consider a particular class of multidimensional nonlinear stochastic differential equations with 0 as a fixed point. The almost sure stability or instability of 0 is determined by the Lyapunov exponent λ for the associated linear system. If parameters in the stochastic differential equation are varied in such a way that λ changes sign from negative to positive then 0 changes from being (almost surely) stable to being (almost surely) unstable and a new stationary probability measure μ appears. There also appears a new Lyapunov exponent \( \tilde \lambda \), say, corresponding to linearizing the original stochastic differential equation along a trajectory with stationary distribution μ. The value of \( \tilde \lambda \) determines stability or instability along trajectories. We show that, under appropriate conditions, the ratio \( \tilde \lambda \)/λ has a limiting value Γ at a bifurcation point, and we give a Khasminskii-Carverhill type formula for Γ. We also provide examples to show that Γ can take both negative and positive values.
Research supported in part by Office of Naval Research contract N00014-96-1-0413.
This is a preview of subscription content, log in via an institution to check access.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Preview
Unable to display preview. Download preview PDF.
Similar content being viewed by others
9 References
L. Arnold. Random dynamical systems. Springer, Berlin Heidelberg New York, 1988.
L. Arnold, N. Sri Namachchivaya and K. Schenk-Hoppé. Toward an understanding of stochastic Hopf bifurcation. Internat. J. Bifur. Chaos Appl. Sci. Engrg 6:1947–1975, 1996.
P.H. Baxendale. Brownian motions in the diffeomorphism group I. Compositio Math. 53:19–50, 1984.
P.H. Baxendale. Asymptotic behaviour of stochastic flows of diffeomorphisms. In Stochastic processes and their applications. Proc. Nagoya 1985. (K. Itô and T. Hida, eds.) Lect. Notes Math. 1203:1–19. Springer, Berlin Heidelberg New York, 1986.
P. H. Baxendale. Invariant measures for nonlinear stochastic differential equations. In Lyapunov Exponents. Proc. Oberwolfach 1990. (L. Arnold, H. Crauel and J.-P. Eckmann, eds) Lect. Notes Math. 1486:123–140. Springer, Berlin Heidelberg New York, 1991.
P. H. Baxendale. A stochastic Hopf bifurcation. Probab. Th. Rel. Fields 99:581–616, 1994.
P.H. Baxendale and T.E. Harris. Isotropic stochastic flows. Ann. Probab. 14:1155–1179, 1986.
A.P. Carverhill. A formula for the Lyapunov numbers of a stochastic flow. Application to a perturbation theorem. Stochastics 14:209–226, 1985.
H. Crauel and F. Flandoli. Attractors for random dynamical systems. Probab. Theory Relat. Fields 100:365–393, 1994.
R. Z. Khas’minskii. Necessary and sufficient conditions for the asymptotic stability of linear stochastic systems. Theory Probab. Appl. 12:144–147, 1967.
R.Z. Khas’minskii. Stochastic stability of differential equations. Sijthoff and Noordhoff, Alphen aan den Rijn, 1980.
H. Kunita. Stochastic flows and stochastic differential equations. Cambridge University Press, 1990.
H. Kunita and S. Watanabe. On square integrable martingales. Nagoya Math. J. 30:209–245, 1967.
N. Sri Namachchivaya. Stochastic bifurcation. Appl. Math. and Comp. 38:101–159, 1990.
A. Yaglom. Some classes of random fields in n-dimensional space, related to stationary random processes. Theory Probab. Appl. 2:273–320, 1957.
Rights and permissions
Copyright information
© 1999 Springer-Verlag New York, Inc.
About this chapter
Cite this chapter
Baxendale, P.H. (1999). Stability Along Trajectories at a Stochastic Bifurcation Point. In: Stochastic Dynamics. Springer, New York, NY. https://doi.org/10.1007/0-387-22655-9_1
Download citation
DOI: https://doi.org/10.1007/0-387-22655-9_1
Publisher Name: Springer, New York, NY
Print ISBN: 978-0-387-98512-1
Online ISBN: 978-0-387-22655-2
eBook Packages: Springer Book Archive