Keywords

1 Introduction

Thermal convection is a key phenomenon for heat and mass transfer and mixing. Bénard [1] considered a horizontal layer. After Bénard, a lot of researchers have studied practical problems of thermal convection.

Here, we considers thermal convection in a cavity (or a container). One of the simplest cavities is a cube. Especially for thermal convection in a cube, for example Pallares et al. [2,3,4] numerically showed three-dimensional flow structures in the cubic cavity for moderate Rayleigh numbers Ra < 6.0 × 104. They reported seven different steady-flow structures; namely, four kinds of single-roll structures (referred to as S1, S2, S3 and S7), two kinds of four-roll structures (referred to as S5 and S6), and one kind of a toroidal-roll structure (referred to as S4).

In this report, we reports a new flow structure in laminar and steady thermal convection as one facet of conductive diversity. This flow structure involves three downwelling and three matching upwellings in a cube heated from below and chilled from above, as well as our past studies [5,6,7,8] This flow structure is not transient but stable. So, it is commonly observed in various situations, such as in a stationary cube under the gravity and in an oscillating cube under the gravity or zero gravity besides.

2 System Modelling

2.1 Model and Governing Equation

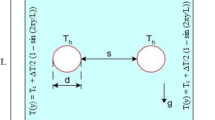
Figure 1 shows the current model. The model is the flow in a cubic cavity with a length scale H*. We analyse the thermal convection assuming incompressible flow with a constant Prandtl number Pr = 7.1 (water) in the non-gravitational field. A pair of opposed walls are taken to be isothermal, and the temperature one of them (hereinafter, referred to as a cold wall) is greater than the other (hereinafter, referred to as a hot wall). The four walls beside the cold and hot walls (hereinafter, referred to as sidewalls) are conductive.

Fig. 1
figure 1

Computational domain, together with coordinate system and boundary conditions

Full size image

The governing equations are the continuity equation, the dimensionless Navier-Stokes equations with the Boussinesq approximation and a dimensionless energy equation. They are as follows;

$$ \nabla \cdot \varvec{u} = 0 $$
(1)
$$ \frac{{D\varvec{u}}}{Dt} = - \nabla p + Pr\Delta \varvec{u} + Ra_{\eta } PrT\,\sin \omega t{\mathbf{e}}_{z} $$
(2)

and

$$ \frac{DT}{Dt} = \Delta T $$
(3)

where \( \varvec{u} = \left( {u,v,w} \right) \), t, p, T, e z and ω denote velocity vector, time, pressure, tempera-ture, the unit vector in the z direction and (angular) frequency, respectively. We the definitions of Ra η and Pr in the following subsection.The governing equations Eqs. (1)–(3) are solved by a finitedifference method based on the MAC method with the FTCS scheme using a staggeredmesh system.

2.2 Governing Parameter

Non-dimensional governing parameters are as follows;

$$ \begin{aligned} Ra_{\eta } & = \frac{{\eta^{*} \beta^{*} \left( {T_{\text{h}}^{*} - T_{\text{c}}^{*} } \right)H^{*3} }}{{\nu^{*} \alpha^{*} }},\,pr = \frac{{\nu^{*} }}{{\alpha^{*} }},\,\omega = \frac{{\omega^{*} H^{*} }}{{\alpha^{*} }}\,{\text{and}} \\ Ra & = \frac{{g^{*} \beta^{*} \left( {T_{h}^{*} - T_{c}^{*} } \right)H^{*3} }}{{\nu^{*} \alpha^{*} }}, \\ \end{aligned} $$
(4)

where β*, ν*and η* denote thermal expansion coefficient, kinetic viscosity and acceleration amplitude, respectively. In the present study, Pr is fixed to 7.1 (water). The test ranges of Ra η and ω are from 5.0 × 103 to 1.1 × 104 and from 1.0 × 100 to 1.0 × 103, respectively.

As global indicators, we considers such physical quantities as a spatially-averaged kinetic energy \( \overline{K} \). These are defined as follows.

$$ \overline{K} = \frac{1}{V}\iiint {\left. {\left\{ {\frac{1}{2}\left( {u^{2} + v^{2} + w^{2} } \right)} \right.} \right\}dxdydz} $$
(5)

3 Results and Discussion

3.1 Flow Structure Sα

Figure 2 shows a result of the present computations for Ra = 8.0 × 104 and Pr = 7.1, being based on many preliminary computations over wide ranges of system parameters. The figure is a perspective view looking downward. Three-dimensional flow structure is visualised by iso-kinetic-energy surfaces of 0.38 × Kmax, where Kmax is the maximum value of kinetic energy K.

Fig. 2
figure 2

Perspective view (look-down view) of the flow structure visualised by iso-kinetic-energy surfaces of 0.38 × Kmax for Ra = 8.0 × 104 and Pr = 7.1. The value of w is represented by gray scale

Full size image

Brightness of the surfaces represents the value of w which is shown as a legend on the lower right of the figure. The flow visualisation based on K is more suitable for the concerning laminar problem to grasp the whole three-dimentional structure, than other physical quantities such as velocity vector u, temperature T, vorticity vector and the second invariant of the velocity-gradient tensor.

From Fig. 2, we can see a pair of trident currents on thermal convection in a cube, which consist of three ascending streams and three matching descending streams. we name this flow structure Sα. Until now, this trident convection in a cube have not been reported, mainly because of the dependency of initial condition. According to past studies [5, 6], we usually expect another flow structure with two ascending and two descending streams which is referred to as S5 for Ra = 8.0 × 104 and Pr = 7.1. Actually, the author observes the S5, if we start our computation with such an initial condition as the conductive state or if we compute with very-slowly increasing/decreasing Ra from zero/infinity.

However, this initial-condition dependency does not necessarily mean weak stability of the trident convection. As an example for this, we can consider the time development of \( \overline{K} \) for Ra = 8.0 × 104 and Pr = 7.1 in a stationary cube under the gravity. we regard \( \overline{K} \) as a global indicator representing the systemʼs state. \( \overline{K} \) is defined by such a physical quantity as a spatially-averaged value of K over the whole volume of a cube. Then, as initial condition, we use the steady solution of S2 with a relatively-small \( \overline{K} = 20.2 \) for Ra = 1.0 × 104 and Pr = 7.1, instead of the S5 or instead of the conductive state. Such time development of \( \overline{K} \) show that the trident convection steadily and permanently appears at t > 0.06, after the initial transient process where another flow structure of one ascending and one descending streams (the S2) is dominant.

As another example to show the durability of the trident convection, we cosider the thermal convection in an oscillating cube instead of a stationary cubeunder the gravity or zero gravity. More specifically, the forced sinusoidal oscillationis parallel to the given temperature gradient direction. Figure 3a is a sample of the forcedly-oscillating cube under the gravity. Figure 3b is another sample of the forcedly-oscillating cube under zero gravity. Both the samples are rigorously periodic except for the first start-up period of computation at t ˂ 2π/ω, while we see high-frequency fluctuations due to higher harmonics. Then, we consider only the third period at t = 4π/ω − 6π/ω in each figure. There exist the time-intervals where \( \overline{K} \) becomes completely zero (or in the conductive state) each forcing cycle. Convective motion always starts with the S4 in each forcing cycle. We can confirm the appearance of the trident convection S9 at t = (4π + 1.01)/ω − (4π + 2.07)/ω in Fig. 3a and at t = (4π + 1.49)/ω − (4π + 2.95)/ω in Fig. 3b, together with other flow structures like the S2, the S4.

Fig. 3
figure 3

Time development of \( \overline{K} \), together with \( Ra \, + \, Ra_{\eta } \sin \omega t \) in figure (a) and \( Ra_{\eta } \sin \omega t \) in figure (b). In each figure, denotes \( \overline{K} \), and denotes \( Ra \, + \, Ra_{\eta } \sin \omega t \) or \( Ra_{\eta } \sin \omega t \) in figure (a) is at Ra = 1.0 × 104 and the S5. In both the samples, the duration times of the S9 are effectively long with enough large values of \( \overline{K} \)

Full size image

4 Conclusions

To summarise, we have reported a pair of trident currents on thermal convection in a cube. This previously-unidentified flow is rather robust, and can be observed not only in a stationary cube under the gravity, but also in an oscillating cube under the gravity and zero gravity. This finding implies a diversity of three-dimensional Rayleigh-Bénard convection, even if we suppose laminar and steady flow with very simple boundary condition.