Domino Effects and Industrial Risks: Integrated Probabilistic Framework – Case of Tsunamis Effects

Abstract

This paper presents an integrated probabilistic framework that deals with the industrial accidents and domino effects that may occur in an industrial plant. The particular case of tsunamis is detailed in the present paper: simplified models for the inundations depths and run-ups as well as their mechanical effects on industrial tanks.

The initial accident may be caused by severe service conditions in any of the tanks either under or at atmospheric pressure, or triggered by a natural hazard such as earthquake, tsunami or extreme floods for instance. This initial event generates, in general, a set of structural fragments, a fire ball, a blast wave as well as critical losses of containment (liquid and gas release and loss). The surrounding facilities may suffer serious damages and may also be a new source of accident and explosion generating afterwards a new sequence of structural fragments, fire ball, blast wave and confinement loss. The structural fragments, the blast wave form and the features of the fire ball can be described following database and feedback collected from past accidents.

The surrounding tanks might be under or at atmospheric pressure, and might be buried or not, or protected by physical barriers such as walls. The vulnerability of the potential targets should therefore be investigated in order to assess the risk of propagation of the accidents since cascading sequences of accidents, explosions and fires may take place within the industrial plant, giving rise to the domino effect that threatens any industrial plant.

The present research describes the risk of domino effect occurrence. The methodology is developed so that it can be operational and valid for any industrial site. It is supposed to be valid for a set of sizes, forms and kinds of tanks as well as a given geometric disposal on the industrial site. The interaction and the behavior of the targets affected or impacted by the first explosion effects should be described thanks to adequate simplified or sophisticated mechanical models: perforation and penetration of metal fragments when they impact surrounding tanks, as well as global failure such as overturning, buckling, excessive bending or shear effects, etc. The vulnerability analysis is detailed for the case of tanks under the mechanical effects generated by tsunamis.

15.1 Introduction

The important quantity of hazardous substances which are produced, confined or treated in industrial plants may generate, under given severe conditions, explosions, fires and fragmentations of the tanks where they are stored or of the pipelines in which they are transported. Due to internal or external causes, an initial sequence of a severe accident may be triggered and may propagate affecting the tanks, pipelines, power lines and facilities erected in their vicinity. This propagation may result in catastrophic consequences: structural damages as well as human losses or severe injuries.

Actually, the existing literature and reports on past accidents show that industrial accidents may generate at the same time blast waves, projection of structural fragments (in general, parts of exploded tanks), fireballs causing thermal radiation or thermal effects as well as loss of confinement with ejection of gases and liquids, that may be flammable or toxic. (Abbasi and Abbasi 2007; ARIA (website); Holden 1988; Lees 2005).

The analysis and modeling of the whole events and sub-sequent events and effects (mechanical, thermal, chemical, etc.) is a complex scientific and multidisciplinary challenge, (Abbasi and Abbasi 2007; Ali and Li 2008; Antonioni et al. 2009; Børvik et al. 2003; Corbet et al. 1995; Cozzani and Salzano 2004; Marhavilas et al. 2011; Mebarki et al. 2009a, b; Mingguang and Juncheng 2008; Neilson 1985; Ohte et al. 1982; Ruiz et al. 1989; Seveso Inspection Tool 2009; Talaslidis et al. 2004; TNO 2005a, b; Tsamopoulos 2004; UniversitÁ degli Studi di Torino; van den Berg 1985; Xie 2007):

• Triggering event: description of the triggering event (the first accident in any of the existing tanks within an industrial plant). The probability of occurrence as well as the thermodynamic and mechanical conditions for the first accident has to be well known and described. Past accidents and existing databases may also be helpful. The case of tsunamis generated by earthquakes is detailed in this paper.

• Subsequent effects and propagation: detailed description of the sub-events such as fragments generation and ejection, blast wave and the pressure front, fireballs and the thermal front and flows, and ejection of gases and liquids (loss of confinement/containment) requires mechanical modeling and also probabilistic distribution of the involved parameters (input and output).

• Interaction and effects on the surrounding tanks and facilities: simplified or sophisticated behavior of the affected targets and their response to the sub-events are required either by analytical, by testing or numerical approaches. For instance, partial penetration or perforation of the impacted tanks should be adequately evaluated as well as the local or global damages suffered by the target facilities (tanks, pipelines, etc.). The same requirements hold for the other sub-events such as blast waves and fireballs. The confinement losses may cause indirect effect such as fire ignitions, for instance.

• Successive sequences of accidents: the series of events and sub-events that take place within the industrial plant once a triggering event hits any of the existing tanks that are potential sources for domino effect initiation require time and dynamic analysis. Numerical analyses are helpful.

Once the domino effect is studied in deep details, its socio-economic consequences combined to the probability of its occurrence result in an expected cost of the domino effect. Investments and protective measures can then objectively be evaluated through an optimization of the global generalized cost. This concept is very helpful for the stakeholders in order to mitigate the potential disaster, and protect adequately the most sensitive and highly strategic installations.

15.2 Integrated Probabilistic Framework for Industrial Explosions and Domino Effects

15.2.1 General Plant: set of Tanks as Sources of Industrial Accidents and Sequence of Accidents

Let us consider any industrial site that may contain several tanks, either under pressure or at atmospheric pressure, see Fig. 15.1. Each of these tanks can be the source of accident or explosion due to either internal or external causes:

Fig. 15.1

First accident sequence within an industrial plant and its propagation

• Internal causes: critical corrosion, weakened welding, excessive cracking, over pressure or a critical temperature of the stored gas or liquid, handling accidental damage to critical, etc.

• External causes: malicious or malevolent acts, natural events such as strong earthquakes or tsunamis as well as extreme floods, explosions or impacts, fires and thermal effects, lightning, etc.

Therefore, each tank can be denoted as a Source “S” of industrial hazard as it may be damaged, may also explode and may generate threats (mechanical, chemical, thermal, etc.) to the surrounding facilities, and other tanks. Let us then denote these potential sources of industrial hazard as sources S(i) with i = 1 up to N_s, N_s being the number of tanks erected within the industrial site under study.

When an accident affects a given source S(i), such as an explosion or fire for instance, it can generate one or various events, i.e.:

• A set of structural fragments (plates, end-cups…): each generated fragment can be ejected from the tank source and become therefore a projectile,

• A fire ball and thermal effects,

• A blast wave, and

• A loss of confinement (containment: gas and liquid losses).

Let us denote E₁ ^g the event that corresponds to occurrence of any first accident (explosion for instance, impact, fire, etc.) that occurs within the entire industrial plant at a given starting instant t₀. Its probability of occurrence should be evaluated during any given reference period, T_ref, that may correspond for instance to the expected plant lifetime. This probability is defined during the entire reference period as:

$$ \mathrm{P}\left({\mathrm{E}}_1^{\mathrm{g}}\right)=\mathrm{P}\left({\left.{\mathrm{E}}_1^{\mathrm{g}}\right|}_{{\mathrm{T}}_{\mathrm{REF}}}\right){,\mathrm{during}\ \mathrm{the}\ \mathrm{reference}\ \mathrm{period}\ \mathrm{T}}_{\mathrm{REF}} $$

(15.1)

If this first accident gives rise to any of the subsequent events among fragments, fire ball, blast wave and confinement loss, one should calculate the risk of such propagation event E₁ ^propa since they may generate threats against the surrounding facilities (tanks, etc.). The probability of the propagation event is defined as:

$$ \mathrm{P}\left({\mathrm{E}}_1^{\mathrm{propa}}\right)=\mathrm{P}\left({\left.{\mathrm{E}}_1^{\mathrm{propa}}\right|}_{{\mathrm{E}}_1^{\mathrm{g}}}\right).\mathrm{P}\left({\mathrm{E}}_1^{\mathrm{g}}\right) $$

(15.2)

In fact, each explosion may also generate simultaneously these phenomena, i.e. fragments, blast wave, fire ball and confinement loss, see Fig. 15.2.

Fig. 15.2

BLEVE explosion generating projectiles, fire ball as well as blast wave

Each individual event effect or their combined effects may therefore damage the surrounding tanks (or facilities, buildings, etc.). The event E₁ ^damage that corresponds to damage caused to the surrounding tanks has its occurrence probability defined as:

$$ \mathrm{P}\left({\mathrm{E}}_1^{\mathrm{damage}}\right)=\mathrm{P}\left({\left.{\mathrm{E}}_1^{\mathrm{damage}}\right|}_{{\mathrm{E}}_1^{\mathrm{propa}}}\right)\mathrm{P}\left({\left.{\mathrm{E}}_1^{\mathrm{propa}}\right|}_{{\mathrm{E}}_1^{\mathrm{g}}}\right).\mathrm{P}\left({\mathrm{E}}_1^{\mathrm{g}}\right) $$

(15.3)

For the case where the tanks erected in the vicinity of the initial accidents are affected by the propagation event and are therefore suffering mechanical damages, they may then give rise to a new sequence and cascading accidents for instance. One should say that a new sequence E₂ ^g is taking place within the industrial plant, triggering the secondary sequence of explosions and accidents, leading then to the rise of the so-called “domino effect”. Its probability of occurrence is therefore estimated as, see Fig. 15.3:

Fig. 15.3

Successive sequences of accidents/explosions within an industrial plant and domino effect: case of fragments impact, for instance

$$ \mathrm{P}\left({\mathrm{E}}_2^{\mathrm{g}}\right)=\mathrm{P}\left({\left.{\mathrm{E}}_2^{\mathrm{g}}\right|}_{{\mathrm{E}}_1^{\mathrm{DAMAGE}}}\right).\mathrm{P}\left({\left.{\mathrm{E}}_1^{\mathrm{damage}}\right|}_{{\mathrm{E}}_1^{\mathrm{PROPA}}}\right).\mathrm{P}\left({\left.{\mathrm{E}}_1^{\mathrm{propa}}\right|}_{{\mathrm{E}}_1^{\mathrm{G}}}\right).\mathrm{P}\left({\mathrm{E}}_1^{\mathrm{g}}\right) $$

(15.4)

For the general purpose, one could say that any sequence E_n ^g, where n is the range of the accidents sequence (n ≥ 1), can give rise to an additional sequence (n + 1) with a probability defined as:

$$ \mathrm{P}\left({\mathrm{E}}_{\mathrm{n}+1}^{\mathrm{g}}\right)=\mathrm{P}\left({\left.{\mathrm{E}}_{\mathrm{n}+1}^{\mathrm{g}}\right|}_{{\mathrm{E}}_{\mathrm{n}}^{\mathrm{damage}}}\right).\mathrm{P}\left({\left.{\mathrm{E}}_{\mathrm{n}}^{\mathrm{damage}}\right|}_{{\mathrm{E}}_{\mathrm{n}}^{\mathrm{propa}}}\right).\mathrm{P}\left({\left.{\mathrm{E}}_{\mathrm{n}}^{\mathrm{propa}}\right|}_{{\mathrm{E}}_{\mathrm{n}}^{\mathrm{g}}}\right).\mathrm{P}\left({\mathrm{E}}_{\mathrm{n}}^{\mathrm{g}}\right) $$

(15.5)

15.2.2 First Sequence of Accidents

In order to develop a general framework and use general notations, let us therefore denote the accident at the source S(i) as the event E_s(i), see Figs. 15.1 and 15.4:

Fig. 15.4

Industrial plants: (a) Two main kinds of tanks; (b) Case of a Japanese refinery damaged by fires that occurred during the sequence earthquake-tsunami Tohuku, March 2011 (Author’s pictures)

$$ \begin{array}{l}{\mathrm{E}}_{\mathrm{s}}\left(\mathrm{i}\right)={\mathrm{E}}_{\mathrm{a}}\left(\mathrm{i}\right)\cup {\mathrm{E}}_{\mathrm{p}}\left(\mathrm{i}\right)\\ {}\kern2em =\left\{{}_{{\mathrm{E}}_{\mathrm{p}}\left(\mathrm{i}\right):\mathrm{if}\ \mathrm{S}\left(\mathrm{i}\right)=\mathrm{Tank}\ \mathrm{Under}\ \mathrm{Pressure}}^{{\mathrm{E}}_{\mathrm{a}}\left(\mathrm{i}\right):\mathrm{if}\ \mathrm{S}\left(\mathrm{i}\right)=\mathrm{Tank}\ \mathrm{at}\ \mathrm{Atmospheric}\ \mathrm{Pressure}}\right.\\ {}\kern-0.35em \mathrm{with}\ \mathrm{i}=1..{\mathrm{N}}_{\mathrm{s}}\end{array} $$

(15.6)

having a probability of occurrence defined as the scalar product:

$$ \mathrm{P}\left({\mathrm{E}}_{\mathrm{s}}\left(\mathrm{i}\right)\right)=\underset{\bar{\mkern6mu}}{1_{\mathrm{S}}}\left(\mathrm{i}\right).\underset{\bar{\mkern6mu}}{{\mathrm{P}}_{\mathrm{S}}}\left(\mathrm{i}\right) $$

(15.7)

Where: E_s(i) becomes either E_a(i) for an atmospheric tank or E_p(i) for an under pressure tank, if these two kinds are the only potential sources of accidents and explosions, with respective probabilities of occurrence denoted P(E_a(i)) and P(E_p(i));

∪ = events union symbol

$$ {1}_{\underset{\bar{\mkern6mu}}{\mathrm{S}}}(i)=\left({}_{1_{\mathrm{S}\mathrm{p}}}^{1_{\mathrm{S}\mathrm{a}}}\right):\mathrm{Identity}\ \mathrm{vector}\ \mathrm{of}\ \mathrm{the}\ \mathrm{tank} $$

(15.8)

$$ {1}_{\mathrm{Sa}}=\left\{{}_{0:\kern0.8em \boldsymbol{e}\mathrm{lse}}^{1:\kern0.8em \mathrm{if}\ \mathrm{S}\left(\mathrm{i}\right)=\mathrm{Tank}\ \mathrm{Atmospheric}\ \mathrm{Pressure}}\right. $$

(15.9)

$$ \mathrm{so}\ \mathrm{that}:{1}_{\underset{\bar{\mkern6mu}}{\mathrm{S}}}(i)={1}_{\underset{\bar{\mkern6mu}}{\mathrm{a}}}\mathrm{with}\ {1}_{\underset{\bar{\mkern6mu}}{\mathrm{a}}}\left({}_0^1\right) $$

(15.10)

and,

$$ {1}_{\mathrm{Sa}}=\left\{{}_{0:\kern0.8em \boldsymbol{e}\mathrm{lse}}^{1:\kern0.8em \mathrm{if}\ \mathrm{S}\left(\mathrm{i}\right)=\mathrm{Tank}\ \mathrm{under}\ \mathrm{Pressure}}\right. $$

(15.11)

$$ \mathrm{So}\ \mathrm{that}:{1}_{\underset{\bar{\mkern6mu}}{\mathrm{S}}}(i)={1}_{\underset{\bar{\mkern6mu}}{\mathrm{p}}}\mathrm{with}\ {1}_{\underset{\bar{\mkern6mu}}{\mathrm{p}}}=\left({}_1^0\right) $$

(15.12)

$$ \begin{array}{l}{\mathrm{P}}_{\underset{\bar{\mkern6mu}}{\mathrm{S}}}(i)=\left({}_{\mathrm{P}\left({\mathrm{E}}_{\mathrm{p}}\left(\mathrm{i}\right)\right)}^{\mathrm{P}\left({\mathrm{E}}_{\mathrm{a}}\left(\mathrm{i}\right)\right)}\right)\\ {}\kern3.5em :\mathrm{Probability}\ \mathrm{vector}\ \mathrm{for}\ \mathrm{the}\ \mathrm{source}\ \mathrm{tank}\ \mathrm{S}\left(\mathrm{i}\right)\ \mathrm{according}\ \mathrm{to}\ \mathrm{its}\ \mathrm{nature}\end{array} $$

(15.13)

$$ \mathrm{So}\ \mathrm{t}\mathrm{hat}:{\mathrm{P}}_{\underset{\bar{\mkern6mu}}{\mathrm{S}}}(i)=\left(\begin{array}{c}\hfill \mathrm{P}\left({\mathrm{E}}_{\mathrm{a}}\left(\mathrm{i}\right)\right)\hfill \\ {}\hfill 0\hfill \end{array}\right)\mathrm{for}\ \mathrm{a}\ \mathrm{t}\mathrm{a}\mathrm{nk}\ \mathrm{a}\mathrm{t}\ \mathrm{a}\mathrm{t}\mathrm{mospheric}\ \mathrm{pressure} $$

(15.14)

$$ \mathrm{and}\ {\mathrm{P}}_{\underset{\bar{\mkern6mu}}{\mathrm{S}}}(i)=\left(\begin{array}{c}\hfill 0\hfill \\ {}\hfill \mathrm{P}\left({\mathrm{E}}_{\mathrm{p}}\left(\mathrm{i}\right)\right)\hfill \end{array}\right)\mathrm{for}\ \mathrm{a}\ \mathrm{tank}\ \mathrm{under}\ \mathrm{pressure}. $$

(15.15)

Within the industrial plant, under the simplified hypothesis that only two different kinds of tanks are erected within the plant under study, the number of tanks is so that:

$$ {\mathrm{N}}_{\mathrm{S}}={\mathrm{N}}_{\mathrm{a}}+{\mathrm{N}}_{\mathrm{p}}=\mathrm{total}\ \mathrm{number}\ \mathrm{of}\ \mathrm{tanks},\mathrm{i}.\mathrm{e}.\mathrm{atmospheric}\ \mathrm{as}\ \mathrm{well}\ \mathrm{as}\ \mathrm{under}\ \mathrm{pressure}\ \mathrm{tanks} $$

(15.16)

$$ {\mathrm{N}}_{\mathrm{a}}={\displaystyle \sum_{\mathrm{i}=1}^{{\mathrm{N}}_{\mathrm{S}}}\underset{\bar{\mkern6mu}}{1_{\mathrm{S}}}\left(\mathrm{i}\right).\underset{\bar{\mkern6mu}}{1_{\mathrm{a}}}}:\mathrm{Total}\ \mathrm{number}\ \mathrm{of}\ \mathrm{tanks}\ \mathrm{at}\ \mathrm{at}\mathrm{mospheric}\ \mathrm{pressure} $$

(15.17)

$$ {\mathrm{N}}_{\mathrm{p}}={\displaystyle \sum_{\mathrm{i}=1}^{{\mathrm{N}}_{\mathrm{S}}}\underset{\bar{\mkern6mu}}{1_{\mathrm{S}}}}\left(\mathrm{i}\right).\underset{\bar{\mkern6mu}}{1_{\mathrm{p}}}:\mathrm{Total}\;\mathrm{number}\;\mathrm{of}\;\mathrm{under}\;\mathrm{pressure}\;\mathrm{tanks} $$

(15.18)

15.2.3 Occurrence Probability of the First Accident Within the Plant During a Reference Period, T _ref

The first sequence of accidents that might occur in a plant can be triggered by various causes. Each triggering cause might be described by theoretical considerations or on the basis of past accidents: probabilistic distributions, fuzzy sets, expert judgement, etc.

15.2.3.1 Hypothesis 1: Case of Homogeneous Sub-populations Within the Whole Population of Tanks

Let us consider the simplified case of an industrial plant where each category of tanks (atmospheric or under pressure in the present case) is considered as “homogeneous”, i.e. having same design, same construction period, same conditions of use and service, storing the same category of gas or liquids, getting in failure or explosive situation under the same conditions, etc. During a given period of existence, each category presents given ratios of severe accidents. From database of past accidents or through theoretical modelling and simulations, one may therefore consider an average annual rate of accident denoted:

$$ \lambda \left(\mathrm{i}\right)=\underset{\bar{\mkern6mu}}{1_{\mathrm{S}}}\left(\mathrm{i}\right).\underset{\bar{\mkern6mu}}{\lambda_S}(i)\ \mathrm{with}\ \underset{\bar{\mkern6mu}}{\lambda_{\mathrm{S}}}(i)=\left({}_{\lambda_{\mathrm{p}}\left(\mathrm{i}\right)}^{\lambda_{\mathrm{a}}\left(\mathrm{i}\right)}\right) $$

(15.19)

with: $\underset{\bar{\mkern6mu}}{\lambda_{\mathrm{S}}}(i)$ = vector of average annual accident ratios for the source tank S(i) according to its category, i = 1 up to N_s.

N ote : The case of malevolent acts requires specific analysis in order to evaluate or predict the annual ratios and the risk of occurrence. In the following parts of the paper, we do not consider this particular case although its consequences could be catastrophic with devastating effects.

Furthermore, the probability of failure of an elementary event becomes in this context:

$$ \underset{\bar{\mkern6mu}}{{\mathrm{P}}_{\mathrm{S}}}(i)=\left({}_0^{{\mathrm{P}}_{\mathrm{a}}}\right)\mathrm{assuming}\ \mathrm{P}\left({\mathrm{E}}_{\mathrm{a}}\left(\mathrm{i}\right)\right)={\mathrm{P}}_{\mathrm{a}},\forall \mathrm{i}=1..{\mathrm{k}}_{\mathrm{a}},\mathrm{for}\ \mathrm{each}\ \mathrm{of}\ \mathrm{the}\ \mathrm{atmospheric}\ \mathrm{tanks} $$

(15.20)

$$ \underset{\bar{\mkern6mu}}{{\mathrm{P}}_{\mathrm{S}}}(i)=\left({}_{{\mathrm{P}}_{\mathrm{p}}}^0\right)\mathrm{assuming}\ \mathrm{P}\left({\mathrm{E}}_{\mathrm{p}}\left(\mathrm{i}\right)\right)={\mathrm{P}}_{\mathrm{p}},\forall \mathrm{i}=1..{\mathrm{k}}_{\mathrm{p}},\mathrm{for}\ \mathrm{each}\ \mathrm{of}\ \mathrm{the}\ \mathrm{under}\ \mathrm{pressure}\ \mathrm{tanks} $$

(15.21)

15.2.3.2 Hypothesis 2: Particular Case of First Sequence of Accidents

Let us consider the general case of a first accident concerning simultaneously a total number k of tanks:

$$ {\mathrm{k}=\mathrm{k}}_{\mathrm{a}}{+\mathrm{k}}_{\mathrm{p}};\kern0.5em {\mathrm{k}}_{\mathrm{a}}\in \left[1;{\mathrm{N}}_{\mathrm{a}}\right];\kern0.5em {\mathrm{k}}_{\mathrm{p}}\in \left[{1;\mathrm{N}}_{\mathrm{p}}\right] $$

(15.22)

Where: k_a, k_p = total number of atmospheric and under pressure tanks, respectively.

The probability of this event can then be derived from the Binomial distribution. Under the hypothesis of “homogeneous” sub-categories, i.e. atmospheric or under pressure tanks, and probabilistic independency between two distinct individual events, this probability of occurrence is then:

$$ \begin{array}{l}\mathrm{P}\left({\mathrm{E}}_{\mathrm{a}}\left(\mathrm{i}=1..{\mathrm{k}}_{\mathrm{a}}\right)\cap {\mathrm{E}}_{\mathrm{p}}\left(\mathrm{j}=1..{\mathrm{k}}_{\mathrm{p}}\right)\right)=\mathrm{P}\left(\left({\displaystyle \underset{\mathrm{i}=1}{\overset{{\mathrm{k}}_{\mathrm{a}}}{\cap }}{\mathrm{E}}_{\mathrm{a}}}\left(\mathrm{i}\right)\right)\cap \left({\displaystyle \underset{\mathrm{j}=1}{\overset{{\mathrm{k}}_{\mathrm{p}}}{\cap }}{\mathrm{E}}_{\mathrm{p}}}\left(\mathrm{j}\right)\right)\right)\kern1em \\ {}\kern1em =\left[\left(\begin{array}{c}\hfill {\mathrm{N}}_{\mathrm{a}}\hfill \\ {}\hfill {\mathrm{k}}_{\mathrm{a}}\hfill \end{array}\right).{\left({\mathrm{P}}_{\mathrm{a}}\right)}^{{\mathrm{k}}_{\mathrm{a}}}.{\left(1-{\mathrm{P}}_{\mathrm{a}}\right)}^{{\mathrm{N}}_{\mathrm{a}}-{\mathrm{k}}_{\mathrm{a}}}\right].\left[\left(\begin{array}{c}\hfill {\mathrm{N}}_{\mathrm{p}}\hfill \\ {}\hfill {\mathrm{k}}_{\mathrm{p}}\hfill \end{array}\right).{\left({\mathrm{P}}_{\mathrm{p}}\right)}^{{\mathrm{k}}_{\mathrm{p}}}.{\left(1-{\mathrm{P}}_{\mathrm{p}}\Big)\right)}^{{\mathrm{N}}_{\mathrm{p}}-{\mathrm{k}}_{\mathrm{p}}}\right]\end{array} $$

(15.23)

Where the binomial coefficients are:

$$ \left(\begin{array}{c}\hfill {\mathrm{N}}_{\mathrm{a}}\hfill \\ {}\hfill {\mathrm{k}}_{\mathrm{a}}\hfill \end{array}\right)={C}_{{\mathrm{N}}_{\mathrm{a}}}^{{\mathrm{k}}_{\mathrm{a}}}=\frac{{\mathrm{N}}_{\mathrm{a}}!}{\left({\mathrm{k}}_{\mathrm{a}}!\right).\left({\mathrm{N}}_{\mathrm{a}}-{\mathrm{k}}_{\mathrm{a}}\right)!} $$

(15.24)

and

$$ \left(\begin{array}{c}\hfill {\mathrm{N}}_{\mathrm{p}}\hfill \\ {}\hfill {\mathrm{k}}_{\mathrm{p}}\hfill \end{array}\right)={C}_{{\mathrm{N}}_{\mathrm{p}}}^{{\mathrm{k}}_{\mathrm{p}}}=\frac{{\mathrm{N}}_{\mathrm{p}}!}{\left({\mathrm{k}}_{\mathrm{p}}!\right).\left({\mathrm{N}}_{\mathrm{p}}-{\mathrm{k}}_{\mathrm{p}}\right)!} $$

(15.25)

15.2.3.2.1 Particular Case: Only One Accident Occurs Once at a Given Time t₁

The particular case is the event, denoted E₁, which corresponds to one accident taking place once in either an under pressure or an atmospheric tank. As the simultaneous accident of the two different tank categories is supposed to present a null probability of occurrence, this event E ^g₁ global to the entire industrial plant, has a probability of occurrence:

$$ \begin{array}{c}\mathrm{P}\left({\mathrm{E}}_1^{\mathrm{g}}\right)=\left[\left(\begin{array}{c}\hfill {\mathrm{N}}_{\mathrm{a}}\hfill \\ {}\hfill 1\hfill \end{array}\right).{\left({\mathrm{P}}_{\mathrm{a}}\right)}^1.{\left(1-{\mathrm{P}}_{\mathrm{a}}\right)}^{{\mathrm{N}}_{\mathrm{a}}-1}\right]+\left[\left(\begin{array}{c}\hfill {\mathrm{N}}_{\mathrm{p}}\hfill \\ {}\hfill 1\hfill \end{array}\right).{\left({\mathrm{P}}_{\mathrm{p}}\right)}^1.{\left(1-{\mathrm{P}}_{\mathrm{p}}\Big)\right)}^{{\mathrm{N}}_{\mathrm{p}}-1}\right]\\ {}\kern-1.31em =\left[{\mathrm{N}}_{\mathrm{a}}.{\left({\mathrm{P}}_{\mathrm{a}}\right)}^1.{\left(1-{\mathrm{P}}_{\mathrm{a}}\right)}^{{\mathrm{N}}_{\mathrm{a}}-1}\right]+\left[{\mathrm{N}}_{\mathrm{p}}.{\left({\mathrm{P}}_{\mathrm{p}}\right)}^1.{\left(1-{\mathrm{P}}_{\mathrm{p}}\Big)\right)}^{{\mathrm{N}}_{\mathrm{p}}-1}\right]\end{array} $$

(15.26)

15.2.3.2.2 Number of Accidents that Affect One Tank: During a Reference Period T_ref

As the explosions are rare events, we can assume that the number N_e(i) of explosions of a given tank S(i), i = 1 up to N_s, follows adequately a Poisson distribution with an average annual rate λ(i) evaluated from collected database on industrial accidents. Therefore, the probability of having N_e(i) of explosions affecting the tank under study during the reference period T_REF (expected lifetime of the industrial plant, for instance) becomes, (Mebarki et al. 2008a):

$$ \mathrm{P}\left({\mathrm{E}}_{\mathrm{s}}\left(\mathrm{i}\right)\right)=\frac{{\left(\lambda (i){.\mathrm{T}}_{\mathrm{REF}}\right)}^{{\mathrm{N}}_{\mathrm{e}}}{.\mathrm{e}}^{-\left(\lambda (i){.\mathrm{T}}_{\mathrm{REF}}\right)}}{{\mathrm{N}}_{\mathrm{e}}!} $$

(15.27)

leading then, respectively to P(E_a) and P(E_p) for the two kinds of tanks erected within the concerned plant:

$$ {\mathrm{P}}_{\mathrm{a}}={\mathrm{P}\Big(\mathrm{E}}_{\mathrm{a}}\Big)=\frac{{\left({\uplambda}_{\mathrm{a}}{.\mathrm{T}}_{\mathrm{REF}}\right)}^{{\mathrm{N}}_{\mathrm{e}}}{.\mathrm{e}}^{-\left({\uplambda}_{\mathrm{a}}{.\mathrm{T}}_{\mathrm{REF}}\right)}}{{\mathrm{N}}_{\mathrm{e}}!} $$

(15.28)

and

$$ {\mathrm{P}}_{\mathrm{p}}={\mathrm{P}\Big(\mathrm{E}}_{\mathrm{p}}\Big)=\frac{{\left({\uplambda}_{\mathrm{p}}{.\mathrm{T}}_{\mathrm{REF}}\right)}^{{\mathrm{N}}_{\mathrm{e}}}{.\mathrm{e}}^{-\left({\uplambda}_{\mathrm{p}}{.\mathrm{T}}_{\mathrm{REF}}\right)}}{{\mathrm{N}}_{\mathrm{e}}!} $$

(15.29)

Where: T_ref = Reference period time [unit: in years, as λ is the average annual ratio].

15.2.3.2.3 Particular Case: k = 1

Let us consider the case of an initial accident E₁(i), occurring at the source S(i) only once during the reference period. The corresponding probabilities of occurrence becomes for each category of tanks:

$$ \mathrm{P}\left({\left.{\mathrm{E}}_1\left(\mathrm{i}\right)\right|}_{{\mathrm{T}}_{\mathrm{REF}}}\right)=\mathrm{P}\left({\left.{\mathrm{E}}_{\mathrm{s}}\left(\mathrm{i}\right)\right|}_{{\mathrm{T}}_{\mathrm{REF}}}\right)=\left(\lambda \left(\mathrm{i}\right).{\mathrm{T}}_{\mathrm{REF}}\right).{\mathrm{e}}^{-\left(\lambda \left(\mathrm{i}\right).{\mathrm{T}}_{\mathrm{REF}}\right)} $$

(15.30)

as N_e = 1, leading then to:

$$ {\mathrm{P}}_{\mathrm{a}}=\left({\lambda}_{\mathrm{a}}.{\mathrm{T}}_{\mathrm{REF}}\right).{\mathrm{e}}^{-\left({\lambda}_{\mathrm{a}}.{\mathrm{T}}_{\mathrm{REF}}\right)}{\mathrm{a}\mathrm{nd}\ \mathrm{P}}_{\mathrm{p}}=\left({\lambda}_{\mathrm{p}}.{\mathrm{T}}_{\mathrm{REF}}\right) $$

(15.31)

since: k_a = 1 or k_p = 1.

15.2.3.2.4 Synthesis

The event corresponding to any first accident within the entire plant during the whole reference period E ^g₁ is also defined as:

$$ {\left.{\mathrm{E}}_1^{\mathrm{g}}\right|}_{{\mathrm{T}}_{{}_{\mathrm{ref}}}}={\displaystyle \underset{\mathrm{i}=1}{\overset{{\mathrm{N}}_{\mathrm{s}}}{\cup }}\left({\left.{\mathrm{E}}_1\left(\mathrm{i}\right)\right|}_{{\mathrm{T}}_{{}_{\mathrm{ref}}}}\right)} $$

(15.32)

From the above developments, the probability of occurrence of only one accident, once within the industrial plant, during the entire reference period is therefore explicitly written as:

$$ \begin{array}{c}\mathrm{P}\left({\left.{\mathrm{E}}_1^{\mathrm{g}}\right|}_{{\mathrm{T}}_{\mathrm{ref}}}\right)=\left[{\mathrm{N}}_{\mathrm{a}}.\left(\left({\lambda}_{\mathrm{a}}.{\mathrm{T}}_{\mathrm{REF}}\right).{\mathrm{e}}^{-\left({\lambda}_{\mathrm{a}}.{\mathrm{T}}_{\mathrm{REF}}\right)}\right).{\left(1-\left({\lambda}_{\mathrm{a}}.{\mathrm{T}}_{\mathrm{REF}}\right).{\mathrm{e}}^{-\left({\lambda}_{\mathrm{a}}.{\mathrm{T}}_{\mathrm{REF}}\right)}\right)}^{{\mathrm{N}}_{\mathrm{a}}-1}\right]\\ {}\kern1.5em +\left[{\mathrm{N}}_{\mathrm{p}}.\left(\left({\uplambda}_{\mathrm{p}}{.\mathrm{T}}_{\mathrm{REF}}\right){.\mathrm{e}}^{-\left({\uplambda}_{\mathrm{p}}{.\mathrm{T}}_{\mathrm{REF}}\right)}\right).{\left(1-\left({\uplambda}_{\mathrm{p}}{.\mathrm{T}}_{\mathrm{REF}}\right){.\mathrm{e}}^{-\left({\uplambda}_{\mathrm{p}}{.\mathrm{T}}_{\mathrm{REF}}\right)}\right)}^{{\mathrm{N}}_{\mathrm{p}}-1}\right]\end{array} $$

(15.33)

15.2.4 Consequences of the First Accident Triggered Within the Plant During a Reference Period, T _ref

15.2.4.1 Subsequent Events and Threats Generated by a Given Accident

When an accident is triggered (such as BLEVE effect, for instance), a sequence of events might take place as explained above, see Figs. 15.1, 15.2, 15.3, 15.4 and 15.5:

Fig. 15.5

BLEVE explosion generating projectiles, fire ball as well as blast wave: (a) Fragments generation and projectile impacts; (b) Blast wave and pressure/depression front

• A set of structural fragments (plates, end-cups…): event E_F(i) since each generated fragment can be ejected from the tank source and become therefore a projectile.

• A fire ball and thermal effects: event E_T(i)

• A blast wave: event E_W(i)

• A release or loss of liquid and gas (loss of confinement): event E_C(i).

These events may generate mechanical, thermo-mechanical or chemical effects that can affect the surrounding facilities or installations, and even persons on charge of the plant control and security. Our present purpose is to analyse the unfavourable effect on the tanks erected in the vicinity and that might be severely damaged so that they might give rise to a new sequence of accidents and explosions. These successive sequences of damages and explosions, and their subsequent events and consequences, are important steps when dealing with the so-called domino effect.

Therefore, let us consider the case of the first accident triggering at instant t₁ within the industrial plant under study:

$$ {\left.{\mathrm{E}}_1^{\mathrm{g}}\right|}_{{\mathrm{T}}_{\mathrm{ref}}}={\displaystyle \underset{\mathrm{i}=1}{\overset{{\mathrm{N}}_{\mathrm{s}}}{\cup }}\left({\left.{\mathrm{E}}_1\left(\mathrm{i}\right)\right|}_{{\mathrm{T}}_{{}_{\mathrm{ref}}}}\right),\kern0.5em \mathrm{i}.\mathrm{e}.{\mathrm{E}}_{\mathrm{s}}\left(\mathrm{i}\right)={\mathrm{E}}_1\left(\mathrm{i}\right){\ \mathrm{t}\mathrm{riggered}\ \mathrm{at}\ \mathrm{an}\ \mathrm{i}\mathrm{nitial}\ \mathrm{t}\mathrm{i}\mathrm{me}\ \mathrm{t}}_1\left(\mathrm{i}\right)} $$

(15.34)

Let us consider a global axial system as a reference system for the whole plant. The various sources are erected in their respective locations defined by the position vector x _s (.), see Figs. 15.5 and 15.6:

Fig. 15.6

Fragments ejected as projectiles that may impact surrounding target tanks

$$ \underset{\bar{\mkern6mu}}{{\mathrm{x}}_{\mathrm{s}}}\left(\mathrm{i}\right)=\kern0.5em \left(\begin{array}{c}\hfill {\mathrm{x}}_{\mathrm{s}}\left(\mathrm{i}\right)\hfill \\ {}\hfill {\mathrm{y}}_{\mathrm{s}}\left(\mathrm{i}\right)\hfill \\ {}\hfill {\mathrm{z}}_{\mathrm{s}}\left(\mathrm{i}\right)\hfill \end{array}\right),\mathrm{i}={1\ \mathrm{up}\ \mathrm{to}\ \mathrm{N}}_{\mathrm{s}} $$

(15.35)

The elementary threat events propagating from the source accident S(i) may affect and impact the surrounding tanks that becomes, once the initial sequence triggered at time t₁(i), as potential targets T(j), j = 1 up to N_s. The various tanks and targets erected within the considered plant, are placed in the location described by x _t (.), see Figs. 15.5 and 15.6:

$$ \underset{\bar{\mkern6mu}}{{\mathrm{x}}_{\mathrm{t}}}\left(\mathrm{j}\right)=\kern0.5em \left(\begin{array}{c}\hfill {\mathrm{x}}_{\mathrm{t}}\left(\mathrm{j}\right)\hfill \\ {}\hfill {\mathrm{y}}_{\mathrm{t}}\left(\mathrm{j}\right)\hfill \\ {}\hfill {\mathrm{z}}_{\mathrm{t}}\left(\mathrm{j}\right)\hfill \end{array}\right),\mathrm{j}={1\ \mathrm{up}\ \mathrm{to}\ \mathrm{N}}_{\mathrm{s}} $$

(15.36)

In fact, all the tanks can be considered as potential source of accident S(i) as well as potential targets T(i) under the effects of the threats generated at the source, i.e.:

$$ \underset{\bar{\mkern6mu}}{{\mathrm{x}}_{\mathrm{s}}}\left(\mathrm{i}\right)=\underset{\bar{\mkern6mu}}{{\mathrm{x}}_{\mathrm{t}}}\left(\mathrm{i}\right),\mathrm{j}={1\ \mathrm{up}\ \mathrm{to}\ \mathrm{N}}_{\mathrm{s}} $$

(15.37)

15.2.4.2 Propagation of the First Accident and Threats to the Surrounding Tanks and Facilities

This generation of events that may threaten the neighbourhood corresponds to the propagation event, denoted E_propa(i):

$$ {\mathrm{E}}_1^{\mathrm{propa}}\left(\mathrm{i}\right)\left|{}_{{\mathrm{E}}_1\left(\mathrm{i}\right)}\right.={\mathrm{E}}_{\mathrm{F}}\left(\mathrm{i}\right){\displaystyle \cup {\mathrm{E}}_{\mathrm{T}}\left(\mathrm{i}\right){\displaystyle \cup {\mathrm{E}}_{\mathrm{W}}\left(\mathrm{i}\right)}}{\displaystyle \cup {\mathrm{E}}_{\mathrm{C}}\left(\mathrm{i}\right)} $$

(15.38)

Therefore, the apparition of first threats to the surrounding tanks and facilities results from the first accident and propagation events:

$$ {\mathrm{E}}_1\left(\mathrm{i}\right){\displaystyle \cap \left({\mathrm{E}}_1^{\mathrm{propa}}\left(\mathrm{i}\right)\left|{}_{{\mathrm{E}}_1\left(\mathrm{i}\right)}\right.\right)}=\kern0.5em \left({\mathrm{E}}_1\left(\mathrm{i}\right)\right){\displaystyle \cap \left({\mathrm{E}}_{\mathrm{F}}\left(\mathrm{i}\right){\displaystyle \cup {\mathrm{E}}_{\mathrm{T}}\left(\mathrm{i}\right){\displaystyle \cup {\mathrm{E}}_{\mathrm{W}}\left(\mathrm{i}\right)}}{\displaystyle \cup {\mathrm{E}}_{\mathrm{C}}\left(\mathrm{i}\right)}\right)} $$

(15.39)

The corresponding probability of propagation event is then defined as:

$$ \mathrm{P}\left(\left({\mathrm{E}}_1^{\mathrm{propa}}\left(\mathrm{i}\right)\right)\right)={\displaystyle \sum_{\mathrm{i}=1}^{{\mathrm{N}}_{\mathrm{s}}}{\mathrm{P}\Big(\mathrm{E}}_1^{\mathrm{propa}}\left(\mathrm{i}\right)\left|{}_{{\mathrm{E}}_1\left(\mathrm{i}\right)}\right.\Big)}{.\mathrm{P}\Big(\mathrm{E}}_1\left(\mathrm{i}\right)\Big) $$

(15.40)

15.2.4.2.1 Propagation of the Accident: Ejected Structural Fragments as Projectiles

The first accident at the source tank S(i), i = 1 up to N_s, occurring at the starting time t₁(i) may give rise to a set of N_f(i) structural fragments (denoted F(i,k), k = 1 up to N_f(i)) that are ejected from this source and may impact other tanks or facilities that cross their trajectory, i.e.:

$$ {\mathrm{E}}_{\mathrm{F}}\left(\mathrm{i}\right)={\displaystyle \underset{\mathrm{k}=1}{\overset{{\mathrm{N}}_{\mathrm{f}}\left(\mathrm{i}\right)}{\cup }}{\mathrm{E}}_{\mathrm{f}\mathrm{rag}}\left(\mathrm{i},\mathrm{k}\right)} $$

(15.41)

Its occurrence probability can be estimated according to collected database inputs or from theoretical and simulation approaches that provide therefore:

$$ {\mathrm{P}}_{\mathrm{F}}\left(\mathrm{i}\right)=\mathrm{P}\left({\mathrm{E}}_{\mathrm{F}}\left(\mathrm{i}\right)\left|{}_{{\mathrm{E}}_1\left(\mathrm{i}\right)}\right.\right).\mathrm{P}\left({\mathrm{E}}_1\left(\mathrm{i}\right)\right) $$

(15.42)

From database analysis of past accidents and existing bibliography reports as well as theoretical approaches, one can establish the probabilistic distributions concerning the main governing parameters of the fragments motion and energy, i.e. (Mebarki et al. 2007; 2008a, b, 2009a, b):

• The number of fragments: N_f(i)

• The ratio α(i) of total internal energy E_internal(i) within the source tank that is transformed into kinetic energy ejecting the fragments and transferred to them during their motion around the source: E_kin(i)

  $$ {\mathrm{E}}_{\mathrm{kin}}\left(\mathrm{i}\right)=\upalpha {.\mathrm{E}}_{\mathrm{internal}}\left(\mathrm{i}\right) $$

  (15.43)

• The form (among plate, end-cup, oblong end-cup, etc.) and the dimensions of each fragment

• The mass of each fragment F(i,k): m_p(i,j) with i = 1 up to Ns and k = 1 up to N_f(i)

• The initial velocity: v_p(i,k) at instant t₁(i)

  $$ \underset{\bar{\mkern6mu}}{{\mathrm{v}}_{\mathrm{p}}}\left(\mathrm{i},\mathrm{k}\right)={\dot{\underset{\bar{\mkern6mu}}{\mathrm{x}}}}_{\mathrm{p}}\left(\mathrm{i},\mathrm{k}\right)={\left.\left(\begin{array}{c}\hfill {\mathrm{v}}_{\mathrm{x}}\left(\mathrm{i},\mathrm{k}\right)\hfill \\ {}\hfill {\mathrm{v}}_{\mathrm{y}}\left(\mathrm{i},\mathrm{k}\right)\hfill \\ {}\hfill {\mathrm{v}}_{\mathrm{z}}\left(\mathrm{i},\mathrm{k}\right)\hfill \end{array}\right)\right|}_{\mathrm{t}={\mathrm{t}}_1\left(\mathrm{i}\right)} $$

  (15.44)

• The initial angles of ejection: event θ(i,k)

  $$ \underset{\bar{\mkern6mu}}{\uptheta_{\mathrm{p}}}\left(\mathrm{i},\mathrm{k}\right)={\left.\left(\begin{array}{c}\hfill {\uptheta}_{\mathrm{x}}\left(\mathrm{i},\mathrm{k}\right)\hfill \\ {}\hfill {\uptheta}_{\mathrm{y}}\left(\mathrm{i},\mathrm{k}\right)\hfill \\ {}\hfill {\uptheta}_{\mathrm{z}}\left(\mathrm{i},\mathrm{k}\right)\hfill \end{array}\right)\right|}_{\mathrm{t}={\mathrm{t}}_1\left(\mathrm{i}\right)} $$

  (15.45)

At any instant t ≥ t₁(i), the motion of each fragment can be studied according to adopted values for the drag and lift coefficients, i.e. the position x _p (.), velocity v _p (.) and acceleration g _p (.):

$$ {\underset{\bar{\mkern6mu}}{\mathrm{x}}}_{\mathrm{p}}\left(\mathrm{i},\mathrm{k}\right)={\left.\left(\begin{array}{c}\hfill {\mathrm{x}}_{\mathrm{p}}\left(\mathrm{i},\mathrm{k}\right)\hfill \\ {}\hfill {\mathrm{y}}_{\mathrm{p}}\left(\mathrm{i},\mathrm{k}\right)\hfill \\ {}\hfill {\mathrm{z}}_{\mathrm{p}}\left(\mathrm{i},\mathrm{k}\right)\hfill \end{array}\right)\right|}_{\mathrm{t}\ge {\mathrm{t}}_1\left(\mathrm{i}\right);}\underset{\bar{\mkern6mu}}{{\mathrm{v}}_{\mathrm{p}}}\left(\mathrm{i},\mathrm{k}\right)={\left.\left(\begin{array}{c}\hfill {\dot{\mathrm{x}}}_{\mathrm{p}}\left(\mathrm{i},\mathrm{k}\right)\hfill \\ {}\hfill {\dot{\mathrm{y}}}_{\mathrm{p}}\left(\mathrm{i},\mathrm{k}\right)\hfill \\ {}\hfill {\dot{\mathrm{z}}}_{\mathrm{p}}\left(\mathrm{i},\mathrm{k}\right)\hfill \end{array}\right)\right|}_{\mathrm{t}\ge {\mathrm{t}}_1\left(\mathrm{i}\right);}\underset{\bar{\mkern6mu}}{\gamma_{\mathrm{p}}}\left(\mathrm{i},\mathrm{k}\right)={\left.\left(\begin{array}{c}\hfill {\ddot{\mathrm{x}}}_{\mathrm{p}}\left(\mathrm{i},\mathrm{k}\right)\hfill \\ {}\hfill {\ddot{\mathrm{y}}}_{\mathrm{p}}\left(\mathrm{i},\mathrm{k}\right)\hfill \\ {}\hfill {\ddot{\mathrm{z}}}_{\mathrm{p}}\left(\mathrm{i},\mathrm{k}\right)\hfill \end{array}\right)\right|}_{\mathrm{t}\ge {\mathrm{t}}_1\left(\mathrm{i}\right)} $$

(15.46)

On the basis of its motion and the relative location of the whole other potential targets, i.e. the tanks T(j), j = 1 up to N_s, one can define the possible impacts on the tank T(j) by the impact indicator:

$$ \underset{\bar{\mkern6mu}}{1_{\mathrm{p}}}\left(\mathrm{i},\mathrm{k},\mathrm{j}\right)=\left\{\begin{array}{c}\hfill 1:\mathrm{if}\ \mathrm{fragment}\ \mathrm{F}\left(\mathrm{i},\mathrm{k}\right)\ \mathrm{impacts}\ \mathrm{t}\mathrm{arget}\ \mathrm{T}\left(\mathrm{j}\right){\ \mathrm{at}\ \mathrm{instant}\ \mathrm{t}}_{\mathrm{impact}}\left(\mathrm{i},\mathrm{k},\mathrm{j}\right)\hfill \\ {}\hfill 0:\mathrm{else}\hfill \end{array}\right. $$

(15.47)

The tank T(j) is impacted by at least one or a rain of the fragments ejected from source S(i) if the impact indicator is equal to 1, i.e.:

$$ {\underset{\bar{\mkern6mu}}{1}}_{\mathrm{impact}}\left(\mathrm{i},\mathrm{k},\mathrm{j}\right)=\mathrm{Min}\left\{\begin{array}{c}\hfill 1\hfill \\ {}\hfill {\displaystyle \sum_{\mathrm{k}=1}^{{\mathrm{N}}_{\mathrm{f}}\left(\mathrm{i}\right)}\underset{\bar{\mkern6mu}}{1_{\mathrm{p}}}\left(\mathrm{i},\mathrm{k},\mathrm{j}\right)}\hfill \end{array}\right\} $$

(15.48)

The impact event on the tank T(j) under the set of projectiles due to source S(i) is then:

$$ {\mathrm{E}}_{\mathrm{F}}^{\mathrm{impact}}\left(\mathrm{j}\right)={\displaystyle \underset{\mathrm{k}=1}{\overset{{\mathrm{N}}_{\mathrm{f}}\left(\mathrm{i}\right)}{\cup }}\left({\mathrm{E}}_{\mathrm{F}}^{\mathrm{impact}}\left(\mathrm{i},\mathrm{k},\mathrm{j}\right)\cap {\mathrm{E}}_{\mathrm{f}\mathrm{rag}}\left(\mathrm{i},\mathrm{k}\right)\right)} $$

(15.49)

Where:

• Event corresponding to tank T_j impacted by the fragment F(i,k)= E ^impact_F (i,k,j)

• Event corresponding to existence of a fragment F(i,k)= E_frag(i,k)

When there is an impact, the mechanical models that are adopted should provide the damage generated to the impacted tank. A set of mechanical limit state functions tells whether the impacted tank T(j) reaches its defined :

• Limit state of local damages: perforation, partial penetration, cracks, excessive stress or strains, etc.

• Limit state of global damages: over-lapping, anchors rupture, sliding, buckling, etc.

• The corresponding probability of damage can be obtained by analytical or numeric approaches. Due to the complexity of the analysis, Monte Carlo simulations are the common tool used in order to estimate this value:

  $$ \mathrm{P}\left({\mathrm{E}}_{\mathrm{F}}^{\mathrm{damage}}\left(\mathrm{j}\right)\right)={\displaystyle \sum_{\mathrm{k}=1}^{{\mathrm{N}}_{\mathrm{f}}\left(\mathrm{i}\right)}\mathrm{P}\left({\mathrm{E}}_{\mathrm{F}}^{\mathrm{damage}}\left.\left(\mathrm{j}\right)\right)\left|{}_{{\mathrm{E}}_{\mathrm{F}}^{\mathrm{impact}}\left(\mathrm{j}\right)}\right.\right).\mathrm{P}\left({\mathrm{E}}_{\mathrm{F}}^{\mathrm{impact}}\left(\mathrm{j}\right)\left|{}_{{\mathrm{E}}_{\mathrm{f}\mathrm{rag}}\left(\mathrm{i},\mathrm{k}\right)}\right.\right).\mathrm{P}\left({\mathrm{E}}_{\mathrm{f}\mathrm{rag}}\left(\mathrm{i},\mathrm{k}\right)\right)} $$

  (15.50)

15.2.4.2.2 Propagation of the Accident: Fire Ball Generates Thermal Effects

The first accident at the source tank S(i), i = 1 up to N_s, may generate thermal field that may affect significantly other tanks or facilities erected in the source vicinity, see Fig. 15.7. This event and its probability of occurrence are respectively defined as:

Fig. 15.7

Fires and threats to surrounding facilities: Case of a Japanese refinery damaged by fires that occurred during the sequence earthquake-tsunami Tohuku, March 2011 (Author’s pictures)

$$ {\mathrm{E}}_{\mathrm{T}}\left(\mathrm{i}\right)\kern0.15em {\mathrm{with}\ \mathrm{P}}_{\mathrm{T}}\left(\mathrm{i}\right)=\mathrm{P}\left({\left.{\mathrm{E}}_{\mathrm{T}}\left(\mathrm{i}\right)\right|}_{{\mathrm{E}}_1\left(\mathrm{i}\right)}\right).\mathrm{P}\left({\mathrm{E}}_1\left(\mathrm{i}\right)\right) $$

(15.51)

This occurrence probability can be estimated according to collected database inputs or from theoretical and simulation approaches. The event generates thermal fields and flows than can be described by:

$$ \mathrm{T}\left(\mathrm{i},\underset{\bar{\mkern6mu}}{{\mathrm{x}}_{\mathrm{s}}}\left(\mathrm{i}\right),\underset{\bar{\mkern6mu}}{{\mathrm{x}}_{\mathrm{t}}}\left(\mathrm{i}\right),\mathrm{t}-{\mathrm{t}}_1\left(\mathrm{i}\right)\right)=\mathrm{thermal}\ \mathrm{field} $$

(15.52)

$$ {\varPhi}_{\mathrm{T}}\left(\mathrm{i},\underset{\bar{\mkern6mu}}{{\mathrm{x}}_{\mathrm{s}}}\left(\mathrm{i}\right),\underset{\bar{\mkern6mu}}{{\mathrm{x}}_{\mathrm{t}}}\left(\mathrm{i}\right),\mathrm{t}-{\mathrm{t}}_1\left(\mathrm{i}\right)\right)=\mathrm{thermal}\ \mathrm{flow} $$

(15.53)

The effect of the thermal event on the tank T(j) due to source S(i) can produce direct as well as indirect (such as ignition of surrounding gases or liquids and fire generation) global or local damages. The corresponding probability of damage can be obtained by analytical or numeric approaches. Due to the complexity of the analysis, Monte Carlo simulations are the common tool used in order to estimate this value:

$$ \mathrm{P}\left({\mathrm{E}}_{\mathrm{T}}^{\mathrm{damage}}\left(\mathrm{j}\right)\right)=\mathrm{P}\left({\mathrm{E}}_{\mathrm{T}}^{\mathrm{damage}}\left(\mathrm{j}\right)\left|{}_{{\mathrm{E}}_{\mathrm{T}}\left(\mathrm{j}\right)}\right)\right).\mathrm{P}\left({\mathrm{E}}_{\mathrm{T}}\left(\mathrm{i}\right)\right) $$

(15.54)

15.2.4.2.3 Propagation of the Accident: Blast Wave

The first accident at the source tank S(i), i = 1 up to N_s, may give rise to a blast wave that generates high positive and negative pressures around the source and may affect mechanically the surrounding tanks and facilities. This event and its probability of occurrence are respectively defined as:

$$ {\mathrm{E}}_{\mathrm{W}}\left(\mathrm{i}\right){\ \mathrm{with}\ \mathrm{P}}_{\mathrm{W}}\left(\mathrm{i}\right)=\mathrm{P}\left({\left.{\mathrm{E}}_{\mathrm{W}}\left(\mathrm{i}\right)\right|}_{{\mathrm{E}}_1\left(\mathrm{i}\right)}\right).\mathrm{P}\left({\mathrm{E}}_1\left(\mathrm{i}\right)\right) $$

(15.55)

This occurrence probability can be estimated according to collected database inputs or from theoretical and simulation approaches. The event generates a pressure field and front wave than can be described by:

$$ \mathrm{p}\left(\mathrm{i},\underset{\bar{\mkern6mu}}{{\mathrm{x}}_{\mathrm{s}}}\left(\mathrm{i}\right),\underset{\bar{\mkern6mu}}{{\mathrm{x}}_{\mathrm{t}}}\left(\mathrm{i}\right),\mathrm{t}-{\mathrm{t}}_1\left(\mathrm{i}\right)\right)=\mathrm{pressure}\ \mathrm{field} $$

(15.56)

The effect of this blast wave on the tank T(j) due to source S(i) can produce global or local damages. The corresponding probability of damage can be obtained by analytical or numeric approaches. Due to the complexity of the analysis, Monte Carlo simulations are the common tool used in order to estimate this value:

$$ \mathrm{P}\left({\mathrm{E}}_{\mathrm{W}}^{\mathrm{damage}}\left(\mathrm{j}\right)\right)=\mathrm{P}\left({\left.{\mathrm{E}}_{\mathrm{W}}^{\mathrm{damage}}\left(\mathrm{j}\right)\right|}_{{\mathrm{E}}_{\mathrm{W}}\left(\mathrm{j}\right)}\right).\mathrm{P}\left({\mathrm{E}}_{\mathrm{W}}\left(\mathrm{i}\right)\right) $$

(15.57)

15.2.4.2.4 Propagation of the Accident: Loss of Confinement

The first accident at the source tank S(i), i = 1 up to N_s, may cause loss of confinements with ejection of gases or liquids that may be indirect sources of fires or explosions. This event and its probability of occurrence are respectively defined as:

$$ {\mathrm{E}}_{\mathrm{C}}\left(\mathrm{i}\right){\ \mathrm{with}\ \mathrm{P}}_{\mathrm{C}}\left(\mathrm{i}\right)=\mathrm{P}\left({\left.{\mathrm{E}}_{\mathrm{C}}\left(\mathrm{i}\right)\right|}_{{\mathrm{E}}_1\left(\mathrm{i}\right)}\right).\mathrm{P}\left({\mathrm{E}}_1\left(\mathrm{i}\right)\right) $$

(15.58)

This occurrence probability can be estimated according to collected database inputs or from theoretical and simulation approaches. This event generates loss of confinement that releases liquids and gases and it can, therefore, give rise to a fire ignition or explosions, for instance. This confinement loss can be expressed by a critical volume of losses within a critical time (global volume or rate of volume release, in a half hour or an hour or a day, for instance) than can be described by:

$$ \mathrm{v}\left(\mathrm{i},\underset{\bar{\mkern6mu}}{{\mathrm{x}}_s}\left(\mathrm{i}\right),\underset{\bar{\mkern6mu}}{{\mathrm{x}}_{\mathrm{t}}}\left(\mathrm{i}\right),\mathrm{t}-{\mathrm{t}}_1\left(\mathrm{i}\right)\right)=\mathrm{volume}\ \mathrm{of}\ \mathrm{confinement}\ \mathrm{loss}\ \mathrm{and}\ \mathrm{products}\ \mathrm{release} $$

(15.59)

The effect of this confinement loss on the tank T(j) due to source S(i) can produce global or local damages. The corresponding probability of damage can be obtained by analytical or numeric approaches. Due to the complexity of the analysis, Monte Carlo simulations are the common tool used in order to estimate this value:

$$ \mathrm{P}\left({\mathrm{E}}_{\mathrm{C}}^{\mathrm{damage}}\left(\mathrm{j}\right)\right)=\mathrm{P}\left({\mathrm{E}}_{\mathrm{C}}^{\mathrm{damage}}\left(\mathrm{j}\right)\left|{}_{{\mathrm{E}}_{\mathrm{C}}\left(\mathrm{j}\right)}\right)\right).\mathrm{P}\left({\mathrm{E}}_{\mathrm{C}}\left(\mathrm{i}\right)\right) $$

(15.60)

15.2.4.3 Total Energy and Momentum: Conservation Requirements

When an accident is triggered, one should verify that the conservation requirements are satisfied, i.e.:

Momentum conservation of the ejected fragments and products (liquids or gases) for the tank S(i), i =, i.e. up to N_s,

$$ {\overrightarrow{\mathrm{p}}}_{\mathrm{initial}}\left(\mathrm{i}\right)={\overrightarrow{\mathrm{p}}}_{\operatorname{int}\mathrm{ernal}}\left(\mathrm{i}\right)+{\overrightarrow{\mathrm{p}}}_{\mathrm{external}}\left(\mathrm{i}\right)\ \mathrm{with}\ {\overrightarrow{\mathrm{p}}}_{\mathrm{internal}}=\overrightarrow{0} $$

(15.61)

$$ {\overrightarrow{\mathrm{p}}}_{\mathrm{external}}\left(\mathrm{i}\right)\ne \overrightarrow{0}:\mathrm{in}\ \mathrm{case}\ \mathrm{of}\ \mathrm{a}\ \mathrm{prior}\ \mathrm{impact}\ \mathrm{for}\ \mathrm{instance} $$

(15.62)

$$ {\overrightarrow{\mathrm{p}}}_{\mathrm{initial}}\left(\mathrm{i}\right)={\overrightarrow{\mathrm{p}}}_{\mathrm{fragments}}\left(\mathrm{i}\right)+{\overrightarrow{\mathrm{p}}}_{\mathrm{fluids}}\left(\mathrm{i}\right) $$

(15.63)

$$ {\overrightarrow{\mathrm{p}}}_{\mathrm{f}\mathrm{ragments}}={\displaystyle \sum_{\mathrm{k}=1}^{{\mathrm{N}}_{\mathrm{f}}}{\mathrm{m}}_{\mathrm{p}}\left(\mathrm{k}\right).{\overrightarrow{\boldsymbol{v}}}_{\mathrm{p}}\left(\mathrm{k}\right)}\kern0.5em \mathrm{and}\kern0.5em {\overrightarrow{\mathrm{p}}}_{\mathrm{f}\mathrm{luids}}={\displaystyle \underset{{\mathrm{V}}_{\mathrm{g}}}{\int }{\uprho}_{\mathrm{G}}}.{\boldsymbol{v}}_{\mathrm{G}}{.\mathrm{dV}}_{\mathrm{g}}+{\displaystyle \underset{{\mathrm{V}}_{\mathrm{l}}}{\int }{\uprho}_{\mathrm{L}}}.{\boldsymbol{v}}_{\mathrm{L}}{.\mathrm{dV}}_l $$

(15.64)

Where: ${\overrightarrow{\mathrm{p}}}_{\mathrm{initial}}\left(\mathrm{i}\right)$ = initial momentum in the considered tank S(i); ${\overrightarrow{\mathrm{p}}}_{\mathrm{external}}\left(\mathrm{i}\right)$ = momentum provided by the external cause such as a prior external impact on the tank, for instance; ${\overrightarrow{\mathrm{p}}}_{\mathrm{fragments}}\left(\mathrm{i}\right)$ = total momentum of the fragments; ${\overrightarrow{\mathrm{p}}}_{\mathrm{fluids}}\left(\mathrm{i}\right)$ = total momentum of the ejected fluids (liquids and gases); m_p, v _p, N_f = mass, velocity of each fragment among the total set of N_f ejected fragments, r_G, v _G, V_g = density, velocity and volume of the gas part, respectively; r_L, v _L, V_l = density or specific weight (if g included), velocity and volume of the liquid part, respectively.

Energy conservation since the total initial energy (internal energy and external energy in case of impacts, for instance) is partly transformed into kinetic energy (fragments, gases and liquids), thermal energy, blast energy, dissipated energy and residual energy, i.e.

$$ {\mathrm{E}}_{\mathrm{initial}}\left(\mathrm{i}\right)={\mathrm{E}}_{\mathrm{internal}}\left(\mathrm{i}\right)+{\mathrm{E}}_{\mathrm{external}}\left(\mathrm{i}\right){\kern0.5em \mathrm{with}\kern0.5em \mathrm{E}}_{\mathrm{internal}}\ne 0 $$

(15.65)

$$ {\mathrm{E}}_{\mathrm{external}}\left(\mathrm{i}\right)\ne 0:\mathrm{in}\ \mathrm{case}\ \mathrm{of}\ \mathrm{a}\ \mathrm{prior}\ \mathrm{impact}\ \mathrm{or}\ \mathrm{thermal}\ \mathrm{flow},\mathrm{for}\ \mathrm{instance} $$

(15.66)

$$ {\mathrm{E}}_{\mathrm{initial}}\left(\mathrm{i}\right)={\mathrm{E}}_{\mathrm{solids}}\left(\mathrm{i}\right)+{\mathrm{E}}_{\mathrm{fluids}}\left(\mathrm{i}\right)+{\mathrm{E}}_{\mathrm{blast}\hbox{-} \mathrm{wave}}\left(\mathrm{i}\right)+{\mathrm{E}}_{\mathrm{thermal}\hbox{-} \mathrm{effect}}\left(\mathrm{i}\right)+{\mathrm{E}}_{\mathrm{dissipated}}\left(\mathrm{i}\right)+{\mathrm{E}}_{\mathrm{residual}}\left(\mathrm{i}\right) $$

(15.67)

$$ {\mathrm{E}}_{\mathrm{f}\mathrm{ragments}}={\displaystyle \sum_{\mathrm{k}=1}^{{\mathrm{N}}_{\mathrm{f}}}\left(\frac{1}{2}{\mathrm{m}}_{\mathrm{p}}\left(\mathrm{k}\right).{v}_{\mathrm{p}}^2\left(\mathrm{k}\right)\right)} $$

(15.68)

$$ {\mathrm{E}}_{\mathrm{fluids}}\left(\mathrm{i}\right)={\displaystyle \underset{{\mathrm{V}}_{\mathrm{g}}}{\int}\frac{1}{2}{\uprho}_{\mathrm{G}}}.{v}_{\mathrm{G}}^2{.\mathrm{dV}}_{\mathrm{g}}+{\displaystyle \underset{{\mathrm{V}}_{\mathrm{l}}}{\int }{\uprho}_{\mathrm{L}}}.{v}_{\mathrm{L}}^2.\mathrm{dV} $$

(15.69)

Where: E_internal (i) = internal energy in the considered tank S(i); E_external (i) = external energy provided by the external cause such as a prior external impact on the tank, for instance; E_initial (i) = initial total energy in the considered tank S(i); E_fragments (i) = total kinetic energy of the fragments; E_fluids (i) = total kinetic energy of the ejected fluids (liquids and gases); E_blast-wave (i) = total energy transported by the blast wave; E_{thermal-effect} (i) = total energy transported by the fire ball; E_dissipated (i) = total energy dissipated in order to trigger the explosion and fragmentation of the considered tank (such as plastic deformation, cracking and propagation, fragmentation, chemical reactions and transformation, etc.); and E_residual (i) = total residual energy remaining in the damaged tank (residual products and solids, etc.).

15.2.5 General Purpose of the Domino Effect Study and Use of the Occurrence Probability for Decision Making

The industrial accidents have in general several consequences of great importance, such as:

• Socio-economic and employees jobs losses due to production interruption, reconstruction, repair or strengthening of the industrial plant

• Environmental consequences in case of containment losses such as liquids or gases

• Fires that threaten the whole plant as well as the surrounding buildings, facilities, installations, etc.

• Threats to the health and physical integrity of the employees and inhabitants in case of toxic products release, etc.

In order to reduce or mitigate the disaster, one may consider either, see Fig. 15.8:

Fig. 15.8

Protective measures regarding potential sources or targets

• Reduction of the hazards and threats by isolating the sources of possible accidents

• Reduction of the vulnerability of the potential targets by protective measures better design, barriers and protections, erection of buildings, tanks and installations with large relative security distances

• Regular inspections and severe security measures, etc.

• Protection against confinement losses such as containment and retention basins in case of petrol oil, for instance.

• Use of automatic protective systems such as shutdown systems, alarms, fire protection, etc.

From a theoretical point of view, let us consider that the site under study (industrial plant, surrounding buildings, facilities and other plants, strategic installations, operational headquarters, etc. is so that the total expected cost of losses is:

$$ {\mathrm{C}}_{\mathrm{losses}}^{\mathrm{g}}={\displaystyle \sum_{k=1}^n\mathrm{P}\left({\mathrm{E}}_k^{\mathrm{g}}\right).{\mathrm{C}}_{\mathrm{k}}^{\mathrm{g}}}\kern0.62em \mathrm{where}\kern0.5em \mathrm{P}\left({\mathrm{E}}_k^{\mathrm{g}}\right)=\mathrm{P}\left({\mathrm{E}}_k^{\mathrm{g}}\left|{}_{{\mathrm{E}}_{\mathrm{k}-1}^{\mathrm{damage}}}\right.\right).\mathrm{P}\left({\mathrm{E}}_{\mathrm{k}-1}^{\mathrm{damage}}\left|{}_{{\mathrm{E}}_{\mathrm{k}-1}^{\mathrm{propa}}}\right.\right).\mathrm{P}\left({\mathrm{E}}_{\mathrm{k}-1}^{\mathrm{propa}}\left|{}_{{\mathrm{E}}_{\mathrm{k}-1}^{\mathrm{g}}}\right.\right).\mathrm{P}\left({\mathrm{E}}_{\mathrm{k}-1}^{\mathrm{g}}\right) $$

(15.70)

where : C ^g_k = socio-economic losses as consequences of the sequence k of the domino effect, k = 1 up to the range n of sequences under study (until the total plant is destroyed or reaches a given threshold of destruction, C ^g_losses = mathematical expected value of the socio-economic consequences on the whole industrial plant and its concerned vicinity.

In order to mitigate the industrial disaster, the stockholders may decide to adopt several protective solutions in order to optimise the economic investments so that to reach the optimal global cost:

$$ {\mathrm{C}}_{\mathrm{opt}}^{\mathrm{g}}=\mathrm{Min}\left\{{\mathrm{C}}_0^{\mathrm{g}}+{\Delta \mathrm{C}}_0^{\mathrm{g}}+{\displaystyle \sum_{k=1}^n\left(\mathrm{P}\left({\mathrm{E}}_k^{\mathrm{g}}\right)+\Delta \mathrm{P}\left({\mathrm{E}}_k^{\mathrm{g}}\right)\right).{\mathrm{C}}_{\mathrm{g}}}\right\} $$

(15.71)

Where: C ^g_opt = optimal global cost of the entire zone (industrial plant and its affected surroundings), C ^g₀ = initial global cost before adopting any additional protective measures so that the initial risk of domino effect P(E ^g _k ) is reduced by ΔP(E ^g _k ) as consequence of the protective measures.

In fact, this optimal global cost seems easy to be theoretically calculated. However, several aspects such as respect of human life, pollutions and aggressive products release, reactions of the public opinion and political decisions make this optimization not so easy to be reached in practice. However, this theoretical formulation may also be helpful in prospecting objective investments and accompanying measures (survey and early warning systems, automatic control and shutdowns, protective barriers, vicinity planning and organization) that result in risk reduction, disaster mitigation and satisfy resilience and quick recovery requirements.

15.3 Applications and Sensitivity Analysis

15.3.1 Risk of Failure

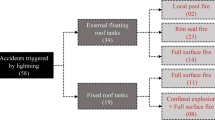
The present application considers the case of tanks with various filling levels, see Table 15.1. It is restricted to the analysis of the fragments impacts and the blast wave. For sake of simplicity, the thermal flows effects and the containment losses effects are not included in the present results. They are done separately.

Table 15.1 Probabilistic description of the structural fragments (Mebarki et al. 2009a, b)

Monte Carlo simulations are used in order to evaluate the probability of impacts as well as the probability of failure.

15.3.2 Structural Fragments: Probability of Impacts and Risk of Failure

The probability of failure is obtained by Monte Carlo simulations as, (Mebarki et al. 2007, 2008a, b, 2009a, b; Mingguang and Juncheng 2008):

$$ {\mathrm{P}}_{\mathrm{rup}}={\displaystyle \sum_{k=1}^{{\mathrm{N}}_{\mathrm{sim}}}\frac{1_{\left(\mathrm{State}\ \mathrm{of}\ \mathrm{a}\ \mathrm{target}\ne 0\right)}(k)}{{\mathrm{N}}_{\mathrm{sim}}}} $$

(15.72)

With:

$$ {1}_{\left(\mathrm{State}\ \mathrm{of}\ \mathrm{a}\ \mathrm{target}\ne 0\right)}(k)=\left[\begin{array}{c}\hfill 1\ \mathrm{if}\ \mathrm{a}\ \mathrm{target}\ \mathrm{is}\ \mathrm{completely}\ \mathrm{damaged}\hfill \\ {}\hfill 0\ \mathrm{else}\hfill \end{array}\right. $$

(15.73)

And: N_sim = total number of simulations.

Furthermore, a uniform random variable, H, is considered in order to express the filling level of a source tank. Its values range within the interval] 0; H_max], where H_max is the maximal filling level of a tank.

The trajectory of the fragments and their impact on target tanks are evaluated according to existing developments (Mebarki et al. 2009a, b).

15.3.3 Blast Waves: Effects on Target Tanks and Risk of Failure

After an explosion, a blast wave propagates through the air and in contact with surrounding tanks it can produce mechanical effects on affected tanks, resulting in:

• Excessive bending,

• Tank overturning,

• Global buckling,

• Tank sliding on the ground surface, and

• Excessive shear and bending of the target anchors.

15.3.4 Results and Comments

15.3.4.1 Comparison Between the Proposed Theoretical Models and the Experimental Results

Obviously, the results depend intimately on the accuracy of the models concerning the governing parameters: fragments description, their trajectory, their impacts and perforation of the impacted targets. The number of structural fragments is described by a theoretical probabilistic model which results are reported in Fig. 15.9a, in the case of BLEVE phenomena. The kinetic energy at ejection of the fragments, as shown in Fig. 15.9b, and their initial horizontal angles are also described by adequate models, derived from experimental observations in the case of spherical as well as cylindrical metal tanks, (Mebarki et al. 2009a).

Fig. 15.9

Explosion and structural fragments. (a) BLEVE and Number of fragments (Mebarki et al. 2009a); (b) Kinetic energy of the fragments, (Mebarki et al. 2009a); (c) Distances of fragments impacts in the real case of Mexico accident (Mebarki et al. 2009b)

Furthermore, the simulation of explosion and fragments trajectory is run for the case of Mexico accident: a good accordance is obtained between the observed distances of fragments impacts and the theoretical prediction as shown in Fig. 15.9c, (Mebarki et al. 2009b).

The interaction of the impacted tanks and the metals fragments is described by simplified models of penetration and perforation, whereas the fragments are considered as rigid rods. A set of experimental results is collected for various incidence angles and velocities at impact. The proposed simplified models for perforation and penetration provide good predictions of the penetration depth and velocities after perforation, (Mebarki et al. 2007, 2008b).

15.3.4.2 Sensitivity Analysis and Numerical Simulations

For illustrative purposes, the source tank is cylindrical and pressurized, whereas the target tank is at atmospheric pressure, see Table 15.2. The source tank content is liquefied propane. Target tank is supposed without any liquefied content, full of evaporated gas-oxygen mixture. Under this assumption, secondary effects due to liquid dynamic movement are intentionally neglected and, on the other side, a target tank is more prone to mechanical damage.

Table 15.2 Case study: source and target tanks definition

The target tank is located at a distance of 100 m from the source tank. Considering the origin of coordinate system at the centre of source tank, the centre of the target tank is then located at (100 m: horizontal distance, 0 m, 6 m: height). Table 15.3 provides the results obtained from the simulations, i.e.: projectiles impacting the target, distribution of projectiles and distribution of projectiles kinetic energy at the impact on the ground for each angular sector, see Table 15.3 and Fig. 15.10a.

Table 15.3 Properties of the structural fragments ejected as projectiles impacting the target

Fig. 15.10

Distribution of projectiles and failure risk. (a) Iso-values of impact probability around the cylindrical source tank; (b) Iso-values of tanks failure probability under the projectiles impact (P_f,imp) and the overpressure wave (P_f,w)

From the results of simulations for projectiles impact and overpressure wave effect on the atmospheric tank it is possible to compare levels of risk from each phenomena and for different levels of damage. It is found that overpressure waves can produce significant damages at the near field. On the other hand, risk of projectiles impact is much lower at the near field but projectiles can trigger the domino effect at much higher distance.

Figure 15.10b shows that the probability of failure from the blast wave is 3–7 times greater than the probability of failure produced by projectiles. Also, in this model only massive projectiles are considered as the potential projectiles and small, light projectiles are intentionally neglected.

15.4 Natural Hazards as Triggering Events: Tsunamis Caused by Quakes

15.4.1 Theoretical Frameworks and Required Steps

Natural hazards such as quakes, tsunamis, storms, floods, lightening, extreme winds, tornados and typhoons for instance may trigger domino effects sequences in industrial plants. Actually, the tanks and facilities may be damaged and the damages may propagate until disastrous situations take rise as it happened during the 2011 Eastern Japan Great Earthquake Disaster (Norio et al. 2011; Goto et al. 2011): quakes and tsunamis effects have caused serious and disastrous damages.

Therefore, mitigation and risk reduction for the case of coastal industrial plants, for instance, require four steps:

• Hazard modeling: description of the maximum inputs generated by the natural hazard (PGA: Peak Ground Acceleration for the quakes, PWH: Peak Water Heights and Run-ups for the tsunamis, for instance).

• System vulnerability, fragility and limit state functions: definition of the limit states that should not be reached by the systems under study in order to avoid occurrence of system failure (rupture of the industrial tanks or critical release of products after mechanical damages and impacts for instance) (Askan and Yucemen 2010; Reese et al. 2011).

• Reliability and risk analysis: assessment of the limit states occurrence probability, i.e. probability of failure. Actually, the hazard and the vulnerability are described within a probabilistic framework in order to express the error models and cover the uncertainties and heterogeneities (Eckert et al. 2012; Leone et al. 2011).

• And decision making by optimization: definition of protective measures and required investments in order to reduce the expected risks and socio-economic losses (protective barriers, coastal walls, early warning systems, real time survey, etc.), (Beltrami and Risio 2011; Grasso and Singh 2008; Jin and Lin 2011; van Zijll de Jong et al. 2011; Wilson et al. 2011).

15.4.2 Hazard Modeling: Simplified and Probabilistic Models – Case of Tsunamis

The present work focuses on the particular case of tsunamis. Several authors have investigated the description and modeling of the tsunamis in order to predict the Peak Water Heights and Run-ups (Burwell et al. 2007; Cheung et al. 2011; Constantin 2009; Demetracopoulos et al. 1994; Flouri et al. 2011; Haugen et al. 2005; Heidarzadeh et al. 2009; Helal and Mehanna 2008; Kharif and Pelinovsky 2005; Liu et al. 2009; Lovholt et al. 2011; Madsen 2010; Nandasena et al. 2011; Pophet et al. 2011; Sladen et al. 2007; Todorovska et al. 2002; Ward 2011; Wijetunge 2006; Zhang et al. 2009; Zhao et al. 2011).

Simplified models have been developed for quick evaluation of the Peak Water Heights and Run-ups. The model denoted cMD (coupled Magnitude-Distance) has proved to be efficient in predicting the Peak Ground Acceleration for various soil conditions (Mebarki 2009c):

$$ \frac{A}{g}=\frac{e^{-\beta .{D}_h}}{1+{D}_h.{e}^{-\left({M}_w-{M}_0\right)}} $$

(15.74)

Where: A [in m/s²] = Peak Ground Acceleration, g [in m/s²] = gravity acceleration, D_h [in km] = hypocentral central, M_w = moment magnitude of the earthquake, and two fitting constants (β and a threshold magnitude M₀). The error model associated to the PGA is assumed to follow a Gamma distribution; a Log-Normal distribution is also acceptable.

This form of the model and the distribution of the error model are adopted to derive the Peak Water Height (PWH) of the tsunamis in the sea zone far from the shoreline, see Fig. 15.11:

Fig. 15.11

Tsunami path from the epicentral zone towards the shore and inlands

$$ \frac{H}{H_0}=\frac{e^{-\beta .{D}_h}}{1+{D}_h.{e}^{-\left({M}_w-{M}_o\right)}} $$

(15.75)

Where: H [in m] = Peak Water Height, D_h [in km] = hypocentral central, M_w = moment magnitude of the earthquake, and three fitting constants β, M₀ = threshold magnitude and H₀ [in m] = equivalent uplift height at the epicentral zone. The error model associated to the PWH is also assumed to follow a Gamma distribution.

The constant H₀ is adopted according to Abe’s proposal (Abe 1993):

$$ \log \left({H}_0\right)=0.5{M}_w-3.3+C $$

(15.76)

Where: C = constant as fitting parameter depending on the kind of subduction zone.

Furthermore, the velocity of the tsunami waves is also an important parameter that governs the impact loads on the structures and facilities. It is derived from the Peak Water Height as follows:

$$ V=\sqrt{g\left(h+H\right)} $$

(15.77)

Near the shoreline, the seabed may have a regular or disturbed slope from an interface zone (the seabed is not considered as horizontal) up to the shoreline, see Fig. 15.12. Of course, these interface zone and average slope until the shoreline depend on the local topography and bathymetry. For sake of simplification, sometimes one could assume a straight line from the interface until the shoreline. The Peak Water Height at the shoreline is obtained by energy conservation, when no attenuation is considered, i.e.:

Fig. 15.12

Run-up and slopes between interface zones and shorelines

$$ {H}_{sl}^2.\sqrt{H_{sl}}={H}_{\operatorname{int}}^2.\sqrt{\left({h}_{\operatorname{int}}+{H}_{\operatorname{int}}\right)} $$

(15.78)

Due to the attenuation of energy, the final Peak Water Height at the shoreline becomes:

$$ {H}_{sl}={\left({H}_{\operatorname{int}}^2.\sqrt{\left({h}_{\operatorname{int}}+{H}_{\operatorname{int}}\right)}\right)}^{\raisebox{1ex}{$2$}\!\left/ \!\raisebox{-1ex}{$3$}\right.}.\frac{e^{-\beta .{D}_{\operatorname{int}}}}{1+{D}_{\operatorname{int}}.{e}^{-\left({M}_w-{M}_o\right)}} $$

(15.79)

Where: H_sl [in m] = Peak Water Height at the shoreline, H_int [in m] = Peak Water Height at the interface zone, h_int [in m] = depth of the sea at the interface zone, D_int [in km] = Horizontal projection of the distance from the interface zone towards the shoreline.

For illustrative purposes, the model is run for the case of the tsunami and Peak Water Heights observed during the earthquake Akita Oki, Japan on May25, 1983 with moment magnitude M_w = 7.9 (Abe 1995). According to the bathymetry collected for the zones under study (GEBCO 2012), the proposed model provides theoretical values that are in good accordance with the observed heights (H_obs), see Fig. 15.13. Furthermore, the theoretical confidence interval [H_5% up to H_95%] contains 95 % of the experimental values, i.e. more than the acceptable ratio of 90 %. A gamma distribution is considered for the error model with a coefficient of variation C_v = 45 %.

Fig. 15.13

Tsunamis height: H_obs = observed value; G_mean, G_5%, G_95% = Predicted Mean value, and fractiles 5 % and 95 % respectively.

15.4.3 Industrial Tanks and Vulnerability Under Tsunamis Effects

Under the quake and tsunami effects, the industrial tanks and facilities may suffer serious damages. These latters may reach serious intensities and their effect may propagate and might give rise to disastrous situation.

The tsunami may generate various effects and cause different damages to the structures and facilities at the coastal zones, under the mechanical loads, (ASCE 2010; ATC 2008, 2011; Batdorf 1974; FEMA; INERIS 2011; Nishi 2012; Goto 2008; Lukkunaprasit et al. 2009; Naito et al. 2012; Palermo and Nistor 2008; Saatçioğlu 2009; Sakakiyama et al. 2009; USGS 2011; Yeh 2008), see Fig. 15.14:

Fig. 15.14

Mechanical loadings, pressures and impacts on the industrial plants and facilities

• Hydrodynamic forces

• Hydrostatic pressures

• Buoyancy forces, and

• Debris impacts (boulders, cars, ships, etc.).

Various resulting damages may affect the industrial tanks and facilities:

• Excessive stresses under bending, shear and axial effects

• Stability, Sliding and overturning

• Lateral and longitudinal buckling

• Perforation, loss and release of flammable products (liquid, gas) that may produce fireballs and explosions.

15.4.4 Fragility Curves and Risk of Failure

Regarding any of the possible damages described above, the safety or failure of the affected structures depends on the loads, denoted S and its capacity to stand these loads, denoted R. The loads depend on the quake and tsunami inputs, i.e. PGA and PWH in the present case. The resistance depends on the geometry, external supports, the filling ratio of the tank, the thermodynamic conditions of the products contained by the tank, and the constitutive materials. Therefore, the loads as the resistances can be described by random variables. The probability of failure of the tank or facility under study is then defined as:

$$ {P}_f=\left(R-S\le 0\right) $$

(15.80)

This risk of failure is in general calculated by Monte Carlo simulations or level 2 methods, (Mebarki et al. 2008a). For industrial plants, it is worth to establish fragility curves that express the probability of exceeding given damage levels according to the governing input parameters such as PGA or PWH, for instance.

Various sophisticated or simplified methods can be used to investigate the response of cylindrical tanks to external pressures (Batford 1974; CCH 2000; CEN 2007; Chen and Rotter 2012; Godoy 2007; INERIS 2011; Koshimura and Namegaya 2009; Nistor et al. 2010a, 2010b; Suguino and Iwabuchi 2008; USGS 2011).

Figure 15.15 illustrates the fragility curve that corresponds to the case of a cylindrical tank with a slenderness (Tank height/Tank radius = L/r = 2.7), the filling ratio being considered as a uniform random variable and the limit state under study is the lateral buckling of the tank. For the present case, the risk of buckling is 0.27 for a Peak water height H = 4 m.

Fig. 15.15

Flowchart for fragility curve elaboration

Once the fragility curves have been calibrated for given typologies, it becomes easy to predict the risk of disaster within an entire industrial plant against potential upcoming hazards. The protective measures can therefore be adopted according to the level of expected risks and their socio-economic consequences.

15.5 General Conclusions

The domino effect is a cascading sequence of accidents and explosions, propagating from an initial source to the surrounding tanks in an industrial plant. Under these successive sequences, the industrial plant and the facilities, constructions and also human beings are severely threatened and may suffer important and irreversible losses.

The study of this effect from the initial possible accident until the dissemination within the plant requires multi-disciplinary approaches. The purpose of the present study if to provide a theoretical formulation that describes and evaluates the risk of occurrence of the triggering event, due to external or internal causes, the propagation effect as it may produce four sub-events, mainly: fragments ejected as projectiles, blast waves, fire balls as well as loss of confinement.

Probabilistic formulation is developed in order to describe the occurrence of the first event, as well as the probability of occurrence of the four sub-events. The interaction between the surrounding vessels and facilities and the mechanical or thermal effects of these sub-events may cause global or local damage as well as possible fire ignition and explosion. They may take rise within the affected targets or their immediate vicinity such as pipelines and power lines, for instance. Mechanical, thermo-mechanical and also chemo-thermo-mechanical analyses are therefore required in order to quantify the resulting effect on the considered targets (tanks or power lines impacted, heated, blasted, etc.). In the case of impact by structural fragments for instance, penetration and perforation as well as interaction projectile-impacted tank need the use of adequate material and structural behaviours, performed in general by numerical simulations.

The present study provides the theoretical aspects that should be considered for a detailed analysis of the domino effect. Relying on these developments, numerical simulations can be performed and sensitivity analysis as well as critical scenarios can be studied.

For instance, for the case considered in this study, it is found that overpressure waves can produce significant damage at the near field. On the other hand, risk of projectiles impact is much lower at the near field but projectiles can trigger the domino effect at much higher distance. Actually, the probability of failure from the blast wave is 3 –7 times greater than the probability of failure produced by projectiles. Also, in this model only massive projectiles are considered as the potential projectiles and small, light projectiles are intentionally neglected.

For a whole industrial plant that may suffer quakes or tsunamis effects, it is required to describe the input parameters (Peak ground Acceleration for quakes, or Peak Water Height for tsunamis) by physical models affected by probabilistic distributions. As many effects and damages may be caused to the concerned components of the plant (tanks, pipelines, and other facilities), it is necessary to consider the whole possible limit states in order to describe the state of the components under study (excessive stresses, stability, sliding, perforation, etc.). Probabilistic descriptions of the inputs and components responses are helpful in order to calibrate the fragility curves of each generic category of component. The risk analysis under potential natural hazards becomes therefore easy to perform for an entire industrial plant. The protective measures derive from an optimisation process: theoretically, by balance between possible investments and socio-economic consequences of disaster occurrence.

Relying on the expected numeric results, one may perform an optimisation of the generalised utility function (or costs) resulting from initial costs and expected socio-economic consequences. Adequate investments and protective options can then be objectively decided by the stakeholders in order to mitigate the potential disasters and aim a quick recovery as well as resilience.