Keywords

1 Introduction

The request for more functionality on a smaller physical area makes the design of modern RF (radio frequency) integrated circuits increasingly more complicated. Modern RF chips for mobile devices, for instance, typically have an FM radio, Blue-tooth, and GPS on one chip. Each of these functionalities are implemented with Voltage Controlled Oscillators (VCOs), that are designed to oscillate at certain different frequencies. Such oscillators are influenced by unintended (parasitic) signals coming from other blocks (such as Power Amplifiers) or from other oscillators, via for instance (unintended) inductive coupling through the substrate. A possibly undesired consequence of the perturbation is that the oscillators lock to a frequency different than designed for, or show pulling, in which case the oscillators are perturbed from their free running orbit without locking. This makes floor planning, i.e., determining the locations for the functional blocks, one of the most challenging tasks in RF chip design.

Our motivation comes from the design of RF systems, where oscillators play an important role [4, 8, 12, 21] in, for instance, high-frequency phase locked loops (PLLs). The nonlinear dynamics of interest include changes in the frequency spectrum of the oscillator due to small noise signals (an effect known as jitter [8]), which may lead to pulling or locking of the oscillator to a different frequency and may cause the oscillator to malfunction. Since both phase and amplitude dynamics are strongly nonlinear and spread over separated time scales [17], simulation is difficult. Accurate simulation requires very small time steps during time integration, resulting in unacceptable simulation times that block the design flow. Furthermore, transient simulation only gives limited understanding of the causes and mechanisms of the pulling and locking effects. Oscillators appear in many other physical systems and applications, see e.g. [2, 18].

Here we use the nonlinear phase macromodel introduced and developed in [8, 10, 11, 16, 17, 25]. Contrary to linear macromodels [1, 16, 21], the nonlinear phase macromodel is able to capture nonlinear effects such as injection locking. Because the macromodel replaces the original oscillator system by a single scalar equation, simulation times are decreased while the nonlinear oscillator effects can still be studied without loss of accuracy. We use the macromodels to predict the behavior of inductively coupled oscillators.

In some applications one exploits the coupling of oscillators. To reduce clockskew (clocksignals becoming out of phase), for instance, oscillators can be coupled via transmission lines [9]. Since accurate models for transmission lines can be large, this may lead to increased simulation times. We show how model order reduction techniques [3, 5, 22] can be used to decrease simulation times without unacceptable loss of accuracy.

The paper is organized as follows. Section 9.2 gives a summary of the phase noise theory. In Sect. 9.3 we show how the phase noise theory can be used to analyze oscillator–balun coupling. In Sect. 9.4, we explain the coupling of oscillators via transmission lines. Application of model order reduction techniques in simulation of coupled oscillators is described in Sect. 9.5 Numerical results are presented in Sect. 9.6 and Sect. 9.7 concludes.

2 Phase Noise Analysis of Oscillators

A general free-running oscillator is described as an autonomous system of differential equations:

$${\frac{\hbox{d}q(x)}{\hbox{d}t}}+j(x)=0,$$
(9.1a)
$$x(0)=x(T),$$
(9.1b)

where \(x(t)\in{\mathbb{R}}^n\) are the state variables, T is the period of the free running oscillator, which is in general unknown, \(q, j : {\mathbb{R}}^n \rightarrow {\mathbb{R}}^n\) are (nonlinear) functions and n is the system size. The solution of (9.1a, b) is called periodic steady state (PSS) and is denoted by x pss . Although finding a PSS solution can be a challenging task in itself, we will not discuss this in the present paper and refer to, for example, [6, 10, 13–15, 23].

A general oscillator under perturbation can be expressed as a system of differential equations

$${\frac{\hbox{d}q(x)}{\hbox{d}t}}+j(x)=b(t),$$
(9.2)

where \(b(t)\in{\mathbb{R}}^n\) are perturbations to the free running oscillator. For small perturbations b(t) it can be shown [8] that the solution of (9.2) can be approximated by

$$x_p(t)=x_{pss}(t+\alpha(t)),$$
(9.3)

where \(\alpha(t)\in{\mathbb{R}}\) is called the phase shift, which satisfies the following scalar nonlinear differential equation:

$$\dot{\alpha}(t)=V^T(t+\alpha(t))\cdot b(t),$$
(9.4a)
$$\alpha(0)=0,$$
(9.4b)

with \(V(t)\in{\mathbb{R}}^n\) being the perturbation projection vector (PPV) [8] of (9.2). The PPV is a periodic function with the same period as the oscillator and can efficiently be computed directly from the PPS solution, see e.g. [7].

3 Oscillator Coupled to a Balun

In this section we consider the mathematical model of an oscillator inductively coupled to a balun. A balun is an electrical transformer that can transform balanced signals to unbalanced signals and vice versa. A schematic view of an LC oscillator coupled to a balun with mutual inductors is given in Fig. 9.1. The corresponding mathematical model is given by the following set of equations:

$$ C_1{\frac{\hbox{d}v_1(t)}{\hbox{d}t}}+{\frac{v_1(t)}{R_1}}+i_1(t)+S\tanh\left({\frac{G_n}{S}}v_1(t)\right)=0,$$
(9.5a)
$$L_1{\frac{\hbox{d}i_1(t)}{\hbox{d}t}}+M_{12}{\frac{\hbox{d} i_2(t)}{\hbox{d}t}}+M_{13}{\frac{\hbox{d}i_3(t)}{\hbox{d} t}}-v_1(t)=0,$$
(9.5b)
$$C_2{\frac{\hbox{d}v_2(t)}{\hbox{d} t}}+{\frac{v_2(t)}{R_2}}+i_2(t)+I(t)=0,$$
(9.5c)
$$L_2{\frac{\hbox{d}i_2(t)}{\hbox{d}t}}+M_{12}{\frac{\hbox{d}i_1(t)}{\hbox{d}t}}+M_{23}{\frac{\hbox{d}i_3(t)}{\hbox{d} t}} -v_2(t)=0,$$
(9.5d)
$$C_3{\frac{\hbox{d}v_3(t)}{\hbox{d} t}}+{\frac{v_3(t)}{R_3}}+i_3(t)=0,$$
(9.5e)
$$L_3{\frac{\hbox{d}i_3(t)}{\hbox{d} t}} +M_{13}{\frac{\hbox{d}i_1(t)}{\hbox{d} t}}+M_{23}{\frac{\hbox{d}i_2(t)}{\hbox{d} t}} -v_3(t)=0,$$
(9.5f)

where \(M_{ij}=k_{ij}\sqrt{L_iL_j},\; i,j=1,2,3, \;i<j\) is the mutual inductance and k ij is the coupling factor. The parameters of the nonlinear resistor are S = 1/R 1 and \(G_n=-1.1/R_1\) and the current injection in the primary balun is denoted by I(t).

Fig. 9.1
figure 1

Oscillator coupled with a balun

Full size image

For small coupling factors we can consider \(M_{12}{\frac{\hbox{d}i_2(t)}{\hbox{d}t}}+M_{13} {\frac{\hbox{d}i_3(t)}{\hbox{d}t}}\) in (9.5b) as a small perturbation to the oscillator and apply the phase shift macromodel to (9.5a)–(9.5b). Then the reduced model corresponding to (9.5a)–(9.5b) is

$${\frac{\hbox{d}\alpha(t)}{\hbox{d}t}}= V^T(t+\alpha(t))\cdot\left( \begin{array}{c} 0 \\ -M_{12}{\frac{\hbox{d}i_2(t)}{\hbox{d}t}}-M_{13}{\frac{\hbox{d}i_3(t)}{\hbox{d} t}}\\ \end{array}\right), $$
(9.6)

where V is the PPV of the oscillator. The balun is described by a linear circuit (9.5c)–(9.5f) which can be written in a more compact form:

$$ E{\frac{\hbox{d}x(t)}{\hbox{d}t}}=Ax(t)+Bu(t), $$
(9.7)

where

$$ E=\left(\begin{array}{cccc} C_2 &0&0&0 \\ 0&L_2 &0&M_{23} \\ 0 &0&C_3&0 \\ 0&M_{23} &0&L_3 \\ \end{array}\right), \quad A=\left( \begin{array}{cccc} -{\frac{1}{R_2}} &-1&0&0 \\ 1&0 &0&0 \\ 0 &0&-{\frac{1}{R_3}}&0 \\ 0&0 &1&0 \\ \end{array}\right), $$
(9.8a)
$$ B=\left( \begin{array}{cc} -1 &0\\ 0&-M_{12}\\ 0&0\\ 0&-M_{13}\\ \end{array}\right), \quad x(t)=\left( \begin{array}{c} v_2(t)\\ i_2(t)\\ v_3(t)\\ i_3(t) \\ \end{array}\right), \quad u(t)=\left( \begin{array}{c} I(t)\\ {\frac{{d}i_1(t)}{{d}t}}\\ \end{array}\right). $$
(9.8b)

With these notations (9.6) and (9.7) can be written in the following form

$$ {\frac{\hbox{d}\alpha(t)}{\hbox{d}t}}= V^T(t+\alpha(t))\cdot \left( \begin{array}{c} 0 \\ {\frac{{d}y(t)}{{d}t}} \end{array}\right) $$
(9.9a)
$$ E{\frac{\hbox{d}x(t)}{\hbox{d}t}}=Ax(t)+Bu(t), $$
(9.9b)
$$ y(t)=\fancyscript{C}^T x, $$
(9.9c)

where \(\\fancyscript{C}^T=(0,-M_{12},0,-M_{13})\) and i 1(t) is computed by using (9.3).

4 Oscillator Coupling to a Transmission Line

In some applications oscillators are coupled via transmission lines. By coupling oscillators via transmission lines, for instance, one can reduce the clockskew in clock distribution networks [9]. Accurate models for transmission lines may contain up to thousands or millions of RLC components [26]. Furthermore, the oscillators or the components that perturb (couple to) the oscillators can consist of many RLC components, for instance when ones takes into account parasitic effects. Since simulation times usually increase with the number of elements, one would like to limit the number of (parasitic) components as much as possible, without losing accuracy.

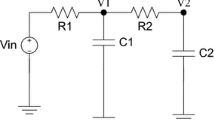
For the sake of simplicity in this paper we consider an RC transmission line coupled to an oscillator, see Fig. 9.2. Using phase macromodel for oscillator and by applying Kirchhoff’s current law to the transmission line circuit, we obtain the following set of differential equations:

$${\frac{\hbox{d}\alpha(t)}{\hbox{d}t}}= V^T(t+\alpha(t))\cdot \left( \begin{array}{c} {\frac{y(t)-v(t)}{R_1}} \\ 0 \\ \end{array}\right) $$
(9.10a)
$$E{\frac{\hbox{d}x(t)}{\hbox{d}t}}=Ax(t)+Bu(t),$$
(9.10b)
$$y(t)=\\fancyscript{C}^Tx,$$
(9.10c)

where

$$E=\hbox{diag}(C_1,C_2,\ldots,C_n), \quad A=\hbox{tridiag}\left({\frac{1}{R_i}},-{\frac{1}{R_i}}-{\frac{1}{R_{i+1}}}, {\frac{1}{R_{i+1}}}\right),$$
(9.11a)
$$B=\left(\begin{array}{cc} {\frac{1}{R_1}} &0\\ 0&0\\ \vdots&\vdots\\ 0&1 \\ \end{array} \right), \quad x(t)=\left( \begin{array}{c} v_1(t)\\ v_2(t)\\ \vdots\\ v_n(t) \\ \end{array} \right), \quad u(t)=\left( \begin{array}{c} v(t)\\ I(t)\\ \end{array} \right), \quad \fancyscript{C}=\left( \begin{array}{c} 1\\ 0\\ \vdots\\ 0 \\ \end{array}\right).$$
(9.11b)
Fig. 9.2
figure 2

Oscillator coupled to a transmission line

Full size image

In a similar way the phase macromodel of two oscillators coupled via a transmission line, see Fig. 9.3, is given by the following equations:

$$ {\frac{\hbox{d}\alpha_1(t)}{\hbox{d} t}}= V_1^T(t+\alpha_1(t))\cdot \left( \begin{array}{c} {\frac{v_1(t)-v(t)}{R_1}} \\ 0 \\ \end{array}\right) $$
(9.12a)
$$ E{\frac{\hbox{d}x(t)}{\hbox{d}t}}=Ax(t)+Bu(t), $$
(9.12b)
$$ {\frac{\hbox{d}\alpha_2(t)}{\hbox{d}t}}= V_2^T(t+\alpha_2(t))\cdot \left(\begin{array}{c} {\frac{v_n(t)-v_0(t)}{R_{n+1}}} \\ 0 \\ \end{array}\right), $$
(9.12c)

where α1(t) and α2(t) (V 1 and V 2) are phase shifts (PPV’s) of the corresponding oscillator. The matrices E, A and x are given by (9.11a, b) and

$$ B=\left(\begin{array}{cc} {\frac{1}{R_1}} &0\\ 0&0\\ \vdots&\vdots\\ 0&{\frac{1}{R_{n+1}}} \\ \end{array}\right), \quad u(t)=\left(\begin{array}{c} v(t)\\ v_0(t)\\ \end{array}\right). $$
(9.13)
Fig. 9.3
figure 3

Two oscillators coupled via a transmission line

Full size image

5 Model Order Reduction

Model order reduction (MOR) techniques [3, 5, 22] can be used to reduce the number of elements significantly. Here we show how model order reduction can be used for the analysis of oscillator perturbation effects as well. Since the main focus is to show how MOR techniques can be used (and not which technique is the most suitable), we limit the discussion here to balanced truncation [20]. For other methods, see, e.g., [3, 5, 22].

Given any dynamical system (A, B, C)

$$ E{\frac{\hbox{d}x(t)}{\hbox{d}t}}=Ax(t)+Bu(t), \quad y(t)=C^T x, $$

and assuming E = I, balanced truncation [20] consists of first computing a balancing transformation \(V\in{\mathbb{R}}^{n\times n}\), where B and C are input-to-state and state-to-output vectors, respectively. The balanced system \((V^TAV,V^TB,V^TC)\) has the nice property that the Hankel singular valuesFootnote 1 are easily available. A reduced order model can be constructed by selecting the columns of V that correspond to the k < n largest Hankel singular values. With \(V_k\in{\mathbb{R}}^{n\times k}\) having as columns these k columns, the reduced order model (of order k) becomes \((V_k^TAV_k,V_k^TB,V_k^TC)\). If E ≠ I is nonsingular, balanced truncation can be applied to \((E^{-1}A,E^{-1}B,C)\). For more details on balanced truncation, see [5, 20, 22].

In this paper we apply model order reduction to linear circuits that are coupled to oscillators, and the relevant equations for each problem describing linear circuits have the form of (9.9b)–(9.9c). For each problem the corresponding matrices E, A, B, and C can be identified readily, see (9.8a, b), (9.11a, b), (9.13) and note \(C\equiv\fancyscript{C}\). We use Matlab [19] implementation for balanced truncation to obtain reduced order models:

Note that we can apply balanced truncation because E is nonsingular. It is well known that in many cases in circuit simulation the system is a descriptor system and hence E is singular. Although generalizations of balanced truncation to descriptor systems exist [22, 24], other MOR techniques such as Krylov subspace methods and modal approximation might be more appropriate. We refer the reader to [3, 5, 22] for a good introduction to such techniques and MOR in general.

6 Numerical Experiments

In all the numerical experiments the simulations are run until \(T_{\rm{final}}= 6\times10^{-7}\,\hbox{s}\) with fixed time step τ = 10−11. In this section all the numerical results done with the phase macromodel combined with MOR technique are called macromodel-MOR simulation.

We compare our results with simulation results of the full circuit (no macromodeling, no model order reduction), hereafter full-simulation. Because the full circuit represents a stiff ODE, we use the Matlab built-in ODE solver ode15s with relative tolerance set to 10−7 (larger values of relative tolerance result in incorrect solutions) to achieve a comparable accuracy with the macromodel-MOR simulation results. In all experiments we observed that the simulation time of the macromodel-MOR technique is typically five times faster than full-simulation times.

6.1 Oscillator Coupled to a Balun

Consider an oscillator coupled to a balun as shown in Fig. 9.1 with the following parameters values:

Oscillator

Primary balun

Secondary balun

\(L_1= 0.64\times 10^{-9}\,\hbox{H}\)

\(L_2=1.10\times 10^{-9}\,\hbox{H}\)

\(L_3=3.60\times 10^{-9}\,\hbox{H}\)

\(C_1=1.71\times 10^{-12}\,\hbox{F}\)

\(C_2=4.00\times 10^{-12}\,\hbox{F}\)

\(C_3=1.22\times 10^{-12}\,\hbox{F}\)

\(R_1= 50\,\Upomega\)

\( R_2= 40\,\Upomega\)

\( R_2= 60\,\Upomega\)

The coefficients of the mutual inductive couplings are \(k_{12}=10^{-3}, k_{13}=5.96\times 10^{-3}, k_{23}=9.33\times 10^{-3}. \) The injected current in the primary balun is of the form

$$ I(t)=A_{\rm{inj}}\sin(2\pi(f_0-f_{\rm{off}})t), $$
(9.14)

where \(f_0=4.8\,\hbox{GHz}\) is the oscillator’s free running frequency, \(f_{\rm{off}}\) is the offset frequency and \(A_{\rm{inj}}\) is the current amplitude.

Simulation results of (9.9a, b, c) are shown in Fig. 9.4, where the frequency is plotted versus power spectral density (PSDFootnote 2). The obtained results by the macromodel-MOR technique with \(\hbox{mor}\_ \hbox{dim}=2\) provide a good approximation to the full-simulation results. We note that for the injected current with \(A_{\rm{inj}}=10^{-1}\,\hbox{A}\) the oscillator is locked to the injected signal. Similar results are also obtained for the balun.

Fig. 9.4
figure 4

Comparison of the output spectrum of the oscillator coupled to a balun obtained by the macromodel-full and the macromodel-MOR simulations for an increasing injected current amplitude \(A_{\rm{inj}}\) and an offset frequency \(f_{\rm{off}}=20\,\hbox{MHz}\)

Full size image

6.2 Oscillators Coupled with Transmission Lines

In this section we consider two academic examples, where transmission lines are modeled with RC components.

6.2.1 Single Oscillator Coupled to a Transmission Line

Let us consider the same oscillator as given in the previous section, now coupled to a transmission line, see Fig. 9.2. The size of the transmission line is n = 100 with the following parameters: \(C_1=\cdots=C_n=10^{-2}\,\hbox{pF}, R_1=40\,\hbox{k}\Upomega, R_2=\cdots=R_n=1\,\Upomega.\) The injected current has the form (9.14) with \(A_{\rm{inj}}=10^{-2}\,\hbox{A}\) and \(f_{\rm{off}}=20\,\hbox{MHz}.\) Dimension of the reduced system is \(\hbox{mor}\_ \hbox{dim}=18.\) Simulation results of (9.10a, b, c) around the first and third harmonics (this oscillator does not have a second harmonic) are shown in Fig. 9.5. The macromodel-MOR method, using techniques described in Sect. 9.5, gives a good approximation to the full simulation results.

Fig. 9.5
figure 5

Comparison of the output spectrum around the first and third harmonics of the oscillator coupled to a transmission line, cf. Fig. 9.2. a First harmonic. b Third harmonic

Full size image

6.2.2 Two LC Oscillators Coupled via a Transmission Line

For this experiment we consider two LC oscillators coupled via a transmission line with the mathematical model given by (9.12a, b, c). The first oscillator has a free running frequency \(f_1=4.8\,\hbox{GHz}\) and is described in Sect. 9.6.1. The second LC oscillator has the following parameter values: \(R_0=50\,\Upomega, L_0=0.64\,\hbox{nH}, C_0=1.87\,\hbox{pF}\) and a free running frequency \(f_2=4.6\,\hbox{GHz}.\) The size of the transmission line is n = 100 with the following parameters: \( C_1=\cdots=C_n=10^{-2}\,\hbox{pF}, R_1=R_{n+1}=4\,\hbox{k}\Upomega, R_2=\cdots=R_n=0.001\,\Upomega.\) Dimension of the reduced system is \(\hbox{mor}\_ \hbox{dim}=16.\) Numerical simulation results are given in Fig. 9.6. As can be seen from the spectra of Fig. 9.6, the macromodel-MOR and full simulation results match well: with both simulation methods the carrier and beat frequencies are the same with a comparable PSD.

Fig. 9.6
figure 6

Comparison of the output spectrum around the first and third harmonics of two oscillators coupled via a transmission line. \(f_2=4.6\,\hbox{GHz}:\) a first harmonic, b third harmonic; \(f_1=4.8\,\hbox{GHz}:\) c first harmonic, d third harmonic

Full size image

7 Conclusion

In this paper we have used nonlinear phase macromodels to accurately predict the behavior of individual and mutually coupled voltage controlled oscillators under perturbation. Several types of coupling have been described, including oscillator–balun coupling. For small perturbations, the nonlinear phase macromodels can also be used to produce results with accuracy comparable to full circuit simulations [11]. In addition, model order reduction techniques have been applied to transmission lines that couple oscillators. With these techniques, reduced-order models could be obtained that decreased simulation times while preserving the required accuracy.