1 Introduction

In the last decades, models which generalize that of the free relativistic particle by adding terms depending on the curvatures of the worldline to the action functional have been studied by both physicists and mathematicians. This trend is considered to have begun when Polyakov [13] expanded the Nambu-Goto action of the propagating string with a term computed from the second fundamental form of the so-called worldsheet, that is, the surface traced by the moving string, in order to study the phase structure of the dynamics. This idea was soon applied to the one-dimensional case. Pisarski [8] added a curvature term to the usual relativistic particle Lagrangian, but did not conceive it as representing the motion of particles and only studied its statistical properties by means of renormalization techniques. The crucial step appears to have been made by Plyushchay [9, 10], who understood that these models can be used to describe spinning particles. Plyushchay studied the dynamics arising from several functionals containing the extrinsic curvatures of the worldline, both classically and when quantized, using physical techniques such as the generalized Hamiltonian formalism of Dirac to handle Lagrangians containing higher derivatives.

Later, more researchers, including mathematicians and mathematical physicists, became interested in these constructions. One of their appeals is that, in contrast to previous models, no additional variables are required to implement the spinning degrees of freedom. They are fully intrinsic in that they only require the original spacetime instead of needing to resort to extra structures whose physical significance is not clear. Furthermore, the introduction of terms containing the curvatures of the worldline is compatible with the Poincaré invariance that is demanded of a relativistic particle model. Another advantage is that, although they were initially formulated on Minkowski spacetime by Plyushchay, Nesterenko and others, they can be readily generalized to other curved backgrounds thanks to their intrinsic nature. All these characteristics can be summed up by saying that such functionals allow to produce new relativistic particle models in an elegant and geometrical manner, and the study of their trajectories is a problem of both physical and purely mathematical interest.

Concerning the applications of the aforementioned models, it is not clear whether they furnish physically sensible particles living in spacetime, in general, as they oftentimes give tachyonic solutions. However, functionals depending on the curvature, torsion and other higher curvatures of the particle worldline, while not directly having the interpretation of describing real particles, can be found in several relevant contexts both inside and outside physics. Some of them are:

  • An action containing a term linear in the torsion features in studies of gauge field theories in low dimensionality [11, 12]. A peculiar phenomenon associated with this term is the Fermi-Bose transmutation, in which bosonic excitations of the field behave as if they were fermionic. More generally, the torsion term is connected with the excitations exhibiting fractional or anyonic statistics [15]. It has been suggested that one may gain insights into superconductivity, the quantum Hall effect and other planar physics by means of these models.

  • Curvature terms are relevant in the study of the Gauss-Landau-Hall problem [2], which can be thought of as the characterization of trajectories of charged particles under the influence of magnetic fields in curved backgrounds.

  • Some authors have used functionals depending on the first curvature to model polymer physics and, in particular, the behaviour of proteins [4].

The findings we present in this article are partially based on [1, 5]. In the first, the trajectories of the model whose action functional contains only a term linear in the curvature are characterized with a three-dimensional generalized Robertson-Walker spacetime as the background. In the second, a similar study is carried out for the functional linear in the torsion of the worldline, this time both in three-dimensional generalized Robertson-Walker and standard static spacetimes. The aim of this text is to extend the first study to standard static spacetimes and to collect and discuss all the results.

2 Generalities

2.1 Calculus of Variations

Given a three-dimensional time-oriented Lorentzian manifold \((\mathcal {M},g)\), we will consider functionals

$$\begin{aligned} \mathcal {S} : \varGamma \longrightarrow \mathbb {R}, \qquad \qquad \gamma \longmapsto \int _{\gamma } \mathcal {L} \; ds, \end{aligned}$$
(1)

Here, \(\varGamma \) is a suitable space of curves \(\gamma : I \subseteq \mathbb {R} \longrightarrow \mathcal {M}\), s is the arc-length parameter for the Lorentzian metric g and \(\mathcal {L}\) is a smooth function, usually known as the Lagrangian in a physical context. Trajectories of relativistic particles are identified with the critical curves of such functionals.

In order to find such trajectories, we require some basic knowledge from variational calculus on manifolds as found e.g. in [6], of which we provide a brief summary. We first note that, when given an appropriate differentiable manifold structure, the tangent space at a curve \(\gamma \in \varGamma \), \(T_{\gamma }\varGamma \) can be naturally identified with the space of smooth vector fields \(W \in \mathfrak {X}(\gamma )\).

In order to establish whether \(\gamma : [a,b] \longrightarrow \mathcal {M}\) is a critical point of a functional of the type that we will study, let us consider a variation of the curve given by a smooth two-parameter map \(\varOmega : (-\epsilon , \epsilon ) \times [a,b] \longrightarrow \mathcal {M}\) such that \(\varOmega (0,t) = \gamma (t)\). We introduce the vector fields

$$\begin{aligned} V(z,t) = \frac{\partial \varOmega }{\partial t}(z,t) \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; W(z,t) = \frac{\partial \varOmega }{\partial z}(z,t) \end{aligned}$$
(2)

Then, V(z, t) is tangent to each curve in \(\varOmega \). In particular, \(W(t) = W(0,t) \in \mathfrak {X}(\gamma )\). The vector field W(t) is known as a variational vector field. In fact, for any curve \(\gamma \) and variational vector field \(W \in T_{\gamma }\varGamma \), there always exists a variation \(\varOmega \) such that \(\varOmega (0,t) = \gamma (t)\) and \(\partial \varOmega / \partial z (0,t) = W(t)\), given by \(\varOmega (u,t) = \exp _{\gamma (t)}(uW(t))\) .

2.2 Equations of Motion

We begin by introducing the Frenet frame of a curve in a three-dimensional spacetime, which will be of much use in the following. Let \(\gamma : I \subseteq \mathbb {R} \longrightarrow \mathcal {M}\) be a curve such that \(\nabla _T T \ne 0\), where T is the normalized tangent vector along \(\gamma \). Define N to be the result of normalizing \(\nabla _T T\). Then

$$\begin{aligned} \nabla _T T = \epsilon _2 \kappa N \end{aligned}$$

where \(\kappa \; \>\; 0\) is the curvature of \(\gamma \) and \(\epsilon _2 = g(N, N) = \pm 1\). Now, let B be a unit vector along \(\gamma \) such that \(\{ T, N, B \}\) is a positively oriented basis. We have

$$\begin{aligned} \nabla _T N = -\epsilon _1 \kappa T + \epsilon _3 \tau N \qquad \qquad \nabla _T B = -\epsilon _2 \tau N \end{aligned}$$
(3)

where \(\tau \) is the torsion of \(\gamma \), which can be positive, negative or zero, and \(\epsilon _1 = g(T,T) = \pm 1, \epsilon _3 = g(B,B) = \pm 1\). The basis \(\{ T, N, B \}\) is called the Frenet frame of \(\gamma \). In case \(\nabla _T T = 0\) or, in other words, \(\gamma \) is a geodesic, we define its curvature and torsion to be \(\kappa = 0, \tau = 0\). We will occasionally make use of the two-dimensional Frenet frame \(\{ T, N \}\), where the vector fields satisfy

$$\begin{aligned} \nabla _T T = \epsilon _2 \kappa N \qquad \qquad \nabla _T N = -\epsilon _1 \kappa T \end{aligned}$$
(4)

and, in this case, \(\kappa \) is allowed to be negative.

We shall study the following functionals

$$\begin{aligned} \mathcal {S}_1(\gamma ) = \int _{\gamma } \kappa \, ds \qquad \qquad \mathcal {S}_2(\gamma ) = \int _{\gamma } \tau \, ds \end{aligned}$$
(5)

Note that all geodesics are trivially critical points of \(\mathcal {S}_1\), but this is not the case for \(\mathcal {S}_2\). In order to find the non-trivial critical points of these functionals, that is, those which are not geodesics, we apply the formalism introduced in the preceding section: one chooses a variation \(\varOmega \) with associated variational vector field W and base curve \(\gamma : [a,b] \longrightarrow \mathcal {M}\) and evaluates, for instance, \(\mathcal {S}_1\) on \(\varOmega \)

$$\begin{aligned} \mathcal {S}_1[\varOmega (z,t)] = \int _a^b \, \kappa (\gamma _z(t)) v_z(t) \, dt \end{aligned}$$
(6)

where \(\gamma _z(t) = \varOmega (z,t)\) and \(v_z(t) = \sqrt{|g(\gamma _z'(t), \gamma _z'(t))|}\). Then, \(\gamma \) is a critical point of \(\mathcal {S}_1\) if and only if

$$\begin{aligned} \frac{\partial }{\partial z} \bigg |_{z=0} \mathcal {S}_1[\varOmega (z,t)] = 0 \end{aligned}$$
(7)

for any variation \(\varOmega \) constructed from \(\gamma \). Explicitly, we can write

$$\begin{aligned} \frac{\partial }{\partial z} \mathcal {S}_1[\varOmega (z,t)] = \int _{\gamma _z} W(\kappa v) \, dt = \int _{\gamma _z} [W(\kappa ) \, v + W(v) \, \kappa ] \, dt \end{aligned}$$
(8)

and similarly for \(\mathcal {S}_2\). The usual rules of derivation are then used in conjunction with expressions such as \(v^2 = \epsilon _1 g(V, V), \kappa = g(\nabla _T T, N), \tau = -g(\nabla _T B, N)\) or the definition of the curvature tensor \(R(X,Y)Z = \nabla _X \nabla _Y Z - \nabla _Y \nabla _Z X - \nabla _{[X,Y]}Z\) (for more details, see [5]). So as to ensure the vanishing of the boundary terms resulting from this computation, we take the space of curves to be the so-called clamped curves

$$\begin{aligned}&\varGamma = \{ \gamma : [a,b] \longrightarrow \mathcal {M} :\nonumber \\&\gamma (a) = p, \gamma (b) = q, T(a) = x_1, T(b) = x_2, N(a) = y_1, N(b) = y_2 \} \end{aligned}$$
(9)

Then, we have

Proposition 1

Let \((M,g), \mathcal {S}_1, \mathcal {S}_2\) as above. Then, \(\gamma \in \varGamma \) with \(\kappa \ne 0\) is a critical point of \(\mathcal {S}_1\) if [1]

$$\begin{aligned} R(N,T)T - \epsilon _2 \epsilon _3 \tau ^2\,N - \epsilon _3 \tau ' B = 0 \end{aligned}$$
(10)

On the other hand, \(\gamma \in \varGamma \) is a critical point of \(\mathcal {S}_2\) if [5]

$$\begin{aligned} R(B,N)T - \epsilon _1 \kappa ' B + \epsilon _1 \epsilon _2 \kappa \tau N = 0 \end{aligned}$$
(11)

3 Set up

As mentioned in the introduction, we will consider two large families of three-dimensional spacetimes. First, the generalized Robertson-Walker (GRW) spacetimes are Lorentzian warped products [7] of the type \(I \times _f S\) where \(I \subseteq \mathbb {R}\) is an open interval and (S, g) is a Riemannian surface. This is to say that they are the manifold \(I \times S\) equipped with the Lorentzian product

$$\begin{aligned} g^f = -dt^2 + f^2 \, g \end{aligned}$$
(12)

where \(f : I \longrightarrow \mathbb {R}\) is a strictly positive function known as the warping function. Cosmological models used to successfully describe the universe on large scales are particular cases of GRWs, as well as (Anti-)de Sitter spacetimes, which play a very relevant role in some speculative ideas within the realm of theoretical physics.

Second, a standard static spacetime is a different kind of warped product in which the manifold is still \(I \times S\) but the metric is given by

$$\begin{aligned} g^f = -f^2 \, dt^2 + g \end{aligned}$$
(13)

where \(f : S \longrightarrow \mathbb {R}\). The motivation for these spacetimes is as follows: a spacetime is said to be stationary if it has enough symmetry to admit a global timelike Killing field U. Furthermore, if U is irrotational, meaning that for any point p in the spacetime and tangent vectors \(X, Y \in U_p^{\bot }\)

$$\begin{aligned} g(\nabla _X U, Y) - g(\nabla _Y U, X) = 0 \end{aligned}$$
(14)

then the spacetime is static. Standard static spacetimes are static in this sense. Moreover, any static spacetime is locally a standard static spacetime [14]. We remark that the exterior Schwarzschild solution, which is the simplest black hole model, is a standard static spacetime. More generally, there exist static regions in the (Anti)-de Sitter-Schwarzschild spacetime, which corresponds to a Schwarzschild black hole inside de Sitter or Anti-de Sitter spacetime.

Although the two families of spacetimes are generally quite different, they both reduce to an ordinary Lorentzian product if the warping function f is a constant, and the metrics coincide except for at most a constant factor. This will be used later to check consistency between results.

A particular property of the above spacetimes that is exploited in the calculations leading to the results that will be presented is that they can be foliated by spacelike slices as defined by

$$\begin{aligned} S(t) = \{t \} \times S = \{(t,p) : p \in S \} \end{aligned}$$
(15)

Then, trajectories of the functionals \(\mathcal {S}_1\) and \(\mathcal {S}_2\) having the form \(\gamma _t(s) = (t, \gamma (s))\), where \(\gamma \) is a curve in S, are sought. Note that this will only produce spacelike solutions which, when interpreted as particles, would be identified with tachyons.

4 Trajectories in Generalized Robertson-Walker Spacetimes

4.1 Frenet Frame

Consider a curve \(\gamma (s)\) in S with Frenet frame \(\{T,N \}\). In order to write the equations of motion for curves of the form \(\gamma _t(s) = (t, \gamma (s))\), with a fixed t in the domain of the warping function, we need to compute a Frenet frame for \(\gamma _t(s)\), which we will denote \(\{T^f, N^f, B^f \}\), where \(T^f = T/f\), as well as the curvature \(\kappa ^f\) and torsion \(\tau ^f\) of \(\gamma _t(s)\) as a function of \(\kappa \), f and their derivatives. This computation can be found in [1]. The relevant results include

$$\begin{aligned} \nabla f = -\dot{f} \partial _t \end{aligned}$$
(16)
$$\begin{aligned} \kappa ^f = \frac{\sqrt{\epsilon _2 (\kappa ^2 -\dot{f}^2)}}{f} \end{aligned}$$
(17)
$$\begin{aligned} N^f = \frac{\epsilon _2}{\sqrt{\epsilon _2 (\kappa ^2 -\dot{f}^2)}} (\kappa \xi + \dot{f} \partial _t) \end{aligned}$$
(18)
$$\begin{aligned} B^f = \frac{\epsilon _2}{\sqrt{\epsilon _2 (\kappa ^2 -\dot{f}^2)}} (\dot{f} \xi + \kappa \partial _t) \end{aligned}$$
(19)
$$\begin{aligned} \tau ^f = \frac{\epsilon _2 \kappa ' \dot{f}}{f(\kappa ^2 -\dot{f}^2)} \end{aligned}$$
(20)

where \(\epsilon _2, \epsilon _3\) are the signature of the causal characters of \(N^f, B^f\), respectively, \(\kappa \) is the curvature of \(\gamma (s)\) and \(\xi = N/f\).

4.2 The Curvature Functional

An extensive study of this case was carried out in [1], where it was found the following:

Proposition 2

Consider a three-dimensional generalized Robertson-Walker spacetime and a curve \(\gamma _t\) as above. Then, \(\gamma _t\) is a critical point of \(\mathcal {S}_1\) if and only if

$$\begin{aligned} \frac{G+\dot{f}^2}{f^2}\kappa = -\kappa (\tau ^f)^2 - \epsilon _3 \dot{f} (\tau ^f)' \end{aligned}$$
(21)
$$\begin{aligned} -\frac{\dot{f}\ddot{f}}{f} = \dot{f}(\tau ^f)^2 + \epsilon _3 \kappa (\tau ^f)' \end{aligned}$$
(22)

where G is the Gaussian curvature of the slice.

Proposition 3

Consider a three-dimensional generalized Robertson-Walker spacetime and a curve \(\gamma _t\) as above. If \(\gamma _t\) lies in a critical slice, that is, \(\dot{f}=0\), then \(\gamma _t\) is a critical point of \(\mathcal {S}_1\) if and only if either \(\gamma \) is a geodesic or G vanishes along \(\gamma \).

Proposition 4

For a three-dimensional generalized Robertson-Walker spacetime and a curve \(\gamma _t\) as above, solutions such that \(\kappa \) is not constant can only be found in slices satisying \(G \;<\; 0, \ddot{f}(t) \; <\; 0\) and \(-f(t)\ddot{f}(t)+\dot{f}(t)^2=1\). In such a slice, these curves are \(\gamma _t = (t, \gamma (s))\) where \(\gamma (s)\) is parametrized by arclength and

$$\begin{aligned} \kappa (s) = -\dot{f}(t) \tanh \left( \displaystyle \pm \sqrt{-f(t)\ddot{f}(t)s} + c \right) \end{aligned}$$
(23)

where c is an integration constant.

4.3 The Torsion Functional

In this case, we evaluate (11) using the results of Sect. 4.1. It is found that [5]

$$\begin{aligned} R^f(B^f, N^f)T^f = 0 \end{aligned}$$
(24)

and the equations of motion become

$$\begin{aligned} (\kappa ^f)' B^f - \epsilon _2 \kappa ^f \tau ^f N^f = 0 \end{aligned}$$
(25)

It is seen that this gives the following two equations

$$\begin{aligned} \dot{f} \kappa \kappa ' \left( \displaystyle 1 - \frac{\epsilon _2}{f} \right) = 0 \end{aligned}$$
(26)
$$\begin{aligned} \frac{\kappa '}{f} \left( \displaystyle \kappa ^2 - \frac{\dot{f}^2}{f} \right) = 0 \end{aligned}$$
(27)

which can only hold simultaneously if \(\kappa ' = 0\), so

Proposition 5

Consider a three-dimensional generalized Robertson-Walker spacetime and a curve \(\gamma _t = (t, \gamma (t))\) as earlier. \(\gamma _t\) is a critical point of \(\mathcal {S}_2\) if and only if \(\gamma \) has constant curvature.

5 Trajectories in Standard Static Spacetimes

5.1 Frenet Frame

Let us now consider the standard static case whose metric \(g^f\) is given by (13). A \(g^f\)-orthonormal frame for \(\gamma _t(s) = (t, \gamma (s))\) is \(\{ T, N, \partial _t/f \}\). Our aim, as usual, is to determine a Frenet frame \(\{ T^f = T, N^f, B^f \}\). Note that here T, N are tangent to the base space whereas \(\partial _t/f\) is tangent to the fiber. Then, so long as \(\kappa \ne 0\)

$$\begin{aligned} \nabla ^f_{T^f}T^f = \nabla _T T = \kappa N \end{aligned}$$
(28)

from which it follows that \(\kappa ^f = \kappa \) and \(N^f = N\). The only choice is then \(B^f = \partial _t/f\). Let us find the torsion of \(\gamma _t\)

$$\begin{aligned} \nabla ^f_{T^f}B^f = \nabla _T^f \left( \displaystyle \frac{\partial _t}{f} \right) = \frac{1}{f} \nabla _T^f \partial _t + T\left( \displaystyle \frac{1}{f} \right) \partial _t \end{aligned}$$
(29)

Making use of the required equation for warped products, we get

$$\begin{aligned} \nabla ^f_{T^f} B^f = \left[ \displaystyle \frac{T(f)}{f^2} - \frac{T(f)}{f^2} \right] \partial _t = 0 \end{aligned}$$
(30)

so these curves have \(\tau ^f = 0\). Note that the above results are not valid if \(\kappa = 0\), when both \(\gamma \) and \(\gamma _t\) are geodesics.

5.2 The Curvature Functional

When particularizing to a curve \(\gamma _t\) living in a slice, the equations of motion (10) reduce to

$$\begin{aligned} R^f(N^f,T^f)T^f = 0 \end{aligned}$$
(31)

Using the corresponding formula for the curvature tensor on a warped product, this is

$$\begin{aligned} R(N,T)T = 0 \Longrightarrow G = 0 \end{aligned}$$
(32)

and we conclude that

Proposition 6

Consider a three-dimensional standard static spacetime and a curve \(\gamma _t = (t, \gamma (s))\) as above. \(\gamma _t\) is a critical point of \(\mathcal {S}_1\) if and only if either \(\gamma \) is a geodesic or G vanishes along \(\gamma \).

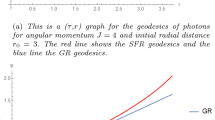
Examples: In any standard static spacetime whose slices are flat, any curve \(\gamma \) in S provides a trajectory \(\gamma _t = (t, \gamma (s))\) of the curvature functional. In particular, this is so if \(S = \mathbb {R}^2\) endowed with the usual metric.

For a less obvious scenario, consider the ruled surfaces [3]. When inmersed in \(\mathbb {R}^3\), these can be defined as follows. Let \(J \subset \mathbb {R}\) be an interval and \(\alpha (t), w(t)\) be differentiable maps \(\alpha : J \longrightarrow \mathbb {R}^3\), \(w : J \longrightarrow \mathbb {R}^3\). For each \(t \in J\), consider the line passing through point \(\alpha (t)\) and having the direction of vector w(t). Thus, the pair \(\{ \alpha (t), w(t) \}\) defines a family of lines in \(\mathbb {R}^3\). When taken together, all the lines trace a surface which admits the parametrization

$$\begin{aligned} x(t,v) = \alpha (t) + vw(t) \end{aligned}$$
(33)

with \(t \in J, w \in \mathbb {R}\). One calls this surface the ruled surface generated by \(\{ \alpha (t), w(t) \}\) and the lines defined by the pair \(\{ \alpha (t), w(t) \}\) are the rulings of the surface.

The aspect of such constructions that is of relevance for our study is that some ruled surfaces contain rulings along which the Gaussian curvature vanishes (with the usual metric). For this, we need the concept of line of striction. This is a line \(\beta (t)\) belonging to the surface such that \(\beta '(t)\) and \(w'(t)\) are orthogonal for any \(t \in J\). Then, the Gaussian curvature along a ruling is zero if it meets the striction line at a singular point, that is, a point such that \(x_t \times x_v = 0\) where \(\times \) denotes the cross product in \(\mathbb {R}^3\). This produces less trivial critical curves of \(\mathcal {S}_1\). Particular instances of ruled surfaces known as developable surfaces have identically zero Gaussian curvature.

5.3 The Torsion Functional

Using the results of Sect. 5.1 to compute (11), we expand the curvature tensor

$$\begin{aligned} R^f(B^f, N^f)T^f = R^f \left( \displaystyle \frac{\partial _t}{f}, N \right) T = -\frac{1}{f} \frac{H^f(N,T)}{f} \partial _t \end{aligned}$$
(34)

Writing \(\nabla f\) in the orthonormal basis \(\{T, N \}\), the Hessian can be put [5]

$$\begin{aligned} H^f(N,T) = \kappa \langle \nabla f, T \rangle + \langle \nabla f, N \rangle '. \end{aligned}$$
(35)

where the angular brackets indicate the scalar product on the slice. In this way, (11) becomes

$$\begin{aligned} \frac{1}{f^2} (\kappa \langle \nabla f, T \rangle + \langle \nabla f, N \rangle ') \partial _t + \frac{1}{f} \epsilon _1 \kappa ' \partial _t = 0, \end{aligned}$$
(36)

and, making a few manipulations, we arrive at the proposition

Proposition 7

Consider a three-dimensional standard static spacetime and a curve \(\gamma _t = (t, \gamma (t))\). \(\gamma _t\) is a critical point of \(\mathcal {S}_2\) if and only if

$$\begin{aligned} \epsilon _1 \kappa ' + \frac{1}{f} \langle \nabla f, T \rangle \kappa + \frac{1}{f} \langle \nabla f, N \rangle ' = 0. \end{aligned}$$
(37)

holds.

Furthermore, it can be shown that

Proposition 8

In a three-dimensional standard static spacetime, any curve \(\gamma _t(s)=(t,\gamma (s))\) is solution of equation (37) if and only if it satisfies

$$\begin{aligned} \tilde{\kappa } = \frac{C}{f^2} \end{aligned}$$
(38)

where \(\tilde{\kappa }\) is the curvature of \(\gamma \) with respect to the metric \(\tilde{g} = e^{-2 \ln \, f} g\) and C is a constant. Hence, for each \(C\in \mathbb {R}\) there exist critical points of \(\mathcal {S}_2\) obeying (38). In particular, the geodesics of the conformal metric \(\tilde{g}\) satisfy the equations of motion (by considering \(C=0\)).

We remark that the above is an existence result for non-trivial critical points of \(\mathcal {S}_2\) on three-dimensional standard static spacetimes.

6 Discussion

  • Of the preceding findings, Propositions 5 and 6, concerning critical points of the curvature functional on standard static spacetimes and of the torsion functional on GRW spacetimes, have the remarkable property that they provide trajectories which do not depend on the warping function. In other words, such critical points can be directly known from the geometry of the slice.

  • We may check if the results are consistent. For instance, if the warping function is constant, both GRWs and standard static spacetimes reduce to the same Lorentzian product (except for at most a constant factor in the metric). Thus, the critical curves of the form \(\gamma _t = (t, \gamma (s))\) when \(\dot{f}=0\) should be the same in the two cases for a given functional. Concerning the curvature functional, this is obvious since the conditions a critical curve must meet in Proposition 3, which assumes \(\dot{f}=0\), coincide with those of Proposition 6 for any value of \(\dot{f}\). For the case of the torsion functional, Proposition 5, again independently of \(\dot{f}\), states that we should look for curves with \(\kappa '=0\). If we now set \(\nabla f = 0\) in (37) of Proposition 7, we again find the requirement \(\kappa '=0\).