The Transfer Impedance of Capacitive Electrodes and Insulated Wires on the Ground Surface

Abstract

This article presents a formula for the capacitance of a thin disk over a conducting plane corrected for the edge effects and offers a number of generalized expressions for assessing the transfer impedance of capacitive electrodes over ground with finite conductivity. It is shown that the transfer impedance of an insulated wire on the ground in a wide frequency range is described by the Cole–Cole formula with an exponent parameter from 0.9 to 1.

INTRODUCTION

The method of capacitive excitation and reception of an electric field in the ground was proposed almost half a century ago and has since been actively used in low-frequency electrical exploration (Timofeev, 1974; Nakhabtsev et al., 1985; Grard and Tabbagh, 1991; Shima et al., 1996; Dashevsky et al., 2005; Kuras et al., 2006; Przyklenk et al., 2016; Gruzdev et al., 2020; Shlykov et al., 2020). Parasitic capacitive effects can greatly distort the results of measuring the electric field with conventional galvanic receiving lines, especially when working in conditions of poor electrode grounding (Zonge and Hughes, 1985; Zorin and Yakovlev, 2021). In this regard, the refinement of the expressions used to estimate the magnitude of the transient impedance of capacitive electrodes and insulated lines is an urgent scientific problem (Hordt et al., 2013).

The classical approach to calculating the transfer impedance Z of a capacitive electrode assumes that the resistance of the ground to the flowing current is negligible. In this case, Z = 1/iωC, where i is the imaginary unit, ω is the circular frequency, and C is the capacitance, which for the simplest models can be expressed analytically (Kuras et al., 2006). However, in some situations, the resistivity of the ground also affects the transfer impedance of the capacitive electrode, as a result of which its dependence on frequency may deviate noticeably from the formula Z = 1/iωC. Therefore, for the correct calculation of the impedance, numerical methods should be used (Hordt et al., 2013). In the present work, we present a number of simple analytical formulas that allow obtaining approximate estimates of the magnitude and frequency dependence of the transfer impedance of various types of capacitive electrodes over the ground with finite conductivity.

MATERIALS AND METHODS

Grounding resistance of galvanic electrodes. We consider a grounded electrical line AB, which is supplied with voltage ΔU_AB. The flowing current is equal to I_AB = ΔU_AB/R_AB, where R_AB is the contact resistance or grounding resistance of the AB line. The voltage drop inside the copper wires and metal electrodes can be neglected; therefore, ΔU_AB = U_A – U_B where U_A and U_B are the electric field potentials on the surface of electrodes A and B, respectively. If the dimensions of the electrodes are much smaller than the distance between them, the potential of each of them does not depend either on this distance or on the parameters of the other electrode and, therefore, is equal to the potential of a similar solitary electrode.

Thus, provided that the distance between the electrodes is many times greater than their dimensions R_AB = R_A + R_B, where R_A = U_A/I_A and R_B = U_B/I_B are the grounding resistances of A and B solitary electrodes, respectively (hereinafter, the direction of the current flowing from the solitary electrode is taken as positive).

The contact resistance of a solitary hemispherical electrode of radius r in a medium with resistivity ρ can be found as follows. Due to spherical symmetry (Fig. 1), at any point M of the earth’s surface, which is at a distance x ≥ r from the center of the electrode, the electric current is directed strictly radially and is characterized by the density j = I/2πx². According to Ohm’s law, the electric field at a given point is directed in exactly the same way and has a strength of E = jρ = Iρ/2πx². The potential U at the point M is uniquely determined from the equation E = –grad(U) = –dU/dx and the boundary condition U(∞) = 0, whence U(x) = Iρ/2πx. Thus, the contact resistance of a hemispherical electrode is R = U(r)/I = ρ/2πr.

Fig. 1.

An illustration for the derivation of the formula for the grounding resistance of a hemispherical electrode.

The calculation of the grounding resistance of non-spherical electrodes is a more difficult problem (Ollendorf, 1928; Wait, 1982). To solve it, it is convenient to use the methods and existing results of a well-developed theory of electrostatics, whose problems have a direct mathematical analogy with the problems of direct current distribution. In particular, using this analogy, it is easy to show (Smythe, 1950, §6.14; Bursian, 1972, §6) that the grounding resistance of an arbitrary electrode placed in a homogeneous half-space with resistivity ρ is equal to:

$$R = \frac{{2\rho \varepsilon }}{C} = \rho K,$$

(1)

where C is the capacitance of an electrode of the same shape (complemented upward by the symmetrical reflection of its grounded part) in a homogeneous space with permittivity ε.

Thus, the grounding resistance of any galvanic electrode is directly proportional to the resistivity of the medium in which it is placed. In this case, the geometric grounding factor K depends only on the configuration of the area of the earth’s surface in contact with the electrode (the smaller the characteristic dimensions of this area, the greater the value of K). From the previously obtained formula for the transfer resistance of a grounded hemisphere, it follows that for a hemispherical electrode of radius r, the geometric grounding factor is equal to:

$${{K}_{{{\text{HS}}}}}\left( r \right) = \frac{1}{{2\pi r}}.$$

(2)

For electrodes of a more complex shape, it is convenient to determine the value of K by applying formula (1) to the known solutions of the corresponding problems of electrostatics. For example, the capacity of a solitary disk represented as an infinitely oblate spheroid (Landau and Lifshitz, 1960, §4) or cylinder (Falco et al., 2003) is equal to 8εr, whence for a disk electrode of the radius r pressed against the earth’s surface, we obtain

$${{K}_{{\text{D}}}}\left( r \right) = \frac{1}{{4r}}.$$

(3)

Similarly, the capacity of a strongly prolate spheroid (Landau and Lifshitz, 1960, §4]) or cylinder (Jackson, 2000) is 2πεl/ln(l/r), from which the following estimates of the K value for a pressed to the ground bare wire of radius r and length l ⪢ r may be obtained:

$${{K}_{{\text{W}}}}\left( {r,l} \right) = \frac{1}{{\pi l}}\ln \left( {\frac{l}{r}} \right),$$

(4)

For a classic stake electrode with radius r and the length of the grounded part l ⪢ r:

$${{K}_{{\text{S}}}}\left( {r,l} \right) = \frac{1}{{2\pi l}}\ln \left( {\frac{{2l}}{r}} \right).$$

(5)

In conclusion, we note that the grounding resistance of a solitary electrode practically does not depend on the properties of the external environment outside its immediate vicinity, comparable to the characteristic dimensions of the electrode (Bursian, 1972, §8; Kaufman and Anderson, 2010, §2.10). Thus, all the above expressions are applicable not only to a homogeneous half-space, but also to real geological media with a fairly homogeneous upper layer (of course, provided that the rocks of this layer are in close contact with the entire working surface of the electrode).

Transfer impedance of capacitive electrodes. We consider the line AB connected to capacitive (i.e., located close to the ground, but not in direct galvanic contact with it) electrodes. The current flowing through it is I_AB = ΔU_AB/Z_AB, where Z_AB = Z_A + Z_B is called the transfer impedance of the line AB, and Z_A = U_A/I_A and Z_B = U_B/I_B is the transfer impedance of A and B solitary electrodes, respectively.

The formula for calculating the transfer impedance of a solitary capacitive electrode over a perfectly conducting half-space is as follows (Kuras et al., 2006):

$$Z = \frac{1}{{i\omega C}}.$$

(6)

The value of the electrode capacitance C is determined by the configuration of the electrode–ground system and, in some cases, can be expressed analytically. As the first example (not encountered in practice, but simple and illustrative), let us take a hemispherical electrode of radius r covered with an insulating layer of thickness d and pressed into the ground. For very small values of d, the capacitance of such an electrode is equal to half the capacitance of the corresponding spherical capacitor:

$${{C}_{{{\text{HS}}}}}\left( {r,d,\varepsilon } \right) = \frac{{2\pi {{r}^{2}}\varepsilon }}{d},$$

(7)

where ε is the dielectric constant of the insulating layer.

The capacitance of a disk electrode of radius r located above the ground at a low height h is usually estimated using the classical flat capacitor formula (Kuras et al., 2006; Hordt et al., 2013):

$${{C}_{{\text{D}}}}\left( {r,h} \right) = \frac{{\pi {{r}^{2}}{{\varepsilon }_{0}}}}{h},$$

(8a)

where ε₀ is the permittivity of air (vacuum). This expression does not take into account the influence of edge effects. A more precise formula for a thin disk of finite dimensions at k = h/r ⪡ 1 can be written as follows (see Appendix):

$$C_{{\text{D}}}^{*}\left( {r,h} \right) = \frac{{\pi {{r}^{2}}{{\varepsilon }_{0}}}}{h}\left[ {1 + \frac{{4k}}{\pi }\left( {k - \ln ek} \right)} \right].$$

(8b)

It follows from the above expressions that in the general case $C_{{\text{D}}}^{*}$ > C_D; however, the difference between these two estimates decreases as the disk approaches the ground and tends to zero as k → 0. In the most important from a practical point of view range of k from 1/5 to 1/50, formula (8b) gives the average value $C_{{\text{D}}}^{*}$ → 1.15C_D, which is in excellent agreement with the known numerical estimates of the edge-effect influence on the capacitance of an elementary thin plate above a conducting plane (Sakurai and Tamaru, 1983; Kuras et al., 2006).

Finally, the capacitance of a thin long wire of radius r raised above the ground by a small height (h) compared to its length (l) is (Smythe, 1950, §4.14):

$${{C}_{{\text{W}}}}\left( {r,l,h} \right) = \frac{{2\pi l{{\varepsilon }_{0}}}}{{\text{arccosh} \left( {\frac{h}{r}} \right)}}.$$

(9)

Correction for ground resistance. Since the earth resistivity is negligible compared to the effective resistivity of the air, it can be assumed that the entire current from the capacitive electrode flows into the ground and only then spreads over the ground to “infinity.” If, in this case, the area S of the earth’s surface, through which the current enters the lower half-space, is close to equipotential, then the transfer impedance of the electrode decomposes into the sum of the reactance of the air and the galvanic resistance of the earth. Since the latter has the form (1), it is natural to search for the generalized expression (6) for the impedance of a capacitive electrode over a half-space with resistivity ρ in the form:

$$Z = \frac{1}{{i\omega C}} + \rho G.$$

(10)

The value of G plays the role of the geometric “grounding” factor of the capacitive electrode and, therefore, is determined by the configuration of the area S (the smaller its characteristic dimensions, the greater the value of G). As the electrode approaches the ground, its capacitance increases, and the dimensions of the S region decrease, which leads to an increase in the relative contribution of the correction term ρG. We emphasize that S in the general case can not always be considered an equipotential surface, so the representation of the impedance in the form (10) with frequency-independent parameters C and G is only a convenient first approximation, whose accuracy for each type of electrode should be checked separately using analytical or numerical evaluations.

As a rule, the active resistance of the earth makes an insignificant contribution to the total value of the transfer impedance, and it is customary to neglect it (Shima et al., 1996; Kuras et al., 2006). However, in some cases it can reach and even exceed the air reactance, which can be easily shown using the following thought experiment. We consider again a hemispherical electrode of radius r, grounded in a homogeneous conducting half-space with resistivity ρ (Fig. 2a). As was shown earlier, its contact resistance is R = ρ/2πr. If we remove the electrode from the ground, apply an insulating layer of thickness d ⪡ r on its surface, and place it back into the ground (Fig. 2b), application of the formulas (6), (7) to estimate the impedance of the resulting capacitive electrode gives Z_HS = d/2πr²iωε, which cannot be true for ρ ≠ 0, because it leads to paradoxical results. Indeed, at very small d, the value d/2πr²iωε tends to zero, whence it would follow that the deposition of a sufficiently thin insulating layer on a grounded electrode does not increase, but, on the contrary, reduces its contact resistance.

Fig. 2.

A diagram of a theoretical experiment with a hemispherical electrode. The letters indicate the models: grounded electrode (a), capacitive electrode (b) and an equivalent grounded capacitor model (c).

For a correct assessment of Z_HS, we replace the electrode model under consideration with an equivalent model of a hemispherical capacitor immersed in the ground (Fig. 2c). For small d, the capacitance of such a capacitor is d/2πr²ε = C_HS(r, d, ε), grounding resistance of the outer capacitor plate in accordance with formulas (1) and (2) is equal to ρK_HS(r + d) ≈ ρK_HS(r), whence for the total impedance of the circuit we finally obtain:

$${{Z}_{{{\text{HS}}}}} = \frac{1}{{i\omega {{C}_{{{\text{HS}}}}}\left( {r,d,\varepsilon } \right)}} + \rho {{K}_{{{\text{HS}}}}}\left( r \right).$$

(11)

We note that the same result can be obtained using the expression for the contact resistance of a hemispherical electrode of radius r surrounded by a concentric layer with resistivity ρ₁ and thickness d (Bursian, 1972, §8; Wait, 1982):

$$R = \frac{{{{\rho }_{1}}d}}{{2\pi r\left( {r + d} \right)}} + \frac{\rho }{{2\pi \left( {r + d} \right)}}.$$

(12)

Indeed, taking into account the fact that in the model under consideration d ⪡ r, substitution of the effective resistivity of the insulator ρ₁ = (iωε)^–1 into formula (12 ) gives (11).

Let us find a generalized expression for the transfer impedance Z_W of a thin long wire with a radius r ⪡ h and a length l ⪢ h raised to a height h above the ground. To do this, we first assume that the resistivity of the ground ρ coincides with the effective air resistivity ρ₀ = 1/iωε₀, i.e., that the wire is located in empty space, and consider the behavior of the potential U₀ at a distance x ⪡ l from the axis of the wire. Due to cylindrical symmetry (Fig. 3a) at any point M, located at a distance x ≥ r from the wire, the current density is equal to j₀ = I/2πlx, whence E₀ = Iρ₀/2πlx. The potential at the point M is uniquely determined from the equation E₀ = –dU₀/dx and boundary condition U₀(r) = I/iωC = (Iρ₀/2πl)ln(l/r), where C = 2πε₀l/ln(l/r) is the given above capacitance of a long wire in vacuum, from where U₀(x) = (Iρ₀/2πl)ln(l/x).

Fig. 3.

An illustration for the derivation of the formula for the transfer impedance of a thin long wire raised above the ground. See text for explanations.

Let us return to the original problem where ρ ⪡ ρ₀. An abrupt change in the resistivity of the medium at the air–ground boundary leads to the appearance of secondary charges on it, which change the structure of the electric field in the air (Fig. 3b). To determine the value of the additional potential ΔU caused by these charges on the wire surface, we use the method of mirror reflections, according to which (Grard and Tabbagh, 1991; Kaufman and Anderson, 2010, §3.6):

$$\Delta U = \frac{{\rho - {{\rho }_{0}}}}{{\rho + {{\rho }_{0}}}}{{U}_{0}}\left( {2h} \right) = \left[ {\frac{{2\rho }}{{\rho + {{\rho }_{0}}}} - 1} \right]\frac{{I{{\rho }_{0}}}}{{2\pi l}}\ln \frac{l}{{2h}}.$$

(13)

As (ρ + ρ₀) ≈ ρ₀ = 1/iωε₀, the total potential of the wire U_W is equal to:

$$U = {{U}_{0}}\left( r \right) + \Delta U = \frac{1}{{i\omega \times 2\pi l{{\varepsilon }_{0}}}}\ln \frac{{2h}}{r} + \frac{{I\rho }}{{\pi l}}\ln \frac{l}{{2h}}.$$

(14)

Since ln(2h/r) ≈ arccosh(h/r), taking into account (4), (6), and (9), we finally obtain

$${{Z}_{{\text{W}}}} = \frac{{{{U}_{{\text{W}}}}}}{I} = \frac{1}{{i\omega {{C}_{{\text{W}}}}\left( {r,l,h} \right)}} + \rho {{K}_{{\text{W}}}}\left( {2h,l} \right).$$

(15)

In conclusion, we will try to determine the coefficients of formula (10) that are best suited for the transfer impedance Z_D of a thin disk of radius r raised to a height h = kr above the ground with resistivity ρ. To do this, we note that for vanishingly small values of k the desired formula must satisfy the following limit conditions: Z_D = 1/iωC_D(r, h) for ρ → 0 and Z_D = K_D(r) ρ with h → 0, whence

$${{Z}_{{\text{D}}}} = \frac{1}{{i\omega {{C}_{{\text{D}}}}\left( {r,h} \right)}} + \rho {{K}_{{\text{D}}}}\left( r \right).$$

(16a)

Formula (16a) can be naturally generalized to the case 0 ≤ k ⪡ 1 by replacing C_D with $C_{{\text{D}}}^{*}$ and r with r*:

$$Z_{{\text{D}}}^{*} = \frac{1}{{i\omega C_{{\text{D}}}^{*}\left( {r,h} \right)}} + \rho {{K}_{{\text{D}}}}\left( {r{\text{*}}} \right),$$

(16b)

where r* = $\sqrt {{{C}_{{\text{D}}}}{\text{*/}}{{C}_{{\text{D}}}}}$ is the effective radius of the area S, through which the current flowing from both sides of the disk electrode raised into the air enters the ground.

Numerical simulation. To estimate the accuracy of formula (16b), numerical simulation was performed in the Disc5 program (P. Weidelt), which allows one to calculate the value of the transfer impedance of a thin disk of radius R raised to an arbitrary height h above the ground with an effective resistivity ρ = ρ_DC/(1 + iωερ_DC) (Hordt et al., 2013).

Simulation results for R = 0.2 m and different values of ρ_DC, ε and h are shown in Fig. 4. The solid red line in the figure shows the impedance modulus curves obtained using the Disc5 software; the black dotted line shows the results of applying the classical flat capacitor formula (6, 8a); finally, the black dashed line shows the results of applying the modified formula (16b) for a disk above the ground with an effective resistivity ρ = ρ_DC/(1 + iωερ_DC).

Fig. 4.

Comparison of classical and modified formulas for estimating the transfer impedance of a disk electrode with numerical calculations in the Disc5 software (P. Weidelt). The electrical properties of the lower half-space and the height of the electrode above it are shown in the figure, the radius of the electrode in all cases is 20 cm. See the text for explanations.

The first series of graphs (Figs. 4a–4c) allows one to compare the behavior of $\left| {Z_{{\text{D}}}^{*}} \right|$ for different values of ρ_DC = 1 kOhm, 10 kOhm, 100 kOhm. As seen in Figs. 4a–4c, the left branches of all curves lie on the asymptote 1/ω$C_{{\text{D}}}^{*}$(r, h); however, starting from a certain moment, the graphs flatten out, practically ceasing to decrease with frequency. This indicates that the first term in formula (16b) becomes less than the second and the value of $Z_{{\text{D}}}^{*}$ begins to be determined not by the reactance of the electrode–ground system, but by the galvanic resistance of the lower half-space, which at ρ ≈ ρ_DC is frequency independent. Since the ratio of the second term to the first is proportional to ωρ, an increase in ρ_DC of the model by 10 times, as expected, leads to the plot $\left| {Z_{{\text{D}}}^{*}} \right|$ deviating from the low-frequency asymptote by a decade earlier (Figs. 4b and 4c). With a further increase in frequency, sooner or later, the displacement currents begin to dominate in the ground: ρ ≈ 1/iωε, as a result of which the impedance graphs go to their right asymptote, also inversely proportional to frequency. It is important to note that all the curves obtained by formula (16b) practically coincide with the results of the corresponding numerical calculations, except for high-frequency asymptotes, where they give slightly overestimated values, the reasons for which are considered below.

The second series of graphs (Figs. 4d–4f) allows one to compare the behavior of $\left| {Z_{{\text{D}}}^{*}} \right|$ for different values of ε: 10ε₀, 3ε₀, ε₀. As seen in Figs. 4d–4f, the smaller ε the lower is the accuracy of the impedance estimate in the region of the highest frequencies. This is due to the violation of the condition ρ ⪡ ρ₀ used in the generalized formula (10), which for high frequencies is equivalent to the condition ε ⪢ ε₀. At ε ~ ε₀, part of the current propagates to “infinity” directly through the air, thereby reducing the actual value of the contact impedance. However, as follows from Fig. 4, for majority of real rocks with ε around 10ε₀ this effect does not lead to significant distortion.

The third series of graphs (Figs. 4g–4i) clearly confirms the earlier conclusion that the difference in the frequency dependence of the capacitive electrode impedance from formula (6) is the greater, the closer the electrode to the ground. In addition, it allows us to estimate the practical limits of the condition k = h/R ⪡ 1 for formulas (6, 8a) and (16b). At h = 0.1 cm (k = 0.005) in the low-frequency region, both formulas practically coincide with numerical calculations. At h = 1 cm (k = 0.05), the left asymptote for formula (16b) remains valid, while for formula (8a) that does not take into account the edge effects, it turns out to be overestimated by ~15%. Finally, for h = 10 cm (k = 0.5), any estimates based on the condition k ⪡ 1 give significantly (>30%) overestimated impedance values over the entire frequency range.

Thus, the proposed in the article approach to asses and take into account the influence of the finite conductivity of the earth on the transfer impedance of the disk electrode is fully consistent with the results of numerical simulation.

Capacitive electrodes with distributed parameters. Systems with distributed parameters, which, for example, include insulated lines (Veshev, 1980, §3.2), are of particular interest from the point of view of the influence of earth resistance on the transfer impedance of capacitive electrodes. If the impedance Z_i of each elementary section of such a line is described by the formula Z_i = 1/iωC_i + R_i, where C_i is capacitance of the i-th section of the wire, and R_i is the galvanic resistance of the corresponding region of the lower half-space, then the total impedance of the wire is equal to:

$$Z = {{\left( {\sum\limits_i {\frac{{i\omega {{C}_{i}}}}{{1 + i\omega {{R}_{i}}{{C}_{i}}}}} } \right)}^{{ - 1}}}.$$

(17)

Using auxiliary variables $\tilde {R}$ = 1/Σ_i$R_{i}^{{ - 1}}$, γ_i = $\tilde {R}$/R_i and τ_i = R_iC_i it is convenient to rewrite formula (17) in the following form:

$$Z = \tilde {R}{{\left( {\sum\limits_i {{{\gamma }_{i}}\frac{{i\omega {{\tau }_{i}}}}{{1 + i\omega {{\tau }_{i}}}}} } \right)}^{{ - 1}}},$$

(18a)

$$\sum\limits_i {{{\gamma }_{i}}} = 1.$$

(18b)

In the expression given in parentheses, it is easy to recognize the kernel of the discrete version of the generalized Debye formula (Debye decomposition), which is widely used in the modern induced polarization method (Patella, 2003; Nordsiek and Weller, 2008; Zorin, 2015). An important feature of the Debye decomposition is that it is the most general of all possible forms of representation of the frequency characteristics of relaxation systems (Shuey and Johnson, 1973) and in many practical cases can be replaced by simpler empirical and semi-empirical models (Pelton et al., 1983). The most famous example of such a model is the Cole–Cole formula (Cole, K.S. and Cole, R.H., 1941), which, when applied to expression (18), gives the following estimate of the insulated line transfer impedance:

$$Z = \tilde {R}{{\left( {\frac{{{{{\left( {i\omega {{\tau }_{0}}} \right)}}^{\alpha }}}}{{1 + {{{\left( {i\omega {{\tau }_{0}}} \right)}}^{\alpha }}}}} \right)}^{{ - 1}}} = \frac{{\tilde {R}}}{{{{{\left( {i\omega {{\tau }_{0}}} \right)}}^{\alpha }}}} + \tilde {R},$$

(19)

where the mean value of τ_i is characterized by the time constant τ₀, and the variance of its distribution is characterized by the difference from unity of the parameter α ∈ [0; 1]. If ωτ₀ ⪡ 1, formula (19) is additionally simplified by discarding the second term and introducing the additional notation X = τ₀$\tilde {R}$^–1/α, which is more convenient for describing the impedance of the Cole–Cole element (Pelton et al., 1983), whence:

$$Z = \frac{1}{{{{{\left( {i\omega X} \right)}}^{\alpha }}}}.$$

(20)

RESULTS AND DISCUSSION

Field measurements. It has been shown above that the transfer impedance of a real insulated line on a non-uniform ground surface is better described using a Cole–Cole element than a simple capacitor. To test this statement, in May 2021 at the Aleksandrovka geophysical field camp of Lomonosov Moscow State University (Aleksanova et al., 2018), we conducted the following field experiment.

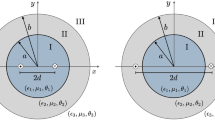
On a field covered with short grass, with a soil resistivity of about 0.5 kOhm m, a set up was installed, the scheme of which is shown in Fig. 5. A long line, which was a 50-m piece of insulated copper wire grounded at the far end through a resistor of R₀ = 1 MOhm, was connected to one of the terminals of the impedance meter. The second terminal was grounded in close proximity to the device without an additional resistor (the grounding resistance of the second electrode was <1 kOhm, so its effect on the transfer impedance of the entire system can be neglected). To exclude possible galvanic leakage through microdamages of the insulation, a piece of wire used for the long line was taken from a new package for the purity of the experiment. As an impedance meter was used the electromagnetic receiver NORD (Nord-West Ltd.), which has the capability to carry out a broadband assessment of the transfer impedance of the connected lines with an error of less than 1% of the amplitude and 1° phase in the range of values from 1 kOhm to 1 MOhm.

Fig. 5.

The scheme of the setup for the experiment on measuring the transfer impedance of a real insulated line ~50 m long in a wide frequency band.

The classical equivalent circuit (Zonge and Hughes, 1985) for the setup used in the experiment is shown in the lower left corner of Fig. 5. In this circuit, the capacitive current leakage from the wire is modeled using an ideal capacitor, and the effective contact impedance of the entire line becomes the following:

$$Z = {{R}_{0}}\frac{1}{{1 + i\omega {{R}_{0}}C}}.$$

(21)

The modified equivalent circuit is shown in the lower right corner of Fig. 5. In this scheme, the capacitor is replaced by a Cole-Cole element and the earth resistance to the capacitive current flowing from the wire is added, which gives the following impedance formula:

$$Z = {{R}_{0}}\frac{{1 + \tilde {R}{{{\left( {i\omega X} \right)}}^{\alpha }}}}{{1 + \left( {{{R}_{0}} + \tilde {R}} \right){{{\left( {i\omega X} \right)}}^{\alpha }}}}.$$

(22)

The field experiment was carried out in dry cloudy weather at a temperature of 15–20°C and consisted of three multifrequency measurements of the transfer impedance Z, which were then approximated using formulas (21) or (22). Before the first measurement, the long line laid out on the grass was trampled under foot, as a result of which the average distance from the wire to the ground was about 1–2 cm. Before the second measurement, the line wire was additionally spilled with fresh water to simulate operation in wet weather. Finally, before the third measurement, the long line was buried in the ground to a shallow (up to 5 cm) depth, which is a common fieldwork practice in the magnetotelluric method (Chave and Jones, 2012, §9.2).

The results of the approximation of the obtained data using the classical formula (21) are shown in Fig. 6. The strong dependence of the capacitive leakage on the experimental conditions is most noticeable. Thus, the selected values of the effective capacitance of the long line were ~2 nF for a dry wire, ~4 nF for a wet wire, and ~20 nF for a buried wire. Interestingly, taking into account the parameters of the wire used, formula (9) gives the maximum achievable capacitance values of 3–4 nF. This in particular suggests that to estimate the capacitance of insulated lines according to formula (9) in wet weather, the outer radius of the wire (r + d) can be taken as the height h, where d is the insulation thickness. To estimate the capacitance of the buried line, instead of (9), the formula for a cylindrical capacitor should be used (Smythe, 1950, §2.04):

$$C = \frac{{2\pi L}}{{\ln \frac{{r + d}}{r}}}{{\varepsilon }_{1}},$$

(23)

which gives better agreement with experiment values of about 15–20 nF.

Fig. 6.

The results of approximation of the measured data with an equivalent circuit in which capacitive leakage from a wire is modeled by an ideal capacitor.

The second important conclusion from the Fig. 6 data is in the fact that the classical formula (21) accurately describes only the results of the experiment with a dry wire on the grass. Indeed, for a wet wire, the calculated fitting error exceeds the instrumental accuracy by several times, and for a buried wire, even by an order of magnitude. The reason for such poor curve fitting was that in the last two tests the line impedance decreased with frequency much more slowly than ~1/ω, which cannot be modeled within the framework of a classical single-capacitor circuit.

The results of data approximation using the modified formula (22) are shown in Fig. 7. For all measurements, the fitting error is close to the instrumental accuracy, which suggests the correctness of the proposed equivalent circuit. In this case, the obtained values of the power parameter α for dry, wet, and buried wires were ~0.98, ~0.97, and ~0.93, respectively.

Fig. 7.

The results of the approximation of the measured data using an equivalent circuit in which the capacitive leakage from the wire is modeled by the Cole-Cole element.

CONCLUSIONS

In electrical exploration, it is generally accepted that the transfer impedance of an arbitrary capacitive electrode is a function of the form Z = 1/iωC with a frequency-independent coefficient C. This statement is based on the assumption that the resistance of the lower half-space is negligible. In many practical situations, this assumption is absolutely justified, but if the electrode is located close to the underlying medium (especially in wet weather), then its resistance can make a significant contribution to the transfer impedance of the electrode. In such cases, extensive sections appear on the graph of the dependence of the transfer impedance modulus on frequency, within which it decreases more slowly than ~1/ω.

For a quantitative description of these effects, the authors proposed a number of approximate formulas for calculating the transfer impedance of capacitive electrodes over a medium with a known resistivity. In this study, an expression refined for the influence of edge effects was also obtained for the capacitance of a thin disk over a conducting plane. Finally, it is shown that to approximate the transfer impedance of real insulated lines, it is more correct to use equivalent circuits with a Cole–Cole element instead of an ideal capacitor.

APPENDIX

We present the derivation of the usual (8a) and refined (8b) formulas for the capacitance of a thin disk of radius r located at a low height h = kr above a conducting half-space (earth). For this purpose, we assume that the earth potential is equal to zero, and the potential of the disk electrode is equal to φ_D. By definition, the capacity of a disk is C_D = q/φ_D, where q is the total induced charge on its surface.

If we neglect the influence of edge effects, i.e., assume that the electric field in the air is uniform and exists only between the disk and the ground, then the total disk charge q₀ is distributed uniformly over its lower surface, whence q₀ = σ₀πr², where σ₀ is the surface charge density. The earth charge of opposite sign –q₀ is also distributed uniformly on the area of the daylight surface under the disk and is characterized by the density σ₀. Since the elementary surface charge σ₀ leads to the appearance of a normal field component in air E_n = σ₀/2ε₀ (Kaufman and Anderson, 2010, §1.41), the total field over the disk is equal to σ₀/2ε₀ – σ₀/2ε₀ = 0, and under the disk, it is equal to E = σ₀/2ε₀ + σ₀/2ε₀ = σ₀/ε₀. Due to the homogeneity of the field at any point between the disk and the ground E = φ_D/h, whence σ₀ = φ_Dε₀/h, and for the capacitance C_D of the disk electrode, we obtain formula (8a):

$${{C}_{{\text{D}}}} = \frac{{{{q}_{0}}}}{{{{\varphi }_{{\text{D}}}}}} = \frac{{{{\sigma }_{0}}\pi {{r}^{2}}}}{{{{\varphi }_{{\text{D}}}}}} = \frac{{\pi {{r}^{2}}{{\varepsilon }_{0}}}}{h}.$$

(A1)

A real disk electrode of finite dimensions is characterized by edge effects, which manifest themselves in the fact that the field in air exists not only under, but also above the disk (Fig. 8a). We consider the point M on the upper side of the disk, which is at a distance x from its edge (Fig. 8b). The existence of the normal component of the electric field E_n(x) directly above the disk indicates that it has an additional charge Δ_q with density Δσ(x) = E_n(x)2ε₀. If you do not come close to the edge of the disk (Fig. 8c), then its elevation above the ground can be neglected, and the task of determining E_n(x) is reduced to determining the field at the contact of two flat surfaces with different potentials. As shown in (Landau and Lifshitz, 1960, §22), in such a model the field lines are arcs of circles centered at the point of contact, along which the potential varies linearly. Drawing such an arc through the point M (Fig. 8b), we obtain that

$${{E}_{n}}\left( x \right) = - \frac{{d\varphi \left( {\alpha ,x} \right)}}{{dn\left( {\alpha ,x} \right)}} = \frac{{\frac{{{{\varphi }_{{\text{D}}}}}}{\pi }d\alpha }}{{xd\alpha }} = \frac{{{{\varphi }_{{\text{D}}}}}}{{\pi x}},$$

(A2)

whence the surface density of the additional charge at the point M is equal to

$$\Delta \sigma \left( x \right) = \frac{{2{{\varepsilon }_{0}}{{\varphi }_{{\text{D}}}}}}{{\pi x}}.$$

(A3)

Fig. 8.

An illustration for the derivation of the formula refined for the influence of edge effects for the capacitance of a thin disk over a conducting half-space. See text for explanations.

To estimate Δq, we integrate Δσ over the entire disk surface, minus a thin strip of width h along its edge, where E_n is not described by the equation (A2):

$$\begin{gathered} \Delta q = \int\limits_0^{2\pi } {\int\limits_0^{r - h} {\Delta \sigma \left( {R - \tau } \right)\tau d\tau d\theta } } \\ = 4{{\varepsilon }_{0}}{{\varphi }_{{\text{D}}}}\left( { - r\ln \frac{h}{r} - r + h} \right). \\ \end{gathered} $$

(A4)

Let us rewrite equation (A4) using the substitution h = kr:

$$\begin{gathered} \Delta q = 4{{\varepsilon }_{0}}{{\varphi }_{{\text{D}}}}r\left( { - \ln k - 1 + k} \right) \\ = 4{{\varepsilon }_{0}}{{\varphi }_{{\text{D}}}}r\left( {k - \ln ek} \right). \\ \end{gathered} $$

(A5)

Finally, dividing the total charge of the disk q = q₀ + Δq by its potential φ_D, we obtain the disk electrode capacitance formula (8b) refined for the influence of edge effects:

$${{C}_{{\text{D}}}} = \frac{{{{q}_{0}} + \Delta q}}{{{{\varphi }_{{\text{D}}}}}} = \frac{{\pi {{r}^{2}}{{\varepsilon }_{0}}}}{h}\left[ {1 + \frac{{4k}}{\pi }\left( {k - \ln ek} \right)} \right].$$

(A6)