INTRODUCTION

A characteristic distinguishing feature of composites is the nonadditivity of the contributions of components to its properties (emergence) [1–3]. Structural element of the composite, in which there is a formation of such systemic effects, is the phase boundary “matrix material–dispersed phase.” The interaction of components at this boundary leads to the appearance in the matrix material of a phase that differs in structure parameters and properties from the matrix material in the bulk (far from the interface phases). In some information sources, such a phase was called the “film phase” (see, for example, [4, 5]). The presence of such a phase in the matrix material affects not only the structure-sensitive properties of the composite (mechanical, deformation properties, resistance to operational factors), but also its volume [6].

The work of Rusanov [7] showed that the change in the properties of a liquid (matrix material) in contact with a solid surface (dispersed phase) under otherwise equal conditions depends on the van der Waals interaction constant

$$\rho = {{\rho }_{0}} + \frac{{\pi \rho _{0}^{2}(A_{B}^{'}\rho {\kern 1pt} '\, - {{A}_{B}}{{\rho }_{0}})}}{6}\frac{1}{{{{h}^{3}}}},$$
(1)

where \(A_{B}^{'}\) and AB are the constants of the van der Waals interactions of liquid molecules with a solid surface and between liquid molecules, ρ0 and ρ′ are the densities of the liquid and the solid surface, and h is a distance from the solid surface.

Equation (1) not only demonstrates that, in the presence of interaction between liquid molecules (matrix material) and the surface of a solid body (dispersed phase), a layer is formed with different physical properties (film phase of the matrix material), but it also makes it possible to calculate its thickness. This makes it possible to implement a new principle for the design of composites based on the formation of a synergistic effect owing to the overlap of the layers of the film phase of the matrix material formed on the dispersed phase of the composite.

The key element of this method of designing composites is to establish the value of the van der Waals interaction constant. In the theory of Deryagin, Landau, Verwey, and Overbeck (DLVO theory) [8], there is a directly proportional relationship of the constant of van der Waals interaction to the Hamaker constant:

$$A = \frac{{{{\tau }^{2}}N_{{\text{A}}}^{2}}}{{{{V}_{m}}}}{{A}_{B}},$$
(2)

where A is the Hamaker constant, Vm is the molar volume, and NA is the Avogadro constant.

On the basis of Eq. (2), the Hamaker constant can be a criterion for assessing the correctness of the choice of disperse components, the composition of which contributes to the manifestation of a systemic synergistic effect. The scientific foundation for such an approach can be the theory of molecular interaction between macrobodies proposed by Deryagin et al. [9]. So, to characterize the forces of molecular (dispersion) interaction in condensed systems, the constant A proposed by H.C. Hamaker (Hamaker constant) is used. According to [10, 11], the Hamaker constant characterizes the interaction energy of particles separated by an interlayer of the third phase. However, with this classical approach, performing an experiment to determine A is a rather difficult task. At the same time, obtaining the Hamaker constant as an estimated quantitative criterion characterizing, for example, the enhancement of the dispersion interaction is not only an interesting scientific problem but also a problem of great practical importance.

The practical implementation of such a solution can be associated with the initial stage of the selection of raw materials, which consists in the experimental determination of the Hamaker constant of the composition of the raw mixture, the combination of components in which gives the maximum value of A. So, if we designate the Hamaker constant of the composition as Acom and a similar parameter, for example, for two assumed raw materials components Аx and Аy, then, on the basis of [8], the following mathematical expression (3) is true:

$${{A}_{{{\text{com}}}}} \approx {{A}_{{xy}}} = \sqrt {{{A}_{x}}{{A}_{y}}} .$$
(3)

The value of the Hamaker constant for some substances (and systems) can be found in the reference literature, for example, [10, 12, 13]. However, in our opinion, the developed theoretical provisions of the physical chemistry of surface phenomena [8] allow us to successfully apply the method of calculating the Hamaker constant based on determining and comparing the equilibrium wetting angle (θ0) of the surface of the analyzed material with reference (with a known value of surface tension, σlic) working fluids. In this case, it should be noted that this approach makes it possible to determine not the absolute value of the desired value, but the possible analogous value of the constant. Thus, according to the Frumkin–Deryagin equation, the equilibrium wetting angle is related to the disjoining pressure, П(h), arising in the liquid film, by the following mathematical formula:

$$\cos {{\theta }_{0}} = 1 - \frac{1}{{{{\sigma }_{{{\text{lic}}}}}}}\int\limits_{{{h}_{{\min }}}}^\infty {\Pi (h){{d}_{h}},} $$
(4)

where hmin is the smallest film thickness that corresponds to the van der Waals distance (0.24 nm) [14].

However, it should be noted that, in the theory of B.V. Deryagin, the dependence of the wetting angle on the value of the disjoining pressure (4) was determined for the case of a liquid film located between the plates of a solid body. In the proposed approach, in contrast to the classical version, the interaction of a liquid drop with a surface formed by compacted fine particles is considered. However, despite this difference, the experimental results obtained give grounds to apply the mathematical apparatus created in the theory of molecular interaction to this system. It should also be noted that there are objective experimental difficulties in reproducibly determining the equilibrium wetting angle of a liquid drop on the surface of a solid body in the transition zone between the bulk liquid and a flat equilibrium film with a thickness hmin (transition of the liquid profile into a flat equilibrium film). Note that the formation of a thin film of a wetting liquid on the surface of a solid that is in equilibrium with the bulk phase is not always observed. This fact is explained by the difference in the disjoining pressure isotherms, which, in turn, are determined by the nature of the surface forces acting in the system under consideration [15]. At the same time, the wetting angle of the same surface by a film and the bulk phase of a liquid is determined only by the physicochemical properties of the liquid itself (at constant external factors); therefore, in our studies, to characterize the wetting process (disregarding at this stage of research the characteristics of a thin film), we introduce the concept of an analog of the Hamaker constant (Am) determined by the equilibrium wetting angle of the surface by the bulk phase of a liquid drop. Taking into account the equation proposed by Hamaker for the attractive force (dispersion interaction) between bodies having the shape of a plane for a unit surface \(\left( { - \frac{A}{{6\pi {{h}^{3}}}}} \right)\) [9] and the functional relationship between the disjoining pressure and the dispersion interaction [12], Eq. (4) takes the following form:

$$\cos {{\theta }_{0}} = 1 + \frac{{{{A}_{m}}}}{{6\pi {{\sigma }_{{{\text{lic}}}}}}}\int\limits_{{{h}_{{\min }}}}^\infty {\frac{{{{d}_{h}}}}{{{{h}^{3}}}}.} $$
(5)

Then

$$\cos {{\theta }_{0}} = 1 + \frac{{{{A}_{m}}}}{{6\pi {{\sigma }_{{{\text{lic}}}}}}} \cdot \left( { - \frac{1}{{2\left( {\frac{1}{{h_{\infty }^{2}}} - \frac{1}{{h_{{\min }}^{2}}}} \right)}}} \right).$$
(6)

Given that \(\frac{1}{{h_{\infty }^{2}}}\) \( \ll \) \(\frac{1}{{h_{{\min }}^{2}}}\), we can write

$$\cos {{\theta }_{0}} = 1 + \frac{{{{A}_{m}}}}{{12\pi {{\sigma }_{{{\text{lic}}}}}h_{{\min }}^{2}}}$$
(7)

or

$$\cos {{\theta }_{0}} - 1 = \frac{{{{A}_{m}}}}{{12\pi {{\sigma }_{{{\text{lic}}}}}h_{{\min }}^{2}}}.$$
(8)

It is not possible to calculate Аm according to expression (8), on the basis of the values of the surface tension of the wetting liquid, since in this case (taking into account that cosθ takes values from +1 to –1), the Hamaker constant will be negative or equal to zero. However, having the functional dependence cosθ0 – 1 = f(\(\sigma _{{{\text{lic}}}}^{{ - 1}}\)) obtained on the basis of experimental measurement of the equilibrium wetting angles of the surface of a dispersed material with liquids with known values of surface tension, we can calculate the constant Аm of the analyzed material as the tangent of the slope of the straight line multiplied by 12π\(h_{{\min }}^{2}\). As shown by experiments [16], this approach shows that the Hamaker constant or its analog can take both positive and negative values. The variant when Аm < 0 arises in the case of mutual repulsion of the contacting bodies. At the same time, it should be noted that the Hamaker constant is a characteristic of van der Waals interactions in a three-phase system: two particles and an interlayer between them [10]. Therefore, when implementing a concept based on changing the properties of one of the media (in this work, changing the concentration of a water-alcohol mixture) to determine the Hamaker constant, the constant value A cannot be obtained in principle (since each composition of the liquid mixture will have its own constant). However, in our opinion, the functional dependence (8) established for liquids of the same nature (water–ethanol) with a small range of variation in concentration parameters allows us to calculate a certain constant of this ternary system, which we called an analog of the Hamaker constant.

In previous studies [16], we showed the possibility of experimentally determining the value of the analog of the Hamaker constant (Am), which characterizes the energy of dispersion interaction of the surface of a solid body in contact with a water–ethanol (weakly polar) solution (Am01). Here, the index “0” denotes the liquid phase, and the index “1” denotes the phase in contact with the surface of the analyzed solid. To determine Am01, the functional dependence (8) was used:

$$\cos {{\theta }_{0}} = 1 + \frac{{{{A}_{{m01}}}}}{{12\pi {{\sigma }_{{{\text{lic}}}}}{{h}^{2}}}}.$$
(9)

The Am01 value is functionally related to the properties of the solution (A00) and directly to the solid phase (A11) according to the equation [9, 17]

$${{A}_{{m01}}} = {{A}_{{11}}} + {{A}_{{00}}} - \sqrt {{{A}_{{11}}}{{A}_{{00}}}} .$$
(10)

By algebraic transformations from Eq. (10), it is possible to obtain an expression for calculating A11 from the known values of Am01 and A00. So,

$$\begin{gathered} {{A}_{{m01}}} = {{A}_{{11}}} + {{A}_{{00}}} - 2\sqrt {{{A}_{{11}}}{{A}_{{00}}}} , \\ {{A}_{{m01}}} = {{(\sqrt {{{A}_{{11}}}} )}^{2}} - 2\sqrt {{{A}_{{11}}}{{A}_{{00}}}} + {{(\sqrt {{{A}_{{00}}}} )}^{2}}, \\ \end{gathered} $$
$$\begin{gathered} {{A}_{{m01}}} = {{(\sqrt {{{A}_{{11}}}} - \sqrt {{{A}_{{00}}}} )}^{2}}, \\ \sqrt {{{A}_{{m01}}}} = \sqrt {{{A}_{{11}}}} - \sqrt {{{A}_{{00}}}} , \\ \sqrt {{{A}_{{11}}}} = \sqrt {{{A}_{{m01}}}} + \sqrt {{{A}_{{00}}}} , \\ \end{gathered} $$
(11)
$$\begin{gathered} {{A}_{{11}}} = {{(\sqrt {{{A}_{{m01}}}} + \sqrt {{{A}_{{00}}}} )}^{2}}, \\ {{A}_{{11}}} = {{A}_{{m01}}} + 2\sqrt {{{A}_{{m01}}}{{A}_{{00}}}} + {{A}_{{00}}}. \\ \end{gathered} $$

To conduct experiments to determine the wetting angle of the surface of prototypes, a number of weakly polar solutions with a surface tension of not more than 35 mN/m are required. This condition is provided by using a water–ethanol system with a mass content of alcohol from 100 to 60%. In [18], an algorithm for calculating A00 for monohydric alcohols is given. So, for absolute ethanol A00 = 3.91 × 10–20 J,

$${{A}_{{00}}} = {{\pi }^{2}}{{N}^{2}}{{C}_{{00}}},$$
(12)

where N is the number of atoms (molecules) of a substance per unit volume; C00 is the London constant for ethanol,

$${{C}_{{00}}} = \frac{3}{4}{{\alpha }^{2}}I$$
(13)

and

$$\alpha = \frac{{3{{R}_{0}}}}{{4\pi {{N}_{{\text{A}}}}}},$$
(14)

where α and I are the polarization and ionization potential of organic matter; NA is the Avogadro constant; and R0 is the molecular refraction calculated by the Lorentz–Lorentz formula taking into account the molecular weight based on measurements of the density (ρ) and refractive index (n) of alcohol [19]. So, for absolute ethanol (at 20°C), n = 1.3614 and ρ = 0.7893 g/cm3 [20]. The ionization potential of ethanol is 11.7 eV [21]. Our calculation of A00 for 100% ethanol, taking into account the volume of the drop applied by the dispenser to the surface of the test sample of 0.00954 cm3, made it possible to obtain the value of 3.88 × 10–20 J. The obtained value of the Hamaker constant for ethanol allows us, in our opinion, to make the following important assumption. Let us take A00 = 3.91 × 10–20 J as an absolute value; then the relative error of our calculation of the constant A00 is 0.8%. On the basis of this fact, the values of the Hamaker constant, which vary in the range (3.88 ± 0.03) × 10–20 J, can be considered a constant value.

In this work, according to the experimental data obtained in the study of compositions from fine powders of basalt and polymineral sand [22], the results of testing the presented algorithm for calculating A11 (basalt powder) as a constant of the dispersion interaction of fine particles of the same nature and A12 for samples in which part of the dispersed phase (1—basalt) is replaced by fine particles (2—polymineral sand) are presented.

It should be noted that an important component of successful experiments to determine the equilibrium wetting angle is taking into account the time factor associated with the contact of the working fluid with the analyzed surface, which is determined by the properties of the surface itself and the physicochemical characteristics of the working fluid [23, 24].

EXPERIMENTAL

Screenings of basalt from the Myandukha deposit (Plesetsky district of Arkhangelsk oblast) and silty polymineral quartz-feldspathic pit sand from the Kholmogorskoye deposit (Kholmogorsky district of Arkhangelsk oblast) were chosen as the objects of study. The elemental composition of the raw material was determined by X-ray fluorescence spectroscopy at the Center for Collective Use of Northern (Arctic) Federal University Arktika on a Shimadzu EDX 800 HS spectrometer. Grinding of basalt and sand was carried out by joint mechanical dry grinding for 20 min at 420 rpm with large grinding bodies 2 cm in diameter on a Retsch PM100 planetary ball mill. The size of the obtained fine particles was determined on a Delsa Nano analyzer.

For grinding, we used fractions of basalt and sand which had previously passed through a sieve with a mesh size of 1.25 mm, and the uniformity of mixing of the components was not specifically controlled, assuming that a uniform mutual distribution of the components in the mixture was achieved in 20 min from joint grinding.

In addition, in the experiment, we did not set out to determine the change in the dimensional parameters of the particles of the composition, which should not change significantly when the components are mixed, since in this case there is no formation of chemical bonds between fine particles, and the interaction is carried out only through forces of van der Waals nature.

To determine the equilibrium wetting angle of highly dispersed materials, prototypes were made by compacting the studied compositions of basalt and sand (in given ratios), under a load of 1.5 kPa, in metal molds with a diameter of 10 mm.

The surface tension of aqueous solutions of alcohol (technical hydrolytic alcohol, 96%, was used for research) and the wetting angle of the surface were measured at a temperature of 20°C on a DSA-20E (EasyDrop) goniometer. The limit of permissible absolute error in measuring the wetting angle of the goniometer DSA-20E (EasyDrop) was ±0.3°. When carrying out experiments to determine the wetting angle, a specialized computer program selected the time of the first contact of the liquid with the surface of the analyzed sample, which in all experiments varied in the range of 1.0 ± 0.5 s. This is due to the fact that, over the specified period of time, the processes of evaporation and diffusion of liquid into the bulk phase, capillary, and other phenomena associated with surface inhomogeneity can be neglected. In addition, the good reproducibility of the measurement results may indicate the existence of a pseudo-equilibrium state at the interface in this time interval. The contour of the drops of the working fluid was processed by the software using the segment method (the wetting angle was less than 30°). All experiments were accompanied by obligatory parallel measurements. Such an experimental technique made it possible to reduce the scatter in the values of parallel measurements of the wetting angle from 2° to 1°. To average the results, three values of the wetting angle were used, the difference between which did not exceed 0.5°, which in turn changed the range of values of the cosine of this angle (in the range of measured angles of 18°–25°) from ±0.003 to ±0.004.

The constants Am011 and Am012 were calculated on the basis of the values of the tangent of the slope of the functional dependence cosθ0 − 1 = f(\(\sigma _{{{\text{lic}}}}^{{ - 1}}\)). Constants A11 and A12 (experimental samples) and A00 (water-alcohol solutions) were calculated by expressions (11) and (12), respectively.

RESULT AND DISCUSSION

The calculated A00 values for ethanol solutions in the range of alcohol volume concentrations of 40–96%, their molecular refraction, and surface tension are presented in Table 1.

Table 1. Physicochemical characteristics of aqueous solutions of ethanol
Full size table

The calculations performed showed that, in the range of alcohol concentrations from 96 to 60 vol %, the A00 value remains practically constant; therefore, for further calculations, A00 = 3.92 × 10–20 J was used (as the arithmetic mean value).

The chemical composition of raw materials presented in terms of the content of oxides (as a percentage of the total mass) showed the presence of the main components presented in Table 2.

Table 2. Chemical composition of raw material based on oxide content (percentage of total weight)
Full size table

The measured dimensional characteristics of basalt and sand samples allow us to conclude that, under the given grinding modes, a rather narrow fraction in terms of dispersion and good reproducibility of the results is achieved (Table 3).

Table 3. Indicators of dispersed systems
Full size table

To calculate the constants Am011 and Am012, we constructed the functional dependences cosθ0 – 1 = f(\(\sigma _{{{\text{lic}}}}^{{ - 1}}\)) for all series of the experiment, which are linear in nature with a high value of the approximation reliability coefficient (r2). The values of the coefficients of these linear dependences and the obtained constants are presented in Table 3.

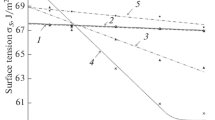
It should be noted that, for all series of the experiment, the linear dependence cosθ0 – 1 = (1/σlic) contains the free term of the equation “b,” which has an almost constant value equal to (–0.20 ± 0.02) (for the composition “basalt–sand”), which, on the basis of Eq. (7), should be equal to zero. The physical meaning of this constant can follow from the following considerations. At Am011 (Am012) = 0, the constant b = cosθ0 − 1. This means that there is some contact angle constant for the given system, the value of which is determined by the nature of the interacting substances (for a system formed only by basalt particles, this constant does not fit into the marked interval and is equal to ‒0.15). This fact (on the basis of the provisions of the theory of surface forces by B.V. Deryagin) can be associated with the formation of a wetting film that is in equilibrium with a bulk liquid (drop). The condition of their equilibrium is determined by the equality of the disjoining pressure in the film Π(h) and the capillary pressure in the drop. With the existence of such equilibrium, it is possible to assume the equality of the properties of the liquid and interlayer, which is realized under the assumption of the conditional equality of the Hamaker constants Am0 and Am1. Then, from Eq. (9), Аm01 = 0. A flat film located in the sphere of action of the surface forces of the substrate, upon transition to the bulk liquid, forms a transition zone, where the surface forces still continue to act. Only outside the range of action of these forces does the liquid acquire bulk properties. The value of the constant “b” is probably related to the contact angle of the transition zone (the angle of inclination of the tangent to the surface). Thus, for the surface formed by finely dispersed basalt particles, θ = 32°, and for the finely dispersed “basalt–sand” composition, θ = 37° (Fig. 1).

Fig. 1.
figure 1

Schematic profile of a liquid droplet on the sample surface: (1) sample surface; (2) a drop of liquid.

Full size image

The functional dependence (A11, A12) = f(Cs) is characterized by a second degree polynomial equation (Fig. 2), which has the following analytical expression (r2 = 0.92):

$$\begin{gathered} ({{A}_{{11}}},{{A}_{{12}}}) = - {{10}^{{ - 23}}}C_{s}^{2} + 8.15 \times {{10}^{{ - 22}}}{{C}_{s}} \\ + \;7.38 \times {{10}^{{ - 20}}}. \\ \end{gathered} $$
(15)
Fig. 2.
figure 2

Functional dependence (A11, A12) = f(Cs).

Full size image

The obtained functional dependence (Fig. 2) shows that the composition consisting of fine powders of basalt and sand is characterized by the maximum value of the Hamaker constant at a certain mass ratio of components. The ratio of the components of the mixture of these powder materials at which the maximum van der Waals dispersion effect appears can be calculated as follows.

Differentiating Eq. (15) with respect to Cs, we obtained an expression for the derivative related to the functional dependence of the form \(\frac{{d{{A}_{{11}}},{{A}_{{12}}}}}{{d{{C}_{s}}}}\) = f(Cs):

$$({{A}_{{11}}},{{A}_{{12}}}) = - 2 \times {{10}^{{ - 23}}}{{C}_{s}} + 8.15 \times {{10}^{{ - 22}}}.$$
(16)

Considering that the derivative of function (15) at the maximum point is equal to 0, we obtain Cs ≈ 41%. Thus, the ratio of components for the finely dispersed composition “basalt–sand” characterized by the maximum value of the Hamaker constant is as follows: 59% basalt and 41% polymineral sand.

FINDINGS

1. For the selection of raw materials when creating a composite material, it is proposed to use the value of the Hamaker constant calculated from the value of the analog component and making it possible to evaluate their dispersion interaction. It has been established that the value of the analog Hamaker constant is a function of the response of the nature of the analyzed materials, and the existence of a functional relationship between the Hamaker constant and the composition of the tested samples makes it possible to justify the choice of a quantitative combination of components that give the maximum van der Waals effect.

2. An assumption is made about the presence of a constant characteristic of a thin film that wets the analyzed surface. Moreover, this thin film has a wetting angle of the transition region “film–bulk phase” whose value is determined by the nature of the surface and the properties of the wetting liquid. The value of this parameter is calculated on the basis of the functional dependence relating the equilibrium wetting angle and the surface tension of the liquids used. The wetting angle of the transition region can be an additional quantitative criterion for the selection of naturally compatible materials.