1 INTRODUCTION

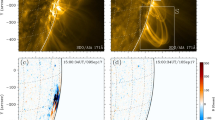
According to the standard flare model, the energy release volume is located at the top of a coronal magnetic loop. This model assumes that during the flare, the increasing number of magnetic field lines is involved in the magnetic reconnection, the loop top rises and the distance between the foot-points increases. However, there are cases of deviation from the standard scenario (see, e.g., Li and Gan, 2005; Zhou et al., 2013), when the height of the loop decreases in the impulsive phase of the flare, and then the loop shrinkage changes to upward motion and expansion. One example of the change in the loop height during a flare on August 24, 2002 at a frequency of 35 GHz is shown in Fig. 1 (Li and Gan, 2005). Radio fluxes at 17 and 35 GHz are also shown here, as well as fluxes of soft X-ray radiation, according to GOES-08 data. From Fig. 1, it follows that, during the impulsive phase of the flare, the large loop radius decreases from 2.3 × 109 cm to 1.5 × 109 cm within a time Δτ = 540 s. Then, within about the same time, the radius increases to a pre-flare value and continues to grow.

Fig. 1.
figure 1

Top panel: SXR light curves from GOES-08. Middle panel: Time history of the radius of the 34 GHz radio flare loop. The solid lines represent the linear fitting. Bottom panel: 17 and 35 GHz light curves obtained with Nobeyama Radio Polarimeter. The two vertical dotted lines mark the times of the beginning and the 1–8 Å maximum of the flare, and the two vertical solid lines indicate the shrinkage period (Li and Gan, 2005).

Full size image

We will show that the height of the magnetic loop depends not only on the balance of magnetic pressure, magnetic tension and gravity, but also on the magnitude of the electric current flowing in the loop. Moreover, the analysis of the loop shrinkage during the impulsive phase of the flare allows us to determine some important characteristics of the flare process: in particular, the evolution of the electric current and loop resistance, as well as the power of the thermal component of the flare. We will investigate the cause of the increase in the electrical resistance of the loop, which in this case plays the role of a flare trigger.

2 THE HEIGHT OF THE CURRENT-CARRYING LOOP

Consider a magnetic loop (Fig. 2) with an electric current J, which flows from one foot-point of the loop to the other through the coronal part of the loop and closes under the photosphere at a depth \({{\tau }_{{5000}}} = 1\), where the conductivity is isotropic (Zaitsev et al., 2000). In Fig. 2, \({{h}_{1}},{{h}_{2}}\) and \(\bar {h}\) are the large, small, and middle radii of the loop, respectively, \({{B}_{z}},{{B}_{\varphi }}\) are the longitudinal and azimuthal components of the magnetic field. The loop is approximated by a half-ring for simplicity. An electric current is generated by the emf located at the foot-points of the magnetic loop and arising from the interaction of convective flows of photospheric plasma with the magnetic field of the loop.

Fig. 2.
figure 2

A sketch of current-carrying flare loop. A tongues of external plasma penetrating into the flux tube near chromosphere foot-points due to R–T instability are indicated as dark areas. As the result of R–T instability the cross-section of current channel at loop foot-points is getting smaller.

Full size image

The following forces act on the loop top, determining its equilibrium in the vertical direction:

—Upward pressure force of the magnetic field associated with the curvature of the field’s lines in inhomogeneous atmosphere and referred to the unit volume

$${{F}_{1}} = \frac{1}{{{{h}_{1}} - {{h}_{2}}}}\Delta \left( {\frac{{B_{z}^{2} + B_{\varphi }^{2}}}{{8\pi }}} \right) = \frac{{\bar {B}_{z}^{2} + \bar {B}_{\varphi }^{2}}}{{4\pi \bar {h}}}.$$
(1)

—Downward magnetic tension force

$${{F}_{2}} = - \frac{{\bar {B}_{z}^{2}}}{{4\pi \bar {h}}}.$$
(2)

—Upward Ampére force associated with the photospheric current

$${{F}_{3}} = \frac{{2{{J}^{2}}}}{{\pi r_{c}^{2}{{c}^{2}}\bar {h}}},$$
(3)

where \({{r}_{c}} = {{({{h}_{1}} - {{h}_{2}})} \mathord{\left/ {\vphantom {{({{h}_{1}} - {{h}_{2}})} 2}} \right. \kern-0em} 2}\) is the half-thickness of the loop in the coronal part.

—Downward gravitational force

$${{F}_{4}} = - \rho g.$$
(4)

—The force acting on the loop from the external magnetic field. The direction of this force (upward or downward) depends on the mutual orientation of the current at the loop top and the external magnetic field:

$${{F}_{5}} = \frac{{J{{B}_{{{\text{ext}}}}}(z)\sin \theta }}{{\pi r_{c}^{2}c}},$$
(5)

where \({{B}_{{{\text{ext}}}}}(z)\) is the height-dependent external magnetic field, which for simplicity is considered to be directed horizontally, \(\theta \) is the angle between the current J and the magnetic field Bext. When analyzing the vertical equilibrium of the loop, we will not take into account the F5 force. For example, if the external horizontal magnetic field changes with height as \({{B}_{{{\text{ext}}}}}(z) = {{B}_{0}}({{{{z}_{0}}} \mathord{\left/ {\vphantom {{{{z}_{0}}} z}} \right. \kern-0em} z})\) (van Tend and Kuperus, 1978), then at B0—100 G, z0 = 108 cm, sin θ ≈ 0.3, and the influence of the external magnetic field on the loop shrinkage can be neglected in comparison with F3 for J  > 1010 A. Magnetic pressure and tension act approximately equally on the loop along its entire length, while the expressions for the forces F3 and F5 are valid for horizontal sections of the loop near the loop top and its surroundings. From Eqs. (1) and (2) it follows that the part of magnetic pressure associated with the longitudinal component of the magnetic field is compensated by magnetic tension. Therefore, keeping in mind that \({{\bar {B}}_{\varphi }} = {{2J} \mathord{\left/ {\vphantom {{2J} {c{{r}_{c}}}}} \right. \kern-0em} {c{{r}_{c}}}}\) and assuming F5 ⪡ F1 + F2, we can write the equilibrium condition for the loop top:

$$\frac{{3{{J}^{2}}}}{{{{c}^{2}}\bar {h}}}\frac{1}{{\pi r_{c}^{2}}} - \rho g = 0.$$
(6)

Thus, the average height of the loop is proportional to the square of the electric current in the loop:

$$\bar {h} = \frac{{3{{J}^{2}}}}{{{{c}^{2}}gM}},\,\,\,\,M = \pi r_{c}^{2}\rho .$$
(7)

3 THE LOOP SHRINKAGE AND INCREASE IN RESISTANCE

From Eq. (7) it follows that the height of the loop top (LT) is determined by the balance of Ampére force, magnetic pressure, and gravity. Therefore, the loop shrinkage during the impulsive phase should indicate a decrease in the current in the loop. This decrease may be due to a change in the resistance of the loop as an equivalent electrical (RLC) circuit and/or a change in the loop inductance. The relative small change in the electric current is associated with a change in the loop top height by the ratio:

$$\frac{{\Delta J}}{{{{J}_{1}}}} = \frac{1}{2}\frac{{{{{\bar {h}}}_{2}} - {{{\bar {h}}}_{1}}}}{{{{{\bar {h}}}_{1}}}},$$
(8)

where \({{\bar {h}}_{1}}\) and \({{\bar {h}}_{2}}\) are the average heights of the loop before and after the shrinkage, respectively. The observed change in the current is rather small indeed. For example, for the X3.1 flare on August 24, 2002 \({{\Delta J} \mathord{\left/ {\vphantom {{\Delta J} {{{J}_{1}}}}} \right. \kern-0em} {{{J}_{1}}}}\) = –0.175; for the X 7.1 flare on January 20, 2005 \({{\Delta J} \mathord{\left/ {\vphantom {{\Delta J} {{{J}_{1}}}}} \right. \kern-0em} {{{J}_{1}}}}\) = –0.107.

In addition, a current variation is an adiabatic process with respect to the period of eigen oscillations of the loop as an equivalent RLC-circuit, \(\sqrt {LC} \approx 10{\kern 1pt} - {\kern 1pt} 100\) s (Zaitsev et al., 2000). Hence the slow changes in the electric current in a flare magnetic loop can be described by the equation

$$\frac{1}{{{{c}^{2}}}}\frac{{dL}}{{dt}}J + \frac{1}{{{{c}^{2}}}}\frac{{dJ}}{{dt}}L + R(J)J = \varepsilon J.$$
(9)

Here, \(\varepsilon J = \left| {{{V}_{r}}} \right|{{{{l}_{1}}J} \mathord{\left/ {\vphantom {{{{l}_{1}}J} {{{c}^{2}}r}}} \right. \kern-0em} {{{c}^{2}}r}}\) is the photospheric emf, which is determined by the parameters of photospheric convection at the foot-point of the loop: the radial velocity component \({{V}_{r}}\), the scale of the emf in height \({{l}_{1}}\), and the radius of the loop foot-point r. \(R(J) = \xi {{J}^{2}}\) is the resistance of the chromospheric part of the loop associated with the Cowling conductivity and depending on the magnitude of the electric current, where

$$\xi \approx \frac{{1.5l{{\chi }^{2}}}}{{\pi {{r}^{4}}n(2 - \chi ){{m}_{i}}\nu _{{ia}}^{{\text{'}}}{{c}^{4}}}}.$$
(10)

Here, \(\chi = {{{{n}_{a}}} \mathord{\left/ {\vphantom {{{{n}_{a}}} {(n + {{n}_{a}})}}} \right. \kern-0em} {(n + {{n}_{a}})}}\), n and na are the number densities of electrons and neutral atoms, respectively, \(\nu _{{ia}}^{{\text{'}}} \approx 2.25 \times {{10}^{{ - 11}}}\chi (n + {{n}_{a}})\sqrt T \) is the effective frequency of collisions of ions with neutrals, T is the temperature in the chromospheric foot-points of the magnetic loop, \(l \approx 5 \times {{10}^{7}}\) cm is the characteristic scale of the chromosphere in the region of the temperature minimum. For loop inductance, we can use the well-known formula for wire with large \(\bar {h}\) and small \({{r}_{c}}\) radii (Landau and Lifshitz, 1960):

$${{L}_{{1,2}}} \approx 4\pi {{\bar {h}}_{{1,2}}}\left( {\ln \frac{{8{{{\bar {h}}}_{{1,2}}}}}{{{{r}_{c}}}} - \frac{7}{4}} \right).$$
(11)

A relative change in the loop inductance in the course of shrinkage is \({{\Delta L} \mathord{\left/ {\vphantom {{\Delta L} {{{L}_{1}}}}} \right. \kern-0em} {{{L}_{1}}}} = {{({{L}_{2}} - {{L}_{1}})} \mathord{\left/ {\vphantom {{({{L}_{2}} - {{L}_{1}})} {{{L}_{1}}}}} \right. \kern-0em} {{{L}_{1}}}}\). From Eq. (9) it follows that the current value before the onset of the impulsive phase is determined by the equation

$$J_{1}^{2} = {\varepsilon \mathord{\left/ {\vphantom {\varepsilon \xi }} \right. \kern-0em} \xi }.$$
(12)

If the convection parameters in the photospheric footpoints of the magnetic loop (i.e. the value of ε) do not change during the flare, i.e. the photosphere is not modified by a flare process (no white light flare, for example), the magnitude of the electric current and the loop size determined by the current can only vary as a result of a change in resistance \(R(J).\) Let us estimate to what value the resistance of the electric circuit should increase in order to lead to the observed loop shrinkage (as well as the loop inductance L) over the time Δτ. Assuming \(R(J) = \xi {{({{J}_{1}} + \Delta J)}^{2}} + \Delta R\), \({{dL} \mathord{\left/ {\vphantom {{dL} {dt}}} \right. \kern-0em} {dt}} \approx {{\Delta L} \mathord{\left/ {\vphantom {{\Delta L} {\Delta \tau }}} \right. \kern-0em} {\Delta \tau }},\) and \({{dJ} \mathord{\left/ {\vphantom {{dJ} {dt}}} \right. \kern-0em} {dt}} \approx {{\Delta J} \mathord{\left/ {\vphantom {{\Delta J} {\Delta \tau }}} \right. \kern-0em} {\Delta \tau }}\), after linearization of Eq. 9 under the assumption \(\left| {{{\Delta J} \mathord{\left/ {\vphantom {{\Delta J} {{{J}_{1}}}}} \right. \kern-0em} {{{J}_{1}}}}} \right| \ll 1\) we obtain

$$\Delta R = - \left( {2\varepsilon + \frac{{{{L}_{1}}}}{{\Delta \tau {{c}^{2}}}}} \right)\frac{{\Delta J}}{{{{J}_{1}}}} - \frac{1}{{{{c}^{2}}}}\frac{{\Delta L}}{{\Delta \tau }}.$$
(13)

For typical parameters of the photospheric emf \({{V}_{r}} = {{10}^{5}}\) cm s–1, \({{l}_{1}} \approx r \approx {{10}^{7}}\) cm, the first term in brackets in Eq. (13) can be neglected, i.e. a change in the emf concentrated at the loop foot-points weakly affects the change in the inductance of the electrical circuit, i.e. its size. In this case, the main factor initiating the loop shrinkage is the change in the resistance of the loop as an RLC-circuit. For example, for the flare of August 24, 2002, assuming \({{r}_{c}} = {{10}^{8}}\) cm, we obtain \({{L}_{1}} \approx 4 \times {{10}^{{10}}}\) cm ≈ 40 H, \({{L}_{2}} \approx 2.2 \times {{10}^{{10}}}\) cm ≈ 22 H. For Δτ = 540 s, \({{\Delta J} \mathord{\left/ {\vphantom {{\Delta J} {{{J}_{1}}}}} \right. \kern-0em} {{{J}_{1}}}}\) = −0.175, \(\Delta L = - 0.45{{L}_{1}}\), from Eq. (13) we obtain the value by which the loop resistance increases during the impulsive phase of the flare, \(\Delta R = 5.1 \times {{10}^{{ - 14}}}\,{\text{cgs}} \approx 4.6 \times {{10}^{{ - 2}}}\) Ohm. The resistance of the electric circuit before the flare, as follows from Eq. (9), is determined from the relation \(R({{J}_{1}}) = \xi {{J}_{1}}^{2}\). Thus, we find \(R({{J}_{1}})\) = \(5.5 \times {{10}^{{ - 16}}}\,{\text{cgs}} \approx 5 \times {{10}^{{ - 4}}}\) Ohm.

Hence, the resistance of the magnetic loop in the impulsive phase of the flare increases by approximately two orders of magnitude, while the electric current changes insignificantly compared to the initial value \({{J}_{1}}\), due to the large loop inductance. To determine the extent to which this conclusion is typical for loop shrinkages, we make the estimates for the flare on January 20, 2005. For this flare, the loop height decreased from \({{\bar {h}}_{1}} = 3.4 \times {{10}^{9}}\) cm to \({{\bar {h}}_{2}} = 2.8 \times {{10}^{9}}\) cm over the time \(\Delta \tau = 300\) s, which corresponds to an increase in the loop resistance during the impulsive phase by \(\Delta R = 7.2 \times {{10}^{{ - 14}}}\,{\text{cgs}} \approx 6.5 \times {{10}^{{ - 2}}}\) Ohm, i.e., as it was in the first case, by about two orders of magnitude.

4 THE REASON FOR INCREASE IN RESISTANCE AND POWER OF JOULE HEATING

The reason for the increase in the loop resistance during the impulsive phase of the flare may be the Rayleigh–Taylor (R–T) instability in the chromosphere foot-points of the magnetic loop (Fig. 2). As a result of R–T instability, the external plasma penetrates the loop at a speed \({{V}_{r}} < 0\). Let, for example, \({{V}_{r}} = {{{{V}_{0}}r} \mathord{\left/ {\vphantom {{{{V}_{0}}r} {{{r}_{0}}}}} \right. \kern-0em} {{{r}_{0}}}}\) where \({{r}_{0}}\) is the radius of the tube in the region of instability. As a result, perturbations of the magnetic field \({{B}_{\varphi }}\) and \({{B}_{z}}\) arise in this region. The field perturbation \({{B}_{\varphi }}\) leaves the instability domain in the form of a nonlinear Alfvén wave and leads to the generation of fast electrons manifesting their presence in microwave and hard X-rays (Zaitsev et al., 2016). The field perturbation \({{B}_{z}}\) remains in the instability domain and leads to a decrease in the cross section of the current channel. As it follows from Eq. (10), the circuit resistance depends quadratically on the channel cross section and can increase with a decrease in the cross section, leading to a decrease in the current and to the loop shrinkage.

The effect of reducing the cross section of the current channel due to R–T instability can be shown in the following example. Assume, for example, that the component of the magnetic field \({{B}_{z}}\) in a vertical magnetic flux tube depends on the radial coordinate as follows:

$$B_{z}^{2}(r) = B_{z}^{2}(0) - C({{{{r}^{2}}} \mathord{\left/ {\vphantom {{{{r}^{2}}} {r_{0}^{2}}}} \right. \kern-0em} {r_{0}^{2}}}),\,\,\,\,r \leqslant {{r}_{0}}.$$
(14)

When a tongue of external plasma penetrates into the flux tube due to the R–T instability, the component \({{B}_{z}}\) will change as follows (Stepanov et al., 2012):

$$\begin{gathered} {{B}_{z}}(r,t) = {{B}_{Z}}(0)\exp \left( { - \frac{{{{V}_{0}}t}}{{{{r}_{0}}}}} \right){{\left[ {1 - C\frac{{{{r}^{2}}}}{{r_{0}^{2}}}\exp \left( { - \frac{{2{{V}_{0}}t}}{{{{r}_{0}}}}} \right)} \right]}^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-0em} 2}}}}, \\ r \leqslant {{r}_{0}}\exp \left( {\frac{{{{V}_{0}}t}}{{{{r}_{0}}}}} \right). \\ \end{gathered} $$
(15)

Since \({{V}_{0}} < 0\), the effective radius of the flux tube, as follows from Eq. (15), decreases with the development of R–T instability. The magnitude of the electric current in the magnetic loop before the start of the impulsive phase of the flare is found from Eqs. (10) and (12):

$${{J}_{1}} = {{\left[ {\frac{{\left| {{{V}_{r}}} \right|\pi {{r}^{3}}{{c}^{2}}n{{m}_{i}}(2 - \chi ){{l}_{1}}\nu _{{ia}}^{{\text{'}}}}}{{1.5{{\chi }^{2}}l}}} \right]}^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-0em} 2}}}}.$$
(16)

Supposing \(n = {{10}^{{11}}}\) cm–3, \({{n}_{a}} = {{10}^{{15}}}\) сm–3, \(T = {{10}^{4}}\,{\text{K}}\), \({{V}_{r}} = {{10}^{5}}\) сm s–1, \(r = {{10}^{7}}\) сm, we obtain the value of the electric current in the magnetic loop before the impulsive phase: \({{J}_{1}} \approx 1.2 \times {{10}^{{20}}}\,{\text{cgs}}\) ≈ 3.6 × 1010 А. Since the electric current in the impulsive phase varies slightly compared to the initial value \({{J}_{1}}\), it is possible to estimate the rate of Joule losses before and during the impulsive phase. For the flare of August 24, 2002, we obtain \({{W}_{1}} = R({{J}_{1}})J_{1}^{2} \approx 7.5 \times {{10}^{{24}}}\) erg s–1, \({{W}_{{fl}}} \approx \Delta RJ_{1}^{2} \approx 7 \times {{10}^{{26}}}\) erg s–1. Thus, the Joule heating rate increases by two orders of magnitude during the impulsive phase. Joule loss in the impulsive phase of the flare is \(0.5{\kern 1pt} {{W}_{{fl}}}\Delta \tau \) ≈ 1.9 × 1029 erg. This value is about 60% of the non-potential energy originally stored in the loop, \({{L{{J}^{2}}} \mathord{\left/ {\vphantom {{L{{J}^{2}}} {2{{c}^{2}}}}} \right. \kern-0em} {2{{c}^{2}}}} \approx 3 \times {{10}^{{29}}}\) erg. Estimates for the flare on January 20, 2005 (Zhou et al., 2013), with similar parameters of the chromosphere and photosphere convection, lead to similar values of the electric current energy stored in the magnetic loop \({{L{{J}^{2}}} \mathord{\left/ {\vphantom {{L{{J}^{2}}} {2{{c}^{2}}}}} \right. \kern-0em} {2{{c}^{2}}}} \approx 1.1 \times {{10}^{{30}}}\) erg and the Joule losses initiating plasma heating, \(0.5{{W}_{{fl}}}\Delta \tau \approx \) 7 × 1029 erg. In this case, heating of the chromospheric foot-points leads to an increase in the degree of ionization and a decrease in \(\chi \). As a result, the circuit resistance decreases (Cowling conductivity increases) and the current increases with a continuously acting photospheric emf, leading to a subsequent increase in the size of the magnetic loop (Fig. 1).

5 CONCLUSION

We have shown that the height of the loop top depends not only on the balance of magnetic pressure, tension and gravity, but also on the magnitude of the electric current in the loop. In this case, the role of electric current in the loop equilibrium equation turns out to be more significant than that in the case of a horizontal current-carrying filament (Kuperus and Raadu, 1974). It was also shown that the flare process for a current-carrying loop is accompanied by a significant (~ two orders of magnitude) increase in the loop resistance with a slight (≤ 20%) decrease in the magnitude of the electric current compared to the initial value due to the large loop inductance.

The reason for the increase in loop resistance during the impulsive phase of the flare can be the Rayleigh-Taylor instability in the chromospheric foot-points of the magnetic loop. As a result of the instability, the external plasma penetrates into the loop and reduces the channel cross section with the current (see Eq. (15)). This increases the resistance of the chromospheric part of the loop (Eq. (10)) and Joule dissipation rate by approximately two orders of magnitude, providing the thermal component of the flare. The current value is maintained at a level close to the preflare value due to the non-potential energy stored in the magnetic loop before the flare. The Rayleigh–Taylor instability in this case plays the role of a flare trigger.

The time of increasing of the loop top height after the shrinkage to a pre-flare value, as it follows from Fig. 1, is ~500 s. Estimates have shown that during this time the loop resistance decreases by approximately two orders of magnitude and close to that before the flare. Further heating of the flaring plasma leads to additional growth in the loop height.