Reform mathematics teaching calls attention to mathematics classroom talk (hereafter, math-talk) (Brodie, 2013; Conner et al., 2014; Hufferd-Ackles et al., 2004; Walshaw & Anthony, 2008). In math-talk, students have the opportunity to listen to explanations (Hintz & Tyson, 2015), share insights and ideas (Barron, 2003), observe the strategies of other (Walshaw & Anthony, 2008), engage in collective argumentation (Conner et al., 2014) and explain one’s reasoning of a phenomenon (Jackson et al., 2013). However, research suggests that talk in the mathematics classroom is usually relatively constrained (Brodie, 2011) and does not always allow for development and extension of students’ mathematical understanding (Fraivillig et al., 1999). Students’ engagement in math-talk is usually reduced to observational reports (Nilsson, 2019) and a practice of show-and-tell (Stein et al., 2008). Hence, if we believe in the power of math-talk for the learning of mathematics, there is a need to investigate further how teachers can support the development of math-talk that builds on students’ ideas and informal strategies and encourage mathematical activities such as analysing, making connections, explaining, inferring and generalizing (Fraivillig et al., 1999).

Digital technologyFootnote 1 has reached increased attention regarding collective learning processes (White, 2018). It is suggested that it can provide students and teachers with new forms of physical interactions, sharing of a product of activity and verbal forms of communication for joint mathematical work. However, for many teachers, it is challenging to shape and lead a technology-rich teaching practice (Hegedus et al., 2017), and much is still unknown about how to exploit the potential of powerful technologies for mathematics learning (Drijvers, 2019). With this background, the present study engages in a design experiment (Cobb et al., 2003), with the aim of contributing with knowledge on how technology can support math-talks. We address the following research question: How can collective inferential reasoning be orchestrated in a technology-enhanced learning environment?

We approach our research question via the theories of instrumental orchestration (Trouche, 2004), instrumental genesis (Lonchamp, 2012; Guin & Trouche, 2002) and inferentialism (Brandom, 1994). Instrumental orchestration provides us with an analytical lens on arranging and utilizing technology in the orchestration of math-talk, in terms of a collective instrumental genesis (Drijvers et al., 2010). Inferentialism provides the Game of Giving and Asking for Reasons (GoGAR) as a metaphor to describe how knowledge and meaning-making emerge inferentially within a collective and pragmatic practice of reasoning (Bakhurst, 2011; Brandom, 2000). Before we elaborate on our theoretical approach, we discuss relevant research on math-talk in classrooms and elaborate on its complexity in relation to technology.

Previous Research

In mathematics classrooms where teachers take the social and interactive nature of meaning-making seriously (Lerman, 2000), students are supposed to participate actively in a collective process of investigation, and analyse and reason, rather than wait to answer leading questions from the teacher (Hufferd-Ackles et al., 2004). In such teaching, the teacher is positioned as an orchestrator in supporting student participation. The teacher’s supporting role entails encouraging students to expand on their ideas and to make connections between ideas (Manouchehri & Enderson, 1999). Staples (2007) identified three interrelated thematic actions a teacher can take in supporting math-talk: supporting students in making contributions, establishing and monitoring common ground and guiding the mathematics.

To continue, we will reflect on the complexity for teachers to perform these actions and on how technology can become a resource for teachers to meet challenges in supporting math-talk that includes teacher, technology, students and mathematics (Rezat & Sträßer, 2012). Drijvers (2015) points out that, even though so many studies conclude that there is great potential for technology in mathematics education, teachers still face questions of how, when and where it should be adopted.

The first action—supporting students in making contributions—means eliciting and scaffolding the production of ideas. A mathematical artefact, such as a GeoGebra applet with a drag tool (Leung et al., 2006), provides a teacher with opportunities to support students in making contributions when a student explores geometric problems by clicking and dragging. With the help of the artefact, the teacher can elicit and share mathematical ideas and reasoning (Staples, 2007). However, a teacher merely eliciting answers to closed questions (e.g. through initiation–response–evaluation (IRE) patterns) tends to constrain math-talk (Nilsson, 2019). Teachers need to ask explorative questions and press students to follow up on their contributions (Brodie, 2011).

Since its introduction to mathematics education, technology has been revered for its opportunities to create dynamic and interactive learning environments. Twenty-five years after Kaput’s (1992) seminal review on technology in mathematics education, the shift from static to dynamic media has been one of the key arguments for integrating technology in mathematics teaching (Roschelle et al., 2017). Connected classroom technology (i.e. networked systems of digital devices in the classroom) provide opportunities for teachers to elicit, connect and develop ideas (Irving, 2006). Connected classroom technology allows teachers to monitor students' progress and ideas and to use them in forwarding math-talk (Clark-Wilson, 2010). However, Doerr and Zangor (2000) showed that even though the technology proved to support communication in the whole-class setting, it did not support communication in group work. Their results indicate that we need to investigate further the role of technology in math-talk.

The second action—establishing and monitoring common ground—entails coordinating the class work, creating a shared context between students and maintaining the flow of the math-talk over the duration of a lesson. Students need to be able to contribute with their own ideas and have multiple opportunities to access ideas of their classmates (Eckert, 2017). However, teachers trying to co-ordinate class work through traditional teaching formats, such as presentations and IRE pattern, tend to limit the math-talk to a practice of show-and-tell (Nilsson, 2019; Stein et al., 2008). This can be avoided by making students’ ideas easily available to the whole class by means of resources that offers the opportunity of public displays, which allow students to share ideas with each other (Eckert & Nilsson 2019).

For instance, Hegedus and Moreno-Armella (2009) showed how dynamic representations on a public display can elevate a teacher’s supporting actions to establish mathematical inferences and to monitor and direct the course of math-talk. In contrast, however, Clark-Wilson et al. (2015) conclude that it is challenging for teachers to take full advantage of technology because of the level of mathematical knowledge they require. It is therefore important to purposefully design technology and activity so that the teacher is supported in establishing and monitoring focused math-talk in the classroom (Cusi et al., 2017).

The third action—guiding the mathematics—means attending to the co-construction of ideas, rather than the teacher transmitting ideas to the students. Guiding the mathematics is not an easy task. It is a complex enterprise that requires deep knowledge of students, mathematics and teaching strategies (Clark-Wilson et al., 2015). Adding technology to the teaching may add to this complexity (Lagrange & Monaghan, 2010). Since we cannot assume that teachers will work the same way in a technology-rich environment as they would do in ‘traditional’ classrooms (Drijvers, 2011), there is a need to study how teachers can exploit a configuration of technology for guiding the mathematics in the classroom. Kendal and Stacey (2002), for example, studied a teacher-centred technique in technology-rich environments that guided learning in a step-by-step methodology. Students were offered minimal opportunities to produce their own mathematical explanation, since the teacher controlled the interaction by funnelling (Bauersfeld, 1998). In order to guide and, at the same time, to keep a strong pressure on students to provide mathematical explanations, students should be held accountable for their ideas (Kazemi & Stipek, 2001).

Drijvers (2011) argued, “It is not self-evident that techniques and orchestrations which are used in ‘traditional’ settings can be applied successfully in a technological-rich learning environment” (p. 265). A teacher’s practice is both complex yet stable, and technology may increase the complexity and challenge the stability of teaching (Robert & Rogalski, 2005). As a result, a new repertoire of orchestrations must emerge to consolidate the use of technology into a teaching practice (Drijvers, 2011). In other words, it is necessary to know more about how teachers can design an instrumental orchestration (i.e. exploit available technology) to guide math-talk.

Theoretical Approach

We use the construct of instrumental orchestration (Trouche, 2004) to conceptualize a teacher’s intentional and systematic organization and use of technology to guide math-talk in terms of a collective instrumental genesis (Drijvers et al, 2010). We then conceptualize collective instrumental genesis from the semantic theory of inferentialism and, in particular, from the Game of Giving and Asking for Reasons (GoGAR) (Brandom, 1994).

Instrumental Genesis and Its Orchestration

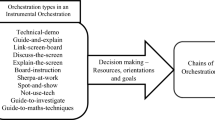
An instrumental orchestration is characterized by carefully designed instruction based on an available didactical configuration with focus on how it may both be exploited and used in a teacher’s didactical performance (Drijvers et al., 2010). A didactical configuration is composed by an artefact or, more often, a complex of artefacts. In the discourse of instrumental orchestration, the artefacts are usually digital, such as calculators, computers and interactive whiteboards. This is also the situation in the present study.

We refer to technical demo (Drijvers et al., 2010), in relation to a f(x) = g(x) task, to explain the concepts of instrumental orchestration. The didactical configuration could consist of an applet or graphing calculator that can generate graphs and a solution for projecting what happens on individual screens to a public screen. The didactical configuration can then be exploited by a teacher to demonstrate how to find intersection points. One exploitation mode could be the possibility of zooming. How a teacher chooses to utilize possible exploitation modes of a didactical configuration in a technical demo refers to a teacher’s didactical performance–ad hoc decisions in the moment to guide in the learning of mathematics. In the case of demonstrating how to find intersection points, a teacher can use the zooming function in her didactical performance to enhance the visualization of intersection point(s) of two graphs.

Instrumental orchestration is linked to instrumental genesis in that an instrumental orchestration is the intentional and systematic organization and use of the various artefacts available by the teacher in a given learning situation, in order to guide students’ instrumental genesis (Lonchamp, 2012; Guin & Trouche, 2002). Instrumental genesis describes a process, which involves the mix of artefacts and schemes the user develops to turn the artefact into an instrument for specific types of tasks (Drijvers et al., 2010). Schemes concern both technical knowledge about the artefact and domain-specific knowledge, such as knowledge in mathematics. In the present study, we will not consider schemes as an individual, psychological construct. Instead, following Drijvers and colleagues’ notion of a collective instrumental genesis, we look at how artefacts take a position and are used as instruments in a social and pragmatic practice of reasoning and meaning-making in mathematics. In particular, we aim at giving account of how teachers orchestrate situations by means of technology, in order to guide a collective instrumental genesis in the frame of the game of giving and asking for reasons (Brandom, 1994).

Inferentialism and Instrumental Math-GoGAR

Inferentialism is Brandom’s (1994) term for his “account of the nature of the conceptual, the nature of meaning, content, and awareness, the nature of what makes us exemplars of Homo sapiens” (Bransen, 2002, p. 374). As beings of reason, things mean something to us. They have content for us, and we understand them in one way rather than another (Derry, 2013). For instance, we have the capacity to understand that the statement “50 percent” is compatible with “one-half”, but incompatible with “one-third”.

In inferentialism, the meaning of a concept is explained in terms of its role in a space of reasons and reasoning (Noorloos et al., 2017). A concept or a claim can take positions both as premises and as consequences; they can both be reasons and be in need of reasons. Imagine that someone is shouting “The house is on fire” because they see and smell smoke. The meaning of what they say and, thus, the concept of fire is inferentially related to antecedents, such as “seeing smoke coming out of a room” and “smoke results from fire”, which lead to consequences such as “leaving the house” and “calling the emergency number”. The meaning of a concept is not fixed but evolves through the inferential connections between the concepts. In other words, “As one becomes gradually familiar with more of the inferences the concept is engaged in, one becomes more familiar with the concept as well” (Noorloos et al., 2017, p. 446).

Inferentialism takes a social and pragmatic stance on meaning-making and understanding (Brandom, 1995). Rather than considering the space in which thought moves, Brandom suggests looking at the normative space of language use (Sellars et al., 1997). Brandom (1994) introduces the game of giving and asking for reasons (GoGAR) as a metaphor to describe how knowledge and meaning-making emerge within a social, collective and pragmatic practice of talk and reasoning (Bakhurst, 2011; Brandom, 2000). Knowing or grasping the content of a claim (e.g. “Fire in the house”) consists of knowing what follows from a claim and what it follows from, what would be evidence for it and what is incompatible with it (Brandom, 2000). For teaching and learning, a math-GoGAR implies a practice where students are engaged in making claims, giving reasons and asking for reasons relevant to mathematics (Nilsson, 2020). In this practice, students also acknowledge claims and reasons, attribute them to others, undertake them or reject them (Schindler & Seidouvy, 2019).

Following an inferentialist perspective, we consider collective instrumental genesis as the process in which an artefact becomes an instrument in GoGAR. In particular, we use the term instrumental math-GoGAR (IM-GoGAR) to label the situation in which technology takes positions in a GoGAR about mathematics. Linking instrumental orchestration to the genesis of IM-GoGAR then concerns how exploitation modes of a didactical configuration are used in teachers’ didactical performance to guide the IM-GoGAR. To illustrate this linking, we return to the example of using graphical calculators to make sense of the intersection point(s) of two graphs. In the context of GoGAR, a teacher could ask students for reasons for the intersection points of two graphs. The teacher can use the didactical configuration to demonstrate and give reasons (and ask for reasons as well) for how the intersections can be found and, so, making explicit the meaning of an intersection point of two graphs. The students can then use the zooming function as an instrument in giving reasons for the solution of f(x) = g(x).The example involves an IM-GoGAR of many reasons related to the concept of intersection points, of which only a few are made explicit here. The main point, however, is how technology is taking a position as an instrument in a collective practice of giving and asking for reasons.

Next, we outline the design experiment used to study how collective inferential reasoning, in terms of IM-GoGAR, can be orchestrated in a technology-enhanced learning environment.

Method

The project this article reports on was a design research project. Design experiments draw on theories and practices of teaching and learning for design decisions to solve specific needs grounded in teaching practice (diSessa & Cobb, 2004; Ruthven et al., 2009).

Participants and Data Collection

The research team consisted of two experienced mathematics teachers, Peter and Eric,Footnote 2 working in lower-secondary school, and two researchers, the authors of this article. This team designed the tasks, analysed the material and made changes during weekly project meetings. Peter taught a Grade 8 class (14–15-year-olds), and Eric taught a Grade 9 class (15–16-year-olds). They were the classes’ regular teachers and had extensive knowledge about the day-to-day work and the students’ previous mathematics experiences. The students in both classes had scant experience with pattern generalization, particularly in searching for and formulating a general expression for a pattern using n. The students had had some experience discussing mathematics in exploratory tasks. However, they were mainly accustomed to receiving teaching based on individual work from textbooks, accompanied by whole-class presentations led by their teacher. In the transcripts, teachers and students are anonymized. They were, however, informed that 100% anonymization could not be guaranteed because of the nature of the project and the official research team.

The data consists of video and audio recordings of classroom teaching and of audio recordings and written notes from project meetings. Each project meeting was a full day in length, and the discussions were recorded (audio only) except for the breaks. Three video-cameras were used during the lessons: one placed in the back of the classroom and a second one in front of the classroom to capture whole-class teaching. Two cameras were directed towards student-groups (one doubling as the back camera) to capture their work in between whole-class teaching and how technology was utilized by the groups. It resulted in a total of approximately 20 h of video material.

Didactical Configuration and Overall Task Design

The design experiment involved two iterations of a series of three consecutive lessons. Iteration 1 was conducted in Eric’s class, and Iteration 2 was conducted in Peter’s class. The technology used in the intervention was common in Swedish mathematics classrooms. This was supposed to keep the complexity low and to help the teachers maintain stability in their teaching practices. This meant that the didactical configuration consisted of all students and the teacher having their own personal computers, as well as a projector directed towards a whiteboard at the front of the class.

The didactical configuration also included three on-line, free-to-use software programs: Socrative (www.socrative.com), Padlet (www.padlet.com) and GeoGebra (www.geogebra.org). Socrative is an on-line response system that allows teachers to post questions which then show up on students’ devices and allows them to post answers. Answers become instantly available for the teacher, and for the students too, if the teacher so wishes. Padlet is an on-line, virtual pin-board that lets students publish pictures, solutions or other types of contributions. These contributions are instantly available for the whole class through each device. GeoGebra is an on- and off-line mathematics application with multiple uses, which offers the possibility to construct applets that are customized for the content in question.

The design experiment was on pattern generalization. The starting point of the IM-GoGAR was that of additive inferences connected to tasks on arithmetic patterns. The end-point was to develop an IM-GoGAR on visual structure reasoning (Rivera, 2010), for making sense of algebraic expressions of far-generalizations.

Lesson 1 focused on how to use the applet for making claims of near and far generalizations of arithmetic patterns. We did this by challenging naïve additive inferences in pattern generalization and supporting the development of the more advanced multiplicative strategy (Rivera, 2010) motivated by far-generalization tasksFootnote 3 (Mouhayar & Jurdak, 2015). The students moved from making near generalizations, making sense of the next stageFootnote 4 in the pattern, to far generalizations, making sense of an arbitrary stage by an algebraic expression.

An example of this progression is to move from focusing on the numerical difference between subsequent elements and instead attending to a pattern to discern repeated visual structure units. Figure 1 shows a visual representation of a chair-pattern task with colour-coded visual structure units. The students were asked to identify an algebraic expression, e.g. n + (n + 1) + n, that matched the colouring. Later, in the same lesson, the students were asked to work the other way around, colour-coding the visual structure units based on a given expression.

Fig. 1
figure 1

First three stages (figur in Swedish) of the chair pattern with colour coding

Full size image

For example, they colour-coded the expression (n + 1) + (n–1) + (n + 1) in the chair pattern. The students could easily colour-code patterns by using a digital paint tool. However, since the task was challenging to many students, the first iteration of the lesson revealed that they became distracted when using the digital paint tool. Many students used it to draw unrelated pictures, and their talk involved little about mathematics. In the subsequent iteration, we instead offered a GeoGebra applet, which contained the same possibilities to colour-code patterns but with less freedom.

In Lesson 2, the teachers orchestrated further opportunities to develop the IM-GoGAR, by adding more complex tasks to the teaching. In this lesson, the students were first asked to work in pairs to create their own patterns and publish them in Padlet. Next, they were asked to formulate algebraic expressions for their classmates’ patterns. Following inferentialism (Brandom, 1994), the teachers were encouraged to push the students to justify their reasoning on these open-ended problems. Therefore, the lesson ended with a whole-class talk on which patterns the students had found easy or difficult to formulate into an expression. Padlet made each pattern accessible to each student, in both group-work and whole-class talk. During the first iteration of Lesson 2, one pair of students posted a pattern that was not an arithmetic sequence but instead a quadratic sequence. This pattern challenged students’ linear perception of patterns. The class moved the IM-GoGAR forward by making inferences on whether this new pattern really was a pattern or not, by exploiting the opportunities of the software to switch to and compare it with other patterns. The discussion resulted in a collective agreement that the increase of elements does not need to be linear to qualify as a pattern, but the elements do need to be predictable. To provide Peter with the opportunity to orchestrate a similar discussion in the second iteration, the project team prepared a quadratic pattern in advance, so Peter could add it to the Padlet if none of the students made such a pattern.

The aim of Lesson 3 was to guide the IM-GoGAR to include ways of using the GeoGebra applet also to solve patterns similar to the quadratic pattern in Lesson 2. It was specially designed to challenge students to use the applet to transform patterns and identify visual structure units to generate algebraic expressions of more complex patterns which are not representations of standard arithmetic sequences.

Figure 2 shows the initial stages of the hexagonal pattern that the students were asked to generalize. The students worked in pairs with tasks distributed through Socrative. The main task utilized a GeoGebra applet with movable dots identical to those in Figs. 2 and 3. The applet enabled students to try out ideas and make inferences by adding and moving dots effortlessly in each stage. Our goal was to orchestrate an IM-GoGAR where students could recognize differences and similarities between the hexagonal and the quadratic pattern (n2). More specifically, our ambition was to guide the students to make inferences between how the two patterns grew and between the transformed hexagonal pattern (see Fig. 3) and the quadratic pattern.

Fig. 2
figure 2

The hexagonal pattern

Full size image
Fig. 3
figure 3

The hexagonal pattern transformed

Full size image

Method of Analysis

Exerting control over design experiments implies the need for analyses prior the intervention (prospective analysis), during the intervention (reflective analysis) and after the intervention (retrospective analysis).

  1. 1.

    The initial design of the interventions was based on theoretical and empirical arguments from a prospective analysis of previous research by the researchers and from the teachers’ teaching experiences.

  2. 2.

    The reflective analysis (the modification of the interventions) was based on the recorded lessons. The analysis culminated in discussions during weekly design meetings between the two teachers and the two researchers on what worked and what needed revision.

  3. 3.

    The retrospective analysis was an overarching analysis by the two researchers of all six lessons. The exploitation modes for orchestrating an IM-GoGAR, which we account for in the “Results” section, emerged from the retrospective analysis.

We followed Bakker’s (2018) advice to make use of the constant comparative method in the retrospective analysis. This method meant that we compared and contrasted teachers’ didactical performance, in relation to how functions of the didactical configuration were exploited to guide the IM-GoGAR. In practice, each researcher identified episodes where teachers used technology in the GoGAR. These episodes were then compared in analysis meetings between the two researchers to discern and agree on exploitation modes.

For example, when comparing an episode from Lesson 3 with one from Lesson 1, we could discern similarities on how the teacher orchestrated the use of markings to give reasons for far generalizations of patterns. During Lesson 1, the teacher prompted students to use different coloured dots to identify different visual structure units. During Lesson 3, the teacher asked a student to give a reason for his claim by dividing the pattern into a square and a rectangle and then circle the visual structure units that justify the solution. Both instances contributed with evidence on how an IM-GoGAR can be guided by means of exploiting opportunities of marking in a technology-enhanced learning environment. We later labelled this exploitation mode as marking flexibility, which is one of three exploitation modes of the “FlexTech” orchestration that emerged from our analyses.

Results

Characteristics of the FlexTech orchestration are three exploitation modes of flexibility, namely, flexibility in constructing, marking and switching. We continue by first giving an overall presentation of the three exploitation modes. Then we will use episodes from Lesson 3 of Iteration 2 to provide empirical arguments from the case of pattern generalization of how the three exploitation modes can be used to guide an IM-GoGAR. Collective reasoning is expressed in how Peter alternated between group-work and whole-class discussions, returned to previous tasks and invited students to build on each other’s reasoning.

Flexibility in constructing means that the didactical configuration enables the building of models or forms of representation. In this study, flexibility in constructing means that the software and the digital projection on the white board offer the opportunity to extend a pattern and to change its visual structure to facilitate visual analysis of the pattern and to reach an algebraic expression that describes it. Flexibility in switching refers to situations when the didactical configuration allows for rapid changes between tasks, representations and student solutions. Flexibility in marking refers to how the didactical configuration can enable the adding of information on a screen by digital as well as non-digital means. A teacher, or a student, circling a group of dots with a pen on the whiteboard provides an example of non-digital marking with the purpose of highlighting how n can be found in a pattern. Flexibility in switching facilitates contrasting activities. For instance, in trying to make students aware of the features of a quadratic pattern, the teacher can contrast the growth of the quadratic pattern with the growth of an arithmetic linear pattern. By such an act of contrast, the teacher gives visual reasons for why the growth of a quadratic pattern is not the growth of an arithmetic pattern.

Now we turn to the second iteration of Lesson 3 to provide details from empirical instances of how flexibility in constructing, marking and switching orchestrated the genesis of an IM-GoGAR to make sense of an algebraic expression of the hexagonal pattern (see Fig. 2).

Constructing Flexibility

Constructing flexibility appeared in two forms during the lesson: (i) in constructing a pattern by adding subsequent stages to it and (ii) in transforming a structure. At the beginning of the lesson, Peter challenged students’ linear perception of patterns by first showing only two stages of the hexagonal pattern (the two first stages in Fig. 2) on the whiteboard (WB). He then asked the students to guess how many dots there would be in the third stage. No further information was given to them. Generalizing the pattern according to the constant difference property (Stacey, 1989), the students suggested that the third stage was made of 11 dots. Peter continued constructing the pattern by adding its third stage. Confronted with this, all students understood that the answer “eleven dots” was wrong and that the correct number of dots was fifteen. So, in his didactical performance, Peter used the flexibility in constructing a non-linear pattern to guide the IM-GoGAR forward on the distinction between linear and non-linear patterns.

He then asked the students to discuss the number of dots in stage four. They worked in groups on their laptops. The first three stages of the pattern were displayed on the students’ screens, and the applet allowed them to construct the fourth stage. When the groups were done, Peter asked Gene to construct the fourth stage on the public display. Figure 4a shows the result of Gene’s initial construction, to which Peter reacted and asked Gene for reasons:

Peter: How are you thinking?

Gene: I thought, three here at the top [pointing at the three purple dots at the top of the third layer] so, one [pointing at the single blue dot], two [pointing at the two green dots at the top of layer two], three [pointing at three purple dots at the top of layer three], four [pointing at the four red dots at the top of layer four].

Fig. 4
figure 4

a, b Gene’s construction of stage four in the hexagonal pattern

Full size image

Peter was probably okay with the reason Gene provided, but being aware of the constructing feature of the configuration, he pushed the IM-GoGAR further:

Peter: Is there someone who would like to help Gene to arrange…, are we satisfied with how they [the dots] are arranged now?

Emma: No, we should bend them in.

Peter: Shall we bend them in?

Emma: Yes.

Peter: Okay, what does that mean?

The reasons why Peter was not satisfied with Gene’s initial construction was not made explicit. But, seeing his doubt and Emma’s statement, “No, we should bend them in” as inferentially related, we can infer that the underlying reason was the need to see the visual structure more clearly between the different layers. Gene acknowledged the concern and made the IM-GoGAR clearer on the visual reason for the number of red dots (see Fig. 4b), by using the possibility of the applet to make changes to the last layer of the hexagonal pattern. Flexibility in constructing was also in play during situations where the teachers helped the students to see the structure in stages and then asked them to add a subsequent stage and continue working on their own.

Marking Flexibility

It was possible to make marks both by digital and by non-digital means, and Peter acted on both to guide the IM-GoGAR for making sense of the hexagonal pattern. In Fig. 4 a and b, we can see that each layer is digitally marked by different colours. After Gene was done with stage four, Peter used these markings to guide the IM-GoGAR on how the pattern behaved and grew. Ardy voiced that the reason for different colours was to show the difference between the numbers of dots of subsequent stages of the pattern. He then reported that stage two increased by five dots, stage three by nine dots, and stage four by thirteen dots. Peter exploited the flexibility to mark arcs between subsequent stages with a non-digital whiteboard pen to make Ardy’s reasoning further explicit in the class. Moreover, over each arc, Peter added the difference in the number of dots between subsequent stages (see Fig. 5). We will elaborate further on marking flexibility when we discuss how the non-digital marking made here was used together with switching flexibility.

Fig. 5
figure 5

Illustration of the growth of the hexagonal pattern

Full size image

Switching Flexibility and Marking Flexibility

The flexibility to switch rapidly between patterns was used to guide the IM-GoGAR in relation to the situation of comparing the hexagonal pattern with the square pattern the students had been working on in Lesson 2b. Peter erased the non-digital markings made on the hexagonal pattern (still visible in Fig. 5) and then switched the picture on the WB so it displayed the four first stages of the square pattern and asked the students if they remembered how they could find an expression of this pattern. Hallie claimed n times n. Peter wrote n•n on the whiteboard and asked Cho to approach it and give reasons for Hallie’s claim. Peter particularly asked him to mark how he found the visual structure unit n (Rivera, 2010) in the stages of the square pattern (Cho’s non-digital markings are shown in Fig. 6).

Cho :Like this, horizontally and then also vertically (marking in the second stage). It is two times two. And then here, it will be like this. And so, three times three (marking in the third stage).

Fig. 6
figure 6

Cho’s non-digital marking of n in stages two and three in the quadratic pattern

Full size image

Peter reminded the class that, with n times n, they can calculate the number of dots in any stage of the square pattern. He did a last check by asking how many dots there would be in stage five. Several students answered twenty-five and thus collectively acknowledged Hallie’s claim and the reasons visualized by Cho that any stage of the pattern could be described algebraically by n times n.

Peter exploited the flexibility of switching to change the display, so it showed the square pattern and the hexagonal pattern at the same time (see Fig. 7). Following a didactical performance of initiation–response–evaluation (referred to in Brodie, 2011), he then asked the students for reasons about the difference between subsequent stages of the square pattern and added to this performance by drawing arcs between the stages and marking the difference over or beneath the arcs. Ardy noticed a pattern.

Peter: Can you see a pattern?

Ardy: The odd numbers.

Peter: The odd numbers?

Ardy: You add two all the time.

Gabe: It increases by plus two.

Fig. 7
figure 7

Non-digital markings explaining inferential relationships between the hexagonal and the quadratic pattern

Full size image

Peter challenged Gabe to give reasons for his claim.

Gabe: It increases by plus two. Three plus two is five, five plus two is seven.

Peter wrote “ + 2” between “3, 5 and 7” on the board (see Fig. 7). Next, he turned to the hexagonal pattern and re-marked the arcs and number of dots that had been made prior to switching to the square pattern. Hence, as can be seen in Fig. 7, what is displayed are the two patterns accompanied with non-digital markings, which make the relationships across the patterns explicit.

Peter: It seems, in some sense, that these [patterns] behave in the same way. All of you that agree on, that it seems as if they behave in the same way, those patterns, raise a hand.

Nearly all the students raised their hands. Peter continued by referring to Cho’s marking of n•n in stage two of the square pattern and then introducing base and height to mark how Cho grouped the dots. Based on that, Hallie subsequently made explicit an inference between the square pattern and the hexagonal pattern that described how they differ. “But wait, the one at the top [the hexagonal pattern], the circles or…, they cannot be calculated by base times height.” We view Hallie’s claim as part of an IM-GoGAR, as it is based on the visual structure of a hexagonal pattern and Peter’s didactical performance of exploiting the flexibility of switching and marking in patterns. Her inference also implied that, when a pattern can be structured with a base and a height, it is possible to find a general expression for the pattern. Next, we will see how Peter used Hallie’s inference to guide the IM-GoGAR by means of constructing flexibility.

A Further Example on Constructing Flexibility

As a response to Hallie, Peter responded:

Peter: Isn’t this interesting […] Say that we could in some way move the dots. Is it possible to get a base and a height with the dots?

He left this as an open question. In the reflective analysis after the lesson, he explained that his intention was to push the students on if they could transform the hexagonal pattern so that the stages took a similar visual structure as in the square pattern, that is, a shape of a base and a height (rectangular shape). He used his computer to show the students how they could manipulate the stages in the hexagonal pattern.

Peter: Now I want you to explore by moving, because these are possible to move, these (moving a dot in stage two) in some way. If it goes like, if it is possible to make these hexagonal numbers by moving these [dots] so they form a base and a height (forming a rectangular shape with his two hands).

What Peter implicitly added to the IM-GoGAR was the inference that moved from, “If we can transform the hexagonal pattern into a visual structure with base and height” to “then we can formulate a general expression of the pattern”. The students worked in their groups for 6 min. When they were done, Peter asked Cho to approach the whiteboard and explain how he could find a base and a height in the hexagonal pattern. Figure 8a shows Cho’s initial method of transforming the hexagonal pattern and his markings on the WB to indicate the base and the height in the pattern. The way in which the GoGAR is instrumental here is, among other things, that the possibility to manipulate dots takes a position in structuring the visual representation of the hexagonal pattern, in order to make sense of the algebraic expression of the pattern. It also involves the possibility of continuing a prior construction in trying to make a structure further explicit. Peter was aware of this possibility when asking the class:

Fig. 8
figure 8

a Cho’s initial transformation and marking of the base and the height in the hexagonal pattern. b Cho’s re-transformation of the hexagonal pattern

Full size image



Peter: Do any of you have a question [for] Cho, so it becomes clearer to you?

The class turned silent, so Peter asked Cho:

Peter: Now [if] you moved away all dots (see Figure 8a), would it be possible to place these dots so it would be like a pattern of, for instance, a rectangle, with all the dots [placed] so it is filled like a rectangle?

As most groups had done, Cho transformed the layout of the dots in stages two, three and four into rectangles (Fig. 8b). The episode shows that some students have made an inference between base and height and a horizontal rod at the bottom and a vertical rod to the left in the stage. In other words, they operate in an IM-GoGAR that includes the inference between a base and a height and between a square and a rectangle, integrated to the possibility to construct stages of patterns with a high degree of flexibility. Peter reminded the class:

Peter: Do you remember the square numbers, then we found squares. We found n times n, that is a square. Two times two is a square. Three times three is a square. And four times four is a square. It would be, because we saw in some way that the hexagonal numbers and the square numbers that they, maybe, are connected. Can we find, in these stages, any squares here? Talk to each other for a while.

Peter used the relationship between the hexagonal numbers and the square numbers to give reasons for how the students should continue to explore the hexagonal pattern. According to his instruction, the students continued using the flexibility in constructing and marking as instruments in finding squares in the visual structure of the stages. When the groups were done, Milan approached the whiteboard and marked squares in the pattern. Peter then asked the class to show where n is, according to the base and the height of the squares that Milan has marked (Fig. 9a).

Fig. 9
figure 9

a Non-digital markings of n defining a square in the hexagonal pattern. b Non-digital markings of n defining a rectangle in the hexagonal pattern

Full size image

Peter told the class that they had found part of the solution but that they still needed to find an expression for the dots that were outside the square (in Fig. 9a). Ardy noted that these dots form a rectangle. Peter wrote the word rektangeln (Swedish for “rectangle”) on the board (Fig. 9b) and introduced a group discussion by asking for reasons on the number of dots in the rectangle:

Peter: Here (pointing to the square), we have found an expression, n times n. How do we express this now (pointing to the rectangle in Figure 9a), by means of the number of the stage? And then, you may be able to use n.

When the groups were done, Peter asked Wilma to explain how her group came to see n in the rectangle in stage three of the hexagonal pattern.

Wilma: Here is, if it is, here is n (marking a line beneath the base with three dots) if it is stage three. And, then you take n minus one here (drawing a vertical line to the right of the same rectangle), and it is two here.

Peter complemented Wilma’s marking by drawing ellipses around the base-dots and the height-dots and then adding n and n – 1 to the two ellipses (see Fig. 9b). Another student now verbally expresses the formula, “n times n minus one”, for the number of dots in the rectangle. Peter took advantage of this contribution by drawing an inference from what the student articulated to the symbolic expression n(n– 1) (Fig. 9b). Peter continued and guided the class to conclude that n•n + n(n – 1) is the general expression of an arbitrary stage of the hexagonal pattern. He then switched the display so that it showed the first six stages of the hexagonal pattern (see Fig. 10). Utilizing switching flexibility, he pushed the IM-GoGAR by asking the students to give further reasons for the validity of the general expression by applying it to stages five and six. Nearly every group was able to apply the expression to stages five and six and to test the validity of their result by visually counting the number of dots in those stages.

Fig. 10
figure 10

The first six stages of the hexagonal pattern

Full size image

Discussion and Conclusion

This study was motivated by the need to further our understanding of orchestrating math-talk with technology that moves beyond a teaching practice of show-and-tell (Stein et al., 2008) and observational reports (Nilsson, 2019). We approached this by addressing the research question: How can collective inferential reasoning be orchestrated in a technology-enhanced learning environment? Analysis of the design experiment resulted in the FlexTech orchestration with a configuration including a shared screen on a whiteboard, a purposeful applet and the exploitation modes of flexibility in constructing, switching and marking. The FlexTech orchestration is built on a didactical configuration common to Swedish mathematics classrooms, and the study shows how the orchestration can be utilized to support the genesis of an IM-GoGAR.

A teacher’s practice is both complex and stable (Robert & Rogalski, 2005). The risk of implementing technology into their practice is to add to its complexity and challenge its stability (Drijvers, 2011). However, the present design experiment shows that technology, common to Swedish mathematics classrooms, can be used without breaking common routines that would lead to instability. In fact, the analysis shows how the exploitation modes of the FlexTech orchestration can support teachers’ didactical performance to increase stability, in terms of guiding the mathematics, making student’s ideas explicit and orchestrating the genesis of an IM-GoGAR.

To elaborate on the significance and usability of the present study, we discuss how the flexibility to construct, switch and mark can provide support to teachers’ didactical performance to orchestrate an IM-GoGAR. We connect the discussion to the three overall kinds of supporting actions identified by Staples (2007): supporting students in making contributions, establishing and monitoring a common ground and guiding the mathematics.

Constructing Flexibility

Research suggests that teachers in mathematics should strive to create a teaching practice that builds on students’ active engagement and contributions and encourages them to develop and share their reasoning (Hufferd-Ackles et al., 2004). A teacher should elicit and scaffold students’ ideas (Staples, 2007) and make their underlying reasoning explicit (Nilsson, 2019), in order to establish a common ground for collective reasoning (Nilsson & Ryve, 2014; Staples, 2007). In the present study, the teachers could act on a digital flexibility in constructing patterns. For instance, the constructing flexibility enabled the teacher to orchestrate near and far generalizations of a pattern by adding subsequent pattern stages or changing the visual structure of the stages. The didactical configuration allowed the teacher to add new stages to the hexagonal pattern rapidly. It also provided the teacher and the students with an opportunity to integrate construction and manipulation of patterns to the IM-GoGAR on visual structure reasoning. Within this IM-GoGAR, the teacher then guided the class through a linear perception of a pattern towards a non-linear perception.

Students need to be prompted for justifications for their reasons when working with open tasks (Kosyvas, 2016), such as those used in Lessons 2 and 3. On this account, the analysis showed how the possibility to transform the hexagonal pattern into a rectangular pattern supported students to make sense of a general expression of the hexagonal pattern by contrasting the pattern with the square pattern. Much like the situation with drag tools in dynamic geometry environments (Leung et al, 2006), the FlexTech orchestration invites exploration of different arrangement, compared to an orchestration with paper and pencil.

Shared screens enabled the teachers to use the software to give and ask for reasons. In group settings, students’ screens acted as shared screens allowing the teacher to engage with and challenge groups of students, which counteracted the effect where individual units inhibit communication, as shown by Doerr and Zangor (2000). Shared screens in group settings also enabled Peter to monitor and select students for whole-class discussions. The example with Cho shows how flexibility in constructing on shared screens enabled Peter to select a student (Cho) to develop his/her idea further in a whole-class setting to guide the IM-GoGAR.

Marking Flexibility

For the changes that require students’ active engagement in the construction of mathematical knowledge, teachers need to “step back in controlling students’ learning activities” (Hoekstra et al., 2009, p. 664). Though teachers need to step back from controlling students’ learning, evidence shows that teachers also need to guide and help students in advancing and structuring the content to be learned (Stein et al., 2008). We argue that marking flexibility permits teachers to balance these two needs. An illustrative example appeared when the class focused on finding a general expression of any stages in the square pattern. To figure out n•n was not an easy task for the class. However, by being able to highlight visual structures (Rivera, 2010) in the applet and communicate their reasoning by marking the base and the height of the stages in the square pattern, students came to understand the meaning of n. The orchestration encouraged students to be active at the whiteboard by interacting with the projected image of the applet. The teacher stood next to the whiteboard and asked for reasons for markings, by using the strategy of active re-voicing (Eckert & Nilsson, 2017), to emphasize important knowledge and ideas. Hence, the present study shows how didactical configurations that allow for marking flexibility (by digital as well as non-digital means) on a public screen can provide teachers means for balancing processes of controlling and guiding the mathematics in orchestrating an IM-GoGAR.

Switching Flexibility

To succeed in eliciting and extending students’ mathematical reasoning, a teacher should encourage the mathematical activities of analysing, making connections and generalizing (Fraivillig et al., 1999). Of course, flexibility in constructing and marking are important to such processes as well, but we claim that flexibility in switching provides specific opportunities to promote these processes. For instance, the opportunity to switch between the hexagonal and the quadratic pattern provided the teachers opportunities to use the familiar quadratic pattern as a frame of reference in making sense of the hexagonal pattern.

Switching flexibility connects to variation theory (Marton et al., 2004), which claims that a resource that allows for switching offers teachers certain opportunities to guide the mathematics (Staples, 2007) through patterns of contrast and comparison between tasks, representations and student solutions. We recognize that switching between tasks, representations and student solutions can be done with non-digital means. However, we claim that flexibility in switching in a digital didactical configuration can provide a flexibility to exploit switching even when it is not planned for. In this account, based on our findings, we argue that switching in a technology-enhanced learning environment can provide a teacher with a certain opportunity for her/his didactical performance to guide an IM-GoGAR.

We end the discussion by briefly reflecting on the argument that the FlexTech orchestration does not make the teaching overly complex and unstable and by pointing out suggestions for further research based on these reflections. The reason the technology provided support was because it added only a limited amount of complexity to the teaching practice. The orchestration builds on a didactical configuration which is common in Swedish mathematics classrooms: laptops, a projector and an ordinary (non-digital) whiteboard. The software used was also simplified. Even though GeoGebra was used in the experiment design, its entire functions were not made available to the students. The software was customized to the specific learning goals, which reduced the complexity of the environment and did not encourage the students to turn their attention away from the tasks. Moreover, the threshold of learning to use the technology was low, because it was not entirely new to the teachers or the students. Hence, the technology was used in a manner that was in line with a teaching practice the teacher believed in and one with which the students were familiar.

Drijvers (2011) calls for further research on investigating new repertoires of instrumental orchestrations. The FlexTech orchestration is one contribution to this call. However, our results also indicate the need to investigate how technology should be designed to be rich enough to invite and enable processes of exploration and investigation, without losing sight of the mathematics. We propose the three modes of flexibility characterized in the present study stand as a good starting point for such investigations. Flexibility in constructing provides teachers with a resource in designing and sequencing tasks and in developing the task in the moment. Flexibility in switching supports how teachers often work with patterns of variation in teaching, which has been formalized and theoretically underpinned in variation theory (Marton et al., 2004). Flexibility in marking contributes to stability in relation to working between digital and non-digital technology.

Being able to mark by non-digital means on the whiteboard was important for highlighting significant content and making reasons explicit. The same effect could have probably been achieved with completely digital means. However, trying to achieve the same effect with only digital means would have increased the technical complexity of the situation, which likely would have reduced the pace and flow of the teaching. Hence, the present design experiment raises questions about the balance between complexity and stability and between teacher-centred and student-centred teaching.

On this account, we encourage researchers to investigate patterns of instrumental orchestration of IM-GoGAR further. We particularly invite them to explore how the FlexTech orchestration can be used as a framework in designing instruction within other areas of mathematics. Connected to the promises of the flexible marking mode, we also encourage further exploration of the bi-directional relationships between digital and non-digital learning technology in the design of an instrumental orchestration of IM-GoGAR.

Finally, the three modes of flexibility may well extend beyond fine-grained analyses that show the role of technology in IM-GoGAR. The modes may also have the potential to help teachers examine their own use of technology. It may help them to see the ways digital technology can be used in combination with non-digital technology effectively to guide learners in developing IM-GoGARs for making sense of and solving tasks in mathematics.