1 Introduction

The foundation is an essential component of the structures as it supports and transfers loads from the superstructure to the underlying soil. A well-built base spreads the loads from the superstructure in such a way that the underlying soil is not highly stressed. The overburdening of soil may result in the settlement of structure or shear failure of the soil. The bearing capacity of the soil is the amount of pressure at which the supporting soil is anticipated to fail in shear. The bearing capacity can be calculated using analytical methods or experimental studies. In recent years, several investigations have been performed on determining the bearing capacity factor Nγ for the strip, circular and conical footings. Conical footings represent spudcan foundations which are generally employed for different offshore structures. Cassidy and Houlsby (2002) provided the solution for finding the load-carrying capacity factors for smooth and rough conical footings resting on the surface of sand following the method of characteristics. In their study, sand friction angles were kept between 5 and 50°, while cone apex angles ranged between 30 and 180°. Lyamin et al. (2007) calculated the bearing capacity of strip, square, circular, and rectangular foundations in sand using finite-element limit analysis for frictional soils following an associated flow rule. Based on analytical results, shape, and depth factors were proposed to determine the bearing capacity of foundations embedded in the sand. Khatri and Kumar (2009) examined the effect of the apex angle β of the conical footing on the bearing capacity factor Nγ by varying the friction angle (ϕ) of sand, obeying an associated flow rule. The influence of β on the plastic zones was also investigated. In another study, Kumar and Khatri (2011) used the lower bound limit analysis in conjunction with finite element and linear programming to calculate the bearing capacity factors for a circular footing due to cohesion, surcharge, and unit weight with various values of ϕ. Chakraborty and Kumar (2015) estimated the bearing capacity factors, Nc, Nq, and Nγ, for numerous combinations of β, ϕ, and δ, using the lower and upper bound axisymmetric formulation of limit analysis combined with finite elements and linear optimization. The variations of Nc, Nq, and Nγ in a bound form for various combinations of ϕ and δ were illustrated and compared to Houlsby and Martin’s stress characteristic solution (Houlsby and Martin 2003). Furthermore, Chakraborty and Kumar (2016) explored the dependence of Nc on footing diameter for a conical foundation utilizing limit analysis in combination with finite elements and linear programming for different cone apex angles. The investigation was carried out using two well-defined ϕ versus σm curves from the literature. According to the analysis, the magnitude of Nγ diminishes with the increase in footing diameter (B) for all cone apex angles. For a rough footing, the decrease of Nγ with an increase in B becomes more widespread. With B greater than 0.45 m approximately for a smooth footing and 0.85 m for a rough footing, the factor Nγ diminishes almost linearly with an increase in B on a log–log scale for which a simplified expression was generated relating Nγ and B. The reduction in Nγ with an increase in B becomes minimal for B bigger than about 8.0 m.

Keawsawasvong (2021a) proposed a novel plastic solution of the bearing capacity factor for conical footings on clays with linearly increasing anisotropic shear strength. With the increase in strength gradient ratio or anisotropic strength ratio, an increase in the bearing capacity factor was reported. But, with the increase in cone apex angle reduction in bearing capacity factor was observed. In another study, Keawsawasvong (2021b) proposed new plastic solutions for the bearing capacity factors of conical footings on rock masses obeying the Hoek–Brown yield criterion. A conical footing was penetrated into a rock mass. The effect of the cone apex angle, the yield parameter, and the geological strength index of a rock mass on the bearing capacity factors were evaluated. Lai et al. (2022) evaluated the bearing capacity of conical footings embedded in anisotropic and inhomogeneous clays using the NGI-ADP model. The bearing capacity factor of conical footings was defined as function of cone apex angle, strength gradient ratio, and anisotropic shear strength ratio. Phuor et al. (2022) computed of the vertical bearing capacity factors a rough conical footing placed on the soil with friction angle ranging from ϕ = 5 to 45° by using the FE-based viscoplastic strain method under the Mohr–Coulomb (MC) yield criterion. It was observed that numerical stability with a high value of friction angle could be improved to some extent by employing the proper values of soil dilation angle. The bearing capacity factors were slightly decreased with the increase of the cone apex angle β = 60° to β = 180°. However, considerable increment in bearing capacity factors was reported with the decrease of β = 60° to β = 30°. Hu et al. (2022) investigated the horizontal bearing capacity factors for conical footing on clay, taking the effects of embedment ratio, foundation–soil interface roughness, conical angle, and soil strength heterogeneity in the account. At a specific embedment, an increment in horizontal bearing capacity factors was reported with strength gradient ratio, but with the increase in cone apex angle, decrement in bearing capacity factors was seen. The roughness factor had the least effect on the bearing capacity factors.

In the past, very little literature has been available on the bearing capacity of rough conical shallow footing resting on the sand. The shallow conical footing laying on the sand at various embedment ratios has not been examined thoroughly, utilizing different friction angles, and cone apex angles.

The present study uses the finite element method to determine the collapse loads for conical footings placed on the sand. The bearing capacity values were determined for various combinations of friction angles (ϕ) and cone apex angles (β) with different embedment ratios. The results obtained from the analysis were compared with those available in the literature.

2 Methodology

In the finite element method, the solution usually refers to the determination of the displacements at each node and the stresses inside each element in the structure subjected to the applied loads. The bearing capacity of a conical foundation lying on the sand was calculated using the finite element methods employing Optum G2. (2019) using the method suggested by Khatri et al. (2022). The footing was modeled with this software using the six-noded triangular gauss element and dividing the whole domain into 10,000 elements. The axisymmetric analysis was performed by utilizing a typical domain in the r-z plane.

During the analysis for conical footing, the axisymmetric condition was considered. The cylindrical coordinates are adopted with r, z, and θ representing radial, vertical, and circumferential directions. There are four non-zero stresses (σr, σz, σθ, and τrz), four non-zero strains (εr, εz, εθ, and ϒrz), and two displacements (ũ and w′) in the r and z-direction, respectively. There is no displacement in the θ direction owing to the symmetry. The displacements in the r and the z directions are independent of θ. Thus the strains and stresses are reduced to as shown in Eqs. (1) and (2), respectively.

$$\gamma r\theta =\gamma z\theta =0$$
(1)
$$\tau r\theta =\tau z\theta =0$$
(2)

The strain–displacement relationships are as follows:

$$\varepsilon r=\frac{\partial u\mathrm{^{\prime}}}{\partial r\mathrm{^{\prime}}},\text{\hspace{0.17em}\hspace{0.33em}}\varepsilon z=\frac{\partial w\mathrm{^{\prime}}}{\partial z\mathrm{^{\prime}}},\text{\hspace{0.33em}}\varepsilon \theta =\frac{\widetilde{u}}{{r}^{\mathrm{^{\prime}}}},\text{\hspace{0.17em}}\gamma rz=\frac{\partial w\mathrm{^{\prime}}}{\partial r\mathrm{^{\prime}}}+\frac{\partial \widetilde{u}}{\partial z}$$
(3)

Every two-dimensional axisymmetric problem was required to satisfy the equilibrium stresses given by Eq. (3) and Eq. (5).

$$\frac{\partial \sigma r}{\partial r}+\frac{\partial \tau rz}{\partial z}+\frac{(\sigma r-\sigma \theta )}{r}=0$$
(4)
$$\frac{\partial \tau rz}{\partial r}+\frac{\partial \sigma z}{\partial z}+\frac{\tau rz}{r}=\gamma$$
(5)

Equations (1–5) can be used for the creation of the stiffness matrix, with the use of shape function for a six-noded triangular element and the relevant constitutive relationships (Potts et al. 2001).

The soil was modelled assuming as Mohr–Coulomb material and followed the non-associated flow rule. The soil domain was discretized using six noded finite triangular elements (linear strain triangle LST), with nodal displacements as unknown variables. The differential equation, strain compatibility equation, and boundary conditions for plane strain and axisymmetric analysis are already reported in Khatri et al. (2022). The strip footing follows the plain-strain condition during its analysis, wherein the strains and stresses considered are given by Eqs. (6) and (7), respectively. Here, the x, y, and z represent the three directions in the cartesian coordinate system, and u′ and v′ implies the displacements in the x and y directions. εx, εy, and εz are known as the longitudinal strains in the x, y, and z directions, respectively, whereas ϒ is called the shear strain. Similarly, σ and τ represent normal and shear stresses.

$$\varepsilon z=\gamma xz=\gamma yz=0$$
(6)
$$\tau xz=\tau yz=0,but\sigma z\ne 0$$
(7)

Furthermore, the strain–displacement relationships for the plane-strain condition are as follows:

$$\varepsilon x=\frac{\partial {u}^{\mathrm{^{\prime}}}}{\partial {x}^{\mathrm{^{\prime}}}},\varepsilon y=\frac{\partial {\nu }^{\mathrm{^{\prime}}}}{\partial {y}^{\mathrm{^{\prime}}}},\gamma xy=\frac{\partial {u}^{\mathrm{^{\prime}}}}{\partial {y}^{\mathrm{^{\prime}}}}+\frac{\partial {\nu }^{\mathrm{^{\prime}}}}{\partial {x}^{\mathrm{^{\prime}}}}$$
(8)

The basic differential equations to satisfy plane strain conditions are given by Eqs. (9) and (10), where the parameter ν represents the Poisson’s ratio.

$$\frac{{\partial }^{2}{u}^{^{\prime}}}{\partial {x}^{2}}+\frac{{\partial }^{2}{u}^{^{\prime}}}{\partial {y}^{2}}=\frac{1+\nu }{2}\left(\frac{{\partial }^{2}{u}^{^{\prime}}}{\partial {y}^{2}}-\frac{{\partial }^{2}{\nu }^{^{\prime}}}{\partial x\partial y}\right)$$
(9)
$$\frac{{\partial }^{2}{\nu }^{^{\prime}}}{\partial {x}^{2}}+\frac{{\partial }^{2}{\nu }^{^{\prime}}}{\partial {y}^{2}}=\frac{1+\nu }{2}\left(\frac{{\partial }^{2}{\nu }^{^{\prime}}}{\partial {x}^{2}}-\frac{{\partial }^{2}{u}^{^{\prime}}}{\partial x\partial y}\right)$$
(10)

It is pertinent to note that an additional plane strain analysis for strip footing is performed herein to determine shape and depth factors for conical footing in line, similar to Lyamin et al. (2007).

3 Problem Domain, Mesh Details, and Material Properties

Optum G2 software was used to conduct this numerical-based analysis. In this regard, the details regarding the problem domain, mesh, material properties used, and methodology are described in the following sections.

3.1 Problem Domain

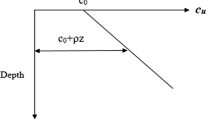
For the sake of presentation, a rough conical footing embedded in the soil domain (represented by ABCE) is shown in Fig. 1, where the load (Q) acts in the downward direction. The horizontal displacement (u) along the line of symmetry (line “AB” in figure) and far-off boundary (line “CE” in figure) was kept at zero. In contrast, along the bottom boundary “BC,” the horizontal displacement (u) and vertical displacement (w) were stated as zero. The parameter B, the diameter of the conical footing, was taken as 2 m. The parameter “t” means the thickness of the footing, which was taken as 0.25 m. L and V denote the length and depth of the selected domain. The values of L and V were computationally arrived at by using several trials like (i) the limits of the plastic zones fall well within the boundaries of the specified problem domain, and (ii) the magnitude of the collapse load remains almost constant even if the domain size was increased further. Approximately the L and V values were kept equal to 5B. No movement was enabled in either the vertical direction (w) or the horizontal direction (u) at the horizontal bottom of the selected domain. A six-noded element was used to model the soil and the footings.

Fig. 1
figure 1

Line diagram of conical footing embedded in the soil

Full size image

3.2 Mesh Details

The whole domain was discretized into the number of elements resulting in meshes. In a given mesh, finer elements accumulate in a region wherein shear failure is evident. The meshes are generated for conical footing with different Df/B values for various β. At ϕ = 40°, the mesh details for conical footing at Df/B = 0 and 1 with β = 30°, β = 90° and β = 180° is shown in Fig. 2. Note that the adaptive finite element meshes with a zone of finer elements shown in Fig. 2 indirectly reflect the failure patterns.

Fig. 2
figure 2

Mesh details for conical footing at ϕ= 40°

Full size image

3.3 Material Properties

The properties of the material used in this analysis are as per Das et al. (2022). The properties of footing are listed in Table 1. The Modulus of elasticity of footing is calculated by E(MPa) = 5000 × (fck)0.5, where fck is the characteristic compressive strength of concrete, and in the present study, it was taken as 20 N/mm2.

Table 1 Properties of footing (Das et al. 2022)
Full size table

The soil is assumed to be elastoplastic and follows the Mohr–Coulomb failure criterion and the non-associated flow rule (ψ < ϕ). Furthermore, the dilation angle was taken as ψ = (ϕ-30°). The properties of sand are listed in Table 2 (Bowles 1996).

Table 2 Properties of sand (Bowles 1996)
Full size table

4 Results and Discussion

The finite element analysis was conducted for conical footing lying on the sand, obeying the non-associated flow rule for various depth ratios (Df/B). The friction angle of sand is varied from 25 to 45°. In each case, the bearing capacity was estimated from the pressure-settlement curves. Furthermore, the bearing capacity was articulated using a non-dimensional parameter termed the bearing capacity ratio (BCR), which is a ratio of the bearing capacity of the conical footing to the bearing capacity of the circular footing. The BCR was calculated for two different scenarios: (1) at failure (BCRu) and (2) at the settlement of 10% (BCR10). Note that the term BCR signifies the bearing capacity improvement brought out with the use of conical footing instead of the circular footing of a similar diameter. A reduction in settlement with the use of conical footing in place of circular footing at a given pressure was arrived at in terms of a settlement reduction factor (SRF), defined in the subsequent section. Like BCR, SRF has been defined as corresponding to the pressure at failure and S/D = 10% of circular footing and termed as SRFu and SRF10, respectively. The variations of all the above parameters are explained in subsequent sections.

4.1 Pressure-settlement variation

Figure 3(a)–(i) illustrate the variation of normalized pressure with normalized settlement for conical foundation resting over sand for different ϕ with β = 30°, 90°, and 180° at Df/B = 0, 0.5, and 1. A normalized or equivalent pressure is found by fractioning the load-carrying capacity of the footing by (γB) and normalized settlement (S/D) is calculated by fractioning the settlement with the diameter of the footing (D). From the obtained plots, it can be observed that the bearing pressure for a given friction angle decreases on increasing apex angle. Also, the bearing pressure increases on increasing embedment depths. The ultimate bearing capacity of footing was determined using the pressure settlement plots using a double tangent method (Khatri et al. 2022). Additionally, the bearing pressure corresponding to S/D = 10% was determined. Using the magnitude of ultimate bearing capacity and bearing pressure corresponding to S/D = 10%, the terms BCRu and BCR10 were computed as defined earlier.

Fig. 3
figure 3

Variation of Q/(AbγD) with S/D (%) for different ϕ with β = 30°, 90°, and 180° at Df/B = 0, 0.5, and 1

Full size image

4.2  Variation of BCRu and BCR10 with β, Df/B and ϕ

Figures 4 and 5, respectively, show the variation of BCRu and BCR10 for various β and ϕ with Df/B = 0, 0.5, and 1. It is worth noting that the bearing capacity decreases on increasing the cone’s apex angle (β). The bearing capacity of conical footing is maximum at β = 30°. Accordingly, the BCRu and BCR10 are maximum at β = 30°. For β = 30°, bearing capacity improvement at failure (BCRu) over circular footing is in the range of 1.25 to 4.5, considering all the friction angles and embedment ratios. In contrast, the bearing capacity improvement corresponding to S/D = 10% (BCR10) ranges from 1.15 to 3.25.

Fig. 4
figure 4

Variation of bearing capacity ratio for different β and different ϕ with a Df/B = 0, b Df/B = 0.5, c Df/B = 1

Full size image
Fig. 5
figure 5

Variation of bearing capacity ratio for different β and different ϕ at failure corresponding to S/D = 10% for a Df/B = 0, b Df/B = 0.5, c Df/B = 1

Full size image

A close observation of Figs. 4 and 5 suggests that the BCRu and BCR10 are maximum for a lower value of friction angle for surface footing (Df/D = 0). However, with an increase in embedment ratio, the trend is reversed. It can be reasoned that in the case of surface footing, a significant improvement is brought out in bearing capacity by changing the type of footing from circular (β= 180°) to conical (β= 30°) alone. However, as embedment depth increases, the bearing capacity improves due to soil shear strength above the base level of the foundation overweighing the change in footing type. Accordingly, the BCRu and BCR10, in the case of embedded footing (Df/D > 0), were higher for a greater friction angle in contrast to the surface footing. Using Figs. 4 and 5, one can select a conical footing of the appropriate apex angle over a circular footing if an improvement in bearing capacity is desired, while friction angle and embedment depth are held constant.

4.3 Variation of SRF at Failure and SRF at 10% Settlement with β at DifferentEmbedment Ratios for ϕ

SRF (settlement reduction factor) is defined as the fraction of the difference in the settlement between the conical footing and the circular footing to the settlement of the circular footing defined at a given pressure. Numerically the SRF is calculated by -:

$$SRF=\left[\frac{{\left(\frac{S}{B}\right)}_{\mathrm{circular}}- {\left(\frac{S}{B}\right)}_{\mathrm{conical}}}{{\left(\frac{S}{B}\right)}_{\mathrm{circular}}}\right]\times 100$$
(11)

The present study defines the SRF as corresponding to pressure i) at failure state (SRFu) and ii) S/D = 10% (SRF10) of circular footing. Figure 6a and b show the variation of SRFu with β at failure for different ϕ at Df/B = 0 and 1, respectively. It can be observed that SRFu decreases on increasing apex angle. It implies that compared to circular footing, the conical footing is more efficient in settlement reduction apart from improvement in bearing capacity. As shown in Fig. 6a, the maximum reduction in settlement for a conical footing with a given apex angle was seen for the footing placed on a surface with the highest friction angle of sand, i.e., ϕ = 45°. However, the SRFu trends for an embedded footing (Df/D = 1) were in reverse order, with the SRF being greatest at the lowest friction angle. It follows that, in terms of settlement reduction, a conical foundation embedded at a given depth will be a viable option, particularly in soil with low shear strength. At β = 30°, settlement reduction over circular footing is in the range of 60 to 80% considering all the friction angles and embedment ratios.

Fig. 6
figure 6

Variation of settlement reduction factor with β at failure for different ϕ at a Df/B = 0, b Df/B = 1

Full size image

Figure 7a and b show the variation of SRF10 corresponding to S/D = 10% with β for different ϕ at Df/B = 0 and 1, respectively. From the curves, it is observed that on increasing the β, SRF decreases which also means improvement in settlement reduction of conical footing decreases with increasing β. At β = 30°, the settlement reduction over circular footing is in the range of 55 to 75% considering all the friction angles and embedment ratios. The settlement reduction over circular footing is maximum for a lower value of friction angle. For a given value of β and ϕ, the SRF10 decreases with an increase in the embedment ratio. Furthermore, SRF10 values were marginally smaller than SRFu.

Fig. 7
figure 7

Variation of settlement reduction factor for different β and different ϕ at failure corresponding to S/D = 10% for a Df/B = 0, b Df/B = 1

Full size image

4.4 Variation and Comparison of Nγ and Nq

From the ultimate bearing capacity obtained using the pressure settlement curve for a footing placed on a surface, the bearing capacity factor Nγ was estimated. Table 3 shows the comparison of Nγ values for conical footing placed on the sand obtained from the present study with Khatri and Kumar (2009). The present Nγ values nearly match the results of Khatri and Kumar (2009). Similarly, the present Nq variation is compared with the result obtained by Lyamin et al. (2007) for strip footing placed on the ground, as shown in Table 4. The result of the present study in terms of Nq is marginally less than those of Lyamin et al. (2007). The Nγ and Nq values obtained in the present study are smaller due to the use of the non-associated flow rule but comparable.

Table 3 Comparison of Nγ values for conical footing placed on the sand
Full size table
Table 4 Comparison of Nq values for strip footing placed on the sand
Full size table

4.5 Variation and Comparison of sγ and sq

The bearing capacity of embedded conical footing can be expressed as:

$${q}_{ult}={qN}_{q}{s}_{q}{d}_{q}+0.5\gamma {BN}_{\gamma }{s}_{\gamma }{d}_{\gamma }$$

In the above expression, the term sq and sγ take care of the plan shape of the footing, while dq and dγ relate to the increase of shear strength with depth which was not accounted for by Terzaghi (1943). The Nq and Nγ in the above equation correspond to the strip footing. Note that if the foundation is placed on a surface, the first term in the above expression related to the surcharge becomes zero. Furthermore, the depth factor dγ in this study was taken as 1, consistent with Lyamin et al. (2007).

Hence, to determine the shape factor sγ, the bearing capacity of conical footing placed on the surface was divided by the bearing capacity of surface strip footing. In this regard, an additional analysis was performed to determine the magnitude of Nγ of strip footing by keeping the width of strip footing (B) equal to the diameter of conical footing (B). Following the above, in the present study, the shape factor sγ was computed for both circular and conical footing, and its variation with cone apex angle (β) was presented. Numerically, the shape factor can also be defined as

$${s}_{\gamma }=\frac{{{(N}_{\gamma })}_{\mathrm{conical}}}{{({N}_{\gamma })}_{\mathrm{strip}}}$$
(12)

The variation of sγ with β and ϕ is shown in Table 5. From this table, it is worth noting that on increasing the apex angle of the cone, the sγ value decreases, which means that on increasing β, the bearing capacity decreases for footing placed on the surface. Furthermore, the comparison of present sγ for circular footing (β=180°) with Lyamin et al. (2007) is also shown in the same table. The current sγ values for circular footing (β=180°) seem to almost match with Lyamin et al. (2007). From Table 5, it can also be observed that the shape factor sγ increases with an increase in friction angle (ϕ) for any given value of β.

Table 5 sγ values calculated for conical footing
Full size table

The shape factor sq corresponding to various embedment depths is calculated by taking a ratio of the difference in the bearing capacity of conical footing at a given embedment depth to the bearing capacity of the footing placed on a surface to the bearing capacity of strip foundation at the same embedment depth (Eq. 13). Numerically the expression of sq is given as follows:

$${s}_{q}=\left[\frac{{q}_{b}-(0.5\times {\mathrm{s}}_{\gamma }\times \gamma \times \mathrm{B}\times {N}_{\gamma })}{{(d}_{q}\times q\times {N}_{q})}\right]$$
(13)

The term qb refers to the bearing capacity of embedded conical footing, while the term 0.5 sγBγNγ relates to the bearing capacity of conical footing placed at the surface. The shape factor sγ is defined in the previous section. The variation of sq for the range of ϕ, Df/B, and ϕ is depicted in Fig. 8a–f. From Fig. 8a–f, it has been observed that on sq value decreases with an increase in apex angle and increases with a rise in friction angle. On increasing embedment depth for a particular value of friction angle, the sq value increases. Accordingly, the bearing capacity also increases. The comparison of present sq values with Lyamin et al. (2007) for circular footing (β = 180°) is indicated in Fig. 9. The current sq values were slightly smaller but comparable with that reported by Lyamin et al. (2007) due to the use of the non-associated flow rule.

Fig. 8
figure 8

Variation sq vs Df/B curves for different ϕ at β a 30°, b 60°, c 90°, d 120°, e 150°, and f 180°

Full size image
Fig. 9
figure 9

Variation and comparison of Sq vs Df/B with different ϕ for circular footing

Full size image

4.6 Variation and Comparison of dq

In the bearing capacity equation, the Nγ term relates to the slip mechanism that develops beneath the footing’s base. It means that the surcharge term, together with depth factor dq captures the impacts of the portion of the mechanism that extends above the base of the footing. For determining dq, the analysis was first performed by embedding the strip footing at different depths, and subsequently, the ultimate bearing capacity was computed using the pressure-settlement curve. Following this, the bearing capacity of the surface footing (0.5BγNγ) was subtracted from the bearing capacity corresponding to the embedded footing. This difference in bearing capacity (qNqdq) is related to the component on account of surcharge due to shear strength above the foundation’s base level. Accordingly, the depth factor dq, which takes care of the shear strength above the foundation’s base level, was determined by dividing the term qNqdq by the bearing capacity of surface strip footing subjected to surcharge, i.e., qNq.

Numerically dq is defined as the depth factor calculated for different embedment depths at different φ by:

$${d}_{q}=\left[\frac{{q}_{bstrip}-\left(0.5\times B\times \gamma \times {N}_{\gamma }\right)}{(q{\times N}_{q})}\right]$$
(14)

where qbstrip is the bearing capacity of the strip foundation at failure for different embedment depths, q is the surcharge (γDf), and Nγ and Nq are the bearing capacity factors for strip footing resting on the ground.

The dq values obtained as per the above-mentioned procedure were compared with those reported by Lyamin et al. (2007). This comparison is shown in Table 6, which suggests that the present dq values were similar to that reported by Lyamin et al. (2007).

Table 6 Comparison of dq values for strip footing on homogeneous sand at various embedment depths
Full size table

4.7 Failure Patterns

The generated failure patterns for conical footing with ϕ = 35° and Df/B = 0 and 1 at β = 30°, 60°, 90°, 120°, 150°, and 180° are shown in Fig. 10a–f and Fig. 11a–f, respectively. The parameter indicated in Fig. 10a–f is the strain in the form of |ε1,p–ε3,p|. The dark red color in this figure implies the material is at failure. Alternatively, the adaptive finite element meshes shown in Fig. 2 indirectly reflect the failure patterns. The failure patterns generally serve two purposes: (1) to visualize the occurrence of failure and (2) to verify the correctness of the obtained results. It should also be noted that the curvilinear collapse pattern or rupture surface was always covered well within the stipulated vertical and horizontal range. It indicates that the analysis domain chosen was adequate. From the failure patterns, it can be seen that the failure surface is not well developed and is not reaching the ground. So, it can be concluded that there may be a possibility of local shear failure.

Fig. 10
figure 10

Generated failure patterns for conical footing with ϕ = 35° and Df/B = 0 at β a 30°, b 60°, c 90°, d 120°, e 150°, and f 180°

Full size image
Fig. 11
figure 11

Generated failure patterns for conical footing with ϕ = 35° and Df/B = 1 at β a 30°, b 60°, c 90°, d 120°, e 150°, and f 180°

Full size image

5 Conclusions

  • Bearing capacity decreases on increasing the cone’s apex angle (β), with the maximum at β = 30°.

  • At β = 30°, bearing capacity improvement over circular footing was in the range of 1.25 to 5, considering all the friction angles and embedment ratios.

  • At a given pressure, settlement increases on increasing the apex angle of the cone and becomes minimum at β = 30°.

  • At β = 30°, settlement reduction over circular footing is in the range of 60% to 80%, considering all the friction angles and embedment ratios.

  • The bearing capacity improvement and settlement reduction over circular footing are maximum for lower friction angle values.

  • For a value of β and ϕ, the BCR decreases with an increase in the embedment ratio.

  • The bearing capacity factors from the present study were marginally smaller than that reported in the literature, while the shape and depth factors were comparable. It implies that the flow rule only affected the bearing capacity factors but not the shape and depth factors.