1 Introduction

The evolution of small- and large-amplitude IASWs in plasmas has been a recent focus of theoretical and experimental investigation. Many investigators have been drawn to the field by the IASWs that may arise under various physical conditions in cold or warm, magnetized or unmagnetized plasmas that also contain positive and negative ions in addition to the normal electrons. The Korteweg–de Vries (KdV) equation arises from a reductive perturbation approach aimed at describing SWs with small yet finite amplitudes. In contrast, the SP [1] approach is employed to study finite amplitude SW structures. Previous research on IA solitons in unmagnetized and magnetized plasmas has produced intriguing theoretical and experimental findings [2,3,4,5,6,7,8,9]. Investigating the features of small-amplitude nonlinear IASWs in a warm magnetoplasma with negative–positive ions and non-thermal electrons led to the Zakharov–Kuznetsov equation (ZK) as a relevant description. The consequence of relativistic effects on the development of SWs has been extensively studied in various contexts, such as laser plasma interactions and astronomical models [10,11,12]. Das and Paul [13] conducted a study on relativistic solitons in an ion–electron plasma based on initial streaming, while Chatterjee and Roychoudhury [14] employed the SP approach with electron inertia to investigate the effect of ion temperature in a relativistic plasma. Additionally, Roychoudhury et al. [15] explored the influence of ion and electron drifts on SW occurrence in relativistic plasma. The pseudopotential method has been widely used in past research on SWs and sheath structures in plasmas due to its high effectiveness [16,17,18,19,20,21,22,23,24,25,26,27,28]. Solitons are crucial nonlinear excitations with numerous applications in nonlinear optics and communication technologies. Kalita et al. [29] ingeniously induced the formation of rapid IA mode compressive solitons in a magnetized plasma, comprising relativistic ions, electrons, and beam ions drifting perpendicularly to the magnetic field. Lee and Choi [30] studied IASWs in a relativistic plasma composed of hot electrons and cool ions using SP approach and a set of completely relativistic two-fluid equations. Alinejad [31] investigated solitons' existence, production, and characteristics in a multicomponent dusty plasma, while Tiwari et al. [32] explored the dynamics of highly charged large dust grains and IA soliton properties in dusty plasma using the Sagdeev potential approach. El-Labany et al. [33] used the Sagdeev potential approach to investigate large-amplitude dust IASWs in a dusty plasma with non-thermal electrons. In the research on nonlinear dust acoustic waves [34], it was found that huge-amplitude rarefactive and compressive solitons occur in an unmagnetized dusty plasma with the effects of vortex-like and non-thermal ion distributions. Additionally, the SP approach was employed to analyze dust acoustic solitary waves (DASWs) in dusty plasma [35] considering the changing non-thermal component in the non-thermal distribution of ions. Kamalam and Ghosh [36] used the SP method to study the behavior of a single ionic acoustic wave in a magnetized plasma made up of warm fluid ions and two types of electrons with different temperatures in the Boltzmann distribution. Also, Nooralishahi and Salem [37] explored the nonlinear behavior of high-amplitude electromagnetic SWs in a magnetized electron–positron plasma through a comprehensive relativistic two-fluid hydrodynamic model. In a separate study, Mushinzimana and Nsengiyumva [38] delved into the characteristics of large-amplitude IA fast mode SWs in a negative ion plasma with kappa electrons, employing the SP method. Their findings revealed that specific parameter values allowed for the coexistence of two distinct structure types, facilitating the propagation of rarefactive and compressive solitons in this plasma. More recently, Almas et al. [39] studied the oblique propagation of arbitrary IASWs in magnetized electron–positron–ion plasmas using the pseudopotential approach. They investigated the effects of various plasma configuration factors on soliton properties in the plasma system, such as positron concentration and parallel and perpendicular ion pressure. It is demonstrated that wave propagation, temperature ratio, and relativistic factor significantly influence solitary excitations. Therefore, the practical interest lies in exploring the impact of non-thermal electrons on the characteristics of IASWs in a negative–positive ion magnetoplasma.

In this study, we embark on a theoretical investigation to explore the possibility of the presence of high-amplitude IASWs in a magnetized plasma comprising warm ions and relativistic electrons. Our main findings indicate that the present plasma system is capable of accommodating both compressive subsonic solitons and rarefactive supersonic and subsonic solitons. The discussion of the normalized nonlinear basic equations can be found in Sect. 2, while in Sect. 3, we present the derivation of the energy integral, enabling the study of SWs with arbitrary amplitudes. Computational results for nonlinear structures in various parametric ranges are examined in Sect. 4. Finally, our overall conclusions are summarized in Sect. 5.

2 Basic equations

We examine the behavior of IA waves in a magnetized plasma made up of warm ions and relativistic electrons. Due to their lighter mass and higher speed, the relativistic electrons are more susceptible to magnetic and relativistic effects, and thus their behavior only varies in the direction of z-axis, i.e., along \(B_{0} \hat{z}\). As electron or ion velocities \(u_{e,i}\) approach the speed of light \(c\), relativistic effects become apparent and modify the nonlinear behavior of plasmas. The dynamics of the magnetized plasma in the (x, z) plane can be described by the following equations, with densities normalized by the equilibrium density \(n_{0}\), distances by the gyroradius \(\rho_{s}\), potential by \(\frac{{T_{e} }}{e}\), speeds by the IA velocity \(c_{s}\), and time by the ion gyroperiod \(\Omega_{i}^{ - 1}\):

$$ \frac{{\partial n_{i} }}{\partial t} + \frac{\partial }{\partial x}\left( {n_{i} u_{ix} } \right) + \frac{\partial }{\partial z}\left( {n_{i} u_{iz} } \right) = 0, $$
(1)
$$ \left( {\frac{\partial }{\partial t} + u_{ix} \frac{\partial }{\partial x} + u_{iz} \frac{\partial }{\partial z}} \right)u_{ix} = - \frac{\partial \varphi }{{\partial x}} + u_{iy} - \frac{\alpha }{{n_{i} }}\frac{{\partial n_{i} }}{\partial x}, $$
(2)
$$ \left( {\frac{\partial }{\partial t} + u_{ix} \frac{\partial }{\partial x} + u_{iz} \frac{\partial }{\partial z}} \right)u_{iy} = - u_{ix} , $$
(3)
$$ \left( {\frac{\partial }{\partial t} + u_{ix} \frac{\partial }{\partial x} + u_{iz} \frac{\partial }{\partial z}} \right)u_{iz} = - \frac{\partial \varphi }{{\partial z}} - \frac{\alpha }{{n_{i} }}\frac{{\partial n_{i} }}{\partial z}, $$
(4)

for the ions and

$$ \frac{{\partial n_{e} }}{\partial t} + \frac{\partial }{\partial z}\left( {n_{e} u_{ez} } \right) = 0, $$
(5)
$$ \left( {\frac{\partial }{\partial t} + u_{ez} \frac{\partial }{\partial z}} \right)\gamma_{ez} u_{ez} = \frac{1}{Q}\left( {\frac{\partial \varphi }{{\partial z}} - \frac{1}{{n_{e} }}\frac{{\partial n_{e} }}{\partial z}} \right), $$
(6)

for the electrons, where \(\gamma_{ez} \, = \left\{ {1 - \left( {\frac{{u_{ez} }}{c}} \right)^{2} } \right\}^{{\frac{ - 1}{2}}} \, = \,1 + \frac{{u_{ez}^{2} }}{{2c^{2} }}\) and \(c\) is the speed of light and \(Q\,\left( { = m_{e} /m_{i} } \right)\) is the electron to ion mass ratio, \(\alpha \, = \,\frac{{T_{i} }}{{T_{e} }}\) and \(\rho_{s} \, = \,c_{s} \Omega_{i}^{ - 1}\). \(i\) and \(e\) correspond to ions and electrons, respectively.

3 Derivation of energy integral

We presume that the wave moves at an angle to the external magnetic field \(B_{0} \hat{z}\), depending on a certain quantity

$$ \xi = k_{x} x + k_{z} z - Mt,k_{x}^{2} + k_{z}^{2} = 1. $$
(7)
$$ M = \frac{{{\text{wave}}\,\;{\text{velocity}}}}{{{\text{acoustic}}\;\,{\text{velocity}}}}. $$

Making use of the new coordinate \(\xi\), we get from (7)

$$ \frac{\partial }{\partial x} = k_{x} \frac{\partial }{\partial \xi },\frac{\partial }{\partial z} = k_{z} \frac{\partial }{\partial \xi },\frac{\partial }{\partial t} = - M\frac{\partial }{\partial \xi }. $$

Upon the introduction of the supplementary variable \(\xi\), as delineated in (7), and making use of the boundary conditions \(u_{ix} = u_{iz} = 0\) at \(n_{i} = 1\) as \(\left| \xi \right| \to \infty\), after integration, Eq. (1) transforms into:

$$ k_{x} u_{ix} + k_{z} u_{iz} = M\left( {1 - \frac{1}{{n_{i} }}} \right). $$
(8)

Using (7) and (8), we can simplify Eqs. (2)–(4) as follows:

$$ M\frac{{\partial u_{ix} }}{\partial \xi } = k_{x} n_{i} \frac{\partial \phi }{{\partial \xi }} - n_{i} u_{iy} + \alpha k_{x} \frac{{\partial n_{i} }}{\partial \xi }, $$
(9)
$$ M\frac{{\partial u_{iy} }}{\partial \xi } = n_{i} u_{ix} , $$
(10)
$$ M\frac{{\partial u_{iz} }}{\partial \xi } = k_{z} n_{i} \frac{\partial \phi }{{\partial \xi }} + \alpha k_{z} \frac{{\partial n_{i} }}{\partial \xi }. $$
(11)

Using (7) in (5) and then integrating, we obtain

$$ u_{ez} = \frac{M}{{k_{z} }}\left( {1 - \frac{1}{{n_{e} }}} \right). $$
(12)

When deriving Eq. (12), we applied the boundary conditions \(u_{ez} = 0\) and \(n_{e} = 1\) as \(\left| \xi \right| \to \infty\).

Using the coordinate \(\xi\) and Eq. (12), integrating Eq. (6) once to give the boundary conditions \(\varphi = 0\) at \(n_{e} = 1\) as \(\left| \xi \right| \to \infty :\)

$$ n_{e} = e^{\varphi } \exp \left[ {\frac{{QM^{2} }}{{2k_{z}^{2} }}\left( {1 - \frac{1}{{n_{e}^{2} }}} \right) + \frac{{QM^{4} }}{{8c^{2} k_{z}^{4} }}\left( {1 - \frac{1}{{n_{e} }}} \right)^{3} \left( {1 + \frac{3}{{n_{e} }}} \right)} \right]. $$
(13)

By leveraging (13) alongside the charge neutrality condition \(n_{e} = n_{i} = n\), Eq. (11) can be integrated to obtain

$$ u_{iz} = \frac{{k_{z} }}{M}\left( {n - 1} \right)\left\{ {1 - \frac{{QM^{2} }}{{k_{z}^{2} }}.\frac{1}{n} - \frac{{QM^{4} }}{{2c^{2} k_{z}^{4} }}.\frac{{\left( {n - 1} \right)^{2} }}{{n^{3} }} + \alpha } \right\}. $$
(14)

Using (14), we can derive from (8)

$$ u_{ix} = \frac{M}{{k_{x} }}\left( {n - 1} \right)\left[ {\frac{1}{n} - \frac{{k_{z}^{2} }}{{M^{2} }}\left\{ {1 + \alpha - \frac{{QM^{2} }}{{k_{z}^{2} }}.\frac{1}{n} - \frac{{QM^{4} }}{{2c^{2} k_{z}^{4} }}.\frac{{\left( {n - 1} \right)^{2} }}{{n^{3} }}} \right\}} \right]. $$
(15)

Upon substituting the value of \(u_{ix}\) from (15) into (9), we can then determine \(u_{iy}\) as

$$ u_{iy} = f\left( n \right)\frac{1}{n}\frac{{{\text{d}}n}}{{{\text{d}}\xi }}, $$
(16)

where \(f\left( n \right) = C_{1} + \frac{{C_{2} }}{{n^{2} }} + \frac{{C_{3} }}{{n^{3} }} + \frac{{C_{4} }}{{n^{4} }}\) with

$$ C_{1} = \left( {k_{x} + \frac{{k_{z}^{2} }}{{k_{x} }}} \right)\left( {1 + \alpha } \right), $$
$$ C_{2} = - \left\{ {\frac{{M^{2} Qk_{x} }}{{k_{z}^{2} }} + \frac{{3M^{4} Qk_{x} }}{{2c^{2} k_{z}^{4} }} + \frac{{M^{2} \left( {1 + Q} \right)}}{{k_{x} }} + \frac{{3QM^{4} }}{{2c^{2} k_{z}^{2} k_{x} }}} \right\}, $$
$$ C_{3} = \frac{{3M^{4} Qk_{x} }}{{c^{2} k_{z}^{4} }} + \frac{{3QM^{4} }}{{c^{2} k_{z}^{2} k_{x} }}, $$
$$ C_{4} = - \left\{ {\frac{{3M^{4} Qk_{x} }}{{2c^{2} k_{z}^{4} }} + \frac{{3QM^{4} }}{{2c^{2} k_{z}^{2} k_{x} }}} \right\}. $$

To obtain (16), we need to employ Eq. (13). From (10), the subsequent expression can be derived by utilizing the values of \(u_{ix}\) and \(u_{iy}\) from (15) and (16), respectively.

$$ \frac{{\text{d}}}{{{\text{d}}\xi }}\left\{ {f\left( n \right)\frac{1}{n}\frac{{{\text{d}}n}}{{{\text{d}}\xi }}} \right\} = \frac{1}{{k_{x} }}\left( {n - 1} \right)\left\{ {1 + Q - \frac{{k_{z}^{2} }}{{M^{2} }}\left( {1 + \alpha } \right).\,n + \frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}.\,\left( {1 - \frac{1}{n}} \right)^{2} } \right\}. $$
(17)

By multiplying both sides of Eq. (17) with the term enclosed in the parenthesis on the left-hand side, we can perform a single integration to obtain

$$ \frac{1}{2}\left( {\frac{dn}{{d\xi }}} \right)^{2} + \psi \left( {n,M,k_{z} ,\alpha } \right) = 0. $$
(18)

Equation (18) is derived using the boundary condition \(\frac{{{\text{d}}n}}{{{\text{d}}\xi }} = 0\), \(n = 1\,\) at \(\xi = \pm \infty\). This Eq. (18) represents the energy integral of an oscillating particle with a unit mass with velocity \(\frac{{{\text{d}}n}}{{{\text{d}}\xi }}\), and position \(n\) in a potential well \(\psi \left( n \right)\). It describes the motion of a particle in a pseudopotential well \(\psi \left( n \right)\), where the pseudoposition is denoted by \(n\), pseudotime by \(\xi\), and pseudovelocity by \(\frac{{{\text{d}}n}}{{{\text{d}}\xi }}\). Because of this, Sagdeev potential is known as a pseudopotential. It can be represented by

$$ \psi \left( {n,M,k_{z} ,\alpha } \right) = X\left( n \right)\,Y\left( n \right), $$
(19)

with

$$ X\left( n \right) = \frac{{n^{2} }}{{\left\{ {f\left( n \right)} \right\}^{2} }}, $$
(20)

and

$$ \begin{aligned} Y\left( n \right) & = - \frac{1}{{k_{x} }}\left[ {\left\{ {\left( {1 + Q + \frac{{k_{z}^{2} }}{{M^{2} }}\left( {1 + \alpha } \right) + \frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)C_{1} } \right\}\left( {n - 1} \right) - \left( {\frac{{C_{1} k_{z}^{2} }}{{2M^{2} }}} \right)} \right.\left( {1 + \alpha } \right)\left( {n^{2} - 1} \right) \\ & - \left\{ {\frac{{C_{2} k_{z}^{2} }}{{M^{2} }}\left( {1 + \alpha } \right) + \left( {1 + Q + \frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)C_{1} } \right\}\log n \\ & - \left\{ {\left( {1 + Q + \frac{{k_{z}^{2} }}{{M^{2} }}\left( {1 + \alpha } \right) + \frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)C_{2} + \left( {\frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)C_{1} - \frac{{C_{3} k_{z}^{2} }}{{M^{2} }}\left( {1 + \alpha } \right)} \right\}\left( {\frac{1}{n} - 1} \right) \\ & - \frac{1}{2}\left\{ {\left( {1 + Q + \frac{{k_{z}^{2} }}{{M^{2} }}\left( {1 + \alpha } \right) + \frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)C_{3} - \left( {1 + Q + \frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)C_{2} - \left( {\frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)C_{1} - \frac{{C_{4} k_{z}^{2} }}{{M^{2} }}\left( {1 + \alpha } \right)} \right\}\left( {\frac{1}{{n^{2} }} - 1} \right) \\ & - \frac{1}{3}\left\{ {\left( {1 + Q + \frac{{k_{z}^{2} }}{{M^{2} }}\left( {1 + \alpha } \right) + \frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)C_{4} - \left( {1 + Q + \frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)C_{3} + \left( {\frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)C_{2} } \right\}\left( {\frac{1}{{n^{3} }} - 1} \right) \\ & + \frac{1}{4}\left\{ {\left( {1 + Q + \frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)C_{4} - \left( {\frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)C_{3} + \left( {\frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)C_{2} } \right\}\left( {\frac{1}{{n^{4} }} - 1} \right) \\ & - \frac{1}{5}\left\{ {\left( {\frac{{3QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)C_{4} - \left( {\frac{{QM^{2} }}{{2c^{2} k_{z}^{2} }}} \right)C_{3} } \right\}\left( {\frac{1}{{n^{5} }} - 1} \right) + \left. {\left( {\frac{{C_{4} QM^{2} }}{{12c^{2} k_{z}^{2} }}} \right)\left( {\frac{1}{{n^{6} }} - 1} \right)} \right]. \\ \end{aligned} $$
(21)

To identify the presence of SWs, we can use the following conditions, denoted by \(\psi \left( {n,M,k_{z} ,\alpha } \right)\) as \(\psi \left( n \right)\):

(i) \(\psi \left( n \right) < 0\), between \(n = 1\) and \(n = N\), where \(N\) represents the wave's amplitude, ensuring that \(\frac{dn}{{d\xi }}\) is real [see Eq. (18)]. The value of \(N\) can be either greater than or less than 1, which determines whether the SW is compressive or rarefactive, respectively.

(ii) At \(n = 1\), \(\psi \left( n \right)\) must reach a maximum, which implies that \(\left[ {\psi^{\prime}\left( n \right)} \right]_{n = 1} = 0\) and \(\left[ {\psi^{\prime\prime}\left( n \right)} \right]_{n = 1} < 0\).

(iii) Near \(n = N\), \(\psi \left( n \right)\) should cross the \(^{\prime}n^{\prime}\) axis from below, and for \(n > N\), \(\psi \left( n \right)\) should be greater than \(0\).

4 Discussion

The nonlinear wave forms of large-amplitude IASWs are investigated in a magnetized warm plasmas with relativistic electrons and non-relativistic ions. Shukla and Yu [40] have demonstrated the existence of compressive \(\left( {N > 1} \right)\) solitons in the absence of electron inertia, considering ion polarization drift and neglecting all nonlinear terms in plasma dynamics. On the contrary, Ivanov [41] has shown the existence of rarefactive \(\left( {N < 1} \right)\) solitons for \(M > k_{z}\), considering full ion nonlinearity. Moreover, Lee and Kan [42] successfully demonstrated compressive solitons \(k_{z} < M < 1\), but they were unable to showcase rarefactive solitons without taking electron inertia into account. Kalita et al. [43] revealed the existence of subsonic \(\left( {M < 1} \right)\) IASWs in a magnetized plasma, where both density hump (compressive) \(\left( {N > 1} \right)\) and density dip (rarefactive) \(\left( {N < 1} \right)\) solitons can coexist in the presence of electrons and relativistic ions. Interestingly, our investigation found compressive \(\left( {N > 1} \right)\) and rarefactive \(\left( {N < 1} \right)\) solitons with intriguing characteristics, including the growth of supersonic and subsonic solitons arising from the relativistic effects of electrons and temperature ratio \(\alpha \left( { = \frac{{T_{i} }}{{T_{e} }}} \right)\).

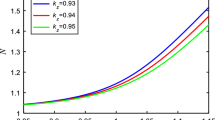
The figures in the study depict high-amplitude, weakly relativistic, compressive solitons with subsonic speed \(M\, = \,0.90\,(s{\text{olid}}\,\;{\text{line}}),\,0.92\,(d{\text{ashed}}\,\;{\text{line}}),\,0.94\,(d{\text{ash}} - {\text{dotted}}\,{\text{line}}),\), which exhibit a concave decrease with \(k_{z}\) at fixed values of \(\alpha \, = \,0.10\) (Fig. 1). The behavior of solitons with high amplitudes \(\left( { > 1} \right)\) differs for small \(k_{z}\) compared to higher \(k_{z}\). The diminishing amplitude of the compressive subsonic soliton as it moves away from the magnetic field can be explained by the interaction between plasma density and magnetic field strength. As the soliton travels further from the magnetic field, the weakening magnetic field diminishes the plasma's capacity to maintain the soliton, resulting in a decrease in amplitude. This effect arises from the interplay between plasma dynamics and magnetic field confinement. In contrast, the amplitudes of slightly supersonic solitons shift from compressive to rarefactive. Nonetheless, as we move away from the magnetic field, the rarefactive solitons demonstrate a decreasing pattern (Fig. 2) in the critical direction of propagation for all \(M\, = \,1.04\,(s{\text{olid}}\,\;{\text{line}}),\,1.06(d{\text{ashed}}\,\;{\text{line}}),\,1.08\,(d{\text{ash}} - {\text{dotted}}\;\,{\text{line}})\) and \(\alpha \, = \,0.10\). As the temperature ratio \(\alpha = \,0.10\,(s{\text{olid}}\,\;{\text{line}}),\,0.15(d{\text{ashed}}\,\;{\text{line}}),\,0.20\,(d{\text{ash}} - {\text{dotted}}\,\;{\text{line}})\) gradually increases and \(k_{z}\) remains fixed, the amplitudes of subsonic and supersonic solitons decrease (as shown in Fig. 3). Furthermore, Fig. 3 demonstrates that the amplitudes exhibit nearly negligible variations as the Mach numbers increase. Figure 4 illustrates that the amplitudes of relativistic, subsonic compressive solitons remain consistently linear as the ion temperature ratio \(\alpha\) increases for all values of \(k_{z} \, = \,0.55\,(s{\text{olid}}\;\,{\text{line}}),\,0.60(d{\text{ashed}}\,\;{\text{line}}),\,0.65\,(d{\text{ash}} - {\text{dotted}}\,\;{\text{line}})\) and \(M\, = \,0.80\). We know that as plasma temperature increases, density typically decreases. Consequently, at higher temperatures, the existence of relativistic compressive, subsonic solitons may be compromised. But, in supersonic (\(M\, = \,1.05\)) solitons, amplitudes transition from compressive to rarefactive (Fig. 5) for the same values of \(k_{z}\) as shown in Fig. 4. Additionally, as the values of \(k_{z}\) increase, the amplitudes decrease for both subsonic and supersonic situations (Figs. 4 and 5).

Fig. 1
figure 1

Variation of compressive subsonic soliton amplitude vs. \(k_{z}\) for all \(M\, = \,0.90\,({\text{solid}}\;\,{\text{line}}),\,0.92\,({\text{dashed}}\;\,{\text{line}}),\,0.94\,({\text{dash}} - {\text{dotted}}\,\;{\text{line}})\) and \(\alpha \, = \,0.10\)

Full size image
Fig. 2
figure 2

Variation of rarefactive supersonic soliton amplitude vs. \(k_{z}\) for all \(M\, = \,1.04\,({\text{solid}}\;\,{\text{line}}),\,1.06({\text{dashed}}\;\,{\text{line}}),\,1.08\,({\text{dash}} - {\text{dotted}}\,\;{\text{line}})\) and \(\alpha \, = \,0.10\)

Full size image
Fig. 3
figure 3

Variation of rarefactive subsonic and supersonic soliton amplitude vs. \(M\) for \(\alpha = \,0.10\,({\text{solid}}\,\;{\text{line}}),\,0.15({\text{dashed}}\,\;{\text{line}}),\,0.20({\text{dash}} - {\text{dotted}}\,\;{\text{line}})\) and \(k_{z} = \,0.7\)

Full size image
Fig. 4
figure 4

Variation of compressive subsonic soliton amplitude vs. \(\alpha\) for all \(k_{z} \, = \,0.55\,({\text{solid}}\,\;{\text{line}}),\,0.60({\text{dashed}}\;\,{\text{line}}),\,0.65\,({\text{dash}} - {\text{dotted}}\;\,{\text{line}})\) and for fixed \(M\, = \,0.80\)

Full size image
Fig. 5
figure 5

Variation of rarefactive supersonic soliton amplitude vs. \(\alpha\) for all \(k_{z} \, = \,0.55\,({\text{solid}}\,\;{\text{line}}),\,0.60 \,({\text{dashed}}\;\,{\text{line}}),\,0.65\,({\text{dash}} - {\text{dotted}}\,\;{\text{line}})\) and for fixed \(M\, = \,1.05\)

Full size image

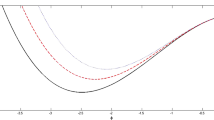
The energy integral (18) displays reflected potential wells characterized by \(\psi \left( n \right)\) in subsonic (\(M\, = \,0.2\)) and supersonic (\(M\, = \,1.01\)) circumstances, exhibiting the soliton amplitudes clearly in Figs. 6 and 7 for \(\alpha \, = \,0.01\) and for all three cases where the SW moves with propagation directions \(k_{z} \, = \,0.17\,({\text{solid}}\;\,{\text{line}}),\,0.175 \, ({\text{dashed}}\,\;{\text{line}}),\,0.18 \, ({\text{dash}} - {\text{dotted}}\,\;{\text{line}})\) and \(k_{z} \, = \,0.78\) \(\,({\text{solid}}\,{\text{line}}),\,0.79 \, ({\text{dashed}}\;\,{\text{line}}),\,0.80 \, ({\text{dash}} - {\text{dotted}}\,\;{\text{line}})\), respectively. Notably, we have observed that as \(k_{z}\) increases, the amplitude and depth of the potential profile decrease for both subsonic and supersonic conditions. The speed of plasma particles significantly affects the formation and characteristics of solitons. In subsonic conditions (Fig. 6), where plasma particles have a comparatively slower velocity, they are confined within a shallower potential well. This shallow potential results in the formation of smaller-amplitude compressive solitons. Conversely, in supersonic conditions (Fig. 7), where plasma particles move at higher velocities, they are trapped in a deeper potential well, leading to the formation of higher-amplitude compressive solitons. However, in the subsonic situation, our observation indicates that if \(k_{z} > 0.21401\), SWs fail to exist, leading to the non-existence of soliton solutions. Consequently, the amplitude of the nonlinear wave patterns is influenced by the external magnetic field \(k_{z}\). However, in Fig. 8, an opposite trend is observed: as the Mach number \(M\) increases, the amplitudes and depth of the potential profile also increase for the fixed values of \(\alpha \, = \,.01\) and \(k_{z} = \,0.79\). In Fig. 9, the decrease in amplitude as the ion-to-electron temperature ratio \(\alpha\) rises, given a constant Mach number (\(M\, = \,0.2\)) and direction cosine (\(k_{z} = \,0.15\)), is fundamentally linked to the behavior of plasma waves. With an increasing temperature ratio, ions play a more significant role in determining plasma dynamics. This increased influence results in a lower amplitude of plasma waves because the heavier ions dampen the wave motion more effectively than the lighter and more mobile electrons. Consequently, as the temperature ratio \(\alpha\) grows, the amplitude of plasma waves diminishes due to the enhanced damping effect of the ions.

Fig. 6
figure 6

Reflection of potential well \(\psi\) for different propagation directions \(k_{z} \, = \,0.17\,({\text{solid}}\,\;{\text{line}}),\,0.175 \, ({\text{dashed}}\,\;{\text{line}}),\,0.18 \, ({\text{dash}} - {\text{dotted}}\;\,{\text{line}})\) when \(M\, = \,0.2\), and \(\alpha \, = \,0.01\)

Full size image
Fig. 7
figure 7

Reflection of potential well \(\psi\) for different propagation directions \(k_{z} \, = \,0.78\,({\text{solid}}\,\;{\text{line}}),\,0.79 \, ({\text{dashed}}\,\;{\text{line}}),\,0.80 \, ({\text{dash}} - {\text{dotted}}\;\,{\text{line}})\) when \(M\, = \,1.01\), and \(\alpha \, = \,0.01\)

Full size image
Fig. 8
figure 8

Reflection of potential well \(\psi\) for different propagation directions \(M = \,0.87\,({\text{solid}}\,\;{\text{line}}),\,0.875 \, ({\text{dashed}}\,\;{\text{line}}),\,0.88\,({\text{dash}} - {\text{dotted}}\;\,{\text{line}})\) when \(\alpha \, = \,.01\), and \(k_{z} = \,0.79\)

Full size image
Fig. 9
figure 9

Reflection of potential well \(\psi\) for different propagation directions \(\alpha = \,0.01\,({\text{solid}}\,\;{\text{line}}),\,0.02 \, ({\text{dashed}}\,\;{\text{line}}),\,0.03\,({\text{dash}} - {\text{dotted}}\,\;{\text{line}})\) when \(M\, = \,0.2\), and \(k_{z} = \,0.15\)

Full size image

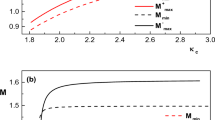
As shown in Fig. 10, the width (\(\Delta = \,N/\sqrt d , \, \,d\) represents its depth for each given set of assigned values of \(M\),\(\alpha\) and \(k_{z}\) to determine \(N\)) of compressive relativistic solitons grows as \(k_{z}\) goes up when the soliton moves in a direction that is close to the direction of the magnetic field for all values of \(M\, = \,1.04\,(\text{solid}\, \text{line}),\,1.06 \, (\text{dashed}\, \text{line}),\,1.08 \, (\text{dash} - \text{dotted}\, \text{line})\) and \(\alpha \, = \,0.10\). We use a reference value of \(Q = 0.00054\) for the lightest ion and maintain \(c = 300\) in all numerical calculations.

Fig. 10
figure 10

Variation of soliton width \(\left( \Delta \right)\) vs. \(k_{z}\) for different Mach numbers \(M\, = \,1.04\,({\text{solid}}\;\,{\text{line}}),\,1.06 \, ({\text{dashed}}\;\,{\text{line}}),\,1.08 \, ({\text{dash}} - {\text{dotted}}\;\,{\text{line}})\) and for fixed \(\alpha \, = \,0.10\)

Full size image

Studying the behavior of large-amplitude IASWs within a warm magnetoplasma characterized by the presence of positive ions and relativistic electrons represents an exciting frontier in the field of plasma physics. Positive ions and relativistic electrons interact in a complicated way, especially when magnetic fields are present. This gives rise to wave structures and dynamics that are different from those seen in plasmas with less complicated arrangements. This study is also very important for astrophysics because many natural places in the universe, like pulsar magnetospheres and active galactic nuclei, have magnetoplasmas with fast electrons and positive ions. The derivation of the Sagdeev potential within this particular plasma system carries significant physical and research significance. This potential plays a pivotal role in understanding critical aspects such as wave stability, energy conservation, and the emergence of nonlinear wave phenomena. Importantly, its applicability extends to both astrophysical and laboratory plasma systems. As a result, it stands as an indispensable tool in advancing our understanding of plasma physics and its diverse applications.

5 Summary

In this comprehensive investigation of large-amplitude IASWs within a plasma consisting of warm positive ions and relativistic electrons, we employ the SP approach to gain deep analytic insights. Our findings indicate the presence of both compressive and rarefactive solitary waves, which manifest in subsonic and supersonic forms under distinct parametric conditions. These solitary waves arise from a complex interplay between nonlinear effects, dispersion, and the specific parameters of the plasma environment. Significantly, the plasma configuration, particularly the ion temperature and the relativistic nature of the electrons, plays a critical role in shaping the formation and dynamics of SWs. Moreover, our research highlights a crucial correlation between the direction cosine \(k_{z}\) of the magnetic field and the amplitude of these waves: as the direction cosine \(k_{z}\) increases, indicating greater alignment with the magnetic field lines, the amplitude of the SWs decreases. This relationship offers valuable insights into how the orientation of the magnetic field influences the behavior of IASWs, enhancing our understanding of their dynamics and potential applications in fields such as controlled nuclear fusion and space physics.