Energy dependence of the electoproduction of vector mesons

Abstract

The energy dependence of the electroproduction of vector mesons is analyzed. Two pomeron model is used assuming that the soft and the hard pomerons are two Regge poles. In the analysis, a Regge amplitude for the photoproduction processes is extended to include the electroproduction processes. In this case the residues of the two poles are assumed to be functions of the photon virtuality. These functions are extracted by fitting the experimental data using the chi- square method. The variation of cross section with energy for different photon virtuality can be obtained by including these functions in the amplitude of the photoproduction process.

1 Introduction

Diffractive electroproduction of vector mesons can be represented by the reaction $\gamma^{*} p \to vp$, where $v = \rho ,w,\varphi ,J/\psi , \ldots$. The hard scattering can be achieved if one or more of the variables $Q^{2} ,t\;{\text{and}}\;m_{v}$ is high, with $Q^{2}$ is the mass of the virtual photon, t is the transferred momentum and $m_{v}$ is the mass of the vector meson. At low values of these variables the process is soft and can be described by the exchange of a soft pomeron [1]. The small t values are considered here.

Experimentally, the $Q^{2}$ dependence is giving by the form [2, 3]

$$ \frac{1}{{(Q^{2} + m_{v}^{2} )^{n} }} $$

(1)

with $n\sim2.3$. In the hard region, the Quantum Chromodynamic calculation can be applied [4–7]. Most of the qualitative properties of the data can be reproduced by such calculations. However, the calculations suffer from uncertainties in the gluon distribution and the vector meson wave function which introduces uncertainty in the overall normalization. Different models have described the $Q^{2}$ dependence using different approaches. Some [8, 9] of these models assume that the coupling of the photon-vector meson vertex to the exchanged pomeron is responsible for this dependence. Some other models use the $Q^{2}$ in the parameters of pomeron trajectory [9, 10]. However, apart from the pQCD models, the variation of the cross section with the energy $w$ for different values of $Q^{2}$ has not been discussed. In this paper, a two pomeron model is used to analyze this variation. Experimentally, this variation is parameterized as $w^{\delta }$. For photoproduction [11–13], there is a pattern of variation of the exponent $\delta$ from light to heavy vector mesons. For $\rho$ and $\varphi \delta \cong 0.22$ the measurement of the $J/\psi$ photoproduction shows a much steeper rise with energy than the light vector mesons with $\delta$ in the range 0.7–0.8. It is also found that $\delta$ for $\varUpsilon$ is about 1.6. The cross section has a smooth dependence on $w$ for small $\delta$ and a steep dependence for higher values of $\delta$. This phenomenon is explained as presence of two pomerons, the soft and the hard pomeron. In the photoproduction the transition from the soft region to the hard region is due to the mass scale. The transition can also be obtained by varying the $Q^{2}$ for electroproduction of $\rho$ and $\varphi$ mesons [14, 15]. The $w^{\delta }$ parameterization for $\sigma_{{\gamma^{*} p \to \rho p}} \left( w \right)$ and $\sigma_{{\gamma^{*} p \to \varphi p}} \left( w \right)$ cross sections for different values of $Q^{2}$ indicates that $\delta$ changes from 0.22 at small $Q^{2}$ as in the photoproduction and increase with increasing $Q^{2}$ up to 0.8–1.1 for highest values of $Q^{2}$. For $J/\psi$ electroproduction [16], $\delta$ is almost constant with $Q^{2}$ and is consistent with that observed for $J/\psi$ photoproduction. This region may be denoted by the constant region. However, QCD models suggest that the pomeron is either a branch point or a sequence of Regge poles but not a single pole. Consequently, the exponent $\delta$ can depend on the hard scale $Q_{G}^{2} = Q^{2} + m_{v}^{2}$. It is found that at equal values of the hard scale, the $\rho$ production is softer than the $J/\psi$ production and the $\delta_{J/\psi }$ is slightly larger than the $\delta_{\rho }$. The $k_{T}$ factorization model [17] suggests that the vacuum exchange can be approximated by soft and hard poles with $Q^{2}$ independent intercepts. Consequently, the exponent $\delta$ is a $Q^{2}$ independent as it is related to these intercepts. In this paper each pomeron is regarded as a single pole with a $Q^{2}$ independent intercept but the residue is a $Q^{2}$ dependent.

2 The model

In the region of small $Q^{2}$ the amplitude for production of vector meson by a transversely polarized virtual photon is expressed in terms of the respective real photon as follows [18]:

$$ A_{{\gamma^{*} p \to Vp}}^{T} = \frac{{m_{v}^{2} }}{{Q^{2} + m_{v}^{2} }}A_{\gamma p \to Vp} \;\left( w \right) $$

(2)

The amplitude $A_{\gamma p \to Vp}$($w)$ can be calculated using the vector meson dominance model (VMD) and Regge theory. The energy dependence of the cross section is similar to that of hadronic cross section and is dominated by a soft pomeron exchange. For high $Q^{2}$ region, the vertex of coupling of the exchanged trajectory to the virtual photon- vector meson vertex becomes $Q^{2}$ dependent. The amplitude in Eq. (2) becomes

$$ A_{{\gamma^{*} p \to Vp}}^{T} = \frac{{m_{v}^{2} }}{{Q^{2} + m_{v}^{2} }} f\left( { Q^{2} } \right)A_{{\gamma^{*} p \to Vp}} \;\left( w \right) $$

(3)

The vertex $f\left( { Q^{2} } \right)$ has been calculated using the quark loop triangle with [19]

$$ f\left( {Q^{2} ,0,m_{v}^{2} } \right) = \frac{{m_{v}^{2 } }}{{Q^{2} + m_{v}^{2} }} \left( {\pi^{2} - ln^{2} \left( {\frac{{1 + \beta_{2} }}{{1 - \beta_{2} }}} \right) + ln^{2} \left( {\frac{{\beta_{1} + 1}}{{\beta_{1} - 1}}} \right)} \right) $$

(4)

All the parameters are defined in Ref [19]. The energy dependence in Eq. (3) is different from that in Eq. (2), since the process is now a hard process which is dominated by a hard pomeron exchange.

The main task is to find the amplitude $A_{{\gamma^{*} p \to Vp}}$ $\left( w \right)$. The start point is to define the photo production amplitude $A_{\gamma p \to Vp}$ $\left( w \right)$. Different models indicate that a better fit for the experimental data is obtained if a hard pomeron term is added to the soft pomeron in the amplitude of the process $\gamma p \to Vp$. This hard pole is attributed to the non hadronic phase of the photon. Thus according to Regge theory, the amplitude of this process is given as [20, 21]:

$$ A\left( {s,t} \right) = \mathop \sum \limits_{i} X_{i} F\left( t \right)G\left( t \right)\left( {\frac{s}{{s_{i} }}} \right)^{{\alpha_{i} \left( t \right) - 1}} e^{{ - \frac{1}{2}\pi \alpha_{i} \left( t \right)}} $$

(5)

The summation is over the exchanged reggeons, soft and hard pomerons. The parameters $X_{i}$ are the coupling of the exchanged trajectories to the interacting particles (residues). These parameters are fixed by the experimental data. The used trajectories ($\alpha_{i} \left( t \right)$) are the reggeons, soft pomeron and hard pomeron. These are respectively as

$$ \begin{aligned} \alpha_{2} \left( t \right) = & \;0.55 + \alpha_{2}^{'} t,\quad \alpha_{2}^{'} = 0.93\;{\text{GeV}}^{ - 2} \\ \alpha_{1} \left( t \right) = & \;1.08 + \alpha_{1}^{'} t,\quad \alpha_{1}^{'} = 0.25\;{\text{GeV}}^{ - 2} \\ \alpha_{0} \left( t \right) = & \;1.44 + \alpha_{0}^{'} t,\quad \alpha_{0}^{'} = 0.1\;{\text{GeV}}^{ - 2} \\ \end{aligned} $$

(6)

The form factor $F\left( t \right)$ is the Dirac form factor for the proton and given as

$$ F\left( t \right) = \frac{{4m^{2} - 2.79t}}{{4m^{2} - t}}\left( {\frac{1}{{1 - {\raise0.7ex\hbox{$t$} \!\mathord{\left/ {\vphantom {t {m_{1} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${m_{1} }$}}}}} \right)^{2} $$

(7)

with m is the proton mass. The function $G\left( t \right)$ is the $\gamma \to v$ transition form factor and given as:

$$\begin{aligned} G_{\rho } \left( t \right) = \frac{1}{{1 - {\raise0.7ex\hbox{$t$} \!\mathord{\left/ {\vphantom {t {m_{2} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${m_{2} }$}}}}\\G_{\varphi } \left( t \right) = \frac{1}{{1 - {\raise0.7ex\hbox{$t$} \!\mathord{\left/ {\vphantom {t {m_{3} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${m_{3} }$}}}} \end{aligned}$$

(8)

where $m_{1} = 0.71 \,{\text{GeV}}^{2}$,$m_{2} = 0.71\, {\text{GeV}}^{2}$ and $m_{3} = 1.5\, {\text{GeV}}^{2}$. For $J/\psi$ the form factor is rather flat in t and is used as a constant. The normalization factor $X_{i}$ are obtained by fitting the experimental data. These for the Rho meson are: $X_{2} = 15.9$, $X_{1} = 6.0$, $X_{0} = 0.036$. For the phi mesons and $J/\psi$ mesons the Zweig’s rules are used, then for phi mesons we have: $X_{1} = 1.49$, $X_{0} = 0.014$ while for $J/\psi$ are $X_{1} = 0.17$, $X_{0} = 0.016$. The factors $s_{i}$ are related to slope trajectories with $s_{i} = {\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 {\alpha_{i}^{'} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${\alpha_{i}^{'} }$}}$. The amplitude is normalized such that $\frac{d\sigma }{dt} = \left| {A\left( {s,t} \right)} \right|^{2}$ in $\mu b\; {\text{GeV}}^{ - 2}$. The integration is performed numerically on t from t = 0 to t = 1 GeV².

For the virtual photon interaction $\gamma^{*} p \to vp$ amplitude, the two pomeron model is also adopted with $Q^{2}$-dependent residues as which are fixed by fitting the experimental data. To compare with the data of the electorproduction, let us write Eq. (3) in the form

$$ A_{{\gamma^{*} p \to Vp}}^{T} = \frac{{m_{v}^{2} }}{{Q^{2} + m_{v}^{2} }} f\left( { Q^{2} } \right)A_{\gamma p \to Vp} \;\left( w \right) $$

(9)

where $A_{\gamma p \to Vp}$($w)$ is the amplitude $A\left( {s,t} \right)$ in Eq. (5). It is used in here instead of the virtual photon amplitude in Eq. (3). The cross section of Eq. (9) is given by:

$$ \frac{d\sigma}{dt} = \left({1 + \epsilon\frac{{Q^{2}}}{{m_{v}^{2}}}} \right)\left| {A_{{\gamma^{*} p \to Vp}}^{T}} \right|^{2} $$

(10)

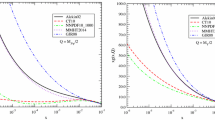
where the contribution from the longitudinal photon has been included in Eq. (10). As usual integrating Eq. (10) numerically, then the variations of the total cross section with energy for fixed $Q^{2}$ is calculated. The results are compared with the data [22, 23]. It is found that Eq. (10) does not match with the data simply because the amplitude $A_{\gamma p \to Vp}$($w)$ in Eq. (9) is the photoproduction amplitude. To match the data, the hard pomeron and the soft pomeron terms in Eq. (10) are multiplied by some factors $c_{0}$ and $c_{1}$ respectively. For simplicity, the coefficient of the reggeon term has been frozen at the same value of the real photon case. These factors are adjusted using the Chi square ($\chi^{2}$) method until good fits for the data are obtained [22, 23]. It is found that all the $\chi^{2}$ values are less than one. The maximum value is 0.68 at $Q^{2} = 11.9$ GeV². These encouraging results assume that the performed fits are reasonable. The obtained fits are also compared with those given by the $w^{\delta }$ method. The resulting $c_{0}$ and $c_{1}$ factors are denoted by the weights. The hard pomeron weights $\left( { c_{0} } \right)$ for $\rho$, $\varphi$ and $J/\psi$ are plotted together in Fig. 1(a) as a function of $Q^{2}$, while those of the soft pomeron weights $\left( {c_{1} } \right)$ are given in Fig. 1(b).

Fig. 1

(a) Hard pomeron weights and (b) soft pomeron weights as functions of $Q^{2}$ for Rho, Phi and $J/\psi$ mesons production

The following equations are used to fit the variations of the pomeron weights with $Q^{2}$ in Fig. 1:

For the Rho mesons

$$ c_{1} = {1 \mathord{\left/ {\vphantom {1 {\left( {1 + \frac{{Q^{2} }}{{60Q_{0}^{2} }}} \right)}}} \right. \kern-0pt} {\left( {1 + \frac{{Q^{2} }}{{60Q_{0}^{2} }}} \right)}} $$

(11)

$$ c_{0} = 1 + \left( {\frac{{Q^{2} }}{{Q_{0}^{2} }}} \right)^{0.43} - 0.013\frac{{Q^{2} }}{{Q_{0}^{2} }} ^{ } $$

(12)

while for the Phi mesons we have

$$ c_{1} = {1 \mathord{\left/ {\vphantom {1 {\left( {1 + \frac{{Q^{2} }}{{11Q_{0}^{2} }} } \right)}}} \right. \kern-0pt} {\left( {1 + \frac{{Q^{2} }}{{11Q_{0}^{2} }} } \right)}} $$

(13)

$$ c_{0} = 1 + 0.67\left( {\frac{{Q^{2} }}{{Q_{0}^{2} }} } \right)^{0.38} - 0.003\;\frac{{Q^{2} }}{{Q_{0}^{2} }} $$

(14)

and for $J/\psi$ mesons

$$ c_{1} = {1 \mathord{\left/ {\vphantom {1 {\left( {1 + \frac{{Q^{2} }}{{4.5Q_{0}^{2} }} } \right)}}} \right. \kern-0pt} {\left( {1 + \frac{{Q^{2} }}{{4.5Q_{0}^{2} }} } \right)}} $$

(15)

$$ c_{0} = 1 + 0.18\left( {\frac{{Q^{2} }}{{Q_{0}^{2} }}} \right)^{0.34} - 0.003\;\frac{{Q^{2} }}{{Q_{0}^{2} }} $$

(16)

Here $Q_{0}^{2} = 1{\text{GeV}}^{2}$. It is clear that the hard pomeron weights in Fig. 1(a) for Rho and Phi mesons are increasing with $Q^{2}$, but the rate of the increase is decreasing with $Q^{2}$. Therefore, the curves may tend to constant regions at high values of $Q^{2}$. This arises from the behavior of the steepness of the cross sections. In the constant region, the steepness of the cross section is almost constant with $Q^{2}$ as in the $J/\psi$ case. For the Rho and the phi mesons, the steepness is increasing with $Q^{2}$ but the rate of increase is decreasing. Eventually, the cross sections should tend to constant regions. It is clear that the Phi mesons reaches the constant region faster than the Rho mesons. As $Q^{2}$ increases the hard pomeron dominates the cross section while the contribution from the soft pomeron decreases. This means that the weights of the hard pomeron increase, while those of the soft pomeron decrease as given in Fig. 1(a) and 1(b). As the Rho mesons is in a softer regime, the weight needed to multiply the hard pomeron coefficient in the photoproduction amplitude in Eq. (10) is higher than that of the Phi mesons at the same $Q^{2}$ while that needed for the soft pomeron is lower. On the other hand, as the $J/\psi$ production is a hard process even in the photoproduction process, the variation with $Q^{2}$ is small and the hard weights vary slightly around one as in Fig. 1(a), (b).

The $w^{\delta }$ distribution shows that $\delta_{J/\psi } > \delta_{\varphi } > \delta_{\rho }$, while the present model shows that $c_{0\rho } > c_{0\varphi } > c_{0J/\psi }$. Using Eqs. (11–16) in Eq. (10) to fit the data for Rho mesons [3], the Phi mesons [24] and the $J/\psi$ [25] as given in Fig. 2. It is clear that the extracted equations can reasonably reproduce the experimental data.

Fig. 2

Vector meson electroproduction cross sections as a function of the energy ($w$) for different values of ($Q^{2}$), for: (a) Rho [3], (b) Phi [24] and (c) $J/\psi$ [25] mesons. The curves represent the predictions from the model in terms of Eqs. (11–16). The data points are H1 data

The variation of the cross sections with $Q^{2}$ at fixed values of energy is shown in Fig. 3 for Rho, Phi [22] and $J/\psi$ [23] mesons. We notice reasonable fits for the data in Fig. 3. So far the H1 data are used to justify the calculation. It is found that the ZUES data can be fit by inserting the following forms of the extracted functions in Eq. (10):

Fig. 3

Vector meson electroproduction cross sections as a function of ${\text{Q}}^{2}$, for: (a) Rho [22], (b) Phi [22] and (c) for $J/\psi$ [25], where $w$ = 75 GeV for Rho and Phi and $w$ = 90 GeV for $J/\psi$. The solid curve is the prediction from the model and the data points are the H1 data

For the Rho mesons:

$$ c_{1} = {1 \mathord{\left/ {\vphantom {1 {\left( {1 + \frac{{Q^{2} }}{{70Q_{0}^{2} }} } \right)}}} \right. \kern-0pt} {\left( {1 + \frac{{Q^{2} }}{{70Q_{0}^{2} }} } \right)}} $$

(17)

$$ c_{0} = 1 + 0.83\left( \frac{{Q^{2} }}{{Q_{0}^{2} }}\right)^{0.31} - 0.014\;\frac{{Q^{2} }}{{Q_{0}^{2} }} $$

(18)

while for the Phi mesons we have

$$ c_{1} = {1 \mathord{\left/ {\vphantom {1 {\left( {1 + \frac{{Q^{2} }}{{25Q_{0}^{2} }} } \right)}}} \right. \kern-0pt} {\left( {1 + \frac{{Q^{2} }}{{25Q_{0}^{2} }} } \right)}} $$

(19)

$$ c_{0} = 1 + 0.8\left( {\frac{{Q^{2} }}{{Q_{0}^{2} }}} \right)^{0.4} - 0.003\;\frac{{Q^{2} }}{{Q_{0}^{2} }} $$

(20)

and for $J/\psi$ mesons

$$ c_{1} = {1 \mathord{\left/ {\vphantom {1 {\left( {1 + \frac{{Q^{2} }}{{4 Q_{0}^{2} }} } \right)}}} \right. \kern-0pt} {\left( {1 + \frac{{Q^{2} }}{{4 Q_{0}^{2} }} } \right)}} $$

(21)

$$ c_{0} = 1 + 0.19\left( {\frac{{Q^{2} }}{{Q_{0}^{2} }}^{{}} } \right)^{0.37} - 0.003\;\frac{{Q^{2} }}{{Q_{0}^{2} }} $$

(22)

3 Results and discussion

Two pomeron model is used to analyze the electroproduction of vector mesons. The soft and the hard pomerons are assumed to be Regge poles. Consequently, their Regge trajectories should be independent of the photon virtuality as Regge theory is dealing with on shell particles. Instead, the coefficients of the poles are assumed to involve the photon virtuality. The coefficients are given by those of the photoproduction process multiplied by the weights. These weights are fixed by fitting the data on the variation of the cross sections with energy for different $Q^{2}$.The hard pomeron weights are increasing with $Q^{2}$, but the rate of increase is decreasing until a constant region is reached at very high values of $Q^{2}$. The hard weights of the Rho mesons are higher than those of the Phi mesons at the same $Q^{2}$, while the $J/\psi$ weights are around one. To normalize the increase in the hard weights the soft weights are decreasing as in given in Fig. 1(b). The Chi square method is used to extract the weights. This method works better for higher numbers of data points. Therefore, higher numbers of data points are needed especially at high values of $Q^{2}$ to reveal the constant regions. However, different formulae are obtained by fitting the weights. A reasonable fit for another set of data is obtained by involving these formulae in the photoproduction Regge amplitude. In this analysis, data from H1 are used. The extracted equations can be used to predict the cross sections at any value of $Q^{2}$. However, the ZEUS data may need slight adjustments in the parameters of the extracted equations.

4 Conclusions

The two pomeron model is extended to analyze the energy dependence of the electorproduction of the vector mesons. As in the photoproduction case the soft and the hard pomerons are treated as Regge poles but their residues are assumed to be functions of the photon virtuality $Q^{2}$. The variation of the residues with $Q^{2}$ is extracted by comparing the model with the data using the Chi square method. These functions are denoted by the weight functions. It is found that more data points are needed to use this method and to get more accurate weight functions. The weights functions become almost constant with $Q^{2}$ at high values of $Q^{2}$ for all types of vector mesons. This is clear from the $J/\psi$ data where the variations of the cross section with energy for different values of $Q^{2}$ including the real case are almost parallel. Therefore, its residues reach the constant region faster than those of the other vector mesons. With this model the distribution of the cross section with $Q^{2}$ for different energies is predicted even at high values of $Q^{2}$ and energies.