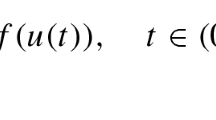A Class of Prescribed Weingarten Curvature Equations for Locally Convex Hypersurfaces with Boundary in $\mathbb {R}^{n+1}$

Abstract

In this paper, we consider a class of prescribed Weingarten curvature equations for strictly locally convex hypersurfaces with boundary in $\mathbb {R}^{n+1}$. Under some sufficient conditions, we obtain an existence result using a two-step continuity process based on the a priori estimates for solutions to prescribed Weingarten curvature equations.

1 Introduction

In this paper, we study the Plateau type problem for strictly locally convex hypersurfaces $\Sigma \subset \mathbb {R}^{n+1}$, which is determined by prescribed Weingarten curvature equations with (k, l)-Hessian quotient of $\lambda [\eta ]$

$$\begin{aligned} \frac{\sigma _k}{\sigma _l}(\lambda [\eta ])=\psi (X, \nu ), \end{aligned}$$

(1.1)

as well as the boundary condition

$$\begin{aligned} \partial \Sigma = \Gamma . \end{aligned}$$

(1.2)

Here $2\le k\le n$, $0\le l\le \min \{n-2, k-1\}$, the (0, 2)-tensor $\eta$ on $\Sigma$ is defined by

$$\begin{aligned} \eta _{ij}= H g_{ij}-h_{ij}, \end{aligned}$$

$g_{ij}$ and $h_{ij}$ are the first and second fundamental forms of $\Sigma$ respectively, H is the mean curvature of $\Sigma$ , $\psi$ $:\mathbb {R}^{n+1}\times \mathbb {S}^n\rightarrow \mathbb {R}$ is a given positive smooth function, X is the position vector of $\Sigma$. $\sigma _k(\lambda [\eta ])$ means the k-th elementary symmetric polynomial $\sigma _k$ applied to the eigenvalues of $g^{-1}\eta$.

Our interest on the solvability of the above problem is motivated by the study of a class of Hessian type equations in complex and real geometry. Harvey-Lawson [26, 28] introduced a class of functions $u\in C^2(\mathbb {C}^n)$, named $(n-1)$-plurisubharmonic, such that the complex Hessian matrix

$$\begin{aligned} \bigg (\Big (\sum _{i=1}^{n}\frac{\partial ^2u}{\partial z_m\partial \overline{z}_m}\Big )\delta _{ij}-\frac{\partial ^2u}{\partial z_i\partial \overline{z}_j}\bigg )_{1\le i,j\le n} \end{aligned}$$

(1.3)

is nonnegative definite. For $(n-1)$-plurisubharmonic functions, one can consider the following complex Monge-Ampère equations

$$\begin{aligned} \textrm{det}\bigg (\Big (\sum _{i=1}^{n}\frac{\partial ^2u}{\partial z_m\partial \overline{z}_m}\Big )\delta _{ij}-\frac{\partial ^2u}{\partial z_i\partial \overline{z}_j}\bigg )=\psi (z), \end{aligned}$$

(1.4)

which is related to the Gauduchon conjecture in complex geometry [12, 36]. For more references, we refer the readers to [10, 11, 37] and references therein.

The Dirichlet problem for equation (1.4) on strictly pseudo-convex domains in $\mathbb {C}^n$ was solved by Li [29]. Tosatti-Weinkove [38, 39] showed that the associated Monge-Ampère equation can be solved on any compact K$\ddot{a}$hler manifold. If the complex Hessian matrix in (1.3) is replaced by real Hessian matrix, Harvey-Lawson [25, 27, 28] also investigated the corresponding real Monge-Ampère equation and solved the Dirichlet problem for equation (1.4) with $f=0$ on suitable domains. Recently, Chu-Jiao [7] established Pogorelov type estimates for the Hessian type equation $\sigma _k(\lambda [\Delta u I- D^2u])=\psi (x, u, Du)$. Then Chen-Tu-Xiang [6] generalized Chu-Jiao’s results to Hessian quotient case.

The Hessian type equation for matrix $\Delta u I -D^2u$ also arises in conformal geometry. Let $(M, g_0)$ be a smooth closed Riemannian manifold $n\ge 3$ and $[g_0]$ denote the conformal class of $g_0$ on M. An interesting problem is to find a metric $g \in [g_0]$ which satisfies the following equation

$$\begin{aligned} \sigma _k(A^{\tau }_{g})=f(x). \end{aligned}$$

(1.5)

where the modified Schouten tensor $A_g^{\tau }$ is defined by

$$\begin{aligned} A^{\tau }_{g}={\frac{1}{{n-2}}} \left( {Ric}_{g}- {\frac{{\tau {R}_{g}}}{{2(n-1)}}}g \right) , \end{aligned}$$

$\tau \in \mathbb {R}, {Ric}_{g}$ and ${R}_{g}$ are the Ricci and scalar curvatures of g respectively. When $\tau =1$ and $f\equiv constant$, $A^{1}_{g}$ is just the Schouten tensor $A_g$ and equation (1.5) is the well-known $\sigma _k$-Yamabe problem. Under the conformal transformation ${g}=\exp {(2u)}g_0$, the problem (1.5) reduces to finding a solution $u\in C^{\infty }(M)$ to the following equation

$$\begin{aligned} \sigma _k(W)=f(x)\exp ({2ku}), \end{aligned}$$

(1.6)

where

$$\begin{aligned} W=\frac{\tau -1}{n-2} \triangle u g_0 -\nabla ^2 u + \frac{\tau -2}{2}|\nabla u|^2 g_0+ du\otimes du +A^{\tau }_{g_0}. \end{aligned}$$

Clearly, the matrix $\Delta u I- D^2u$ naturally appears in (1.6) if $\tau = n-1$. For more details see [24, 32].

Another important example is the Plateau type problem in differential geometry: find a hypersurface $\Sigma$ in $\mathbb {R}^{n+1}$ with prescribed Weingarten curvature and boundary data. That is, we seek to solve

$$\begin{aligned} \sigma _k(\kappa )=\psi (X), \quad \partial \Sigma =\Gamma , \end{aligned}$$

(1.7)

where $\psi$ is a positive function and $\kappa =(\kappa _1,\cdots ,\kappa _n)$ are the principal curvatures of $\Sigma$. For the Plateau type problem (1.7), Caffarelli-Nirenberg-Spruck [2] initiated the study of vertical graphs over strictly convex domains in $\mathbb {R}^n$. Later Guan-Spruck [19] studied radial graphs in $\mathbb {R}^{n+1}$ of the constant Gauss-Kronecker curvature, where they replaced the convexity assumption of the domain by a subsolution condition. It’s worth noting that the subsolution assumption is later widely used for the Dirichlet boundary value problems for general curvature equations (see [8, 14,15,16,17,18, 20, 21]). Recently, Su [33] considered the general prescribed curvature equations (namely $\sigma _k$ is replaced by a general curvature function f) for strictly locally convex hypersurfaces, i.e., hypersurfaces with positive principal curvature everywhere. With the help of the a priori estimates for strictly locally convex radial graphs with prescribed Weingarten curvature and boundary in space forms, Sui [34, 35] established the existence results for problem (1.7) in space forms and Hyperbolic space. Hence, it is natural to study equation (1.1) in the strictly locally convex case with the known boundary data.

We may assume that $\Sigma$ is a star-shaped hypersurface in $\mathbb {R}^{n+1}$ and can be represented as a radial graph

$$\begin{aligned}X(x)=\rho (x)x,~~~~{x}~ \in \overline{\Omega }, \end{aligned}$$

where $\Omega$ is a relatively open portion of $\mathbb {S}^n$ with smooth boundary,

$$\begin{aligned} \partial \Sigma =\Gamma = \phi (x) x, \quad x \in \partial \Omega \subset \mathbb {S}^n. \end{aligned}$$

Then equation (1.1) with boundary condition (1.2) is reduced by the following Dirichlet problem of Hessian quotient type equation

$$\begin{aligned} \left\{ \begin{aligned}&\left( \frac{\sigma _k}{\sigma _l}\right) ^{\frac{1}{k-l}}(\lambda [\eta ])=\psi (X,\nu ),{} & {} \quad {in}~ \Omega ,\\&\rho (x)=\phi (x),{} & {} \quad {on}~\partial \Omega , \end{aligned} \right. \end{aligned}$$

(1.8)

where $\kappa =(\kappa _1, \cdots , \kappa _n)$ is the principal curvatures of hypersurface $\Sigma$ and

$$\begin{aligned} \lambda _i[\eta ]=\sum _{j=1}^n\kappa _j-\kappa _i, \quad \quad \forall ~ i =1,\cdots , n. \end{aligned}$$

We need the following Assumption 1.1 to obtain the a priori estimates.

Assumption 1.1

• (i) Assume ${\overline{\Omega }}$ does not contain any hemisphere.

• (ii) There exists a smooth radial graph $\Sigma ': \overline{X}(x)=\overline{\rho }(x)x$, whose principal curvatures of $\Sigma '$ are greater than a positive constant, satisfying

  $$\begin{aligned} \left\{ \begin{aligned}&\left( \frac{\sigma _k}{\sigma _l}\right) ^{\frac{1}{k-l}}(\lambda [\eta (\overline{X})])>\psi (\overline{X},\nu (\overline{X})),{} & {} \quad {in}~ \Omega ,\\&\overline{\rho }= \phi ,{} & {} \quad \text {on}~ \partial \Omega . \end{aligned} \right. \end{aligned}$$

  (1.9)

We have the following Theorem.

Theorem 1.2

Let $2\le k\le n$, $0\le l\le \min \{k-1, n-2\}$. Suppose that $\Sigma$ is a smooth strictly locally convex hypersurface with a radial graph $X(x)= \rho (x) x$ such that $\rho$ satisfying equation (1.8) with $\rho \le \overline{\rho }$. Under Assumption 1.1, we have

$$\begin{aligned} ||\rho ||_{C^2 } <K, \quad \kappa _i \le K, \end{aligned}$$

where K is a constant depending on $n, k,l, \Omega , \inf \psi , ||\psi ||_{C^{\infty } }, ||\phi ||_{C^{\infty } }, \inf \overline{\rho }$, $||\overline{\rho }||_{C^{\infty } }$ and the convexity of $\overline{\rho }$.

Remark 1.3

Assumption 1.1 (i) introduced in [19, 33, 34] is used to prove $C^0$ and $C^1$ estimates for the solution of equation (1.8). Although Guan-Ren-Wang [23] showed that $C^2$ estimates failed for the classical Hessian quotient type curvature equations

$$\begin{aligned} \frac{\sigma _k(\kappa )}{\sigma _l(\kappa )}=\psi (X, \nu ), \quad 2\le k\le n,~ 0 \le l\le k-1, \end{aligned}$$

the a priori estimates for equation (1.8) are feasible.

Inspired by Su and Sui’s idea [33, 34], in this article we prove an existence result by continuity method and degree theory. The preservation of strictly local convexity of solutions is vital in order to perform the continuity process. Thus we impose the following assumptions on the subsolution and $\psi$ to guarantee the constant rank property for the second fundamental form on $\Sigma$ which is based on the ideas of [35].

Assumption 1.4

• (iii) Assume $\frac{1}{\psi }(X, y)$ is locally convex in X for any $y\in \mathbb {S}^n$.

• (iv) The smooth strictly locally convex radial graph $\Sigma ': \overline{X}(x)=\overline{\rho }(x)x$ satisfies

  $$\begin{aligned} \left[ \left( \ln (\overline{\rho }\underline{\psi }) \right) _{ij} \right] <0\quad \text{ in } ~ \overline{\Omega }, \end{aligned}$$

  where $\underline{\psi }:=\left( \frac{\sigma _k}{\sigma _l}\right) ^{\frac{1}{k-l}}(\lambda [\eta (\overline{X})])$.

Combined with the constant rank theorem, we have the following Theorem.

Theorem 1.5

Let $2\le k\le n$, $0\le l\le \min \{k-1, n-2 \}$, $\phi \equiv \frac{1}{a}$ be a constant determined by (4.7). Under Assumption 1.1 and Assumption 1.4, there exists a smooth, strictly locally convex hypersurface $\Sigma$ which is a radial graph $X(x)= \rho (x) x$ with $\rho \le \overline{\rho }$ in $\Omega$ satisfying equation (1.8).

The organization of the paper is as follows. In Sect. 2 we start with some preliminaries. We deal with the a priori estimates in Sect. 3. In Sect. 4 we complete the proof of Theorem 1.5 by constructing a two-step continuity process.

2 Preliminaries

2.1 Star-Shaped Hypersurfaces in $\mathbb {R}^{n+1}$

Let $\Sigma$ be a star-shaped hypersurface in $\mathbb {R}^{n+1}$ which can be represented by

$$\begin{aligned} X(x)=\rho (x)x, \quad \quad {x}~ \in \overline{\Omega }, \end{aligned}$$

where X is the position vector of the hypersurface $\Sigma$ $\subset \mathbb {R}^{n+1}$.

Let $\nabla$ be the Levi-Civita connection of $\mathbb {S}^n$ and $\{e_1,\cdots , e_n\}$ be a smooth orthonormal frame field on $\overline{\Omega }\subset \mathbb {S}^n$. We set $u(x)=\frac{1}{\rho (x)}$ and push $\{e_i\}$ forward to a frame field $\{\tau _{i}\}$ on $\Sigma$, where $\tau _i= -\frac{\nabla _{e_i} u}{u^2}x+ \frac{1}{u} e_i$. From [33], the metric of $\Sigma$ can be given by

$$\begin{aligned} g_{ij}=\frac{1}{u^2} \delta _{ij} + \frac{1}{u^4} u_i u_j, \end{aligned}$$

and its inverse is given by

$$\begin{aligned}g^{ij}= u^2 (\delta _{ij}- \frac{u_i u_j}{w^2}).\end{aligned}$$

The second fundamental form of $\Sigma$ can be written as

$$\begin{aligned} h_{ij}=\frac{1}{uw}\left( u\delta _{ij} +u_{ij}\right) , \end{aligned}$$

where $w=\sqrt{u^2+|\nabla u|^2}$. The principal curvatures of $\Sigma$ are the eigenvalues of the symmetric matrix $[a_{ij}]$, where

$$\begin{aligned} a_{ij}= & {} \frac{u}{w} \gamma ^{ik} (u \delta _{kl}+u_{kl}) \gamma ^{lj},\\ \gamma ^{ij}= & {} \delta _{ij}-\frac{u_i u_j}{w(u+w)},~~~~\gamma _{ij}= \delta _{ij}+\frac{u_i u_j}{u(u+w)}. \end{aligned}$$

Obviously $\Sigma$ is strictly locally convex if and only if the matrix

$$\begin{aligned}{}[u\delta _{ij}+u_{ij}]>0 ~\quad \text{ in } \Omega . \end{aligned}$$

(2.1)

Hence we say u is strictly locally convex if (2.1) holds.

2.2 Inequalities and Notations

Definition 2.1

A smooth hypersurface $\Sigma \subset \mathbb {R}^{n+1}$ is called $(\eta , k)$-convex if $\lambda [\eta ] \in \Gamma _k$, where $\Gamma _k$ is the Garding’s cone

$$\begin{aligned} \Gamma _{k}=\{\lambda \in \mathbb {R} ^n: \sigma _{j}(\lambda )>0, \forall ~ 1\le j \le k\}. \end{aligned}$$

The following Lemmas (see [31, 40]) will be used later.

Lemma 2.2

Let $\lambda \in \mathbb {R}^n$. For $0\le l<k\le n,$ $r>s\ge 0, k\ge r, l\ge s$, we have the Newton-Maclaurin inequality:

$$\begin{aligned} \big [\frac{\sigma _k(\lambda )/C^k_n}{\sigma _l(\lambda )/C^l_n}\big ]^\frac{1}{k-l} \le \big [\frac{\sigma _r(\lambda )/C^r_n}{\sigma _s(\lambda )/C^s_n}\big ]^{\frac{1}{r-s}},~ \lambda \in \Gamma _k. \end{aligned}$$

Lemma 2.3

Let $[P_{ij}]$ be an $n \times n$ matrix with $\lambda ([P_{ij}])\in \Gamma _2$. Then

$$\begin{aligned} |P_{ij}|\le \sum _{k}P_{kk},~\forall ~1\le i,j\le n. \end{aligned}$$

For convenience, we introduce the following notations:

$$\begin{aligned} G(\eta ):= \left( \frac{\sigma _k(\lambda [\eta ])}{\sigma _l(\lambda [\eta ])}\right) ^{\frac{1}{k-l}},~ G^{ij}:=\frac{\partial G}{ \partial \eta _{ij}},~ G^{ij, rs}:= \frac{\partial ^2 G}{\partial \eta _{ij} \partial \eta _{rs}}. \end{aligned}$$

(2.2)

Thus,

$$\begin{aligned} G^{ij}= \frac{1}{k-l} \left( \frac{\sigma _k(\lambda [\eta ])}{\sigma _l(\lambda [\eta ])}\right) ^{\frac{1}{k-l}-1} \frac{T^{ij}_{k-1}(\eta )\sigma _l(\lambda [\eta ])-\sigma _k(\lambda [\eta ])T^{ij}_{l-1}(\eta )}{\sigma _l^2(\lambda [\eta ])}, \end{aligned}$$

where

$$\begin{aligned} T^{ij}_k(\eta ):= \frac{1}{k!} \left( \begin{array}{c} i_1\cdots i_{k} i \\ j_1\cdots j_{k}j \end{array} \right) \eta _{i_1j_1}\cdots \eta _{i_{k}j_{k}}. \end{aligned}$$

When $\eta =\text{ diag }(\mu _1, \mu _2, \cdots , \mu _n)$ with $\mu _1 \le \mu _2\le \cdots \le \mu _n$, it yields

$$\begin{aligned}G^{11}\ge G^{22} \ge \cdots \ge G^{nn}. \end{aligned}$$

The ellipticity of equation (1.8) is dependent on the following proposition whose proof is similar to Proposition 2.2.3 in [3].

Proposition 2.4

Let $\Sigma$ be a smooth $(\eta , k)$-convex hypersurface in $\mathbb {R}^{n+1}$ and $0\le l< k-1$. Then the operator

$$\begin{aligned} G(\eta )=\left( \frac{\sigma _k(\lambda [\eta ])}{\sigma _{l}(\lambda [\eta ])}\right) ^{\frac{1}{k-l}} \end{aligned}$$

is elliptic and concave with respect to $\eta$. Moreover, we have

$$\begin{aligned} \sum G^{ii} \ge \left( \frac{C_n^k}{C_n^l}\right) ^{\frac{1}{k-l}}. \end{aligned}$$

Let

$$\begin{aligned} Q(\nabla ^2u, \nabla u, u):=G([b_{ij}])= \left( \frac{\sigma _k}{\sigma _l}\right) ^{\frac{1}{k-l}}(\lambda [b_{ij}]), \end{aligned}$$

(2.3)

where

$$\begin{aligned}{}[b_{ij}]=T(A)=\left( \text{ trace }A\right) I-A,\quad A=[a_{ij}]. \end{aligned}$$

(2.4)

Then equation (1.8) can be rewritten as

$$\begin{aligned} \left\{ \begin{aligned}&Q(\nabla ^2u, \nabla u, u)=\psi (x, u, \nabla u) ,{} & {} \quad {in}~ \Omega ,\\&u= \frac{1}{\phi (x)},{} & {} \quad {on}~ \partial \Omega . \end{aligned} \right. \end{aligned}$$

(2.5)

In the later calculation, $(\nabla ^2u, \nabla u, u)$ is replaced by (r, p, u). Denote

$$\begin{aligned} Q^{ij}(r,p,u)= & {} \frac{\partial Q}{\partial r_{ij}}(r,p,u),\quad Q^{i}(r,p,u)=\frac{\partial Q}{\partial p_{i}}(r,p,u),\\ Q_u(r,p,u)= & {} \frac{\partial Q}{\partial u}(r,p,u),\\ \psi _{u}(x,u,p)= & {} \frac{\partial \psi }{\partial u}(x,u,p),\quad \psi ^i(x,u,p)=\frac{\partial \psi }{\partial p_i}(x,u,p). \end{aligned}$$

Then we have

$$\begin{aligned} Q^{ij}=\frac{\partial Q}{\partial u_{ij}}= \frac{u}{w} G^{st} (\delta _{kl}\delta _{st}-\delta _{ks}\delta _{lt}) \gamma ^{ik} \gamma ^{lj},\end{aligned}$$

(2.6)

$$\begin{aligned}Q^{ij, rh}=\frac{\partial ^2 Q}{\partial u_{ij}\partial u_{rh}}= \frac{u^2}{w^2} G^{st, s^\prime t^\prime }(\delta _{kl}\delta _{st}-\delta _{ks}\delta _{lt})(\delta _{k^\prime l^\prime }\delta _{s^\prime t^\prime }-\delta _{k^\prime s^\prime }\delta _{l^\prime t^\prime }) \gamma ^{ik} \gamma ^{lj} \gamma ^{rk^\prime } \gamma ^{hl^\prime }.\end{aligned}$$

Therefore Q is elliptic and concave with respect to $u_{ij}$ for strictly locally convex function u. Moreover, from Assumption 1.1 (ii), the function $\underline{u}=\frac{1}{\overline{\rho }}$ is a subsolution of equation (2.5), i.e.,

$$\begin{aligned} \left\{ \begin{aligned}&Q(\nabla ^2\underline{u}, \nabla \underline{u}, \underline{u})>\psi (x, \underline{u}, \nabla \underline{u}) ,{} & {} \quad {in}~ \Omega ,\\&\underline{u}= \frac{1}{\phi (x)},{} & {} \quad {on}~ \partial \Omega . \end{aligned} \right. \end{aligned}$$

(2.7)

3 A Priori Estimates

In this section, we establish the a priori estimates for strictly locally convex solution u of equation (2.5) with $u\ge \underline{u}$ in $\Omega$. For convenience, we will use a unified notation K to denote a positive constant depending on $n, k, l, \Omega , \inf \underline{u}, ||\underline{u}||_{C^4}$, $||\psi ||_{C^2}$, $||\phi ||_{C^4}$ and $\inf \psi$.

3.1 $C^0$ and $C^1$ Estimates

Firstly we show the following $C^0$ and $C^1$ estimates.

Theorem 3.1

($C^0$ and $C^1$ estimates) Under Assumption 1.1, for any strictly locally convex function u with $u\ge \underline{u}$ in $\Omega$ and $u=\underline{u}$ on $\partial \Omega$, we have

$$\begin{aligned} \frac{1}{K}\le u\le K , ~~~ ~|\nabla u|\le K, \end{aligned}$$

where K is a constant depending on $\Omega , \sup \underline{u}$, $\inf \underline{u}$ and $\sup |\nabla \underline{u}|$.

Proof

$C^0$ and $C^1$ estimates are established in [33, Lemma 3.3], so we omit the proof here. $\square$

3.2 $C^2$ Estimates

Next, we’ll derive the following interior $C^2$ estimates.

Theorem 3.2

Let $2\le k\le n$ and $0\le l\le k-1$. Under Assumption 1.1, let $u\in C^4(\Omega )\cap C^2(\overline{\Omega })$ be a strictly locally convex solution of the Dirichlet problem (2.5) with $u\ge \underline{u}$. Then there exists a constant K depending on n, k, l, $\Omega$, $\inf \underline{u}$, $||\underline{u}||_{C^4}$, $||\psi ||_{C^2}$, $\inf \psi$ such that

$$\begin{aligned}\sup _{\Omega } |\nabla ^2 u| \le K(1+ \sup _{\partial \Omega } |\nabla ^2 u|).\end{aligned}$$

Proof

We omit the proof of Theorem 3.2 since it is similar to Theorem 3.4 in [5]. $\square$

3.2.1 Some Properties

In order to get the boundary estimates of the second derivative of u, we need some properties of the linearized operator. Let $\{e_1, \cdots , e_n\}$ be a local orthonormal frame field on $\mathbb {S}^n$ , $Q^{ij} :=\frac{\partial Q}{\partial u_{ij}}$, $Q^s:=\frac{\partial Q}{\partial u_s}$ and $Q_u:=\frac{\partial Q}{\partial u}$. Define

$$\begin{aligned} Lw:=Q^{ij} w_{ij}+ (Q^s-\psi ^s) w_s, \end{aligned}$$

for a $C^2$ function $w: \Omega \rightarrow \mathbb {R}$. We have the following Lemma.

Lemma 3.3

Let u be a smooth strictly locally convex solution of (2.5) with $u\ge \underline{u}$. Under Assumption 1.1, there exists a positive constant K such that

$$\begin{aligned} |Q^s|\le K, \quad |Q_u|\le K \left( 1+\sum _i Q^{ii}\right) . \end{aligned}$$

Proof

Using (2.4), it follows that

$$\begin{aligned} Q^s= G^{rt}\frac{\partial b_{rt}}{\partial a_{ij}} \frac{\partial a_{ij}}{\partial u_s}, \quad \frac{\partial b_{rt}}{\partial a_{ij}}=(\delta _{ij}\delta _{rt}-\delta _{ir}\delta _{jt}). \end{aligned}$$

Moreover,

$$\begin{aligned} \begin{aligned} \frac{\partial a_{ij}}{\partial u_s}&= u(u \delta _{kl}+u_{kl}) \frac{\partial }{\partial u_s} \left( \frac{\gamma ^{ik} \gamma ^{lj}}{w} \right) = -\frac{u_s}{w^2} a_{ij} +2 \frac{u}{w} (u \delta _{kl}+u_{kl}) \gamma ^{ik} \frac{\partial \gamma ^{lj}}{\partial u_s}\\&=-\frac{u_s}{w^2} a_{ij} +2 a_{ik} \gamma _{kl} \frac{\partial \gamma ^{lj}}{\partial u_s} \end{aligned} \end{aligned}$$

and

$$\begin{aligned} \begin{aligned} \gamma _{kl} \frac{\partial \gamma ^{lj}}{\partial u_s}&=\left( \delta _{kl}+ \frac{u_k u_l}{u(u+w)}\right) \left( \frac{u u_l u_j u_s}{w^3 (u+w)^2} -\frac{u_j \gamma ^{ls}}{w(u+w)} -\frac{u_l \gamma ^{js}}{w(u+w)}\right) \\&=-\frac{u_j \gamma ^{ks}}{w(u+w)}- \frac{u_k \gamma ^{js}}{u(u+w)}. \end{aligned} \end{aligned}$$

Therefore,

$$\begin{aligned} \begin{aligned} Q^s&=-\frac{u_s}{w^2} \left( (\sum _l G^{ll}) \delta _{ij} -G^{ij} \right) a_{ij} -2 \left( \left( \sum _l G^{ll}\right) \delta _{ij} - G^{ij} \right) a_{ik}\\&\quad \left( \frac{u_j \gamma ^{ks}}{w(u+w)}+ \frac{u_k \gamma ^{js}}{u(u+w)} \right) \\&=-\frac{u_s}{w^2} G^{ij} b_{ij}-2 \left( \left( \sum _l G^{ll}\right) \delta _{ij} - G^{ij} \right) a_{ik} \left( \frac{u_j \gamma ^{ks}}{w(u+w)}+ \frac{u_k \gamma ^{js}}{u(u+w)} \right) . \end{aligned} \end{aligned}$$

It is clear that $G^{ij}([b_{ij}])$ and $[b_{ij}]$ can be diagonalized simultaneously by an orthonormal transformation. Then utilizing the $C^0$ and $C^1$ estimates of u, we know that

$$\begin{aligned} |Q^s| \le K \sum _{i=1}^n G^{ii} \lambda _i \le K. \end{aligned}$$

Furthermore, we get

$$\begin{aligned} \begin{aligned} Q_u=&\left( \left( \sum _l G^{ll}\right) \delta _{ij} - G^{ij} \right) \left( \frac{1}{u} a_{ij}- \frac{u}{w^2}a_{ij} +\frac{u}{w} \gamma ^{ik} \gamma ^{kj} + 2a_{ik} \gamma _{kl} \frac{\partial \gamma ^{lj}}{\partial u} \right) \\ =&\frac{|\nabla u|^2}{uw^2}\left( \left( \sum _l G^{ll}\right) \delta _{ij} - G^{ij} \right) a_{ij} +2 \left( \left( \sum _l G^{ll}\right) \delta _{ij} -G^{ij} \right) a_{ik}\frac{u_j u_k}{ uw^2}\\&+ \frac{u}{w} \left( \left( \sum _l G^{ll}\right) \delta _{ij} -G^{ij} \right) \left( \delta _{ij}- \frac{u_i u_j}{w^2}\right) \\ \le&K(1+\sum _i Q^{ii}). \end{aligned} \end{aligned}$$

(3.1)

$\square$

Let t and N be positive constants and d be the distance function to $\partial \Omega$. We define

$$\begin{aligned} v:=u-\underline{u}+td-Nd^2. \end{aligned}$$

(3.2)

We have the following Lemma.

Lemma 3.4

Let u be a smooth strictly locally convex solution of (2.5) with $u\ge \underline{u}$. Fix $x_0\in \partial \Omega$, there exist constants $t, \delta$ sufficiently small and N sufficiently large depending on $\Omega , \inf \underline{u}, ||\underline{u}||_{C^1}, ||\psi ||_{C^1}$ and the convexity of $\underline{u}$ such that

$$\begin{aligned} \left\{ \begin{aligned}&Lv\le -1-\beta \sum Q^{ii},{} & {} \quad in~ \Omega \cap B_{\delta }(x_0),\\&v\ge 0,{} & {} \quad on~ \partial (\Omega \cap B_{\delta }(x_0)), \end{aligned} \right. \end{aligned}$$

(3.3)

where $\beta >0$ depends only on the convexity of $\underline{u}$, $B_{\delta }(x_0)$ is a ball of radius $\delta$ centered at $x_0$.

Proof

Choose $\delta _0>0$ sufficiently small such that d is smooth in $\Omega \cap B_{\delta _0}$ and

$$\begin{aligned} |\nabla d|=1, \quad -C_0I\le \nabla ^2 d\le C_0 I, \end{aligned}$$

where $C_0$ depends only on $\delta _0$ and $\Omega$. Moreover, according to the convexity of $\Sigma '$, we can find $\beta >0$ such that

$$\begin{aligned} {[}\underline{u} \delta _{ij}+ \underline{u}_{ij}] \ge 4 \beta I. \end{aligned}$$

Define $T(A):=(\text{ trace }A) I-A$ for any matrix A. From the concavity of G and the fact that $u\ge \underline{u}$, we get

$$\begin{aligned} \begin{aligned}&G\left( T\left[ \frac{u}{w} \gamma ^{ik} (\underline{u} \delta _{kl}+\underline{u}_{kl}+N (d^2)_{kl}-2\beta \delta _{kl}) \gamma ^{lj}\right] \right) -\psi (X,\nu )\\&\quad =G\left( T\left[ \frac{u}{w} \gamma ^{ik} (\underline{u} \delta _{kl}+\underline{u}_{kl}+N (d^2)_{kl}-2\beta \delta _{kl}) \gamma ^{lj}\right] \right) \\&\quad - G\left( T\left[ \frac{u}{w} \gamma ^{ik} (u \delta _{kl}+u_{kl}) \gamma ^{lj} \right] \right) \\&\quad \le \frac{u}{w} G^{st} (\delta _{kl}\delta _{st}-\delta _{ks}\delta _{lt}) \gamma ^{ik} \gamma ^{lj} (\underline{u} \delta _{ij}+\underline{u}_{ij}+N (d^2)_{ij}-2\beta \delta _{ij}-u\delta _{ij}-u_{ij})\\&\quad =Q^{ij} (\underline{u}-u+Nd^2)_{ij}+(\underline{u}-u) \sum Q^{ii} -2\beta \sum Q^{ii}. \end{aligned} \end{aligned}$$

Then using Lemma 3.3, we obtain

$$\begin{aligned} Lv= & {} Q^{ij}\nabla _{ij} (u-\underline{u}+td-Nd^2)+ (Q^s-\psi ^s) \nabla _s (u-\underline{u}+td-Nd^2)\nonumber \\= & {} Q^{ij}(u-\underline{u}-Nd^2)_{ij}+t(Q^{ij}d_{ij}+(Q^s-\psi ^s)d_s)\nonumber \\{} & {} +(Q^s-\psi ^s)(u-\underline{u})_s-2Nd(Q^s-\psi ^s)d_s\nonumber \\\le & {} \psi (X, \nu )- G\left( T\left[ \frac{u}{w} \gamma ^{ik} (\underline{u} \delta _{kl}+\underline{u}_{kl}+N (d^2)_{kl}-2\beta \delta _{kl}) \gamma ^{lj}\right] \right) \nonumber \\{} & {} +(C_0t-2\beta ) \sum Q^{ii}+K(1+t+2N\delta ). \end{aligned}$$

(3.4)

When we choose $\delta \le \frac{\beta }{2NC_0}$, it follows that

$$\begin{aligned} \underline{u} I + \nabla ^2\underline{u}+N \nabla ^2(d^2)-2\beta I= & {} \underline{u}I +\nabla ^2\underline{u}+2Nd \nabla ^2d+2N \nabla d \otimes \nabla d -2\beta I\nonumber \\\ge & {} \underline{u}I +\nabla ^2\underline{u}+2N \nabla d \otimes \nabla d -3\beta I\nonumber \\\ge & {} 2N \nabla d \otimes \nabla d +\beta I:=\mathcal {H}. \end{aligned}$$

(3.5)

Denote $u[\gamma ^{ij}]$ by $g^{-\frac{1}{2}}$. We have

$$\begin{aligned}{} & {} G\Big (T\Big (\left[ \frac{u}{w} \gamma ^{ik} (\underline{u} \delta _{kl}+\underline{u}_{kl}+N (d^2)_{kl}-2\beta \delta _{kl}) \gamma ^{lj}\right] \Big )\Big )\nonumber \\{} & {} \quad \ge G\Big (T\Big ( \frac{1}{uw} g^{-\frac{1}{2}} \mathcal {H} g^{-\frac{1}{2}}\Big )\Big ) = G\Big (T\Big (\frac{1}{uw} \mathcal {H}^{\frac{1}{2}} g^{-1} \mathcal {H}^{\frac{1}{2}}\Big )\Big )\nonumber \\{} & {} \quad \ge G\Big (T\Big (\frac{1}{uw} \mathcal {H}^{\frac{1}{2}} (\frac{u^4}{w^2}I)\mathcal {H}^{\frac{1}{2}}\Big )\Big )\nonumber \\{} & {} \quad \ge K G\Big (T\Big (\mathcal {H}\Big )\Big ). \end{aligned}$$

(3.6)

Without loss of generality we write $\mathcal {H}=\text{ diag }\{2N+\beta ,\beta ,\cdots ,\beta \}$. Now choose t small enough such that $C_0t+t\le \beta$. By using (3.4), (3.5) and (3.6), it follows that

$$\begin{aligned} Lv\le & {} \psi (X,\nu )-K G(T(\mathcal {H}))+(C_0t-2\beta ) \sum Q^{ii}+K(1+t+2N\delta )\\\le & {} \psi (X,\nu )-K G(T(\mathcal {H}))-\beta \sum Q^{ii}+K(1+\beta +2N\delta ). \end{aligned}$$

Finally we choose N large enough such that (3.3) holds. $\square$

3.2.2 Boundary Estimates

Theorem 3.5

Let $2\le k\le n$, $0\le l\le \min \{k-1, n-2\}$, $u\in C^4(\Omega )\cap C^2(\overline{\Omega })$ be a strictly locally convex solution of the Dirichlet problem (2.5) with $u\ge \underline{u}$. Under Assumption 1.1, there exists a constant K such that

$$\begin{aligned} \max _{\partial \Omega } |\nabla ^2 u| \le K. \end{aligned}$$

Proof

For any point $x_0\in \partial \Omega$, let $\{e_1, \cdots , e_{n}\}$ be a local orthonormal frame field on $\mathbb {S}^n$ around $x_0$. Here $\{e_1, \cdots , e_{n-1}\}$ is a local orthonormal frame field on $\partial \Omega$. $e_n$ is obtained by a parallel translation of the unit inner normal vector field along the geodesic perpendicular to $\partial \Omega$.

$\textbf{Case}~\mathbf {1:}$ Estimates of $u_{\alpha \beta }$ on $\partial \Omega$ for $1\le \alpha ,\beta \le n-1$.

Since $u-\underline{u}=0$ on $\partial \Omega$, we obtain

$$\begin{aligned} (u-\underline{u})_{\alpha }=0, \quad ((u-\underline{u})_\alpha )_\beta =0 \end{aligned}$$

for any $\alpha , \beta \in 1,\cdots , n-1$. Then we have

$$\begin{aligned} (u-\underline{u})_{\alpha \beta }=-(\textbf{n}\cdot \nabla _{\beta }e_{\alpha } ) (u-\underline{u})_n, \end{aligned}$$

where $\textbf{n}$ is the unit inner normal of $\partial \Omega$. It follows that

$$\begin{aligned} |u_{\alpha \beta }(x_0)|\le K,\quad \alpha , \beta = 1,\cdots , n-1. \end{aligned}$$

(3.7)

$\textbf{Case}~\mathbf {2:}$ Estimates of $u_{\alpha n}$ on $\partial \Omega$.

Define

$$\begin{aligned} \Psi := Ev + B\rho ^2, \end{aligned}$$

where v is as in Lemma 3.4, $\rho$ is the distance function to $x_0$ and E, B are large positive constants to be determined later. Note that there exists $C_1$ depending only on $\Omega$ such that

$$\begin{aligned} |\nabla (\Gamma ^l_{ij})|+|\Gamma ^l_{ij}|\le C_1\quad \nabla ^2(\rho ^2)\le C_1 I, \quad 1\le i,j,l\le n. \end{aligned}$$

Using Lemma 3.3 directly, we get

$$\begin{aligned} L\Psi \le E\left( -1-\beta \sum Q^{ii} \right) +BK (1+\sum Q^{ii} ). \end{aligned}$$

(3.8)

Combining (2.6) and Lemma 2.3, we therefore obtain

$$\begin{aligned} Q^{ij}\Gamma ^l_{i\alpha }\big (u_{jl}+u\delta _{jl}\big ) =(\gamma ^{ir}\Gamma ^l_{i\alpha }\gamma _{zl})(G^{st}(\delta _{rh}\delta _{st}-\delta _{rs}\delta _{ht})a_{hz}) \le K. \end{aligned}$$

The term $Q^{ij}\Gamma ^l_{j\alpha }u_{il}$ can be calculated similarly. Hence,

$$\begin{aligned} |L(\nabla _{\alpha } u)|= & {} |Q^{ij}(\nabla _{\alpha } u)_{ij}+(Q^s-\psi ^s)\nabla _{s}(\nabla _{\alpha } u)|\nonumber \\= & {} |Q^{ij}u_{ij\alpha }+(Q^s-\psi ^s)u_{s\alpha }\nonumber \\{} & {} +Q^{ij}\Gamma ^l_{i\alpha }u_{jl}+Q^{ij}\Gamma ^l_{j\alpha }u_{il}+ Q^{ij}(\nabla _{e_\alpha }\Gamma ^l_{ij})u_l+(Q^s-\psi ^s)\Gamma ^l_{s\alpha }u_l|\nonumber \\\le & {} K (1+\sum _i Q^{ii}). \end{aligned}$$

(3.9)

Using the fact $\underline{u}\in C^2(\overline{\Omega })$, we obtain

$$\begin{aligned} \left| L(u-\underline{u})_{\alpha }\right| \le K (1+\sum _i Q^{ii}). \end{aligned}$$

(3.10)

Then we can choose a constant B large enough such that

$$\begin{aligned}\Psi =Ev+B\rho ^2\ge \pm (u-\underline{u})_{\alpha } \quad \text{ on }~\partial (\Omega \cap B_{\delta }(x_0)).\end{aligned}$$

Combining (3.8) and (3.10), we pick $E>>B$ such that

$$\begin{aligned} L(\Psi \pm (u-\underline{u})_{\alpha })\le & {} E\left( -1-\beta \sum Q^{ii} \right) +BK\left( 1+\sum Q^{ii} \right) \\\le & {} 0. \end{aligned}$$

Then by maximum principles, we have

$$\begin{aligned} \Psi \ge \pm (u-\underline{u})_{\alpha } \quad \text{ in }~\Omega \cap B_{\delta }(x_0). \end{aligned}$$

Note that $\Psi (x_0)=(u-\underline{u})_{\alpha }(x_0)=0$, thus

$$\begin{aligned} -\Psi _n(x_0)\le (u-\underline{u})_{\alpha n}(x_0)\le \Psi _n(x_0), \end{aligned}$$

which implies

$$\begin{aligned} |u_{\alpha n}|\le K. \end{aligned}$$

$\textbf{Case}~\mathbf {3:}$ Estimates of $u_{nn}$ on $\partial \Omega$.

It suffices to derive an upper bound $u_{nn}\le K \quad ~\text{ on }~\partial \Omega$. We will divide the following argument into two cases.

$\textbf{Case}~\mathbf {3.1:}$ $k<n$.

Note that $0<\frac{1}{K} \le u \le K$, $|\nabla u|\le K$,

$$\begin{aligned} {[}\gamma ^{ij}] \le K I, \quad |u_{\alpha \beta }|+|u_{\alpha n}| \le K, \quad \alpha , \beta \in 1, \cdots , n-1. \end{aligned}$$

Let $W=[u\delta _{ij}+u_{ij}]$, we may assume $W_{\alpha \beta }=0$ for any $\alpha \ne \beta$ with $\alpha , \beta =1, 2, \cdots , n-1$. Then we can assume that $u_{nn}$ is large enough such that

$$\begin{aligned}\frac{1}{2} W_{nn} > \sum _{\alpha =1}^{n-1} W_{\alpha \alpha }.\end{aligned}$$

It follows that

$$\begin{aligned} T(W)= & {} \left( \begin{array}{cccc} \sum _{i}W_{ii}-W_{11}&{}\cdots &{}0&{}-W_{1n}\\ \vdots &{}\ddots &{}\vdots &{}\vdots \\ 0 &{}\cdots &{}\sum _{i}W_{ii}-W_{n-1,n-1}&{}-W_{n-1,n}\\ -W_{n1}&{}\cdots &{}-W_{n,n-1}&{}\sum _{i}W_{ii}-W_{nn} \end{array} \right) \\\ge & {} \left( \begin{array}{cccc} \frac{1}{2}W_{nn}&{}\cdots &{}0&{}-W_{1n}\\ \vdots &{}\ddots &{}\vdots &{}\vdots \\ 0 &{}\cdots &{}\frac{1}{2}W_{nn}&{}-W_{n-1,n}\\ -W_{n1}&{}\cdots &{}-W_{n,n-1}&{}\sum _{i\ne n}W_{ii} \end{array} \right) :=[P_{ij}]. \end{aligned}$$

It is easily seen that

$$\begin{aligned} \lambda _1([P_{ij}])=\cdots =\lambda _{n-2} ([P_{ij}])= \frac{1}{2} W_{nn}, \ \ \lambda _{n-1}([P_{ij}])\ge \frac{1}{2} W_{nn}, \end{aligned}$$

and

$$\begin{aligned} |\lambda _{n}([P_{ij}])|\le \sum _{i=1}^n\sum _{\alpha =1}^{n-1} |W_{i\alpha }| \le K, \end{aligned}$$

which implies that

$$\begin{aligned} \psi= & {} \frac{\sigma _k}{\sigma _l}^{\frac{1}{k-l}}\left( \sigma _1(\kappa )-\kappa _1, \sigma _1(\kappa )-\kappa _2, \cdots , \sigma _1(\kappa )-\kappa _n\right) = G(T (\frac{u}{w} \gamma W \gamma ))\\\ge & {} K G(T (W)) \ge K \frac{\sigma _k}{\sigma _l}^{\frac{1}{k-l}}\left( \lambda [P_{ij}]\right) \ge K \frac{\sigma _k}{\sigma _l}^{\frac{1}{k-l}} \left( \frac{1}{2} W_{nn}, \cdots , \frac{1}{2} W_{nn}, -K\right) \\> & {} \sup \psi . \end{aligned}$$

This contradiction gives $u_{nn}\le K.$

$\mathbf {Case~3.2:}$ $k=n$.

Now we claim that

$$\begin{aligned} M:=\min _{x\in \partial \Omega } \max _{\eta \in T_x(\partial \Omega ), |\eta |=1} (u+ u_{\eta \eta })\ge K>0, \end{aligned}$$

(3.11)

where $T_x(\partial \Omega )$ denotes the tangent space of $\partial \Omega$ at $x\in \partial \Omega$. To show (3.11), we adopt ideas in [33]. Let $\sigma \in C^{\infty }(\mathbb {S}^n)$ such that

$$\begin{aligned} \Omega =\{\sigma <0\}, \quad \partial \Omega =\{\sigma =0\}, \quad \nabla \sigma =-\textbf{n}\quad \text{ on }~\partial \Omega , \end{aligned}$$

where $\textbf{n}$ is the unit inner normal vector to $\partial \Omega$. We may assume

$$\begin{aligned}||\sigma ||_{C^2}\le C_2,\end{aligned}$$

where $C_2$ depends only on $\Omega$. Note that for any $\eta \in T_x(\partial \Omega )$,

$$\begin{aligned} u_{\eta \eta } = \underline{u}_{\eta \eta } - \textbf{n}\cdot \nabla (u-\underline{u}) \sigma _{\eta \eta } \quad \text{ on }~\partial \Omega . \end{aligned}$$

(3.12)

Suppose that M is achieved at $x_1\in \partial \Omega$ with $\xi \in T_{x_1} (\partial \Omega )$. We choose a local orthonormal frame field ${e_1, \cdots , e_n}$ around $x_1$ such that $e_1|_{x_1} = \xi$ and $(u+u_{11})(x_1) =\max _{\eta \in T_x(\partial \Omega ), |\eta |=1} (u+u_{\eta \eta })(x)$. Hence

$$\begin{aligned} M=u(x_1)+ u_{11}(x_1)=\underline{u}(x_1)+ \underline{u}_{11} (x_1)-\textbf{n}\cdot \nabla (u-\underline{u})(x_1) \sigma _{11}(x_1). \end{aligned}$$

If

$$\begin{aligned} \textbf{n} \cdot \nabla (u-\underline{u})(x_1) \sigma _{11} (x_1) \le \frac{1}{2} (\underline{u}(x_1) + \underline{u}_{11} (x_1)), \end{aligned}$$

the claim is clearly established by the strictly local convexity of $\Sigma$. So we only need to consider the following case

$$\begin{aligned} \textbf{n} \cdot \nabla (u-\underline{u})(x_1) \sigma _{11} (x_1) > \frac{1}{2} (\underline{u}(x_1) + \underline{u}_{11} (x_1)). \end{aligned}$$

Let $\zeta =(\zeta ^1, \cdots , \zeta ^n)$ be defined as

$$\begin{aligned} {\left\{ \begin{array}{ll} \zeta ^1= - \frac{ \sigma _n}{\sqrt{( \sigma _1)^2+(\sigma _n)^2}},\\ \zeta ^j=0, \quad 2\le j\le n-1, \\ \zeta ^n=\frac{ \sigma _1}{\sqrt{( \sigma _1)^2+(\sigma _n)^2}}. \end{array}\right. } \end{aligned}$$

(3.13)

Since $\sigma _{ij} \zeta ^i \zeta ^j$ is continuous and $0\le \textbf{n}\cdot \nabla (u-\underline{u})\le K$ on $\partial \Omega$, there exist $c>0$ and $\delta >0$ such that

$$\begin{aligned} \begin{aligned} \sigma _{ij} \zeta ^i \zeta ^j (x)\ge \frac{1}{2} \sigma _{ij} \zeta ^i \zeta ^j (x_1)=\frac{\sigma _{11} (x_1)}{2}>\frac{\underline{u}(x_1)+\underline{u}_{11}(x_1)}{4\textbf{n}\cdot \nabla (u-\underline{u})(x_1)}\ge c \end{aligned} \end{aligned}$$

(3.14)

for any $x\in \Omega \cap B_{\delta }(x_1)$. Thus the function $\Phi :=\frac{\underline{u}+\underline{u}_{ij} \zeta ^i \zeta ^j-M}{\sigma _{ij} \zeta ^i \zeta ^j}$ is smooth and bounded in $\Omega \cap B_{\delta }(x_1)$. By (3.12), for any $x\in \partial \Omega \cap B_{\delta }(x_1)$, we get

$$\begin{aligned} \underline{u}+\underline{u}_{ij} \zeta ^i \zeta ^j+\left( \nabla (u-\underline{u})\cdot \nabla \sigma \right) \sigma _{ij} \zeta ^i \zeta ^j\ge u+u_{11}\ge M, \end{aligned}$$

which implies that

$$\begin{aligned} \Phi +\nabla (u-\underline{u})\cdot \nabla \sigma \ge 0 \quad \text{ on }~\partial \Omega \cap B_{\delta }(x_1). \end{aligned}$$

(3.15)

Note that

$$\begin{aligned} \begin{aligned} L(\Phi +\nabla (u-\underline{u})\cdot \nabla \sigma )&=Q^{ij} \Phi _{ij} +(Q^s-\psi ^s) \Phi _s+Q^{ij}(\nabla u \cdot \nabla \sigma )_{ij}\\&\quad -Q^{ij}(\nabla \underline{u} \cdot \nabla \sigma )_{ij}\\&\quad +(Q^{s}-\psi ^s)(\nabla u \cdot \nabla \sigma )_{s}-(Q^{s}-\psi ^s)(\nabla \underline{u} \cdot \nabla \sigma )_{s}. \end{aligned}\nonumber \\ \end{aligned}$$

(3.16)

By Lemma 3.3, we have

$$\begin{aligned}{} & {} |Q^{ij} \Phi _{ij}| +|(Q^s-\psi ^s) \Phi _s|+|Q^{ij}(\nabla \underline{u} \cdot \nabla \sigma )_{ij}|\\{} & {} \quad +|(Q^{s}-\psi ^s)(\nabla \underline{u} \cdot \nabla \sigma )_{s}|\le K\left( 1+\sum _i Q^{ii}\right) . \end{aligned}$$

Moreover,

$$\begin{aligned} \begin{aligned}&Q^{ij}(\nabla u \cdot \nabla \sigma )_{ij}+ (Q^{s}-\psi ^s)(\nabla u \cdot \nabla \sigma )_{s}\\&\quad =Q^{ij} (u_{ijp} \sigma _p +u_p \sigma _{ijp}+ 2u_{ip}\sigma _{jp})+(Q^s-\psi ^s)(u_{sp} \sigma _p+u_p\sigma _{sp})\\&\quad =\sigma _p\left( Q^{ij} u_{ijp} +(Q^s-\psi ^s) u_{sp}\right) +u_p\left( Q^{ij} \sigma _{ijp}+(Q^s-\psi ^s) \sigma _{sp}\right) \\ {}&\qquad +2Q^{ij} u_{ip} \sigma _{jp}. \end{aligned}\qquad \end{aligned}$$

(3.17)

It is easy to show that

$$\begin{aligned} \left| u_p(Q^{ij} \sigma _{ijp}+(Q^s-\psi ^s) \sigma _{sp})\right| \le K \left( 1+\sum _i Q^{ii}\right) \end{aligned}$$

(3.18)

and

$$\begin{aligned} \begin{aligned} \left| Q^{ij} u_{ijp}+(Q^s-\psi ^s) u_{sp}\right| \le K \left( 1+\sum _i Q^{ii}\right) . \end{aligned} \end{aligned}$$

(3.19)

Note that $u_{pl}= \frac{w}{u} \gamma _{pi} a_{ij} \gamma _{jl}-u\delta _{pl}$, so

$$\begin{aligned} \begin{aligned} \left| Q^{ij} u_{ip} \sigma _{jp}\right|&=\left| Q^{ij} \left( \frac{w}{u} \gamma _{pl} a_{ls} \gamma _{si}-u\delta _{pi}\right) \sigma _{jp}\right| \\&\le K\left| G^{mn} (\delta _{rh}\delta _{mn}-\delta _{rm}\delta _{hn}) \gamma ^{ir} \gamma ^{hj} \gamma _{jl} a_{ls} \gamma _{si}\right| +K\sum _iQ^{ii}\\&\le K \left( 1+\sum _iQ^{ii}\right) . \end{aligned} \end{aligned}$$

(3.20)

Therefore using (3.16)-(3.20), we have

$$\begin{aligned} {\left\{ \begin{array}{ll} L(\Phi +\nabla (u-\underline{u})\cdot \nabla \sigma )\le K(1+\sum _i Q^{ii}),\quad {in}~\Omega \cap B_{\delta }(x_1),\\ \Phi +\nabla (u-\underline{u})\cdot \nabla \sigma \ge 0 ,\quad \quad \quad {on}~\partial \Omega \cap B_{\delta }(x_1). \end{array}\right. } \end{aligned}$$

By Lemma 3.4, we can choose constants $E>>B>>1$ such that

$$\begin{aligned} {\left\{ \begin{array}{ll} L(Ev+B\rho ^2+\Phi +\nabla (u-\underline{u})\cdot \nabla \sigma )\le 0,\quad {in}~\Omega \cap B_{\delta }(x_1),\\ Ev+B\rho ^2+\Phi +\nabla (u-\underline{u})\cdot \nabla \sigma \ge 0, \quad \quad {on}~\partial (\Omega \cap B_{\delta }(x_1)), \end{array}\right. } \end{aligned}$$

where v is defined as (3.2) and $\rho$ is the distance function to $x_1$. According to the maximum principle, we have

$$\begin{aligned} Ev+B\rho ^2+\Phi +\nabla (u-\underline{u})\cdot \nabla \sigma \ge 0 \quad \quad \text{ in }~\Omega \cap B_{\delta }(x_1). \end{aligned}$$

Note that $(Ev+B\rho ^2+\Phi +\nabla (u-\underline{u})\cdot \nabla \sigma )(x_1)=0$. Hence

$$\begin{aligned} Ev_n(x_1)+\Phi _n(x_1)-(u-\underline{u})_{nn}(x_1)+(u-\underline{u})_{n}(x_1) \sigma _{nn}(x_1)\ge 0, \end{aligned}$$

which implies that $u_{nn}(x_1) \le K$. Thus $|\nabla ^2u(x_1)|\le K$. We can prove (3.11) by a contradiction argument. Suppose

$$\begin{aligned} \max _{\eta \in T_x(\partial \Omega ), |\eta |=1} (u+ u_{\eta \eta }) (x_1) \le \frac{1}{N}\ \text {for}\ N\ \text { large enough}. \end{aligned}$$

Let $W=[u\delta _{ij}+u_{ij}]$ and assume $W_{ij}(x_1)=0, \text {for } i\ne j \text { and } 1 \le i,j\le n-1.$ Thus,

$$\begin{aligned} 0<W\le & {} \left( \begin{array}{cccc} \frac{1}{N}&{}\cdots &{}0&{}W_{1n}\\ \vdots &{} \ddots &{}&{}\vdots \\ 0&{} &{} \frac{1}{N}&{}W_{n-1,n}\\ W_{n1}&{}\cdots &{} W_{n,n-1}&{}W_{nn} \end{array} \right) . \end{aligned}$$

(3.21)

We have

$$\begin{aligned} T(W)\le & {} \left( \begin{array}{cccc} W_{nn}+\frac{n-2}{N}&{}\cdots &{}0&{}-W_{1n}\\ \vdots &{} \ddots &{}&{}\vdots \\ 0&{} &{}W_{nn}+\frac{n-2}{N}&{}-W_{n-1,n}\\ -W_{n1}&{} \cdots &{} -W_{n,n-1}&{}\frac{n-1}{N} \end{array} \right) :=[\bar{P}_{ij}]. \end{aligned}$$

It is then easily seen that

$$\begin{aligned} \lambda _{1}([\bar{P}_{ij}])=\cdots =\lambda _{n-2}([\bar{P}_{ij}])=W_{nn}+\frac{n-2}{N},\\ \lambda _{n-1}([\bar{P}_{ij}])\ge W_{nn}+\frac{n-2}{N},\ \ \lambda _{n}([\bar{P}_{ij}]) \ge 0.\end{aligned}$$

It follows that

$$\begin{aligned} \psi= & {} \frac{\sigma _n}{\sigma _l}^{\frac{1}{n-l}}\left( \sigma _1(\kappa )-\kappa _1, \sigma _1(\kappa )-\kappa _2, \cdots , \sigma _1(\kappa )-\kappa _n\right) \\= & {} G(T (\frac{u}{w} \gamma W \gamma )) \le K G(T (W)) \le K \frac{\sigma _n}{\sigma _l}^{\frac{1}{n-l}}\left( \lambda [\bar{P}_{ij}]\right) \\\le & {} K\left( (W_{nn}+\frac{n-2}{N})^{n-1-l} \frac{n-1}{N}\right) ^{\frac{1}{n-l}}\\< & {} \psi . \end{aligned}$$

This contradiction implies that (3.11) holds.

Note that $a_{ij}(x)= \frac{u}{w} \gamma ^{ik} (u\delta _{kl}+u_{kl}) \gamma ^{lj}$. For any $x\in \partial \Omega$, the estimates of u, $u_i$, $u_{\alpha \beta }$, $u_{n\alpha }$ imply that $\kappa '_{\alpha }$, the eigenvalues of $[a_{\alpha \beta }]_{1\le \alpha , \beta \le n-1}$, have both a positive upper and lower bound, i.e.,

$$\begin{aligned} \frac{1}{K}\le \kappa '_{\alpha }\le K, \quad \alpha =1,\cdots , n-1.\end{aligned}$$

(3.22)

Suppose that $a_{nn}$ tends to infinity. According to the well known Cauchy interlacing inequalities (see [41], p. 103-104), we get

$$\begin{aligned} 0\le \kappa _1\le \kappa '_1\le \kappa _2\le \cdots \le \kappa _{n-1}\le \kappa '_{n-1}\le \kappa _n. \end{aligned}$$

(3.23)

Combining (3.22) and (3.23), it yields

$$\begin{aligned} \begin{aligned} \psi&=\frac{\sigma _n}{\sigma _l}^{\frac{1}{n-l}}\left( \sigma _1(\kappa )-\kappa _1, \sigma _1(\kappa )-\kappa _2, \cdots , \sigma _1(\kappa )-\kappa _n\right) \\&\ge \Big (\frac{\sigma _n}{\sigma _l}\Big (\kappa _n, \cdots , \kappa _n, \frac{n-2}{K}\Big )\Big )^{\frac{1}{n-l}}\\&\ge \Big (K\kappa ^{n-1-l}_{n} \Big )^{\frac{1}{n-l}}>\psi ,\end{aligned} \end{aligned}$$

which is a contradiction. Therefore $a_{nn}\le K$ and thus $u_{nn}\le K$ as required. $\square$

Proof of Theorem 1.2

On account of Theorem 3.1, Theorem 3.2 and Theorem 3.5, Theorem 1.2 is proved. $\square$

4 Existence

The convexity of solutions is a very important prerequisite to perform the continuity process. In order to keep the convexity of solutions, we introduce the following definition.

Definition 4.1

Let $u: \overline{\Omega } \subset \mathbb {S}^n \rightarrow \mathbb {R}$ be a positive smooth function, we say u is spherical convex if

$$\begin{aligned} \left( u_{ij}+u \delta _{ij} \right) \ge 0, \quad \forall ~x\in \overline{\Omega }. \end{aligned}$$

Then we give a constant rank theorem.

Theorem 4.2

Let $\theta : \overline{\Omega } \subset \mathbb {S}^n \rightarrow \mathbb {R}$ be a given negative smooth function. Suppose that $u\in C^4(\Omega )\cap C^2(\overline{\Omega })$ is a solution of equation

$$\begin{aligned} -\left( \frac{\sigma _k}{\sigma _l}\right) ^{-\frac{1}{k-l}}(\lambda [b_{ij}])=\theta (x) \frac{1}{u^p} \end{aligned}$$

(4.1)

satisfying that $A=[a_{ij}]$ is positive semi-definite in ${\Omega }$, where $p\ge 0$, $a_{ij}$ are defined as in (2.4) and $[b_{ij}]=T(A)=(\text{ trace } A)I-A$. If $(-\theta )^{\frac{1}{1+p}}$ is spherical convex, then A is of constant rank in ${\Omega }$.

Proof

See Theorem 3.1 in [4]. $\square$

When the right hand side of the prescribed curvature equation depends on X and $\nu$, we establish the following version of constant rank theorem.

Theorem 4.3

Let $\Sigma$ be a $C^4$ oriented connected compact hypersurface in $\mathbb {R}^{n+1}$ satisfying the prescribed curvature equation

$$\begin{aligned} -\left( \frac{\sigma _k}{\sigma _l}\right) ^{-\frac{1}{k-l}}(\eta )=\theta (X, \nu ), \end{aligned}$$

(4.2)

where $\lambda [\eta ]=\sum _{j=1}^n \kappa _j - \kappa _i$. Suppose $\theta (X, y)$ is locally concave in X variable for any $y\in \mathbb {S}^n$. Then the second fundamental form of $\Sigma$ is of constant rank.

Proof

We set $\widetilde{Q}([a_{ij}])=-\left[ \frac{\sigma _k(\lambda (T[a_{ij}]))}{\sigma _l(\lambda (T[a_{ij}]))}\right] ^{-\frac{1}{k-l}}$, $\widetilde{\theta }= \theta (X, \nu (X))$, equation (4.2) can be rewritten in the following form

$$\begin{aligned} \widetilde{Q}(A)=\widetilde{\theta } \quad \text {in}\ \Omega . \end{aligned}$$

(4.3)

We also set $\widetilde{Q}^{ij}=\frac{\partial \widetilde{Q}}{\partial a_{ij}},\quad \widetilde{Q}^{ij,pq}=\frac{\partial ^2\widetilde{Q}}{\partial a_{ij}\partial a_{pq}}.$ Consider the test function

$$\begin{aligned} \phi (x)=\sigma _{r+1}(A)+\frac{\sigma _{r+2}(A)}{\sigma _{r+1}(A)}, \end{aligned}$$

(4.4)

with the convention that $\sigma _j(A)=0$ if $j<0$ or $j>n$. Note that the test function $\phi$ was first introduced by Bian-Guan in [1], they showed that $\phi$ is $C^{1,1}$ and hence can be tested via defferentiation.

We say that $h(y)\lesssim f(y)$ provided there exist positive constants $c_1$ and $c_2$ such that

$$\begin{aligned} (h-f)(y)\le \left( c_1|\nabla \phi |+c_2\phi \right) (y), \end{aligned}$$

and we write $h(y)\sim f(y)$ if $h(y)\lesssim f(y)$ and $f(y)\lesssim h(y)$. We denote the eigenvalues of A by $\lambda$ where $\lambda _n\ge \cdots \ge \lambda _1$. Let $B=\{1,\cdots ,n-r\}$ and $G=\{n-r+1,\cdots ,n\}$ denote the sets of indices for eigenvalues $\lambda _i$, i.e., $B=\{\lambda _1,\cdots ,\lambda _{n-r}\}$, $G=\{\lambda _{n-r+1},\cdots ,\lambda _{n}\}$. As in [22], for any $i \in B$, using Gauss formula and Weingarten formula, it follows that

$$\begin{aligned} \sum _{i\in B} \widetilde{\theta }_{ii}= & {} \sum _{i\in B} d_X \theta (\nabla _{ii} X)+d^2_X \theta (\nabla _i X, \nabla _i X)+2d_X d_{\nu } \theta (\nabla _i X, \nabla _i \nu )\\{} & {} + d^2_{\nu } \theta (\nabla _i \nu , \nabla _i \nu )+ d^2_{\nu } \theta (\nabla _{ii} \nu )\\\sim & {} d^2_X \theta (\nabla _i X, \nabla _i X)\\\lesssim & {} 0.\end{aligned}$$

Therefore, an argument similar to that in [4] can deduce that

$$\begin{aligned} \sum _{\alpha ,\beta }\widetilde{Q}^{\alpha \beta }\phi _{\alpha \beta }\lesssim & {} \sum _{i\in B}\left[ \sigma _r(G)+\frac{\sigma _1^2(B|i)-\sigma _2(B|i)}{\sigma _1^2(B)}\right] \widetilde{\theta }_{ii} \lesssim 0. \end{aligned}$$

Then by the strong maximum principle, $\phi \equiv 0$ and A is of constant rank r. $\square$

Now we may assume $s\in (1, 2)$ is a fixed small constant such that

$$\begin{aligned} \left( \frac{t}{\varepsilon }+\frac{(1-t)\underline{u}^s }{\underline{\psi }} \right) ^{\frac{1}{s+1}} \quad \quad \text{ is } \text{ spherical } \text{ convex } \text{ for }~t\in [0,1], \end{aligned}$$

(4.5)

where $\varepsilon$ is a fixed small positive constant such that

$$\begin{aligned} \underline{\psi }(x):=Q(\nabla ^2\underline{u}, \nabla \underline{u}, \underline{u})>\psi (x, \underline{u}, \nabla \underline{u})+\varepsilon \sup \underline{u}\quad \quad \text{ in }~\overline{\Omega }. \end{aligned}$$

(4.6)

In fact, note that Assumption 1.4 implies $\left( \ln \frac{\underline{u}}{\underline{\psi }}\right) _{ij} >0.$ It follows

$$\begin{aligned}{} & {} \lim _{s\rightarrow 1} \Big [\Big (\frac{t}{\varepsilon }+ \frac{(1-t)\underline{u}^s}{\underline{\psi }}\Big )^{\frac{1}{s+1}}\Big ]_{ij} + \Big [\frac{t}{\varepsilon }+ \frac{(1-t)\underline{u}^s}{\underline{\psi }} \Big ]^{\frac{1}{s+1}} \delta _{ij}\\{} & {} \quad \ge \Big [\Big (\frac{t}{\varepsilon }+ \frac{(1-t)\underline{u}}{\underline{\psi }}\Big )^{\frac{1}{2}}\Big ]_{ij} +\Big [\frac{t}{\varepsilon }+\frac{(1-t) \underline{u}}{\underline{\psi }} \Big ]^{\frac{1}{2}} \delta _{ij}\\{} & {} \quad \ge \Big (\frac{t}{\varepsilon }+ \frac{(1-t)\underline{u}}{\underline{\psi }} \Big )^{-\frac{3}{2}} \left\{ (1-t)^2\Big [ \frac{1}{4}{\left( \frac{\underline{u}}{\underline{\psi }}\right) ^{2}}\left( \ln \frac{\underline{u}}{\underline{\psi }}\right) _{ij} \Big ]+\frac{t^2}{\varepsilon ^2} \delta _{ij}\right\} \\{} & {} \quad >0, \end{aligned}$$

where in the second inequality we use $\left( \ln \frac{\underline{u}}{\underline{\psi }}\right) _{ij} >0$ and $\frac{\underline{u}}{\underline{\psi }} >0$. Thus there exists $s\in (1, 2)$ such that (4.5) holds. Moreover, let a satisfy

$$\begin{aligned} \frac{ \min \left\{ \varepsilon \underline{u}^s,\ \underline{\psi }, \ \inf \psi \right\} }{n-1} \big (\frac{C_n^l}{C_n^k}\big )^{\frac{1}{k-l}}\ge a. \end{aligned}$$

(4.7)

Then for each $t\in [0,1]$,

$$\begin{aligned}{} & {} \frac{n-1}{n}\left( \frac{C^k_n}{C^l_n}\right) ^{\frac{1}{k-l}} {\sigma _1} (a,\cdots ,a) \nonumber \\{} & {} \quad \le \min \left\{ \left( \frac{t}{\varepsilon }+ \frac{{(1-t)\underline{u}^s}}{\underline{\psi }} \right) ^{-1}\underline{u}^{s},\ \left( \frac{t}{\inf \psi }+\frac{(1-t)}{\varepsilon \underline{u}^{s}} \right) ^{-1} \right\} . \end{aligned}$$

(4.8)

We then apply the classical continuity method and degree theory developed by Li [30] to establish the existence of solution of the Dirichlet problem (1.8). The proof here is similar to [33] and [34], thus only sketch will be given below. Set $v=\ln u$ and

$$\begin{aligned} {[}a_{ij}]= & {} \left[ \frac{e^v}{\sqrt{1+|\nabla v|^2}} \tilde{\gamma }^{ik} (\delta _{kl}+v_k v_l+ v_{kl})\tilde{\gamma }^{lj}\right] \\:= & {} A[v], \end{aligned}$$

moreover

$$\begin{aligned} -A[v]+(\text{ trace }A[v])I:=A^1[v], \quad F(\nabla ^2v, \nabla v, v):=G(A^1[v]), \end{aligned}$$

where

$$\begin{aligned} \tilde{\gamma }^{ik}=\delta _{ik}- \frac{v_i v_k}{(\sqrt{1+|\nabla v|^2}+1)\sqrt{1+|\nabla v|^2}}. \end{aligned}$$

Then equation (1.8) reduces to

$$\begin{aligned} \left\{ \begin{aligned}&F(\nabla ^2v, \nabla v, v)=\psi (x, v, \nabla v),{} & {} \quad {in}~ \Omega ,\\&v=\ln a,{} & {} \quad {on}~ \partial \Omega . \end{aligned} \right. \end{aligned}$$

(4.9)

We set $\underline{v}=\ln \underline{u}$, then equation (2.7) of the subsolution becomes

$$\begin{aligned} \left\{ \begin{aligned}&F(\nabla ^2\underline{v}, \nabla \underline{v}, \underline{v})=\underline{\psi }(x)>\psi (x, \underline{v}, \nabla \underline{v} ) ,{} & {} \quad {in}~ \Omega ,\\&\underline{v}= \ln a,{} & {} \quad {on}~ \partial \Omega . \end{aligned} \right. \end{aligned}$$

(4.10)

Consider the following two auxiliary equations:

$$\begin{aligned} \left\{ \begin{aligned}&F(\nabla ^2v, \nabla v, v)=\left( \frac{t}{\varepsilon }+(1-t) \frac{e^{s\underline{v}}}{\underline{\psi }} \right) ^{-1} e^{sv},{} & {} \quad {in}~ \Omega ,\\&v=\underline{v},{} & {} \quad {on}~ \partial \Omega , \end{aligned} \right. \end{aligned}$$

(4.11)

and

$$\begin{aligned} \left\{ \begin{aligned}&F(\nabla ^2v, \nabla v, v)=\left( \frac{t}{\psi (x, v, \nabla v)}+ \frac{(1-t)}{\varepsilon e^{sv} } \right) ^{-1},{} & {} \quad {in}~ \Omega ,\\&v=\underline{v},{} & {} \quad {on}~ \partial \Omega , \end{aligned} \right. \end{aligned}$$

(4.12)

where $t\in [0,1]$.

Lemma 4.4

Let v be a strictly locally solution of $F(\nabla ^2v, \nabla v, v)=\psi$, then

$$\begin{aligned}F_v:= \frac{\partial F}{ \partial v}= \psi .\end{aligned}$$

Proof

One can easily check that

$$\begin{aligned} F_v=G^{st}(\delta _{ij}\delta _{st}-\delta _{is}\delta _{jt}) a_{ij}=G^{st} \left( \left( \sum _l a_{ll} \right) \delta _{st}-a_{st}\right) =\psi . \end{aligned}$$

$\square$

Lemma 4.5

For any $t\in [0,1]$, the Dirichlet problem (4.11) has at most one strictly locally convex solution v with $v\ge \underline{v}$.

Proof

The proof is similar to the proof of Lemma 4.2 in [33] and Lemma 5.6 in [34], so we omit it. $\square$

Theorem 4.6

Under the assumptions in Theorem 1.5, for any $t\in [0, 1]$, (4.11) has a unique strictly locally convex solution.

Proof

Let

$$\begin{aligned} L: C_0^{2,\alpha }\times [0,1]\rightarrow & {} C^{\alpha }\\ (w,t)\mapsto & {} L(w,t) \end{aligned}$$

where

$$\begin{aligned} L(w,t)=F(\nabla ^2 (w+\underline{v}), \nabla (w+\underline{v}), (w+\underline{v}))-\left( \frac{t}{\varepsilon }+(1-t) \frac{e^{s\underline{v}}}{\underline{\psi }} \right) ^{-1} e^{s(w+\underline{v})}. \end{aligned}$$

We define

$$\begin{aligned} \mathcal {S}=\{t\in [0,1]|L(w,t)=0 \text { has a solution }w \text { with } A[w+\underline{v}]>0\ \text{ in }~ \Omega \}. \end{aligned}$$

As in Theorem 4.4 in [33], we know that $\mathcal {S}$ is open in [0, 1]. It suffices to show that $\mathcal {S}$ is closed in [0, 1]. Suppose that there exist a constant $t_0 \in [0, 1]$ and sequences $\{t_j\}_{j=1}^{\infty }, \{w_j\}_{j=1}^{\infty }$ such that

$$\begin{aligned} t_j\rightarrow t_0 \text { and } L(w_j, t_j)=0.\end{aligned}$$

Due to Lemma 4.5 and $C^2$ estimates established in Sect. 3, we have $w_j\ge 0$ and $v_j=w_j+ \underline{v}$ is a bounded sequence in $C^{2, \alpha }$. Therefore there exists a solution $v_0 =w+\underline{v}$ of equation

$$\begin{aligned} \left\{ \begin{aligned}&-\left( \frac{\sigma _k}{\sigma _l}\right) ^{-\frac{1}{k-l}} \left( \lambda \left( A^1[v_0] \right) \right) =-\left( \frac{t}{\varepsilon }+(1-t) \frac{e^{s\underline{v}}}{\underline{\psi }} \right) e^{-sv_0},{} & {} \quad {in}~ \Omega ,\\&v_0=\ln a,{} & {} \quad {on}~ \partial \Omega , \end{aligned} \right. \end{aligned}$$

with $||w_{j_k}- w||_{C^{2, \alpha } }\rightarrow 0$ and $A[v_0]\ge 0$. Suppose the minimum of $v_0$ is taken at $p_0$. We shall consider two cases.

Case A: $p_0\in \partial \Omega$.

Let $\overline{v}\equiv \ln a$. Then (4.8) implies

$$\begin{aligned} {\sigma _1} \left( \lambda \left( A^1[\overline{v}] \right) \right) \le {\sigma _1} \left( \lambda \left( A^1[{v}_0] \right) \right) . \end{aligned}$$

(4.13)

Then by the comparison principle we have

$$\begin{aligned} v_0\le {\overline{v}}. \end{aligned}$$

(4.14)

Furthermore, we have

$$\begin{aligned} \ln a=v_0(p_0)\le v_0\le \overline{v}= \ln a. \end{aligned}$$

Then we have $A[v_0]>0$.

Case B: $p_0$ is an interior point of $\Omega$.

In this case, by Theorem 4.2 we arrive $A[v_0](p_0)>0$ and hence $A[v_0]>0$ as well. Thus $t_0 \in \mathcal {S}$. Moreover, $\mathcal {S}$ is closed and $\mathcal {S}=[0, 1]$. $\square$

Lemma 4.7

Let $v\ge \underline{v}$ be a strictly locally convex solution of (4.12), then

$$\begin{aligned} v> \underline{v},\quad \quad \big (\textbf{n}\cdot \nabla (v-\underline{v})\big )\Big |_{\partial \Omega }>0, \end{aligned}$$

where $\textbf{n}$ is the unit inner normal of $\partial \Omega$.

Proof

The proof follows by a simple modification of Theorem 5.12 in [34] and is omitted. $\square$

Lemma 4.8

Under the assumptions of Theorem 1.5, for any $t \in [0, 1]$, the Dirichlet problem (4.12) has a strictly locally convex solution v with $v \ge \underline{v}$ in $\Omega$.

Proof

Due to $C^2$ estimates established in Sect. 3, we can apply Evans-Krylov estimates [9, 13] and the classical Schauder theory [13] to obtain

$$\begin{aligned} ||v||_{C^{4, \alpha } }< K \end{aligned}$$

(4.15)

for strictly locally convex solutions of (4.12) with $v\ge \underline{v}$. Note that K is independent of t. Let us consider a bounded open subset

$$\begin{aligned} \mathcal {O}:= \left\{ w\in C_0^{4, \alpha } \bigg | \begin{array}{c} w> 0\quad \text{ in }~\Omega ,\quad \quad \textbf{n}\cdot \nabla w>0\quad \text{ on }~ \partial \Omega , \\ ||w||_{C^{4, \alpha }}< 2K +||\underline{v}||_{C^{4, \alpha }}, \\ A[w+\underline{v}]>0\quad \text{ in }~ \Omega , \end{array} \right\} \end{aligned}$$

and construct a map $M_t[w]: \mathcal {O} \times [0, 1] \longrightarrow C^{2, \alpha }$,

$$\begin{aligned} M_t[w]= & {} F(\nabla ^2 (w+\underline{v}), \nabla (w+\underline{v}), (w+\underline{v}))\\{} & {} -\left( t\psi ^{-1}(x, w+\underline{v}, \nabla (w+\underline{v}))+(1-t) \varepsilon ^{-1} e^{-s(w+\underline{v})} \right) ^{-1}. \end{aligned}$$

Let $v^0$ be the solution of (4.11) at $t=1$, which is unique by Lemma 4.5. We set $w^0:=v^0-\underline{v}$. To proceed further, we need the following Claim.

Claim $M_t[w]=0$ has no solution on $\partial \mathcal {O}$.

In fact, suppose $w_0 \in C_0^{4, \alpha }$ with $M_{t_0}[w_0]=0$, $t_0\in [0, 1]$ and $A[w_0+\underline{v}]\ge 0$. Let $v_0=w_0+\underline{v}$ and $u_0= e^{v_0}$. Then $u_0$ satisfies equation

$$\begin{aligned} \left\{ \begin{aligned}&-\left( \frac{\sigma _k}{\sigma _l}\right) ^{-\frac{1}{k-l}} \left( \lambda [b_{ij}]\right) =- \frac{t}{\psi (X, \nu )}- \frac{(1-t)}{\varepsilon u_0^{s} } ,{} & {} \quad {in}~ \Omega ,\\&u_0= a,{} & {} \quad {on}~ \partial \Omega . \end{aligned} \right. \end{aligned}$$

Recall $\overline{v}\equiv \ln a$, then (4.8) implies

$$\begin{aligned} {\sigma _1} \left( \lambda \left( A^1[\overline{v}] \right) \right) \le {\sigma _1} \left( \lambda \left( A^1[{v}_0] \right) \right) . \end{aligned}$$

(4.16)

Hence by the comparison principle we get

$$\begin{aligned} v_0\le {\overline{v}}. \end{aligned}$$

(4.17)

If $u_0$’s minimum is taken at a boundary point $p_0$, then

$$\begin{aligned} \ln a=v_0(p_0)\le v_0\le \overline{v}= \ln a \end{aligned}$$

and thus $A[v_0]>0$. If the minimum of $u_0$ is taken at an interior point of $\Omega$, then by Theorem 4.3 we have $A[w_0+\underline{v}]>0$ as well. Now using Lemma 4.7 and (4.15), we know that $M_t[w]=0$ has no solution on $\partial \mathcal {O}$.

Therefore, the degree $deg(M_t, \mathcal {O}, 0)$ is well defined for $0 \le t \le 1$. Using the homotopic invariance of the degree [30], we have

$$\begin{aligned} deg(M_0, \mathcal {O}, 0) = deg (M_t, \mathcal {O}, 0). \end{aligned}$$

So we only need to compute $deg(M_0, \mathcal {O}, 0)$. Note that $M_0[w]=0$ has a unique solution $w^0$ in $\mathcal {O}$. The Fr$\acute{e}$chet derivative of $M_{0, w^0}: C^{4, \alpha } \longrightarrow C^{2, \alpha }$ is a linear elliptic operator,

$$\begin{aligned} M_{0, w^0}(h)= F^{ij}\mid _{v^0} h_{ij} +F^i\mid _{v^0} h_i + (F_v\mid _{v^0}- s\varepsilon e^{sv^0})h. \end{aligned}$$

By Lemma 4.4,

$$\begin{aligned} F_v\mid _{v^0}- s\varepsilon e^{sv^0}<0. \end{aligned}$$

So $M_{0, w^0}$ is invertible. Therefore, by the theory in [30],

$$\begin{aligned} deg(M_0, \mathcal {O}, 0) = deg (M_{0, w^0}, B_1, 0)= \pm 1 \ne 0, \end{aligned}$$

where $B_1$ is the unit ball in $C^{4, \alpha }_0$. Thus

$$\begin{aligned} deg(M_t, \mathcal {O}, 0) \ne 0 \end{aligned}$$

for any $t\in [0, 1]$. Hence (4.12) has a strictly locally convex solution for any $t\in [0, 1]$. $\square$

Proof of the Theorem 1.5

The existence result follows from Lemma 4.8. $\square$