The Astrophysical S-factor for the ²H(α, γ) ⁶Li Nuclear Reaction at Low-Energies

Abstract

The alpha radiative capture reactions are the key to understand about primordial nucleosynthesis and the observed abundance of light nucleus in stars. The astrophysical S-factor for the process ²H(α, γ) ⁶Li has been calculated at the low-energies relevant to big-bang nucleosynthesis and in comparison with laboratory data. On the basis of the model, the alpha radiative capture process is studied by using the two- and three-body electromagnetic currents. The bound and resonance states of ⁶Li are calculated via an inverse process, deuteron- α photodisintegration of a ⁶Li nucleus. In comparison with other theoretical approaches and available laboratory data, excellent agreement is achieved for the astrophysical S-factor of this process.

1 Introduction

The observations of ⁶Li in several old metal-poor stars, inside the halo of the galaxy, have been reported in previous studies. The observations exceed the predictions of the Standard Big-Bang Nucleosynthesis model by a factor of 500. In the relevant energy range, no directly measured S-factors were available yet for the main production reaction ²H(α, γ) ⁶Li, while different theoretical estimations have an uncertainty of up to two orders of magnitude. In the standard primordial nucleosynthesis models, the ²H(α, γ) ⁶Li nuclear reaction is the only process by which ⁶Li nucleus is produced (Nollett et al. 1997). No measurable amount of ⁶Li nucleus can be obtained in big-bang nucleosynthesis (BBN) without recourse to exotic scenarios, as the cross-section of this reaction is very small. Therefore, it has never been measured experimentally at such low-energies, and theoretical predictions remain uncertain (Marcucci et al. 2006; Hammache et al. 2010; Mukhamedzhanov et al. 2011).

In experimental studies, few attempts to determine the cross-section for the radiative capture of deuterons on alpha particles have been made using the Coulomb dissociation technique, in which an energetic ⁶Li beam is shot on a target of high nuclear charge. The time-reversed reaction is then studied using virtual photons (Blatt & Weisskopf 1991). In 1991, Kiener et al. bombarded a lead target with a 156 MeV ⁶Li beam and calculated a surprisingly constant S-factor below the resonance. A re-analysis by Hammache et al. (2010) found that these results are affected by highly dominating nuclear processes and have to be regarded as upper limits. This method is specially sensitive to quadrupole E ₂ transitions in the excited ⁶Li nucleus, largely neglecting the electrical (E ₁) and magnetic (M ₁) contributions of dipole transitions. It is also limited by possible background from non-Coulomb, i.e. nuclear breakup (Baur & Rebel 1996). More recently, The LUNA collaborations reported on neutron-induced effects in a high-purity germanium detector that were encountered in a new study of this reaction (Anders et al. 2014). In their experiment, an alpha-beam from the underground accelerator LUNA in Gran Sasso, Italy, and a windowless deuterium gas target are used.

Electroweak processes in light nuclei are interesting from the point of view of few-body nuclear physics. The advent of realistic few-nucleon potentials have enabled calculation of nuclear wave functions and energy observables of low mass nuclei (Carlson & Schiavilla 1998). An accurate solution for a three-body bound state system can now be obtained (Thompson et al. 2004). However, there are still difficulties persisting for the charged particles scattering problem as no standard boundary condition for this system exists when considering the long-range effects of the Coulomb interaction. Certain efforts have been made to solve this problem but the results are limited to cases where only one pair of particles have charge (Kievsky et al. 1997; Deltuva et al. 2005; Nguyen et al. 2012, 2013).

Recently, the cross-section for the radiative capture of deuterons on alpha particles have been computed at the low-energies (Nollett et al. 2001). They considered the final state as a six-body wave function generated by the variational Monte Carlo method from the AV ₁₈ and Urbana IX potentials. They showed that E ₂ contribution of cross-section is dominant and E ₁ contribution is larger than the measured contribution at 2 MeV by a factor of seven. They also calculated explicitly the impulse-approximation of M ₁ contribution, and found to be very small. The cross-section for the process is very small and the amount that could be obtained in big bang nucleosynthesis is extremely small.

But since ⁶Li is sensitive to destruction in stars more than ⁷Li, the amount of destruction of ⁶Li has been used to set limits on the depletion of ⁷Li in halo stars. The abundance of lithium in the atmosphere of stars is inferred from the spectral analysis of their emitted light. Every element leaves a characteristic signature of absorption lines in the spectrum. To reliably understand the relation between their shape and the corresponding abundance, sufficient knowledge about parameters like distance, space motion and the galactic orbit of the star, and atmosphere parameters (radial velocity, effective temperature, gravity and relative metal deficiency [Fe/H]) are necessary. Abundance of ⁷Li is about a factor of three lower than what is predicted as primordial value in big-bang nucleosynthesis (Ryan et al. 2000; Melendez & Ramirez 2004; Rollinde et al. 2005), while ⁶Li abundance is about 1/20 of ⁷Li, even though standard big bang would produce at the most three orders of magnitude lesser amounts of ⁶Li compared to what is detected (Kusukabe et al. 2006). Asplund et al. (2006) analysed high-quality spectra of 24 metal-poor halo dwarfs and subgiants, with signal-to-noise-ratios being typically more than ten times higher than that was available in 1982, with the possibility to infer ⁶Li/ ⁷Li abundance ratios. The high ⁶Li abundance during the early galactic epochs (corresponding to very low metallicity) was difficult to achieve by galactic cosmic-ray spallation and fusion reactions involving alphas. More recently, a contribution to the discussion about the accuracy of the recent ⁶Li observations, and to the question if it is necessary to include new physics into the standard big-bang nucleosynthesis model, has been presented by Anders et al. (2014).

In this work, we study the three-body model for the deuteron–alpha radiative capture within a alpha cluster model inclusive of the two- and three-body conserved currents. In the previous works, the one-, two-, three- and four-alpha radiative capture processes were calculated at stellar energies (Sadeghi 2013; Sadeghi et al. 2014; Sadeghi & Mosavi-Khonsari 2015). We have also presented the three- and four-body type calculations for two- and three-body current conservation interactions to cluster nuclear reactions. We have shown that the consideration of few-body interactions along with two- and three-body allows one to improve description of the laboratory observables.

This paper is organized as follows. In section 2, we describe briefly the Faddeev based theory, interactions between nucleons, deuteron and alpha particles, two- and three-body current conservation operators, the matrix elements for radiative capture and photodisintegration processes. We tabulate the calculated cross-section and the astrophysical S-factor and compare the results with the corresponding available laboratory data and other theoretical findings in section 3. Summary and conclusions follow in section 4.

2 Theoretical framework

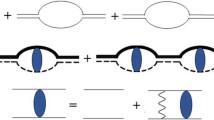
The nuclear electromagnetic operators for charge ρ(q), and current, j(q), are given by integrals of many-body terms that operate on the nucleon degrees of freedom

$$\begin{array}{@{}rcl@{}} \rho(\mathbf{q})&=&\sum\limits_{i} \rho_{i}(\mathbf{q})+\sum\limits_{i<j} \rho_{ij}(\mathbf{q})+\sum\limits_{i<j<k} \rho_{ijk}(\mathbf{q})+ \cdots\ , \\ \mathbf{j}(\mathbf{q})&=&\sum\limits_{i} \mathbf{j}_{i}(\mathbf{q})+\sum\limits_{i<j} \mathbf{j}_{ij}(\mathbf{q})+\sum\limits_{i<j<k} \mathbf{j}_{ijk}(\mathbf{q})+ \cdots \ , \end{array} $$

(1)

where the one-body nuclear electromagnetic operators ρ _i (q) and j _i (q) are derived from the non-relativistic reduction of the covariant single-nucleon current, by expanding in powers of 1/m, m being the nucleon mass. The one-body current conservation form of the electromagnetic current operator at lowest order in 1/m is given by (Carlson & Schiavilla 1998; Sadeghi et al. 2014)

$$ \mathbf{q}\cdot\mathbf{j}_{i}(\mathbf{q})= \left[{{\mathbf{p}_{i}^{2}}\over 2m}, \rho_{i,\text{NR}}(\mathbf{q})\right]. $$

(2)

The two- and three-body current density operators in its Fourier transform by retaining only linear terms in the vector potential is given by (Marcucci et al. 1998)

$$\begin{array}{@{}rcl@{}} v_{ij} &\equiv& v^{0}_{ij} -\int \mathbf{j}_{ij}({\mathbf{x}})\cdot\mathbf{A}({\mathbf{x}}) \textnormal{d}{\mathbf{x}} \ , \\ \mathbf{j}_{ij}(\mathbf{q}) &=&\mathrm{i}\,v_{2,ij}\bigg(\epsilon_{i}{\int}_{\gamma_{ij}}\textnormal{d}\mathbf{s}\,\mathrm{e}^{\mathrm{i}\mathbf{q}\cdot\mathbf{s}} +\epsilon_{j}{\int}_{\gamma^{\prime}_{ji}}\textnormal{d}\mathbf{s}^{\prime}\,\mathrm{e}^{\mathrm{i}\mathbf{q}\cdot\mathbf{s}^{\prime}}\bigg) \,(1+\mathbf{\tau}_{i}\cdot\mathbf{\tau}_{j}) \ . \end{array} $$

$$\begin{array}{@{}rcl@{}} \mathbf{j}_{j;ki}(\mathbf{q})&=&2A_{2\pi}{G_{E}^{V}}(q_{\mu}^{2}) \{X_{ij},\,X_{jk}\}\,(\mathbf{\tau}_{i}\times\mathbf{\tau}_{k})_{z} \,{\int}_{\gamma_{ik}} \mathrm{d}\mathbf{s}\, \mathrm{e}^{\mathrm{i}\mathbf{q}\cdot\mathbf{s}} \\ &&+ \frac{\mathrm{i}}{4}\, A_{2\pi}{G_{E}^{V}}(q_{\mu}^{2}) [X_{ij},\,X_{jk}] \bigg [ (\tau_{i,z}\, \mathbf{\tau}_{j}\cdot\mathbf{\tau}_{k}- \tau_{j,z}\, \mathbf{\tau}_{i}\cdot\mathbf{\tau}_{k}) {\int}_{\beta_{jk}} \mathrm{d}\mathbf{s}\, \mathrm{e}^{\mathrm{i}\mathbf{q}\cdot\mathbf{s}} \\ && + (\tau_{k,z}\, \mathbf{\tau}_{i}\cdot\mathbf{\tau}_{j}- \tau_{j,z}\, \mathbf{\tau}_{i}\cdot\mathbf{\tau}_{k}) {\int}_{\beta_{ij}^{\prime}} \mathrm{d}\mathbf{s}\, \mathrm{e}^{\mathrm{i}\mathbf{q}\cdot\mathbf{s}} \bigg ] \ , \end{array} $$

(3)

where $v^{0}_{ij}$, j _{i j} , j _{j;k i} and A(x) are the isospin-conserving momentum-independent part of the potential, the two-body conserved current, the three-body conserved current and the vector potential, respectively. τ _i and τ _j are the isospin Pauli matrices, and v ₂ is in general functions of the positions and spin operators of the two nucleons that includes the long-range one-pion-exchange component. ${G_{E}^{V}}(q_{\mu }^{2})$ is the isovector combinations of the nucleon electric Sachs form factors and {…} ([…]) denotes the anticommutator (commutator). The parameter A _2π is determined by the ⁶Li binding energy in a Green’s function Monte Carlo calculation.

All of the potentials used contain both central and spin–orbit terms, and the spin–orbit force in the even partial waves is well-constrained by the spacing of the D-wave scattering resonances. While a tensor component could in principle be derived to reproduce the ground-state quadrupole moment, its effect on the scattering data is too small to support its derivation from the scattering phase shifts. At these very low-energies the error estimation derived from studying the changes due to the variation of the free parameters is very small in comparison to the potential model calculation. The most important difference between the used potential and other applied potentials, which give very similar cross-sections in the calculations, is the fitting of Av18 + UIX potential to the phase shifts, to produce the expected parity-dependent potential and long-range behaviour of the operators, with normalization fitted to the Monte Carlo results.

The time reversed photo dissociation cross-section ⁶Li(γ, α) ²He can be expanded into electric and magnetic multipoles. In particular, the electric multipole contribution of order λ has the form (de Diego et al. 2010)

$$ \sigma^{(\lambda)}_{\gamma}(E_{\gamma})=\frac{(2 \pi)^{3} (\lambda+1)}{\lambda [(2 \lambda+1)!!]^{2}} \left(\frac{E_{\gamma}}{\hbar c}\right)^{2 \lambda-1} \frac{\mathrm{d}\mathcal{B}}{\textnormal{d}E} \;, $$

(4)

the strength function $\mathcal {B}$ being

$$ \mathcal{B}(E\lambda,J_{0} \rightarrow J) = \sum\limits_{\mu M} |\langle JM|\mathcal{O}_{E\lambda\mu}|J_{0}M_{0}\rangle|^{2}, $$

(5)

where J ₀, J and M ₀, M are the total angular momenta and their projections of the initial and final states, and all other quantum numbers are collected into n ₀ and n.

The matrix element for the transition J ₀ M ₀→J M is given by (Bohr & Mottelson 1969; Edmonds 1960; Sadeghi et al. 2014)

$$\begin{array}{@{}rcl@{}} &&\langle JM\vert \mathcal{O}_{E\lambda\mu}\vert J_{0} M_{0}\rangle =\left\langle J_{0}M_{0}\lambda\mu|JM\right\rangle \ \frac{\left\langle J\left\Vert \mathcal{O}_{E\lambda}\right\Vert J_{0}\right\rangle }{\sqrt{2J+1}},\\ &&\left\langle J\left\Vert \mathcal{O}_{E\lambda}\right\Vert J_{0}\right\rangle \,=\,\left( -1\right)^{j+I_{a}+J_{0}+\lambda}\!\left[ \left( 2J\,+\,1\right) \left( 2J_{0}\,+\,1\right) \right]^{1/2}\!\left\{\!\!\! \begin{array} [c]{ccc} j & J & I_{a}\\ J_{0} & j_{0} & \lambda \end{array}\!\!\! \right\}\! \left\langle lj\!\left\Vert \mathcal{O}_{E\lambda}\right\Vert l_{0}j_{0}\right\rangle_{J}\!, \end{array} $$

(6)

where the subscript J is a reminder that the matrix element is spin dependent.

The astrophysical S-factor of the ²H(α, γ) ⁶Li radiative capture reaction at low-energies is given by

$$ S(E)=E\sigma(E)\,\text{exp}(2\pi\eta)\ , $$

(7)

where 2π η = 31.29Z ₁ Z ₂(μ/E)^1/2 and σ(E) is the total cross-section. Z ₁ and Z ₂ are the nuclear charges of the interacting nuclei. μ is their reduced mass (in units of a.m.u.), and E is the centre of mass energy (in units of keV). Calculation of S(E) with a centre of mass energy of zero, cannot be measured directly, but instead must be extrapolated from values taken at higher energies.

3 Results and discussion

In this work, the deuteron- α cluster model is used that allows for taking into account the most important features of the two- and three-body α-clusters and the deuteron- α cluster ⁶Li components. An α particle is considered as a boson of spin zero. We derived two-coupled Faddeev equations for the deuteron- α scattering amplitudes. We also derived the results of three-body Faddeev-type calculations for systems of few particles interacting through short-range nuclear interactions plus the long-range Coulomb potentials. Realistic applications of three-body theory to the nuclear reactions only became possible to address in recent years after a reliable and practical momentum-space treatment of the Coulomb interaction was developed by Deltuva et al. (2005). In case of ⁶Li, it is absolutely necessary that system composed of the two- and three-nucleon constituent particles are reasonably described by taking the interactions among these systems as discussed in section 2. In this study, the two- and three-body conservation currents are needed to account for study of photo-nuclear processes (see Sadeghi et al. (2014) for more details).

The cross-section of the ²H(α, γ) ⁶Li reaction at energies E _cm<1 MeV is dominated by radiative E ₂ capture from d waves in the α-deuteron channel into the J ^π = 1⁺ ground state of ⁶Li via a prominent 3⁺ resonance at E _cm = 0.711 MeV. In comparison, E ₁ transitions from p waves to the ⁶Li ground state are strongly suppressed by the isospin selection rule for N = Z nuclei due to the almost equal charge-to-mass ratio of the deuteron and the α particle. Only at very low energies (E _cm≤150 keV), the E ₁ contribution is expected to become larger than the E ₂ capture since the penetrability in p and d waves exhibit a different energy dependence. The astrophysically important quantity is the astrophysical S-factor, S, for the ²H(α, γ) ⁶Li reaction.

For better comparison we separate the calculated S-factor into two different ranges of energy below 1 MeV. In big-bang nucleosynthesis, the ²H(α, γ) ⁶Li process occurs at energies in the range 10 keV ≤E _cm≤ 400 keV. At big-bang nucleosynthesis energies, however, direct measurements are difficult due to extremely low cross-sections (about 29 pb is at E _cm = 100 keV). The astrophysical S-factor for the ²H(α, γ) ⁶Li reaction at 0–0.5 MeV, are compared with the available experimental data from Coulomb dissociation at this range of energies (Kiener et al. 1991) and more recent data by LUNA Collaboration (Anders et al. 2014) in Fig. 1. The results are found to be S(93.3 keV) = 2.54 MeV.nb and S(93.3 keV) = 2.61 MeV.nb in TWB and TWB & THB calculations, in agreement with the recent LUNA Collaboration S(93.3 keV) = 2.6 ± 2.1 MeV.nb.

Figure 1

Results of two-body and two- and three-body values of the calculated total astrophysical S-factors at energies in the range 10 keV ≤E _cm≤ 400 keV, in comparison with the available experimental data from Coulomb dissociation (Kiener et al. 1991) and other theoretical values (Hammache et al. 2010).

The results of calculated S-factor for the ²H(α, γ) ⁶Li radiative capture process at some energies in the range 0.01 MeV ≤E _cm≤ 0.4 MeV are given in Table 1. The table shows the sum of calculated E ₁ and E ₂ contributions of the astrophysical S-factor for the deuteron- α radiative capture process in comparison with the more recent total S-factor laboratory data (Kiener et al. 1991) as well as the theoretical results (Hammache et al. 2010). The re-analysis results by Hammache et al. (2010) have been provided for the most recent indirect measurement at the GSI. Furthermore, due to the weak flux of virtual E ₁ photons, this contribution could not be measured, but only inferred from the theoretical model. From the comparison between predicted and measured Coulomb dissociation cross-sections with virtual E ₂ photons, the applied model could be largely confirmed, and S-factors could be provided for a broad energy range.

Table 1 Theoretical two-body (TWB) and two- and three-body (TWB & THB) values of the calculated total astrophysical S-factors (in MeV.b) at energies in the range 10 keV ≤E _cm≤ 400 keV, in comparison with the available experimental data from Coulomb dissociation (Kiener et al. 1991) and other theoretical values (Hammache et al. 2010).

At higher energies, this reaction has also been studied in direct kinematics, at energies above 1 MeV (Mohr et al. 1994), also in the energy range around the dominant 3⁺ resonance at E _cm = 0.711 MeV. In Fig. 2 and Tables 2 and 3, we represent the results for the calculated total S-factor, around the resonance peak, together with the previous direct data of Mohr et al. (1994) and recent theoretical results (Hammache et al. 2010). A comparison between the calculated total astrophysical S-factors around prominent 3⁺ resonance (E _cm = 0.711 MeV), corresponding with the available experimental data from direct measurements, reveals good agreement especially when the three-body contribution is added.

Figure 2

Results of TWB and TWB & THB values of the calculated total astrophysical S-factors around prominent 3⁺ resonance (E _cm = 0.711 MeV), in comparison with the available experimental data from direct measurements (Mohr et al. 1994) and other theoretical values (Hammache et al. 2010).

Table 2 Theoretical TWB and TWB & THB values of the calculated total astrophysical S-factors (in MeV.b) around prominent 3⁺ resonance (E _cm = 0.711 MeV), in comparison with the available experimental data from direct measurements (Mohr et al. 1994) and other theoretical values (Hammache et al. 2010).

Table 3 Theoretical TWB and TWB & THB values of the calculated total astrophysical S-factors (in MeV.b) at prominent 3⁺ resonance (E _cm = 0.711 MeV), in comparison with the available experimental data.

4 Summary and conclusion

We have studied a three-body approach to compute the deuteron- α radiative capture reaction at astrophysical energies where measurements are impossible and many theoretical studies show different results. In this work, E ₁ and E ₂ contributions of the astrophysical S-factor for the deuteron- α radiative capture process have been calculated. The present calculation is a application of the current conservation realistic potentials method for the ²H(α, γ) ⁶Li nuclear radiative capture reaction. The S-factor is found with(without) three-body interactions, in good agreement with available theoretical results and experiment data.

This method is particularly applicable to very low energy regimes where measurements are impossible. In addition, the method we developed opens new opportunities in addressing few- α, radiative capture reactions at low energy. Specific improvements which will be possible in the near future include the use of improved many-body phenomenological interactions and essentially exact wavefunctions for the many-body cluster bound states.