Introduction. Thermomechanical plastic deformation behavior of metallic materials has integrated the advantages of controlling their geometry and microstructure. The constitutive model is important in the design and simulation of product-forming process and can reflect the evolution of stresses and strains. Dynamic recovery (DRV) and dynamic recrystallization (DRX) are important mechanisms for improving the performance of products, since microstructure of materials is closely related to their dislocation evolution. Complex types of mechanical behavior and microstructure are caused by the concurrent effects of work-hardening (WH), secondary work hardening (SWH), DRV, and DRX during hot deformation. Therefore, dislocation evolution is important for the hot deformation of metallic materials.

In the thermoplastic compressive deformation process of metal materials, stresses firstly increase with strains and then reach their peak values [1,2,3,4,5]. After that, the stress has several possible changing patterns. Firstly, the stress is maintained at the same level, due to the effect of DRV, or fluctuates. Secondly, the stress decreases gradually, due to the effect of DRX. Thirdly, due to the effect of SWH, the stress increases gradually again after the steady state strain is achieved. The DRX steady state is the equilibrium state between DRX softening and WH. After the DRX steady state is realized in the hot deformation process, the steady flow stress and average grain size is achieved. The DRX steady-state behavior of pure copper in the hot deformation process was explored, and the DRX grain size was found to be inversely proportional to flow stress under the steady state with an exponent of 1/2 [6].

The relationship between thermomechanical parameters and the strain hardening and softening kinetics had been extensively explored through some models, especially the Arrhenius hyperbolic-sine equation. The constitutive equations constructed by Sun et al. [7] precisely predicted the peak stress of the Mg–xZn–yEr alloys. Li et al. [8] constructed a constitutive model and explored the effects of strain, deformation temperature and strain rate on the yield stress of Al–Cu–Li–Sc–Zr alloy. The Kocks and Mecking (K–M) model [9,10,11,12,13,14] was used to analyze the evolution of dislocation density. In this K–M model, the microstructural evolution was described with the dislocation density. Huang et al. [10] explored the evolution of the dislocation density of 5754 aluminum alloy by K–M type plots. However, the prediction of the dislocation density evolution of pure copper in DRV, DRX, and SWH processes was seldom reported.

In this study, a refined K–M model of dislocation density evolution was established. The stress-strain data of pure copper were compared and verified to investigate the dislocation and stress evolution rules (WH, DRV, DRX, and SWH) under high-temperature compressive deformation. In addition, based on the dislocation evolution, the metallographic structure, and the DRX mechanism, the effects of temperature and strain rate on the grain size were analyzed.

1. Model Description. The modified K–M model [10] is provided as follows:

$$ \frac{\mathrm{\partial \uprho }}{\mathrm{\partial \upvarepsilon }}={k}_1\sqrt{\uprho}-{k}_2\uprho, $$
(1)

where the multiplication term \( {k}_1\sqrt{\uprho} \) indicates the accumulated moving dislocation density, the annihilation term k2ρ denotes the dislocation annihilation during dynamic softening, while k1 and k2 values are correlated with the strain.

The dislocation density-flow stress relationship is expressed as [14, 15]

$$ \upsigma =\upalpha \upmu b\sqrt{\uprho}, $$
(2)

where α denotes the Taylor constant, μ represents the shear modulus, and b is the Burgers vector.

The stress is correlated with the strain under the same conditions of temperature and strain rate. Equations (1) and (2) can be deduced as

$$ \frac{\mathrm{\partial \uprho }}{\mathrm{\partial \upvarepsilon }}=\frac{2\upsigma \uptheta}{{\left(a\upmu b\right)}^2}, $$
(3)
$$ {k}_1=\frac{2{\uptheta \upsigma}_{sat}}{a\upmu b\left({\upsigma}_{sat}-\upsigma \right)}, $$
(4)
$$ {k}_2=\frac{2\uptheta}{\upsigma_{sat}-\upsigma}, $$
(5)

where σsat is the saturation stress, \( {\upsigma}_{sat}=\upalpha \upmu b\sqrt{\uprho_{sat}} \), ρsat is the dislocation density of saturation state, and θ is the current work-hardening rate, θ= ∂σ/∂ε. Assuming that the entire deformation time ttotal from the initial deformation ε0 to the cumulative true strain εtotal is evenly subdivided into N deformation periods with a time step ∆t, coefficients k1i and k2i in each deformation period can be respectively approximated as constants. When the strain rate \( \overset{\cdot }{\upvarepsilon} \) is constant, the yield stress σi of the ith deformation period is derived as

$$ {\sigma}_i={\sigma}_{sat}-\left({\sigma}_{sat}-{\sigma}_{i-1}\right)\exp \left(-\frac{k_{2i}}{2}\left({\upvarepsilon}_i-{\upvarepsilon}_{i-1}\right.\right). $$
(6)

2. Experimental Results and Discussion. In the simulation, the compressive deformation process of pure copper at high temperatures is subdivided into N deformation periods. The time step of each deformation period is ∆t, and the product of the design time step ∆t and the strain rate \( \overset{\cdot }{\upvarepsilon} \) is 0.002, i.e., \( \overset{\cdot }{\upvarepsilon}\Delta t \) = 0.002. Then, the equivalent strain of the ith deformation period εi = 0.002i. At 700°C, under the strain rates of 0.001 and 0.01 s−1, the stress decreases gradually when the DRX softening effect occurs (Fig. 1). Under the strain rates of 0.1 and 1 s−1, the main deformation mechanism is the DRV. When the SWH occurs, the stress increases gradually. Figure 1 shows the comparison between the stress calculated by Eq. (6) and the experimentally obtained stress. Under the strain rates of 0.001 and 0.01 s−1, the calculated stress basically coincides with the experimentally obtained stress, and the corresponding stress–strain curves are superposed. Under the strain rates of 0.1 and 1 s−1, when the stress is lower than the saturated stress, the calculated stress–strain curves are superposed to the experimental stress–strain curves. When the stress is higher than the saturation stress, the calculated stress–strain curve remains stable indicating that Eq. (6) cannot describe the deformation mechanism of SWH.

Fig. 1
figure 1

Stress–strain curves of pure copper under different strain rates and 700°C.

Full size image

When the SWH stress is higher than the saturation stress, in order to better describe the deformation mechanism of SWH, the saturation stress in Eq. (6) is substituted by the maximum stress. Then, evolutions of the coefficient k2 with strain ε are calculated (Fig. 2). The coefficient k2 varies with strain, indicating the relationship between the strain-hardening rate θ and the stress σ. The stresses calculated with different strain rates are consistent with the experimentally obtained stresses, and all stress–strain curves coincide with each other, indicating that the deformation mechanisms of DRV, DRX, and SWH can be well reflected by the evolution process of the dislocation density.

Fig. 2
figure 2

Evolution of the coefficient k2 with strain ε under different strain rates at 700°C.

Full size image

As shown in Eq. (6), when ε0 ≤ εi ≤ εc and k2i is a constant, Eq. (7) can be used to describe the stress σrec of pure copper DRV:

$$ {\upsigma}_{rec}={\upsigma}_{sat}-\left({\upsigma}_{sat}-{\upsigma}_0\right)\exp \left(-\frac{k_2}{2}\left(\upvarepsilon -{\upvarepsilon}_0\right)\right), $$
(7)

where σ0 and ε0 are the yield stress and its corresponding yield strain, respectively, εc is the critical strain of DRX, k2 is a constant, which can be easily obtained from the linear relationship of \( \ln \frac{\upsigma_{sat}-{\upsigma}_{rec}}{\upsigma_{sat}-{\upsigma}_0} \) and εi − ε0. When εc ≤ εi ≤ εss, the DRX stress σdrx is expressed as [16]:

$$ {\upsigma}_{drx}={\upsigma}_{rec}-\left({\upsigma}_{sat}-{\upsigma}_{ss}\right)\left\{1-\exp \left[-{k}_3{\left(\frac{\upvarepsilon -{\upvarepsilon}_c}{\upvarepsilon_p}\right)}^n\right]\right\}, $$
(8)

where σss is the steady state stress, εp is the peak strain, n is the Avrami’s power, and k3 is a constant. According to Eq. (8), a linear relationship of ln(−ln(1 − Xdrx)) and \( \ln \frac{\upvarepsilon -{\upvarepsilon}_c}{\upvarepsilon_p} \) is easily obtained, and n and k3 values are obtained by the fitting calculation, where Xdrx is the DRX fraction. The DRV stress σrec and DRX stress σdrx are calculated with Eqs. (7) and (8). The calculated stress–strain curve is consistent with the experimental stress–strain curve without considering SWH, indicating that Eqs. (7) and (8) can reflect the stress-strain rules of pure copper DRV and DRX when the strain is smaller than the steady-state strain.

Figure 3 shows curves of calculated (ρ − ρc)/ρc and ε. From DRX to DRX steady state, the curve rises, indicating that the dislocation density increases, and then drops, indicating that DRX consumes a large amount of dislocation. In the DRX deformation process of pure copper, when the strain increases, (ρ − ρc)/ρc value is basically larger than 0, indicating that the dislocation density is higher than the critical value (ρc). The high-temperature compression deformation process of pure copper is dominated by the continuous dynamic recrystallization (CDRX) deformation mechanism. In the curves corresponding to the strain rates of 0.001 and 0.01 s−1, the (ρ − ρc)/ρc value under the DRX steady state is close to 0, indicating that the critical stress σc can be replaced by the steady-state stress σss. With critical stress and steady-state stress exponents, \( n=\partial \left(\ln \overset{\cdot }{\upvarepsilon}\right)/\partial \left(\ln \upsigma \right) \), the corresponding values at 700°C are 6.4 and 5.9, respectively. The same method can be used to calculate stress exponents at other temperatures. Table 1 lists the steady-state stress exponents obtained in previous studies and calculated in this study. The stress exponents remain constant and are close to 6 [17], being insensitive to the processing parameters.

Fig. 3
figure 3

Curves of (ρ − ρc)/ρc as a function of ε under different strain rates at 700°C.

Full size image
Table 1 Steady-State Stress Exponents for the Hot Processing of Pure Copper
Full size table

As shown in Fig. 4, under a strain rate of 0.001 s−1, the corresponding Xdrx/ε value is maximum. The inner deformation energy provides the thermodynamic driving force for DRX nuclei, which is firstly formed at the grain boundary. With an increase in strain, the size of the recrystallized grain increases. The lower strain rate allows the atoms to be sufficiently diffused, and the DRX nuclei grow into recrystallized grains by absorbing the initial microstructure into dynamic recrystallized grains. The recrystallized grains are also easily coarsened. It can be found from Fig. 4 that, under the strain rates of 0.001 and 0.01 s−1, the dynamic recrystallization volume fraction is higher than 99% when the strain reaches steady-state strain. Therefore, it is approximately regarded as an occurrence of a complete dynamic recrystallization process.

Fig. 4
figure 4

Curves of Xdrx/ε as a function of ε at 700°C.

Full size image

After the initiation of DRX, the Xdrx/ε value corresponding to strain rate 1 s−1 increases fastest, and its Xdrx/ε value is higher than Xdrx/ε values corresponding to strain rates of 0.01 and 0.1 s−1, indicating that higher the strain rate, the easier the nucleation process of the recrystallized grain, even if the DRX fraction is smaller. Many small DRX grains appear around initial coarse grains. With an increase in strain, the average size of recrystallized grains and the DRX fraction increase. Furthermore, in a small range of strain, it promotes the recrystallized grains to grow rapidly.

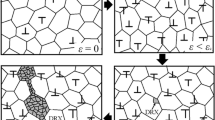
Figure 5 is a typical optical microstructure of pure copper. The initial microstructure (Fig. 5a) is basically replaced by the DRX grain microstructure (Fig. 5b). Figure 5c shows the deformed microstructure with the decline in temperature and rise of strain rate; the grain size of dynamic recrystallization is relatively small but the distribution of its grain size is comparatively non-uniform. The stable size of recrystallized grain is not related to the initial grain size in the equality \( {\upsigma}_{ss}^2{D}_s={\upeta}^2{\upmu}^2b \) [18], where Ds denotes the DRX steady-state grain size and η represents a constant.

Fig. 5
figure 5

Optical microstructures of pure copper under different conditions: (a) initial microstructure; (b) 700°C, 0.01 s−1; (c) 600°C, 1 s−1.

Full size image

In Fig. 6, the DRX steady-state grain size corresponding to the strain rate 1 s−1 is set as a reference value of 1, and the variation patterns of steady-state grain size and logarithmic strain rate are calculated. The relative steady-state grain size increases with the decrease in the strain rate. The higher the temperature, the more obvious the increase of the steady-state grain size. In other words, with a decrease in the deformation temperature, the strain rate increases, thus resulting in a smaller DRX steady-state grain size.

Fig. 6
figure 6

Relationship between the relative steady-state grain size and strain rate.

Full size image

Conclusions. A modified K–M model was established to explore the evolution of dislocation density of pure copper under high-temperature compression deformation. The relationships between the model parameters (WH coefficient, DRV coefficient, and DRX coefficient) and deformation conditions (deformation temperature, strain rate, and strain) were established. The calculated yield stress curves under different conditions were consistent with the experimental results.

The high-temperature compressive deformation of pure copper was dominated by CDRX deformation mechanism. The critical stress and steady-state stress exponents were basically close to six, being insensitive to the processing parameters. The strain and strain rate had a significant effect on the DRX grain size. With a decrease in temperature and increase in strain rate, DRX steady-state grain sizes were reduced.