1 Introduction

The theory of classical thermoelasticity investigates the distributions of thermal stresses caused by the temperature field found from the parabolic type heat conduction equation. The heat conduction of such model is based on the classical Fourier’s law

$$\begin{aligned} {\mathbf{q}} = -K {\text{grad}}T, \end{aligned}$$

relating the heat flux vector q to the temperature gradient \({\text {grad}}T\) [1], where \(K\) is the thermal conductivity. In the non-classical theory of thermoelasticity, the Fourier’s law as well as the heat conduction equation are replaced by more general equations. One can refer to Chandrasekharaiah [2] for a review and presentation of generalized theories. Hetnarski and Ignaczak in their survey article [3] examined five generalizations of the coupled theory and obtained a number of important analytical results.

Goodman and Cowin [4] established a continuum theory for granular materials whose matrix material (or skeletal) is elastic and interstices are voids. The basic concept underlying this theory is that the bulk density of the material is written as the product of two fields- the density field of the matrix material and the volume fraction field (the ratio of the volume occupied by grains to the bulk volume at a point of the material). This representation of the bulk density of the material introduces an additional kinematic variable in the theory. This idea of such representation of the bulk density was employed by Nunziato and Cowin [5] to develop a non-linear theory of elastic material with voids. They developed the constitutive equations for solid like material which are nonconductor of heat and discussed the restrictions imposed on these constitutive equations by thermodynamics. They showed that the change in the volume fraction causes an internal dissipation in the material which is similar to that associated with viscoelastic material. They also considered the dynamic response and derived the general propagation condition on acceleration wave. Later, Cowin and Nunziato [6] developed another theory of linear elastic material with voids for the mathematical study of the mechanical behavior of porous solid. They considered several application of the linear theory by investigating the response of the material to homogeneous deformation, pure bending of beam and small amplitude of acoustic wave. Iesan [7] presented a linear theory for thermo-elastic material with voids. He derived the basic equations and proved the uniqueness of solution, reciprocity relation and variational characterization of solution in the dynamical theory. Later, Cicco and Diaco [8] presented a theory of thermoelastic material with voids without energy dissipation. One can see the literatures [9–21] for various applications of the theory of generalized thermoelasticity in elastic material with voids in one-dimensional space.

Fractional order differential equations have been the focus of many studies due to their frequent appearance in various applications in fluid mechanics, biology, physics, viscoelasticity, mechanics of solids, control theory and engineering. The most important advantage of using differential equation of fractional order in these and other applications is their non-local property. This means that the next state of a system depends not only upon its current state but also upon all of its historical states. This is more realistic and it is one reason why fractional calculus has become more and more popular. The first application of fractional derivative was given by Abel [22] who applied fractional calculus in the solution of an integral equation that arises in the formulation of the tautochrone problem. Caputo [23] gave the definition of fractional derivative of order \(\zeta \in (0,1]\) for absolutely continuous function. Caputo and Mainardi [24, 25], and Caputo [26] found good agreement with experimental results when using fractional derivative for description of viscoelastic material, and established the connection between fractional derivative and the theory of linear viscoelasticity. Oldham and Spanier [27] studied the fractional calculus and proved the generalization of the concept of derivative and integral to a non-integer order. A theoretical basis for the application of fractional calculus to viscoelasticity was given by Bagley and Torvik [28]. Applications of fractional calculus to the theory of viscoelasticity was given by Koeller [29]. Rossikhin and Shitikova [30] presented application of fractional calculus to various problems of mechanics of solids.

Povstenko [31] constructed a quasi-static uncoupled thermoelasticity model based on the heat conduction equation with a fractional order time derivative. He used the Caputo fractional derivative [23], and obtained the stress components corresponding to the fundamental solution of a Cauchy problem for the fractional order heat conduction equation in both the one-dimensional and two-dimensional cases. Povstenko [32] also studied factional Cattaneo-type equation and generalized thermoelasticity. In the last few years, fractional calculus has also been introduced in the field of thermoelasticity [33–38] successfully.

In this paper, the distributions of the temperature, the change in volume fraction, the displacement and the thermal stress in an infinite solid medium with voids are studied in the framework of a theory of generalized thermoelasticity based on the heat conduction equation with a time fractional derivative of order \(0<\zeta \le 1\). We used the Caputo fractional derivative to formulate the fractional heat conduction equation. The fractional heat conduction equation interpolates the standard heat conduction equation for \(\zeta =1\) of Lord–Shulman (L–S model of generalized thermoelasticity [39]). The solution is obtained using the integral transform [40] technique together with an eigenvalue approach method [41–43]. The numerical inversions of the physical variables in the space-time domain are carried out by using the Zakian algorithm [44]. Numerical results are illustrated graphically and analyzed the results.

2 Basic equations and formulation of the problem

Following, Iesan [7], Sherief et al. [35], and Lord & Shulman [39], the governing equations for a homogeneous isotropic generalized thermoelastic material (possessing a center of symmetry) with voids can be put in following form:

Constitutive equations:

$$\begin{aligned} \sigma _{ij}= &\, {} 2\mu e_{ij}+[\lambda e_{kk}+b\Phi -\beta \Theta ]\delta _{ij},\end{aligned}$$
(1)
$$\begin{aligned} h_i= &\, {} \alpha \Phi _{,i},\end{aligned}$$
(2)
$$\begin{aligned} g= &\, {} -be_{kk}-\xi \Phi +m\Theta, \end{aligned}$$
(3)
$$\begin{aligned}&q_i+\tau _0\frac{\partial ^\zeta q_i}{\partial t^\zeta }=-K\Theta _{,i},\,\,0< \zeta \le 1,\end{aligned}$$
(4)
$$\begin{aligned} \rho T_0\eta= &\, {} \rho C_E\Theta +\beta e_{kk}+m\Phi, \end{aligned}$$
(5)

The energy equation for linear theory of thermoelastic material with voids in the presence of heat sources is

$$\begin{aligned} \rho T_0\dot{\eta }=-q_{i,i}+\rho Q, \end{aligned}$$
(6)

Equations of motion:

$$\begin{aligned} \sigma _{ij,j}+\rho F_i=\rho {\ddot{u}}_i, \end{aligned}$$
(7)

Equations of equilibrated forces:

$$\begin{aligned} h_{i,i}+g+\rho l=\rho \chi {\ddot{\Phi}}, \end{aligned}$$
(8)

where \(\sigma _{ij}\) are the components of the stress tensor, \(e_{ij}\) are the components of strain tensor, \(h_i\) are the components of equilibrated stress tensor, \(\Phi \) is the change in volume fraction field, \(\rho \) is the density, \(\eta \) is the entropy per unit mass, g is the intrinsic equilibrated body force, b is the measure of diffusion effects, \(\alpha, \,sm,\,\xi \) are void material parameters, \(q_i\) are the components of heat flux vector, K is the coefficient of thermal conductivity, \(\Theta =T-T_0,\) T is the absolute temperature, \(T_0\) is the temperature of the medium in its natural state assumed to be such that \(\left| \Theta /T_0\right| \ll 1,\) \(F_i, (i=1,2,3)\) are the components of body forces, l is the extrinsic equilibrated force, \(\chi \) is the equilibrated inertia, \(\lambda,\mu \) are Lame’s constants, \(\beta =(3\lambda +2\mu )\alpha _t,\) \(\alpha _t\) is the coefficient of linear thermal expansion, \(\delta _{ij}\) is the Kronecker delta, \(u_i\) are the components of the displacement vector, \(C_E\) is the specific heat at constant strain, \(\tau _0\) is the relaxation time parameter, Q is the internal heat sources, and

$$\begin{aligned} \frac{\partial ^{\zeta }}{\partial t^{\zeta }}f(x,t)=\left\{ \begin{array}{ll} f(x,t)-f(x,0), & {\text{ when }}\quad \zeta \rightarrow 0,\\ I^{1-\zeta }\frac{\partial f(x,t)}{\partial t}, & {\text{ when }}\, 0<\zeta < 1,\\ \frac{\partial f(x,t)}{\partial t}, & {\text{ when} }\, \zeta =1. \end{array}\right. \end{aligned}$$
(9)

In the above definition, \(I^\zeta \) is the Riemann–Liouville fractional integral operator defined as

$$\begin{aligned} I^{\zeta }f(t)=\frac{1}{\Gamma (\zeta )}\int _0^t(t-s)^{\zeta -1}f(s)ds, \end{aligned}$$

where \(\Gamma (\zeta )\) is the well-known Gamma function. A superposed dot represents differentiation with respect to time variable t, and a comma followed by a suffix denotes material derivative and \(i, j = x, y, z\) referee to a general coordinates.

From Eqs. (1)–(8), the field equations in terms of the displacement, volume fraction and temperature field for a homogenous isotropic generalized thermoelastic material with voids and fractional derivative heat transfer subjected to a heat sources in the absence of body forces, and extrinsic equilibrated body forces are

$$\begin{aligned}&\mu u_{i,jj}+(\lambda +\mu )u_{j,ij}+b\Phi _{,i}-\beta \Theta _{,i}=\rho \ddot{u}_i,\end{aligned}$$
(10)
$$\begin{aligned}&\quad K\Theta _{,ii}=\left( 1+\tau _0\frac{\partial ^\zeta }{\partial t^\zeta }\right) \left( \rho C_E\dot{\Theta }+\beta T_0\dot{u}_{k,k}+mT_0\dot{\Phi }-\rho Q\right),\,0<\zeta \le 1,\end{aligned}$$
(11)
$$\begin{aligned}&\quad \alpha \Phi _{,ii}-bu_{k,k}-\xi \Phi +m\Theta =\rho \chi \ddot{\Phi }. \end{aligned}$$
(12)

The homogeneous isotropic infinite thermoelastic solid is unstrained and unstressed initially, but has a uniform temperature distribution \(T_0.\) Let \(x=0\) represents the plane area over which the heat sources Q are situated and the solid occupies the infinite space \(-\infty <x<\infty.\) From the symmetry of the problem, all the physical variables considered depend only on the space variable x and time variable t and thus it follows that for one-dimensional problem, \(u_1=u(x,t),\,u_2=0,\, u_3=0.\) Equations (10)–(12), and Eq. (1) may be put in the following forms:

$$\begin{aligned}&(\lambda +2\mu )\frac{\partial ^2u}{\partial x^2}+b\frac{\partial \Phi }{\partial x}-\beta \frac{\partial \Theta }{\partial x}=\rho \frac{\partial ^2u}{\partial t^2},\end{aligned}$$
(13)
$$\begin{aligned} K\frac{\partial ^2\Theta }{\partial x^2}=\left( 1+\tau _0\frac{\partial ^\zeta }{\partial t^\zeta }\right) \left( \rho C_E\frac{\partial \Theta }{\partial t}+\,\beta T_0\frac{\partial ^2u}{\partial x \partial t}+mT_0\frac{\partial \Phi }{\partial t}-\rho Q\right),\,\,0<\zeta \le 1,\end{aligned}$$
(14)
$$\begin{aligned} \alpha \frac{\partial ^2\Phi }{\partial x^2}-b\frac{\partial u}{\partial x}-\xi \Phi +m\Theta =\rho \chi \frac{\partial ^2\Phi }{\partial t^2},\end{aligned}$$
(15)
$$\begin{aligned}&\quad \sigma _{xx}=(\lambda +2\mu )\frac{\partial u}{\partial x}+b\Phi -\beta \Theta. \end{aligned}$$
(16)

To transform Eqs. (13)–(16) in non-dimensional forms, we will use the following non-dimensional variables

$$\begin{aligned} x'= &\, {} \frac{\widetilde{\omega }}{c_1}x,\,\,u'= \frac{\widetilde{\omega }}{c_1}u,\,\,t'=\widetilde{\omega }t,\,\tau _0'=\widetilde{\omega }^\varsigma \tau _0, \,\sigma _{xx}'=\frac{\sigma _{xx}}{\beta T_0},\\ \Phi '= &\, {} \frac{\widetilde{\omega }^2\chi }{c_1^2}\phi,\,\alpha '=\frac{\alpha }{\rho c_1^2\chi },\,\Theta '=\frac{\Theta }{T_0},\,Q'=\frac{KQ}{\rho c_1^2c_e^2T_0},\,\varepsilon '=\frac{\beta c_1^2}{K\widetilde{\omega }}, \end{aligned}$$

where \(\widetilde{\omega }=\frac{\rho C_Ec_1^2}{K}\) and \(c_1^2=\frac{\lambda +2\mu }{\rho }\).

Using the above variables, Eqs. (13)–(16) take the following forms (omitting the primes for convenience):

$$\begin{aligned}&\frac{\partial ^2u}{\partial x^2}+g_1\frac{\partial \Phi }{\partial x}-g_2\frac{\partial \Theta }{\partial x}=\frac{\partial ^2u}{\partial t^2}\end{aligned}$$
(17)
$$\begin{aligned} g_3\frac{\partial ^2\Phi }{\partial x^2}-g_4\frac{\partial u}{\partial x}-g_5\Phi +g_6\Theta =\frac{\partial ^2\Phi }{\partial t^2}\end{aligned}$$
(18)
$$\begin{aligned} \frac{\partial ^2\Theta }{\partial x^2}=\left( 1+\tau _0\frac{\partial ^\zeta }{\partial t^\zeta }\right) \left[ \frac{\partial \Theta }{\partial t}+\varepsilon \frac{\partial ^2u}{\partial x \partial t}+g_7\frac{\partial \Phi }{\partial t}-Q(x,t)\right],\,0<\zeta \le 1,\end{aligned}$$
(19)
$$\begin{aligned} \sigma _{xx}=g_8\frac{\partial u}{\partial x}+g_9\Phi -\Theta, \end{aligned}$$
(20)

where

$$\begin{aligned} g_1= &\, {} \frac{b}{\rho \chi \widetilde{\omega }^2},\,g_2=\frac{\beta T_0}{\rho c_1^2},\,g_3=\alpha,\,g_4=\frac{b}{\rho c_1^2},\,g_5=\frac{\xi }{\rho \chi \widetilde{\omega }^2},\\ g_6= &\, {} \frac{mT_0}{\rho c_1^2},\,g_7=\frac{mc_1^4}{k\chi \widetilde{\omega }^3},\,g_8=\frac{\rho c_1^2}{\beta T_0},\,g_9=\frac{bc_1^2}{\beta \chi \widetilde{\omega }^2T_0}. \end{aligned}$$

For time-dependent continuous heat sources over the plane \(x=0,\) we may represent it as \(Q(x,t)=Q_0\delta (x)H(t),\) where \(\delta (x)\) is the Dirac’s delta function defined by

$$\begin{aligned} \delta (x)=0\,\,{\text {for}}\,\,x\ne 0;\,\, \int _{-\infty }^{+\infty }\delta (x)dx=1;\,\,{\text{ and }}\,\,\int _{-\infty }^{+\infty }\delta (x)f(x)dx=f(0), \end{aligned}$$

\(H(t)\) is the Heaviside unit step function defined by

$$\begin{aligned} H(t)=\left\{ \begin{array}{ll} 1,&t>0\\ 0,&t\le 0 \end{array}\right., \end{aligned}$$

and \(Q_0\) is a constant.

3 Solution in the Laplace transform domain: eigenvalue approach

Taking the Laplace transform of parameter \(s,\) defined by

$$\begin{aligned} L\left[ f(x,t)\right] =\int _0^\infty \exp (-st)f(x,t)dt=\bar{f}(x,s)\,\,\left( \text{ Re }(s)>0\right), \end{aligned}$$
(21)

on both sides of the Eqs. (17)–(20) (assuming the homogeneous initial conditions), we get

$$\begin{aligned} D^2\bar{u}= &\, {} s^2\bar{u}-g_1D\bar{\Phi }+g_2D\bar{\Theta },\end{aligned}$$
(22)
$$\begin{aligned} D^2\bar{\Phi }= &\, {} \frac{g_4}{g_3}D\bar{u}+\frac{g_5+s^2}{g_3}\bar{\Phi } -\frac{g_6}{g_3}\bar{\Theta },\end{aligned}$$
(23)
$$\begin{aligned} D^2\bar{\Theta }= &\, {} s(1+\tau _0s^\zeta )\left[ \varepsilon D\bar{u}+g_7\bar{\Phi }+\bar{\Theta }-\frac{Q_0\delta (x)}{s^2}\right],\end{aligned}$$
(24)
$$\begin{aligned} \bar{\sigma }_{xx}= &\, {} g_8D\bar{u}+g_9\bar{\Phi }-\bar{\Theta }. \end{aligned}$$
(25)

Following [41–43], Eqs. (22)–(24) can be written in a vector-matrix differential equation as follows:

$$\begin{aligned} D\tilde{v}(x,s)={\mathcal {A}}(s)\tilde{v}(x,s)+\tilde{f}(x,s), \end{aligned}$$
(26)

where

$$\begin{aligned} D= &\, {} \frac{d}{dx},\,\,\tilde{v}(x,s)=\left(\begin{array}{l} \bar{u}\\ \bar{\Phi }\\ \bar{\Theta }\\ D\bar{u}\\ D\bar{\Phi }\\ D\bar{\Theta }\end{array}\right),\,\, {\mathcal {A}}(s)=\left(\begin{array}{llllll} 0 & 0 & 0 & 1 & 0 & 0 \\ 0 & 0 & 0 & 0 & 1 & 0 \\ 0 & 0 & 0 & 0 & 0 & 1 \\ C_{41} & 0 & 0 & 0 & C_{45} & C_{46}\\ 0 & C_{52} & C_{53} & C_{54} & 0 & 0\\ 0 & C_{62} & C_{63} & C_{64} & 0 & 0 \end{array}\right),\,\,\tilde{f}(x,s)=\left(\begin{array}{l} 0\\ 0\\ 0\\ 0\\ 0\\ f_6 \end{array}\right),\nonumber \\ C_{41}= &\, s^2,\,\,C_{45}=-g_1,\,\,C_{46}=g_2,\,\,C_{52}= \frac{g_5+s^2}{g_3},\,\,C_{53}=-\frac{g_6}{g_3},\,\,C_{54}=\frac{g_4}{g_3},\\ C_{62}= &\, g_7s(1+\tau _0s^\zeta ),\,\,C_{63}=s(1+\tau _0s^\zeta ),\,\, C_{64}=\varepsilon s(1+\tau _0s^\zeta ), f_6=-Q_0s^{-1}(1+\tau _0s^\zeta ) \delta (x). \end{aligned}$$

Following the solution methodology through eigenvalue approach [42, 43], we now proceed to solve the vector-matrix differential equation (26). The characteristic equation of the matrix \({\mathcal {A}}(s)\) can be written as

$$\begin{aligned} k^6-Pk^4+Qk^2-R=0, \end{aligned}$$
(27)

where

$$\begin{aligned} P= &\, C_{41}+C_{52}+C_{63}+C_{45}C_{54}+C_{46}C_{64},\\ Q= &\, C_{41}\left( C_{52}+C_{63}\right) +C_{52}C_{63}-C_{53}C_{62}+C_{46}\left( C_{52}C_{64}-C_{54}C_{62}\right) \\&-C_{45}(C_{53}C_{64}-C_{54}C_{63}),\\ R= &\, C_{41}(C_{52} C_{63}-C_{53}C_{62}). \end{aligned}$$

Let \(k^2_1,\) \(k^2_2\) and \(k^2_3\) be the roots of the above characteristic Eq. (27) with positive real parts. Then all the six roots of the above characteristic equation which are also the eigenvalues of the matrix \({\mathcal {A}}(s)\) are of the form

$$\begin{aligned} k=\pm k_1,\,\,\pm k_2,\,\,\pm k_3, \end{aligned}$$

where

$$\begin{aligned} k_1^2= &\, \frac{1}{3}(2p{\text{ sin} }q+P),\\ k_2^2= &\, \frac{-1}{3}\left( p[\sqrt{3}{\text{ cos }}q+{\text{ sin }}q]-P\right),\\ k_3^2= &\, \frac{1}{3}\left( p[\sqrt{3}{\text{ cos} }q-{\text{ sin}}q]+P\right), \end{aligned}$$

and

$$\begin{aligned} p=\sqrt{P^2-3Q},\,\,q=\frac{\text{ sin }^{-1}r}{3}, r=\frac{9PQ-2P^3-27R}{2p^3}. \end{aligned}$$

Suppose \({\mathcal {X}}(k)\) be a right eigenvector corresponding to the eigenvalue \(k\) of the matrix \({\mathcal {A}}(s).\) Then after some simple manipulations, we get

$$\begin{aligned} {\mathcal {X}}(k)=\left(\begin{array}{l} k\left[ C_{45}C_{53}-C_{46}(C_{52}-k^2)\right] \\ \left[ k^2C_{46}C_{54}-C_{53}\left( C_{41}-k^2\right) \right] \\ \left[ (C_{41}-k^2)(C_{52}-k^2)-k^2C_{45}C_{54}\right] \\ k^2\left[ C_{45}C_{53}-C_{46}(C_{52}-k^2)\right] \\ k\left[ k^2C_{46}C_{54}-C_{53}\left( C_{41}-k^2\right) \right] \\ k\left[ (C_{41}-k^2)(C_{52}-k^2)-k^2C_{45}C_{54}\right] \end{array}\right). \end{aligned}$$
(28)

We can easily calculate the eigenvector \({\mathcal {X}}_j\,\,(j=1,2,3)\) corresponding to the eigenvalue \(\pm k_j\,\,(j=1,2,3)\) from (28). For our further reference, we shall use the following notations:

$$\begin{aligned} {\mathcal {X}}_1= &\, {\mathcal {X}}(k_1),\,\,{\mathcal {X}}_2={\mathcal {X}}(-k_1),\,\,{\mathcal {X}}_3={\mathcal {X}}(k_2),\nonumber \\ {\mathcal {X}}_4= &\, {\mathcal {X}}(-k_2),\,\,{\mathcal {X}}_5={\mathcal {X}}(k_3),\,\,{\mathcal {X}}_6={\mathcal {X}}(-k_3). \end{aligned}$$
(29)

We assume the inverse of the matrix \(V=\left(\begin{array}{llllll} {\mathcal {X}}_1,&{\mathcal {X}}_2,&{\mathcal {X}}_3,&{\mathcal {X}}_4,&{\mathcal {X}}_5,&{\mathcal {X}}_6\end{array}\right)\) as

$$\begin{aligned} V^{-1}=(w_{ij}),\,\,i,j=1,2,\ldots,6. \end{aligned}$$

Hence using the expression for \(\tilde{f}(s),\) we can calculate the expression for \(Q_r\) as (see [43] for details):

$$\begin{aligned} Q_r=\sum _ {j=1}^6 w_{rj}f_j=w_{r6}f_6,\,\,r=1,2,\ldots,6. \end{aligned}$$
(30)

As in [43], the solution of the vector-matrix differential equation (26) can be written as

$$\begin{aligned} \tilde{v}=X_2y_2+X_4y_4+X_6y_6, \end{aligned}$$
(31)

where

$$\begin{aligned} y_r=e^{k_rx}\left[ y_re^{-k_rx}\right] _{{x=-\infty }}+e^{k_rx}\int _{-\infty }^x Q_re^{-k_rx}dx,\,x>0. \end{aligned}$$
(32)

Since \(y=V^{-1}\tilde{v}\) and and the field variables in \(\tilde{v}\) vanish at \(x=+\infty,\) we neglect the first term on the right hand side of (32), and we get

$$\begin{aligned} y_2=-\frac{Q_0(1+\tau_{0}s^\zeta )}{s}w_{26}e^{-k_1x},y_4 =-\frac{Q_0(1+\tau_{0}s^\zeta )}{s}w_{46}e^{-k_2x},y_6 =-\frac{Q_0(1+\tau_{0}s^\zeta )}{s}w_{66}e^{-k_3x}\,(x>0), \end{aligned}$$
(33)

since \(y_1,\) \(y_3\) and \(y_5\) are neglected from the physical considerations of the problem.

Thus, we get

$$\begin{aligned} \left(\begin{array}{l} \bar{u}\\ \bar{\Phi }\\ \bar{\Theta }\end{array}\right)=-\left(\begin{array}{l} -k_1\left[ C_{45}C_{53}-C_{46}(C_{52}-k_1^2)\right] \\ \nonumber \left[ k_1^2C_{46}C_{54}-C_{53}\left( C_{41}-k_1^2\right) \right] \\ \left[ (C_{41}-k_1^2)(C_{52}-k_1^2)-k_1^2C_{45}C_{54}\right] \end{array}\right)\frac{Q_0(1+\tau _0s^\zeta )w_{26}}{s}e^{-k_1x}\\ \nonumber -\left(\begin{array}{l} -k_2\left[ C_{45}C_{53}-C_{46}(C_{52}-k_2^2)\right] \\ \left[ k_2^2C_{46}C_{54}-C_{53}\left( C_{41}-k_2^2\right) \right] \\ \left[ (C_{41}-k_2^2)(C_{52}-k_2^2)-k_2^2C_{45}C_{54}\right] \end{array}\right)\frac{Q_0(1+\tau _0s^\zeta )w_{46}}{s}e^{-k_2x}\\ \nonumber -\left(\begin{array}{l} -k_3\left[ C_{45}C_{53}-C_{46}(C_{52}-k_3^2)\right] \\ \left[ k_3^2C_{46}C_{54}-C_{53}\left( C_{41}-k_3^2\right) \right] \\ \left[ (C_{41}-k_3^2)(C_{52}-k_3^2)-k_3^2C_{45}C_{54}\right] \end{array}\right)\frac{Q_0(1+\tau _0s^\zeta )w_{66}}{s}e^{-k_3x} \nonumber \end{aligned}$$

The expressions for \(\bar{u}(x,s),\) \(\bar{\phi }(x,s)\) and \(\bar{\Theta }(x,s)\) can now be written as

$$\begin{aligned} \bar{u}(x,s)= &\, \frac{Q_0(1+\tau _0s^\zeta )}{s}[k_1\{C_{45}C_{53}-C_{46}(C_{52}-k_1^2)\}w_{26}e^{-k_1x}\nonumber \\&+k_2\{C_{45}C_{53}-C_{46}(C_{52}-k_2^2)\}w_{46}e^{-k_2x}\nonumber \\&+k_3\{C_{45}C_{53}-C_{46}(C_{52}-k_3^2)\}w_{66}e^{-k_3x}],\end{aligned}$$
(34)
$$\begin{aligned} \bar{\Phi }(x,s)= &\, -\frac{Q_0(1+\tau _0s^\zeta )}{s}[\{k_1^2C_{46}C_{54}-C_{53}\left( C_{41}-k_1^2\right) \}w_{26}e^{-k_1x}\nonumber \\&+\{k_2^2C_{46}C_{54}-C_{53}\left( C_{41}-k_2^2\right) \}w_{46}e^{-k_2x}\nonumber \\&+\{k_3^2C_{46}C_{54}-C_{53}\left( C_{41}-k_3^2\right) \}w_{66}e^{-k_3x}],\end{aligned}$$
(35)
$$\begin{aligned} \bar{\Theta }(x,s)= &\, -\frac{Q_0(1+\tau _0s^\zeta )}{s}[\{(C_{41}-k_1^2)(C_{52}-k_1^2)-k_1^2C_{45}C_{54}\}w_{26}e^{-k_1x}\nonumber \\&+\{(C_{41}-k_2^2)(C_{52}-k_2^2)-k_2^2C_{45}C_{54}\}w_{46}e^{-k_2x}\nonumber \\&+\{(C_{41}-k_3^2)(C_{52}-k_3^2)-k_3^2C_{45}C_{54}\}w_{66}e^{-k_3x}. \end{aligned}$$
(36)

Using Eqs. (34)–(36) in the Eq. (25), the stress component \(\bar{\tau }_{xx}(x,s)\) can be determined as

$$\begin{aligned} \bar{\sigma }_{xx}(x,s)= &\, -\frac{g_8Q_0(1+\tau _0s^\zeta )}{s}[k^2_1\{C_{45}C_{53}-C_{46}(C_{52}-k_1^2)\}w_{26}e^{-k_1x}\nonumber \\&+k^2_2\{C_{45}C_{53}-C_{46}(C_{52}-k_2^2)\}w_{46}e^{-k_2x}\nonumber \\&+k^2_3\{C_{45}C_{53}-C_{46}(C_{52}-k_3^2)\}w_{66}e^{-k_3x}]\nonumber \\&-\frac{g_9Q_0(1+\tau _0s^\zeta )}{s}[\{k_1^2C_{46}C_{54}-C_{53}\left( C_{41}-k_1^2\right) \}w_{26}e^{-k_1x}\nonumber \\&+\{k_2^2C_{46}C_{54}-C_{53}\left( C_{41}-k_2^2\right) \}w_{46}e^{-k_2x}\nonumber \\&+\{k_3^2C_{46}C_{54}-C_{53}\left( C_{41}-k_3^2\right) \}w_{66}e^{-k_3x}]\nonumber \\&+\frac{Q_0(1+\tau _0s^\zeta )}{s}[\{(C_{41}-k_1^2)(C_{52}-k_1^2)-k_1^2C_{45}C_{54}\}w_{26}e^{-k_1x}\nonumber \\&+\{(C_{41}-k_2^2)(C_{52}-k_2^2)-k_2^2C_{45}C_{54}\}w_{46}e^{-k_2x}\nonumber \\&+\{(C_{41}-k_3^2)(C_{52}-k_3^2)-k_3^2C_{45}C_{54}\}w_{66}e^{-k_3x}. \end{aligned}$$
(37)

4 Numerical results and discussions

For the final solution of the temperature \(\Theta,\) the volume fraction field \(\Phi,\) the displacement \(u\) and the stress \(\sigma _{xx}\) distributions in the time domain, we adopt a numerical inversion method based on the Zakian [44]. In this method, the inverse \(f(t)\) of the Laplace transform \(\bar{f}(s)\) is approximated by the following relation:

$$\begin{aligned} f(t)=\frac{2}{t}\sum _{i=1}^N Re\left[ K_i\bar{f}\left( \frac{\alpha _i}{t}\right) \right] \,(0<t<\infty ), \end{aligned}$$
(38)

where the constants \(K_i\) and \(\alpha _i\) for \(N=5\) are given in Table 1.

Table 1 Five constants for \(\alpha \) and \(K\) for the Zakian method [44]
Full size table

This method is fast and easy to implement, and there is one free parameter, \(N\), to be determined. Thus the solutions of all the physical variables in space-time domain are given by:

$$\begin{aligned} \Theta (x,t)=\frac{2}{t}\sum _{i=1}^N Re\left[ K_i\bar{\Theta }\left( x,\frac{\alpha _i}{t}\right) \right],\end{aligned}$$
(39)
$$\begin{aligned} \Phi (x,t)=\frac{2}{t}\sum _{i=1}^N Re\left[ K_i\bar{\Phi }\left( x,\frac{\alpha _i}{t}\right) \right],\end{aligned}$$
(40)
$$\begin{aligned} u(x,t)=\frac{2}{t}\sum _{i=1}^N Re\left[ K_i\bar{u}\left( x,\frac{\alpha _i}{t}\right) \right],\end{aligned}$$
(41)
$$\begin{aligned} \sigma _{xx}(x,t)=\frac{2}{t}\sum _{i=1}^N Re\left[ K_i\bar{\sigma }_{xx}\left( x,\frac{\alpha _i}{t}\right) \right]. \end{aligned}$$
(42)

To illustrate and compare the theoretical results obtained in the Sect. 3, we now present some numerical results which depict the variations of the temperature, the volume fraction field, the displacement, and the stress component. The material chosen for the purpose of numerical evaluations is magnesium crystal, for which we take the following values of the different physical constants:

$$\begin{aligned} \lambda= &\, 2.17\times 10^{10}\,{\text {Nm}}^{-1},\,\mu =3.278\times 10^{10}\,{\text {Nm}}^{-1},\,\rho =1.74\times 10^3\,{\text {kg}}\,{\text {m}}^3,\,T_0=298^\circ {\text {K}},\\ C_E= &\, 1.04\times 10^3\,{\text{J}}\,{\text {kg}}^{-1}{\text {deg}}^{-1},\,K=1.7\times 10^2\,{\text {W}}\,{\text {m}}^{-1}{\text {deg}}^{-1},\,\beta =2.68\times 10^6\,{\text {Nm}}^{-2}{\text {deg}}^{-1}. \end{aligned}$$

The void parameters are

$$\begin{aligned} \chi= &\, 1.753\times 10^{-15}\,{\text {m}}^2,\,\alpha =3.688\times 10^{-5}\,{\text {N}},\,\xi =1.475\times 10^{10}\,{\text {Nm}}^{-2},\\ b= &\, 1.13849\times 10^{10}\,{\text {Nm}}^{-2},\,m=2\times 10^6\,{\text {Nm}}^{-2}{\text {deg}}^{-1}. \end{aligned}$$

The non-dimensional relaxation time is \(\tau _0=0.02\).

The computations are carried out for \(\zeta =0.5,1.0\) and \(t=0.9\). The results are represented graphically for different positions of \(x\) using MATLAB and Mathematica software. The case \(\zeta =1.0\) indicates the linear Lord & Shulman model with voids and the case \(\zeta =0.5\) indicates the fractional order Lord & Shulman model with voids of generalized thermoelasticity.

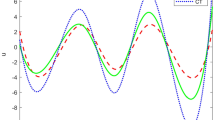
Figures 1, 2, 3 and 4 exhibit the space variations of the field quantities in the context of fractional order generalized thermoelasticity for different values of the fractional parameter \(\zeta \).

Fig. 1
figure 1

Temperature distribution \(\Theta \) at \(t=0.9\) for different values of \(\zeta \)

Full size image
Fig. 2
figure 2

Volume fraction field distribution \(\Phi \) at \(t=0.9\) for different values of \(\zeta \)

Full size image
Fig. 3
figure 3

Displacement distribution \(u\) at \(t=0.9\) for different values of \(\zeta \)

Full size image
Fig. 4
figure 4

Stress distribution \(\sigma _{xx}\) at \(t=0.9\) for different values of \(\zeta \)

Full size image

Figure 1 depicts the variations of the temperature \(\Theta \) with distance \(x\) for different values of \(\zeta \), and it is noticed that in both the cases (i.e., \(\zeta \) = 0.5 and \(\zeta \) = 1.0), maximum value of \(\Theta \) is 0.53 which is on the boundary of the half-space \(x\ge 0\). We observe from the figure that the difference is negligible in the beginning, and with the increase in \(x\), the difference is slightly pronounced upto \(x\le 3.2\); both the series approach to zero. The trends of both the series are alike only upto \(x\le 1.15\).

Figure 2 shows the variations of the volume fraction field \(\Phi \) with \(x\) for different values of \(\zeta \). It is evident from the figure that both the series have similar trend upto \(x\le 1.6\), and finally converge to zero. We notice from the figure that the difference is significant at the beginning, and with the increase in \(x\), the difference is slightly pronounced upto \(x\le 3.0\). In all the cases (\(i.e., \zeta =0.5,1.0\)), \(\Phi \) attains its maximum value at the boundary of half-space.

Figure 3 displays the variations of displacement component \(u\) for different values of \(\zeta \), and it is noticed that in both the cases (i.e., \(\zeta \) = 0.5 and \(\zeta \) = 1.0), \(u\) starts with zero value, which is on the boundary of the half-space. It is also noticed that both the series have similar trend, that is, first increases to a maximum value and then decreases to a minimum value. We observe from the figure that the difference is significant in the range \(0\le x\le 1.4\), and the difference is slightly pronounced for \(x> 1.4\). Both the series approach to zero with the increase of \(x\).

Figure 4 shows the variations of stress component \(\sigma _{xx}\) with distance \(x\) for different values of \(\zeta \). It is evident from the figure that it start with some non-zero negative value on the boundary of the half-space. The difference in both the series is slightly pronounced in the range \(0\le x\le 0.8\) and the difference is significant in the range \(0.8\le x\le 3.5\). After that both the series approach to zero for \(x\ge 3.5\).

Figures 5, 6, 7 and 8 display the temperature, volume fraction field, displacement, and stress distributions for wide range of \(x\) (\(0.0\le x\le 4.0\)) at \(\zeta =0.5\) for different values of the time parameter \(t=0.3,0.5\) and we have noticed that the time parameter \(t\) play significant role on all the studied fields. The increasing of the value of \(t\) causes increasing of the values of all the studied fields and makes the speed of the waves propagation vanishes more rabidly.

Fig. 5
figure 5

Temperature distribution \(\Theta \) at \(\zeta =0.5\) for different \(t\)

Full size image
Fig. 6
figure 6

Volume fraction field distribution \(\Phi \) at \(\zeta =0.5\) for different \(t\)

Full size image
Fig. 7
figure 7

Displacement distribution u at \(\zeta =0.5\) for different t

Full size image
Fig. 8
figure 8

Stress distribution \(\sigma _{xx}\) at \(\zeta =0.5\) for different t

Full size image

5 Concluding remarks

  1. 1.

    The results of this work presents the fractional order generalized thermoelasticity theory with voids as a new improvement and progress in the field of the thermoelasticity with voids subjected to a instanced heat sources. According to this theory, we have to construct a new classification to all the materials according to its fractional parameter \(\zeta \) where this parameter becomes new indicator of its ability to conduct the thermal energy.

  2. 2.

    The method eigenvalue approach [41–43] reduced the problem on vector-matrix differential equation to an algebraic eigenvalue problems and the solutions for the field variables were achieved by determining the eigenvalues and the corresponding eigenvectors of the coefficient matrix. In this method, the physical quantities are directly involved in the formulating of the problem and as such the boundary and initial conditions can be applied directly. This is not in other methods, like State-Space-Approach.

5.1 Application of the model

The formation of one-dimensional void material will help researchers in the material chemistry for developing one-dimensional nanocomposites for applications in pharmaceutical technology and also in environmental chemistry. A nanosized highly luminescent \(LaPo_4:Ce^{3+}, Tb^{3+}\) is nowadays one of the important material for biomedical applications such as fluorescence resonance energy-transfer assays, optical imaging, etc. A new mesoporous hybrid titanium (IV) phosphonate nanomaterial has been synthesized by using Benzene-1,3,5-triphosphonic acid as the organophosphorus source in the absence of any template molecule. The photocurrent generated by sensitizer entrapped titanium phosphonate material is quite higher than other titanium oxide based nanomaterials. Thus, one can design different hybrid titanium phosphonate materials bearing organic functionalities to enhance the efficiency of the photon-to-electron energy transfer process. On the other side, the hybrid titanium phosphonate material with nanoscale porosity may have potential biological applications in drug delivery and in lithium ion batteries [45].