1 Introduction

The studies of mixed-spin (integer (I) and half-integer (HI), HI and HI, I and I spins) systems are an important and interesting research area in statistical physics and condensed matter for the last 25 years. The reason is that the mixed-spin systems have less translational symmetry than their single-spin counterparts and are well adapted to study a certain type of ferrimagnetic materials. Moreover, another reason is that ferrimagnetic materials, which can be studied with mixed-spin systems, are possibly useful for advanced technological applications (see [1–6] and references therein).

Fig. 1
figure 1

The dynamic hysteresis behaviors at different reduced temperatures for the mixed-spin (1, 5/2) systems: a m A, b m B, and c m T. We use w = 0.05 π, d = 0.5, and h = 1.0 Hamiltonian parameters

Full size image

There are many combinations of the mixed I and HI Ising system, such as mixed-spin (1/2, 1), (1, 3/2), (2, 3/2), (1, 5/2), and (2, 5/2) systems. The mixed-spin (1, 5/2) Ising system has been less considered for investigation according to other mixed-spin systems with I and HI spins. We should note that the molecular mixed-spin ferromagnetic chain material MnNi(NO2)4 (ethylenediamine) is known as quasi-one-dimensional material which composed of spin-1 and spin-5/2 [7]. The first study in the literature was done by Deviren et al. [8]. The equilibrium features of the system like hysteresis, magnetization, ground-state phase diagram, susceptibility, specific heat, and internal energy are comprehensively examined on the square and honeycomb lattices by utilizing effective field theory (EFT) in this study. Yessoufou et al. [9] studied mixed-spin (1, 5/2) Blume-Emery-Griffiths (BEG) model on the Bethe lattice (BL) in terms of exact recursion equations and obtained the equilibrium phase diagram of the system. The system illustrates the second- and first-order phase transition temperatures as well as compensation temperatures. Finally, Yiğit and Albayrak [10] studied critical properties of system with equal and unequal crystal fields on BL. They have obtained the thermal behavior of the magnetizations to characterize the nature (continuous or discontinuous) of the transitions and presented equilibrium phase diagrams in the plane of reduced temperature versus crystal field. It is observed that the system shows second- and first-order transitions for equal and unequal crystal fields. Moreover, the phase diagrams illustrate reentrant and compensation behaviors. In addition to these three equilibrium studies in which the equilibrium properties of a mixed-spin system composed of spin-1 and spin-5/2 are investigated in detail, there are only two works on the non-equilibrium behavior of mixed system [11, 12]. Keskin et al. [11] examined dynamic phase transition (DPT) temperatures and dynamic phase diagrams (DPDs) of the mixed-spin (1, 5/2) BEG system by means of DMFA. The dynamic phase diagrams have been obtained in various planes, and a comparison is made with the results of other kinetic mixed-spin Ising systems. These comparisons illustrate that the mixed-spin (1, 5/2) system displays more richer, distinct, and interesting topological phase diagram than the mixed system composed of low values of spin variables. Recently, Özkılıç and Temizer [12] investigated the dynamic phase diagrams and compensation temperatures of the system on alternate layers of a hexagonal lattice by using DMFA. It has been found that the system exhibits five fundamental and nine mixed phases as well as the compensation temperature or N-type behavior in the Néel classification [13].

Conversely, unfortunately, to the best of our knowledge, right now, there are no works related to the dynamic magnetic hysteresis (DMH) properties of the mixed-spin-1 and spin-5/2 Ising system. Hence, the aim of this work is to investigate the DMH properties in a two-dimensional mixed-spin (1, 5/2) Ising system. Especially, we examine the effect of Hamiltonian parameters such as the temperature, crystal field and frequency of oscillating external magnetic on the DMH properties. In addition, this work also aims to find the dynamic coercive field and remanent magnetization.

The organization of the remaining part of this paper is as follows. The dynamic mean-field approximation formulation is given in brief in Section 2. In Section 3, the results and remarks are presented in detail. Finally, we give the conclusion in Section 4.

2 The Dynamic Mean-Field Approximation Formulation

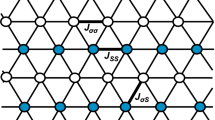
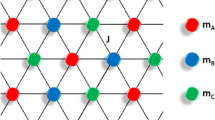
Since the formulation is given and discussed in Refs. [11, 12] in detail, we will briefly summarize it here. The mixed-spin system composed of two sublattices A and B which two are interpenetrating square lattices. The system has two order parameters, namely two average magnetizations < σ>and <S>for the A and B sublattices, respectively, which are the excess of one orientation over the other. The Hamiltonian is described as follows:

$$\begin{array}{@{}rcl@{}} H&=& -J\sum\nolimits_{<ij>} {\sigma_{i} S_{j} -D\left( {\sum\nolimits_{i} {{\sigma_{i}^{2}} +\sum\nolimits_{j} {{S_{j}^{2}}}}} \right)}\\ &&-h(t)\left( {\sum\nolimits_{i} {\sigma_{i} +\sum\nolimits_{j} {S_{j}} } } \right) \end{array} $$
(1)

where σ i and S j take the ± 1.0 and 0.0 values and ± 5/2, ± 3/2, and ± 1/2 values, respectively. The summation index <ij> denotes a summation over all pairs of nearest-neighbor spins. J and D are defined as the exchange constant and crystal field interaction parameter, respectively. The last term in Hamiltonian is an oscillating external magnetic field, and it is given by h(t) = h 0 cos(w t). h 0 and w = 2 π f are the amplitude and frequency of the oscillating field, respectively. The system is in contact with an isothermal heat reservoir at absolute temperature (T A). According to (7) and (9) in Ref. [11] and (11) and (12) in Ref. [12], dynamic MFA equations are described for A and B

$$ {\Omega} \frac{d}{d\xi} m_{\mathrm{A}} \,=\, -m_{\mathrm{A}} \!+\frac{2\sinh \left[ {\beta \left( {m_{\mathrm{B}} +h_{0} \cos (\xi )} \right)} \right]}{\exp (-\!3\beta d)\,+\,2\cosh \left[ {\beta \left( {m_{\mathrm{B}}\! +\!h_{0} \cos (\xi )} \right)} \right]} $$
(2)
$$ {\Omega} \frac{d}{d\xi} m_{\mathrm{B}} =-m_{\mathrm{B}} +\frac{5\exp \left( {\frac{2d}{T}} \right)\sinh \left[ {\frac{5a}{2T}} \right]+3\exp \left( {-\frac{2d}{T}} \right)\sinh \left[ {\frac{3a}{2T}} \right]+\exp \left( {-\frac{4d}{T}} \right)\sinh \left[ {\frac{a}{2T}} \right]}{2\exp \left( {\frac{2d}{T}} \right)\cosh \left[ {\frac{5a}{2T}} \right]+2\exp \left( {-\frac{2d}{T}} \right)\cosh \left[ {\frac{3a}{2T}} \right]+2 \exp \left( {-\frac{4d}{T}} \right)\cosh \left[ {\frac{a}{2T}} \right]} $$
(3)

where a = m A + h 0 cos(ξ), m A = 〈σ〉, m B = 〈S〉, ξ = ω t, \(T=\frac {k_{\mathrm {B}} T_{\mathrm {A}}} {| J |} \), \(h_{0} =\frac {H_{0}} {| J |} \), \(d=\frac {D}{| J |} \), and Ω = τ ω. We fixed J = 1 throughout the paper.

In order to study the DMH behaviors, one should describe the dynamic hysteresis loop area and it is defined as

$$ m_{\mathrm{T}} =-\oint {m(t)dh=-h_{0} \omega \oint {m(t)\cos (\omega t)dt}} $$
(4)

where m T = (m A + m B)/2. The solution and discussion of Eq. (4) will be given in the next section.

3 Results and Discussions

In order to investigate the behavior of the DMH loop with the variation of the Hamiltonian parameters, we can use quantities which are related to the shapes of the hysteresis loop, the coercivity field (high coercivity is the term called for magnetically hard materials and low coercivity for magnetically soft ones), and the remanent magnetization.

Figure 1 is obtained for selected temperature values, namely T = 3.1, 4.25, 4.5, 5.0, 5.5, 6.1, 7.2, 8.7, and 27, in the case of w = 0.05 π, d = 0.5, and h = 1.0, fixed values to investigate the temperature dependence of the DMH behavior of the mixed-spin (1, 5/2) system. We can see that the DMH loop areas increase as the temperature increases and, at a certain temperature, the loop areas decrease with increasing the temperature. With further increase of temperature, the hysteresis loops are very slim. The conventional hysteresis loop is observed around T = 5.0. Hysteresis loop is rectangle type at T = 4.5 and turn into rounded type at T = 5.5. Moreover, for m A, at T = 6.1 value, the single hysteresis loop turns into a triple hysteresis loops, and for m B, it turns into a double hysteresis loops. Triple hysteresis loops consist of three loops in which one central loop and two of them are the inversions of each other about the origin. Note that the presence of central loop is the sign of the ferrimagnetic phase of the system, whether the system shows single and triple loops. While double loops occur under an ac field at temperature slightly above the Curie point, triple loops can develop at temperature slightly below the phase transition [14]. The physical mechanism of the presence of the multiple loops behaviors [15] and classification of hysteresis loop can be found at references [16, 17]. The double hysteresis loops have been observed experimentally in different systems [18–20].

Figure 2a illustrates the coercivity field versus scaled temperature for w = .05 π, d = 0.5, and h = 1.0. It can be seen from the figure that the coercive field decreases with increasing temperature up to certain values of temperature and, with further increase of temperature, coercivity field increases. With increasing temperature within the range 4–6, the remanent magnetization decreases. With the temperature range 7–9, the remanent magnetization is practically constant.

Fig. 2
figure 2

(Color online) a The coercivity field and b the remanent magnetizations, versus temperature for the same parameters as in Fig. 1

Full size image

In the following part of the study, in order to see the effect of the crystal field on the hysteresis behavior, we plot Fig. 3 for the parameters chosen as w = 0.05 π and h = 1.0. In Fig. 3, we depict the hysteresis loops calculated at the temperature T = 5.5 for crystal field values that are changing between −5.0 and 10.0. In this figure, we can see that with increasing crystal field, the area of hysteresis loops becomes bigger and the system displays a single hysteresis loop except for d = 0.5 values. At d = 0.5 value, the single hysteresis loop turns into a triple hysteresis loops. A similar observation has been reported by Akıncı [15] in a crystal field-diluted spin-1 Blume–Capel model. Broad hysteresis loops are seen at d = 1.6, and with further increase of crystal field parameter, the hysteresis loops are very slim. A narrow hysteresis loop implies a small amount of dissipated energy in repeatedly reversing the magnetization. Minimization of energy dissipation has AC electrical application.

Fig. 3
figure 3

Dynamic hysteresis behavior of the mixed-spin (1, 5/2) systems: a m A, b m B, and c m T for different crystal field values. We use w = 0.05 π, T = 5.5, and h = 1.0 Hamiltonian parameters

Full size image

Figure 4a illustrates the coercivity field and Fig. 4b the remanent magnetizations, versus the crystal field for the same parameters as in Fig. 3. It can be seen from the figure that the coercive field increases with increasing positive and negative crystal field values. The remanent magnetizations chance exponentially with increasing crystal field. There are various methods of chancing the coercivity and the remanent magnetizations of magnetic materials, all of which involve in the control of the magnetic domains within the material. Hard magnetic materials have much higher retentivity and coercivity which are useful in memory devices (audio recording, computer disk drives, magnetic cards, etc.).

Fig. 4
figure 4

(Color online) a The coercivity field and b the remanent magnetizations, versus crystal field for the same parameters as in Fig. 3

Full size image

Finally, to better understand the effect of the frequency on the hysteresis loop, we plot Fig. 5. In this figure, we focus our attention on the frequency dependence of the hysteresis loops for the fixed parameters: T = 5.5, d = 0.5, and h = 1.5. The results show some interesting features; as the frequency is increased, the area of hysteresis loops (and also the coercive field and remanent magnetization) is increased until ω reaches the critical value. With increasing of the frequency bigger than 0.06 π, the rectangular shape of the hysteresis loops disappears due to fluctuations. These results are in a good agreement with theoretical results [21].

Fig. 5
figure 5

The dynamic hysteresis behaviors at different frequency for the mixed-spin (1, 5/2) systems: a m A, b m B, and c m T. We use T = 5.5, d = 0.5, and h = 1.5 Hamiltonian parameters

Full size image

4 Conclusion

In this paper, we investigate the dynamic magnetic hysteresis properties in a two-dimensional mixed-spin (1, 5/2) Ising system on a square lattice. We investigated the effects of the temperature and crystal field on dynamic hysteresis properties, and the results are consistent with previous experimental and theoretical works for magnetic systems. The calculated results affirmed that the Hamiltonian parameters of the system affect strongly the hysteresis characteristics and thus can be controlled by these parameters. It is believed that the results could shed light on the development of magnetic device application.