1 Introduction

Optical dielectric relaxation is a crucial phenomenon that influences the overall performance of optical materials. This process involves the response of a dielectric material to an applied electric field, resulting in fluctuations of charge movement within the material. The intricate behavior of optical dielectric relaxation directly affects aspects of optoelectric conductivity, highlighting its importance in the development of efficient photonic devices. Furthermore, studying this phenomenon in depth will enable researchers to tailor material properties and optimize functionalities, paving the way for innovation within the field of optical materials. Optical and optoelectric conductivities are two of the most important parameters that characterize optical materials. Optical and optoelectric conductivities are two of the most important parameters that characterize optical materials [1,2,3]. These properties play a crucial role in determining the performance and efficiency of various devices, such as solar cells, photodetectors, and fiber-optic communication systems. Understanding the relationship between optoelectric conductivity and material composition is vital for developing new and improved materials with desired characteristics. Additionally, advancements in measurement techniques and theoretical models will continue to contribute toward a better comprehension of optoelectric conductivity in various materials [4, 5]. Optical characteristics of many materials, such as thin films, composites, nanopowder, chalcogenides, and oxide glasses, have recently attracted the attention of numerous researchers. This interest is brought on by the compounds' prospective uses in optoelectronic sensors, frequency converters, optical switching instruments, and other optical processing applications [6, 7]. The majority of such materials fall into the dielectric or semiconductor categories. There are two types of opto-structure interactions, linear or nonlinear, depending on how the electric field component of light interacts with polarons/polarizability (P) [8,9,10]. This study aims to identify the relations between electric and optical conductivities and the dielectric relaxation parameters, (dielectric constant and dielectric loss), of sodium borate glass.

2 Experimental description and theoretical procedures

2.1 Experimental work

Only one sample of the chemical composition (72 wt% B2O3–28 wt% Na2O) has been prepared using the fast cooling method. Both B2O3 and Na2O have been weighted then mixed well in a porcelain crucible before being placed in an electric oven set at 900 °C, for two hours. Thereafter, the melt quenched by pouring at room temperature in between two copper plates, where the resulted some of solids were adjusted for optical properties while some others were grinded to be suitable for XRD for the internal phase identification. The optical measurements were obtained using Cary 5000 Varian Double Beam UV–Vis-NIR Spectrometer, of resolution 2 nm.

2.2 Formulation of the problem

There are three ways that electromagnetic wave can interact with a dielectric solid sample: reflectance (R), absorbance (A), and transmittance (T). Where the resulting refractive index of a solid sample with an optical loss should be complex and dispersive (Eq. 1), and in turn composed of real part (refractive index n) and an imaginary part (absorption index K) [11, 12].

$${n}^{*} \left(\lambda \right)= n \left(\lambda \right)+j K (\lambda )$$
(1)

Such concept may be understood by considering the fact that a sort of polarization P proportional to the electric field component E and the average current density i through the sample is produced by incident electromagnetic radiation on a dielectric sample. The kind of electromagnetic waves that are present in a dielectric sample can be identified using Maxwell's equations (Eqs. 2–7) (MKS) [13, 14], where c and εo are the space light velocity and space permittivity.

$$\rho (\mathrm{density of the effective charge carriers})= - \nabla P$$
(2)
$$i (\mathrm{Average current density over the sample voume})= \frac{\partial P}{\partial t}$$
(3)
$$\nabla . E= - \frac{\nabla . P}{{\varepsilon }_{o}}$$
(4)
$$\nabla x E= - \frac{\partial B}{\partial t}$$
(5)
$$\nabla . B=0$$
(6)
$${c}^{2} \nabla x B= \frac{\partial }{\partial t} \left(\frac{P}{{\varepsilon }_{o}}+E\right)$$
(7)

These relationships state that the normal component of the electric field E is not conserved at the interfaces of materials of variable polarizability. So, the electric displacement parameter (Eq. 8) has been introduced as a conservative quantity across the interfaces' actions. The solution of Maxwell's equations resulted in the following plane harmonic waves, (Eqs. 9, 10), their traveling phase velocity vphase is described by Eq. 11, where n is the refractive index.

$$D= {\varepsilon }_{o}+P$$
(8)
$$E= {E}_{o} {e}^{j(\omega t-kr)} \, \text{where} \, k \, \text{is the wave vector}$$
(9)
$$H= {H}_{o} {e}^{j(\omega t-kr)}$$
(10)
$${\mathrm{v}}_{\mathrm{phase}}= \frac{\omega }{k}= \frac{c}{n}$$
(11)

In the case in which the optical absorption takes place, both the wave vector and the refractive index will be complex quantities, which confirms Eq. 1. Where. A common method based on non-destructive measurements of a solid sample's transmittance T (λ) and/or specular reflectance R (λ) over the ultraviolet, visible, and infrared (UV–Vis-IR) areas of the electromagnetic spectrum is widely used for the evaluation of n (λ) and K (λ) of a solid sample [15], as shown in Eqs. 12–14 where A (λ), α and d are the optical absorbance, absorption coefficient, and sample thickness [16].

$$n= \frac{1+ \frac{R}{4}}{1- \frac{R}{4}}+ \sqrt{\frac{R}{{(1-\frac{R}{4})}^{2}}- {(K)}^{2}} \, \text{Linear refractive index}$$
(12)
$$K= \frac{\alpha \lambda }{4\pi } , \, \text{absorption index}$$
(13)
$$\alpha =2.303 \frac{A}{t}$$
(14)

The optical dielectric relaxation ε* can express the loss of energy parameters (see Eq. 15 [17], which consists of a real and imaginary components ε1 and ε2. For unfree damper, the real component (ε1) characterizes the damping of the light propagation through the medium. While the imaginary component is considered as a damping factor describes the amount of energy loss/absorbed within the medium [18, 19].

$${\varepsilon }^{*}= {\varepsilon }_{1}+j{\varepsilon }_{2}$$
(15)

According to Eq. 16 the complex dielectric constant could be expressed in terms of the complex refractive index n* which can be defined as formulated in Eq. 17.

$${\varepsilon }^{*}= {n}^{*2}$$
(16)

where

$${n}^{*}= n +jK$$
(17)

Solving of Eqs. 16 and 17 gives Eqs. 18 and 19

$${\varepsilon }_{1}=\left({n}^{2}- {K}^{2}\right)$$
(18)
$${\varepsilon }_{2}=2n K$$
(19)

2.3 Mathematical derivation

A material's optical conductivity (optoelectronic conductivity) is the relationship between the induced current density and the strength of the generated electric field component of the light. While the ability of a material to carry electricity is known as electrical conductivity, which refers to the ratio between electric field strength and current density. The objective of the current study is to create a novel approximation that precisely links the optical and opto-electric conductivities to the real and imaginary parts of the dielectric constant. Where it’s well known that the conductivity is a complex quantity which proportional to both the angular frequency of the electromagnetic wave and the dielectric relaxation of the medium (Eq. 20) [16, 20].

$${\sigma }^{*} \sim \omega {\varepsilon }^{*}$$
(20)

Mathematically, relation 20 could be re-formulated in the form of Eq. 21, where σ1 and σ2 are the real and imaginary conductivities, respectively.

$${\sigma }_{1}+j{\sigma }_{2}= {C}_{1}\omega {\varepsilon }_{1}+ j{C}_{2}{\omega \varepsilon }_{2}$$
(21)

Using Eqs. 18 and 19 in Eq. 21 lead to new two Equations, Eqs. 22 and 23.

$${\sigma }_{1}= {C}_{1}\omega {\varepsilon }_{1}={C}_{1}\omega \left({n}^{2}- {K}^{2}\right)$$
(22)
$${\sigma }_{2}= {C}_{2}{\omega \varepsilon }_{2}=2{C}_{2} \omega nK$$
(23)

3 Results and discussion

3.1 Internal structural nature identification

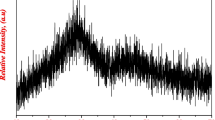
As shown in Fig. 1, the X-ray diffraction XRD pattern shows just only two broad humps without the existence of any sharp peaks which reveals the amorphous nature of the prepared sample. Accordingly, the prepared sample represents a glass solid which makes it an optical material suitable for the current study.

Fig. 1
figure 1

XRD pattern of the prepared sample

Full size image

3.2 Optical characterization

The measured optical parameters A, T%, and R% have been used to extract the refractive and absorption indices of the prepared glass sample according to the well know Eqs. 24 and 25 [16]. The obtained n-values and K-values, in turn, have used to check the validity of the Eqs. 22 and 23, where the values of σ1 and σ2 were calculated using random values for both C1 and C2, as shown in Figs. 2 and 3. On the other side n and K have been used to obtain the optical and electric conductivities using Eqs. 26 and 27 [16], as shown in Figs. 4 and 5.

Fig. 2
figure 2

σ1 versus the wavelength for sodium borate glass sample (72 wt% BO. 28 wt% Na2O), at three different randomly C1-values (0.1, 0.2, and 0.3)

Full size image
Fig. 3
figure 3

σ2 versus the wavelength for sodium borate glass sample (72 wt.% BO. 28 wt.% Na2O), at three different randomly C2-values (0.1, 0.2, and 0.3)

Full size image
Fig. 4
figure 4

σopt versus the wavelength for sodium borate glass sample (72 wt% BO. 28 wt% Na2O)

Full size image
Fig. 5
figure 5

σopt-electric versus the wavelength for sodium borate glass sample (72 wt% BO. 28 wt% Na2O)

Full size image
$$n= \frac{1+ \frac{R}{4}}{1- \frac{R}{4}}+ \sqrt{\frac{R}{{(1-\frac{R}{4})}^{2}}- {(K)}^{2}}$$
(24)
$$K= \frac{\alpha \lambda }{4\pi }= 2.303 \,\, \frac{A}{t} \,\, \frac{\lambda }{4\pi }$$
(25)
$${\sigma }_{\mathrm{opt}}= \frac{\alpha n c}{4\pi }$$
(26)
$${\sigma }_{\mathrm{opoelec}}= \left(\frac{n}{K}\right){\sigma }_{\mathrm{opt}}$$
(27)

By inspecting and comparing Figs. 2, 3, 4, and 5, it's clear that Figs. 2 and 5 show the same behavior as well as the same order of magnitude. Also, Figs. 3 and 4 show the same behavior as well as the same order of magnitude. Interestingly, it can be stated that σ1 and σ2 could refer to the σoptoelectric and σoptical conductivities, respectively.

Now to estimate the values of the unknown constants C1 and C2, the authors using the numerical analysis to simulate the experimental obtained data as shown in Figs. 6 and 7. The simulation process resulted in determining the magnitudes of the dimensionless constants C1 and C2, where it was found that C1 = 1/2 C2, C2 = 1/12.5, and hence Eqs. 24 and 25 will take the forms of Eqs. 28, 29 and 30, respectively.

Fig. 6
figure 6

Simulation between σ2 and σopt

Full size image
Fig. 7
figure 7

Simulation between σ1and σopt-electric

Full size image
$${\sigma }_{optoelec}=\frac{1}{6.25}{\omega \varepsilon }_{1}$$
(28a)
$${\sigma }_{optoelec}=\frac{1}{6.25}\omega \left({n}^{2}\right) where {K}^{2} \ll {n}^{2} ({s}^{-1})$$
(28b)
$${\sigma }_{opt}=\frac{1}{12.5}{\omega \varepsilon }_{2}$$
(29a)
$${\sigma }_{opt}=\frac{1}{6.25}\omega nK ({s}^{-1})$$
(29b)

Equations 28 and 29 are dimensional correct, where Eq. 28 indicates the dependence of the opto-electrical conductivity on the behavior of the real refractive index and then on the behavior of the optical reflectance. While, Eq. 29 shows that the optical conductivity depends on the values of both the refractive and absorption indices (n and K), which means its dependence on the amount of the energy loss within the optical medium.

4 Conclusion

A pure sodium borate glass sample was prepared as an optical material, and then it was exposed to UV–vis. electromagnetic radiation. The recorded optical parameters in the range of 400–800, besides a theoretical modulation process, have been used to estimate new mathematical formulas to describe and calculate the optical and optoelectric conductivities as functions of the dielectric relaxation parameters and/or the dispersion coefficients were developed. Results from the recently devolved equations were very consistent with those from the previously published equations. The newly created equations show that optoelectric depends on the real component of the optical complex dielectric constant, whereas optical conductivity depends on the imaginary part. Such a finding may be regarded as advantageous and contribute to a thorough comprehension of the parameters.