Exploring the Effects of Intrinsic Decoherence on Quantum-Memory-Assisted Entropic Uncertainty Relation in a Heisenberg Spin Chain Model

Abstract

In this paper, we investigate the influence of the intrinsic decoherence on the Quantum-Memory-Assisted Entropic Uncertainty Relation (QMA-EUR) and quantum correlations in a two-qubit XX Heisenberg spin chain coupled to Dzyaloshinskii-Moriya (DM) interaction and subjected to an external non-homogeneous magnetic field. To quantify the quantum correlations presented in our bipartite system, we consider the local quantum uncertainty (LQU) to measure discord-like correlations and the concurrence (C) to detect entanglement. Then, we have revealed that quantum correlations and entropic uncertainty are typically anticorrelated when the system is prepared initially in a pure state. Significantly, we show promising features on the behaviours of quantum correlations and the entropic uncertainty in terms of the system parameters in a variety of initial states. Indeed, we can improve quantum correlations and reduce entropic uncertainty with the control of both DM interaction and the external magnetic field, which is of fundamental importance for quantum precision measurement during quantum information processing, particularly in the context of spin solid-state.

1 Introduction

Incompatible measurements are those that cannot be performed simultaneously with arbitrary precision. The Heisenberg uncertainty principle [1] explains that the position and momentum measurements of a quantum particle are incompatible, which is expressed mathematically as ${\Delta } X\cdot {\Delta } P_{x} \geq \frac {\hbar }{2}$ [2]. In other words, the measurement of one of the two observables disturbs the result of the measurement of the other. This is why one can never simultaneously attribute a defined position and momentum to a particle. Then, regarding two arbitrary incompatible observables Q and R, Kennard [3] and Robertson [4] had generalized this principle to a standard deviation expressed as follows:

$$ {\Delta} Q\cdot {\Delta} R \geq \frac{1}{2}|\langle[Q,R]\rangle| $$

(1)

where ${\Delta } Q=\sqrt {\langle Q^{2}\rangle -\langle Q \rangle ^{2}}$, ${\Delta } R=\sqrt {\langle R^{2}\rangle -\langle R \rangle ^{2}}$ denote the mean quadratic deviations (i.e., the variances) of Q and R respectively and |〈[Q,R]〉| is the mean value of the commutator [Q,R] = QR − RQ in a quantum state |ψ〉. However, the lower bound of inequality 1 is state-dependent; that is if the system is prepared in one of the eigenvectors of Q or R, the inequality will be trivial and [Q,R] = 0. To overcome this deficiency, a new approach to characterize the uncertainty relation, the so-called Entropic Uncertainty Relation (EUR), has been imposed within the framework of quantum information theory as a fundamental concept of measurement processes, and which has led to important applications in this field [5].

The EUR has been proposed by Deutch [6] and then simplified by Kraus [7]. Finally, Maassen and Uffink [8] rewrote an uncertainty relation in terms of Shannon entropy in the following form:

$$ H(Q) + H(R) \geq \log_{2} \frac{1}{c} $$

(2)

where $H(X)= -{\sum }_{m} p_{m} \log _{2} p_{m}$ is the Shannon entropy of the observable X ∈{Q,R}, $p_{m}={\langle \phi ^{X}_{m}}|\rho |{\phi ^{X}_{m}}\rangle$ is the probability of obtaining the m-th outcome, $|{\phi ^{X}_{m}}\rangle$ denoting the eigenvectors of the observables X [9, 10]. The parameter $c \equiv max_{j,k}\lbrace |\langle {\varphi ^{Q}_{j}}|{{\Psi }^{R}_{k}}\rangle |^{2}\rbrace$ is the maximal overlap of the observables Q and R , where $|{\varphi ^{Q}_{j}}\rangle$ and $|{{\Psi }^{R}_{k}}\rangle$ are the eigenvectors of Q and R respectively.

Recently, EUR in the presence of quantum memory was presented by Berta et al. [11] and confirmed experimentally (See Refs. [12, 13]). Also, the new relationship can be interpreted as an uncertainty game between two recognized participators, Alice and Bob. At first, Bob prepares a pair of initially entangled state AB, and then he transmits A related to quantum memory B to Alice. Next, Alice performs a measurement on A with Q or R and announces her measurement decision to Bob via a conventional communication channel. Bob’s task is to estimate as accurately as possible the measurement outcome. Therefore, the new uncertainty principle is known as the Quantum-Memory-Assisted Entropic Uncertainty Relation (QMA-EUR) and given by [11]:

$$ S(Q|B)+S(R|B)\geq S(A|B)+\log_{2}\frac{1}{c} $$

(3)

Where, S(A|B) = S(ρ^AB) − S(ρ_B) represents the conditional von Neumann entropy of the post-measurement state $\rho ^{XB}={\sum }_{o} ({{\Pi }_{o}^{X}}\otimes \mathbb {I_{B}})\rho ^{AB}({{\Pi }_{o}^{X}}\otimes \mathbb {I_{B}})$ with X ∈{Q,R}, and $S(\rho )=-Tr[\rho \log _{2} \rho ]$ [14, 15], ${{\Pi }_{o}^{X}} = |{\phi ^{X}_{o}}\rangle \langle {\phi ^{X}_{o}}|$ is the projector on the eigenspace of X with obtained outcome, and $\mathbb {I_{B}}$ is the identity operator of the Hilbert space ${\mathscr{H}}_{B}$ of B. To compare the inequality 3 with inequality 2, we can deduce that the lower bound given on the Right-Hand Side (RHS) can be minimized if the measured particle A and the particle serving for memory B are entangled since S(A|B) is negative, so Bob’s uncertainty on Alice’s measurements could be reduced. Notably, if the particles are maximally entangled, i.e., S(A|B) = − 1, and if we admit c = 1/2 (such that the observables are those of Pauli), the RHS of inequality 3 is equal to zero. As a result, Bob will perfectly guess Alice’s measurement results.

In addition, the lower bounds are improved by several authors. For example, Pati et al. [16] have suggested that the lower bound of the EUR can be tightened by quantum discord and classical correlations. Further, Pramanik et al. [17] reported a new form of EUR via extractable classical information.

Entanglement represents one of the most significant revolutions in quantum physics [18, 19]. This revolution fascinated many physicists, because of the counter-intuitive notion known by the non-locality [20] of quantum systems, which had caused, at the time, a strong suspicion on the part of A. Einstein [21]. This remarkable property allows two particles to be mysteriously linked whatever the distance between them. Therefore, it becomes impossible to describe each particle independently. The most complete description of the individual subsystems is then an entangled state which contains all the information about these particles.

To detect the quantumness of correlations which are beyond entanglement, many quantifiers have been introduced, and for instance; the local quantum uncertainty (LQU) [22,23,24]. it is a powerful discord-type quantifier that satisfies all the requirements for measuring quantum correlations. Besides, LQU is easily computable, which is not always the case for other quantifiers such as quantum discord [25,26,27] or its geometric variant [28]. Recently, LQU has been used as a quantum criticality witness in multipartite spin systems and the coherence based measure of quantum correlations induced by the Wigner-Yanase skew information [29,30,31]. It is also a key tool in quantum metrology protocols alongside quantum Fisher information [32,33,34,35,36].

Nevertheless, it is difficult to preserve all the time quantum correlations presented in quantum systems, due to the decoherence phenomenon [37], which constitutes a major obstacle in the advancement of quantum information devices [38,39,40,41].

However, it has been recognized that Heisenberg spin chains are promising systems in the field of condensed matter physics [42,43,44], especially for the physical realizations of quantum information processing tasks, where the spins constitute the qubits coupled by the exchange interaction and which are used in the manufacture of quantum computers [45, 46]. Various works have been achieved based on Heisenberg spin chain models to examine QMA-EUR with an inhomogeneous magnetic field along the z-axis and the control of coupling strength between the two spins [47, 48].

In the dynamics of the quantum spin system, the Dzyaloshinskii-Moriya (DM) interaction [49, 50] is a vital spin interaction, which arises from the consideration of spin-orbit coupling effects and can be described by the following expression

$$ H_{DM}\sim \overrightarrow{D}.(\overrightarrow{\sigma_{1}}\times\overrightarrow{\sigma_{2}}),$$

where $\overrightarrow {D}=(D_{x},D_{y},D_{z})$ denotes the DM coupling vector and ${\sigma _{i}^{x}},{\sigma _{i}^{y}}$, and ${\sigma _{i}^{z}}$ are the Pauli matrices associated to the two qubits (i = 1,2).

In this context, Zheng et al. investigated the consequences of mixedness and entanglement on the lower bound and thinness of entropic uncertainty in a Heisenberg model with a DM interaction in the z-axis [51]. Moreover, Wang et al. studied the relationship between quantum coherence and uncertainty relations [52]. Besides, Y. Zhang et al. analyzed QMA-EUR in a two-qubit XX Heisenberg model with DM interaction and an external magnetic field [43]. They also revealed the dynamics of entropic uncertainty and its lower bound in the presence of intrinsic decoherence and noisy environment [53]. The same authors studied the dynamics of QMA-EUR in the XY spin chain environments with DM interaction (See Ref. [54]). N. Zidan [55] examined the effect of long-range interactions on entropic uncertainty in the XY spin chain model coupled to a magnetic field and a DM interaction.

In this work, using Milburn’s dynamical master equation, we investigated the effect of intrinsic decoherence on quantum-memory-assisted entropic uncertainty (QMA-EUR) in a Heisenberg spin system. To clear, we quantify the quantum correlations by local quantum uncertainty (LQU) and the entanglement quantified by the concurrence (C) in a two-qubit XX Heisenberg spin chain model initially prepared in the pure state. Here, we shed light on the effects of physical parameters on the studied system, in particular the nonuniform external magnetic field and the Dzyaloshinkii-Moriya (DM) interaction both directed along the z-axis on our proposed system. This paper is structured as follows. In Section 2, we give the Hamiltonian of the system and evaluate its density matrix as a function of time. In Section 3, we present the properties of QMA-EUR of Pauli observables and the analytical expressions of uncertainty relations. In Section 4, we provide and discuss our results. Finally, we end the paper with conclusions.

2 Theoretical Model

2.1 Hamiltonian

To start, we introduce the Hamiltonian of the two-qubit (spin-$\frac {1}{2}$) XX Heisenberg spin chain model with a Dzyaloshinskii-Moriya interaction (D_z) and non-homogeneous magnetic field (b) both directed along the z-axis which is given by :

$$ H^{AB}=-J(\sigma_{+}^{A}\sigma_{-}^{B}+\sigma_{-}^{A}\sigma_{+}^{B})-\frac{D_{z}}{2}(\sigma _{x}^{A}{\sigma_{y}^{B}}-{\sigma_{y}^{A}}{\sigma_{x}^{B}})-b({\sigma_{z}^{A}}-{\sigma_{z}^{B}}), $$

(4)

where J denotes the coupling constant, $\sigma _{\pm } =\frac {1}{2}({\sigma _{x}\pm i\sigma _{y}})$ are the raising and lowering Pauli operators, ${\sigma ^{j}_{x}}, {\sigma ^{j}_{y}}$ and ${\sigma ^{j}_{z}}$ are the standard Pauli matrices, b is the component along the z-axis of the non-uniform external magnetic field acting on the qubit j(j = A,B). The eingenvalues and the corresponding eigenstates of the Hamiltonian are given by :

$$ E_{1} = 2b, |\varphi_{1}\rangle= cos \left( \frac{\theta_{1}}{2}\right) |00\rangle+sin\left( \frac{\theta_{1}}{2}\right) |11\rangle; $$

(5)

$$ E_{2} = - 2b, |\varphi_{2}\rangle= sin \left( \frac{\theta_{1}}{2}\right) |00\rangle-cos\left( \frac{\theta_{1}}{2}\right) |11\rangle, $$

(6)

$$ E_{3} = \chi , |\varphi_{3}\rangle= cos \left( \frac{\theta_{2}}{2}\right) |01\rangle+e^{-i\varphi} sin\left( \frac{\theta_{2}}{2}\right) |10\rangle, $$

(7)

$$ E_{4} = - \chi , |\varphi_{4}\rangle= sin \left( \frac{\theta_{2}}{2}\right) |01\rangle-e^{-i\varphi} cos\left( \frac{\theta_{2}}{2}\right) |10\rangle, $$

(8)

where 𝜃₁ = kπ / $k \in \mathbb {Z}, tan\left (\theta _{2} \right ) = \frac {\chi }{2 b},$ $tan \left (\varphi \right ) = \frac {D_{z}}{2 J}$ and $\chi = \sqrt {{D_{z}^{2}} + 4 J^{2}}$.

2.2 Intrinsic Decoherence

To take into account the intrinsic decoherence, Milburn [56] had introduced a straightforward adjustment of conventional quantum mechanics by assuming that over sufficiently short time steps, the system does not evolve continuously under unitary evolution, but rather in a stochastic sequence of identical unitary transformations. This assumption led to a modification of the Schrödinger’s equation by incorporating a term responsible for the decay of quantum coherence in the energy eigenstate basis, without any intervention of a reservoir and thus without the usual energy dissipation associated with normal decay. Milburn obtained the subsequent master equation given by

$$ \frac{d\rho^{AB}(t)}{dt}=-i\left[H^{AB},\rho^{AB}(t)\right]-\frac{\gamma}{2}\left[H^{AB},\left[H^{AB},\rho^{AB}(t)\right]\right], $$

(9)

Here, H^AB is the Hamiltonian of the system, ρ^AB(t) denotes the state of the system and γ is the phase decoherence rate. In the right part of (9), the first term generates a coherent unitary time evolution of the system, while the second term, which does not commute with the Hamiltonian represents the decoherence effect on the system. The formal solution of the above equation can be written in operator-sum representation using the Kraus operators M^k as [41, 57, 58]

$$ \rho^{AB}(t) = \sum\limits_{k=0}^{\infty} \frac{t^{k}}{k!}M^{k}(t) \rho^{AB}(0)M^{\dagger k}(t), $$

(10)

where ρ^AB(0) is the density matrix of the initial state and M^k(t) is defined by

$$ M^{k}(t) = H^{k} e^{-iH^{AB}t}e^{-\frac{t \gamma (H^{AB})^{2}}{2}}, $$

(11)

Then, the resulted state can be written as

$$ \rho^{AB}(t) = \sum\limits_{\substack{m,n}}\exp \left[ -\frac{\gamma t}{2}(E_{m} - E_{n})^{2} - i(E_{m} - E_{n})t \right]\\ \times \langle\varphi_{m} |\rho^{AB}(0)|\varphi_{n}\rangle|\varphi_{m}\rangle\langle\varphi_{n}|, $$

(12)

E_m,n and φ_m,n are respectively the eigenvalues and the eigenstates of the Hamiltonien of the system, respectively.

Thereafter, we assume that the system is initially prepared in the pure state:

$$ \rho^{AB}(0) = |\psi_{p}^{AB}(0)\rangle\langle\psi_{p}^{AB}(0)| $$

(13)

where $|\psi _{p}^{AB}(0)\rangle =\sqrt {p}|01\rangle + \sqrt {1 - p}|10\rangle$, p ∈ [0,1] being the degree of entanglement. In the standard basis {|00〉,|01〉,|10〉,|11〉}, ρ^AB(0) can be written as :

$$ \rho^{AB}(0)=\left( \begin{array}{cccc} 0 & 0 & 0 & 0 \\ 0 &p & \sqrt{p(1-p)} & 0 \\ 0 & \sqrt{p(1-p)} &1-p & 0 \\ 0 & 0 & 0 & 0 \end{array} \right) $$

(14)

From (12), the time evolution of ρ^AB(t) in the standard basis {|00〉,|01〉,|10〉,|11〉} can be expressed as :

$$ \rho^{AB}(t)=\left( \begin{array}{cccc} 0 & 0 & 0 & 0 \\ 0 &\rho_{22}(t) & \rho_{23}(t) & 0 \\ 0 & \rho_{32}(t) &\rho_{33}(t) & 0 \\ 0 & 0 & 0 & 0 \end{array} \right) $$

(15)

The diagonal elements of ρ^AB(t) are:

$$ \begin{array}{@{}rcl@{}} \rho_{22}(t)&=& e^{-2\gamma \chi^{2} t} \frac{sin\theta_{2}}{2}\left[(2p-1) sin(\theta_{2})cos(2\chi t)+2\sqrt{p(1-p)}\vphantom{\frac{\theta_{2}}{2}}\right.\\&&\left.\left( sin^{2}\left( \frac{\theta_{2}}{2}\right)cos(\varphi-2\chi t)-cos^{2}\left( \frac{\theta_{2}}{2}\right)cos(\varphi+2\chi t)\right)\right]+ p\left( 1-\frac{sin^{2}\theta_{2}}{2} \right)\\&&+(1-p)\frac{sin^{2}(\theta_{2})}{2} + \sqrt{p(1-p)}\frac{sin(2\theta_{2})}{2}cos\varphi ;\\ \rho_{33}(t)&=& -e^{-2\gamma \chi^{2} t} \frac{sin\theta_{2}}{2}\left[\left( 2p-1\right) sin(\theta_{2})cos(2\chi t)+2\sqrt{p(1-p)}\vphantom{\frac{\theta_{2}}{2}}\right.\\&&\left.\left( sin^{2}\left( \frac{\theta_{2}}{2}\right)cos(\varphi-2\chi t)-cos^{2}\left( \frac{\theta_{2}}{2}\right)cos(\varphi+2\chi t)\right)\right]\\&&+ (1-p)\left( 1 - \frac{sin^{2}\theta_{2}}{2} \right)+p\frac{sin^{2}\theta_{2}}{2}+\sqrt{p(1-p)}\frac{sin(2\theta_{2})}{2}cos\varphi; \end{array} $$

and the off-diagonal elements are:

$$ \begin{array}{@{}rcl@{}} \rho_{23}(t) \!&=&\! \rho_{32}^{*}(t) = e^{-2\gamma \chi^{2} t} e^{i \varphi}\frac{sin\theta_{2}}{2}\left[(2p-1)\left( e^{2i \chi t}sin^{2}\left( \frac{\theta_{2}}{2}\right)- e^{-2i \chi t}cos^{2}\left( \frac{\theta_{2}}{2}\right)\right)\right.\\&&\left.\!+\sqrt{p(1 - p}\left( e^{-i(\varphi-2\chi t)}\mathit{sin}^{4}\left( {}\frac{\theta_{2}}{2}{}\right) + e^{-i(\varphi+2\chi t)}\mathit{cos}^{4}\left( {}\frac{\theta_{2}}{2}{}\right) - \frac{\mathit{sin}^{2}\theta_{2}}{2}e^{i\varphi}\mathit{cos}(2\chi t)\!\right)\!\right]\\&&\!+e^{i\varphi}\frac{sin(2\theta_{2})}{4}\left( 2p-1\right)+e^{i\varphi}\sqrt{p(1-p)}sin^{2}\theta_{2} cos\varphi. \end{array} $$

2.3 Local Quantum Uncertainty (LQU)

Local quantum uncertainty (LQU) quantifies the minimum quantum uncertainty due to a measurement of a local observable on a quantum state [24]. For a bipartite quantum state ρ^AB, LQU is a discord-type quantifier of quantum correlations which is defined by minimizing the the Wigner-Yanase skew information over all local observables K_A acting on qubit A as:

$$ LQU (\rho^{AB}) = \mathscr{U}(\rho^{AB}) \equiv \min_{K_{A}} \mathscr{I}(\rho^{AB}, K_{A} \otimes \mathbb{I}_{B}), $$

(16)

where $\mathbb {I}_{B}$ is the identity operator, while the term:

$$ \mathscr{I}(\rho^{AB}, K_{A} \otimes \mathbb{I}_{B})=-\frac{1}{2}\text{Tr}([\sqrt{\rho^{AB}}, K_{A} \otimes \mathbb{I}_{B}]^{2}) $$

(17)

is the skew information [31, 59] which is an uncertainty relation introduced to provide the statistical nature of measurement errors [35, 60]. By carrying out a minimization on the set of all observables acting on qubit A, we can derive the analytical expression of LQU, for qubits (spin-$\frac {1}{2}$ particles), in the following form [24]:

$$ LQU(\rho^{AB}) = 1 - \max\{ \mu_{1}, \mu_{2}, \mu_{3}\}, $$

(18)

where μ₁,μ₂ and μ₃ are the eigenvalues of the 3 × 3 matrix W defined by its matrix elements:

$$ \omega_{ij} \equiv \text{Tr}\{\sqrt{\rho^{AB}}(\sigma_{i}\otimes \mathbb{I}_{2})\sqrt{\rho^{AB}}(\sigma_{j}\otimes \mathbb{I}_{2})\}, $$

(19)

with i,j = 1,2,3 and σ_i are the usual Pauli matrices.

2.4 Concurrence (C)

The concurrence (C) is a reliable measurement quantifying the amount of entanglement in the system under study. For any bipartite state ρ^AB, C is defined by Wooters formlua [61]:

$$ C (\rho^{AB}) = max \lbrace0, 2{\Lambda}_{max} - \sum\limits_{\substack{i=1}}^{4} {\Lambda}_{i}\rbrace $$

(20)

where Λ_i(i = 1,2,3,4) are the square roots of the eigenvalues of the marix $R = \rho ^{AB} ({\sigma _{y}^{A}} \otimes {\sigma _{y}^{B}}) \rho ^{*AB}({\sigma _{y}^{A}} \otimes {\sigma _{y}^{B}})$. ρ^AB is the density matrix of the bipartite system; it can be either pure or mixed. ρ^∗AB denotes the complex conjugation of ρ^AB in the standard basis. C = 0 corresponds to unentangled state and C = 1 to a maximally entangled state.

3 Quantum-Memory-Assisted Entropic Uncertainty Relation (QMA-EUR)

To probe the dynamical nature of QMA-EUR in the two-qubit spin-$\frac {1}{2}$ Heisenberg XX spin chain, we consider Q ≡ σ_x and R ≡ σ_z as two incompatible observables. After having measured the two Pauli observables, the reduced density operators of the system are therefore respectively

$$ \rho_{\sigma_{x} B}=\frac{1}{2}\left( \begin{array}{cccc} \rho_{33}(t) & 0 & 0 & \rho_{23}(t) \\ 0 &\rho_{22}(t) & \rho_{32}(t) & 0 \\ 0 & \rho_{23}(t) &\rho_{33}(t) & 0 \\ \rho_{32}(t) & 0 & 0 & \rho_{22}(t) \end{array} \right) $$

(21)

$$ \rho_{\sigma_{z} B}=\left( \begin{array}{cccc} 0 & 0 & 0 & 0 \\ 0 &\rho_{22}(t) & 0 & 0 \\ 0 & 0 &\rho_{33}(t) & 0 \\ 0 & 0 & 0 & 0 \end{array} \right) $$

(22)

ρ₂₂(t),ρ₃₃(t),ρ₃₂(t), and ρ₂₃(t) are defined in (15). Then, the von Neumann entropies are given by

$$ S(\rho_{\sigma_{x} B})=S_{bin}\left( \frac{1-\sqrt{1-4\varpi}}{2} \right)+1 $$

(23)

$$ S(\rho_{\sigma_{z} B})=-\rho_{22}(t)\log_{2}\rho_{22}(t)-\rho_{33}(t)\log_{2}\rho_{33}(t) $$

(24)

where S_bin denotes the binary entropy expressed as $S_{bin}({\Xi })=-{\Xi } \log _{2} {\Xi } -(1-{\Xi })\log _{2} (1-{\Xi })$ and ϖ = ρ₂₂(t)ρ₃₃(t) −|ρ₂₃(t)|².

Due to ρ^B = Tr_A(ρ^AB), the eigenvalues of the reduced density matrix B are ρ₂₂ and ρ₃₃, the Left-Hand Side (LHS) of inequality 3 is

$$ U_{L}=S(\rho_{\sigma_{x} B})+S(\rho_{\sigma_{z} B})-2S_{bin}(\rho_{33}(t)) $$

(25)

Returning to (15), one can obtain the eigenvalues of ρ^AB(t) as $\lambda _{1,2}^{AB}=\frac {1}{2}(\tau \pm \sqrt {\tau ^{2}-4\rho _{22}(t)\rho _{33}(t)+4|\rho _{23}(t)|^{2}})$ and $\lambda _{3,4}^{AB}=0$ with τ = ρ₂₂(t) + ρ₃₃(t). Since the overlap c of two Pauli observables is always equal to $\frac {1}{2}$, therefore the lower bound (RHS) of (3) can be expressed analytically as

$$ U_{R}=1-S_{bin}(\rho_{33}(t))-\lambda_{1}^{AB} \log_{2} \lambda_{1}^{AB} -\lambda_{2}^{AB}\log_{2} \lambda_{2}^{AB}. $$

(26)

By substituting the matrix elements of ρ^AB(t) (15) by their expressions into (25) and (26), we can derive the analytical expressions of U_L and U_R corresponding to the situation considered here.

4 Results and Discussion

In this section, we report the variations of the dynamics of the uncertainties U_L, U_R and quantum correlations captured by the local quantum uncertainty LQU, concurrence C, in the Heisenberg XX spin chain model taking into consideration the intrinsic decoherence. Indeed, we examine the influence of the Dzyaloshinkii-Moriya (D_z) interaction and external non-uniform magnetic field (b) on the entropic uncertainty and quantum correlations existing in the system. So, our study will focus on three particular cases of the initial pure state, namely the uncorrelated state, the correlated state, and the maximally correlated state (Bell-state).

Figure 1, shows at t = 0, the anti-correlation of the uncertainties U_L and U_R with LQU and C, so that LQU and C increase when the degree of entanglement p increases, while the uncertainties (U_L ≡ U_R) decrease. Note that C always prevails over LQU. On the other hand, the uncertainties are zero, when the value of p = 0.5 corresponding to the maximally entangled state (Bell-state), while LQU and entanglement reach their maximum and are equal to 1. This indicates that the owner of the memory particle (Bob) can perfectly predict the result of Alice’s measurement. For p = 0 or p = 1 (separable state), we notice the absence of quantum correlations and entanglement (LQU = C = 0) and the uncertainty U_L and its lower bound U_R are equal to 1.

Fig. 1

Plots of LQU, C, U_L and U_R as a function of p

Usefully, U_L ≡ U_R for all p-values, indicating that Berta’s lower bound of inequality 3 is a good quantifier for predicting the result of the measurement when the state is prepared initially in the pure state. Since this characteristic is symmetrical for p = 0.5, consequently, we will restrict our investigation in the range of p ∈ [0,0.5].

In Fig. 2, we introduce the effect of intrinsic decoherence by fixing the value of the decoherence rate γ = 0.02 without DM interaction or external magnetic field b. We plot the temporal evolution of LQU, C and uncertainties (U_L ≡ U_R) for different values of the parameter p, which allows us to display different features of these quantifiers.

Fig. 2

Time evolution of LQU, C and entropic uncertainties U_L ≡ U_R as a function of time t. In all plots we set J = 0.5, D_z = 0,b = 0 and γ = 0.02: (a) p = 0, (b) p = 0.25, and (c) p = 0.5

In Fig. 2a, where we set p = 0, even if the initial state ρ^AB(0) = |10〉〈10| is separable, we show that the system has a capacity to produce an influential entanglement manifested by the oscillations of concurrence C during time t which are finally annihilated when $t \longrightarrow \infty$. On the other hand, we note that LQU keeps a zero value whatever t. Meanwhile, the uncertainties (U_L ≡ U_R), which initially start from the value 1, show oscillations anti-correlated to those exhibited by the concurrence C before stabilizing at the value 1.

Based on this analysis, we assert that the uncertainties of measurement are significantly anti-correlated to the entanglement created by the system and not related to the quantum correlations quantified by LQU.

In the case of a correlated initial state (p = 0.25), Fig. 2b illustrates the improvement of the initial values of the quantum correlations (LQU ≈ 0.75) and of the entanglement (C ≈ 0.87). The concurrence C oscillates and reaches its steady-state ($t \longrightarrow \infty$) at the same initial value, while LQU decreases, without oscillations, with time to finally reach its steady-state at the value of 0.5. On the other hand, one notes the evolution of uncertainties starting from the initial value U_L ≡ U_R ≈ 0.2, in an oscillatory way to reach a steady-state at the value 0.35 when $t\longrightarrow \infty$. Likewise, the oscillatory behavior of the concurrence C and the uncertainties unveils the anti-correlated relationship between these quantities.

Now, by setting p = 0.5, we consider the maximally entangled (correlated) state $|\psi ^{AB}(0)\rangle =\frac {1}{\sqrt {2}}(|01\rangle +|10\rangle )$. As shown in Fig. 2c, the uncertainties accompanying the measurement are zero (i.e., U_L ≡ U_R = 0) while the quantum correlations and entanglement in the system are maximum (i.e., LQU = C = 1) whatever the time t. Therefore, all of these quantities are immune to the effect of intrinsic decoherence in that particular state.

In Fig. 3, we are interesting in the effects at the same time of the Dzyaloshinskii-Moriya interaction (D_z = 1) and the intrinsic decoherence (γ = 0.02) on the dynamics of quantum correlations (LQU and C) and entropic uncertainties (U_L ≡ U_R) in the system. By examining the separable state (p = 0) object of the study presented in Fig. 3a, and as we reported in our recent article [44], we notice a sudden creation of LQU which is manifested by the same oscillatory behavior of concurrence C but with extremely low maxima which disappear more quickly. Also, the uncertainties U_L ≡ U_R and concurrence C reach their steady-states at a shorter time compared to the case where D_z = 0 in Fig. 2a.

Fig. 3

Time evolution of LQU, C and entropic uncertainties U_L ≡ U_R as a function of time t. In all plots we set J = 0.5, D_z = 1,b = 0 and γ = 0.02: (a) p = 0, (b) p = 0.25, and (c) p = 0.5

To increase the degree of entanglement, we set the value p = 0.25, i.e., by choosing a system prepared initially in a correlated state, we see an obvious impact of the DM interaction (Fig. 3b). Besides, we first notice the rise in the amplitudes of the oscillations of the quantities LQU, C, and U_L ≡ U_R. The local quantum uncertainty (LQU) undergoes sudden death at a very early time t before it stabilizes and reaches its steady-state at the value LQU ≈ 0.1 which is very small compared to the case where D_z = 0 (Fig. 2b). In addition, the concurrence C exhibits a decreasing oscillatory behavior and reaches its steady-state at the value C ≈ 0.61 after a short time compared to the case where DM interaction is absent (Fig. 2b), while the uncertainties U_L ≡ U_R exhibit an ascending oscillatory behavior and stabilize at a value greater than those of LQU and C as $t\longrightarrow \infty$.

In Fig. 3c, we repeat the same study, but giving the degree of entanglement p the value 0.5, i.e., the system is prepared initially in a maximally entangled state. So, we find that the DM interaction set off a decay of LQU and C from their maximum value (equal to unity) by performing temporal oscillations before reaching their steady-state at LQU ≈ 0.15 and C ≈ 0.71. Note that LQU is more sensitive to the effect of the DM interaction than concurrence C. Also, the local quantum uncertainty undergoes stronger decoherence than entanglement. However, the uncertainties U_L ≡ U_R, after a very small oscillation, increase continuously and finally stabilize at the value U_L ≡ U_R = 0.6.

Based on these discussions, we can conclude that the DM interaction can enhance the effect of intrinsic decoherence in the system and makes it stronger, so that it can degrade the quantum correlations (LQU and C), which supports the uncertainties of the measurement when the system is initially correlated or maximally correlated. Unfortunately, the decoherence breaks the advantage of the maximally correlated initial state to perfectly guess the measurement result by the owner of the particle serving as memory.

Subsequently, we provide in Fig. 4 temporal evolutions of LQU, C and uncertainties U_L and U_R in the presence of both an external magnetic field (b = 0.3) and intrinsic decoherence (γ = 0.02) and without DM interaction. In Fig. 4a, the system is initially in the separable state, so all quantities experience an oscillatory behaviour during time t, indicating that an external magnetic field b positively reinforces the influence of intrinsic decoherence. Thus, owing to the external magnetic field b, we can use a separable state and makes it correlated because, when $t \longrightarrow \infty$, quantum correlations can reach non-zero steady-state values (LQU ≈ 0.11 and C ≈ 0.44), which contrasts with the results presented in Fig. 2a, when b = 0. However, the uncertainties (U_L ≡ U_R) of the measurement are reduced and stabilize at the value U_L ≡ U_R ≈ 0.85 instead of 1 in the case of Fig. 2a where b = 0.

Fig. 4

Time evolution of LQU, C, entropic uncertaintiy U_L and its lower bound U_R as a function of time t. In all plots we set J = 0.5, D_z = 0,b = 0.3 and γ = 0.02: (a) p = 0, (b) p = 0.25, and (c) p = 0.5

When the initial state is correlated (p = 0.25), we observe a sharp decrease in the initial values of LQU and of C (Fig. 4b). After oscillations, they reach their steady-state for extremely small values compared to the case where b = 0 (Fig. 2b). Note that LQU can be completely destroyed at an early time. Interestingly, the uncertainty U_L differs from its lower bound U_R and crucially expands showing oscillations over time of very large amplitudes and finally reaches a high value U_L ≈ 1.6. As for its lower bound U_R, oscillates with time and reaches its steady-state at the value U_R ≈ 0.83. The gap between the uncertainty U_L and its lower bound U_R is quite clear when $t\longrightarrow \infty$. Therefore, in the light of these results, the external magnetic field b rules negatively if the state is initially correlated by strongly stimulating the effect of decoherence and by favoring the increase of the measurement uncertainty due to the sharp reduction of quantum correlations (LQU and C) within the system.

In the case of the maximally entangled (correlated) initial state (p = 0.5), Fig. 4c exhibits the negative influence of the external magnetic field b on all quantities which show an oscillatory behavior with time t. Note that LQU and C undergo the destructive effect of decoherence due to the magnetic field b and stabilize, when $t\longrightarrow \infty$, at the values LQU ≈ 0.35 and C ≈ 0.74. On the other hand, the uncertainties U_L and U_R are different; U_L shows high amplitude oscillations and finally reaches its steady-state at the value U_L ≈ 1.32, while its lower bound, after minimal amplitude oscillations, stabilizes at the value U_R ≈ 0.53. A comparison with Fig. 4b, we observe that the gap between U_L and U_R is larger in this case. Therefore, although the system is maximally correlated (LQU = C = 1), there is a high measurement uncertainty which prevents the owner of the memory particle from perfectly guessing the result of the measurement. This statement is also violated in the presence of an external magnetic field b.

To fully understand the effect of the DM interaction on our bipartite system, we plot in Fig. 5 the LQU, C and U_L ≡ U_R versus the parameter D_z, in the absence of the external magnetic field b, while keeping the effect of the decoherence (γ = 0.02) for the various initial states characterized by the parameter p. To start, we consider the separable state (p = 0), Fig. 5a proves the ability of the DM interaction to generate small amounts of LQU in a constructive domain of D_z, then displays oscillatory behavior to finally cancel out for large values of D_z, while the entanglement behaves negatively, when the values of D_z increase which oscillates less and less then disappears completely for large values of D_z. On the other hand, the uncertainties U_L ≡ U_R oscillate and can be reduced to an appropriate region of D_z, but as D_z increases they return to the value 1. In Fig. 5b, the system is initially correlated, the quantities LQU and C show oscillations which decrease in amplitude as D_z increases, while the uncertainty of the measurement (U_L ≡ U_R) increases by oscillating more weakly. Furthermore, if the state is initially maximally correlated (LQU = C = 1), Fig. 5c notes that D_z generates a destructive oscillatory behavior of the correlations and entanglement, especially on LQU, while the measurement uncertainties display a monotonic extension as D_z increases. Therefore, we can state that DM interaction (D_z) improves the measurement uncertainties by reducing quantum correlations and entanglement in the system when it is initially correlated.

Fig. 5

Plots of LQU, C entropic uncertainties U_L ≡ U_R as a function of D_z. In all plots we set J = 0.5, t = 20,b = 0 and γ = 0.02: (a) p = 0, (b) p = 0.25, and (c) p = 0.5

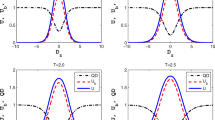
In order to characterize the contribution of the external magnetic field b on our quantifiers, we report, the dynamics of LQU, C and of the uncertainty U_L with its lower bound U_R as a function of the magnetic field b for the various initial states (See Fig. 6). For the separable state (p = 0), the influence of the magnetic field b depicted in Fig. 6a depends on the magnitude of b, so that, when b ≤ 0.6, we view LQU and C increase and after, they reach a maximum. While the uncertainties U_L ≡ U_R decrease to a minimum. Then for b > 0.6, LQU and C decrease monotonically and conversely, the uncertainties U_L ≡ U_R increase progressively and tend towards 1. In short, a controllable magnetic field b can degrade the uncertainty of the measurement, strengthening correlations (especially entanglement) within the system.

Fig. 6

Plots of LQU, C, entropic uncertainty U_L and its lower bound U_R as a function of b. In all plots we set J = 0.5, t = 20,D_z = 0 and γ = 0.02: (a) p = 0, (b) p = 0.25, and (c) p = 0.5

If the system is initially correlated (p = 0.25), we illustrate in Fig. 6b that quantum correlations first drop as the magnetic field b increases, the LQU exhibits a sudden death, then after it performs a weak revival and stabilizes at a non-zero value for large values of b. And the entanglement first decreases for weak magnetic fields and then it increases slightly as b increases. However, the presence of the external magnetic field b crucially inflates the uncertainty U_L and separates it from its lower bound U_R. Also, U_L suddenly increases to a value U_L ≈ 1.8 then it undergoes again downwards and tends towards its lower bound for large values of b, while U_R, increases monotonously and stabilizes at the value U_R ≈ 1.03 for high values of b.

Moreover, we find that the gap between U_L and U_R is larger for values of b close to 0.6, and decreases as b increases. The same behavior is illustrated in Fig. 6c where the initial state is maximally correlated (p = 0.5). Accordingly, when b = 0, LQU and C are both equal to 1. On the other hand, the uncertainties U_L and U_R are zero. This confirms that the maximum amounts of quantum correlations in the system eliminate the measurement uncertainty.

In addition, LQU presents a weak revival for a large value of b (i.e., $b\gtrsim 1$) compared to the case where p = 0.25 in Fig. 6b. Meanwhile, U_L increases up to the value U_L ≈ 1.5 and then decreases to its lower bound U_R as b increases, while U_R monotonically increases and stabilizes at U_R ≈ 0.95. Note that the gap between U_L and U_R is reduced in this case.

5 Conclusion

In summary, we have explored the effect of the intrinsic decoherence on quantum-memory-assited entropic uncertainty (U_L), its lower bound (U_R), the local quantum uncertainty (LQU), and entanglement (C) in the Heisenberg XX spin chain model. In particular, we have proposed a rigorous investigation of the effects of the Dzyaloshinskii-Moriya (DM) interaction and the external non-homogeneous magnetic field b on different initial states characterized by the parameter p. We showed that the Berta’s lower bound is a proper quantifier of the measurement uncertainty when the two-qubit system is prepared initially in a pure state.

The uncorrelated state has very significant features. Indeed, the entanglement is strongly generated and reveals a damped oscillatory behavior of the measurement uncertainty, while LQU remains zero whatever the time. Taking into consideration the DM interaction promotes the appearance of LQU and accelerates the disappearance of the entanglement in the system. Moreover, by controlling the external magnetic field b, we can increase LQU and the concurrence C and save them from complete destruction when the system stabilizes in a steady-state for non-zero values; this is very useful because it reduces the measurement uncertainty.

For the initial correlated state (p = 0.25) and in the absence of DM interaction and the magnetic field, solely the effect of intrinsic decoherence (γ = 0.02) forces the entanglement to exhibits a damped oscillatory behaviour, which is not the case for LQU which decreases continuously, while the uncertainty of the measurement increases as a function of time t. In the presence of the DM interaction, there is an increase in the oscillations of all quantifiers and a fall of LQU and C; this decrease is more pronounced for LQU which highlights its sudden death. This can simply improve the uncertainty of the measurement between the two qubits. The effect of the magnetic field b on this initial state is very destructive. A separation of the uncertainty U_L from its lower bound U_R shows that we can no longer rely on the lower bound as a good quantifier for uncertainty. Moreover, the uncertainty U_L is extremely inflated because of the magnetic field which crucially damages LQU and entanglement in the system, especially LQU which can be totally destroyed at a very early time.

Finally, if the initial state is maximally correlated (p = 0.5), the DM interaction stimulates the intrinsic decoherence effect and decreases the quantum correlations, in particular LQU which undergoes its sudden death very early. This is not the case for the entanglement. In addition, LQU and C exhibit damped oscillations as t increases, while the DM interaction enhances the measurement uncertainty between the two qubits. Besides, the external magnetic field b separates U_L from its lower bound U_R and inflates by showing damped oscillations as a function of time. In this sense, even though the initial state is maximally correlated, the owner of the memory particle cannot perfectly predict the measurement performed by the other part of the system.

At last, we can state that our results could serve a meticulous understanding of the dynamics of quantum correlations, and also shed light on the precision of quantum measurement in practical quantum information processing, especially in the context of spin solid-state.