1 Introduction

Quantum information science is a new discipline based on quantum mechanics, in which quantum teleportation has been widely used. Among them, there are many methods about two-particle teleportation [1,2,3,4,5,6,7,8]. In 2006, Yeo and Chua [9] proposed a four- particle entangled state named χ-type entangled state, which was different from four-particle GHZ and W state. In 2013, Yuan et al. [10] made use of χ-type entangled state to achieve an unknown two-particle teleportation scheme. In 2015, Zhang et al. [11] proposed an unknown two particles teleportation scheme by using two special types state.

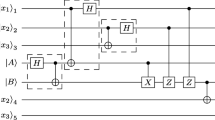
In this paper, we propose two schemes to realize teleportation of two particles by using a special two-particle entangled state as quantum channel. In the first stage, we choose a special two-particle entangled state as a quantum channel, and introduce an auxiliary particle to transfer a two particle unknown state with special coefficients. The sender only uses Bell bases measurement once and Von Neumann measurement, and transfers measurement results to the receiver through classical channel, then the receiver can obtain the state through appropriate unitary transformation. In the second stage, we choose two special two-particle entangled states as quantum channels to transfer a general two-particle unknown state. The sender makes two Bell bases measurement on the system state, and transfers measurement results to the receiver by classical channel, then the receiver takes proper unitary transformation and gets the transmitted state. Contrasting to the scheme proposed by Yuan et al. [10], this paper uses less resource and has a successful probability of 100%. Comparing with the scheme proposed by Zhang et al. [11], this paper proposes two different schemes and it doesn’t need the sender sending the particles to the receiver in advance. Futhermore, we compare the advantages and disadvantages of the two schemes in the end.

2 Teleportation of a Special Two-Particle Quantum State

Alice wants to teleport the following unknown two particle state \(\left | u\right \rangle _{12} \) to Bob,

$$\left| u\right\rangle_{12} =\alpha \left| 00\right\rangle_{12} +\alpha \left| 01\right\rangle_{12} +\Upsilon \left| 10\right\rangle_{12} +\Upsilon \left| 11\right\rangle_{12} $$

where the coefficients are all complex numbers and satisfy normalized condition

$$\left| \alpha \right|^{2}+\left| \gamma \right|^{2}=\frac{1}{2}. $$

We suppose that Alice and Bob share the following special entangled state as quantum channel,

$$ \left| \phi \right\rangle_{3a} =\frac{1}{\sqrt 2} \sum\limits_{i=1}^{2} {\left| \phi \right\rangle_{i}} \otimes \left| \phi ^{\prime}\right\rangle _{i} $$
(1)

where \(\left \{ {\left | \phi \right \rangle _{i}} \right \}_{i=1}^{2} \) constitutes an orthogonal basis of \(\mathbb {C}^{2}\),

$$ \left\{ {{\begin{array}{*{20}l} {\left| \phi \right\rangle_{1} =\cos \theta_{1} \left| 0\right\rangle +\sin \theta_{1} \left| 1\right\rangle} \\ {\left| \phi \right\rangle_{2} =-\sin \theta_{1} \left| 0\right\rangle +\cos \theta_{1} \left| 1\right\rangle} \\ \end{array}} } \right.. $$
(2)

and \(\left \{ {\left | \phi ^{\prime }\right \rangle _{i}} \right \}_{i=1}^{2} \) constitutes another orthogonal basis of \(\mathbb {C}^{2}\),

$$ \left\{ {{\begin{array}{*{20}l} {\left| \phi ^{\prime}\right\rangle_{1} =\cos \theta_{2} \left| 0\right\rangle +\sin \theta_{2} \left| 1\right\rangle} \\ {\left| \phi ^{\prime}\right\rangle_{2} =-\sin \theta_{2} \left| 0\right\rangle +\cos \theta_{2} \left| 1\right\rangle} \\ \end{array}} } \right. $$
(3)

thus we can rewrite \(\left | \phi \right \rangle _{3a} \) as follows,

$$\begin{array}{@{}rcl@{}} \left| \phi \right\rangle_{3a} &\!=\!&\frac{1}{\sqrt 2} \left({\cos \theta_{1} \cos \theta_{2} +\sin \theta_{1} \sin \theta_{2}} \right)\left| 00\right\rangle_{3a} \!+\!\frac{1}{\sqrt 2} \left({\cos \theta_{1} \sin \theta_{2} -\sin \theta_{1} \cos \theta_{2}} \right)\left| 01\right\rangle_{3a} \\ &&+\frac{1}{\sqrt 2} \left({\sin \theta_{1} \cos \theta_{2} \!-\!\cos \theta_{1} \sin \theta_{2}} \right)\left| 10\right\rangle_{3a} \!+\!\frac{1}{\sqrt 2} \left({\cos \theta_{1} \cos \theta _{2} \!+\!\sin \theta_{1} \sin \theta_{2}} \right)\left| 11\right\rangle_{3a} \\ &\!=\!&\frac{1}{\sqrt 2} \left[ {\cos (\theta {} _{1}\!-\!\theta_{2} )\left| 00\right\rangle_{3a} \!-\!\sin (\theta {} _{1}\!-\!\theta _{2} )\left| 01\right\rangle_{3a} \!+\!\sin (\theta {}_{1}\!-\!\theta_{2} )\left| 10\right\rangle_{3a} \!+\!\cos (\theta {} _{1}\!-\!\theta_{2} )\left| 11\right\rangle_{3a}} \right] \\ \end{array} $$

Denote 𝜃 = 𝜃 1 − 𝜃 2. Then

$$\begin{array}{@{}rcl@{}}\left| \phi \right\rangle_{3a} =\frac{1}{\sqrt 2} \left({\cos \theta \left| 00\right\rangle_{3a} -\sin \theta \left| 01\right\rangle_{3a} +\sin \theta \left| 10\right\rangle_{3a} +\cos \theta \left| 11\right\rangle_{3a}} \right) \end{array} $$
(4)

Next, Bob introduces an auxiliary particle with \(\left | \varphi \right \rangle _{b} =\frac {1}{\sqrt 2} \left ({\left | 0\right \rangle +\left | 1\right \rangle } \right )_{b} \), then the state of the combined system is given by

$$\begin{array}{@{}rcl@{}} \left| \Pi \right\rangle_{123ab} &=&\left| u\right\rangle_{12} \otimes \left| \phi \right\rangle_{3a} \otimes \left| \phi \right\rangle_{b} \\ & =&\frac{1}{\sqrt 2} \left({\alpha \left| 00\right\rangle +\alpha \left| 01\right\rangle +\gamma \left| 10\right\rangle +\gamma \left| 11\right\rangle} \right)_{12}\\ & \otimes& \left({\cos \theta \left| 00\right\rangle -\sin \theta \left| 01\right\rangle +\sin \theta \left| 10\right\rangle +\cos \theta \left| 11\right\rangle} \right)_{3a} \otimes \left| \phi \right\rangle_{b} \end{array} $$

where particles 1, 2 and 3 belong to Alice, particles a and b belong to Bob.

Alice first performs Bell state measurement on the qubit pairs (1, 3) in the basis states

$$\left\{ {\left| \phi^{\pm} \right\rangle =\frac{1}{\sqrt 2} \left({\left| 00\right\rangle \pm \left| 11\right\rangle} \right),\left| \psi^{\pm} \right\rangle =\frac{1}{\sqrt 2} \left({\left| 01\right\rangle \pm \left| 10\right\rangle} \right)} \right\},$$

then the results are as follows,

$$\begin{array}{@{}rcl@{}} {~} _{13}\left| \varphi^{+}\right\rangle \left. \Pi \right\rangle_{123ab} &=&\frac{1}{2}\left[ \left({\alpha \cos \theta +\gamma \sin \theta} \right)\left| 00\right\rangle +\left({\gamma \cos \theta -\alpha \sin \theta} \right)\left| 01\right\rangle\right.\\ &&\left. +\left({\alpha \cos \theta +\gamma \sin \theta} \right)\left| 10\right\rangle +\left({\gamma \cos \theta -\alpha \sin \theta} \right)\left| 11\right\rangle \right]_{2a} \left| \varphi \right\rangle_{b} \\ {~} _{13}\left| \varphi^{-}\right\rangle \left. \Pi \right\rangle_{123ab} &=&\frac{1}{2}\left[ \left({\alpha \cos \theta -\gamma \sin \theta} \right)\left| 00\right\rangle -\left({\alpha \sin \theta +\gamma \cos \theta} \right)\left| 01\right\rangle\right.\\ &&\left.+\left(\alpha \cos \theta -\gamma \sin \theta \right)\left| 10\right\rangle -\left({\alpha \sin \theta +\gamma \cos \theta} \right)\left| 11\right\rangle \right]_{2a} \left| \varphi \right\rangle_{b} \\ {~} _{13}\left\langle \psi^{+} \right|\left. \Pi \right\rangle _{123ab} &=&\frac{1}{2}\left[ \left({\alpha \sin \theta +\gamma \cos \theta} \right)\left| 00\right\rangle +\left(\alpha \cos \theta -\gamma \sin \theta \right)\left| 01\right\rangle\right.\\ &&\left.+\left({\alpha \sin \theta +\gamma \cos \theta} \right)\left| 10\right\rangle +\left({\alpha \cos \theta -\gamma \sin \theta} \right)\left| 11\right\rangle \right]_{2a} \left| \varphi \right\rangle_{b}\\ {~} _{13}\left\langle\psi^{-} \right|\left. \Pi \right\rangle _{123ab} &=&\frac{1}{2}\left[ \left({\alpha \sin \theta -\gamma \cos \theta} \right)\left| 00\right\rangle +\left(\alpha \cos \theta +\gamma \sin \theta \right)\left| 01\right\rangle\right.\\ &&\left.+\left({\alpha \sin \theta -\gamma \cos \theta} \right)\left| 10\right\rangle +\left({\alpha \cos \theta +\gamma \sin \theta} \right)\left| 11\right\rangle \right]_{2a} \left| \varphi \right\rangle_{b} \end{array} $$

Choosing \({} _{13}\left | \varphi ^{+}\right \rangle \left . \Pi \right \rangle _{123ab} \) as an example, then we have

$$\begin{array}{@{}rcl@{}} {~} _{13} \left| \varphi^{+}\right\rangle \left. \Pi \right\rangle_{123ab} &=&\frac{1}{2}\left[ \left({\alpha \cos \theta +\gamma \sin \theta} \right)\left| 00\right\rangle +\left({\gamma \cos \theta -\alpha \sin \theta} \right)\left| 01\right\rangle\right.\\ && \left.+\left({\alpha \cos \theta +\gamma \sin \theta} \right)\left| 10\right\rangle +\left({\gamma \cos \theta -\alpha \sin \theta} \right)\left| 11\right\rangle \right]_{2a} \left| \varphi \right\rangle_{b} \\ &=&\frac{1}{2\sqrt 2} [\left({\alpha \cos \theta +\gamma \sin \theta} \right)\left| 001\right\rangle +\left({\gamma \cos \theta -\alpha \sin \theta} \right)\left| 011\right\rangle\\ &&+\left({\alpha \cos \theta +\gamma \sin \theta} \right)\left| 000\right\rangle +\left({\gamma \cos \theta -\alpha \sin \theta} \right)\left| 010\right\rangle \\ &&+\left({\alpha \cos \theta +\gamma \sin \theta} \right)\left| 100\right\rangle +\left({\alpha \cos \theta +\gamma \sin \theta} \right)\left| 101\right\rangle\\ &&+\left({\gamma \cos \theta -\alpha \sin \theta} \right)\left| 111\right\rangle +\left({\gamma \cos \theta -\alpha \sin \theta} \right)\left| 110\right\rangle]_{2ab} \\ &=&\frac{1}{2\sqrt 2} \left[ \left({\alpha \cos \theta +\gamma \sin \theta} \right)\left| 000\right\rangle +\left({\gamma \cos \theta -\alpha \sin \theta} \right)\left| 010\right\rangle\right.\\ &&\left. +\left({\alpha \cos \theta +\gamma \sin \theta} \right)\left| 001\right\rangle +\left({\gamma \cos \theta -\alpha \sin \theta} \right)\left| 011\right\rangle \right]_{2ab} \\ &&+\frac{1}{2\sqrt 2} \left[ \left({\alpha \cos \theta +\gamma \sin \theta} \right)\left| 100\right\rangle +\left({\gamma \cos \theta -\alpha \sin \theta} \right)\left| 110\right\rangle\right.\\ &&\left.+\left({\alpha \cos \theta +\gamma \sin \theta} \right)\left| 101\right\rangle +\left({\gamma \cos \theta -\alpha \sin \theta} \right)\left| 111\right\rangle \right]_{2ab} \end{array} $$

If Alice takes Von Neumann measurement on the particle 2, then the results are always same no matter the measurement is \(\left | 0\right \rangle _{2} \) and \(\left | 1\right \rangle _{2} \), that is

$$\begin{array}{@{}rcl@{}} \left| \Pi \right\rangle_{ab}& \!=\!&\frac{1}{2\sqrt 2} \left[ \left({\alpha \cos \theta \!+\!\gamma \sin \theta} \right)\left| 00\right\rangle \!+\!\left({\gamma \cos \theta -\alpha \sin \theta} \right)\left| 10\right\rangle +\left({\alpha \cos \theta +\gamma \sin \theta} \right)\left| 01\right\rangle\right.\\ &&\left.+\left({\gamma \cos \theta -\alpha \sin \theta} \right)\left| 11\right\rangle \right]_{ab} \end{array} $$

After Alice tells the measurement results to Bob through classical channel, Bob can obtain the transmitted state by the following unitary transformation,

$$U=\left({{\begin{array}{*{20}c} {\cos \theta} & 0 & {-\sin \theta} & 0 \\ 0 & {\cos \theta} & 0 & {-\sin \theta} \\ {\sin \theta} & 0 & {\cos \theta} & 0 \\ 0 & {\sin \theta} & 0 & {\cos \theta} \\ \end{array}} } \right) $$

and the successful probability is

$$\begin{array}{@{}rcl@{}} &&\frac{1}{8}\left({2\left({\alpha \cos \theta +\gamma \sin \theta} \right)^{2}+2\left({\gamma \cos \theta -\alpha \sin \theta} \right)^{2}} \right) \times 8 \\ &=&\frac{1}{4}\left({\left| \alpha \right|^{2}\left({\cos^{2}\theta +\sin ^{2}\theta} \right)+\left| \gamma \right|^{2}\left({\cos^{2}\theta +\sin ^{2}\theta} \right)} \right)\times 8 \\ &=&1 \\ \end{array} $$

In short, this section first chooses a special two-particle entangled state as the quantum channel, then introduces an auxiliary particle b. The two-particle state can be transfered from Alice to Bob after once Bell based measurement and Von Neumann measurements by Alice, and correponding unitary transformation by Bob. The process is simple and the idea is clear, but the coefficients of the unknown two particle state are limited. In order to realize the teleportation of general two-particle state, we next propose the second scheme.

3 Teleportation of the General Two-Particle State

Alice wants to teleport the following general two-particle state \(\left | u\right \rangle _{12} \),

$$\left| u\right\rangle_{12} =\alpha \left| 00\right\rangle_{12} +\beta \left| 01\right\rangle_{12} +\Upsilon \left| 10\right\rangle_{12} +\delta \left| 11\right\rangle_{12} $$

where the coefficients are complex numbers and satisfy the normalized condition

$$\left| \alpha \right|^{2}+\left| \beta \right|^{2}+\left| \gamma \right|^{2}+\left| \delta \right|^{2}=1. $$

Suppose that Alice and Bob share the following two special entangled states as quantum channel,

$$\begin{array}{@{}rcl@{}} \left| \phi \right\rangle_{3a} =\frac{1}{\sqrt 2} \left[ {\cos \theta \left| 00\right\rangle -\sin \theta \left| 01\right\rangle +\sin \theta \left| 10\right\rangle +\cos \theta \left| 11\right\rangle} \right]_{3a} \\ \left| \phi \right\rangle_{4b} =\frac{1}{\sqrt 2} \left[ {\cos \theta \left| 00\right\rangle -\sin \theta \left| 01\right\rangle +\sin \theta \left| 10\right\rangle +\cos \theta \left| 11\right\rangle} \right]_{4b} \\ \end{array} $$

then the state of the combined system is given by

$$\begin{array}{@{}rcl@{}} \left| \Pi \right\rangle_{123a4b} &=&\left| u\right\rangle_{12} \otimes \left| \phi \right\rangle_{3a} \otimes \left| \phi \right\rangle_{4b} \\ &=&\frac{1}{2}\left({\alpha \left| 00\right\rangle +\beta \left| 01\right\rangle +\gamma \left| 10\right\rangle +\delta \left| 11\right\rangle} \right)_{12} \otimes \left(\cos \theta \left| 00\right\rangle -\sin \theta \left| 01\right\rangle\right.\\ &&\qquad\left.+\sin \theta \left| 10\right\rangle +\cos \theta \left| 11\right\rangle \right)_{3a} \\ &\otimes& \left({\cos \theta \left| 00\right\rangle -\sin \theta \left| 01\right\rangle +\sin \theta \left| 10\right\rangle +\cos \theta \left| 11\right\rangle} \right)_{4b} \end{array} $$

where particles 1, 2, 3 and 4 belong to Alice, particles a and b belong to Bob.

Alice first performs Bell state measurement on the qubit pairs (1, 3), the results are as follows:

$$\begin{array}{@{}rcl@{}} {~} _{13}\left| \varphi^{+}\right\rangle \left. \Pi \right\rangle_{123a4b} &=&\frac{1}{2}\left[ \left({\alpha \cos \theta +\gamma \sin \theta} \right)\left| 00\right\rangle +\left({\gamma \cos \theta -\alpha \sin \theta} \right)\left| 01\right\rangle\right.\\ &&\left. +\left({\beta \cos \theta +\delta \sin \theta} \right)\left| 10\right\rangle +\left({\delta \cos \theta -\beta \sin \theta} \right)\left| 11\right\rangle \right]_{2a} \otimes \left| \varphi \right\rangle_{4b} \\ {~} _{13}\left| \varphi^{-}\right\rangle \left. \Pi \right\rangle_{123a4b} &=&\frac{1}{2}\left[ \left({\alpha \cos \theta -\gamma \sin \theta} \right)\left| 00\right\rangle -\left({\alpha \sin \theta +\gamma \cos \theta} \right)\left| 01\right\rangle\right.\\ &&\left.+\left({\beta \cos \theta -\delta \sin \theta} \right)\left| 10\right\rangle -\left({\beta \sin \theta +\delta \cos \theta} \right)\left| 11\right\rangle \right]_{2a} \otimes \left| \varphi \right\rangle_{4b} \\ {~} _{13}\left| \psi^{+}\right\rangle \left. \Pi \right\rangle_{123a4b} &=&\frac{1}{2}\left[ \left({\alpha \sin \theta +\gamma \cos \theta} \right)\left| 00\right\rangle +\left({\alpha \cos \theta -\gamma \sin \theta} \right)\left| 01\right\rangle\right.\\ &&\left.+\left({\beta \sin \theta +\delta \cos \theta} \right)\left| 10\right\rangle +\left({\beta \cos \theta -\delta \sin \theta} \right)\left| 11\right\rangle \right]_{2a} \otimes \left| \varphi \right\rangle_{4b} \\ {~} _{13}\left| \psi^{-}\right\rangle \left. \Pi \right\rangle_{123a4b} &=&\frac{1}{2}\left[ \left({\alpha \sin \theta -\gamma \cos \theta} \right)\left| 00\right\rangle +\left({\alpha \cos \theta +\gamma \sin \theta} \right)\left| 01\right\rangle\right.\\ &&\left.+\left({\beta \sin \theta -\delta \cos \theta} \right)\left| 10\right\rangle +\left({\beta \cos \theta +\delta \sin \theta} \right)\left| 11\right\rangle \right]_{2a} \otimes \left| \varphi \right\rangle_{4b} \end{array} $$

Choosing \(_{13} \left \langle \varphi ^{+}\right |\Pi \rangle _{123a4b} \) as an example, and Alice performs Bell state measurement on the qubit pairs (2, 4), then the results are as follows:

$$\begin{array}{@{}rcl@{}} {~} _{24}\left\langle\phi^{+}\right|{} _{13}\left\langle\phi^{+}\right|\left. \Pi \right\rangle_{123a4b} &\!=\!&\frac{1}{4}\left({\alpha \frac{1+\cos 2\theta} {2}\!+\!\gamma \frac{\sin 2\theta} {2}+\beta \frac{\sin 2\theta} {2}+\delta \frac{1-\cos 2\theta} {2}} \right)\left| 00\right\rangle_{ab}\\ && \!+\frac{1}{4}\left({-\alpha \frac{\sin 2\theta} {2}\!-\!\gamma \frac{1+\cos 2\theta} {2}\!+\!\beta \frac{1+\cos 2\theta} {2}+\delta \frac{\sin 2\theta} {2}} \right)\left| 01\right\rangle \\ &&\!+\frac{1}{4}\left({-\alpha \frac{\sin 2\theta} {2}\!+\!\gamma \frac{1\!+\!\cos 2\theta} {2}\!-\!\beta \frac{1\!-\!\cos 2\theta} {2}\!+\!\delta \frac{\sin 2\theta} {2}} \right)\left| 10\right\rangle_{ab}\\ &&\!+\frac{1}{4}\left({\alpha \frac{1+\cos 2\theta} {2}\!-\!\gamma \frac{\sin 2\theta} {2}\!-\!\beta \frac{\sin 2\theta} {2}\!+\!\delta \frac{1+\cos 2\theta} {2}} \right)\left| 11\right\rangle_{ab}\\ {~} _{24}\left\langle\phi^{-}\right|{} _{13}\left\langle\phi^{+}\right|\left. \Pi \right\rangle_{123a4b} &\!=\!&\frac{1}{4}\left({\alpha \frac{1+\cos 2\theta} {2}\!+\!\gamma \frac{\sin 2\theta} {2}\!-\!\beta \frac{\sin 2\theta} {2}-\delta \frac{1-\cos 2\theta} {2}} \right)\left| 00\right\rangle_{ab} \\ &&\!+\frac{1}{4}\left({-\alpha \frac{\sin 2\theta} {2}\!-\!\gamma \frac{1\!-\!\cos 2\theta} {2}\!-\!\beta \frac{1\!+\!\cos 2\theta} {2}\!-\!\delta \frac{\sin 2\theta} {2}} \right)\left| 01\right\rangle_{ab} \\ &&\!+\frac{1}{4}\left({-\alpha \frac{\sin 2\theta} {2}\!+\!\gamma \frac{1\!+\!\cos 2\theta} {2}\!+\!\beta \frac{1\!-\!\cos 2\theta} {2}\!-\!\delta \frac{\sin 2\theta} {2}} \right)\left| 10\right\rangle_{ab}\\ &&\!+\frac{1}{4}\left({\alpha \frac{1-\cos 2\theta} {2}\!-\!\gamma \frac{\sin 2\theta} {2}\!+\!\beta \frac{\sin 2\theta} {2}\!-\!\delta \frac{1+\cos 2\theta} {2}} \right)\left| 11\right\rangle_{ab} \end{array} $$
$$\begin{array}{@{}rcl@{}} {~} _{24}\left\langle\Psi^{+}\right|{} _{13}\left\langle\phi^{+}\right|\left. \Pi \right\rangle_{123a4b} &\!=\!&\frac{1}{4}\left({\alpha \frac{\sin 2\theta} {2}+\gamma \frac{1-\cos 2\theta} {2}+\beta \cos^{2}\theta +\delta \frac{\sin 2\theta} {2}} \right)\left| 00\right\rangle_{ab}\\ && \!+\frac{1}{4}\left({\alpha \frac{1+\cos 2\theta} {2}\!+\!\gamma \frac{\sin 2\theta} {2}\!-\!\beta \frac{\sin 2\theta} {2}\!-\!\delta \frac{1\!-\!\cos 2\theta} {2}} \right)\left| 01\right\rangle_{ab} \\ &&\!+\frac{1}{4}\left({\!-\alpha \frac{1\!-\!\cos 2\theta} {2}\!+\!\gamma \frac{\sin 2\theta} {2}\!-\!\beta \frac{\sin 2\theta} {2}\!+\!\delta \frac{1\!+\!\cos 2\theta} {2}} \right)\left| 10\right\rangle_{ab}\\ &&\!+\frac{1}{4}\left({\!-\alpha \frac{\sin 2\theta} {2}\!+\!\gamma \frac{1\!+\!\cos 2\theta} {2}\!+\!\beta \frac{1\!-\!\cos 2\theta} {2}\!-\!\delta \frac{\sin 2\theta} {2}} \right)\left| 11\right\rangle_{ab} \\ {~} _{24}\left\langle\Psi^{-}\right|{} _{13}\left\langle\phi^{+}\right|\left. \Pi \right\rangle_{123a4b} &\!=\!&\frac{1}{4}\left({\alpha \frac{\sin 2\theta} {2}\!+\!\gamma \frac{1\!-\!\cos 2\theta} {2}\!-\!\beta \frac{1\!+\!\cos 2\theta} {2}-\delta \frac{\sin 2\theta} {2}} \right)\left| 00\right\rangle_{ab}\\ && \!+\frac{1}{4}\left({\alpha \frac{1\!+\!\cos 2\theta} {2}\!+\!\gamma \frac{\sin 2\theta} {2}\!+\!\beta \frac{\sin 2\theta} {2}+\delta \frac{1-\cos 2\theta} {2}} \right)\left| 01\right\rangle_{ab} \\ &&\!+\frac{1}{4}\left({\!-\alpha \frac{1\!-\!\cos 2\theta} {2}\!+\!\gamma \frac{\sin 2\theta} {2}\!+\!\beta \frac{\sin 2\theta} {2}\!-\!\delta \frac{1\!+\!\cos 2\theta} {2}} \right)\left| 10\right\rangle_{ab}\\ &&\!+\frac{1}{4}\left({\!-\alpha \frac{\sin 2\theta} {2}\!+\!\gamma \frac{1\!+\!\cos 2\theta} {2}\!-\!\beta \frac{1\!-\!\cos 2\theta} {2}\!+\!\delta \frac{\sin 2\theta} {2}} \right)\left| 11\right\rangle_{ab} \\ \end{array} $$

Alice tell the measurement results to Bob through classical channel, Bob then reproduce the transmitted state by following unitary transformation,

$$U=\left({{\begin{array}{*{20}c} {\frac{1+\cos 2\theta} {2}} & {\frac{-\sin 2\theta} {2}} &{\frac{-\sin 2\theta} {2}} & {\frac{1-\cos 2\theta} {2}} \\ {\frac{\sin 2\theta} {2}} & {\frac{1+\cos 2\theta} {2}} &{\frac{-1+\cos 2\theta} {2}} & {\frac{-\sin 2\theta} {2}} \\ {\frac{\sin 2\theta} {2}} & {\frac{-1+\cos 2\theta} {2}} &{\frac{1+\cos 2\theta} {2}} & {\frac{-\sin 2\theta} {2}} \\ {\frac{1-\cos 2\theta} {2}} & {\frac{\sin 2\theta} {2}} &{\frac{\sin 2\theta} {2}} & {\frac{1+\cos 2\theta} {2}} \\ \end{array}} } \right) $$

and the probability of successful is \(\frac {1}{16}\times 16=1\).

This programmer can also be used to transfer the unknown two particle state \(\left | u\right \rangle _{12} =\alpha \left | 00\right \rangle _{12} +\alpha \left | 01\right \rangle _{12} +\Upsilon \left | 10\right \rangle _{12} +\Upsilon \left | 11\right \rangle _{12} \) in Section 2. If we choose \(_{24} \left \langle \phi ^{+}\right |_{13} \left \langle \phi ^{+} \right |\left .\Pi \right \rangle _{123a4b} \) as an example, then the result is

$$\begin{array}{@{}rcl@{}} {~} _{24}\left\langle\phi^{+}\right|{} _{13}\left\langle\phi^{+}\right|\left. \Pi \right\rangle_{123a4b}& \!=\!&\frac{1}{4}\left({\alpha \frac{1+\cos 2\theta} {2}\!+\!\gamma \frac{\sin 2\theta} {2}\!+\!\alpha \frac{\sin 2\theta} {2}+\gamma \frac{1-\cos 2\theta} {2}} \right)\left| 00\right\rangle_{ab}\\ && \!+\frac{1}{4}\left({\!-\alpha \frac{\sin 2\theta} {2}\!-\!\gamma \frac{1\!-\!\cos 2\theta} {2}\!+\!\alpha \frac{1\!+\!\cos 2\theta} {2}\!+\!\gamma \frac{\sin 2\theta} {2}} \right)\left| 01\right\rangle_{ab} \\ &&\!+\frac{1}{4}\left({\!-\alpha \frac{\sin 2\theta} {2}\!+\!\gamma \frac{1\!+\!\cos 2\theta} {2}\!-\!\alpha \frac{1\!-\!\cos 2\theta} {2}\!+\!\gamma \frac{\sin 2\theta} {2}} \right)\left| 10\right\rangle_{ab}\\ &&\!+\frac{1}{4}\left({\alpha \frac{1\!-\!\cos 2\theta} {2}\!-\!\gamma \frac{\sin 2\theta} {2}\!-\!\alpha \frac{\sin 2\theta} {2}+\gamma \frac{1+\cos 2\theta} {2}} \right)\left| 11\right\rangle_{ab} \\ \end{array} $$

Thus the teleportation of the general two particle state is still successful.

4 Conclusion

In this paper, we have introduced two schemes of teleporting unknown two-particle state by choosing special two-particle state as the channel. The advantages of the first scheme is that it only uses a two-particle state as the channel, and introduces an auxiliary particle, through a Bell bases measurement, Von Neumann measurement and an unitary transformation. It has simple process and less calculation, but the coefficients of transmitted state are limited. The advantages of the second scheme is that it uses two-particle states as channel. It is easy to teleport state after twice Bell bases measurements and an unitary transformation. But it needs much more calculation, and the process is relatively cumbersome. The above two schemes have their own characteristics which both provide new idea for two-particle state teleportation.