1 Introduction

Lithium-ion batteries have been widely used in portable electronic equipment, electric vehicles, wind and solar energy, and energy storage. The development of lithium-ion batteries is also an important part of the new energy revolution [1, 2]. In particular, electric vehicle technology calls for lithium-ion batteries with higher energy densities and better safety performance. Using solid electrolytes can effectively increase the volumetric energy density of a battery [3]. Meanwhile, burning and explosion of the lithium-ion battery induced by a short circuit and thermal runaway in the battery can be avoided by applying solid electrolytes [4,5,6]. Therefore, in recent years, the solid-state lithium-ion battery has received extensive attention and has become an important component of new commercial energy storage technology [7, 8].

However, the interface between the active material and solid electrolyte is one of the key factors obstructing the development of the solid-state lithium-ion battery [9]. The electrochemical reaction at the interface is the core of the matter transport and energy storage/release in the lithium-ion battery. The microscopic defects [10,11,12,13] and interphase [14,15,16] greatly impact the electrochemical reaction at the interface in the solid electrode. For example, Han et al. [10] found that microscopic interfacial defects caused an impedance as high as 1710 \(\Omega /\hbox {cm}^{{2}}\) at the interface between a garnet electrolyte and lithium metal. Tallarek et al. [11] found that the micropores at the interface between a \(\hbox {LiCoO}_{{2}}\) cathode and sulfide solid electrolyte significantly increased the tortuosity of the transport channel of lithium ions, making matter transport more difficult in the electrode. Meanwhile, contact loss between the active material and solid electrolyte at the mesoscopic level also degraded the performance of a solid-state battery [17]. Deveaux et al. [18] observed that after charging–discharging cycles of a \(\hbox {LiFePO}_{{4}}\)/SEO/Li battery, there was serious contact loss at the interface between the active material and solid electrolyte. Koerver et al. observed that after the first charging–discharging cycle of a \(\hbox {NCM/Li}_{{3}}\hbox {PS}_{{4}}/\hbox {Li}\) battery, the contact loss between the NCM particles and solid electrolyte led to an increase in the internal resistance and a significant decrease in discharge capacity [19]. These interface issues can be improved to some extent by imposing external constraints and developing new material systems [12, 13], but it is still challenging to ensure perfect contact at the interface between the active material and solid electrolyte [20].

Contact loss impacts matter transport. First, as a result of contact loss, reduction in the contact surface causes the ion/electron flow to converge, which increases the difficulty of matter transport between the solid electrolyte and active material [21]. Second, contact loss at the interface changes the degree to which the solid electrolyte constrains the active material and significantly affects the stress field in the active material. The influence of stress on matter transport cannot be ignored [22,23,24]. Lithium ions/atoms tend to diffuse from the compressive stress area to the tensile stress area, so stress assists lithium ion/atom diffusion [22]. Most of the related theoretical work focuses on mechanical-chemical coupling in a single active particle. For an active particle with unconstrained surfaces, mechanical–chemical coupling is realized by introducing the influence of hydrostatic stress into the chemical potential and considering the deformation caused by lithium diffusion in the mechanical constitutive relationship [25,26,27,28]. The mechanical–electrochemical coupling within the active materials in solid-state lithium-ion batteries is similar to that in lithium-ion batteries with liquid electrolytes [29]. However, the constraint of the solid electrolyte must be taken into account because the constraint of inactive materials has shown to lead to considerably high stress in the active material, which significantly affects lithium ion/atom transport [23, 24]. There is still little theoretical and modeling work considering the impact of interfacial contact loss on mechanical–chemical coupling in solid-state lithium-ion batteries. In this work, we simulate the delithiation and relaxation of the cathode material in a solid-state lithium-ion battery considering contact loss at the interface between the active material and solid electrolyte. We investigate the influence of the degree of contact loss on the mechanical–chemical coupling fields and the effect of the elastic modulus of the solid electrolyte on the coupling of the active material.

2 Modeling

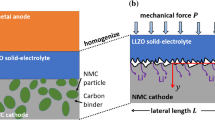
Figure 1a shows the schematic of the cathode of a solid-state lithium-ion battery. The yellow part represents the solid electrolyte. The red part denotes the active particles. The gray area represents the current collector. The active particles are assumed to be uniformly distributed in the solid electrolyte. A core-shell model is established. This model consists of an active particle and the solid electrolyte surrounding it, as shown in Fig. 1a. In the model, r represents the radius of the active particle, and d denotes the thickness of the external solid electrolyte shell. There is contact loss at the interface between the active particle and solid electrolyte, as shown in Fig. 1b. At the interface where contact loss occurs, the active particle is separated from the solid electrolyte. To express the degree of interfacial contact loss, a contact factor is defined as \(\beta =A_{c}\)/\(A_{p}\), where \(A_{c}\) represents the area of the contact region between the active particle and solid electrolyte, and \(A_{p}\) denotes the total surface area of the active particle. Then, \(A_{p}\)-\(A_{c}\) is the area of the contact loss region. When the battery is charging, the cathode material undergoes delithiation. During this process, lithium ions extract from the active particle and get into the solid electrolyte through the contact region. As delithiation begins, the active particle starts to shrink, as shown in Fig. 1b. The delithiation and the following relaxation of the active particle will be simulated, and the mechanical-chemical coupled fields will be analyzed. The interfacial contact loss is assumed not to evolve during the delithiation, meaning the contact factor remains constant during the delithiation.

Fig. 1
figure 1

a Schematic of the cathode and core-shell particle model in a solid-state lithium-ion battery. b Schematic of 50% contact loss at the interface between the active particle (AP) and solid electrolyte (SE)

Full size image

The driving force for the movement of lithium ions is the chemical potential gradient. The total chemical potential \(\mu _{t}\) can be written as:

$$\begin{aligned}&\mu _{t} =\mu _{0} +\mu _{c} +\mu _{m} \end{aligned}$$
(1a)
$$\begin{aligned}&\mu _{c} =RT\ln X \end{aligned}$$
(1b)
$$\begin{aligned}&\mu _{m} =-\Omega \sigma _{h} \end{aligned}$$
(1c)

where \(\mu _{{0}}\) is the reference chemical potential, which is constant; \(\mu _{c}\) is the chemical part of the total chemical potential and is attributed to the molar fraction X of lithium ions given by \(X=c\)/\(c_{\mathrm {max}}\), where c is the current concentration and \(c_{\mathrm {max}}\) the saturated concentration; \({\mu }_{m}\) is the mechanical part of the total chemical potential, which is attributed to the hydrostatic stress \(\sigma _{h}\). The hydrostatic stress is given by \(\sigma _{h}=(\sigma _{{1}}+\sigma _{{2}}+\sigma _{{3}})\)/3, where \(\sigma _{{1}}\), \(\sigma _{{2}}\), and \(\sigma _{{3}}\) are the three principal stresses. R is the gas constant and T the absolute temperature. \(\Omega \) is the partial molar volume of the active material.

The lithium ion transport in the electrolyte is assumed to be very fast, so the concentration in it is almost constant and does not vary with time. Therefore, diffusion in the solid electrolyte is not considered. Diffusion in the active particle is described by Fick’s law:

$$\begin{aligned} \frac{\partial c}{\partial t}+\nabla \cdot J=0 \end{aligned}$$
(2)

The flux J can be derived from the total chemical potential as:

$$\begin{aligned} J=-\frac{Dc}{RT}\nabla \mu _{t} \end{aligned}$$
(3)

where D is the diffusion coefficient of lithium ions in the active material. From Eq. (1) for the total chemical potential, the effect of the stress can be introduced into the flux J, which is expressed as [28, 30]

$$\begin{aligned} J=-D\left( {\nabla c-\frac{\Omega c}{RT}\nabla \sigma _{h} } \right) \end{aligned}$$
(4)

Substituting the flux into Eq. (6) into Eq. (4), we get the governing equation of diffusion within the active particle:

$$\begin{aligned} \frac{\partial c}{\partial t}-D\left( {\nabla ^{2}c-\frac{\Omega }{RT}\nabla c\cdot \nabla \sigma _{h} -\frac{\Omega c}{RT}\nabla ^{2}\sigma _{h} } \right) =0 \end{aligned}$$
(5)

Before the battery is charged, the cathode material is filled with lithium ions and assumed to be in a stress-free state, which means \({c}={c}_{\mathrm {max}}\) and \({\sigma _{ij}}=0\) at \({t}=0\). The initial state of the solid electrolyte is also assumed to be stress-free. Once charging begins, the cathode material starts to delithiate and internal stress begins to rise. Figure 1b is the projection of the three-dimensional core-shell cell in the zx plane. The solid blue line at the interface represents the contact between the active particle and solid electrolyte, while the black dashed line at the interface is where contact loss occurs between them. This model adopts constant current charging method. The transport path of the lithium ions is blocked at the interface where contact loss occurs. Therefore, the flux is zero (\({J}=0\)) at the contact loss region of the interface. At the interface where the active particle and solid electrolyte remain in contact, lithium ions can go through normally, and the flux through this section of the interface can be expressed as:

$$\begin{aligned} J=-D\left( {\nabla c-\frac{\Omega c}{RT}\nabla \sigma _{h} } \right) =\frac{i_{n} }{\beta F} \end{aligned}$$
(6)

where \(i_{n}\) is the reference current density at the particle surface for the case without contact loss, and F is the Faraday constant. Under the condition of constant current charging, the total amount of lithium ions extracted from the active particle should be the same at any time, so the contact factor \({\beta }\) is introduced in the expression of the flux in Eq. (6).

The equilibrium equations for the active particle and solid electrolyte are, respectively,

$$\begin{aligned} \sigma _{ij,j} =0,\;{\sigma }'_{ij,j} =0 \end{aligned}$$
(7a, b)

where \({\sigma _{ij}}\) and \({\sigma }^{{'}}_{ij}\) represent the stress tensors for the active particle and solid electrolyte, respectively. The subscript comma denotes partial differentiation with respect to the following coordinate. The strain–displacement relations are

$$\begin{aligned} \varepsilon _{ij} =\frac{1}{2}\left( {u_{i,j} +u_{j,i} } \right) ,\;{\varepsilon }'_{ij} =\frac{1}{2}\left( {{u}'_{i,j} +{u}'_{j,i} } \right) \end{aligned}$$
(8a, b)

where \(\varepsilon _{ij}\) and \({\varepsilon }^{{'}}_{ij}\) represent the strain tensors for the active particle and solid electrolyte, respectively; \(u_i\) and \({u}^{{'}}_{i}\) denote the displacement components for the active particle and solid electrolyte, respectively.

Both the active particle and solid electrolyte are considered to be isotropic and homogeneous. Considering the coupling effect of diffusion, the constitutive relationship for the active material is [26, 31]

$$\begin{aligned} \varepsilon _{ij} =\left( {\frac{1+\nu }{E_\mathrm{AP} }\sigma _{ij} -\frac{\nu }{E_\mathrm{AP} }\sigma _{kk} \delta _{ij} } \right) +\frac{\Omega \Delta c}{3}\delta _{ij} \end{aligned}$$
(9)

Regardless of diffusion in the electrolyte, the constitutive relationship for the electrolyte is

$$\begin{aligned} {\varepsilon }'_{ij} =\left( {\frac{1+{\nu }'}{E_\mathrm{SE} }{\sigma }'_{ij} -\frac{{\nu }'}{E_\mathrm{SE} }{\sigma }'_{kk} \delta _{ij} } \right) \end{aligned}$$
(10)

where \({E}_{\mathrm {AP}}\) and \({E}_{\mathrm {SE}}\) are the elastic moduli of the active particle and solid electrolyte, respectively; \(\nu \) and \({\nu }^{{'}}\) are the Poisson’s ratios for the active material and solid electrolyte, respectively; and \({\delta _{ij}}\) is the Kronecker symbol.

Because the composite electrode is usually closely compacted, the displacements in the solid electrolyte away from the active particle are assumed to be constrained. Therefore, the displacement constraint conditions are prescribed at the boundary of the solid electrolyte (the outer surface in Fig 1b):

$$\begin{aligned} {u}'_{i} =0 \end{aligned}$$
(11)

At the interface where contact loss occurs, the active particle is separated from the solid electrolyte and free surfaces form. Therefore, tractions at the free surfaces are zero, namely \({\sigma _{ij}n_{j}}={\sigma }^{{'}}_{ij}n^{{'}}_{j}=0\), where \({n_{j}}\) and \({n}^{{'}}_{j}\) are the components of the unit normal vector at the surfaces of the active particle and solid electrolyte, respectively. A perfect bond is assumed between the active particle and solid electrolyte at the interface where they are in contact, indicating that the tractions and displacements at the interface should satisfy the continuity condition:

$$\begin{aligned} \sigma _{ij} n_{j} ={\sigma }'_{ij} {n}'_{j} ,\;u_{i} ={u}'_{i} \end{aligned}$$
(12a, b)

3 Results and Discussion

Table 1 Material properties
Full size table

According to the parameters of common cathode materials [32], the values \(3\times 10^{{4}} {\mathrm { mol/m}}^{{3}}\) and \(\hbox {2.33\,m}^{{3}}{\mathrm {/mol}}\) are selected for \({c}_{\mathrm {max}}\) and \({\Omega }\), respectively. Then, the expansion coefficient of the active material is \({\varOmega c}_{\mathrm {max}}=7\%\) . The value \(1\times 10^{{-13}}\,\mathrm {m}^{{2}}/\hbox {s}\) is selected for the diffusion coefficient of lithium ions in the active particle. The elastic modulus of the active particle is given as \({E}_{\mathrm {AP}}=100\hbox { GPa}\). The value of \({E}_{\mathrm {SE}}\) is fixed at 150 GPa except when examining the influence of different elastic moduli. The values of other constants and parameters for numerical calculations are shown in Table 1. The finite element method is adopted to solve the fully coupled problem. The mechanical module and PDE module in the software COMSOL are applied to solve the initial boundary value problem.

3.1 Evolution of the Coupled Fields During Delithiation and Relaxation

Fig. 2
figure 2

Evolution of concentration in the active particle when a the active particle is in full contact with the solid electrolyte and b 50% contact loss occurs at the interface between the particle and solid electrolyte. The value of \(c_{\mathrm {max }}\) can be found in Table 1

Full size image

Figure 2 shows the evolution of concentration in the active particle during delithiation for full contact (\(\beta =1\)) and 50% contact loss (\(\beta =0.5\)). In the case of 50% contact loss, the upper half surface of the particle (\(\hbox {z}\ge 0\)) stays in contact with the solid electrolyte, while the lower half surface of the particle (\(\hbox {z}< 0\)) is separated from the electrolyte. That is, contact loss occurs at the lower half interface between the active particle and solid electrolyte. The comparison shows that during the entire delithiation, the concentration distribution is more uneven in the active particle with interfacial contact loss. It is interesting that for 50% contact loss, the concentration is high near the contact boundary and relatively low away from the contact boundary, although lithium ions in the particle can get out freely through the contact part of the interface. This concentration distribution is clearly observed as early as at delithiation time \(t=500\hbox { s}\). In the subsequent delithiation, lithium ions can transport to the contact boundary against the concentration gradient. That is, typical uphill diffusion occurs. A similar phenomenon has been observed in all-solid-state lithium-ion batteries via experiments but not explained in detail [33]. In response to this phenomenon, the early delithiation is further analyzed under the condition of 50% contact loss (\(\beta =0.5\)), as shown in Fig. 3.

Fig. 3
figure 3

a Evolution of the concentration and stress in the particle during the first 500 s of delithiation; b evolution of the concentration along the z-axis in the first 500 s of delithiation, where the red triangle represents the maximum concentration point and the black circle represents the minimum concentration point; c change in positions of the maximum and minimum concentration points on the z-axis with time; d evolution of internal stress along the z-axis in the first 500 s of delithiation; e influence of mechanical–chemical coupling on the concentration distribution in the first 500 s of delithiation, where the solid line corresponds to the case that considers the influence of stress on diffusion, while the dotted line corresponds to the case that does not include the effect of stress on diffusion

Full size image

Figure 3a shows the evolution of the lithium concentration and the stress within the active particle during the first 500 s of delithiation for 50% contact loss. To show the change in concentration distribution more clearly, Fig. 3b presents the evolution of the concentration along the z-axis in the first 500 s of delithiation. Before 100 s, the delithiation has just begun and the lithium ions near the contact boundary move out rapidly. As a result, the concentration at the top of the particle is the lowest and the concentration at the bottom of the particle is the highest, as shown in Fig. 3b. However, when the delithiation reaches 150 s, the minimum concentration point has moved down to the lower half of the particle, while the maximum concentration point has moved up. At 500 s, the positions of the maximum and minimum concentration points have been completely swapped: The minimum concentration appears at the bottom of the particle, and the maximum concentration is at the top of the particle. From Fig. 3a, b, it can be seen that as the delithiation continues, the concentration in the upper half of the particle whose boundary remains in contact with the solid electrolyte gradually becomes higher than that in the lower half of the particle where contact loss happens at the boundary and then uphill diffusion appears. Figure 3b shows that this transition of the concentration distribution begins at 100 s and completes at 150 s during delithiation.

For further verification, Fig. 3c shows the positions of the maximum and minimum concentrations on the z-axis as functions of time. The maximum and minimum concentration points start to move at a delithiation time of 100 s. From 100 s to 500 s, the positions of the maximum and minimum concentrations keep varying until they are completely swapped. Before 100 s and after 500 s, the positions of the maximum and minimum concentrations are fixed. The stress contours in Fig. 3a show that the gradient of the stress distribution increases as delithiation occurs. Figure 3d shows the evolution of the stress distribution on the z-axis over time. As delithiation continues, compressive stress begins to appear in the lower half of the particle. The compressive stress and the tensile stress in the upper half of the particle gradually increase. The influence of stress on matter transport cannot be ignored (Fig. 3e). Much research has shown that lithium ions are more likely to diffuse from the compressive stress zone to the tensile stress zone. Considering the evolution of the stress distribution in Fig. 3d, it is concluded that the compressive stress in the lower half of the particle assists lithium ions in flowing rapidly into the upper half of the particle, causing the concentration in the upper half to rise rapidly. As previously mentioned, it is the total potential gradient that drives lithium ions to move. Therefore, the phenomenon of uphill diffusion is essentially the result of competition between chemical driving force and mechanical driving force, which will be discussed next.

Fig. 4
figure 4

Distribution of the total chemical potential \(\mu _{t}\), chemical part \(\mu _{c}\) of the total chemical potential, and mechanical part \(\mu _{m}\) of the total chemical potential along the z-axis at delithiation times of a 5 s, b 50 s, c 150 s, and d 500 s

Full size image

Figure 4 shows the evolution of the chemical potential along the z-axis in the particle during the first 500 s of delithiation. The total chemical potential \({\mu }_{t}\) consists of the chemical part \({\mu }_{c}\) and mechanical part \({\mu }_{m}\). Without loss of generality, the reference chemical potential \(\mu _{0}\) is assumed to be zero. The distribution of \({\mu }_{t}\) correlates with the boundary flux. The contact loss causes changes in the interfacial constraint between the active particle and the solid electrolyte, which complicates the stress distribution in the particle. The nonuniformity of the stress distribution increases significantly as delithiation continues, as shown in Fig. 3a–d. This results in a noticeable increase in the gradient of the mechanical part \({\mu }_{m}\) of the total potential, as shown in Fig. 4. This means the mechanical driving force grows for lithium diffusion. When the boundary flux is fixed, a proper distribution of the total chemical potential \({\mu }_{t}\) should be maintained. However, the gradient of \({\mu }_{m}\) grows quite rapidly. Therefore, to avoid the unexpected rapid increase in the total chemical potential gradient, the gradient of \({\mu }_{c}\) has to be opposite to that of the mechanical part, as shown in Fig. 4c, d. Under the mechanical driving force, the lithium ions eventually transport against the concentration gradient and result in the uphill diffusion.

Fig. 5
figure 5

a Concentration distribution in the active particle before and after relaxation for full contact (\(\beta =1\)) and 50% contact loss (\(\beta =0.5\)) at delithiation time \(t=500\hbox { s}\); b stress distribution in the active particle before and after relaxation for full contact (\(\beta =1\)) and 50% contact loss (\(\beta =0.5\)) at delithiation time \(t=500\hbox { s}\); c evolution of the concentration distribution along the z-axis in the particle for 50% contact loss (\(\beta =0.5\)) during relaxation; d evolution of the stress distribution along the z-axis in the particle for 50% contact loss (\(\beta =0.5\)) during relaxation; e evolution of the total chemical potential distribution along the z-axis in the particle for 50% contact loss (\(\beta =0.5\)) during relaxation; f evolution of the total chemical potential distribution along the z-axis in the particle for full contact (\(\beta =1\)) during relaxation

Full size image

At delithiation time \(t=500\hbox { s}\), the boundary flux is cut off and the active particle is allowed to relax for a sufficient time. Figure 5 presents the evolutions of the concentration and stress within the active particle for 50% contact loss (\(\beta =0.5\)) and full contact (\(\beta =1\)). After relaxation, the concentration and stress distributions in the active particle without contact loss become uniform, as shown in Fig. 5a, b, while in the active particle with half contact loss, the concentration and stress gradients still exist and are even greater than before the relaxation, as shown in Fig. 5c. The boundary flow is zero during the relaxation, which means there is no more exchange of matter between the inside and outside of the particle. However, the gradient of the chemical potential inside the particle still exists to further drive the lithium diffusion until an equilibrium state is reached and the total chemical potential becomes equal everywhere, as shown in Fig. 5e, f. For 50% contact loss, the mechanical part \({\mu }_{m}\) of the total chemical potential still dominates over the chemical part \({\mu }_{c}\) at the beginning of the relaxation, driving lithium ions to continue moving from the lower half of the particle to its upper half, even though the concentration in the lower half is lower than in the upper half. During relaxation, lithium ions no longer escape from the active particle, so the lithium ions coming from the lower half cause a local volume expansion of the upper half and the consequent release of tensile stress in the upper half generated during the previous delithiation, as shown in Fig. 5d. The decrease in the stress gradient causes the gradient of \({\mu }_{m}\) to decrease, as shown in Fig. 5e. The active particle reaches the equilibrium state at a relaxation time of 250 s, and the concentration and stress distributions no longer vary with time (Fig. 5e, f). Because of the nonuniform residual stress, the gradient of \({\mu }_{m}\) does not disappear at the end of relaxation. Therefore, to satisfy the final equilibrium condition, the gradient of \({\mu }_{c}\), which is opposite to that of \({\mu }_{\mathrm {m}}\), has to increase during relaxation and remain at the end of relaxation (Fig. 5e). This explains why the uphill diffusion continues during relaxation in the case of contact loss, as shown in Fig. 5d. For full contact, the distributions of the total chemical potential and its two parts change in a similar way during the relaxation and become uniform in the active particle after relaxation, as shown in Fig. 5f. Uphill diffusion does not appear when no contact loss occurs at the boundary.

Fig. 6
figure 6

a Contour plot of the concentration in the particle at the end of delithiation; b evolution of the concentration distribution along the z-axis before delithiation ends; c evolution of the flux \(q_{z}\) along the z-axis during delithiation; d distributions of the total potential and its two components along the z-axis at 2300 s and the end of delithiation

Full size image

Figure 6a presents the contour plot of the concentration in the particle at the end of delithiation for 50% contact loss. As mentioned above, the concentration in the region close to the contact boundary remains relatively high, and the maximum concentration always appears at the contact boundary, except at the very beginning of the delithiation. However, at the end of delithiation, the concentration in the area close to the contact boundary is quite low and the concentration at the contact boundary becomes the minimum, as shown in Fig. 6a. Figure 6b further shows that when the particle is delithiated to 2200 s, the concentration at the contact boundary is still the maximum, but after 2200 s, it drops fast and first reaches zero at the end of delithiation. As the tensile stress rises in the upper area of the particle before 2200 s, lithium ions move rapidly from the lower area to the upper area. Figure 6c shows that the lithium ion flux in the upper area is even higher than the boundary flux during the first half of delithiation. However, because of the fixed boundary flux, some of the lithium ions are temporarily held back and pile up in the upper area rather than being extracted out, resulting in the high concentration of lithium in this area. As delithiation continues, lithium in the lower part of the particle is gradually evacuated. To continuously satisfy the boundary flux at the late stage of delithiation, it is necessary to consume the lithium in the area of high concentration. Therefore, there is a substantial decrease in the concentration in the upper half of the particle, especially in the area close to the contact boundary (Fig. 6b), and the concentration at the contact boundary first drops to zero and triggers the termination of delithiation. This is consistent with the variation in the chemical potential. Figure 6d shows that \({\mu }_{m}\) still dominates the total chemical potential at 2300 s. However, at the end of delithiation, \({\mu }_{c}\) or the concentration gradient becomes the main driving force for lithium diffusion.

Fig. 7
figure 7

a Evolution of the concentration distribution along the z-axis during relaxation after delithiation stops; b evolution of the stress distribution along the z-axis near the end of delithiation; c evolution of the stress distribution along the z-axis during relaxation after delithiation stops; d evolution of the distribution of the total chemical potential and its two components along the z-axis during relaxation after delithiation stops

Full size image

As delithiation proceeds, the tensile stress and stress gradient in the particle continue to increase. The maximum tensile stress at the end of delithiation (Fig. 7b) is nearly an order of magnitude higher than that at 500 s of delithiation. The stress generated in the particle is affected by two factors, namely the concentration gradient and interfacial constraint by the solid electrolyte. As delithiation continues, the influence of the interfacial constraint on the internal stress gradually increases. In the later stage of delithiation, the influence exerted by the interfacial constraint becomes dominant, while the effect of the concentration gradient becomes negligible. Figure 7a shows that after relaxation begins, the concentration in most areas starts to decrease, while the concentration in the area close to the contact boundary begins to increase quickly. More uphill diffusion occurs, which is similar to the relaxation after delithiation for 500 s. During the relaxation, the influence of the interfacial constraint on stress stops increasing and stays nearly the same as that at the end of delithiation. Therefore, as explained above, the stress distribution hardly changes during the relaxation owing to the negligible influence of the concentration gradient, as shown in Fig. 7c. However, during the relaxation after delithiation for 500 s, the stress in the particle is affected by the change in the concentration distribution because the influence of the interfacial constraint is small. There is no change in stress distribution during the relaxation after delithiation for 2300 s, so the distribution of \({\mu }_{m}\) remains basically unchanged, as shown in Fig. 7d. After the particle is fully relaxed, all systems in the particle should come to equilibrium and the total chemical potential should be equal everywhere. The distribution of \({\mu }_{m}\) remains unchanged during relaxation, so the gradient of \({\mu }_{c}\), which is opposite to that of \({\mu }_{m}\), must increase until the end of relaxation, as shown in Fig. 7d.

3.2 Influence of the Degree of Contact Loss

Fig. 8
figure 8

Influence of the degree of contact loss on a the concentration distribution in the particle, b the stress distribution in the particle, c the concentration distribution along the z-axis, and d the stress distribution along the z-axis. The delithiation time is \(t=1000\hbox { s}\)

Full size image

Figure 8 shows the influence of the degree of contact loss on the concentration and stress in the particle at delithiation time \(t=1000\hbox { s}\). The gradients of the concentration and stress distributions in the particle with contact loss (\(\beta \ne 1\)) are more obvious than when the particle has full contact (\(\beta =1\)) with the solid electrolyte. The concentration and stress distributions in the particle without contact loss have insignificant gradients. For full contact, the tensile stress at the center is low and that at the boundary is high; the concentration at the center is high, while that at the boundary is low, which means no uphill diffusion occurs. Furthermore, the overall tensile stress in the particle increases with decreasing contact loss, as shown in Fig. 8b, d. Driven by the mechanical driving force, lithium ions flow rapidly from the compressive stress area (the area with lower tensile stress) into the tensile stress area (the area with higher tensile stress), causing the local concentration in the former to decrease sharply. This phenomenon is especially obvious for large degrees of contact loss, as shown in Fig. 8a, c.

3.3 Influence of the Type of Solid Electrolyte

Table 2 Elastic moduli of different solid electrolytes
Full size table

There are many kinds of solid electrolytes. Table 2 lists the elastic moduli of several solid electrolyte materials commonly used. Among them, the elastic moduli of the oxide electrolytes are relatively large, especially the crystalline oxide electrolytes such as perovskite and garnet, whose elastic moduli are usually 100-200 GPa. It is usually difficult to compact and sinter such rigid electrolytes during preparation of an electrode, and contact loss between the active material and electrolyte is very likely [40, 41]. In contrast, most sulfide solid electrolytes are soft, with common elastic moduli of 10-40 GPa. Mechanical pressure can be applied to improve their poor contact with active materials [42]. Figure 9 shows the influence of the elastic modulus of the solid electrolyte on the concentration and stress distributions during delithiation for 50% contact loss. The values of the modulus \(E_{\mathrm {SE}}\) of the solid electrolyte are 15 GPa, 50 GPa, 100 GPa, 150 GPa, and 200 GPa. It can be seen that a more rigid solid electrolyte more strongly restricts deformation of the active particle, which leads to a higher tensile stress and larger stress distribution gradient in the particle. The high tensile stress at the contact boundary is likely to crack and damage a brittle electrolyte material [43, 44]. A soft electrolyte weakly constrains and better adapts to the deformation of the active material. However, electrolytes should not be too soft because a solid electrolyte with a relatively high modulus can better suppress growth of lithium dendrites [45]. Figure 9 also shows that for particles with contact loss with the solid electrolyte, the uphill diffusion and concentration gradient increase as the elastic modulus of the solid electrolyte increases.

Fig. 9
figure 9

Influence of the elastic modulus of the solid electrolyte on a the concentration distribution in the particle, and b the stress distribution in the particle. The degree of contact loss is \(\beta =0.5\), and the delithiation time is \(t=1000\hbox { s}\)

Full size image

4 Conclusion

We have simulated the mechanical–chemical coupling during delithiation and relaxation of a cathode in a solid-state lithium-ion battery. Contact loss at the interface between the active particle and solid electrolyte was considered. Uphill diffusion appears early during delithiation and continues during the rest of delithiation and the following relaxation. This phenomenon was explained by analyzing the chemical potential. Contact loss at the interface changes the degree to which the solid electrolyte constrains the active particle and greatly influences the stress and stress gradient in the active particle. As delithiation proceeds, the stress and stress gradient become considerably large, and the mechanical part of the total chemical potential becomes dominant over the chemical part, which leads to the uphill diffusion. In the latter stage of delithiation, the influence of the interfacial constraint on the stress becomes dominant, while the effect of the concentration gradient becomes negligible. After relaxation, the concentration and stress do not become uniform in a particle with contact loss, and their gradients become even greater than before relaxation. The influence of the degree of contact loss on the mechanical–chemical coupling was investigated. The overall tensile stress in the active particle increases with decreasing contact loss, causing a sharp decrease in local concentration. We also checked the effect of the elastic modulus of the solid electrolyte on the coupling of the active material. A more rigid solid electrolyte more strongly restricts the active particle, which leads to a higher tensile stress and larger stress gradient in the particle. As the elastic modulus of the solid electrolyte increases, both the uphill diffusion and concentration gradient increase.