1 Introduction

The shear strength of a soil is influenced by the particle size distribution, particle shape, water content, direction of principal stresses, intermediate principal stress and so forth. When a slope is excavated, the soil is often subjected to three various principal stresses. Hollow cylinder torsion tests and true triaxial compression tests can be used to measure the shear responses of the soil in a three-dimensional stress state. A parameter is often employed to quantify the relative magnitude of the intermediate principal stress. The parameter is defined as:

$$ b = {{\left( {\sigma_{2} - \sigma_{3} } \right)} \mathord{\left/ {\vphantom {{\left( {\sigma_{2} - \sigma_{3} } \right)} {\left( {\sigma_{1} - \sigma_{3} } \right)}}} \right. \kern-\nulldelimiterspace} {\left( {\sigma_{1} - \sigma_{3} } \right)}} $$
(1)

where \(\sigma_{1}\), \(\sigma_{2}\) and \(\sigma_{3}\) are the major, intermediate and minor principal stresses, respectively. Some experimental studies [1,2,3,4,5,6] were centered on the effect of the b value on the shear responses of soils. These studies indicated that both the peak and critical friction angles first increase and then decrease as the b value increases. However, these experimental studies mainly focused on the macroscale mechanical responses rather than the microscale mechanical responses. The discrete element method (DEM) is a good method for obtaining the macroscale and microscale mechanical responses of granular materials [7,8,9]. Many investigators [10,11,12,13,14,15,16,17,18] have employed the DEM to numerically investigate the effect of the b value on the shear responses of granular materials. In these numerical studies, the microscale mechanical responses, including the coordination number, sliding contacts, principal fabrics, particle rotations, and geometrical and mechanical anisotropies, were examined. However, the object of these numerical studies is the granular materials with narrow particle size distributions rather than the binary granular mixtures with wide particle size distributions.

Some natural slopes are composed of binary granular mixtures. The coarse particles and fine particles are the two components of binary granular mixtures. The coarse particles can be rocks, gravels and sands, and the fine particles can be sands, silts and clays [19,20,21]. The properties of binary granular mixtures are more complex than those of ordinary homogeneous geotechnical materials because of the nature of two components. Therefore, it is particularly important to investigate binary granular mixtures. Although some researchers [19,20,21,22,23,24,25,26] have studied binary granular mixtures, few studies have been conducted on the effect of the b value on the macroscale and microscale mechanical responses of binary granular mixtures via the DEM. The contacts in a binary granular mixture can be categorized as coarse–coarse (cc), coarse–fine (cf) and fine–fine (ff) contacts. According to the percentage contributions of the three contact types to the shear strength, three thresholds of the fine content (FC) were used to classify binary granular mixtures as four types, i.e., “underfilled”, “interactive-underfilled”, “interactive-overfilled”, and “overfilled” [27]. Note that the terms “underfilled” and “overfilled” used in dam engineering [28] were used. However, it is not clear whether the b value affects the classification of binary granular mixtures. In addition, the mechanisms by which the b value affects the macroscale shear behaviors of binary granular mixtures with different FCs are not clear. Based on the above considerations, numerical simulations about the effect of the b value on the shear responses of binary granular mixtures were performed using the DEM in this study.

The main aim of this study is to systemically analyze the effect of the intermediate principal stress on the shear responses of binary granular mixtures with different FCs. Many true triaxial compression tests were performed on binary granular mixtures via the DEM. This work was arranged as follows. First, the numerical simulation process was presented. Then, the macroscale responses, contributions of contact types, microscale responses, and anisotropies of contacts and contact forces were examined. Lastly, some main conclusions were obtained.

2 Numerical simulation process

2.1 Coarse and fine particles

Binary granular mixtures were composed of coarse and fine particles. In this study, the coarse and fine particles can be viewed as gravels and sands, respectively. The geometries of coarse particles can be obtained by scanning real gravels with a three-dimensional scanner called Wiiboox Reeyee. The scan file for each gravel is a file suffixed by stl. 36 gravels with various shapes were scanned, and their scan images are shown in Fig. 1. Each scan image has 1500 triangular meshes. Then, the scan files were imported into PFC3D [29], and the geometries of the gravels were filled with overlapping subspheres [30]. Accordingly, these multisphere particles approximately replaced coarse particles. The number of subspheres of each coarse particle is 168–362. In reality, some sands are round in shape, such as Ottawa sands [31,32,33]. In addition, the use of spherical fine particles can significantly improve the computational efficiency. Based on the two facts, the fine particles were treated as spherical particles in this study. Note that all particles are unbreakable. The volume of a coarse particle is equal to that of a sphere with a diameter of 15 mm, and the diameter of a fine particle is 3.37 mm. Accordingly, a particle size ratio of 4.45 was used in this study, consistent with that of Lopez et al. [27, 34]. Lade et al. [35] and Shire et al. [36] found a phenomenon. The minimum void ratio of a binary granular mixture (FC ≈ 30%) decreases sharply in the beginning as the particle size ratio increases. When the particle size ratio is beyond 6.5, the effect of the particle size ratio on the minimum void ratio is much less pronounced. Thus, the behaviors of binary granular mixtures can be expected to change as the particle size ratio exceeds 6.5. The FC ranges from 0 to 100% in intervals of 10%. Before the specimen generation, the number of coarse particles was given. Accordingly, the number of fine particles could be determined according to the FC. For all binary granular mixtures, the numbers of coarse and fine particles are shown in Table 1.

Fig. 1
figure 1

Scan images of 36 differently shaped gravels

Full size image
Table 1 Basic information of the specimens
Full size table

2.2 Specimen generation

The DEM program PFC3D [29] was employed to execute the numerical true triaxial compression tests. The linear contact model was employed to describe the inter-particle and wall-particle interactions. Table 2 provides the parameters of the linear contact model. Horn and Deere [37] discovered that the friction coefficient of saturated quartz particles is 0.42–0.45. Rowe [38] found that the friction coefficient of saturated quartz particles is 0.40–0.60. Senetakis et al. [39, 40] discovered that the friction coefficient of saturated quartz particles is 0.12–0.35. In this study, the inter-particle friction coefficient during the test was set to 0.5, identical with that used by Guo and Zhao [41, 42], Gu et al. [43] and Gong et al. [24]. Coetzee [44] calibrated the effective modulus of the contacts between crushed rock particles as 0.38 × 108 Pa to 1.14 × 108 Pa using PFC3D. In this study, the effective modulus was set to 1.0 × 108 Pa, identical with that used by Gong et al. [24] and approximate to that used by Guo and Zhao [41, 42] and Gu et al. [43]. Goldenberg and Goldhirsch [45] deemed that the normal-to-tangential stiffness ratio should be in the range of (1.0, 1.5) for real granular materials. In this study, the normal-to-tangential stiffness ratio was set to 4/3. Jiang et al. [46] pointed out that the damping factor has no significant effect on a quasi-static test and selected its value as 0.7 by trial and error. In this study, the damping factor was set to 0.7, identical with that used by Gu et al. [43] and Gong et al. [24].

Table 2 Parameters of the linear contact model
Full size table

First, all particles without overlap were stochastically distributed in a large cube area formed by six rigid walls. To obtain isotropically dense specimens, the gravitational acceleration and the inter-particle and wall-particle friction coefficients were temporarily remained at zero. Then, the walls and particles were moved inward. To stop particles from exiting the cube area, all particles were calmed every 10 steps until 20,000 steps. Subsequently, a servo-control mechanism was employed to isotropically compress all particles. Finally, if the ratio of the average unbalanced force to the average contact force was smaller than 1.0 × 10–4 and the deviation of the wall stress from 200 kPa was smaller than 1 kPa, the specimen equilibrated. Frictionless particles were used, thus all specimens had a relative density of 100%, in line with Lopez et al. [27, 34]. The dimensions and initial porosities of all specimens before shear are also included in Table 1. Figure 2 presents the specimens of binary granular mixtures with FC = 20%, 40% and 60% before shear.

Fig. 2
figure 2

Specimens of binary granular mixtures with FC = 20%, 40% and 60% before shear

Full size image

2.3 True triaxial compression

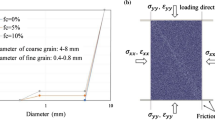
When the specimen generation was completed, the inter-particle friction coefficient was adjusted to 0.5 and the wall-particle friction coefficient was remained at zero. In drained true triaxial compression tests, \(\sigma_{1}\) was changed by shifting the top wall down at an invariable velocity and \(\sigma_{3}\) was maintained at 200 kPa by a servo-control mechanism. \(\sigma_{2}\) varied with \(\sigma_{1}\) by a servo-control mechanism, meanwhile, the b value remained constant. In this study, b = 0, 0.2, 0.4, 0.6, 0.8 and 1. The loading rate should be small enough to make tests quasi-static. This can be described by the inertia parameter I [47], which should be less than 2.5 × 10–3 [48], as follows:

$$ I = \frac{{{\text{d}}\varepsilon_{1} }}{{{\text{d}}t}}d\sqrt {{\rho \mathord{\left/ {\vphantom {\rho {\sigma_{3} }}} \right. \kern-\nulldelimiterspace} {\sigma_{3} }}} $$
(2)

where \(\frac{{{\text{d}}\varepsilon_{1} }}{{{\text{d}}t}}\) denotes the axial strain rate, \(d\) denotes the mean of the diameters of all particles, and \(\rho\) denotes the particle density. The loading rate of the top wall was set to 0.05 m/s, therefore \(I\) was smaller than 1.0 × 10–3 in this study. Following Zhou et al. [18], the true triaxial compression tests were terminated when the axial strain \(\varepsilon_{1}\) reached 40%.

3 Analyses of simulation results

3.1 Macroscale responses

Using the microscale quantities in an entire specimen, the stress tensor \(\sigma_{{{\text{ij}}}}\) is determined by [49]:

$$ \sigma_{ij} = \frac{1}{V}\sum\limits_{c = 1}^{C} {f_{i}^{c} } d_{j}^{c} $$
(3)

where \(V\) denotes the volume of the specimen, \(C\) denotes the number of contacts, and \(f_{i}^{c}\) and \(d_{j}^{c}\) denote the ith component of the contact force and the jth component of the branch vector at contact c, respectively. The average stress \(p\) and the deviatoric stress \(q\) are defined as:

$$ p = {{\sigma_{ii} } \mathord{\left/ {\vphantom {{\sigma_{ii} } 3}} \right. \kern-\nulldelimiterspace} 3} $$
(4)
$$ q = \sqrt {\frac{3}{2}\sigma_{ij}^{\prime } \sigma_{ij}^{\prime } } $$
(5)

where \(\sigma_{ij}^{\prime }\) denotes the deviatoric part of \(\sigma_{ij}\). In this study, the friction angle \(\phi\) of binary granular mixtures is calculated by:

$$ \sin \phi = \frac{{\sigma_{1} - \sigma_{3} }}{{\sigma_{1} + \sigma_{3} }} = \frac{{\left( {{q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}} \right) \cdot {1 \mathord{\left/ {\vphantom {1 {\left( {1 - b + b^{2} } \right)^{0.5} }}} \right. \kern-\nulldelimiterspace} {\left( {1 - b + b^{2} } \right)^{0.5} }}}}{{2 + \left( {{q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}} \right) \cdot {{\left( {{1 \mathord{\left/ {\vphantom {1 {3 - {{2b} \mathord{\left/ {\vphantom {{2b} 3}} \right. \kern-\nulldelimiterspace} 3}}}} \right. \kern-\nulldelimiterspace} {3 - {{2b} \mathord{\left/ {\vphantom {{2b} 3}} \right. \kern-\nulldelimiterspace} 3}}}} \right)} \mathord{\left/ {\vphantom {{\left( {{1 \mathord{\left/ {\vphantom {1 {3 - {{2b} \mathord{\left/ {\vphantom {{2b} 3}} \right. \kern-\nulldelimiterspace} 3}}}} \right. \kern-\nulldelimiterspace} {3 - {{2b} \mathord{\left/ {\vphantom {{2b} 3}} \right. \kern-\nulldelimiterspace} 3}}}} \right)} {\left( {1 - b + b^{2} } \right)^{0.5} }}} \right. \kern-\nulldelimiterspace} {\left( {1 - b + b^{2} } \right)^{0.5} }}}} $$
(6)

The axial strain \(\varepsilon_{{1}}\), the lateral strains \(\varepsilon_{{2}}\) and \(\varepsilon_{{3}}\), the volumetric strain \(\varepsilon_{{\text{v}}}\), and the deviatoric strain \(\varepsilon_{{\text{d}}}\) are given by:

$$ \varepsilon_{1} = {{\left( {L_{1,0} - L_{1} } \right)} \mathord{\left/ {\vphantom {{\left( {L_{1,0} - L_{1} } \right)} {L_{1,0} }}} \right. \kern-\nulldelimiterspace} {L_{1,0} }} $$
(7a)
$$ \varepsilon_{2} = {{\left( {L_{2,0} - L_{2} } \right)} \mathord{\left/ {\vphantom {{\left( {L_{2,0} - L_{2} } \right)} {L_{2,0} }}} \right. \kern-\nulldelimiterspace} {L_{2,0} }} $$
(7b)
$$ \varepsilon_{3} = {{\left( {L_{3,0} - L_{3} } \right)} \mathord{\left/ {\vphantom {{\left( {L_{3,0} - L_{3} } \right)} {L_{3,0} }}} \right. \kern-\nulldelimiterspace} {L_{3,0} }} $$
(7c)
$$ \varepsilon_{{\text{v}}} = {{\left( {V_{0} - V} \right)} \mathord{\left/ {\vphantom {{\left( {V_{0} - V} \right)} {V_{0} }}} \right. \kern-\nulldelimiterspace} {V_{0} }} $$
(8)
$$ \varepsilon_{{\text{d}}} = \frac{\sqrt 2 }{3}\sqrt {\left( {\varepsilon_{1} - \varepsilon_{2} } \right)^{2} + \left( {\varepsilon_{2} - \varepsilon_{3} } \right)^{2} + \left( {\varepsilon_{3} - \varepsilon_{1} } \right)^{2} } $$
(9)

where \(L_{1,0}\), \(L_{2,0}\) and \(L_{3,0}\) are the dimensions of the specimen before shear, respectively; \(L_{1}\), \(L_{2}\) and \(L_{3}\) are the dimensions of the specimen during the test, respectively; \(V_{0}\) and \(V\) are the volumes of the specimen before shear and during the test, respectively. The dilatancy angle \(\psi\) is given by [50]:

$$ \sin \psi = - \frac{{{{{\text{d}}\varepsilon_{{\text{v}}} } \mathord{\left/ {\vphantom {{{\text{d}}\varepsilon_{{\text{v}}} } {{\text{d}}\varepsilon_{{1}} }}} \right. \kern-\nulldelimiterspace} {{\text{d}}\varepsilon_{{1}} }}}}{{2 - {{{\text{d}}\varepsilon_{{\text{v}}} } \mathord{\left/ {\vphantom {{{\text{d}}\varepsilon_{{\text{v}}} } {{\text{d}}\varepsilon_{{1}} }}} \right. \kern-\nulldelimiterspace} {{\text{d}}\varepsilon_{{1}} }}}} $$
(10)

To study the size effect, three specimens (FC = 30%) with different sizes were prepared and tested (b = 0). The dimensions of three specimens are 131 × 130 × 130 mm, 164 × 163 × 163 mm and 206 × 205 × 206 mm, respectively. Namely, the ratios of the minimum specimen size to the maximum particle size are 8.7, 10.9 and 13.7, respectively. The computational efficiency of the largest specimen is extremely low, therefore the largest specimen was not compressed to the axial strain of 40%. Figure 3 shows the evolutions of the stress ratio \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\) and volumetric strain \(\varepsilon_{{\text{v}}}\) versus the axial strain \(\varepsilon_{1}\) for the specimens with different sizes when FC = 30% and b = 0. Clearly, the specimen size has a slight effect on \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p} - \varepsilon_{1}\) and \(\varepsilon_{{\text{v}}} - \varepsilon_{1}\) relationships of binary granular mixtures. Nie et al. [51] found that the specimen size has a slight effect on \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p} - \varepsilon_{1}\) and \(\varepsilon_{{\text{v}}} - \varepsilon_{1}\) relationships when the ratio of the minimum specimen size to the maximum particle size ranges from about 10 to about 20. Hence, it is reasonable to use the smallest specimen (131 × 130 × 130 mm) to improve the computational efficiency. To exclude the specimen size effect, Jamiolkowski et al. [52] suggested that the ratio of the minimum specimen size to the maximum particle size should be at least 5 and that the ideal ratio was 8. ASTM suggested that the ratio should not be less than 6. The industry standard of China: Code for Soil Test of Railway Engineering (TB 10102-2010, J 1135-2010) suggested that the ratio should not be less than 5. In this paper, the ratios of all specimens are larger than 8.5. Based on the above numerical simulation results and those recommendations for laboratory tests, it can be concluded that the size effect in this study is very limited. In future work, the numerical simulations of much larger specimens are needed to further confirm the size effect.

Fig. 3
figure 3

Evolutions of the a stress ratio and b volumetric strain versus the axial strain for the specimens with different sizes when FC = 30% and b = 0

Full size image

Figure 4 presents the evolutions of the stress ratio \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\) with the axial strain \(\varepsilon_{1}\) for binary granular mixtures with FC = 10% and FC = 50%. With increasing \(\varepsilon_{1}\), \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\) increases rapidly to a crest and then slowly decreases to a basically steady value. Additionally, the b value affects \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\); that is, \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\) generally decreases with an increase in the b value. Figure 5 illustrates the variations in the stress ratio \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\) with the FC at the peak and critical states. As shown in Fig. 5a, with increasing FC, \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\) gradually decreases to a basically steady value; with increasing b, \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\) generally decreases. The variation trends of the curves in Fig. 5b basically resemble those in Fig. 5a. Using Eq. (6), the peak friction angle \(\phi_{{\text{p}}}\) and the critical friction angle \(\phi_{{\text{c}}}\) were calculated, as shown in Fig. 6. In Fig. 6a, for a specific FC, as the b value increases, \(\phi_{{\text{p}}}\) first increases to a crest and then decreases. For a specific b, when FC ≤ 40%, \(\phi_{{\text{p}}}\) decreases with increasing FC; when FC ≥ 50%, \(\phi_{{\text{p}}}\) varies within a very small range. The variation trends of \(\phi_{{\text{c}}}\) with respect to the b value and the FC in Fig. 6b are roughly similar to those of \(\phi_{{\text{p}}}\) in Fig. 6a. O’Sullivan et al. [13] used a simple buckling model to explain why the b value influences the friction angles of granular materials. When the b value changes, there is a change in the lateral support provided by the force chains along the directions of intermediate and minor principal stresses; the change in the lateral support directly influences the buckling of strong force chains along the direction of the major principal stress. For a binary granular mixture with a specific FC and a specific shear state, with increasing b, the maximum and minimum friction angles (i.e., \(\phi_{\max }\) and \(\phi_{{{\text{m}} {\text{in}}}}\)) were observed. Note that \(\phi_{{{\text{m}} {\text{in}}}}\) always occurs at b = 0, whereas \(\phi_{\max }\) always occurs at b = 0.4 or b = 0.6.

Fig. 4
figure 4

Evolutions of the stress ratio with the axial strain for binary granular mixtures with a FC = 10% and b FC = 50%

Full size image
Fig. 5
figure 5

Variations in the stress ratio with the FC at the a peak and b critical states

Full size image
Fig. 6
figure 6

Variations in the a peak and b critical friction angles with the b value

Full size image

To compare the mechanical responses of the binary granular mixtures with different coarse particle shapes, additional numerical simulations were performed on the binary granular mixtures (FC = 0%, 10%, 20%, 30%, 40%, 60%, 80% and 100%) with spherical coarse particles. For the sake of conciseness, Fig. 7a presents the variation in the friction angle versus the FC when b = 0.4. For mixtures with real gravel-shaped coarse particles, with increasing FC, both \(\phi_{{\text{p}}}\) and \(\phi_{{\text{c}}}\) gradually decreases to a generally steady value. For mixtures with spherical coarse particles, \(\phi_{{\text{p}}}\) shows its maximum at FC = 30%, whereas the FC has a negligible effect on \(\phi_{{\text{c}}}\). Additionally, when FC ≤ 40%, for a given FC, the friction angle of a mixture with real gravel-shaped coarse particles is clearly larger than that of a mixture with spherical coarse particles. Figure 7b presents the variation in the difference between the maximum and minimum friction angles \(\phi_{\max } - \phi_{\min }\) versus the FC with increasing b. For mixtures with real gravel-shaped coarse particles, when FC ≤ 40%, as the FC increases, \(\phi_{p\max } - \phi_{p\min }\) (\(\phi_{\max } - \phi_{\min }\) at the peak state) gradually decreases; when FC ≥ 40%, as the FC increases, \(\phi_{p\max } - \phi_{p\min }\) is generally stable. For mixtures with spherical coarse particles, the FC has a negligible effect on \(\phi_{p\max } - \phi_{p\min }\). Regardless of the shapes of coarse particles, the FC has a negligible effect on \(\phi_{{{\text{c}}\max }} - \phi_{{{\text{c}}\min }}\) (\(\phi_{\max } - \phi_{\min }\) at the critical state). In addition, for a given FC, \(\phi_{p\max } - \phi_{p\min }\) is larger than \(\phi_{{{\text{c}}\max }} - \phi_{{{\text{c}}\min }}\). Figure 7 indicates that the shapes of coarse particles play an important role in the peak and critical friction angles of binary granular mixtures.

Fig. 7
figure 7

a Variation in the friction angle versus the FC when b = 0.4; b variation in the difference between the maximum and minimum friction angles versus the FC with increasing b

Full size image

Figure 8 presents the evolutions of the volumetric strain \(\varepsilon_{{\text{v}}}\) versus the axial strain \(\varepsilon_{{1}}\) for binary granular mixtures with FC = 10% and FC = 50%. With increasing \(\varepsilon_{{1}}\), the specimen initially contracts and then dilates. In the initial loading stage, the contraction of the specimen increases with increasing b. As the loading continues (\(\varepsilon_{{1}}\) ≤ 20% for FC = 10%, \(\varepsilon_{{1}}\) ≤ 10% for FC = 50%), the dilation of the specimen increases with increasing b. In the final loading stage, the dilation of the specimen first decreases and then increases with increasing b. Figure 9 presents the evolution of the dilatancy angle \(\psi\) versus the axial strain \(\varepsilon_{{1}}\) for the binary granular mixture with FC = 50%. As \(\varepsilon_{{1}}\) increases, \(\psi\) first increases to a crest and then decreases to a value close to zero. It was observed that the b value affects the peak dilatancy angle. Figure 10 shows the variation in the peak dilatancy angle \(\psi_{{\text{p}}}\) versus the b value. As the b value increases, \(\psi_{{\text{p}}}\) roughly linearly increases. For a given b value, the \(\psi_{{\text{p}}}\) values of the binary granular mixtures with FC ≤ 20% are obviously larger than those of the binary granular mixtures with FC ≥ 30%. For a binary granular mixture with a specific FC, with increasing b, the maximum and minimum peak dilatancy angles (i.e., \(\psi_{{{\text{p}}\max }}\) and \(\psi_{{{\text{p}}\min }}\)) were observed. Note that \(\psi_{{{\text{p}}\min }}\) always occurs at b = 0, whereas \(\psi_{{{\text{p}}\max }}\) always occurs at b = 1.

Fig. 8
figure 8

Evolutions of the volumetric strain versus the axial strain for binary granular mixtures with a FC = 10% and b FC = 50%

Full size image
Fig. 9
figure 9

Evolution of the dilatancy angle versus the axial strain for the binary granular mixture with FC = 50%

Full size image
Fig. 10
figure 10

Variation in the peak dilatancy angle versus the b value

Full size image

For the sake of conciseness, Fig. 11a presents the variation in the peak dilatancy angle versus the FC when b = 0.4. For mixtures with real gravel-shaped coarse particles, the \(\psi_{{\text{p}}}\) values of mixtures with FC ≤ 20% are obviously larger than those of mixtures with FC ≥ 30%. For mixtures with spherical coarse particles, \(\psi_{{\text{p}}}\) shows its maximum at FC = 30%. Additionally, when FC ≤ 20%, for a given FC, \(\psi_{{\text{p}}}\) of a mixture with real gravel-shaped coarse particles is clearly larger than that of a mixture with spherical coarse particles. Figure 11b presents the variation in the difference between the maximum and minimum peak dilatancy angles \(\psi_{{{\text{pmax}}}} - \psi_{{{\text{pmin}}}}\) versus the FC with increasing b. For mixtures with real gravel-shaped coarse particles, when FC ≤ 30%, \(\psi_{{{\text{pmax}}}} { - }\psi_{{{\text{pmin}}}}\) roughly decreases with increasing FC; with a further increase in the FC, there is no significant change in \(\psi_{{{\text{pmax}}}} - \psi_{{{\text{pmin}}}}\). For mixtures with spherical coarse particles, the FC has a negligible effect on \(\psi_{{{\text{pmax}}}} - \psi_{{{\text{pmin}}}}\). Figure 11 indicates that the shapes of coarse particles play an important role in the peak dilatancy angles of binary granular mixtures.

Fig. 11
figure 11

a Variation in the peak dilatancy angle versus the FC when b = 0.4; b variation in the difference between the maximum and minimum peak dilatancy angles versus the FC with increasing b

Full size image

In this study, a concept called “change in the void ratio” \(R_{{{\text{vc}}}} - R_{{{\text{vi}}}}\) was used, where \(R_{{{\text{vc}}}}\) and \(R_{{{\text{vi}}}}\) are the void ratios of a specimen at the critical and initial states, respectively. Figure 12a and b show the relationships between the change in the void ratio \(R_{{{\text{vc}}}} - R_{{{\text{vi}}}}\) and the peak friction angle \(\phi_{{\text{p}}}\) and the peak dilatancy angle \(\psi_{{\text{p}}}\). Obviously, for a given b value, the data for binary granular mixtures with different FCs can be fitted by \(\phi_{{\text{p}}} = m\left( {R_{{{\text{vc}}}} - R_{{{\text{vi}}}} } \right) + n\) or \(\psi_{{\text{p}}} = k\left( {R_{{{\text{vc}}}} - R_{{{\text{vi}}}} } \right) + l\). This finding means that a larger \(R_{{{\text{vc}}}} - R_{{{\text{vi}}}}\) will lead to a larger \(\phi_{{\text{p}}}\) and \(\psi_{{\text{p}}}\) for a given b value. The insets show the variations in the slope and the intercept versus the b value. In the insets in Fig. 12a, with increasing b, m increases to a plateau and n initially increases and then decreases. In the insets in Fig. 12b, with increasing b, both k and l increase. These findings mean that the two linear relationships depend on the b value. Figure 12c shows the relationship between the change in the void ratio \(R_{{{\text{vc}}}} - R_{{{\text{vi}}}}\) and the modified peak dilatancy angle \({{\psi_{{\text{p}}} } \mathord{\left/ {\vphantom {{\psi_{{\text{p}}} } {{\text{e}}^{0.52b} }}} \right. \kern-\nulldelimiterspace} {{\text{e}}^{0.52b} }}\). Obviously, the data can be fitted by \({{\psi_{{\text{p}}} } \mathord{\left/ {\vphantom {{\psi_{{\text{p}}} } {{\text{e}}^{0.52b} }}} \right. \kern-\nulldelimiterspace} {{\text{e}}^{0.52b} }}{ = 3}0.725\left( {R_{{{\text{vc}}}} { - }R_{{{\text{vi}}}} } \right){ + }14.424\). This finding indicates that the acceptable equation \(\psi_{{\text{p}}} {\text{ = e}}^{0.52b} \left[ {{3}0.725\left( {R_{{{\text{vc}}}} { - }R_{{{\text{vi}}}} } \right){ + }14.424} \right]\) can be used to predict the peak dilatancy angle of a binary granular mixture with a given b value and a given FC.

Fig. 12
figure 12

Relationships between the change in the void ratio and the a peak friction angle, b peak dilatancy angle and c modified peak dilatancy angle

Full size image

Figure 13 presents the evolutions of the lateral strains (\(\varepsilon_{{2}}\) and \(\varepsilon_{3}\)) and the deviatoric strain \(\varepsilon_{{\text{d}}}\) with the axial strain \(\varepsilon_{{1}}\) for the binary granular mixture with FC = 50%. In Fig. 13a, when b ≤ 0.2, \(\varepsilon_{{2}}\) decreases with increasing \(\varepsilon_{{1}}\); when b = 0.4, \(\varepsilon_{{2}}\) generally remains at 0 with increasing \(\varepsilon_{{1}}\); when b ≥ 0.6, \(\varepsilon_{{2}}\) increases with increasing \(\varepsilon_{{1}}\). Additionally, \(\varepsilon_{{2}}\) increases with an increase in the b value. In Fig. 13b, \(\varepsilon_{{3}}\) decreases with increasing \(\varepsilon_{{1}}\); \(\varepsilon_{{3}}\) decreases with an increase in the b value. In Fig. 13c, \(\varepsilon_{{\text{d}}}\) increases with increasing \(\varepsilon_{{1}}\); \(\varepsilon_{{\text{d}}}\) increases with increasing b. Zhao and Guo [53] and Zhou et al. [54] used a parameter to characterize the coaxiality of the contacts and stresses. Similarly, a parameter can be used to characterize the coaxiality of the strain increments and stresses. The coaxiality parameter can be defined as:

$$ A = \frac{{tr\left( {\alpha_{ij}^{\prime } \beta_{ij}^{\prime } } \right)}}{{\sqrt {\alpha_{ij}^{\prime } \alpha_{ij}^{\prime } } \sqrt {\beta_{ij}^{\prime } \beta_{ij}^{\prime } } }} $$
(11)
Fig. 13
figure 13

Evolutions of the lateral strains and the deviatoric strain with the axial strain for the binary granular mixture with FC = 50%

Full size image

where \(\alpha_{ij}^{\prime }\) and \(\beta_{ij}^{\prime }\) are the deviatoric tensors of \(\alpha_{ij}\) and \(\beta_{ij}\), respectively. The expressions of \(\alpha_{ij}\) and \(\beta_{ij}\) are as follows:

$$ \alpha_{ij} { = }\left[ {\begin{array}{*{20}c} {{\text{d}}\varepsilon_{1} } & {} & {} \\ {} & {{\text{d}}\varepsilon_{2} } & {} \\ {} & {} & {{\text{d}}\varepsilon_{3} } \\ \end{array} } \right] $$
(12a)
$$ \beta_{ij} { = }\left[ {\begin{array}{*{20}c} {\sigma_{1} } & {} & {} \\ {} & {\sigma_{2} } & {} \\ {} & {} & {\sigma_{3} } \\ \end{array} } \right] $$
(12b)

where \({\text{d}}\varepsilon_{1}\), \({\text{d}}\varepsilon_{2}\) and \({\text{d}}\varepsilon_{3}\) are the strain increments corresponding to \(\varepsilon_{{1}}\), \(\varepsilon_{{2}}\) and \(\varepsilon_{{3}}\), respectively. Figure 14 presents the variations in the coaxiality parameter A with the FC at the peak and critical states. When b = 0 and 1, A ≈ 1, which means that the strain increments and the stresses are coaxial. For a specific FC, with increasing b, A first decreases and then increases, which means that an increase in b causes the coaxiality to first weaken and then strengthen. In Fig. 14a, for b = 0, 0.6, 0.8 and 1, A hardly changes with increasing FC. For b = 0.2 and 0.4, A increases with increasing FC. In Fig. 14b, for b = 0, 0.6, 0.8 and 1, A remains roughly unchanged with increasing FC. For b = 0.2, A first increases and then decreases with increasing FC. For b = 0.4, A increases with increasing FC. These findings indicate that the FC affects the coaxiality when b = 0.2 and 0.4 and that the FC has no effect on the co-axiality when b = 0, 0.6, 0.8 and 1.

Fig. 14
figure 14

Variations in the coaxiality parameter with the FC at the a peak and b critical states

Full size image

3.2 Contributions of contact types

Because the particles in the specimen of a binary granular mixture (0% < FC < 100%) include coarse and fine particles, the contacts in the specimen can be categorized as cc, cf and ff contacts. The stress ratio can be written as the sum of three stress ratios:

$$ \frac{q}{p} = \frac{{q_{{{\text{cc}}}} }}{p} + \frac{{q_{{{\text{cf}}}} }}{p} + \frac{{q_{{{\text{ff}}}} }}{p} $$
(13)

where \(q_{{{\text{cc}}}}\), \(q_{{{\text{cf}}}}\) and \(q_{{{\text{ff}}}}\) are the deviatoric stresses of the cc, cf and ff contacts, respectively. Their values can be obtained based on Eqs. (3) and (5) by restricting the cc, cf and ff contacts, respectively. Figure 15 depicts the variations in the stress ratios of the cc, cf and ff contacts \({{q_{{{\text{sub}}}} } \mathord{\left/ {\vphantom {{q_{{{\text{sub}}}} } p}} \right. \kern-\nulldelimiterspace} p}\) (\({{q_{{{\text{sub}}}} } \mathord{\left/ {\vphantom {{q_{{{\text{sub}}}} } p}} \right. \kern-\nulldelimiterspace} p}\) represents \({{q_{{{\text{cc}}}} } \mathord{\left/ {\vphantom {{q_{{{\text{cc}}}} } p}} \right. \kern-\nulldelimiterspace} p}\), \({{q_{{{\text{cf}}}} } \mathord{\left/ {\vphantom {{q_{{{\text{cf}}}} } p}} \right. \kern-\nulldelimiterspace} p}\) and \({{q_{{{\text{ff}}}} } \mathord{\left/ {\vphantom {{q_{{{\text{ff}}}} } p}} \right. \kern-\nulldelimiterspace} p}\)) versus the FC. Clearly, the b value influences \({{q_{{{\text{sub}}}} } \mathord{\left/ {\vphantom {{q_{{{\text{sub}}}} } p}} \right. \kern-\nulldelimiterspace} p}\). For a given FC, the three stress ratios generally decrease as the b value increases, which leads to the fact that the stress ratio \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\) generally decreases as the b value increases as shown in Fig. 5. The variations in the percentage contributions of the cc, cf and ff contacts to the stress ratio \(PC_{{{\text{sub}}}}\) (\(PC_{{{\text{sub}}}}\) represents \(PC_{{{\text{cc}}}}\), \(PC_{{{\text{cf}}}}\) and \(PC_{{{\text{ff}}}}\)) with the FC are depicted in Fig. 16. According to Lopez et al. [27], considering the percentage contributions of the cc, cf and ff contacts, the binary granular mixtures can be classified as several types by three thresholds of the FC (i.e., \({\text{FC}}_{1}\), \({\text{FC}}_{2}\) and \({\text{FC}}_{3}\), as shown in Fig. 16). Specifically, as FC < \({\text{FC}}_{1}\) (\(PC_{{{\text{cc}}}} > PC_{{{\text{cf}}}} > PC_{{{\text{ff}}}}\)), the binary granular mixtures are underfilled. As \({\text{FC}}_{1}\) < FC < \({\text{FC}}_{2}\) (\(PC_{{{\text{cf}}}} > PC_{{{\text{cc}}}} > PC_{{{\text{ff}}}}\)), the binary granular mixtures are interactive-underfilled. As \({\text{FC}}_{2} < {\text{FC}} < {\text{FC}}_{3}\) (\(PC_{{{\text{cf}}}} > PC_{{{\text{ff}}}} > PC_{{{\text{cc}}}}\)), the binary granular mixtures are interactive-overfilled. As FC > \({\text{FC}}_{3}\) (\(PC_{{{\text{ff}}}}\) > \(PC_{{{\text{cf}}}}\) > \(PC_{{{\text{cc}}}}\)), the binary granular mixtures are overfilled. Interestingly, at both the peak and critical states, the b value has an insignificant influence on the three thresholds of the FC. \({\text{FC}}_{1}\) ≈ 30%, \({\text{FC}}_{2}\) ≈ 44% and \({\text{FC}}_{3}\) ≈ 60% at the peak state, while \({\text{FC}}_{1}\) ≈ 30%, \({\text{FC}}_{2}\) ≈ 51% and \({\text{FC}}_{3}\) ≈ 68% at the critical state. This finding indicates that the b value generally has no influence on the classification of binary granular mixtures.

Fig. 15
figure 15

Variations in the stress ratios of the cc, cf and ff contacts versus the FC at the a peak and b critical states

Full size image
Fig. 16
figure 16

Variations in the percentage contributions of the cc, cf and ff contacts to the stress ratio with the FC at the a peak and b critical states

Full size image

The contacts in a specimen can also be categorized as strong and weak contacts. The contact forces of strong contacts are greater than or equals the mean contact force, while the contact forces of weak contacts are less than the mean contact force [55]. The stress ratio can be written as the sum of two stress ratios:

$$ \frac{q}{p} = \frac{{q_{{\text{s}}} }}{p} + \frac{{q_{{\text{w}}} }}{p} $$
(14)

where \(q_{{\text{s}}}\) and \(q_{{\text{w}}}\) are the deviatoric stresses of the strong and weak contacts, respectively. Their values can be calculated based on Eqs. (3) and (5) by restricting strong and weak contacts. Figure 17 depicts the variations in the stress ratios of strong and weak contacts \({{q_{{{\text{sub}}}} } \mathord{\left/ {\vphantom {{q_{{{\text{sub}}}} } p}} \right. \kern-\nulldelimiterspace} p}\) (\({{q_{{{\text{sub}}}} } \mathord{\left/ {\vphantom {{q_{{{\text{sub}}}} } p}} \right. \kern-\nulldelimiterspace} p}\) represents \({{q_{{\text{s}}} } \mathord{\left/ {\vphantom {{q_{{\text{s}}} } p}} \right. \kern-\nulldelimiterspace} p}\) and \({{q_{{\text{w}}} } \mathord{\left/ {\vphantom {{q_{{\text{w}}} } p}} \right. \kern-\nulldelimiterspace} p}\)) with the FC. Evidently, the b value influences \({{q_{{{\text{sub}}}} } \mathord{\left/ {\vphantom {{q_{{{\text{sub}}}} } p}} \right. \kern-\nulldelimiterspace} p}\). In Fig. 17a, as regards strong contacts, for a specific FC, as the b value increases, \({{q_{{\text{s}}} } \mathord{\left/ {\vphantom {{q_{{\text{s}}} } p}} \right. \kern-\nulldelimiterspace} p}\) decreases. As regards weak contacts, when FC ≤ 10%, for a specific FC, as the b value increases, \({{q_{{\text{w}}} } \mathord{\left/ {\vphantom {{q_{{\text{w}}} } p}} \right. \kern-\nulldelimiterspace} p}\) roughly decreases. When FC ≥ 20%, for a given FC, \({{q_{{\text{w}}} } \mathord{\left/ {\vphantom {{q_{{\text{w}}} } p}} \right. \kern-\nulldelimiterspace} p}\) generally increases with increasing b. Figure 17a indicates why \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\) varies with the b value in Fig. 5a. When FC ≤ 10%, with increasing b, decreases in \({{q_{{\text{s}}} } \mathord{\left/ {\vphantom {{q_{{\text{s}}} } p}} \right. \kern-\nulldelimiterspace} p}\) and \({{q_{{\text{w}}} } \mathord{\left/ {\vphantom {{q_{{\text{w}}} } p}} \right. \kern-\nulldelimiterspace} p}\) result in a decrease in \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\). When FC ≥ 20%, with increasing b, a decrease in \({{q_{{\text{s}}} } \mathord{\left/ {\vphantom {{q_{{\text{s}}} } p}} \right. \kern-\nulldelimiterspace} p}\) exceeds an increase in \({{q_{{\text{w}}} } \mathord{\left/ {\vphantom {{q_{{\text{w}}} } p}} \right. \kern-\nulldelimiterspace} p}\), resulting in a decrease in \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\). In Fig. 17b, as regards strong contacts, for a given FC, with increasing b, \({{q_{{\text{s}}} } \mathord{\left/ {\vphantom {{q_{{\text{s}}} } p}} \right. \kern-\nulldelimiterspace} p}\) roughly decreases. As regards weak contacts, \({{q_{{\text{w}}} } \mathord{\left/ {\vphantom {{q_{{\text{w}}} } p}} \right. \kern-\nulldelimiterspace} p}\) is roughly unaffected by the b value. Figure 17b manifests why \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\) varies with the b value in Fig. 5b. For a given FC, with increasing b, a rough decrease in \({{q_{{\text{s}}} } \mathord{\left/ {\vphantom {{q_{{\text{s}}} } p}} \right. \kern-\nulldelimiterspace} p}\) results in a rough decrease in \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\). Figure 18 depicts the variations in the percentage contributions of strong and weak contacts to the stress ratio \(PC_{{{\text{sub}}}}\) (\(PC_{{{\text{sub}}}}\) represents \(PC_{{\text{s}}}\) and \(PC_{{\text{w}}}\)) with the FC. Obviously, \(PC_{{\text{s}}}\) is larger than 75% and \(PC_{{\text{w}}}\) is smaller than 25%, which indicate that the strong contacts are the major contributor to the stress ratio. In addition, the b value influences \(PC_{{\text{s}}}\) and \(PC_{{\text{w}}}\). For a specific FC, \(PC_{{\text{s}}}\) decreases and \(PC_{{\text{w}}}\) increases with increasing b.

Fig. 17
figure 17

Variations in the stress ratios of strong and weak contacts with the FC at the a peak and b critical states

Full size image
Fig. 18
figure 18

Variations in the percentage contributions of strong and weak contacts to the stress ratio with the FC at the a peak and b critical states

Full size image

3.3 Microscale responses

As suggested by Minh and Cheng [56] and Shire et al. [36], the partial coordination number \(Z_{{{\text{cc}}}}\) for a specimen comprising of spherical coarse and fine particles can be calculated by:

$$ Z_{{{\text{cc}}}} = {{2C_{{{\text{cc}}}} } \mathord{\left/ {\vphantom {{2C_{{{\text{cc}}}} } {N_{{{\text{cp}}}} }}} \right. \kern-\nulldelimiterspace} {N_{{{\text{cp}}}} }} $$
(15)

where \(C_{{{\text{cc}}}}\) is the number of cc contacts and \(N_{{{\text{cp}}}}\) is the number of coarse particles. As indicated in the literature [57,58,59], the coordination number has two definitions for multisphere particles. One is the average number of particles in contact with a particle, and the other is the number of contacts between subspheres constituting various multisphere particles divided by the number of multisphere particles. Because every irregular coarse particle consists of overlapping subspheres, the partial coordination number of binary granular mixtures in this study also has two definitions similar to those of the coordination number, as follows:

$$ Z_{{{\text{cc}}}}^{{{\text{pp}}}} = {{2C_{{{\text{cc}}}}^{{{\text{pp}}}} } \mathord{\left/ {\vphantom {{2C_{{{\text{cc}}}}^{{{\text{pp}}}} } {N_{{{\text{cp}}}} }}} \right. \kern-\nulldelimiterspace} {N_{{{\text{cp}}}} }} $$
(16)
$$ Z_{{{\text{cc}}}}^{{{\text{ss}}}} = {{2C_{{{\text{cc}}}}^{{{\text{ss}}}} } \mathord{\left/ {\vphantom {{2C_{{{\text{cc}}}}^{{{\text{ss}}}} } {N_{{{\text{cp}}}} }}} \right. \kern-\nulldelimiterspace} {N_{{{\text{cp}}}} }} $$
(17)

where \(Z_{{{\text{cc}}}}^{{{\text{pp}}}}\) is the partial coordination number based on the “particle–particle” approach; \(C_{{{\text{cc}}}}^{{{\text{pp}}}}\) is the number of contacts between various multisphere coarse particles (not between subspheres); \(Z_{{{\text{cc}}}}^{{{\text{ss}}}}\) is the partial coordination number based on the “subsphere-subsphere” approach; and \(C_{{{\text{cc}}}}^{{{\text{ss}}}}\) is the number of contacts between subspheres constituting various multisphere coarse particles.

Figure 19 depicts the variations in the partial coordination number based on the “particle–particle” approach \(Z_{{{\text{cc}}}}^{{{\text{pp}}}}\) versus the FC. \(Z_{{{\text{cc}}}}^{{{\text{pp}}}}\) gradually decreases with increasing FC at the peak and critical states because the coarse particles are gradually separated by the fine particles. However, it was observed that the b value has an insignificant effect on \(Z_{{{\text{cc}}}}^{{{\text{pp}}}}\). As indicated in Fig. 16, when binary granular mixtures change from an underfilled structure to an interactive-underfilled structure at the peak and critical states, FC1 ≈ 30%. Figure 20 depicts the probability distributions of the number of coarse particles in contact with a coarse particle \(N^{{{\text{cc}}}}\) for FC = 30%. Obviously, these probability distributions show unimodal characteristics. At the peak state, the probabilities of \(N^{{{\text{cc}}}} { = }3\) and \(N^{{{\text{cc}}}} { = }4\) are the largest, around 25%, followed by that of \(N^{{{\text{cc}}}} { = }5\), around 20%. At the critical state, the probabilities of \(N^{{{\text{cc}}}} { = }2\) and \(N^{{{\text{cc}}}} { = }3\) are the largest, around 25%, followed by those of \(N^{{{\text{cc}}}} { = }1\) and \(N^{{{\text{cc}}}} { = }4\).

Fig. 19
figure 19

Variations in the partial coordination number based on the “particle–particle” approach versus the FC at the a peak and b critical states

Full size image
Fig. 20
figure 20

Probability distributions of the number of coarse particles in contact with a coarse particle for FC = 30% at the a peak and b critical states

Full size image

Figure 21 depicts the variations in the partial coordination number based on the “subsphere–subsphere” approach \(Z_{{{\text{cc}}}}^{{{\text{ss}}}}\) versus the FC. Interestingly, the b value influences \(Z_{{{\text{cc}}}}^{{{\text{ss}}}}\). Specifically, at the peak state, for a given FC, an increase in the b value leads to an increase in \(Z_{{{\text{cc}}}}^{{{\text{ss}}}}\) when FC ≤ 60%, which indicates that the interlocking between coarse particles is enhanced. At the critical state, for a given FC, with increasing b, \(Z_{{{\text{cc}}}}^{{{\text{ss}}}}\) first increases and then decreases when FC ≤ 60%, which indicates that the interlocking between coarse particles is first strengthened and then weakened. At both the peak and critical states, the b value almost has no effect on \(Z_{{{\text{cc}}}}^{{{\text{ss}}}}\) when FC > 60%, which can be ascribed to the truth that the coarse particles are suspended in the fine particle matrix when FC > 60%. Figure 22 depicts the relationship between the relative peak dilatancy angle \({{\psi_{{\text{p}}} } \mathord{\left/ {\vphantom {{\psi_{{\text{p}}} } {\psi_{{{\text{p100}}}} }}} \right. \kern-\nulldelimiterspace} {\psi_{{{\text{p100}}}} }}\) and the difference between the partial coordination numbers based on the “subsphere–subsphere” approach at the peak and critical states \(\left( {Z_{{{\text{cc}}}}^{{{\text{ss}}}} } \right)_{{\text{p}}} - \left( {Z_{{{\text{cc}}}}^{{{\text{ss}}}} } \right)_{{\text{c}}}\), where \(\psi_{{{\text{p100}}}}\) is the peak dilatancy angle of the binary granular mixture with FC = 100%, having the same b value with \(\psi_{{\text{p}}}\). Clearly, the data can be fitted by \({{\psi_{{\text{p}}} } \mathord{\left/ {\vphantom {{\psi_{{\text{p}}} } {\psi_{{{\text{p100}}}} }}} \right. \kern-\nulldelimiterspace} {\psi_{{{\text{p100}}}} }}{ = 0}{\text{.0395}}\left[ {\left( {Z_{{{\text{cc}}}}^{{{\text{ss}}}} } \right)_{{\text{p}}} - \left( {Z_{{{\text{cc}}}}^{{{\text{ss}}}} } \right)_{{\text{c}}} } \right]{ + }1.007\). This finding means that the peak dilatancy angle is related to the partial coordination number based on the “subsphere–subsphere” approach for a binary granular mixture.

Fig. 21
figure 21

Variations in the partial coordination number based on the “subsphere–subsphere” approach versus the FC at the a peak and b critical states

Full size image
Fig. 22
figure 22

Relationship between the relative peak dilatancy angle and the difference between the partial coordination numbers based on the “subsphere–subsphere” approach at the peak and critical states

Full size image

In three dimensions, the rotation of each particle is a vector which has three components about the x, y and z axes. In this study, the magnitude of the vector, called a rotation angle, was used. Figure 23 shows the variations in the average rotation angles of coarse and fine particles (i.e., \(w_{{\text{c}}}\) and \(w_{{\text{f}}}\)) with the b value at the axial strain of 40%. Obviously, for a binary granular mixture with a specific FC (0% < FC < 100%) and b value, \(w_{{\text{f}}}\) is larger than \(w_{{\text{c}}}\). The result means that spherical fine particles play a major role in harmonizing the deformation of a specimen, while irregular coarse particles play a minor role. Furthermore, for a given b value, with increasing FC (FC < 100%), \(w_{{\text{c}}}\) and \(w_{{\text{f}}}\) generally decrease.

Fig. 23
figure 23

Variations in the average rotation angles of a coarse and b fine particles with the b value at the axial strain of 40%

Full size image

3.4 Anisotropies of contacts and contact forces

Oda [60] and Satake [61] suggested a quantity to measure the fabric anisotropy, which is expressed as:

$$ \phi_{{{\text{ij}}}} = \int_{\Omega } {E\left( {\mathbf{n}} \right)} \;n_{i} n_{j} {\text{d}}{\mathbf{n}} = \frac{1}{C}\sum {n_{i} n_{j} } $$
(18)

where \({\mathbf{n}}\) denotes a unit vector along a direction; \(n_{i}\) and \(n_{j}\) are the ith component and the jth component of \({\mathbf{n}}\), respectively; \(\Omega\) represents all possible contact directions; \(E\left( {\mathbf{n}} \right)\) denotes the probability density of the directions of the contacts; \(C\) is the number of contacts. \(E\left( {\mathbf{n}} \right)\) is written as [62]:

$$ E\left( {\mathbf{n}} \right) = \frac{1}{{4{\uppi }}}\left( {1 + a_{ij}^{c} n_{i} n_{j} } \right) $$
(19)

where \(a_{ij}^{c}\) is the tensor to describe the directional distribution of contacts and is deduced as [63]:

$$ a_{ij}^{c} = \frac{15}{2}\phi_{ij}^{\prime } $$
(20)

where \(\phi_{ij}^{\prime }\) denotes the deviatoric tensor of \(\phi_{ij}\). Contact forces can be divided into the normal contact forces and the tangential contact forces, whose anisotropies can be quantified as [41]:

$$ F_{ij}^{n} = \frac{1}{{4{\uppi }}}\int_{\Omega } {\overline{f}}^{n} \left( {\mathbf{n}} \right)n_{i} n_{j} {\text{d}}{\mathbf{n}} = \sum {\frac{{f_{n} n_{i} n_{j} }}{{C\left( {1 + a_{kl}^{c} n_{k} n_{l} } \right)}}} $$
(21)
$$ F_{ij}^{t} = \frac{1}{{4{\uppi }}}\int_{\Omega } {\overline{f}}^{{\text{t}}} \left( {\mathbf{n}} \right)t_{i} n_{j} {\text{d}}{\mathbf{n}} = \sum {\frac{{f_{t} t_{i} n_{j} }}{{C\left( {1 + a_{kl}^{c} n_{k} n_{l} } \right)}}} $$
(22)
$$ \overline{f}^{n} \left( {\mathbf{n}} \right) = \overline{f}_{0} \left( {1 + a_{ij}^{n} n_{i} n_{j} } \right) $$
(23)
$$ \overline{f}_{i}^{t} \left( {\mathbf{n}} \right) = \overline{f}_{0} \left( {a_{ij}^{t} n_{j} - \left( {a_{kl}^{t} n_{k} n_{l} } \right)n_{i} } \right) $$
(24)

where \(a_{ij}^{n}\) and \(a_{ij}^{t}\) are the tensors to describe the distributions of the directions of normal and tangential contact forces, respectively. They are determined by:

$$ a_{ij}^{n} = \frac{{15F_{ij}^{{n}^{\prime }} }}{{2\overline{f}_{0} }}\quad a_{ij}^{t} = \frac{{15F_{ij}^{{t}^{\prime }} }}{{3\overline{f}_{0} }}\quad \overline{f}_{0} = F_{ii}^{n} $$
(25)

where \(F_{ij}^{{n}^{\prime }}\) and \(F_{ij}^{{t}^{\prime }}\) are the deviatoric parts of \(F_{ij}^{n}\) and \(F_{ij}^{t}\), respectively. The scalar \(a_{*}\) is used to quantify the degrees of the anisotropies of contacts and contact forces:

$$ a_{*} = {\text{sign}}\left( {S_{r} } \right)\sqrt {\frac{3}{2}a*_{ij} a*_{ij} } $$
(26)

where a* represents the anisotropy of contacts \(a_{{\text{c}}}\), the anisotropy of normal contact forces \(a_{n}\) and the anisotropy of tangential contact forces \(a_{{\text{t}}}\); \(S_{{\text{r}}}\) represents a normalized scalar of the product of \(a_{ij}^{*}\) and \(\sigma_{ij}^{\prime }\) [41].

During the true triaxial compression test, the contacts, contact forces and branch vectors in a specimen are anisotropic. Note that the anisotropies of contacts, contact forces and branch vectors can be used to describe the microscale mechanisms of the stress ratio \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\) when the specimen is subjected to axisymmetric loading (i.e., b = 0); that is, the stress ratio approaches to the sum of the anisotropies of contacts, contact forces and branch vectors [41]. According to the previous studies [18, 64] investigating the shear behaviors of specimens composed of spherical particles, when b ≠ 0, the stress–force–fabric relationship is not accurate enough. Nevertheless, to some extent, the anisotropies can help us to further understand the microscale mechanisms of the stress ratio changing with the b value for binary granular mixtures with various FCs. In addition, compared with the anisotropies of contacts and contact forces, the anisotropy of branch vectors is very small. Thus, this study mainly focused on the anisotropies of the contacts and contact forces.

Figures 24a–c illustrate the anisotropies at the peak state. In Fig. 24a, when FC ≤ 10%, \(a_{{\text{c}}}\) generally decreases with increasing b. However, when FC ≥ 40%, \(a_{{\text{c}}}\) generally increases with increasing b, consistent with the result obtained by Zhou et al. [18], who investigated the effect of the b value on the shear behaviors of spheres with a narrow gradation. In Fig. 24b, \(a_{n}\) basically decreases with increasing b. In Fig. 24c, with increasing b, \(a_{{\text{t}}}\) generally decreases. To some extent, a combination of Fig. 24a–c manifests the microscale mechanisms of the dependence of the stress ratio \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\) on the b value at the peak state as shown in Fig. 5a. When FC ≤ 10%, with increasing b, decreases in \(a_{{\text{c}}}\), \(a_{{\text{n}}}\) and \(a_{{\text{t}}}\) result in a decrease in \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\). When 20% ≤ FC ≤ 30%, with increasing b, decreases in both \(a_{{\text{n}}}\) and \(a_{{\text{t}}}\) lead to a decrease in \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\). When FC ≥ 40%, with increasing b, the sum of decreases in both \(a_{{\text{n}}}\) and \(a_{{\text{t}}}\) exceeds an increase in \(a_{{\text{c}}}\), causing a decrease in \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\). Figure 24d–f illustrate the anisotropies at the critical state. In Fig. 24d, when FC ≤ 20%, \(a_{{\text{c}}}\) generally decreases with increasing b. However, when FC ≥ 30%, \(a_{{\text{c}}}\) is generally independent of the b value. In Fig. 24e, \(a_{{\text{n}}}\) basically decreases with increasing b. In Fig. 24f, \(a_{{\text{t}}}\) basically decreases with increasing b. To some extent, a combination of Fig. 24d–f makes us seize the microscale mechanisms of the dependence of the stress ratio \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\) on the b value at the critical state as shown in Fig. 5b. When FC ≤ 20%, with increasing b, a decrease in \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\) can be attributed to the decreases in \(a_{{\text{c}}}\), \(a_{{\text{n}}}\) and \(a_{{\text{t}}}\). When FC ≥ 30%, with increasing b, decreases in both \(a_{{\text{n}}}\) and \(a_{{\text{t}}}\) result in a decrease in \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\).

Fig. 24
figure 24

Anisotropies at the (a–c) peak and (d–f) critical states

Full size image

4 Conclusions

The effect of the b value on the shear responses of binary granular mixtures with various FCs was investigated via the DEM numerical simulations of true triaxial compression tests. The binary granular mixtures were composed of coarse particles with real gravel shapes and fine particles with sphere shapes, and the ratio of the coarse particle size to the fine particle size is 4.45. The FC ranges from 0 to 100% in intervals of 10%. The main findings are summarized as follows.

For a specific FC, as the b value increases, both \(\phi_{{\text{p}}}\) and \(\phi_{{\text{c}}}\) first increase to a crest and then decrease. For a specific FC, as the b value increases, \(\psi_{{\text{p}}}\) roughly linearly increases. It was found that the shapes of coarse particles play an important role in the peak and critical friction angles and the peak dilatancy angles of binary granular mixtures.

For binary granular mixtures with different FCs and an identical b value, a linear relationship between the peak friction angle (or the peak dilatancy angle) and the change in the void ratio was found. Additionally, an acceptable equation was proposed to predict the peak dilatancy angle of a binary granular mixture. The FC affects the coaxiality when b = 0.2 and 0.4 and the FC has no effect on the coaxiality when b = 0, 0.6, 0.8 and 1. Specifically, at the peak state, with increasing FC, for b = 0.2 and 0.4, the coaxiality parameter A increases. At the critical state, with increasing FC, for b = 0.2, A first increases and then decreases; for b = 0.4, A increases.

The b value has an insignificant influence on \(Z_{{{\text{cc}}}}^{{{\text{pp}}}}\). At the peak state, an increase in the b value leads to an increase in \(Z_{{{\text{cc}}}}^{{{\text{ss}}}}\) when FC ≤ 60%, indicating that the interlocking between coarse particles is enhanced. At the critical state, \(Z_{{{\text{cc}}}}^{{{\text{ss}}}}\) first increases and then decreases with increasing b when FC ≤ 60%, indicating that the interlocking between coarse particles is first strengthened and then weakened. Interestingly, there is a linear relationship between the relative peak dilatancy angle and the difference between the partial coordination numbers based on the “subsphere–subsphere” approach at the peak and critical states.

It was found that the b value generally has no influence on the classification of binary granular mixtures at both the peak and critical states by examining the percentage contributions of cc, cf and ff contacts. The analyses of \({{q_{{{\text{cc}}}} } \mathord{\left/ {\vphantom {{q_{{{\text{cc}}}} } p}} \right. \kern-\nulldelimiterspace} p}\), \({{q_{{{\text{cf}}}} } \mathord{\left/ {\vphantom {{q_{{{\text{cf}}}} } p}} \right. \kern-\nulldelimiterspace} p}\), \({{q_{{{\text{ff}}}} } \mathord{\left/ {\vphantom {{q_{{{\text{ff}}}} } p}} \right. \kern-\nulldelimiterspace} p}\), \({{q_{{\text{s}}} } \mathord{\left/ {\vphantom {{q_{{\text{s}}} } p}} \right. \kern-\nulldelimiterspace} p}\) and \({{q_{{\text{w}}} } \mathord{\left/ {\vphantom {{q_{{\text{w}}} } p}} \right. \kern-\nulldelimiterspace} p}\) indicate why \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\) varies with the b value. To some extent, the examination of anisotropies helps us to further understand the microscale mechanisms that underly the dependence of \({q \mathord{\left/ {\vphantom {q p}} \right. \kern-\nulldelimiterspace} p}\) on the b value.