1 Introduction

Since wireless sensor networks and low power devices have experienced remarkable growth in recent years, which raises the possibility of harvesting energy of the environment to replace the chemical batteries that raise maintenance, environment and size issues currently (Ling et al. 2013; Karami and Inman 2012; Roseveare and Natarajan 2013). Therefore, research has recently been focused on harvesting mechanical energy, especially on converting vibration energy into electric energy, and three mechanisms have been proposed: piezoelectric, electromagnetic and electrostatic (Harne and Wang 2013; Mitcheson et al. 2007; Li et al. 2014; Crovetto et al. 2014). Compared with piezoelectric and electromagnetic energy harvesters, the electrostatic systems have advantages of both compatibility with MEMS processes and small size. Using variable capacitors, induced charges from an external voltage bias (Paracha et al. 2009) or pre-charged (Sakane et al. 2008; Sterken et al. 2007; Wen Lo et al. 2008; Naruse et al. 2009; Crovetto et al. 2013; Wang and Hansen 2013) electrets can move back and forth through an external load and power is generated when proof mass structure resonates according to the vibration source.

Among, electret-based vibration energy harvesters (E-VEHs) can be divided into two types, in-plane (Miki et al. 2010; Renaud et al. 2013; Crovetto et al. 2014) and out-of-plane (Boisseau et al. 2011; Chiu and Lee 2012; Asanuma et al. 2013; Chiu and Lee 2012; Asanuma et al. 2015), depending on the vibration direction. In-plane E-VEHs operate under vibration parallel to the electrets surface, whereas out-of-plane E-VEHs do so under vibration normal to the electrets surface. Compared with out-of-plane E-VEHs, in-plane E-VEHs can achieve high air capacitance vibration by lowering the air gap without pull-in, in which the in-plane E-VEHs can output more power. However, the patterning processing for electrets lead to higher production costs. Out-of-plane E-VEHs have lower production costs because they lack this patterning process and are of a simple device structure. Driven by the merit of low production costs, more and more researchers focus on out-of-plane E-VEHs and attempt to improve their performances.

Because of the coupling equations of out-of-plane E-VEHs cannot be solved analytically, therefore some previous works had focused on improving the performances of out-of-plane E-VEHs by numerical optimization techniques. Biosseau et al. (2011) employed a silicon-based oscillator with a cantilever structure and Teflon FEP-based electrets numerically optimized the resistive load, the electrets length parallel to the beam, and the initial air gap between the oscillator and the electrets face. Chiu and Lee (2012) employed a flexible printed circuit and Si3N4/Si3N4SiO2.SiO2 bilayer electrets, numerically optimized the load resistance and the initial air gap. However, these previous efforts focused solely on enhancing the output power and did not closely investigate changes in the frequency bandwidths within the numerical optimization. Asanuma and Hara (2015) employed CYTOP electrets to optimize the initial air gap to maximize the output power and to widen the frequency bandwidth. But they neglected another important parameter that the load resistance not only changes the output power but also can adjust resonance frequency and frequency bandwidth of out-of-plane E-VEHs.

In this study, we investigate the dependence of output power, resonance frequency, and half power bandwidth on load resistance in out-of-plane E-VEHs, with the external acceleration and electrets surface potential held constant. First, the mechanical–electrical coupling model of out-of-plane E-VEHs is built. Second, the frequency response function of output power versus acceleration excitation is obtained by Fourier transform. At last, we numerically investigate the performances of the harvester, and then experimentally evaluate these optimal designs and compare the real device performances with the numerically predictions.

2 Out-of-plane E-VEHs with double-clamped beam

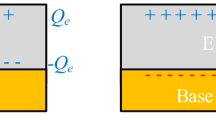
An electrostatic harvester unit able to turn vibrations into electricity using electrets can be found in Fig. 1, it is composed of a counter-electrode and an electrode on which is deposited electrets, spaced by air gap and connected by electrical load (here a load resistance). The electrets have constant surface charges Q, due to electrical induction and charges conservation, the sum of charge on the electrode Q b and on the counter-electrode Q t equals the charges Q on electrets:

Fig. 1
figure 1

Electrostatic converter using electrets

Full size image
$$Q = Q_{b} + Q_{t}$$
(1)

When a vibration occurs, it induces a reorganization of charges between the electrode and the counter-electrode through the load resistance. This induces a current i across the load resistance and part of the mechanical energy is then turned into electricity.

The equivalent electrostatic model of the electrostatic converter is presented in Fig. 2. Where, V s is the surface voltage of electrets and C(t) is the capacitance between the counter-electrode and the electrode. C(t) corresponds to the serial capacitance formed by the constant capacitance C 2(C 2 = ɛ 0 ɛ e A/d) of the electrets dielectric material and the variable capacitance C 1(t)(C 1(t) = ɛ 0 A/(g 0 − x(t))) of the air gap. Kirchhoff’s law gives the differential equation that governs the electrostatic system:

Fig. 2
figure 2

Equivalent electric model of the electrostatic converter

Full size image
$$\frac{{Q_{b} \left( t \right)}}{{C_{2} }} - \frac{{Q_{t} \left( t \right)}}{{C_{1} \left( t \right)}} = U = R\frac{{dQ_{t} \left( t \right)}}{dt}$$
(2)

According to Eq. (1), Eq. (2) can be simplified to the following form:

$$\frac{{V_{s} }}{R} - \frac{{Q_{t} \left( t \right)}}{RC\left( t \right)} = \frac{{dQ_{t} \left( t \right)}}{dt}$$
(3)

Where, \(C\left( t \right) = \frac{{\varepsilon_{0} A}}{{g_{0} - x\left( t \right) + {d \mathord{\left/ {\vphantom {d {\varepsilon_{e} }}} \right. \kern-0pt} {\varepsilon_{e} }}}}\), ɛ e is the relative permittivity of the electrets and ɛ 0 is the absolute permeability of air. And A represents the surface area of the electrets, g 0 represents the initial air gap between the electret surface and the counter-electrode, d represents the thickness of the electrets and x(t) represents the transient displacement of the mass. Surface potential V s (V s  = Q/C 2) could be considered as a monotonous linear function of charges Q.

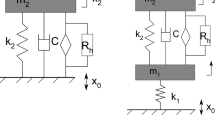
E-VEHs is a typical type of vibration energy harvester can be modeled as a mobile mass suspended to a support by a spring and damped by forces. When a vibration occurs, it induces a relative displacement of the mobile mass compared to the frame showed in Fig. 3a.

Fig. 3
figure 3

Clamped-clamped E-VEHs. a Schematic diagram, b prototype

Full size image

In this paper, a small E-VEHs prototype was fabricated in order to verify our predictions. We employed a 300 μm thick stainless steel film to fabricate a clamped–clamped beam oscillator. A 1.5 cm × 1.5 cm × 1 cm ceramic mass was added at the middle of the beam to increase the output power. A PP polymer electrets film (China. Shanghai Electret Materials Technology Co. LTD) with −500 V surface potential was prepared using the point-to-grid corona-discharge method. The charges are implanted onto a 30 μm thick PP polymer film which was spin-coated on a rigid copper plate with the help of a copper double-tape. The copper foil was uniformly adhered to the surface of the ceramic body as a counter-electrode. As shown in Fig. 3b, the harvester was assembled and set on the shaker. A high speed photographer was used to measure the peak-to-peak amplitude of the centre of the oscillator.

3 Analytical model of out-of-plane E-VEHs

To analyze the main performances of out-of-plane E-VEHs and to identify the load resistance’s effect, it is necessary to find coupled mechanical and electrostatic equations that rule the out-of-plane E-VEHs. The double-clamped beam with a mass at its middle can be modeled as a damped mass-spring structure. The mechanical friction force means the material energy dissipation of the beam can be modeled as viscous force and the electrostatic force is the derivative of the electrostatic energy of the capacitor W e with respect to the displacement x(t). W e is equal to the charge on the upper electrode Q t squared, divided by twice the capacitance as a function of time C(t). Therefore, the mechanical system is ruled by:

$$m\mathop x\limits^{ \cdot \cdot } \left( t \right) + b\mathop x\limits^{ \cdot } \left( t \right) + kx\left( t \right) - \frac{d}{dx}\left( {\frac{1}{2}\frac{{Q_{t}^{2} }}{C\left( t \right)}} \right) - mg = ma\left( t \right)$$
(3)
$$\frac{{dQ_{t} \left( t \right)}}{dt} = \frac{{V_{s} }}{R} - \frac{{Q_{t} \left( t \right)}}{RC\left( t \right)}$$
(4)

Where, V s is the surface voltage of the electrets and C(t) is the capacitance between the counter-electrode and the electrode. Capacitance C(t)corresponds to the serial capacitance formed by the constant capacitance C 2 of the electrets dielectric material and the variable capacitance C 1(t) of the air gap. Nevertheless, it is not possible to get an analytical expression for x(t) and Q t (t). Coincidentally, the V-EVHs output voltage is positively related to the amplitude of the mass, a linear model can be derived to describe the characteristics of the performances qualitatively when the amplitude of the mass is small. The charge Q t (t) on movable electrode can be written as Q t (t) = Q 10 + ΔQ t (t), where \(Q_{10} = {Q \mathord{\left/ {\vphantom {Q {\left( {1 + \frac{{\varepsilon_{e} g_{0} }}{d}} \right)}}} \right. \kern-0pt} {\left( {1 + \frac{{\varepsilon_{e} g_{0} }}{d}} \right)}}\) is the bias charge only related to the size of the structure. The charge Q b (t) on fixed electrode can be written as Q b (t) = Q 20 − ΔQ t (t), where \(Q_{20} = \frac{Q}{{1 + \frac{d}{{\varepsilon_{e} g_{0} }}}}\) is the bias charge also only related to the size of the structure ΔQ t (t) is the time-varying component of Q t (t) and Q b (t) caused by the vibration, according to Eq. (2), a governing equation of ΔQ t (t) can be derived as follow:

$$\frac{{Q_{20} + \Delta Q\left( t \right)}}{{C_{2} }} - \frac{{Q_{10} - \Delta Q\left( t \right)}}{{C_{1} \left( t \right)}} = R\frac{d\Delta Q\left( t \right)}{dt}$$
(5)

Equation (5) is developed as follow:

$$\frac{x\left( t \right)}{{g_{0} }} + \frac{\Delta Q\left( t \right)}{{Q_{10} }}\frac{x\left( t \right)}{{g_{0} }} = \frac{{\mathop {\Delta Q\left( t \right)}\limits^{ \bullet } }}{{Q_{10} }}\frac{{\varepsilon_{0} AR}}{{g_{0} }} + \frac{\Delta Q\left( t \right)}{{Q_{10} }}\frac{d}{{g_{0} \varepsilon_{e} }} + \frac{\Delta Q\left( t \right)}{{Q_{10} }}$$
(6)

Since both ΔQ(t) and x(t) are the amount of change over time and smaller than Q 10 and g 0, ΔQ(t) is a function of x(t). When x(t) is small relative to g 0, the second term on the left side of the above Eq. (6) can be ignored, a linear equation can be obtained:

$$R\frac{{d\Delta Q_{t} \left( t \right)}}{dt} + \frac{{\Delta Q_{t} \left( t \right)}}{{C_{0} }} = E_{10} x\left( t \right)$$
(7)

where, C 0 = ɛ 0 A(g 0 + d/dɛ e ) and E 10 = Q 10/ɛ 0 A are the dc components of the capacitance C(t) and the electric field in the gap, respectively.

Therefore, the mechanical system can be modified as follow:

$$m\mathop x\limits^{ \cdot \cdot } \left( t \right) + b\mathop {x\left( t \right)}\limits^{ \cdot } + kx\left( t \right) - E_{10} \Delta Q_{t} \left( t \right) = ma\left( t \right) + \frac{1}{2}Q_{10} E_{10} + mg$$
(8)

By means of Fourier transform, time-varying component of charge induced on the counter-electrode is derived from the Eq. (8):

$$\Delta \tilde{Q}_{1} \left( \omega \right) = \frac{{E_{10} C_{0} }}{{1 + j\omega RC_{0} }}\tilde{x}\left( \omega \right)$$
(9)

Where, ω is the excitation frequency. Similarly, frequency response function of amplitude versus acceleration excitation can be obtained by Fourier transform, which is expressed as:

$$\frac{{\tilde{x}\left( \omega \right)}}{{\tilde{a}\left( \omega \right)}} = \frac{m}{{ - m\omega^{2} + k - \frac{{E_{10}^{2} C_{0} }}{{\left( {1 + \left( {\omega RC_{0} } \right)^{2} } \right)}} + j\omega \left( {b + \frac{{E_{10}^{2} C_{0}^{2} R}}{{\left( {1 + \left( {\omega RC_{0} } \right)^{2} } \right)}}} \right)}}$$
(10)

Then the amplitude of mass at any frequency ω is:

$$x_{M} = \frac{{ma_{M} }}{{\sqrt {\left[ { - m\omega^{2} + k - \frac{{E_{10}^{2} C_{0} }}{{\left( {1 + \left( {\omega RC_{0} } \right)^{2} } \right)}}} \right]^{2} + \left[ {\omega \left( {b + \frac{{E_{10}^{2} C_{0}^{2} R}}{{\left( {1 + \left( {\omega RC_{0} } \right)^{2} } \right)}}} \right)} \right]^{2} } }}$$
(11)

a M is the amplitude of external acceleration. As illustrated in Eq. (11), the vibration characteristics of the out-of-plane E-VEHs are affected by the electrostatic force. In particular, the coupling effect of the electrostatic force not only changes the effective stiffness but also changes the effective damping. Thus, the effective stiffness and effective damping coefficient of the energy harvester at any excitation frequency ω are shown, respectively, as

$$k_{eff} = k - \frac{{E_{10}^{2} C_{0} }}{{\left( {1 + \left( {\omega RC_{0} } \right)^{2} } \right)}}$$
(12)
$$b_{eff} = b + \frac{{E_{10}^{2} C_{0}^{2} R}}{{\left( {1 + \left( {\omega RC_{0} } \right)^{2} } \right)}}$$
(13)

The current |I| flows through resistance load R is

$$\left| I \right| = \frac{{\omega E_{10} C_{0} }}{{\sqrt {1 + \left( {\omega RC_{0} } \right)^{2} } }}x_{M}$$
(14)

Thus, the output power of the out-of-plane E-VEHs can be calculated by

$$P_{out} = \frac{{R\omega^{2} E_{10}^{2} C_{0}^{2} }}{{2 + 2\left( {\omega RC_{0} } \right)^{2} }}x_{M}^{2}$$
(15)

Now, we solve Eqs. (11), (12), (13), and (15) by substituting parameters found in Table 1, and evaluate the mass amplitude, effective damping, effective stiffness, and frequency bandwidth of the E-VEHs. For simplicity of the calculation, this study employed the constant viscous damping obtained from the experimental value of the damping coefficient and natural frequency. We measured these values using the same mechanical vibrating system as shown in Fig. 3 but without the charging PTFE polymer by using free decay oscillation method with the help of high speed photography.

Table 1 Fixed parameters of out-of-plane E-VEHs
Full size table

Figure 4 shows theoretical analysis of performances of E-VEHs with versus load resistance when the initial air gap g 0 = 300 μm and the external acceleration were 1 ms−2. The effective damping increased from 0.325 N/(m/s) without electret with respect to the load resistance increased until reaching a maximum 0.343 N/(m/s) at 290 MΩ then decreased as Fig. 4a. The effective stiffness decreased with respect to the load resistance increased in the whole process as Fig. 4b shows. Similar with effective damping, an optimal load existed to reach the maximum output power at 270 MΩ. Despite the analytical results maybe not very accurate compared with simulation results, but it can describe the characteristics of E-VEHs qualitatively.

Fig. 4
figure 4

Performances of E-VEHs with respect to load resistance a effective damping, b effective stiffness, c output power, d mass absolute amplitude

Full size image

4 Numerical results of performances

Next, we will numerically solve the differential Eqs. (3) use Matlab/Simulink by substituting parameters found in Table 1 to evaluate the output power, resonance frequency, and frequency bandwidth of the E-VEHs.

In addition to initial air gap, load resonance is another important parameter for out-of-plane E-VEHs which have been overlooked by previous works. Output power P out and the mass absolute amplitude x M with respect to load resistance were given in Fig. 4c, d. There exists an optimal load resistance to reach maximum power, different from output power P out , there also exists a load resistance to impede the amplitude x M to reach the minimum value to obtain a maximum bandwidth. Load resistance changes harvester’s performances by charging the electrostatic force F elec between the electrets and the counter-electrode. Electrostatic force F elec is a complex periodic function under periodic external excitation so that it’s hard to describe in previous studies. But according to Eq. (8), F elec  can be divided into two parts, constant electrostatic force F static and variable electrostatic force F(t). Constant electrostatic force F static works with gravity of mass together change the initial air gap which had been described detailed in H Asanuma’s (Chiu and Lee 2012) work. Variable electrostatic force F(t) can be divided into two parts also, viscous electrostatic force F c (t), which changes E-VEHs’ damping coefficient, and elastic electrostatic force F k (t), which changes E-VEHs’ stiffness. Therefore, viscous electrostatic force F c (t) affects E-VEHs’ half power bandwidth and electrostatic force F k (t) affects E-VEHs’ resonance frequency.

Figure 5 shows the simulation performances of E-VEHs with versus load resistance when the initial air gap g 0 = 300 μm and the external acceleration were 1 ms−2. The resonance frequency decreased with load resistance decreased, but the half power bandwidth’s variation trend looks more attractive than the resonance frequency drift. There exists a load resistance to reach the maximum half power bandwidth, the half power bandwidth reaches a maximum and then decreased with further increases load resistance. The half power bandwidth Δf reaches a maximum 3.6 Hz at R = 310 MΩ, and Δf = 3.2 Hz when the output power reach maximum at R = 218 MΩ.

Fig. 5
figure 5

Numerical results of the a resonance frequency and, b half power bandwidth with respect to load resistance, c output power at resonance frequency as load resistance increases, d mass absolute amplitude at resonance frequency as load resistance increases

Full size image

Figure 5c gives output power with respect to load resistances at resonance frequency. When electret size, initial air gap, and external acceleration held constant, the output power reached a maximum of 0.044 mW at 218 MΩ and 0.0414 mW at 310 MΩ when bandwidths reach maximum. When load resistance was changed from 218 to 310 MΩ, the output power is reduced 5.9%, but half power bandwidth is increased 18.75%.

There exists difference of maximum output load resistance between analytical and simulation results as we can see in Figs. 4c and 5c. To maximize the power output delivered to the load resistance in the analytical model, the two impedances, R and 1/(ωC 0), should be matched. When g 0 = 0.3 mm, C 0 = 7.2 pF. When the excitation frequency f is 82.7 Hz, 1/(ωC 0) ≈ 270 MΩ. In the simulation model, C(t) is a quantity that changes over time as Fig. 6 shows:

Fig. 6
figure 6

C(t) change along with time

Full size image

The capacitance curve is not a standard positive or cosine curve. The average capacitance C(t) m is 8.8 pF, When the excitation frequency f = 82.7 Hz, 1/(ωC(t) m ) ≈ 218 MΩ.

5 Experiment results and discussions

Here, we will experimentally investigate the numerical predictions that (1) an optimal value for load resistance to maximize the output power, (2) the half power bandwidth exists an maximum as load resistance increases, (3) resonance frequency decreases as load resistance increases. The parameters used in experiment are the same values as used in the numerical investigation, showed in Table 1.

In order to test output characteristics of electret-based energy harvester and validate the analytical calculation results, a out-of-plane E-VEHs prototype was fabricated. First, some inherent characteristics of the damped mass-spring structure are measured by free decay oscillation method with the help of high speed photography. The damped mass-spring structure’s natural frequency is 82.6 Hz. The equivalent measuring output voltage circuit refers to Fig. 7, experimental setup shows in Fig. 8a. The output voltage is measured on the 10 MΩ internal impedance (with 3.3 pF parasitic capacitance) of the probe in series with the load resistance. The whole setup of the device is mounted on the vibrating shaker which is connected to a signal generator through a power amplifier. Lead wire from the electrode is connected across a load resistance to counter-electrode. The current generated in the circuit due to external vibration is captured by an oscilloscope. In addition, an accelerometer is used to record vibration acceleration. A load resistance box contained five 1 MΩ load resistances, five 10 MΩ load resistances, five 100 MΩ load resistances, a 5 MΩ load resistance, a 50 MΩ load resistance, and a 500 MΩ load resistance was assembled as Fig. 8b. The load resistance box allowed us obtain a variable load resistance range from 1 to 1000 MΩ.

Fig. 7
figure 7

Equipment of measuring output voltage

Full size image
Fig. 8
figure 8

a Experimental setup, b load resistance box

Full size image

Figure 9a shows the experimental output voltage amplitude of R = 10 MΩ during frequency sweep up and down when the initial air gap g 0 = 300 μm under 1 m/s 2external acceleration. It shows that the nonlinear of double-beam in our experiment is not obvious for small displacement. So we ignore the nonlinear stiffness of double-beam in the aforementioned dynamical equation is reasonable. Figure 9b shows the output voltage amplitude of R = 10 MΩ varies with acceleration amplitude when the initial air gap g 0 = 300 μm. When the acceleration amplitude is less than 0.7 m/s2, the voltage value obtained by the Eq. (7) is consistent with the simulation result and the experimental result, and the voltage amplitude is linearly related to the acceleration amplitude. When the acceleration amplitude is greater than 0.7 m/s2, the voltage amplitude obtained by Eq. (7) is smaller than that obtained by simulation and experiment, because the nonlinearity of the system becomes more and more obvious as the acceleration amplitude increases and the Eq. (7) is no longer applicable as a linear equation. Therefore, when the ratio of the amplitude of the mass to the air gap is less than 0.28, the theoretical model given in this paper is established.

Fig. 9
figure 9

a Output voltage amplitude varies with frequency experimental, b output voltage amplitude varies with acceleration amplitude analytical, numerical and experimental, c normalized mass amplitude varies with acceleration amplitude analytical, numerical and experimental

Full size image

Figure 10 shows experimental output power, half power bandwidth, resonance frequency, and mass amplitude of out-of-plane E-VEHs when the external acceleration is 1 ms−2 corresponding to the numerical predictions shown in Fig. 5. An optimal load resistance R = 60 MΩ exists to reach the maximum output power 0.028 mW during frequency up-sweep and 0.0274 mW during frequency down-sweep. The half power bandwidth reach a maximum Δf = 3.1 Hz during frequency up-sweep and a maximum Δf = 3.2 Hz during frequency down-sweep when load resistance R = 330 MΩ. But, the variations of resonance frequency are not obvious when load resistance increases from 10 to 510 MΩ, the maximum frequency drift is less than 0.5%. When the load resistance increases from 60 to 330 MΩ, the half power bandwidth increases 58.8% during frequency up-sweep and 52.9% during frequency down-sweep, output power decreases 33.2% during frequency up-sweep and 34.6% during frequency down-sweep, respectively. However, the narrow half power bandwidth is not good for practical use, since E-VEHs desperately require wider half power bandwidth, as well as higher output power. Therefore, the load resistance should be placed between 60 and 330 MΩ, while ensuring a higher output power can also get a larger bandwidth in practical applications.

Fig. 10
figure 10

Experimental results of a output power, b half power bandwidth, c resonance frequency, d mass amplitude respect to load resistance, e power respect to frequency sweep

Full size image

While similar overall trends were seen, there exist large discrepancies between the absolute values of output power for the numerical and experimental results. The difference of output power results from surface potential decay of PP electrets and a parasitic capacitance built into the experiment step especially when using high-value resistors. Because E-VEHs’ output power is positively related to the square of the surface potential. A slight potential decay of PP electrets’ surface potential will greatly reduce the output power. The maximum capacitance variation of our E-VEHs type remains only 11.9 pF. Thus, a parasitic capacitance, even with only several decades pF, will significantly decrease the relative capacitance variation and thus the output power. Despite the large differences between the absolute values of output power for the numerical and experimental results, but it but does not obstruct our main conclusions in this paper: (1) an optimum value exists in the load resistance to maximize the output power; (2) enhanced electrostatic forces with decreasing the load resistance emphasize the soft spring effect, which lowers the resonance frequency; (3) a load resistance exists to maximize the half power bandwidth; (4) the load resistance should be placed between maximum power load resistance and maximum bandwidth load resistance, while ensuring a higher output power can also get a larger bandwidth in practical applications.

6 Conclusions

In this paper, we have presented an investigation of load resistance optimization for output power and half power bandwidth in out-of-plane electret-based vibration energy harvesters. First, an analytical model of small signal is presented to describe the characteristics of out-of-plane E-VEHs. Then, the frequency response function of current versus acceleration excitation was obtained by Fourier transform. In particular, the coupling effect of the electrostatic force not only changes the system’s effective stiffness but also changes it’s effective damping. Second, We investigated the dependence of output power, resonance frequency and half power bandwidth on the load resistance for electret-based out-of-plane vibration energy harvesters, both numerically and experimentally. The numerical investigation predicted the following results: (1) an optimum value exists in the load resistance to maximize the output power; (2) enhanced electrostatic forces with decreasing the load resistance emphasize the soft spring effect, which lowers the resonance frequency; (3) a load resistance exists to maximize the half power bandwidth. The experimental results showed behaviors consistent with the numerical predictions. The output power reach a maximum 0.028 mW during frequency up-sweep and 0.0274 mW during frequency down-sweep at optimum load resistance 60 MΩ when the external acceleration were 1 ms−2 and 82.3 Hz, respectively. When increasing the load resistance to 330 MΩ, the half power bandwidth increased to 3.1 Hz during frequency up-sweep and 3.2 Hz during frequency down-sweep, increased 58.8 and 52.9% over optimum load resistance, respectively. The peak output power at load resistance 330 MΩ was 0.0187 mW during frequency up-sweep and 0.0179 mW during frequency down-sweep, when the external acceleration were 1 ms−2 and 82.3 Hz, respectively. Therefore, the load resistance should be placed between maximum power load resistance and maximum bandwidth load resistance, while ensuring a higher output power can also get a larger bandwidth in practical applications.