1 Introduction

Micro-/nanopositioning systems capable of precise parallel alignment have been widely used in many fields such as micromachining, nanoimprint lithography, optics element alignment, fiber assembly, etc (Zhang et al. 2006; Teo et al. 2014; Lin and Chen 2008). For parallel alignment, an orientation stage that can realize out-of-plane adjustment is often needed to make proper alignment between two flat surfaces.

A number of orientation stages have been developed in the literature, among which the most common one refers to the \(Z-{{\theta }_{x}}-{{\theta }_{y}}\) stage (Fung and Lin 2009; Lee et al. 2013). However, since the rotational center is often located inside the stage, the output platform of such stage suffers excessive lateral movement which is harmful and must be avoided (Qu et al. 2014). For example, in nanoimprint lithography, the excessive lateral motion can lead to un-neglected offset between the template and substrate, which may destroy the transfered images (Choi et al. 2001). To overcome this problem, an orientation stage with remote-center-of-motion (RCM) characteristic can be employed. For such a RCM stage, its rotational center locates at the tip of the end-effector which is remote from the system, so that the excessive lateral movement can be eliminated (Qu et al. 2014).

For generating a RCM stage, the simplest way is to employ the circular prismatic joint or spherical bearing, where the RCM motion is guaranteed by the rigid curve structure (Lum et al. 2006; Du et al. 2013). Such mechanisms possess simple structures, while high-precision manufacturing is needed to achieve desired motion accuracy. Another RCM guiding mechanism refers to the instant four-bar-linkage mechanism, such as the isosceles-trapezoid four-bar mechanism (Xu et al. 2008; Sarajlic et al. 2010). However, the actuation radius of such mechanism is restricted by the structural parameters, which leads to a constrained motion range especially when an actuator with short stroke is employed. In contrast, RCM stages based on parallelogram-four-bar-linkage mechanism do not have this limitation since the actuators can be installed close to the base of the stage, where the actuation radius can be decreased (Qu et al. 2014).

Besides, in order to improve the performance of the micro-/nanopositioning system, piezoelectric stack actuators (PSAs) combined with flexure-based compliant mechanisms are often employed (Polit and Dong 2011; Choi and Kim 2006). The merits of fast response, large blocking force and high resolution for PSA and the advantages of no backlash, no friction, and easy to manufacture for compliant mechanisms can provide the system with good dynamic performance and high positioning accuracy. A piezoactuated compliant RCM stage based on parallelogram-four-bar-linkage mechanism has been developed by the authors previously (Qu et al. 2014). However, due to the limited stroke of the PSA, the rotational range of the stage is confined to less than 1.5 mrad which may not be sufficient for many micropositioning occasions. Therefore, this paper is focused on the design and implementing of new parallelogram-based compliant RCM stage with large rotational range in view of its promising prospects.

In order to obtain a larger rotational range, a PSA with larger stroke can be employed. However, the larger stroke of the PSA, the bigger size and higher price it will take, which makes it undesireable in practice. As an alternative, mechanical displacement amplifiers are popular devices to amplify the stroke of PSA in order to achieve large output displacements (Yong et al. 2009). A literature review indicates that the commonly used displacement amplifiers are based on three types including lever mechanism (Yong et al. 2009; Choi et al. 2007), Scott-Russell mechanism (Ha et al. 2006; Tian et al. 2009) and bridge-type mechanism (Xu and Li 2011; Lobontiu and Garcia 2003). By comparison, the bridge-type amplifier possesses larger displacement amplification ratio but smaller output stiffness, while the Scott-Russell mechanism has a smaller displacement amplification ratio but larger output stiffness. In contrast to these two types of amplifiers, lever mechanism has the highest output stifness while its drawback lies in the relative larger dimensions. Considering that the output stiffness is the most important evaluation index of the amplifier when actuating a RCM guiding mechanism, the lever mechanism is chosen as the amplifier in this paper. Specifically in our design, an output-stiffness enhanced lever amplifier (OELA) is presented, where two identical single lever mechanisms are combined in parallel via a rigid connecting linkage. By such design, the output stiffness of the amplifier can be enlarged as twice as before without enlarging the dimensions of the lever. Besides, the outer RCM guiding mechanism is designed with a compact symmetric double parallelogram structure, where two identical parallelogram-four-bar-linkage mechanisms are employed to guide the stage to perform RCM movement with high rotational precision. Based on the proposed OELA and RCM guiding mechanism, a novel piezoactuated compliant RCM stage with large rotational range has been implemented.

In the rest of this paper, the design procedure of the compliant RCM stage is presented in Sect. 2. In Sect. 3, analytical models for the prediction of kinematics, statics, stress, and dynamics properties of the stage are established in detail. Then, in Sect. 4, the dimensional optimization is performed and the models are validated by finite element analysis (FEA). After that, a prototype of the stage is fabricated and its performance is tested in Sect. 5. Finally, Sect. 6 concludes this paper.

2 Mechanism design

2.1 Parallelogram-based RCM guiding mechanism

A RCM stage refers to the one that the rotational center of the output platform is remote from the stage itself. Compared with other RCM stages, parallelogram-based RCM stage has a flexibly adjusted movement range since the actuators can be installed close to the base of the stage.

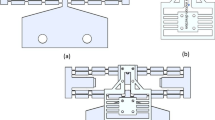
A typical parallelogram-based RCM module is shown in Fig. 1a. When an external force \(f\) is applied on the linkage \(BE\), the module moves to a new position (as indicated by the dotted lines) and the point \(E\) rotates around a virtual remote joint \(O\) due to the geometric constraints of the parallelogram \(ABCD\) and \(CDOE\). Therefore, the point \(O\) can be served as the RCM point and a simple RCM module is generated.

Based on the simple RCM module, an available RCM stage can be constructed as shown in Fig. 1b, where two identical parallelogram RCM modules arranged in symmetric configuration are employed to form the output platform. To decrease the dimensions of the stage, a symmetric double parallelogram mechanism has been proposed in the authors’ previous work (Qu et al. 2014), see Fig. 1c. Through such design, a more compact stage is generated and what’s more, the number of the actuators is cut down from two to one. The final designed stage with flexure hinge-based joints and a PSA is shown in Fig. 1d.

Fig. 1
figure 1

a Typical parallelogram-based RCM module. b Constructing a RCM stage with two parallelogram-based RCM modules. c Constructing a RCM stage with a symmetric double parallelogram mechanism. d Previous design of the compliant RCM stage

Full size image

One merit of the parallelogram-based RCM stage refers to its unconstrained actuation radius. As illustrated in Fig. 2a, the actuation radius can be defined as the distance between the rotational center and the point where the actuation force is applied. And the actuation radius can be calculated by

$$\begin{aligned} {{r}_{a}}=\frac{{{d}_{in}}}{\sin {{\theta }_{in}}}, \end{aligned}$$
(1)

where \({{d}_{in}}\) represents the input displacement and \({{\theta }_{in}}\) denotes the rotational angle of the linkage. It is known that the maximum input displacement is constrained by the stroke of the selected PSA. Therefore, if a large rotational angle were wanted, the actuation radius must be made as small as possible. For parallelogram-based RCM stage, the actuation point can be chosen close to the base so as to decrease the actuation radius, as illustrated in Fig. 2b.

Fig. 2
figure 2

Schematic diagram of a the actuation radius and b choosing the actuation point so as to decrease the actuation radius

Full size image

Nevertheless, due to the short stroke of the adopted PSA, the rotational range of the previously designed RCM stage is limited to 1.45 mrad, which is not sufficient for many applications. Therefore, this paper is concentrated on implementing a new parallelogram-based RCM stage with large rotational range. The designed procedure is detailed as follows.

2.2 Output-stiffness enhanced lever amplifier

In order to realize a large range of rotational positioning, mechanical displacement amplifiers are often employed. Compared with other amplifiers, lever amplifier has the advantages of large output stiffness and high efficiency. Figure 3a shows a conventional flexure-based lever amplifier, where the amplification ratio is given by \(\left( {{l}_{1}}+{{l}_{2}} \right)/{{l}_{1}}\). To obtain a large amplification ratio without enlarging the overall dimensions of the mechanism, a small value of \({l}_{1}\) rather than a large value of \({l}_{2}\) is desired.

However, for lever amplifier, decrease of \({l}_{1}\) is at the expense of decrease of the output stiffness, which may cut down the amplification ratio in turn. Since the lever amplifier will be cascaded between the PSA and the RCM guiding mechanism in this design, large enough output stiffness of the lever amplifier should be guaranteed to drive the RCM guiding mechanism. To overcome this problem, an output-stiffness enhanced lever amplifier (OELA) is presented in this paper, as illustrated in Fig. 3b. The OELA is composed of two identical single lever mechanisms that are combined in parallel. By such design, the output stiffness of the amplifier can be enlarged as twice as before without changing the length of the lever. Therefore, compared with single lever amplifier, the OELA has more ability to obtain a larger amplification ratio under the same external load.

Fig. 3
figure 3

a Conventional flexure-based lever amplifier. b Proposed OELA

Full size image

2.3 Compliant RCM stage based on the OELA

The schematic diagram of the designed compliant RCM stage based on the OELA is shown in Fig. 4. It can be observed that the stage owns a monolithic mechanical structure, where eight flexural hinges (\(E\) to \(I\), \({G}'\) to \({I}'\)) with identical mechanical dimensions are elaborately arranged to compose a single double parallelogram mechanism which is served as the outer RCM guiding mechanism. The OELA is embedded in the RCM guiding mechanism and it is composed of two identical lever mechanisms which are combined together in parallel through a rigid connecting linkage.

It should be noted that the configuration of the RCM guiding mechanism is a little different from the one as illustrated in Fig. 2b, where the lower base of the RCM guiding mechanism is reversely placed. The reason lies in that by such design, more internal spaces could be generated to embed the OELA.

Besides, right circular flexure hinges are adopted as the rotational joints since they possess the smallest magnitude of center-shift compared to other types (Zelenika et al. 2009). The stage is actuated by a PSA which is fixed at the base. And the lowest rigid linkage \(I{I}'\) acts as the output platform which can rotate around the RCM point of the system under the actuation of the PSA.

Fig. 4
figure 4

Proposed compliant RCM stage based on the OELA

Full size image

3 Modeling of the stage

As far as modeling methods is concerned, the pseudo-rigid-body-model (PRBM) method is preferred since it can simplify the design and modeling of compliant mechanisms greatly (Howell 2001). In this section, PRBM-based approach is employed to evaluate the performances of the proposed stage.

3.1 Kinematic modeling

According to the PRBM method, a flexure-based compliant mechanism can be converted into an analogous rigid-body mechanism by replacing each flexure hinge with a one degree-of-freedom (DOF) revolute joint attached with a torsional spring, as shown in Fig. 5. During the kinematic analysis, the drift of the rotational centers and the stiffness of the flexure hinges are not taken into consideration. Besides, due to the symmetric configuration, only half of the inner OELA and outer RCM guiding mechanism are considered.

The two kinematic models are shown in Fig. 6a, b, respectively, where \(i (i = O, A, \ldots ,{I}')\) denotes the rotational centers of flexure hinges and \(P\) represents the input platform of the RCM stage.

Fig. 5
figure 5

Parameters of the right circular flexure hinge and its equivalent PRBM

Full size image

The fundamental structure of the inner OELA and the outer RCM guiding mechanism can both be treated as a special five-bar linkage mechanism, respectively. When the input platform is driven by the PSA with a displacement \({{x}_{in}}\), the output linkage \(I{I}'\) will rotate around the RCM point with an angle \({{\theta }_{out}}\).

To find the relationship between the input displacement and the output angle of the RCM stage, the instantaneous velocities of the point P and linkage \(I{I}'\) are utilized. The instantaneous velocity centers of the corresponding linkages can be determined by intersecting the perpendicular lines of the velocity directions of two different points on the linkage, as shown in Fig. 6. Thus, the instantaneous velocities of the points A to G can be obtained, respectively, and given as follows:

$$\begin{aligned} {{v}_{A}}&={{v}_{P}}={{\omega }_{1}}\cdot {{l}_{OA}}\end{aligned}$$
(2a)
$$\begin{aligned} {{v}_{B}}&={{\omega }_{1}}\cdot {{l}_{OB}}\end{aligned}$$
(2b)
$$\begin{aligned} {{v}_{C}}&={{\omega }_{1}}\cdot {{l}_{OC}}={{\omega }_{2}}\cdot {{l}_{{{O}_{1}}C}}\end{aligned}$$
(2c)
$$\begin{aligned} {{v}_{D}}&={{\omega }_{2}}\cdot {{l}_{{{O}_{1}}D}}={{\omega }_{3}}\cdot {{l}_{ED}}\end{aligned}$$
(2d)
$$\begin{aligned} {{v}_{G}}&={{\omega }_{3}}\cdot {{l}_{EG}}, \end{aligned}$$
(2e)

where \({{\omega }_{1}}\), \({{\omega }_{2}}\) and \({{\omega }_{3}}\) are the instantaneous angular velocities of the linkage \(OC\), \(CD\), and \(EG\), respectively, and \({{l}_{OA}}\), \({{l}_{OC}}, \ldots,\,\) \({{l}_{EG}}\) denote the distances between the two corresponding points, respectively.

Fig. 6
figure 6

Velocity diagram of the a OELA and b RCM guiding mechanism

Full size image

Due to the geometric constraints of the parallelograms \(EGHF\) and \(E{G}'{H}'F\), linkages \(GI\) and \({G}'{I}'\) perform translational motions during this procedure, which indicates that the velocities of the points \(I\) and \({I}'\) equal to that of points \(G\) and \({G}'\), respectively. Therefore, the instantaneous rotational center of the output linkage \(I{I}'\) can be determined as \({{O}_{2}}\) which is illustrated in Fig. 6b, and the instantaneous angular velocity of the linkage \(I{I}'\) can be calculated as

$$\begin{aligned} {{\omega }_{4}}=\frac{{{v}_{I}}}{{{l}_{{{O}_{2}}I}}}=\frac{{{v}_{G}}}{{{l}_{EG}}}={{\omega }_{3}}, \end{aligned}$$
(3)

where \({{v}_{I}}\) denotes the instantaneous velocity of the point \(I\), and \({{l}_{{{O}_{2}}I}}\) represents the distance between the point \({O}_{2}\) and \(I\), respectively. It is noted that the point \({O}_{2}\) along with the other five points (\(I, H, F, {H}', {I}'\)) constructs two new parallelograms, namely, \(FHI{O}_{2}\) and \(F{H}'{I}'{O}_{2}\). Therefore, the instantaneous velocity center \({O}_{2}\) is served as the RCM point of the stage.

According to (2) and (3), and assume that the deformations of flexure hinges are very small and within the micrometer level, the rotational angle of the output platform can be deduced as

$$\begin{aligned} {{\theta }_{out}}=\frac{{{x}_{in}}{{l}_{OC}}{{l}_{{{O}_{1}}D}}}{{{l}_{OA}}{{l}_{{{O}_{1}}C}}{{l}_{ED}}}=\frac{{{x}_{in}}{{l}_{OC}}}{{{l}_{OA}}{{r}_{a}}}, \end{aligned}$$
(4)

where \({r}_{a}\) denotes the actuation radius as aforementioned and is illustrated in Fig. 6a. It can be seen from the Eq. (4) that there exists a linear relationship between the output angle and the input displacement. And the ratio of output-to-input is mainly determined by the length of each linkage, where the ratio increases as \({{l}_{OC}}\) increases while as \({{l}_{OA}}\) and \({r}_{a}\) decrease.

3.2 Static modeling

In this section, the static modeling of the RCM stage is performed with the PRBM approach to describe the force-deflection relationship of flexure hinges. When a small displacement \({x}_{in}\) generated by the PSA is applied on the input platform of the RCM stage, the angle increments \({{\theta }_{out}}\), \({{\theta }_{1}}\sim {{\theta }_{3}}\) for all the moving linkages can be expressed as shown in Fig. 7. Then the rotational angles \({{\psi }_{O}},{{\psi }_{A}}\sim {{\psi }_{I}}\) of the flexure hinges \(O,A\sim I\) can be derived separately as follows:

$$\begin{aligned} {{\psi }_{O}}&={{\psi }_{A}}={{\psi }_{B}}={{\theta }_{1}}=\frac{{{x}_{in}}}{{{l}_{OA}}}\end{aligned}$$
(5a)
$$\begin{aligned} {{\psi }_{C}}&={{\theta }_{1}}+{{\theta }_{2}}=\frac{{{x}_{in}}}{{{l}_{OA}}}+\frac{{{x}_{in}}{{l}_{OC}}}{{{l}_{OA}}{{l}_{CD}}\cot \alpha }\end{aligned}$$
(5b)
$$\begin{aligned} {{\psi }_{D}}&={{\theta }_{2}}+{{\theta }_{3}}=\frac{{{x}_{in}}{{l}_{OC}}}{{{l}_{OA}}{{l}_{CD}}\cot \alpha }+\frac{{{x}_{in}}{{l}_{OC}}}{{{l}_{OA}}{{r}_{a}}}\end{aligned}$$
(5c)
$$\begin{aligned} {{\psi }_{E}}&={{\psi }_{F}}={{\psi }_{G}}={{\psi }_{H}}={{\theta }_{3}}=\frac{{{x}_{in}}{{l}_{OC}}}{{{l}_{OA}}{{r}_{a}}}\end{aligned}$$
(5d)
$$\begin{aligned} {{\psi }_{I}}&={{\theta }_{out}}=\frac{{{x}_{in}}{{l}_{OC}}}{{{l}_{OA}}{{r}_{a}}}={{\theta }_{3}}, \end{aligned}$$
(5e)

where \(\alpha\) denotes the angle made by linkages \(DE\) and \(EG\), which is shown in Fig. 6a, and satisfies

$$\begin{aligned} \cot \alpha =\frac{{{r}_{a}}}{{{r}_{D}}+{{w}_{R}}+\left( {{{t}_{E}}}/{2}\; \right) }, \end{aligned}$$
(6)

where \({{r}_{D}}\) is the radius of the flexure hinge \(D\), \({{w}_{R}}\) is the width of the linkages of the RCM guiding mechanism, and \({t}_{E}\) represents the thickness of the flexure hinge \(E\).

Fig. 7
figure 7

Deformation diagram of the a OELA and b RCM guiding mechanism

Full size image

According to the PRBM approach, each right circular flexure hinge of the RCM stage can be transformed into an equivalent revolute joint with a torsional spring, and the stiffness of the torsional spring can be given as (Paros 1965)

$$\begin{aligned} {{K}_{i}}=\frac{2Ew{{t}_{i}{}^{{5}/{2}\;}}}{9\pi {{{r}_{i}}^{{1}/{2}\;}}}, \quad i=O,\, A,\ldots,I \end{aligned}$$
(7)

where \(E\) denotes the Young’s modulus of the material, \(w\), \({t}_{i}\) and \({r}_{i}\) are the height, thickness and radius of the \(i\)th right circular flexure hinge, respectively, which are elaborated in Fig. 5. Here we assume that all the flexure hinges have identical height \(w\).

Therefore, the torque \({M}_{i}\), generated at the rotational center of the \(i\)th flexure hinge, can be obtained as

$$\begin{aligned} {{M}_{i}}=-{{K}_{i}}{{\psi }_{i}}, \quad i=O,\,A,\ldots ,I \end{aligned}$$
(8)

where the negative sign indicates that the torque has the opposite direction with the rotational motion of the flexure hinges. Assuming that an input force \({F}_{in}\) is applied at the input platform, the corresponding input displacement can be noted as \({x}_{in}\). For calculating the input stiffness of the stage, the Castigliano’s first theorem is adopted which is expressed as

$$\begin{aligned} {{F}_{in}}=\frac{\partial {{U}_{s}}}{\partial {{x}_{in}}}, \end{aligned}$$
(9)

where \({U}_{s}\) denotes the deformation energy of the stage and it is given as

$$\begin{aligned} {{U}_{s}}=-\sum \limits _{i=O}^{I}{{{M}_{i}}{{\psi }_{i}}}+\sum \limits _{i=A,E,F}{\frac{1}{2}{{M}_{i}}{{\psi }_{i}}}. \end{aligned}$$
(10)

Hence, the relationship between the input force and the input displacement can be determined in view of (5), (8), (9) and (10), and the input stiffness of the RCM stage can be derived via \({{K}_{in}}={{{F}_{in}}}/{{{x}_{in}}}\;\), which is given as

$$\begin{aligned} {{K}_{in}}&=\frac{2{{K}_{O}}+{{K}_{A}}+2{{K}_{B}}}{{{l}_{OA}}^{2}}+2{{\left( \frac{{{l}_{CD}}\cot \alpha +{{l}_{OC}}}{{{l}_{OA}}{{l}_{CD}}\cot \alpha } \right) }^{2}}{{K}_{C}} \nonumber \\&+2{{\left( \frac{{{r}_{a}}{{l}_{OC}}+{{l}_{OC}}{{l}_{CD}}\cot \alpha }{{{r}_{a}}{{l}_{OA}}{{l}_{CD}}\cot \alpha } \right) }^{2}}{{K}_{D}}\nonumber \\&+\sum \limits _{i=E}^{F}{{{\left( \frac{{{l}_{OC}}}{{{r}_{a}}{{l}_{OA}}} \right) }^{2}}{{K}_{i}}}+2\sum \limits _{j=G}^{I}{{{\left( \frac{{{l}_{OC}}}{{{r}_{a}}{{l}_{OA}}} \right) }^{2}}{{K}_{j}}}. \end{aligned}$$
(11)

From Eq. (11), it can be seen that the input stiffness of the RCM stage is determined by its geometrical parameters along with the stiffness of each torsional spring. Besides, in view of Eq. (7), it is noted that the parameter of thickness has a great impact on the stiffness of the flexure hinge, which inspires us to assign \({t}_{i}\) with different values to meet different stiffness requirements. That is, for the inner OELA, the thickness of the hinges can be made as relative large values to achieve high output stiffness, while for the outer RCM guiding mechanism, the thickness values can be made as small as possible to acquire small input stiffness. In this way, the amplication efficiency of the OELA can be improved and therefore, the output displacement can be enlarged.

Considering the stress constraints, the maximum stress \({{\sigma }_{max}}\) in the flexure hinge induced by the maximum output of the PSA must be lower than the allowable stress, which can be expressed by

$$\begin{aligned} {{\sigma }_{\max }}\le \frac{{{\sigma }_{y}}}{n}, \end{aligned}$$
(12)

where \({{\sigma }_{y}}\) denotes the yield strength of the material, \(n\in \left( 1,\infty \right)\) represents the assigned safety factor. For a circular flexure hinge, the maximum stress \({{\sigma }_{max}}\) occurs at the outermost surface of the thinnest portion of the hinge, and it can be computed as (Smith et al. 1997)

$$\begin{aligned} {{\sigma }_{max}}=\frac{E{{\left( 1+\beta \right) }^{{9}/{20}\;}}}{{{\beta }^{2}}f\left( \beta \right) }{{\psi }_{max}}, \end{aligned}$$
(13)

where \(\beta =\frac{t}{2r}\) is a dimensionless geometry factor with a valid range of \(0<\beta <2.3\), \(f\left( \beta \right)\) is a dimensionless compliance factor defined by

$$\begin{aligned} f\left( \beta \right) =\frac{3+4\beta +2{{\beta }^{2}}}{\left( 1+\beta \right) {{\left( 2\beta +{{\beta }^{2}} \right) }^{2}}}+\frac{6\left( 1+\beta \right) {{\tan }^{-1}}\sqrt{{2}/{\beta }\;+1}}{{{\left( 2\beta +{{\beta }^{2}} \right) }^{{5}/{2}\;}}}, \end{aligned}$$
(14)

and \({{\psi }_{max}}\) represents the maximum rotational angle of the hinge which is made as

$$\begin{aligned} {{\psi }_{max}}=\max \left\{ {{\psi }_{O}},{{\psi }_{A}},\ldots ,{{\psi }_{I}} \right\}. \end{aligned}$$
(15)

Assuming that \({{l}_{OC}}>{{r}_{a}}\), it can be seen from Eq. (5) that the maximum rotation angle generates at the flexure hinge \(D\). Let \({{Q}_{s}}\) denotes the stroke of the adopted PSA, according to (5), (12) and (13), the following relationship can be derived:

$$\begin{aligned} \frac{{{l}_{OC}}}{{{l}_{OA}}{{l}_{CD}}\cot \alpha }+\frac{{{l}_{OC}}}{{{l}_{OA}}{{r}_{a}}}\le \frac{{{\beta }^{2}}f\left( \beta \right) {{\sigma }_{y}}}{E{{\left( 1+\beta \right) }^{{9}/{20}\;}}n{{Q}_{s}}}, \end{aligned}$$
(16)

which provides a guideline for choosing the architecture parameters of the stage in order to prevent the materials from inelastic deformation.

3.3 Dynamic modeling

From the dynamic point of view, a PSA can be approximately equivalent to an undamped mass-spring system with a lumped mass \({{m}_{p}}\) and a linear stiffness \({{K}_{p}}\). Due to the utilization of flexure hinges, the RCM stage has negligible friction and thus almost no damping. In the current research, the Lagrange’s equation is adopted to evaluate the dynamic property of the stage and the input displacement variable \({x}_{in}\) is chosen as the generalized coordinate.

Since the kinetic energy is induced by the movements of the PSA and rigid linkages, the total kinetic energy of the entire system can be expressed as

$$\begin{aligned} T=&\frac{1}{2}\left( {{m}_{in}}+{{m}_{p}} \right) {{\dot{x}}_{in}}^{2}+\frac{1}{2}{{m}_{B{B}^{\prime}}}{{v}_{B}}^{2}+{{m}_{GI}}{{v}_{G}}^{2}+{{I}_{OC}}{{\omega }_{1}}^{2}+{{I}_{CD}}{{\omega }_{2}}^{2} \nonumber \\&+\frac{1}{2}\left( {{I}_{GE{G}^{\prime}}}+{{I}_{HF{H}^{\prime}}}+{{I}_{I{I}^{\prime}}}\right) {{\omega }_{3}}^{2}, \end{aligned}$$
(17)

where \({{m}_{in}}\), \({{m}_{B{B}'}}\) and \({{m}_{GI}}\) separately represent the mass of the input platform and linkage \(B{B}'\) and \(GI\), and \({{I}_{OC}}\), \({{I}_{CD}}\), \({{I}_{GE{G}'}}\), \({{I}_{HF{H}'}}\) and \({{I}_{I{I}'}}\) denotes the moment of inertia of the linkages \(OC\) to \(I{I}'\) with respect to their corresponding instantaneous centers, respectively.

On the other hand, the potential energy is given by the elastic deformations of the PSA and flexure hinges. And the total potential energy of the entire system can be expressed as

$$\begin{aligned} U&=\frac{1}{2}{{K}_{p}}{{x}_{in}}^{2}+\sum \limits _{i=O}^{I}{{{K}_{i}}{{\psi }_{i}}^{2}}-\sum \limits _{i=A,E,F}{\frac{1}{2}{{K}_{i}}{{\psi }_{i}}^{2}} \nonumber \\&={{U}_{p}}+{{U}_{s}}, \end{aligned}$$
(18)

where \({U}_{p}\) and \({U}_{s}\) denote the potential energy of the PSA and the RCM stage, respectively.

Therefore, the dynamic equation of the mechanism can be developed using Lagrange equation as follows:

$$\begin{aligned} \frac{d}{dt}\left( \frac{\partial T}{\partial {{{\dot{x}}}_{in}}} \right) -\frac{\partial T}{\partial {{x}_{in}}}+\frac{\partial U}{\partial {{x}_{in}}}={{F}_{in}}. \end{aligned}$$
(19)

By substituting Eqs. (17), (18) into (19), considering (2) and (11), and let the input force \({{F}_{in}}\) equal to zero, the dynamic equation that describes a free motion of the stage can be obtained as

$$\begin{aligned} M{{\ddot{x}}_{in}}+K{{x}_{in}}=0, \end{aligned}$$
(20)

where \(M\) and \(K\) represent the equivalent mass and equivalent stiffness of the system, and they are separately defined by

$$\begin{aligned} M&={{m}_{p}}+{{m}_{in}}+\frac{{{m}_{B{B}^{\prime}}}{{l}_{OB}}^{2}}{{{l}_{OA}}^{2}}+\frac{2{{m}_{GI}}{{l}_{OC}}^{2}{{l}_{EG}}^{2}}{{{l}_{OA}}^{2}{{r}_{a}}^{2}}+\frac{2{{I}_{OC}}}{{{l}_{OA}}^{2}}+\frac{2{{I}_{CD}}{{l}_{OC}}^{2}{{\tan }^{2}}\alpha }{{{l}_{OA}}^{2}{{l}_{CD}}^{2}}\nonumber \\&\quad +\frac{\left( {{I}_{GE{G}^{\prime}}}+{{I}_{HF{H}^{\prime}}}+{{I}_{I{I}^{\prime}}} \right) {{l}_{OC}}^{2}}{{{l}_{OA}}^{2}{{r}_{a}}^{2}}\nonumber \\&={{m}_{p}}+{{m}_{s}} \end{aligned}$$
(21)
$$\begin{aligned} K={{K}_{p}}+{{K}_{in}}, \end{aligned}$$
(22)

where \({{m}_{s}}\) represents the equivalent mass of the stage.

After that, the natural frequency of the system can be obtained from the following equation:

$$\begin{aligned} f=\frac{1}{2\pi }\sqrt{\frac{K}{M}}, \end{aligned}$$
(23)

which has the unit Hertz.

4 Dimensional optimization and FEA validation

4.1 Optimal design

Through the above analyses, it is noted that the dimensions of the stage are critical to the performances in terms of kinematics, statics and dynamics. Therefore, it is necessary to optimize the dimensions so as to obtain an ideal structure for practical application. We choose the first resonance frequency of the stage as the objective of the optimization since it is a key factor that determines the dynamic performance of the system.

The variables to be optimized are the parameters of \({l}_{OA},{l}_{OC},{l}_{CD},{l}_{EG},{t}_{i},{t}_{o},r\) and \({r}_{a}\), where \({t}_{i}\) and \({t}_{o}\) represent the thickness of the flexure hinges for the inner OELA and outer RCM guiding mechanism, respectively. The constraints of the optimization are described as follows:

  1. 1.

    Input stiffness The input stiffness of the stage should not exceed the stiffness of the adopted PSA, i.e., \({{K}_{p}}=\text {48}\text {.9 N/} {\upmu }\text {m}\). Moreover, since large input stiffness of the stage will reduce the output displacement of the PSA, an additional safety factor \(\eta\) = 5 is added, where the constraint for input stiffness is given as

    $$\begin{aligned} {{K}_{in}}\le \frac{{{K}_{p}}}{\eta }. \end{aligned}$$
    (24)
  2. 2.

    Maximum stress The maximum stress generated at the flexure hinges should be lower than the allowable stress to avoid material failure during the movements. The constraint expression can be guaranteed by Eq. (16), where the safety factor \(n\) is chosen as 3. Besides, the computation accuracy of Eq. (13) is constrained by the parameter of the circular flexure hinge, i.e., \(0<\frac{t}{2r}<2.3\).

  3. 3.

    Working space The stroke of the adopted PSA is \({Q}_{s}=17.4\pm 2.0\,\upmu \!\!\text { m}\). According to Eq. (4), if a minimum output angle \(\theta _{out}^{req}=8\,\text {mrad}\) is required, the following constraint should be satisfied:

    $$\begin{aligned} \frac{{{l}_{OC}}}{{{l}_{OA}}{{r}_{a}}}\ge \frac{\theta _{out}^{req}}{{{Q}_{s}}}. \end{aligned}$$
    (25)
  4. 4.

    Range of parameters The ranges of dimensions for the eight variables are chosen as follows: 12 mm \(\le {{l}_{OA}}\le\) 30 mm, 20 mm \(\le {{l}_{OC}}\le\) 60 mm, 12 mm \(\le {{l}_{CD}}\le\) 30 mm, 25 mm \(\le {{l}_{EG}}\le\) 100 mm, 0.2 mm \(\le {{t}_{i}}\le\) 2.5 mm, 0.2 mm \(\le {{t}_{o}}\le\) 2.5 mm, 1 mm \(\le {r}\le\) 5 mm, 2 mm \(\le {{r}_{a}}\le\) 15 mm. The minimum value of the four distances and \({r}_{a}\) are restricted in order to provide enough fixing and assembly spaces. And the lower bounds of \({t}_{i}\), \({t}_{o}\) and \(r\) are constrained by the technological requirements of the wire-electrical discharge machining (WEDM) process. The upper bounds for the four distances and two radiuses are all limited so as to generate a compact structure. Besides, the thickness of the fabricated material \(w\) and the widths of the lever arm, linkage \({l}_{CD}\) and all the other linkages are specified as 10, 16, 8 and 13 mm, respectively. The parameters \(\alpha\), \({l}_{OB}\) and \({l}_{GI}\) are not considered in this optimization work since they can be determined by other variables according to Eq. (6) and proper assembly considerations.

The objective function for minimization can be taken as:

$$\begin{aligned} f\left( \mathbf {n} \right) =\frac{1}{{{f}_{s}}}=\frac{2\pi }{\sqrt{{{{K}_{in}}}/{{{m}_{s}}}\;}}, \end{aligned}$$
(26)

where \(\mathbf {n}={{\left[ {l}_{OA},{l}_{OC},{l}_{CD},{l}_{EG},{t}_{i},{t}_{o}, r, {r}_{a} \right] }^{T}}\) is the group of variables to be optimized, \({{{f}_{s}}}\) denotes the natural frequency of the stage without consideration of the PSA. The optimization is implemented with the MATLAB optimization toolbox, and the optimal results are: \({l}_{OA}=12\,\text {mm},\) \({l}_{OC}=45.6\,\text {mm}\), \({l}_{CD}=15.7\,\text {mm}\), \({l}_{EG}=59.6\,\text {mm}\), \({t}_{i}=0.63\,\text {mm}\), \({t}_{o}=0.34\,\text {mm}\), \(r=2.1\,\text {mm}\), and \({r}_{a}=5.7\,\text {mm}\).

The final architectural parameters according to the optimized results and the parameters of the material are listed in Table 1. Then the analytical results of static and dynamic performances of the stage can be obtained by (11) and (23), respectively, where the results show that the input stiffness is \(10.2\,{\text {N}}/{\upmu \text {m}}\;\), and the first resonance frequency of the stage without PSA is 43.5 Hz.

Table 1 Parameters of the RCM stage
Full size table

4.2 FEA validation

In order to evaluate the performances of the stage, FEA simulations are carried out with ANSYS Workbench 12. The deformation of the flexures and rigid linkages, stress distribution, input stiffness and dynamic behavior of the stage are studied, respectively.

When a displacement of \(20\,\upmu \!\!\text { m}\) is applied at the input platform along the \(x\) direction, the deformation behaviors of the flexure elements as well as moving linkages are shown in Fig. 8. It can be clearly seen that both of the two parallelogram mechanisms which compose the RCM guiding mechanism strictly follow a parallel trajectory and the output platform is capable of implementing the rotational motion as expected.

Besides, the resulting stress distribution within the whole RCM stage is provided as shown in Fig. 9. It indicates that the stress is only distributed in the flexure elements and the moving linkages almost do not generate any deformations. The maximum stress is 172.2 MPa, which is much lower than the yield strength of the material.

Fig. 8
figure 8

Deformation behavior of the proposed RCM stage

Full size image
Fig. 9
figure 9

Stress distribution of the proposed RCM stage

Full size image

Moreover, to evaluate the input stiffness and kinematic performances of the stage, a group of displacements from 2 to \(20\,\upmu \text {m}\) are applied at the input platform. The exerted input forces and the rotational angles of the output platform are plotted in Fig. 10. It can be observed that the input stiffness evaluated by the FEA is \(9.9\,{\text {N}}/{\upmu \text {m}}\), which is very close to the analytical result (\(10.2\,{\text {N}}/{\upmu \text {m}}\)). The maximum output angle of the stage on the basis of FEA is 9.6 mrad, which is less than the analytical value (12.7 mrad) with a deviation of \(24\,\%.\) This deviation is mainly attributed to the displacement loss induced by the lever amplifier. In fact, the amplification ratio of the lever according to the FEA result is 3.3, which is lower than the theory value of 3.8. Nevertheless, the input-output relation curve obtained by FEA exhibits well linearity, and a further FEA analysis reveals that the attainable maximum output angle for the stage is 29.2 mrad before the maximum stress reaches the yield strength of the material. Besides, to verify the rotational precision of the output platform, the movement of the RCM point is monitored during the simulation, and the shift values are plotted in Fig. 11. Compared with the rotational displacement \({d}_{o}\) of the output platform, which can be calculated as \({{d}_{o}}={l}_{EG}{{\theta }_{out}}\), the maximum center shift of the RCM point accounts for 1.4 % of the latter. The difference for the FEA result from analytical model is attributed to the compression or extension deformations of the flexure hinges that are not considered in the PRBM.

Fig. 10
figure 10

Stiffness and output angle of the stage based on FEA and analytical models

Full size image
Fig. 11
figure 11

Center shift of the RCM point compared with rotational displacement by FEA

Full size image

Finally, model analysis is performed to evaluate the dynamic performance of the stage. For the free vibration, the first four mode shapes of the stage are illustrated in Fig. 12, and the four corresponding resonance frequencies are 41.1, 172.2, 362.2 and 647.1 Hz, respectively. It can be seen that the first resonance frequency evaluated by the FEA coincides with the calculated result of 43.5 Hz. And the first resonance mode is about the rotary motion in the plane and the rests relate to out-of-plane movements, which indicates that the RCM stage owns one single rotational DOF.

Fig. 12
figure 12

First four model shapes of the RCM stage

Full size image

5 Prototype development and experimental studies

In this section, the prototype of the RCM stage is described and its performances are verified by means of experimental studies.

5.1 Prototype development

The prototype of the stage designed with optimal parameters is fabricated using the WEDM technique from a piece of AL7075-T651 material. The stage possesses a dimension of \(140\,\text {mm}\times 96\,\text {mm}\times 10\,\text {mm}\). Concerning the actuator, a piezoelectric stack actuator (AE0505D16F, produced by NEC TOKIN, Inc.) combined with a benchtop piezo controller (BPC303 from Thorlabs) are selected by considering the force and size requirements. The nominal stroke, stiffness, and blocking force of the actuator are \(17.4\pm 2.0\,\text { }\!\!\upmu \!\!\text { m}\), \(48.9\,{\text {N}}/{\upmu \text {m}}\;\), and 850 N, respectively. To test the rotational angle of the output platform, two capacitive sensors (CPL190, probe model: C8-2.0-2.0, from Lion Precision, Inc.) are adopted to measure the displacements of the two side linkages (\(GI\) and \({G}'{I}'\)) along \(x\) directions. Then the rotational angle can be calculated according to Fig. 13, which is given as

$$\begin{aligned} {{\theta }_{out}}=\arccos \left( \frac{{{l}_{I{I}'}}+{{\Delta }_{xI}}-{{\Delta }_{x{I}'}}}{{{l}_{I{I}'}}} \right) , \end{aligned}$$
(27)

where \({\Delta }_{xI}\) and \({\Delta }_{x{I}'}\) denote the measured displacements of the joints \(I\) and \({I}'\) along the x-axis, respectively.

Fig. 13
figure 13

Schematic diagram of computing the output angle of the stage and center shift of the RCM point

Full size image

The analog voltage outputs of the capacitive sensors are collected and transformed by a peripheral component interconnect (PCI)-based data acquisition (DAQ) board (PCI-1816 with 16-bit A/D convectors, from Advantech Co., Ltd), and the digital outputs of the DAQ board are then read by a personal computer through PCIE bus. Besides, in order to preload the PSA, two shim blocks are employed where a high precision adjustment is assured to provides the PSA with essential contact on the input terminal of the stage. The preloading theory for the two shim blocks is described in Li et al. (2013). The experimental setup is shown in Fig. 14.

Fig. 14
figure 14

Experimental setup of the stage for performance tests

Full size image

5.2 Experimental studies

First, the output angle and input displacement of the RCM stage are tested. When the input platform is driven by the PSA to translate along the x-direction, the displacements of the two joints \(I\) and \({I}'\) in the x-axis are measured by two capacitive sensors. Then the rotational angle of the output platform is calculated according to (27). The results are plotted in Fig. 15. It is observed that the stage owns the maximum output angle of 6.96 mrad under the maximum input voltage of 150 V. Compared with the previously designed RCM stage, the newly developed stage possesses a much larger rotational range (nearly quintuple). To verify the input displacement of the stage, the mobile displacement of the input platform is monitored by a capacitive sensor, and the results are also shown in Fig. 15. One can see that the maximum displacement is approximately \(14.9\,\upmu \text {m}\), which is less than the nominal stroke of the PSA. This may be attributed to the effect of the stiffness of flexure hinges and the preloaded force. Besides, we can see that both of the two curves exhibit hysteresis behaviors, which are induced by the inherent nonlinear characteristic of the PSA. The hysteresis effect can be reduced by designing an appropriate control scheme (Gu et al. 2014).

Fig. 15
figure 15

Experimental results of the input displacement and output angle of the stage versus the driving voltage

Full size image

To further verify the relationship between the input displacement and output angle, Fig. 15 is redrawed where the input displacement is chosen as the abscissa, as illustrated in Fig. 16. It can be seen that the hysteresis effect does not affect the linear relationship between the input displacement and output angle. Moreover, compared with the simulation results, the output angle measured by experiment is about \(8.6\,\%\) smaller than that predicted by FEA. The small difference is mainly induced by the manufacturing and measuring errors.

Fig. 16
figure 16

Output angle versus the input displacement

Full size image

Second, the rotational precision of the output platform is tested. With the input displacement applied at the input platform, the displacements of the two joints \(I\) and \({I}'\) along \(x\) and \(y\) directions are measured by the capacitive sensors, respectively. Then the component values of the center shift (\(\delta x\) and \(\delta y\)) can be derived in view of Fig. 13, which are separately given as

$$\begin{aligned} \delta x&={{l}_{I{I}'}}\frac{\sin \left( \beta +{{\theta }_{out}} \right) -\sin \beta }{2\sin \beta }-{{\Delta }_{xI}}\end{aligned}$$
(28a)
$$\begin{aligned} \delta y&={{l}_{I{I}'}}\frac{\cos \beta -\cos \left( \beta +{{\theta }_{out}} \right) }{2\sin \beta }-{{\Delta }_{yI}}, \end{aligned}$$
(28b)

where \(\beta\) represents the initial angle of the linkage \({l}_{EG},\) namely, the included angle between the linkage \({l}_{EG}\) and y-axis, and the value of \({\theta }_{out}\) has been obtained at the first step. The test results are plotted in Fig. 17, where we can see that the maximum shift of the RCM point is less than \(9.2\,\upmu \text {m}\). Compared with the rotational displacement of the output platform (\(415.9\,\upmu \text {m}\)), the maximum center shift of the RCM point only accounts for 2.2 % of the latter, which indicates that the designed stage possesses high rotational precision.

Fig. 17
figure 17

Experimental results of center shift compared with rotational displacement

Full size image

In addition, to test the natural frequency of the RCM stage, an impulse force is exerted on the movable stage by a modal hammer when the PSA is not mounted. The time response and corresponding frequency response obtained by FFT are shown in Fig. 18a, b, respectively. As one can see, the first natural frequency is around 36 Hz, which shows a deviation of 12.4 % relative to the FEA result. The deviation may be caused by the mass of the measuring bracket and machining imperfections.

Fig. 18
figure 18

Frequency test of the stage: a time-domain response and b frequency spectrum

Full size image

In the future work, more direct measurement method such as computer vision-based approach will be adopted to improve the measurement accuracy. Besides, closed-loop control scheme will be implemented to eliminate the hysteresis and creep behaviors of the PSA and therefore, further improve the positioning accuracy of the stage.

6 Conclusion

A flexure-based compliant micropositioning stage with remote-center-of-motion (RCM) characteristic is developed in this paper. The RCM guiding structure is designed with a symmetric double-parallelogram mechanism which possesses smaller actuation radius and higher rotational precision in contrast to conventional instantaneous RCM mechanism and rigid RCM mechanism. To overcome the short stroke of the PSA, an output-stiffness enhanced lever amplifier (OELA) is designed to amplify the input displacement at the input platform to realize desired rotational range. Analytical models have been established to predict kinematics, statics, and dynamics of the RCM stage, which are validated by the finite element analysis (FEA). Besides, dimensional optimization is conducted to maximize the first resonance frequency of the stage. Then the prototype of the stage is fabricated and the performances are tested. Experimental results indicate that the RCM stage has a rotational range of 6.96 mrad which is much bigger than that of the previous design (1.45 mrad), while the maximum center shift of the RCM point is less than \(9.2\,\upmu \text {m}\), both of which validate the effectiveness of the proposed scheme.