The Electoral College, battleground states, and rule-utilitarian voting

Abstract

We extend the Feddersen and Sandroni (Am Econ Rev 96(4):1271–1282, 2006) voter turnout model to include partisan districts, a battleground district, and an Electoral College. We find that expected voter turnout by a single party is highest in the battleground district, followed by the party’s majority district, which in turn is followed by the party’s minority district. Total turnout is higher in the battleground district than in the partisan districts, but the gap decreases as the level of disagreement in the partisan districts increases. Lastly, turnout in the battleground district decreases as the partisan districts become more competitive.

1 Introduction

In United States presidential elections, not all votes are equal. The winner is determined through the Electoral College, which typically awards all Electoral seats in the state to the candidate with the majority of votes in that state. The winner-take-all nature of the Electoral College gives some citizens a higher chance of being pivotal than others (Gelman et al. 2012). Both George W. Bush in 2000 and Donald Trump in 2016 won the presidency without winning the popular vote. Fewer than 1000 additional votes for Al Gore in Florida could have changed the result of the 2000 presidential election, while an additional million votes for Gore in Texas would have made no difference (Federal Election Commission 2001). Several studies have found that voter turnout is higher in these so-called battleground states (McDonald 2004; Lipsitz 2009; Cebula 2001; Cebula and Meads 2008). This paper explores whether a rule-utilitarian voting model can explain this result. We consider a model with 3 districts: a conservative district, a liberal district, and a battleground district. To win the election, a candidate must win two of the three districts. Citizens with positive voting costs must decide whether to turn out or abstain.

The simplest way to think about voting is that people should vote if the benefit from voting is greater than the cost. Following Downs (1957), suppose p is the probability that an individual affects the outcome of an election (that is the probability that the individual makes or breaks a tie), B is the increase in utility an individual receives by having their preferred party win the election, and c is some strictly positive cost of voting. Then an individual should vote if $pB>c$.

The problem with this explanation is that as the number of voting citizens increases, the probability of being pivotal likely decreases towards zero. For any positive voting cost, there should exist an electorate large enough to discourage an individual from voting. Turnout in presidential elections is high, even though the number of ballots cast is large. One possible way to get around this problem is to redefine the benefits from voting. Perhaps people get some increase in utility related to duty from casting a ballot, possibly from a sense of patriotism. Following Riker and Ordeshook (1968), let D be the utility gain from casting a ballot, then an individual should vote if $pB+D>c$.

This approach is theoretically uninteresting. In large elections in which pB is close to zero, it states that those who vote do so because they get utility from voting. It is equivalent to assuming that voting costs are negative for those who turn out to vote. However, it does have one advantage. When talking to individuals about why they vote, many people refer to a sense of civic duty or patriotism (Blais and Achen 2010). Duty most likely has some role to play since the individual probability of being pivotal is negligible in any large election.

Another approach is to think more seriously about what it means “to do one’s part” in the context of an election. This is the approach that Feddersen and Sandroni (2006) take. They consider a model in which a random fraction of voters are ethical, but the action ethical voters take is endogenous to the model. They define duty as following a voting rule, that if followed by all individuals of the same type, would maximize social welfare of that type. This does not mean that individuals should always turn out. The benefit of an individual turning out to vote might be outweighed by the cost. Thus, the socially optimal rule will be a cutoff rule, in which an individual turns out to vote if their voting costs are below the cutoff and otherwise they abstain. A consequence of the model is that voters turn out in large elections even though they are never individually pivotal. Similarly, Coate and Conlin (2004) develop a model in which they assume that voters get utility from following a rule that maximizes the welfare of only their group. They then structurally estimate the model using Texas Liquor referenda and find that it outperforms many simpler models.¹

The work of Feddersen and Sandroni (2006) and Coate and Conlin (2004) considers large elections in which no individual is pivotal and the winner is determined through the popular vote.² This paper attempts to extend the rule-utilitarian logic of these papers to elections with a winner-take-all Electoral College.³ In addition to finding properties that are analogous to those in Feddersen and Sandroni (2006), we demonstrate that rule-utilitarian voters will turn out at higher rates in battleground states than in partisan states, even though voters in battleground states are never individually pivotal. Furthermore, partisan states that are more contested (and are thus similar to battleground states) have higher turnout than partisan states that are less contested.

These results are similar to the competition and underdog effects first described in Levine and Palfrey (2007) in the context of the pivotal-voter model. The competition effect states that turnout should be higher in elections that would be closer if all voters were to cast ballots. The underdog effect states that smaller groups turn out at a higher rate, but not enough to win the election on average (Levine and Palfrey 2007; Faravelli et al. 2015). Both of these effects are a feature of the Feddersen and Sandroni (2006) model, but the first ethical voting model to use the terminology is Ali and Lin (2013). The competition effect holds in our model, but the underdog effect holds only when making comparisons within a district, and not for comparisons between districts. For example, in the partisan district, the minority party has a higher cutoff rule than the majority party (the underdog effect). However, sometimes the minority party in the partisan district has a lower cutoff than either party in the battleground district. We also find that turnout decreases in the battleground district as the partisan districts become more contested. This occurs because if the battleground district is not unique as the only close election, then the probability that the battleground district is pivotal in the Electoral College decreases. We refer to this as the Electoral College effect.

While our model most closely resembles the U.S. Electoral College, it also contributes to the literature on other electoral systems that pick heads of government through indirect elections. In Westminster democracies, districts elect members of parliament, and then the parliament picks the head of government through majority rule. Thus, the Prime Minister needs the majority of seats in parliament, but not necessarily the popular vote. In 1951, Winston Churchill (leader of the Conservative Party) was elected Prime Minister, although the Labour Party won the popular vote.

2 The Electoral College model

Consider an election between two candidates: candidate 1 (a liberal) and candidate 2 (a conservative). There are two types of citizens. Type 1 strictly prefers candidate 1, and type 2 strictly prefers candidate 2. Suppose there are three districts with a continuum of citizens in each district. District 1 leans liberal, district 2 leans conservative, and district 3 is split between the two candidates. More precisely, the fraction of citizens who support the conservative is k in district 1, $1-k$ in district 2, and $\frac{1}{2}$ in district 3, with $k \in \left( 0, \frac{1}{2} \right)$. District 3 is the battleground district.

The parameter k is the level of disagreement within the partisan districts. When k increases, the probability that two random voters in the district prefer the same candidate decreases. For k close to zero, the liberal candidate has near universal support in the liberal district and the conservative candidate has near universal support in the conservative district. Thus, for low levels of k, there are low levels of disagreement within the partisan districts. Low levels of disagreement within the partisan districts also implies that there are high levels of disagreement between the liberal and conservative districts. In Feddersen and Sandroni (2006), k measures the level of disagreement in the population. In our model, the population is by definition split (that is to say national support for each party is 50 percent if all three districts are the same size). However, the geographic distribution of voters is not uniform across districts. In the battleground district, there is more disagreement about who should win the election than in a partisan district, where there is a clear majority.

Citizens must decide whether to vote for candidate 1, vote for candidate 2, or abstain. Voting is costly, and the cost of voting for individual i is $c_i$ which is uniformly distributed on $\left[ 0, \bar{c} \right]$. Voting costs are distributed independently across i and independent of all other random variables in the model. Individual i observes the realization of her own voting costs before deciding whether to vote, but only knows the distribution from which other individuals’ voting costs are drawn.⁴

To win a district, a candidate must receive the majority of votes cast in that district, and to win the election, a candidate must win two of the three districts. In the event of a tie, the winner of the district is determined through the flip of a fair coin.

A strategy in this setting is a function from voting costs to the set of actions. Since all voters have strict preferences over candidates, if a voter turns out to vote, then they will vote for their most preferred candidate. Voters follow a cutoff strategy in which they turn out if their voting costs are below some critical cutoff and abstain otherwise.

Following Feddersen and Sandroni (2006), if a type 1 or type 2 individual decided the group-rule for their type, then they would pick lotteries over outcomes according to the following utility functions:

$$\begin{aligned} U_1(p, w, \phi )= & {} pw-\phi \end{aligned}$$

(1)

$$\begin{aligned} U_2(p, w, \phi )= & {} (1-p)w-\phi , \end{aligned}$$

(2)

where p is the probability that candidate 1 wins the election, w is the importance of the election, and $\phi$ is the expected total social cost of voting. Holding p fixed, the group-rule decision maker prefers lower turnout because it induces lower social cost from voting.⁵

Without entering duty into the model, there does not exist an equilibrium in which a strictly positive fraction of voters cast ballots. A strictly positive fraction of voters turning out implies that each voter is pivotal with probability zero and would be better off abstaining. There also cannot exist an equilibrium in which no one turns out, because then voters would have strictly positive probabilities of being pivotal, and voters with sufficiently low voting costs would be better off casting ballots. As in Feddersen and Sandroni (2006), we assume that some fraction of agents are ethical agents, and they get utility from “doing their part”. In this setting, doing their part means following a turnout rule that if followed by all other ethical agents of the same type and district would maximize the expected utility of that type as written in Eqs. (1) and (2), holding fixed the turnout rules of everyone else. The ethical agents will get utility $D>\bar{c}$ for doing their part, and thus, it will always be to their benefit to do so. Other agents get zero utility from doing their part, and since voting is costly, these agents will abstain.

Suppose that the fraction of type j agents in district d who are ethical is $q_j^d$ for $j \in \{ 1, 2 \}$ and $d \in \{1, 2, 3 \}$. Further assume that $q_j^d$ is a random variable that is distributed uniformly over the unit interval and is independent across j, d, and all other random variables in the model.

An equilibrium is characterized by six cutoff points, one for each district and type. Suppose the ethical agents use the cutoff points $\sigma _j^d$, where $j \in \{ 1, 2 \}$ indexes the type and $d \in \{1, 2, 3 \}$ indexes the district. Then candidate 1 wins district 1 if:

$$\begin{aligned} (1-k)q_1^1\sigma _1^1 > kq_2^1 \sigma _2^1 \Leftrightarrow \frac{q_2^1}{q_1^1} < \frac{(1-k)\sigma _1^1}{k \sigma _2^1}. \end{aligned}$$

(3)

Define $p_d(\sigma _1^d, \sigma _2^d)$ to be the probability that candidate 1 wins district d given the cutoff points $\left( \sigma _1^d, \sigma _2^d \right)$. Then for district 1, we get that

$$\begin{aligned} p_1(\sigma _1^1, \sigma _2^1)=F\left( \frac{(1-k)\sigma _1^1}{k \sigma _2^1} \right) \end{aligned}$$

(4)

where F is the cumulative probability distribution of the random variable $\frac{q_2^1}{q_1^1}$.

By symmetry, we get that

$$\begin{aligned} p_2(\sigma _1^2, \sigma _2^2)= & {} F\left( \frac{k\sigma _1^2}{(1-k) \sigma _2^2} \right) \end{aligned}$$

(5)

$$\begin{aligned} p_3(\sigma _1^3, \sigma _2^3)= & {} F\left( \frac{ \frac{1}{2}\sigma _1^3}{ \frac{1}{2} \sigma _2^3} \right) =F\left( \frac{\sigma _1^3}{\sigma _2^3} \right) . \end{aligned}$$

(6)

There are four events in which candidate 1 wins the Electoral College. This is when candidate 1 wins districts 1 and 2, districts 1 and 3, districts 2 and 3, or all 3 districts. Define $\sigma =\left( \sigma _1^1, \sigma _1^2, \sigma _1^3, \sigma _2^1, \sigma _2^2, \sigma _2^3 \right)$, and let $p(\sigma )$ be the probability that candidate 1 wins the election given the rule profile $\sigma$. Then by the independence of the q’s,

$$\begin{aligned} p(\sigma )= & {} F\left( \frac{(1-k)\sigma _1^1}{k \sigma _2^1} \right) F\left( \frac{k\sigma _1^2}{(1-k) \sigma _2^2} \right) \nonumber \\&+F\left( \frac{(1-k)\sigma _1^1}{k \sigma _2^1} \right) \left( 1-F\left( \frac{k\sigma _1^2}{(1-k) \sigma _2^2} \right) \right) F\left( \frac{\sigma _1^3}{\sigma _2^3} \right) \nonumber \\&+\left( 1-F\left( \frac{(1-k)\sigma _1^1}{k \sigma _2^1} \right) \right) F\left( \frac{k\sigma _1^2}{(1-k) \sigma _2^2}\right) F\left( \frac{\sigma _1^3}{\sigma _2^3} \right) . \end{aligned}$$

(7)

Equation 7 gives us the probability of candidate 1 winning the election as a function of parameters of the model and choice variables. To find the equilibrium strategies, we must now find $\phi$ as a function of parameters and choice variables. Because only the ratio of w to $\bar{c}$ matters, we normalize $\bar{c}$ to 1. It follows that expected total social cost of the election is

$$\begin{aligned} \phi (\sigma )= & {} (1-k)E(q_1^1)\sigma _1^1 \int _0^{\sigma _1^1}\frac{1}{\sigma _1^1}x \partial x+ kE(q_2^1)\sigma _2^1 \int _0^{\sigma _2^1}\frac{1}{\sigma _2^1}x \partial x\nonumber \\&+kE(q_1^2)\sigma _1^2 \int _0^{\sigma _1^2 }\frac{1}{\sigma _1^2 }x \partial x + (1-k)E(q_2^2)\sigma _2^2 \int _0^{\sigma _2^2}\frac{1}{\sigma _2^2}x \partial x \nonumber \\&+\frac{1}{2}E(q_1^3)\sigma _1^3 \int _0^{\sigma _1^3}\frac{1}{\sigma _1^3}x \partial x+ \frac{1}{2}E(q_2^3) \sigma _2^3 \int _0^{\sigma _2^3}\frac{1}{\sigma _2^3}x \partial x \nonumber \\= & {} \frac{1}{4}\!\bigg [ (1-k)(\sigma _1^1)^2 + k(\sigma _2^1)^2 + k(\sigma _1^2)^2 +\! (1-k)(\sigma _2^2)^2\! +\frac{1}{2}(\sigma _1^3)^2 + \frac{1}{2}(\sigma _2^3)^2 \bigg ]\quad . \end{aligned}$$

(8)

Given a rule profile $\sigma$, define induced payoffs $R_1(\sigma )=wp(\sigma )-\phi (\sigma )$ for type 1 individuals and $R_2(\sigma )=w(1-p(\sigma ))-\phi (\sigma )$ for type 2 individuals. Further, let $\sigma _j=(\sigma _j^1, \sigma _j^2, \sigma _j^3) \in \left[ 0, \bar{c} \right] ^3$, which is the vector of cutoffs type j uses in the three districts. Notice that $\sigma =\sigma _1 \times \sigma _2 = \left( \sigma _1^1, \sigma _1^2, \sigma _1^3, \sigma _2^1, \sigma _2^2, \sigma _2^3 \right) \in \left[ 0, \bar{c} \right] ^6.$ We can now define a consistent equilibrium, developed by Feddersen and Sandroni (2006), in this model.

Definition

A rule profile $\sigma ^*$ is consistent if the following two conditions hold:

• $R_1(\sigma _1^*, \sigma _2^*) \ge R_1(\sigma _1, \sigma _2^*)$ for every $\sigma _1 \in \left[ 0, 1 \right] ^3$

• $R_2(\sigma _1^*, \sigma _2^*) \ge R_2(\sigma _1^*, \sigma _2)$ for every $\sigma _2 \in \left[ 0, 1 \right] ^3$.

If a rule profile is not consistent, then individuals of at least one type in one district must believe that their type would be better off if they deviated to a different rule. We search for a symmetric equilibrium in which both types follow the same strategy in minority districts, majority districts, and in the battleground district. The parameters, random variables, and choice variables of the model are summarized in Table 1.

Table 1 Summary of variables and parameters

3 Results

We now turn to the properties of the symmetric consistent equilibrium. Because the model is symmetric, the probability that the conservative candidate wins the election is equal to the probability that the liberal candidate wins the election and is $\frac{1}{2}$ in equilibrium. Closed-form solutions for the symmetric equilibrium in terms of the parameters k and w and proofs of these properties are in the Appendix.

For the Propositions, we restrict the parameters to those that yield an interior solution. These equilibria are the most interesting because there is some abstention. In practice, this means that the importance of the election cannot be too high and the level of disagreement cannot be too low. Exactly how low the importance of the election must be to get an interior equilibrium depends on the level of disagreement.⁶

Proposition 1

Suppose w and k are such that we have an interior equilibrium. Then the following holds: the equilibrium cutoff rule is decreasing in k for the minority and battleground districts, but increasing in k for the majority district. Furthermore, if the level of disagreement is low ($k \in (0,\frac{1}{5}]$), then the equilibrium cutoff is highest in the minority district. If the level of disagreement is high ($k \in (\frac{1}{5},\frac{1}{2})$), then the equilibrium cutoff is highest in the battleground district. The cutoff is always lowest in the majority district.

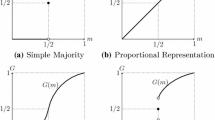
Proposition 1 is displayed graphically in Fig. 1. Proposition 1 implies that the cutoff rule is always the lowest in the majority district. However, the relative ordering of the cutoff rules in the battleground and minority districts depends on the level of disagreement in the partisan districts. For low levels of disagreement, the cutoff rule is higher in the minority district; for high levels of disagreement, the cutoff rule is higher in the battleground district.

Fig. 1

Symmetric consistent equilibrium cutoffs. For purposes of example, $w=0.6$

In the FS model, the cutoff rule is higher for the minority party. This is the underdog effect–smaller groups use higher cutoff rules. In the Electoral College model, since ${\sigma _1^1}^* \le {\sigma _1^2}^*$, the underdog effect holds when making comparisons within districts, but not when making comparisons across districts. This is because if $k > \frac{1}{5}$, then the cutoff rules for both parties are higher in the battleground district than for the minority party in the partisan districts. As k increases, the minority party becomes less of an underdog, thus decreasing the underdog effect. The cutoff rules converge as k approaches $\frac{1}{2}$ because then every district is a battleground district.

The cutoff rule for the battleground district decreases in k for an entirely different reason. For the battleground district to matter in the Electoral College, the other two partisan districts must be split; otherwise, the district is not pivotal. If k is close to 0, then the partisan districts will be split with high probability. As the partisan districts become more like the battleground district, then there will be a non-trivial probability that a single party will win both partisan districts. Thus, the probability that the battleground district is pivotal decreases with k. Consequently, the optimal cutoff in the battleground district decreases as well. We refer to this as the Electoral College effect. Note that as k varies, the Electoral College effect only applies to the battleground district and not the partisan districts. This is because in a symmetric equilibrium, each party has a one half chance of winning the battleground district and the probability that they win their majority district equals one minus the probability they win their minority district (i.e., $p_3=\frac{1}{2}$ and $p_1=1-p_2$). This implies that the probability that a partisan district is pivotal is constant at $\frac{1}{2}$.

Proposition 2

Suppose w and k are such that we have an interior equilibrium. Then the following holds: expected turnout by a single party is highest in the battleground district, followed by the party’s majority district, which in turn is followed by the party’s minority district. Expected turnout by a single party is increasing in k for the party’s majority and minority districts, but decreasing in k for the battleground district.

Figure 2 demonstrates Proposition 2. To turn out, a voter must both be ethical and have voting costs below the cutoff rule. Therefore, expected turnout for a single type is the fraction of voters who are ethical times the group size times the cutoff rule. This implies that expected turnout for a single party is $\frac{1}{2}(1-k)\sigma _1^1$ in the majority district, $\frac{1}{2}k\sigma _1^2$ in the minority district, and $\frac{1}{2}\frac{1}{2}\sigma _1^3$ in the battleground district. Proposition 2 is the competition effect in this model. Turnout is higher in the battleground district, because the election is more contested. As the level of disagreement in the partisan districts increases, the partisan districts are more similar to the battleground district. Parties must turn out more to defend their majority district, and also increase turnout in the minority district in an attempt to out-mobilize the opposing party’s majority. Turnout decreases in the battleground district because that district is no longer unique as the only contested election. For example, if Texas and California began to resemble Florida politically, then turnout would increase in Texas and California because their states are more competitive (the competition effect). Turnout might decrease in Florida because now Florida would no longer be unique as one of the few large swing states and now has a lower probability of being pivotal in the Electoral College (the Electoral College effect). Conversely, if the partisan districts become more partisan, then the probability that the battleground district is pivotal in the general election increases and voter turnout in the battleground district should increase accordingly.

Fig. 2

Expected turnout by type. For purposes of example, $w=0.6$. Expected turnout by a single party is $\frac{1}{2}(1-k)\sigma _1^1$ in the majority district, $\frac{1}{2}k\sigma _1^2$ in the minority district, and $\frac{1}{2}\frac{1}{2}\sigma _1^3$ in the battleground district

Proposition 3

Suppose w and k are such that we have an interior equilibrium. Then the following holds: total turnout of both parties is decreasing in k for the battleground district and increasing in k for the partisan districts. As k approaches $\frac{1}{2}$, expected turnout in all three districts converge.

This Proposition is displayed in Fig. 3. Note that in this model, turnout is higher in battleground districts than in partisan districts even though no individual vote is pivotal and there are no politicians strategically campaigning.

Fig. 3

Expected turnout by district. For purposes of example, $w=0.6$

Proposition 4

Suppose w and k are such that we have an interior equilibrium. Then the following holds: As k increases towards $\frac{1}{2}$, the probability of a party winning their majority district decreases, the probability of winning their minority district increases, and the probability of winning the battleground district is constant at $\frac{1}{2}$.

Proposition 4 states that even though the minority party turns out at a higher rate than the majority party in the partisan districts, the majority party is still large enough that they are more likely to win the election. This is a common feature of the underdog effect (Levine and Palfrey 2007; Faravelli et al. 2015). Although turnout varies with k in the battleground district, since the equilibrium is symmetric, it increases identically for both the liberal and conservative parties.

4 Discussion and conclusion

This model considers the implications of a winner-take-all Electoral College when political support for candidates is not independently distributed across districts and some random fraction of voters behave ethically. We find that voter turnout is higher in the battleground district than in the partisan districts even though no citizen is individually pivotal. Previous empirical studies have attributed this to strategic campaigns and advertising. However, our paper shows that this is not necessary for turnout to be higher in battleground states. We also show that the underdog effect holds, but only when making comparisons within districts. The underdog effect does not hold when comparing turnout in the battleground state to turnout in the partisan states.

Our model makes similar predictions to those that appear in Feddersen and Sandroni (2006), but we allow for the voting behavior in a district to be endogenous to voting behavior in other districts. A result of this endogeneity is that turnout in battleground states decreases as the partisan states become more contested, a feature we term the Electoral College effect. This effect occurs because if partisan districts are more competitive, then the probability that the battleground district will be pivotal in the Electoral College decreases. A possibility for future research is to extend the model to 51 states, allow each state to have a different number of electors, and relax the symmetry assumption. This would allow for structural estimation of the parameters of the model in the spirit of Coate and Conlin (2004).

Appendices

Appendix A: Solving for the symmetric equilibrium

1.1 Interior solution

We restrict our search to symmetric equilibria in which $\sigma _1^{1*}=\sigma _2^{2*} =\alpha$, $\sigma _1^{2*}=\sigma _2^{1*} =\beta$, and $\sigma _1^{3*}=\sigma _2^{3*}=\gamma$. The CDF for the ratio of two independent standard uniform random variables is

$$\begin{aligned} F(z) = \left\{ \begin{array}{ll} \frac{z}{2} &{} \text {if }z \le 1;\\ 1-\frac{1}{2z} &{} \text {if }z >1.\end{array} \right. \end{aligned}$$

The payoff functions that each party seeks to maximize are $R_1(\sigma ) = wp(\sigma )-\phi (\sigma )$ and $R_2(\sigma ) = w(1-p(\sigma ))-\phi (\sigma )$. We can write $p(\sigma )=p_1p_2 + p_1(1-p_2)p_3+(1-p_1)p_2p_3$, where $p_1= F\left( \frac{(1-k)\sigma _1^1}{k \sigma _2^1} \right)$, $p_2 = F\left( \frac{k\sigma _1^2}{(1-k) \sigma _2^2} \right)$, and $p_3 = F\left( \frac{\sigma _1^3}{\sigma _2^3} \right)$. With respect to the partisan district cutoff rules ($\sigma _1^1$, $\sigma _1^2$, $\sigma _2^1$ and $\sigma _2^2$), $p_3$ is constant and is $\frac{1}{2}$ in a symmetric equilibrium. This allows the simplification $p(\sigma )=\frac{1}{2}(p_1+p_2)$ when evaluating the FOCs in the partisan districts. Taking the first order conditions and evaluating at $\sigma _1^1=\sigma _2^2=\alpha$ and $\sigma _1^2=\sigma _2^2=\beta$ gives us that

$$\begin{aligned} \frac{\partial {R_1}}{\partial {\sigma _1^1}} = \frac{\partial {R_2}}{\partial {\sigma _2^2}} = \frac{w}{2}\frac{1-k}{k}\frac{1}{\beta }f\Bigg (\frac{1-k}{k}\frac{\alpha }{\beta }\Bigg ) -\frac{1}{2}(1-k)\alpha \end{aligned}$$

and

$$\begin{aligned} \frac{\partial {R_1}}{\partial {\sigma _1^2}} = \frac{\partial {R_2}}{\partial {\sigma _2^1}} = \frac{w}{2}\frac{k}{1-k}\frac{1}{\alpha }f\Bigg (\frac{k}{1-k}\frac{\beta }{\alpha }\Bigg ) -\frac{1}{2}k\beta . \end{aligned}$$

For the third condition we first simplify $R_1$. Note that the $p_1$ and $p_2$ are constant with respect to battleground district cutoffs and $p_1=1-p_2$ in a symmetric equilibrium. Then, $p(\sigma )=p_1p_2 +(2p_1^2-2p_1+1)p_3,$ which gives us the last FOC (evaluated at $\alpha$, $\beta$, and $\gamma$):

$$\begin{aligned} \frac{\partial {R_1}}{\partial {\sigma _1^3}} = \frac{\partial {R_2}}{\partial {\sigma _2^3}} = w\frac{1}{2\gamma }\Bigg (2F^2\Bigg (\frac{1-k}{k}\frac{\alpha }{\beta }\Bigg ) - 2F\Bigg (\frac{1-k}{k}\frac{\alpha }{\beta }\Bigg )+1\Bigg )-\frac{\gamma }{4}. \end{aligned}$$

For $\frac{1-k}{k}\frac{\alpha }{\beta } >1$, the first two first order conditions are:

$$\begin{aligned} \beta = \frac{(1-k)^2}{k}\frac{2}{w}\alpha ^3,\quad \alpha = \frac{w}{2}\frac{1}{1-k}\frac{1}{\beta } \end{aligned}$$

and give

$$\begin{aligned} \alpha= & {} \sigma _1^{1*}=\sqrt{w}\left( \frac{k}{4(1-k)^3}\right) ^{\frac{1}{4}} \end{aligned}$$

(9)

$$\begin{aligned} \beta= & {} \sigma _1^{2*}=\sqrt{w}\frac{1}{(4k(1-k))^{\frac{1}{4}}} \end{aligned}$$

(10)

$$\begin{aligned} \gamma= & {} \sigma _1^{3*}=\sqrt{w}\left[ \frac{k}{1-k}-2\left( \frac{k}{1-k}\right) ^{\frac{1}{2}}+2\right] ^{\frac{1}{2}} \end{aligned}$$

(11)

The above equilibrium is the unique symmetric interior solution. To see this, suppose $\frac{1-k}{k}\frac{\alpha }{\beta } \le 1$. Re-taking the FOCs gives $\frac{\alpha }{\beta }=\sqrt{\frac{k}{1-k}}$, which implies $k\ge \frac{1}{2}$, which is a contradiction.

1.2 Boundary equilibria

Since $k, w > 0$, it follows that $\alpha , \beta , \gamma > 0$. Thus, as long as the election has positive importance, no optimal $\sigma$ will ever be on the 0 boundary. Since the ordering of the cutoffs is dependent on k, we have two cases.

1.2.1 Low levels of disagreement: $k \in (0, \frac{1}{5}]$

By Proposition 1, we know that $\sigma _1^{1*}\le \sigma _1^{3*}\le \sigma _1^{2*}$. Thus, when $k<\frac{1}{5}$, the equilibrium takes on one of four cases: (1) the interior solution; (2) only $\sigma _1^{2*}$ is on the boundary; (3) only $\sigma _1^{2*}$ and $\sigma _1^{3*}$ are on the boundary; or (4) all three cutoffs are on the boundary. The previous subsection details the interior solutions.

Case 2: Taking $\sigma _1^{2*} = 1$ and retaking FOCs for $\sigma _1^{3*}$ and $\sigma _1^{1*}$ gives boundary equilibrium of

$$\begin{aligned} {\sigma ^1_1}^*&= \left( \frac{kw}{2(1-k)^2}\right) ^{\frac{1}{3}}, \quad {\sigma _1^3}^* = \left[ \left( \frac{4wk^4}{(1-k)^2} \right) ^{\frac{1}{3}}-\left( \frac{16w^2k^2}{(1-k)}\right) ^{\frac{1}{3}} +2w\right] ^{\frac{1}{2}},\nonumber \\ {\sigma _1^2}^*&= 1 . \end{aligned}$$

The interval is:

$$\begin{aligned} \left[ \left( \frac{4wk^4}{(1-k)^2} \right) ^{\frac{1}{3}}-\left( \frac{16w^2k^2}{(1-k)}\right) ^{\frac{1}{3}} +2w\right] ^{\frac{1}{2}}<1,\quad \frac{1}{w} \le \frac{1}{(4k(1-k))^{\frac{1}{2}}}. \end{aligned}$$

Case 3: Taking both $\sigma _1^{2*}$ and $\sigma _1^{3*}$ on the boundary and retaking the FOCs yields

$$\begin{aligned} {\sigma ^1_1}^* = \left( \frac{kw}{2(1-k)^2}\right) ^{\frac{1}{3}}, \quad {\sigma _1^3}^* = 1, \quad {\sigma _1^2}^* = 1. \quad \end{aligned}$$

The interval is:

$$\begin{aligned} \frac{k}{2(1-k)^2} < \frac{1}{w}, 1 \le \left[ \left( \frac{4wk^4}{(1-k)^2} \right) ^{\frac{1}{3}}-\left( \frac{16w^2k^2}{(1-k)}\right) ^{\frac{1}{3}} +2w\right] ^{\frac{1}{2}}. \end{aligned}$$

Case 4: All three cutoffs are on the boundary when $\sigma _1^{1*}$ from Case 3 reaches 1. The interval is

$$\begin{aligned} \frac{1}{w} \le \frac{k}{2(1-k)^2}. \end{aligned}$$

1.2.2 High-levels of disagreement: $k \in (\frac{1}{5}, \frac{1}{2})$

By Property 1, the cutoffs are now ordered $\sigma _1^{1*} \le \sigma _1^{2*} \le \sigma _1^{3*}$. Setting ${\sigma _1^3}^* = 1$ and retaking the FOCs for $\sigma _1^2$ and $\sigma _1^1$ yields that

$$\begin{aligned} {\sigma ^1_1}^* =\frac{w^{\frac{1}{2}}k^{\frac{1}{4}}}{(4(1-k)^3)^\frac{1}{4}}, \quad {\sigma _1^2}^* =\frac{w^{\frac{1}{2}}}{(4k(1-k))^{\frac{1}{4}}}, \quad {\sigma _1^3}^* = 1. \end{aligned}$$

The interval is:

$$\begin{aligned} \frac{1}{(4k(1-k))^{\frac{1}{2}}} < \frac{1}{w} \le \left[ \frac{k}{1-k}-2\left( \frac{k}{1-k}\right) ^{\frac{1}{2}} +2\right] . \end{aligned}$$

When both the largest and second largest cutoffs are at the boundary, we get that

$$\begin{aligned} {\sigma ^1_1}^* = \left( \frac{kw}{2(1-k)^2}\right) ^{\frac{1}{3}},\quad {\sigma _1^2}^* = 1,\quad {\sigma _1^3}^* = 1. \end{aligned}$$

The interval is:

$$\begin{aligned} \frac{k}{2(1-k)^2} < \frac{1}{w} \le \frac{1}{(4k(1-k))^{\frac{1}{2}}}. \end{aligned}$$

All three cutoffs are on the boundary when $\sigma _1^1$ from the previous case reaches 1, which occurs when

$$\begin{aligned} \frac{1}{w} \le \frac{k}{2(1-k)^2}. \end{aligned}$$

Appendix B: Proofs of properties

1.1 Proposition 1

We first show that cutoff rules are decreasing in k for the minority and battleground districts, and increasing in k for the majority district. The derivative of the interior solutions from Eqs. 9, 10, and 11 are:

$$\begin{aligned} \frac{\partial {\sigma _1^1}^*}{\partial k}= & {} \frac{\sqrt{2w}}{2}\left( \frac{ \frac{1}{4} (1-k)^{\frac{3}{4}} k^{-\frac{3}{4}} + k^\frac{1}{4} \frac{3}{4} (1-k)^ {-\frac{1}{4}} }{(1-k)^\frac{3}{2}} \right) \\ \frac{\partial {\sigma _1^2}^*}{\partial k}= & {} -\sqrt{w} \frac{ \frac{1}{4}(4-8k) }{ (4k(1-k))^\frac{5}{4}} \\ \frac{\partial {\sigma _1^3}^*}{\partial k}= & {} \frac{\sqrt{w}}{(1-k)^2\sqrt{k}} \times \frac{\sqrt{k}-\sqrt{1-k}}{2 \left( \frac{k}{1-k}-2 \left( \frac{k}{1-k} \right) ^\frac{1}{2}+2 \right) ^\frac{1}{2}}. \end{aligned}$$

The partial derivative for the majority district, $\frac{\partial {\sigma _1^1}^*}{\partial k}$, is clearly positive, implying that the cutoff rule in the majority district is increasing in k. Since $k<\frac{1}{2}$, $\frac{\partial {\sigma _1^2}^*}{\partial k}$ is negative, implying the cutoff rule in the minority district is decreasing in k. Since $\sqrt{k}-\sqrt{1-k}<0$ for all $k<\frac{1}{2}$, it follows that the cutoff rule is decreasing for the battleground district.

We next show that the cutoff rule in the majority district is always lower. First note that all of the cutoff rules converge to $\sqrt{w}$ if $k=\frac{1}{2}$. Since the cutoff rule is increasing in k for the majority district, this must be the maximum cutoff for that district. Since the cutoff rules are decreasing in the minority and battleground district, this is the minimum cutoff for those districts. Thus, the majority district always has the lowest cutoff.

Lastly, we show that for high levels of disagreement ($k>\frac{1}{5}$), the cutoff is higher in the battleground district than in the minority district, and that for low levels of disagreement ($k<\frac{1}{5}$), the cutoff rule is higher in the minority district. With much algebra, it can be shown that the majority district has a higher cutoff rule if and only if $20k^3-24k^2+9k-1$ is positive. This polynomial is negative for k in $(0,\frac{1}{5})$ and positive for k in $(\frac{1}{5},\frac{1}{2})$.

1.2 Proposition 2

The Proposition states $\frac{1}{2}\frac{1}{2}\sigma _1^{3*}> \frac{1}{2}(1-k)\sigma _1^{1*} > \frac{1}{2}k\sigma _1^{2*}.$ First we show that $\frac{1}{2}\sigma _1^{3*}>\frac{1}{2}w^{\frac{1}{2}}>(1-k)\sigma _1^{1*}$. Some algebra will show that $\frac{1}{2}\sigma _1^{3*}>\frac{1}{2}w^{\frac{1}{2}}$ if and only if $4k^2-4k+1$ is positive, which is true for all $k \in (0,\frac{1}{2})$ since the polynomial has a global minimum of 0 at $k=\frac{1}{2}$.

Next, $\frac{1}{2}w^{\frac{1}{2}}>(1-k)\sigma _1^{1*} \Longleftrightarrow \frac{1}{4} > (1-k)k$. This inequality is assured by $k \in (0,\frac{1}{2})$. Thus we have that $\frac{1}{2}\frac{1}{2}\sigma _1^{3*} > \frac{1}{2}(1-k)\sigma _1^{1*}$.

Next,

$$\begin{aligned} (1-k)\sigma _1^{1*}> & {} k\sigma _1^{2*}\\\Longleftrightarrow & {} \frac{k^{\frac{1}{4}}(1-k)}{(1-k)^{\frac{3}{4}}}> \frac{k}{((1-k)k)^{\frac{1}{4}}}. \\\Longleftrightarrow & {} k(1-2k)> 0, \end{aligned}$$

which holds since $k \in (0, \frac{1}{2})$, and gives $\frac{1}{2}(1-k)\sigma _1^{1*} > \frac{1}{2}k\sigma _1^{2*}$, which finishes the proof of the first part of the Proposition.

The proof of Proposition 1 showed that $\frac{d\sigma _1^{3*}}{dk}>0$, so it follows that a party’s expected turnout $\frac{1}{2}\frac{1}{2}\frac{d\sigma _1^{3*}}{dk}>0$ also.

Expected turnout for the majority party is

$$\begin{aligned} \frac{1}{2}(1-k)\sigma _1^{1*} = \frac{1}{2}\left( \frac{w}{2}\right) ^{\frac{1}{2}}\big ((1-k)k)^{\frac{1}{4}}. \end{aligned}$$

Its derivative is

$$\begin{aligned} \frac{1}{8}\big (\frac{w}{2}\big )^{\frac{1}{2}}\frac{1-2k}{\left( k-k^2\right) ^{\frac{3}{4}}}>0 \end{aligned}$$

since $k \in (0, \frac{1}{2})$. Thus expected turnout for a party’s majority district is increasing in k.

Expected total turnout in a party’s minority district is

$$\begin{aligned} \frac{1}{2}k\sigma _1^{2*} = \frac{1}{2}\left( \frac{w}{2}\right) ^{\frac{1}{2}}\frac{k^{\frac{3}{4}}}{(1-k)^{\frac{1}{4}}}. \end{aligned}$$

Its derivative is

$$\begin{aligned} \frac{1}{2}\left( \frac{w}{2}\right) ^{\frac{1}{2}}\frac{\frac{3}{4}\bigg (\frac{1-k)}{k}\bigg )^{\frac{1}{4}}+\frac{1}{4}\bigg (\frac{k}{1-k}\bigg )^{\frac{3}{4}}}{(1-k)^{\frac{1}{2}}} > 0, \end{aligned}$$

and thus expected turnout for the minority party is increasing in k.

1.3 Proposition 3

Expected total turnout for each party is increasing in k for the majority and minority in the partisan districts. Thus, the total expected turnout in a partisan district is also increasing in k. Both parties’ expected turnout is decreasing in k for the battleground district, so expected total turnout is also decreasing in k.

To see that the expected total turnout in the partisan districts converges to that of the battleground district, consider:

$$\begin{aligned} \lim _{k\rightarrow \frac{1}{2}} \frac{1}{2}\frac{1}{2}\sigma _1^{3*} + \frac{1}{2}\frac{1}{2}\sigma _2^{3*} = \lim _{k\rightarrow \frac{1}{2}} \frac{1}{2}\sqrt{w}\Bigg (\frac{k}{1-k} - 2\bigg (\frac{k}{1-k}\bigg )^{\frac{1}{2}}+2\Bigg )^{\frac{1}{2}} = \frac{1}{2}\sqrt{w} \end{aligned}$$

and

$$\begin{aligned} \lim _{k \rightarrow \frac{1}{2}} \frac{1}{2}(1-k)\sigma _1^{1*} + \frac{1}{2}k\sigma _2^{1*}= & {} \lim _{k\rightarrow \frac{1}{2}} \frac{1}{2}(1-k)\sqrt{w}\frac{k^{\frac{1}{4}}}{(4(1-k)^3)^{\frac{1}{4}}} \nonumber \\&+ \frac{1}{2}k\sqrt{w}\frac{1}{(4(k(1-k))^{\frac{1}{4}}} \nonumber \\= & {} \frac{1}{4}\sqrt{w}+\frac{1}{4}\sqrt{w}= \frac{1}{2}\sqrt{w}. \end{aligned}$$

1.4 Proposition 4

The Proposition states that as k approaches $\frac{1}{2}$, the probability of winning a party’s majority district decreases, the probability of the party winning its minority district increases, and the probability a party wins the battleground district stays constant at $\frac{1}{2}$.

To see this, consider the probability of party 1 winning its majority district:

$$\begin{aligned} F\Bigg (\frac{(1-k)\sigma _1^{1*}}{k\sigma _2^{1*}}\Bigg ) = 1 - \frac{k\sigma _2^{1*}}{2(1-k)\sigma _1^{1*}} = 1 - \frac{1}{2}\Bigg (\frac{k}{1-k}\Bigg )^{\frac{1}{2}}. \end{aligned}$$

Notice that this quantity approaches $\frac{1}{2}$ as $k \rightarrow \frac{1}{2}$. Taking the derivative with respect to k gives

$$\begin{aligned} \frac{\partial F\left( \frac{(1-k)\sigma _1^{1*}}{k\sigma _2^{1*}}\right) }{\partial k} = -\frac{1}{4}\frac{1}{k^{\frac{1}{2}}{(1-k)^{\frac{3}{2}}}}, \end{aligned}$$

which is negative for $k \in (1, \frac{1}{2})$. Thus the probability that a party wins their majority district is decreasing in k.

The probability of party 1 winning district 2 is

$$\begin{aligned} F\left( \frac{(1-k)\sigma _2^{2*}}{k\sigma _1^{2*}}\right) = \frac{k\sigma _1^{2*}}{2(1-k)\sigma _2^{2*}} = \frac{1}{2}\Bigg (\frac{k}{1-k}\Bigg )^{\frac{1}{2}}. \end{aligned}$$

This quantity is increasing in k by the same argument that the previous result is decreasing in k.

Finally, the probability that candidate 1 wins the battleground district is

$$\begin{aligned} F\left( \frac{\frac{1}{2}\sigma _1^{3*}}{\frac{1}{2}\sigma _2^{3*}}\right) = F(1) = \frac{1}{2}. \end{aligned}$$