Optical characterization and dispersion discussions of the novel thermally evaporated thin a-S_50-xGe₁₀Cd_xTe₄₀ films

Abstract

In this article, the authors have been interested in synthesizing and studying the novel amorphous thin S_50-xGe₁₀Cd_xTe₄₀ films (SGCT) (0 $\le$ x $\le$ 15 at.%) from their glassy bulk compositions. The authors have deposited thin films on pure, transparent, and well-pre-cleaned glass substrates using the thermal evaporation route under a vacuum $\approx {10}^{-4}$ Pa. Both the deposition rate and film thickness are fixed during depositing SGCT-films at 10 nm/s and 750 nm, respectively. Film samples have been characterized via X-ray diffraction, energy-dispersive X-ray spectroscopy, and optical spectrophotometric measurements for reflection and transmission in the 500–2500 nm spectral range. Based on Minkov's envelope method, the reflection spectra are employed to get the thickness, d, and refractive index, n, of films. The n-values have been found to increase from 2.755 to 3.300 as the Cd-percentage increases. The single effective oscillator model is used to get dispersion energies and parameters. The dispersion energy increases from 16.11 to 16.73 eV, while the single effective oscillator energy decreases from 3.23 eV to 2.65 eV. Tauc’s and Urbach’s energies (E_g and E_e, respectively) have been determined where Eg decreased from 1.60 to 1.31 (eV), while Ee increased from 0.0276 to 0.0644 (eV) as the Cd content was increased.

1 Introduction

During the past few years, there has been considerable and increasing concern about the properties of non-crystalline chalcogenide solid materials. This interest still exists and is still growing very rapidly because of the distinctive and distinguished properties of these materials. Where the optical, electrical, and thermal properties of these chalcogenide glasses, ChG can be controlled, tailored, and customized according to the necessary usage or application [1,2,3]. These materials have several unique advantages that excel over other materials, such as high packing density, mass-replication, fast data rate, and higher signals with respect to noise, as well as their highest immunity to imperfections or disorders [1,2,3,4]. Moreover, the semiconducting chalcogenide glassy materials are transparent in the infrared region, and the band gaps have variable values depending on the constituent elements, methods of preparation, and the applied preparative conditions. As a result, these ChG have a wide range of applications and are found in various optoelectronic devices [3,4,5,6].

Moreover, to employ these ChG materials in optical fibers and microscopic lenses, glass materials must show their ability to form high glass, thermal stability, and high windows, as well as their rheological and mechanical characteristics should be good. It is observed that the mixed chalcogenide systems, such as S-Ge-Te or/and Se-Ge-Te, have wide glass-forming regions [7, 8]. Furthermore, the addition of a cadmium component may create more configuration disorders and defect states within the forbidden gap of the host lattice of the Ge–S–Te glasses, and therefore, affect their physical, optical, and electrical characteristics [8,9,10].

Moreover, the addition of Cd-element to the host lattice of Ge–S-Te System leads to improving the optical, electrical and optoelectrical properties of the produced composition and their thin films. The formed new compositions will combine many properties of the binary and ternary systems of CdS, CdTe, CdSTe, Cd-Ge-Te, Cd-S-Ge which are characterized by their high absorption coefficient in the visible, NIR, and IR optical regions [9,10,11,12]. Along with this, adding the Cd to the S-Ge-Te System will decrease the optical bandgap width [11,12,13,14]. Thus, adding Cd can help in tuning and controlling the optical gap energy via Changing the compositional elements and the atomic fractions of consistent elements [12,13,14,15]. Thus, these glassy materials have been developed to be used in far-infrared sensors, FIR optical fibers, and to be useful in sensing CO₂ gas or constructing IR-telescopes for detecting biological life outside of the planet Earth and on exoplanets [6,7,8,9].

In addition, the present chalcogenide semiconducting thin-film samples of the S_50-xGe₁₀Cd_xTe₄₀ glassy systems can be used in many technological applications and modern industries such as photo-electrochemical solar cells, photoconductive and photovoltaic cells, NIR-photodetectors, microelectronics and electronics industries, power generation, communications, photoelectricity, optoelectronic devices, as well as catalytic materials. and many others [12,13,14,15].

On the other hand, most of the thin-film physical properties depend on the chemical compositional elements that constitute the film material, the preparation method, and the thickness of the films [9, 16]. The behavior of thin films is also specified by their microstructure, morphological features, chemical composition, purity degree of elements, and homogeneity of constituents [17,18,19,20].

The samples' preparation of chalcogenide thin films can be achieved in many ways, whether physically or chemically, such as magnetron sputtering, spin coating, electron beam evaporation, thermal deposition, atomic layer deposition, chemical vapor deposition, pulsed laser deposition, and many others [19,20,21]. It is difficult to prepare chalcogenide films with complex chemical compositions (ternary, quaternary, etc.) and to have good stoichiometry, a uniform and homogeneous thickness, are well adhered to the substrate, and have good optical properties [17,18,19,20]. So, the selection of the preparation method is necessary. Therefore, the authors studied the previous method and chose the thermal evaporation technique to prepare the thin films of the S_50-xGe₁₀Cd_xTe₄₀, SGCT chalcogenides. The thermal evaporation method is one of the physical vapor deposition (PVD) methods, as it is one of the simplest methods. In this method, a resistive heat source (Molybdenum boat or a resistance-heated quartz crucible) evaporates the bulk sample in a vacuum to get the desired thin-film sample [21,22,23,24]. The compositional material is heated in a high vacuum jar until vapor pressure is obtained. The advantage of this method is that the distance of the source from the substrate, the deposition rate, film thickness, and its homogeneity can be controlled, and several film samples can be deposited in one run for use in several different measurements [24, 25].

On the other hand, film thicknesses and optical constants viz. refractive index (n), extinction coefficient (k), absorption coefficient (α), optical gap energy (E_g), and many other optical parameters may be estimated based on transmittance (T) and reflectance (R) or absorbance (A) spectrophotometric measurements [26, 27]. There are several methods that can be applied to calculate these parameters, according to the form of T and R-spectra [28,29,30]. Generally, these methods are relatively simple but depend on the skill of using the software programs [21,22,23,24,25,26,27,28,29,30,31,32]. There is the Minkov Envelope Method, MEM, which is based on optical reflectance, R-spectra [21, 27,28,29] and the Swanepoel Envelope Method, SEM, which is based on optical transmittance, T-spectra [22, 30,31,32,33] at normal incidence. It has been applied to thin films that have been deposited on transparent substrates. Noting that these methods do not need to know the thickness of the thin films, it is also possible to determine the film thickness using these envelop methods very easily. The authors had used these methods successfully before and were able to study the optical properties and dispersion parameters [32,33,34].

The aim of this article is to prepare homogeneous amorphous S_50-xGe₁₀Cd_xTe₄₀ thin-film samples having a uniform thickness. Then comes the study of the optical and dispersion parameters of SGCT films. The authors will use the envelope method, suggested by Minkov and Ruiz-Ruíz-Pérez et al. [27, 28] for the reflection spectra to estimate the thickness, d, the refractive index, n, and the absorbance, x, values of the films. The authors will also study the electronic polarizability of these films. The authors will characterize their samples by using X-ray diffraction (XRD), energy dispersive X-ray analysis (EDX), and VIS–NIR-spectroscopic measurements. Table 1 reports the most important parameters of the elements of the present studied glassy S_50-xGe₁₀Cd_xTe₄₀ system and will be used to deduce the parameters that will be investigated [35,36,37,38,39].

Table 1 The elements physical parameters of the present glassy S_50-xGe₁₀Cd_xTe₄₀ system [35,36,37,38,39]

2 Experimental procedures

2.1 Materials and preparation of samples

Six glassy alloys of the system S_50-xGe₁₀Cd_xTe₄₀, SGCT (where x = 0.0, 3.0, 6.0, 9.0, 12.0, and 15.0 at. %) have been synthesized using the powder of elements of S, Ge, Cd, and Te. These elemental powders have a high purity degree, which reaches 5 N, and have been purchased from Sigma Aldrich Chemicals Company. Bulk glassy samples of these S_50-xGe₁₀Cd_xTe₄₀ compositions were prepared using the melt-quenching route of the mixture of their elements. Where the four elements were weighed according to their atomic weight percentages and then placed in quartz ampoules. These ampoules were tightly sealed after being well evacuated under a pressure of about 1.2 × 10^–3 Pa. The ampoules containing the mixture of the four components (S, Ge, Cd, and Te) were placed in a vibrating electric oven. The oven temperature was gradually increased from room temperature to a temperature of 1000 °C, and this was done in two stages.

The first stage started from room temperature up to 600 °C, where the heating rate was 3 °C/min, with the temperature degree of the vibrating electric furnace fixed for about 30 min when reaching every hundred degrees. It should be noted that the furnace with the ampoules was periodically rocked to ensure the homogeneity of the contents of the ampoules. While the second stage started at 600 °C and continue to 1000 °C, where the heating rate was lower (2 °C/min), with the continuous shaking of the oven. Noting that the oven temperature was fixed for about 60 min when the temperature was 700 °C, then 800 °C, and then 900 °C. After the temperature reached 1000 °C, the oven temperature was stabilized at this temperature (1000 °C) for about 12 h with continuous shaking of the oven, including the ampoules, to ensure the homogeneity of the contents of the ampoules. The ampoules were then placed into an ice-water bath. The glassy alloys extracted from each ampoule were used in the preparation of SGCT in the form of thin films.

The thermal evaporation method was used to deposit the thin films on microscopic glass substrates, which were well pre-cleaned before. The glass substrates were cleaned with detergent and a mixture of acids, then washed and rinsed thoroughly with alcohol, then double-distilled water, and then dried by ultrasound [40, 41]. The used coating unit was Denton Vacuum 502-A, which was evacuated to a pressure of about 10⁻⁴ Pa. It is worth mentioning that during the film depositing process on the glass substrate, the substrates were periodically rotated to get film samples with a homogenous and uniform thickness. Both the rate of deposition of films and the thicknesses were controlled to be constant at 10 nm/s and 750 nm, respectively. The used thickness controller was a Denton quartz crystal monitor, model DTM-100. The as-deposited films were kept for 24 h within the evaporation jar in the dark to reach the metastable thermodynamic equilibrium state, as reported by Abkowitz [42]. After preparing the thin films, the thickness of the films was checked by utilizing the stylus mechanical method (SMM) using a profilometer of Sloan-Dektak-II [12]. The error in thickness measurement was within ± 6 nm. The Archimedes principle has been used to set the value of volumetric mass-density for the prepared samples. The toluene liquid was used as a non-solvent immersion liquid. The density of each sample has been measured several times and the mean values have been taken for each sample. The details of the experimental density measurements were published in previous works [43, 44]. The density measurements have been carried out at room temperature. The error in the density measurements is within $\pm$ 0.8%.

2.2 Used characterization tools

The prepared S_50-xGe₁₀Cd_xTe₄₀ films were checked by X-ray diffraction employing a Philips X-ray diffractometer, Model (1710), to know the crystallinity/non-crystallinity state of the prepared samples. The source used was k_α-Cu and the wavelength was 1.54184 nm. The diffractometer was operated at an energy of 8.042 keV. The percentages of constituent elements in each sample were also checked using the energy-dispersive X-ray spectroscopic analysis employing the Link analytical EDX-technique. It was found that the error in detecting the percentage of elements in each sample was less than 1%. The optical properties of the present S-Ge-Cd-Te thin films were investigated via the Shimadzu computer-controlled double beam spectrophotometer of the model (2101 UV–Vis–NIR) in the spectral range 500–2500 nm, where the transmission, T, and reflection, R, of S_50-xGe₁₀Cd_xTe₄₀ film samples were measured in this range. The envelope method was applied to the reflection spectra of SGCT-films in that range. The two envelopes of the maximum reflection (R_M) and the minimum one (R_m) were determined for all reflections by applying the software of OriginPro (Origin-Lab Corp) version 2019b [22, 23].

3 Results and discussions

3.1 Verification of samples

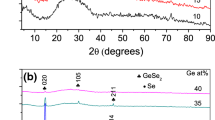
The crystal structural nature of the prepared S_50-xGe₁₀Cd_xTe₄₀ thin-film samples in terms of their crystallinity or non-crystallinity was verified using X-ray diffraction (XRD). The obtained diffraction patterns, as depicted in Fig. 1, show that they are completely free of any diffraction lines and the absence of any discrete or sharp peaks, which confirms that all prepared thin film samples have been formed in the amorphous phase. The diffractograms also showed that there is a wide hump that appears for almost all films in the position between diffraction angles 20° and 37°. The appearance of this apparent hump is attributed to the amorphous nature of the used glass substrates. This observation has been reported in many similar previous works [45, 46].

Fig. 1

X-ray diffractograms of the a-S_50-xGe₁₀Cd_xTe₄₀ chalcogenide films

Moreover, the atomic percentages of the compositional elements of the thermally deposited S-Ge-Cd-Te thin films have also been verified using the energy-dispersive X-ray spectroscopy (EDX) technique. The result of the EDX-scanning process showed that the percentages of the constituent elements of the samples correspond to a very close extent to the selected percentages, based on which the samples were prepared. Table 2 shows this convergence between the selected elemental percentages and those that were inferred by the EDX technique. It is worth noting here that the scanning process of films was carried out several times and at several different points along the length of the film, and the arithmetic mean of all the readings was taken.

Table 2 Percentages of constituent elements of a-S_50-xGe₁₀Cd_xTe₄₀ thin-film samples as detected by EDX technique and those that were selected to prepare these samples

3.2 Reflection and transmission spectra

The study of the reflection, R, and transmission, T, spectra of film samples play a crucial role in understanding the optical properties of the material as well as learning about its optoelectronic nature [18, 19]. The R-and T-spectra can be interpreted from the perspective of the interaction between the incident electromagnetic waves and the semiconductor film. The two figures, Figs. 2 and 3, illustrate the reflection, R, and transmission, T, spectra of the present film samples of the chalcogenide glassy S_50-xGe₁₀Cd_xTe₄₀ system. These two figures of a-S_50-xGe₁₀Cd_xTe₄₀ films show distinct interference fringes along the near-infrared (NIR) region. The relative intensity of R-spectra may reach about 36% for the first film sample (x = 0.0) and increase to reach about 46% for the film sample of the Cd-content (x = 15.0%). For the first sample with zero Cd content, the relative intensity of the transmission spectra is around 80%, and it slightly drops to 77% for the sample with 15 at.% Cd content.

Fig. 2

Reflection spectra of amorphous S_50-xGe₁₀Cd_xTe₄₀ thin films

Fig. 3

Transmission spectra of the quaternary amorphous thin S_50-xGe₁₀Cd_xTe₄₀ films

Moreover, it is found that the distinct interference fringes for the R-spectra almost exactly match what is found in the T-spectra, that is, the tops of R meet the bottoms of T and vice versa. Thus, in the NIR region, the sum of R and T is almost unity (R + T = 1), which indicates minimizing both the absorption and scattering of EM waves by the present S-Ge-Cd-Te films. While in the short-range order of the wavelengths in the visible region, anyone can observe that the sum of R and T is less than unity, where in this region, the absorption of EM waves is considerable and should be taken into consideration. This is due to electron capture owing to the reaction between the photons and electron polarization that has a resonance effect between the incident photons and the electron polarization in the oscillating electric field. This is what makes the propagation of electromagnetic waves in the visible region somewhat slower than their speed through the near-infrared region [20, 37, 47].

Furthermore, from Fig. 3 of the transmission spectra, it can be observed that the absorption edge of the T-spectra is redshifted, i.e., towards the higher wavelengths. This is owing to the effect of Cd-doping, which may cause some perturbations, disturbances, and disorder. This, in turn, leads to the generation of localized states or a band tail in the forbidden gap. Thus, the shrinkage of the bandgap width leads to this redshift of the absorption edge [26, 48]. This will be discussed in detail when studying the absorption coefficient of the current SGCT films and finding the optical energy gap values. Many similar results have been reported in the literature for chalcogenide thin films [48,49,50].

3.3 Determination of film thickness and refractive index

The R(λ) spectra of prepared S-Ge-Cd-Te thin-film samples show interference fringes, as shown in Fig. 2 and for more details in Fig. 4, as a typical sample. Thus, if the R(λ) -spectra have been identified by two limits (two of which are the interference maxima (R_M) and the interference minima (R_m)), then they were enveloped to apply the Minkov Envelope Method, MEM, as illustrated in Fig. 4 [27, 28]. Since the tangential positions of both envelopes of the reflection spectra (R_M(λ) and R_m(λ)) and those of the index of refraction of the glass substrate (n_s) are well-known, then the Newton–Rafson method [23] can be considered as:

$${{R}_{M}} \left( \lambda \right)= {{R}_{M}} \left( n,x \right) {and} {{R}_{m}} \left( \lambda \right)= {{R}_{m}} \left( n,x \right)$$

(1)

where ${R}_{M}\left(\lambda \right)$ and ${R}_{M}\left(n,x\right)$ are the interference maxima of the reflection spectra and refractive index (n), while their interference minima are ${R}_{m}\left(\lambda \right)$ and ${R}_{m}\left(n,x\right)$, respectively. Noting that the ${R}_{M}\left(\lambda \right)$ and ${R}_{m}\left(\lambda \right)$ of the reflection spectra have the following Eqs. [23]:

Fig. 4

Reflection spectra of the a-S₅₀Ge₁₀Cd₀Te₄₀ film, as a typical example, where R_x is the film sample reflection, and R_s is the substrate reflection

$${R}_{M},{R}_{m }= \frac{{\left(ah\pm bcx\right)}^{2}}{{\left(bh\pm acx\right)}^{2}}+ \frac{g {x}^{2}}{{\left(bh\pm acx\right)}^{2}\left({b}^{2}f\pm 2abchx+{a}^{3}{ex}^{2}\right)}$$

(2)

Here, ($\pm )$ refers to the upper and lower envelopes, respectively, i.e., (+ 1) for the upper one and (−1) for the lower one. Therefore, the system of these transcendental Eqs. (1) can be numerically solved and the numerical values of both (n) and (x) can be obtained. For each interference extreme, the solution of Eq. (1) supplies an initial approximation for the refractive index (n₁), and absorbance (x₁), for the present thin S-Ge-Cd-Te films, as reported in Table 2. It is worthy of note that it is substantial to consider the main interference fringes Eq that is given as: 2nd = mλ [23, 24], whereas (m) is equal to an integer for each minimum and a half-integer for each maximum of the reflection spectra, R(λ)-spectra. Also, (λ) are the tangential spectral positions of the upper and lower envelopes for the R(λ)-spectra.

Now, suppose that ${n}_{1(2)}^{{^{\prime}}{^{\prime}}}$ is an index of refraction belonging to the set of${n}_{1}$, and ${n}_{1(2)}^{^{\prime}}$ is another index of refraction belonging to the set of ${n}_{2}$, where n₁ and n₂ are the values of two successive and adjacent fringes (either maxima or minima) at the two wavelengths ${\lambda }^{^{\prime}}$ and ${\lambda }^{{^{\prime}}{^{\prime}}}.$ Thus, the first approximated value of the thickness ${d}^{^{\prime}}$ can be expressed as follows [23]:

$$d_1 = \frac{{\lambda ^{\prime}\lambda ^{\prime \prime}}}{{n^{\prime \prime}\lambda ^{\prime} - n^{\prime}\lambda ^{\prime \prime}}}$$

(3)

Therefore, the average approximated value of the thickness is the sum of all calculated values by using Eq. (3) of (${d}_{1}$) divided by their numbers, i.e., (${d}_{1}=\overline{{d }_{1}}).$ Then, by substituting the values of (${d}_{1}$) and (${n}_{1}$) into the Eq.: 2nd = m λ to get the order number (${\mathrm{m}}_{1}$) for each tangent position. It is worthy to note that the film thickness accuracy can be increased by considering the exact integer- or half-integer values of the calculated values of (m) of each tangential extreme position (λ), and then re-compute the new film thickness value (d₂) using the interference fringes Eq., 2nd = m λ, again, by using the value of n₁. Subsequently, the film thickness (d) can be obtained as the average value of all computed thicknesses for each sample. This is what is shown in Fig. 2, for all S_50-xGe₁₀Cd_xTe₄₀ film samples, and for more clarity, in Fig. 4 of the first sample (S₅₀Ge₁₀Te₄₀) to the possibility of following up the form of interference fingers as well as the envelopes of the ${R}_{M}\left(\lambda \right), {R}_{m}\left(\lambda \right)$ and R_s.

The estimated values of both λ, R_M, R_m, n₁, d₁, m_o, m, d₂, and n₂ of all used reflectance spectra of the amorphous ${{\mathrm{S}}_{50-\mathrm{x}}\mathrm{Ge}}_{10}{\mathrm{Cd}}_{\mathrm{x}}{\mathrm{Te}}_{40} (0.0\le \mathrm{x }\le 15.0\mathrm{ at}.\mathrm{\%})$ films have been listed in Table 3. These values have been deduced from the reflectance interference fringes of Fig. 2. Therefore, Eq. (1) can be dissolved for (n) at each wavelength (λ) and thus, the refractive index, n₂ of each film sample can be obtained [23, 24]. Moreover, the representation of (I/2) against (n/λ), as illustrated in Fig. 5 for the present amorphous S_50-xGe₁₀Cd_xTe₄₀ thin films, to determine the film thickness and the first-order value m₁ for all deposited films. Then, using Cauchy dispersion Eq., which is given as n(λ) = a + b/λ², it can get the two constants (a and b) via using two derived values of each (n₂) and (λ). Therefore, it can extrapolate the refractive index spectral dependence along the studied spectral range (500–2500 nm), as represented in Fig. 6. This figure shows that the refractive index, n, dramatically reduces with the wavelength increment up to 1250 nm. Then, the value of refractive indices tends to almost become constant for each film sample, after any further increment of the wavelength.

Table 3 The inferred values of some optical parameters of ${{\mathrm{S}}_{50-\mathrm{x}}\mathrm{Ge}}_{10}{\mathrm{Cd}}_{\mathrm{x}}{\mathrm{Te}}_{40} (0.0\le \mathrm{x }\le 15.0\mathrm{ at}.\mathrm{\%})$ films applying Minkov model on the reflectance spectra of Fig. 2

Fig. 5

Representation of (I/2) Versus (n/λ) for the a-S_50-xGe₁₀Cd_xTe₄₀ films

Fig. 6

Spectral behavior of the refractive index of thin a-S_50-xGe₁₀Cd_xTe₄₀ films

Furthermore, Fig. 6 shows that the refractive index increases as the Cd-content increases. At the middle of the visible region, at about 560 nm, the n-value increases from 3.233 to 4.708, while at the end of the visible region, increases from 2.755 to 3.300. Along with the NIR-region, the n-value increases from 2.582 to 2.942 as Cd-content increases from zero to 15.0%. Furthermore, the increased values of (n) can be attributed to the enlarged values of the polarizability that are related to the Cd-element [49], where the Cd atom has a larger size than that of S (This subject will be studied in detail in the next topics). Similar results have been observed in the literature for Ge-chalcogenide thin films [24, 44, 51].

It should be noted that the refractive index (n₂) values computed from the reflection interference fringes have been represented as black solid dots of larger sizes (see Fig. 6), while the other n-values deduced from Cauchy-dispersion Eq. have been represented as colored dots (except in the first sample, where the n-values have been represented as black hemisphere points). This increases the values of (n). The increased values of (n) are a good match with the redshift of the absorption edge. Similar reported findings have been observed for other chalcogenide thin films [33, 46].

3.4 Electronic polarizability of films

The electronic polarizability (${\alpha }_{el}$) of ions of optical materials is one of the most important optical properties that must be known to have the possibility of employing this material in one of the appropriate applications. Polarizability is closely related to applicability in the fields of optics and electronics. It has been found that electronic polarizability is one of the main reasons behind the nonlinear optical properties of materials when exposed to the falling of electromagnetic waves of considerable intensity. Therefore, the nonlinear responses of materials are governed by their polarizability, ${\alpha }_{el}$. Therefore, it is necessary to design and manufacture efficient nonlinear optical materials based on the interrelationships among nonlinear optical, optoelectrical, and electronic properties that should be easily understandable and accessible. The volumetric-mass density (mass density), ρ_exp and molar volume, V_m of the optical material also play a main role in studying electronic polarization, and hence, these two parameters will be studied first.

3.4.1 Density and molar volume

The study of density explores the changes in the structural features of amorphous materials. It has a significant impact on structural softening, geometrical configuration changes, and interstitial space dimensions in glassy materials [43, 52]. The density of the present glassy samples has been experimentally measured using the Archimedes Principle, employing toluene as a non-solvent immersion liquid. The experimental density values, ρ_exp of S_50-xGe₁₀Cd_xTe₄₀ samples have been determined using Archimedes' principal Eq. that is given as [20, 43]:

$${\rho }_{exp}= {\rho }_{tn}\left( {m}_{air} \left/ \left({{m}_{tn}}- {{m}_{air}}\right)\right.\right)$$

Here, ${\rho }_{tn}$ is the toluene density, ${m}_{air}$ and ${m}_{tn}$ are the mass of the sample in air and in toluene, respectively. The density of each sample was measured three times at room temperature, and the average value was considered. The calculated mean values of ${\rho }_{exp}$ for S_50-xGe₁₀Cd_xTe₄₀ samples have been tabulated in Table 4. It is observed that the ${\rho }_{exp}$ values increase from 3.986 g/cm³ to 4.718 g/cm³ with increasing Cd-content from zero to 15.0 at.%.

Table 4 Estimated values of optical and physical parameters of a-${{\mathrm{S}}_{50-\mathrm{x}}\mathrm{Ge}}_{10}{{\mathrm{Cd}}_{\mathrm{x}}\mathrm{Te}}_{40}$ samples

The obtained values of ${\rho }_{exp}$ have been represented graphically in Fig. 7a and fitted linearly to get the following empirical relationship: ${\rho }_{exp}$ (gm/cm³) = 3.886 + 0.03 x. The increasing values of ${\rho }_{exp}$ are attributed to the density of the Cd-element (8.650 gm/cm³), which is larger than that of the S-element (1.960 gm/cm³) and is larger than the other elemental components of the present samples (see Table 1). It is worthy of mention that the error in measuring the density is less than $\pm 1.0\%.$

Fig. 7

Dependence of the a Density, b Molar volume of chalcogenide thin S_50-xGe₁₀Cd_xTe₄₀ films upon the cd-content percent

Furthermore, the molar volume, V_m can be obtained from this Eq. [20, 43]: ${V}_{m} = A.W./{\rho }_{exp}$, where A.W. is the atomic weight of the sample. The values of V_m have been computed and tabulated in Table 4 and are graphically illustrated in Fig. 7b. The graph between V_m and Cd-content was linearly fitted to get this experimental Eq.: V_m (cm³/mol) = 19.144 + 0.053x. It has been seen that although the relationship between V_m and ρ_exp is an inverse relationship, the V_m values increase with an increase in the Cd-percentage. This can be attributed to the cadmium element, which has the largest density, but its molar volume is the smallest one (12.995 cm³/mol) compared to the rest of the sample elements, and its fractional percentage is also small (Please refer to Table 1). This result has been reported in many published literature [20, 43]. Depending on the measured values of density, the experimental error of V_m was about 0.7%. Thus, the precision of the determined values of the molar volume, V_m was better than 99.3%.

3.4.2 The average molar refraction and reflection loss

Duffy, Dimitrov, and Sakka have observed a strong relationship between electrical polarization and many linear optical quantities, especially the index of refraction of material (n) [54, 55]. These scholars were able to suggest an approach that gives a relationship between the covalent/ionic nature of the material and the optical parameters. For the non-crystalline materials (isotropic ones), Lorentz–Lorenz also formulated an expression that gives the relationship between the refractive index (n), the density (ρ), the molar volume (V_m), and the average molar refraction (R_m) [64, 67]. This formula is given as [53,54,55]:

$${R}_{m}= \frac{\left({n}^{2}-1\right)}{\left({n}^{2}+2\right)}\times \frac{{M}_{m}}{\rho }= \frac{\left({n}^{2}-1\right)}{\left({n}^{2}+2\right)}\times {V}_{m}$$

(4)

Here, ${M}_{m}$ and ρ are the molecular weight and the density of the film sample, while the refractive index term $\left({n}^{2}-1\right)/\left({n}^{2}+2\right)$ is called the reflection loss (RL) of the present studied film sample, thus RL = $\left({n}^{2}-1\right)/\left({n}^{2}+2\right)$. The value of RL of the S_50-xGe₁₀Cd_xTe₄₀ amorphous film samples has been calculated, tabulated in Table 4, and then represented as a function of Cd-content in Fig. 8-a.

Fig. 8

Dependence of the a Reflection loss, RL, and b average molar refraction, R_m of amorphous thin S_50-xGe₁₀Cd_xTe₄₀ films upon the cd-content percent

The RL value increases from 0.654 to 0.719 as the Cd content increases from zero to 15%. The linear relationship that describes this increase of RL values as a function of the Cd-content percentage (x) is empirically given as: RL = 0.656 + 0.004x. The average molar refraction (R_m) values have been computed, listed in Table 4, and plotted as a function of Cd content as shown in Fig. 8-b. Furthermore, it was noted that the R_m values have increased from 12.197 cm³/mol to 13.165 cm³/mol as Cd-percentage (x) increases from zero to 15%. The linear empirical relationship that describes this increase is given as: R_m = 12.187 + 0.09x.

3.4.3 Determination of the electronic polarizability, ${\boldsymbol{\alpha }}_{{\varvec{e}}{\varvec{l}}}$

The extent of electronic responses to electromagnetic field applications in electronic clouds is defined as electronic polarizability (${\alpha }_{el}$) of this material. The electronic polarizability (measured in Ǻ³) of the material is correlated to the value of the molar refraction (R_m), via the Clausius-Mosotti relationship. Further, the electronic polarizability is proportional to the R_m values, which correlates to the sample's non-crystalline nature. The electronic polarizability of chalcogenide S_50-xGe₁₀Cd_xTe₄₀ amorphous films may be estimated using the Lorentz-Lorenz relationship and the Clausius-Mosotti local-field polarizability model as follows [53,54,55,56]:

$${\alpha }_{el }= \frac{3{R}_{m}}{4\pi {N}_{m}}=\frac{3}{4\pi {N}_{m}}\times \frac{\left({n}^{2}-1\right)}{\left({n}^{2}+2\right)}\times {V}_{m}$$

(5)

where ${N}_{m}$ is the number of atoms or molecules per volume. Thus, by using the refractive index spectra, the spectra of ${\alpha }_{el}$ for the amorphous S_50-xGe₁₀Cd_xTe₄₀ film samples can be obtained and then graphically represented and depicted in Fig. 9. It is observed that the spectral behavior of ${\alpha }_{el}$ is almost like that of the refractive index (n), where ${\alpha }_{el}$ is directly proportional to both (n) and (RL). Thus, if a graph is plotted between ${\alpha }_{el}$ and n, the result will be a straight line [57, 58]. This result revealed the amorphous state of the present thin films, which is consistent with what is supposed by Zemel et al. [57].

Fig. 9

The electronic polarizability spectral dependence, ${\mathrm{\alpha }}_{\mathrm{el}}$ of thin a-S_50-xGe₁₀Cd_xTe₄₀ films

Moreover, the values of ${\alpha }_{el}$ for film samples have been determined and plotted as a function of the Cd-content % (x) in Fig. 10a. This figure shows that the value of ${\alpha }_{el}$ increases from 4.834 Ǻ³ to become 5.218 Ǻ³ as the Cd-concentration percentage increases according to this empirical Eq.: ${\alpha }_{el}$(Ǻ³) = 4.840 + 0.03 x, where (x) is the molecular fraction of the cadmium. This increase in ${\alpha }_{el}$ values is due to the increasing values of both the refractive index, n, reflection loss, RL, and average molar refraction, R_m.

Fig. 10

Dependence of the a Electronic polarizability, ${\mathrm{\alpha }}_{\mathrm{el}}$ and b Metallization parameter, M_e of the a-S_50-xGe₁₀Cd_xTe₄₀ thin films upon the cd-content %

3.4.4 Metallization parameter, ${{\varvec{M}}}_{{\varvec{e}}}$

The metallization of the covalency parameter, ${M}_{e}$ describes the physical behavior of the material (metal or non-metal). If the value of ${M}_{e}$ is greater than unity, then the sample will have a metallic behavior, but if the value is less than unity, the sample will behave as an insulating material. It is not possible for the value of ${M}_{e}$ to equal one at all, as ${M}_{e}$ approaches zero, the transition to the metallic nature occurs. Thereby, the value of this parameter can be calculated from this simple form [55, 59]:${M}_{e}=1-\left({R}_{m} \left/ {V}_{m}\right.\right)$, and by simplifying using Eq. (4), ${M}_{e}$ can be rewritten as:${M}_{e}= 3/\left({n}^{2}+2\right)$. Thus, the M-values of the present films can be determined (see Table 4 and Fig. 10b, A straight-line equation in the form of ${M}_{e}$ = 0.346 – 0.004 x, which was fitted to the relationship between ${M}_{e}$ and the ratio of Cd-presence (x). As it is obvious, the M-values decrease from 0.346 to 0.284 as Cd-content increases from zero to 15.0 at. %, i.e., all ${M}_{e}$-values are less than one. Therefore, these film samples are non-metals, but they have an amorphous nature, as suggested by Duffy, Dimitrov, and Sakka [54, 55]. In addition, these studied film samples are polarizable cations, where their M-values range between 0.346 and 0.284 [55,56,57,58]. Along with, and as suggested by Dimitrov, Sakka, and Duffy, the low value ${M}_{e}$ confirms that these S_50-xGe₁₀Cd_xTe₄₀films have lower energy band-gap values. This result affirms the obtained electronic polarizability results [55,56,57,58,59,60]. Similar results have been reported in the literature for the chalcogenide systems [61, 62].

3.5 Dispersion studies

The dispersion parameters, including the dispersion energies, are considered the essential parameters that affect and determine many optical properties of materials. Therefore, when designing communication devices and spectra dispersion systems, it is necessary to study and investigate these important parameters for the materials used. The dispersion energies are the most crucial quantities that should be studied on this topic, where many other parameters can be deduced with their knowledge [63].

3.5.1 The single effective oscillator model, SEOM

This model, SEOM, was the most effective model that could study and discuss the relationship between the material refractive index (n) and the dispersion energies, which are the dispersion energy, E_d, and the energy of the effective oscillator, E_o. Wemple-Di-Domenico (WDD) were able to formulate the equation of this SEOM-model, so this model is sometimes called the WDD-model. This model also links these two energy parameters and the incident energy of photons (hν) through the following form [63, 64]:

$$\left({n}^{2}-1\right)=\frac{{E}_{o}\times {E}_{d}}{\left[{E}_{o}^{2}- {\left(h\nu \right)}^{2}\right]}$$

(6a)

Or

$${\left({n}^{2}-1\right)}^{-1}= \frac{{E}_{o}}{{E}_{d}}- \frac{{\left(h\nu \right)}^{2}}{{E}_{o}\times {E}_{d}}$$

(6b)

Therefore, if a graph is plotted between the refractive index term ${\left({n}^{2}-1\right)}^{-1}$ (on the ordinate) and the square of the photon energy ${\left(\mathrm{h\nu }\right)}^{2}$, on the abscissa, it will give a straight line that intersects the y-axis in the positive direction. This intersection gives the ratio between E_o and E_d, (i.e., the intersection = ${E}_{\mathrm{o}}/{E}_{\mathrm{d}}$), while the slope of this straight line is ${\left({E}_{\mathrm{o}}\times {E}_{\mathrm{d}}\right)}^{-1}$. The figure that shows the relationship between ${\left({n}^{2}-1\right)}^{-1}$ and ${\left(\mathrm{h\nu }\right)}^{2}$ of the a-S_50-xGe₁₀Cd_xTe₄₀ thin films is illustrated in Fig. 11. Thus, from the intersection parts and the slope of the straight lines, it could determine the dispersion and effective oscillator energies (E_d and E_o, respectively) and then other dispersion parameters. The estimated values of (E_d and E_o) have been computed, listed in Table 5, and then represented as functions of the Cd-content % in Fig. 12a and b. It can be seen that the values of the dispersion energy, E_d increase according to this experimental linear fitted formula: E_d (eV) = 16.109 + 0.042 x, while the values of the single effective oscillator energy, E_o, decrease according to this empirical linear fitted formula: E_o (eV) = 3.204−0.037 x, as the Cd-percentage (x) increases from zero to 15.0 at.%. Obviously, the obtained values of E_d are not large values ($\approx$ 16 eV), which means that the average strength of interband optical transitions is not large, and therefore, the changes in the structural order of the material are limited when adding the Cd-element [64,65,66].

Fig. 11

Variation of the refractive index factor (n²−1)⁻¹versus of (hν)² of a-S_50-xGe₁₀Cd_xTe₄₀ films

Table 5 Determined values of the optical dispersion energies and parameters of thin-film samples of a-S_50-xGe₁₀Cd_xTe₄₀ system

Fig. 12

Dependence of both the E_d, E_o, E_g and the ratio E_o/E_g upon the cd-ratio within the amorphous thin S_50-xGe₁₀Cd_xTe₄₀ films

But at the same time, these structural changes are slightly increased with the addition of more cadmium, where E_d increases from 16.11 eV to 16.73 eV. In particular, the localized states near the conduction and valence bands or the tail localized states have a strong effect on the electronic transition and the optical absorption (as will be discussed in the next topics) and thus will lead to the shrinkage of the optical band gap. Such tail localized states will lead to an increment in the band tail width and, in turn, increase the Urbach energy [63,64,65,66,67,68]. Further, the average bandgap energy, or what is commonly known as the single effective oscillator energy, E_o gives good quantitive information about the band structure of the material [64,65,66,67,68]. In the present study, as shown in Fig. 12b, E_o decreases from 3.23 eV to 2.65. This is fully consistent with the results of the E_d as the localized states lead to a shrinkage in the width of the optical gap [69, 70]. Wemple-Di-Domenico (WDD) has assumed that the optical bandgap energy, E_g is equal to half of the E_o value, i.e., ${E}_{o}\approx {2 E}_{g}$, which was verified for the present S-Ge-Cd-Te thin films in Fig. 12d [70]. The optical band gap values will be studied in detail in the future using the absorption spectra and Tauc’s plots.

On the other hand, by knowing the values of both E_o and E_d, many dispersion parameters can be obtained, such as the lattice dielectric constant (${\varepsilon }_{L})$, static refractive index (${n}_{o})$, and the moments (${M}_{-1}$ and ${M}_{-3})$. These dispersion parameters can be computed from the following Eqs. [64, 65, 70]:

$$\varepsilon_{L} = 1 + \frac{{E_{d} }}{{E_{o} }};\,n_{o} = \sqrt {1 + \frac{{E_{d} }}{{E_{o} }}} ;M_{( - 1)} = \frac{{E_{d} }}{{E_{o} }}\,and\,M_{( - 3)} = \frac{{M_{( - 1)} }}{{E_{o}^{2} }};$$

(7)

Therefore, these four dispersion parameters (${\varepsilon }_{L}$, n_o, and the moments ${M}_{-1}$ and ${M}_{-3}$) can be computed, listed in Table 5, and graphically plotted as functions of the Cd-content percentage in Fig. 13a–d, respectively. It can be observed that the values of these four parameters are increasing as the increment of the Cd-content percentage (x), according to the following empirical Eqs.:

$$\varepsilon_{L} \, = \,6.002\, + \,0.085 \, x; \, n_{o} \, = \,2.453\, + \,0.016 \, x;$$

$$M_{ - 1} \, = \,6.380\, + \,0.011 \, x;{\text{and}}\,{\mathbf{M}}_{{ - {\mathbf{3}}}} \, = \,0.613\, + \,0.020x.$$

Fig. 13

Representations of the ε_L, n_o, M_-1 and M_-3 values as functions of the cd-percentage of the amorphous S_50-xGe₁₀Cd_xTe₄₀ thin films

The increasing values of these four dispersion parameters can be attributed to the increasing values of E_d, and at the same time, the decreasing of E_o, where the four dispersion parameters have been derived from the ratio of (E_d/E_o). Moreover, E_d increases due to the increase in the percentage of cadmium, which leads to more disturbances or disorder in the forbidden band gap and therefore, increases the density of the localized states and in turn increases the band tail width [69, 70]. These results are consistent with the electronic transitions and the determinations of the optical energy gap (E_g) and the energy of the band tail width (E_e) using Tauc and Urbach Eqs., as will be discussed in the next sections. Furthermore, the obtained results are consistent with many similar semiconducting films [18, 33, 71, 72].

3.5.2 Charge carrier concentrations

The lattice dielectric constant (ε_L) of glassy materials, is related to the ionic, electronic, dipolar, and space charge polarization. The contribution of the space charge is dependent upon the quality and purity of the studied glassy samples. In general, the effect of the contribution of the space charges is extremely noteworthy in the high-wavelength (low-energy) region. The analysis of the accurate data of the refractive index can give good data about the lattice dielectric constant (ε_L) along with the ratio between the concentration of the charge carriers and the effective mass (N/m*). These data can be obtained from the study of the dispersion vibration modes and the contribution of free carriers, which is given by this dispersion Eq. [18, 57]:

$${n}^{2}={\varepsilon }_{L}-\left(\frac{{e}^{2}}{{{\pi c}^{2}\varepsilon }_{o}}\right){\left(\frac{N}{{m}^{*}}\right)\lambda }^{2}$$

(8)

where, (n) is the refractive index, (ε_L) is the lattice dielectric constant of studied samples, (e = 1.6 × 10^–19 C) is the electronic charge, (ε_o = 8.85 × 10^–12 F/m) is air permittivity, and (c = 3 × 10⁸ m/s) is the light speed in air. Thus, according to Eq. (8), the graphical representation of (n²) versus (λ²) should give a straight line that intersects the ordinate at a certain value equal to (ε_L). The slope of this line is $\left(\frac{{{\varvec{e}}}^{2}}{{{{\varvec{\pi}}{\varvec{c}}}^{2}{\varvec{\varepsilon}}}_{{\varvec{o}}}}\right)\left(\frac{N}{{{\varvec{m}}}^{\boldsymbol{*}}}\right)$, from which it is possible to obtain the value of the ratio $\left(\frac{N}{{{\varvec{m}}}^{\boldsymbol{*}}}\right)$ [57]. Thus, by applying these procedures to the present amorphous thin S_50-xGe₁₀Cd_xTe₄₀films, the authors have been able to obtain the illustrated figure, Fig. 14.

Fig. 14

The dependence of (n²) on (λ²) of the chalcogenide thin a-S_50-xGe₁₀Cd_xTe₄₀ films

As shown, the general behavior of this figure is almost like that of the refractive index, Fig. 6. The inset of this figure shows the linear portions of the spectra in the high-wavelength region. Consequently, the intersected part of the Y-axis by each straight line was set and the slope of each line has been calculated to estimate the values of each of (ε_L) and $\left(\frac{N}{{{\varvec{m}}}^{\boldsymbol{*}}}\right)$. Accordingly, these values were computed and then listed in Table 5. It is obvious that the values of (ε_L) increase from 6.002 to 7.277, according to the obtained Eq.: ${\varepsilon }_{L}$ = 6.002 + 0.085 x. The increase of (${\varepsilon }_{L}$)-values can be attributed to the Cd-addition that leads to an increment of the displacement current density (J) when the photons propagate along with the films because of the time-varying of the applied electric field [38]. Therefore, the lattice dielectric constant values increase. The obtained values from Fig. 14 are almost consistent with the obtained values from the equation (ε_L = 1 + ${(E}_{d}/{E}_{o}$)) of the dispersion energies. This indicates the accuracy of the accounts and the shown graphics. It should also be noted that the values of ε_L are much greater than that of the static refractive index [38, 63].

Further, the value of the ratio of the charge carrier concentration and the effective mass (N/m*) increases from 8.39 × 10⁵⁵ kg⁻¹ m⁻³ to 16.29 × 10⁵⁵ kg⁻¹ m⁻³. Figure 15a depicts the dependence of this ratio upon the Cd-percentage within the films of a-S_50-xGe₁₀Cd_xTe₄₀system and the relationship between them is given by this experimental Eq.: N/m*(kg−1 m⁻³) = (8.244 + 0.504 x) × 10⁵⁵.

Fig. 15

The dependence of (N/m*), (N), (λ_o), and (S_o) upon the Cd-percentage in the samples of the thin a-S_50-xGe₁₀Cd_xTe₄₀ films

Moreover, the value of the charge carrier concentrations can also be calculated using Q. Shen et al. suggestion [73]. They assumed that the relationship between the effective mass (m*) and the electron rest mass (m_o) is given as (m*/m_o = 0.44), therefore, m* = 4.008 × 10^–31 kg [72,73,74]. Therefore, the charge carrier concentrations (N) can be obtained, then tabulated in Table 5, and represented in Fig. 15b. The experimental Eq. that describes the link between this number (N) and the Cd-content % (x) is given as follows: N (m⁻³) = (3.305 + 0.202 x)) × 10²⁵. Obviously, the N-values increase from 3.363 × 10²⁵ (m⁻³) to 6.526 × 10²⁵ (m⁻³) as Cd-percent increases from zero to 15 at. %. These increased values of the charge carrier concentrations (N) agree completely with the increment of the displacement current density (J) when adding more Cd to the host lattice of the glassy Ge–S–Te system [70, 74].

3.5.3 Classical dispersion theory and Sellmeier’s parameters

The oscillator strength and oscillator wavelength (S_o, λ_o, respectively) are commonly called Sellmeier's parameters. These parameters can be evaluated by using the classical dispersion theory at high wavelengths (or low photon energies, $\mathrm{h\nu })$. Sellmeier has assumed that [75,76,77,78]:

$${\left({n}^{2}-1\right)}^{-1}=\frac{1}{\left({n}_{o}^{2}-1\right)}-\frac{1}{\left({n}_{o}^{2}-1\right){\lambda }^{2}} {{\lambda }^{2}}_{o}$$

(9)

Here, (n and n_o) are the ordinary and static refractive indices, respectively, while ($\uplambda$ and λ_o) are the wavelength of the incident photons and the oscillator wavelength, respectively. Equation (9) can be rearranged and substituted by λ = hc/hν, to get [76,77,78]:

$${\left({n}^{2}-1\right)}^{-1}=\frac{1}{\left({n}_{o}^{2}-1\right)}-\frac{{h\nu }^{2}}{\left({n}_{o}^{2}-1\right){hc}^{2}} {{\lambda }^{2}}_{o}$$

(10)

Referring to Eq. (6b):

$${\left({n}^{2}-1\right)}^{-1}= \frac{{E}_{o}}{{E}_{d}}- \frac{{\left(h\nu \right)}^{2}}{{E}_{o}\times {E}_{d}}$$

Thus, by a comparison between the last two Eqs., it can be concluded that:

$$\frac{1}{\left({n}_{o}^{2}-1\right)}= \frac{{E}_{o}}{{E}_{d}} \mathrm{ and } \frac{1}{\left({n}_{o}^{2}-1\right)} {{\lambda }^{2}}_{o} \frac{{\left(h\nu \right)}^{2}}{{\left(hc\right)}^{2}} = \frac{{\left(h\nu \right)}^{2}}{{E}_{d}{E}_{o}}$$

3.5.3.1 Hence: $\frac{1}{\left({n}_{o}^{2}-1\right)}= \frac{{E}_{o}}{{E}_{d} }\ \mathrm{and} \ \frac{1}{\left({n}_{o}^{2}-1\right)} \frac{{{\lambda }^{2}}_{o}}{{\left(hc\right)}^{2}} = \frac{1}{{E}_{d}{E}_{o}}$

Consequently [33, 34]:

$$E_{o} = ~\frac{{\left( {hc} \right)}}{{\lambda _{o} }}~~~~~~~~~\& ~~~~~~~~E_{d} = ~\left( {n_{o}^{2} - 1} \right)~\frac{{\left( {hc} \right)}}{{\lambda _{o} }}~~~~~~\& ~~~~~~S_{o} = ~\frac{{\left( {n_{o}^{2} - 1} \right)}}{{\lambda _{o} ^{2} }} = \frac{{E_{d} /E_{o} }}{{\lambda _{o} ^{2} }}$$

(11)

whereh = 6.626 × 10^–34J.S, which is Planck's constant, and c = 3 × 10⁸ m/s, which is the photon speed in air. Thus, by knowledge of the dispersion energy values $({\mathrm{E}}_{\mathrm{d}}{\mathrm{ and E}}_{\mathrm{o}})$, one can compute Sellmeier's parameters. The estimated ${\lambda }_{o}$ and ${\mathrm{S}}_{\mathrm{o}} \mathrm{values}$ are tabulated in Table 5, too. Further, the dependence on each ${\uplambda }_{\mathrm{o}}$ and ${\mathrm{S }}_{\mathrm{o}}$ upon the Cd-content % has been illustrated in Fig. 15c and d, respectively. The experimental equations, which describe the relationship between (${\uplambda }_{\mathrm{o}}$ and ${\mathrm{S }}_{\mathrm{o}}$) from one side, and Cd-content % (x) from the other side are given as follows:

$$\lambda_{0} \left( {nm} \right)\, = \,386.5\, + \,5.34\, \times \,{\text{and}}\,S_{o} \left( {m^{ - 2} } \right)\, = \,\left( {3.341-0.031 \, x} \right)\, \times \,10^{13} .$$

As shown in these figures, the value of ${\uplambda }_{\mathrm{o}}$ increases and that of S_o decreases as the Cd-ratio increases. It is worthwhile to mention that the values of both λ_o and S_o can be obtained from the graphical representation of the refractive index factor ${\left({n}^{2}-1\right)}^{-1}$ versus the reciprocal of $({\uplambda }^{-2})$ according to the following Eq. [77, 78]:

$$\left( {n^{2} - 1} \right)^{{ - 1}} = \frac{1}{{S_{o} \lambda _{o}^{{2~}} }}~ - ~\frac{1}{{S_{o} \lambda ^{2} }}$$

(12)

Thus, it can obtain a straight line, which will intersect the Y-axis at a value equal to (S_oλ²_o)⁻¹, while the slope of this line is equal to (1/S_o) as represented in Fig. 16. Consequently, the (S_o and λ_o) values could be determined. It is worth mentioning that the graphical deduced values of both (S_o and λ_o) almost have the same values that are computed from Eq. (11) and tabulated in Table 5.

Fig. 16

Variation of the refractive index factor (n²−1)⁻¹versus λ⁻² of a-S_50-xGe₁₀Cd_xTe₄₀ films

3.6 Absorption coefficient and transition energy

After determining the values of the refractive index (n₂) and obtaining its spectra over the studied range of the wavelength (500–2500 nm), as shown in Fig. 6, it became possible to calculate the values of the absorption coefficient (α) and obtain its spectra over the same spectral range. The values of the absorbance x(λ) have already been calculated by solving Eq. (1) for the upper envelope (R_M) of the reflectance spectra (Fig. 2), and then were recorded in Table 3. The absorption coefficient (α) of studied a-S_50-xGe₁₀Cd_xTe₄₀ films can be inferred by knowing the values of both the thickness ($\overline{d }$) and absorbance (x₂) from the following Eq. [38, 68]: α = $-\left(1 \left/\overline{d}\right.\right) ln ({x}_{2})$.

Consequently, the spectra values of (α) along the studied range could be obtained and then plotted in Fig. 17. Obviously, and as illustrated in Fig. 17, the absorption coefficient values (α) are also ranged between 10⁴ cm⁻¹ and 10⁵ cm⁻¹, along with its spectra shift towards the higher wavelengths, i.e., there is a redshift of the absorption edge of S_50-xGe₁₀Cd_xTe₄₀ films by the increment of the Cd-content. This is fully consistent with the behavior of both the reflectance and transmittance, Figs. 2 and 3, respectively. This redshift can be attributed to the addition of more Cd to the host Ge–S–Te lattice, which causes some structural and disorder changes [79, 80]. This behavior was observed for many Ge-chalcogenide thin films that were doped by a metallic element [33, 50, 68].

Fig. 17

The spectral variation of the absorption coefficient, α of thin a-S_50-xGe₁₀Cd_xTe₄₀ films

On the other hand, the relationship between the incident energy of photons (hν) and (α) was established by Mott and Davis for semiconductor materials in the high absorption region and then was developed by Tauc, who assumed the final equation of accounting for the optical band gap's energy, E_g [68, 69]. This energy, E_g is sometimes called Tauc’s energy, which is given as:

$${(\mathrm{\alpha h\nu })}^{\updelta }= {\mathrm{B}}^{\updelta }(\mathrm{h\nu }-{\mathrm{E}}_{\mathrm{g}})$$

(13)

where (B) is a constant, called the band tailing parameter. This parameter is an energy-independent parameter, but it is dependent on the electronic transition probability. It has been calculated, listed in Table 4, and found to decrease from 116.21 (cm/eV)^0.5 to 55.21 (cm/eV)^0.5 as Cd-content increases from zero to 15 at.%. While E_g is the optical bandgap (or Tauc's) energy, and according to the Mott and Davis model, MDM of the density of localized states near the mobility edges, this energy is the energy required for electronic transition for the forbidden optical gap [68, 69, 80]. Further, the exponent parameter (m) is equal to either 2 for the allowed direct transition or 1/2 for the allowed indirect one. According to MDM, this parameter for non-crystalline materials is usually equal to one-half (${\varvec{\updelta}}$ = 1/2).

Tauc also suggested that the relationship between ${(\alpha h\nu )}^{{\varvec{\updelta}}}$ and $(h\nu )$ must be graphically plotted, and the graph that gives the longest straight line to the curve gives the type of electronic transition. Indeed, the authors plot the relationship twice (when ${\varvec{\updelta}}$ = 2 and 1/2), and they have found that the longest straight-line is obtained when ${\varvec{\updelta}}$ = 1/2, which is consistent with the Mott and Davis model for non-crystalline materials [68, 69, 78, 80]. Figure 18 shows the plot of ${(\alpha h\nu )}^{1/2}$ and $(h\nu )$ and the extrapolations of the obtained line intersect the x-axis at a point that gives E_g, directly, and all the obtained E_g values of the present amorphous S_50-xGe₁₀Cd_xTe₄₀ films have been listed in Table 5.

Fig. 18

The graphical relationship between ${(\mathrm{\alpha h\nu })}^{1/2}$ and $(\mathrm{h\nu })$ of the amorphous

It is observed that the optical energy value decreases from 1.60 eV to 1.31 eV. Moreover, Tauc's energy values, E_g have been represented as functions of the Cd-content in Fig. 12c, and the obtained graph is linearly fitted to get this experimental Eq.: E_g (eV) = 1.595—0.019 x. The optical bandgap energy is slightly decreased due to increasing the Cd-ratio from zero to 15 at.%, where increasing of cadmium has twofold effects. First, it is a metallic element, and it increases at the expense of sulfur. Second, Cd-additions lead to slightly increased band tail width due to increased density of localized states formed at the edges of the mobility bands [78, 80,81,82,83,84,85,86,87]. Furthermore, and according to Sanderson's assumption, the shrinkage of the bandgap is related to the increment of Cd-content owing to the decrease in both the electronegativity and the average stabilization energy values of the sample, which were caused by adding more Cd [73]. Where the electronegativity mean value of the film composition decreases since the Cd-element has the lowest electronegativity value (see Table 1). It is worth mentioning that the extensions of the obtained straight lines of the relationship between ${(\alpha h\nu )}^{0.5}$ and$(h\nu )$, Fig. 18 are coming together at one point. This point is called the focal point, FP (Please, see Fig. 18), the energy of FP (E_FP = 2.24 eV) and ${\sqrt{\alpha h\nu }}_{\mathrm{FP}}=702 {cm}^{-0.5}{eV}^{0.5}$. This indicates that the band tail parameter B is strongly dependent on the electronic transition and the formed tail width [78].

3.7 Urbach Energy of a–S_50-xGe₁₀Cd_x Te ₄₀ thin films

According to Urbach Form, the absorption coefficient is related to the photon energy by this simple Eq. [79, 80,81,82,83,84,85,86,87]: $\alpha = {\alpha }_{o }exp(h\nu$/E_e) or ln $\alpha$ = ln $\alpha$₀ +$h\nu$/E_e.Thus, plotting a graph between (ln $\alpha )$ and $(h\nu )$ gives a straight line that has a slope equal to (1/E_e). Thus, the band tail width energy, E_e can be estimated. Figure 19 illustrates the relationship between (ln $\alpha )$ and $(h\nu )$ of S_50-xGe₁₀Cd_xTe₄₀. Thus, it can determine the value of E_e of the present film samples and then listed them in Table 5, two. The value of E_e increases from 0.0276 eV to 0.0644 eV, as the Cd-content percentage increases from zero to 15 at.%. As it is observed, the formed band tail width is slightly increased because of increasing the percentage of the Cd metal, and its effect on disturbance and disorder is limited.

Fig. 19

Representation of ln ($\alpha )$ Vs. $(\mathrm{h\nu })$ for the amorphous S_50-xGe₁₀Cd_xTe₄₀ thin films

Moreover, by comparing Tauc's energy values, E_g with those obtained from the SEOM-model of Wemple-Di-Domenico, there is a good match between the two methods. The value of the ratio between the single effect oscillator energy, E_o and Tauc's energy, E_g (E_o/E_g) was calculated and found to be almost equal to 2 for all film samples of the S_50-xGe₁₀Cd_xTe₄₀ system [26, 80,81,82,83,84,85]. The values of this ratio have been listed in Table 5, and then represented graphically in Fig. 12d, too. It is obvious from Table 5 and Fig. 12d, which were linearly fitted, that the ratio between E_o and E_g is almost equal to two for all studied films.

Consequently, it can be concluded that the effect of adding more Cd to the host lattice of the S₅₀Ge₁₀Te₄₀ system is that it causes a slight increase in the density of localized states formed in the optical forbidden gap, as the value of E_e is slightly increased. This increase also leads to a simple increase in the band tail width [79, 84]. Thus, it was found that the optical bandgap energy (E_g) decreases with the addition of more cadmium, where adding Cd to the Ge–S-Te glassy system leads to some modifications in the structural features of the system, which in turn leads to improving the optical properties of film samples, especially their refractive indices, dispersion properties, and band gap energies.

4 Conclusions

This article has been dedicated to manufacturing the novel chalcogenide S_50-xGe₁₀Cd_xTe₄₀ ($0.0\le x\le 15 at. \%$), SGCT glassy films. The optical transmission and reflection of thin films have also been measured using the spectrophotometer in the optical range from 500 to 2500 nm. Using the envelope Minkov method of the optical reflection spectra, it could calculate the film thicknesses and complex index of refraction. The refractive indices of these films have been appointed at all interference fringes. Then, Couchy's relationship can be used to calculate the refractive indices for all wavelengths along with the studied spectral range. Using the single effective oscillator model supposed by the Wemple-Di-Domenico, of the dispersion, it could determine the dispersion energy, E_d, the single effective oscillator, E_o, and many other dispersion parameters. The optical energy gap as supposed by Wemple-Di-Domenico was estimated and found to be in good match with what was studied by Tauc’s Plots. Many dispersion parameters have been determined and discussed, such as the lattice dielectric constant ε_L, the static refractive index, n_o, and the moments (M_-1 and M_-3). Moreover, the Sellmeier parameters were also investigated depending upon the classical dispersion theory and the ratio of (N/m*), the oscillator wavelength, λ_o and the oscillator strength, S_o have been estimated and discussed. The electronic polarizability, ${\alpha }_{el}$ of the thin film samples have been also studied and discussed, depending upon the measurements of the volumetric-mass density of samples, the reflection loss, RL and the average molar refraction values, R_m. Using the spectral measurements of the reflection, the authors have determined the absorption coefficient, α, which was found in the range of${ 10}^{4} cm\le \alpha \le {10}^{5} cm$. The electronic transition of the forbidden band gap was arising due to an indirect allowed transition. The optical band gap energy, E_g has been determined by using Tauc's plots. From these plots, it was found that E_g value decreases from 1.600 eV to become 1.309 eV and the band tail parameter, B decreases from 1078 (cm⁻¹/eV)^0.5 to become 743 (cm/eV)^0.5 when the Cd-content percent increases from zero to 15.0 at.%. On the contrary, the band tail width energy, E_e, which has been determined by using Urbach assumption, was found to increase from 267 × 10^–4 eV to become 644 × 10^–4 eV.