Phonon frequencies, mechanical and optoelectronic properties for ${\mathbf{InP}}_{{\mathbf{x}}} {\mathbf{As}}_{{\mathbf{y}}} {\mathbf{Sb}}_{{1 - {\mathbf{x}} - {\mathbf{y}}}}$/InAs alloys under the influence of pressure

Abstract

The pressure dependence of electronic and optical properties for InP_xAs_ySb_1−x−y alloys lattice matched to InAs substrate has been investigated. The mechanical properties and the phonon frequencies for InP_xAs_ySb_1−x−y/InAs system under the effect of pressure have been studied. The physical properties of the binary components InP, InAs and InSb that constitute the quaternary alloy were used in this analysis. The study achieved using the empirical pseudo-potential approach (EPM) under the virtual crystal approximation (VCA). Our findings were found to be in good agreement with experimental and theoretical data at p = 0 kbar. Our results may serve as reference for the future experimental values at high pressures. The pressure dependence of the fundamental properties of InP_xAs_ySb_1−x−y/InAs system such as optical constants and modes of lattice vibration has not been fully studied. So, we have concentrated on these properties under the effect of pressure.

1 Introduction

The III–V semiconductors are major materials used in manufacturing high-speed electronics, long-wavelength devices [1,2,3,4,5]. In the recent years, the ternary and quaternary compounds of the III–V groups have been the subject to several experimental and hypothetical analysis [6,7,8,9]. The binary compounds InP, InAs and InSb form continuous series of alloys denoted by InP_xAs_ySb_1–x–y over the entire range of the composition parameters of x and y. The study of such quaternary alloys is making use of the data available for the binary compounds which constitute them. It has many applications as a source and detector of long-wavelength light communication systems. The recent usage of such alloy is designing low-loss optical fibers in the 2–4 μm wavelength region [10,11,12,13,14]. InP_xAs_ySb_1–x–y lattice matched to InAs is also used to build low-temperature TPV cells (thermo-photovoltaic cells) in the range of 0.35–0.5 eV [15]. It is important to examine the electrical, optical, mechanical and thermal properties of semiconductor compounds, as this regulates us in the planning of high-performance electronic devices [13, 16,17,18,19,20,21,22,23]. The InP_xAs_ySb_1–x–y quaternary structure has an important role in the electronic applications. So, we have studied thoroughly its basic properties such as electronic, optical properties and lattice vibration modes and their dependence on pressure. The quaternary InP_xAs_ySb_1–x–y alloy can be lattice matched to two different substrates, InAs and GaSb, and in this work, we used InAs substrate.

The EPM is one of several techniques for determining the band structure of semiconductor materials [16, 24]. Many studies have been done on the effects of pressure and temperature on the physical properties of materials [24,25,26,27,28,29]. Sadao Adachi [10] studied the band gaps and refractive indices of InPAsSb, GaInAsSb and AlGaAsSb. To the best of our knowledge, the optical, electronic, mechanical and vibrational properties in InP_xAs_ySb_1–x–y/InAs system under the effect of pressure have not been fully studied so far.

In the present work, the pressure dependence of optoelectronic and vibrational properties for the quaternary alloy InP_xAs_ySb_1–x–y lattice matched to InAs was studied. Our calculations were based on the empirical pseudo-potential method (EPM) [30,31,32] with the virtual crystal approximation (VCA) [33, 34].

2 Computational method

The calculations were performed using essentially the (EPM) under the (VCA). Based on this method, one can deduce the pseudo-potential form factors of ${\text{InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y}$ lattice matched to InAs substrate at zero pressure. The lattice matching condition is given by $x = ay + b,$ where x and y are the compositional parameters as reported by Adachi [11], where:

$$a = \frac{{a_{{{\text{InAs}}}} - a_{{{\text{InSb}}}} }}{{a_{{{\text{InP}}}} - a_{{{\text{InSb}}}} }}$$

(1)

$$b = \frac{{a_{{{\text{Substrate}}}} - a_{{{\text{InSb}}}} }}{{a_{{{\text{InP}}}} - a_{{{\text{InSb}}}} }}$$

(2)

It was found that the lattice matching condition for InAs substrate between x and y:

$$x = 0.68933 - 0.68933 y$$

(3)

The energy eigenvalues of the binary compounds which constitute the quaternary alloy could be determined by solving the secular determinant in numerical techniques [35,36,37].

$$\left\| {\left( {\frac{1}{2}\left| {{\mathbf{k}} + \mathop {\mathbf{G}}\limits^{\prime } } \right|^{2} - {\text{E}}_{{n{\mathbf{k}}}} } \right)\delta_{{{\mathbf{G}}\mathop {\mathbf{G}}\limits^{\prime } }} + \sum\limits_{{{\mathbf{G}} \ne \mathop {\mathbf{G}}\limits^{\prime } }} {V\left( {\left| {\Delta {\mathbf{G}}} \right|} \right)} } \right\| = 0$$

(4)

where G and $\mathbf{k}$ are the reciprocal lattice vector and the wave vector, respectively. The electronic energy band gaps could be calculated from the obtained energy eigenvalues. More details about this method stated in Refs. [35,36,37]. The form factors have been obtained by fitting the band gap energies of AlP and AlSb at the high symmetric points L(0.5,0.5,0.5), Γ(0,0,0) and X(0,0,1) in the Brillouin zone to the experimental values. The dimension of our eigen-value problem is a (65 × 65) matrix which provides generally good convergence. The pseudo-potential form factors and the lattice constant for the quaternary alloy could be determined as follow [11]:

$$W\left( {x,y} \right) = xW_{{{\text{InP}}}} + yW_{{{\text{InAs}}}} + \left( {1 - x - y} \right)W_{{{\text{InSb}}}}$$

(5)

$$a\left( {x,y} \right) = xa_{{{\text{InP}}}} + ya_{{{\text{InAs}}}} + \left( {1 - x - y} \right)a_{{{\text{InSb}}}}$$

(6)

The calculated direct $n$ and indirect energy band gaps of ${\text{InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y}$/InAs system are shown in Table 1. It should be mentioned that the pressure range in our calculations was performed through the stability of the crystal structure. As a matter of fact, in the pressure range 0–120 kbar, the structure of the material under investigation was still zinc blende. The polarity $\alpha_{p}$ of the alloys of interest could be determined by using Vogl's relation [38]. The elastic constants $({\text{c}}_{11} ,\;{\text{c}}_{12} ,\;{\text{c}}_{44} )$ and elastic moduli (bulk$B_{u}$, shear $C_{{\text{s}}}$, young $Y_{o}$) could be investigated according to Refs.[30, 39,40,41,42]. By knowing the fundamental energy band gap, the calculations of optical properties could be determined as refractive index, high frequency dielectric constant $\varepsilon_{\infty }$ and static dielectric constant $\varepsilon_{{\text{s}}}$. The longitudinal and transverse phonon frequencies $\omega_{{{\text{LO}}}} , \omega_{{{\text{TO}}}}$ could be determined by solving the Lyddane–Sachs–Teller relations [43, 44].

$$\frac{{\omega_{{{\text{TO}}}}^{2} }}{{\omega_{{{\text{LO}}}}^{2} }} = \frac{{\varepsilon_{\infty } }}{{\varepsilon_{s} }}$$

(7)

$$\omega_{{{\text{LO}}}}^{2} - \omega_{{{\text{TO}}}}^{2} = \frac{{4\pi \left( {e_{T}^{*} } \right)^{2} e^{2} }}{{M\Omega_{o} \varepsilon_{\infty } }}$$

(8)

where ε_∞,ε_s M and Ω₀ are the optical dielectric constant, static dielectric constant, twice of reduced mass and the volume occupied by one atom, respectively. The calculations of InP_xAs_ySb_1–x–y alloys are done using our own code based on MATLAB language.

Table 1 Energy gaps of ${\text{InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y} /{\text{InAs}}$ at different values of pressure at constant values of compositional parameter

3 Results and discussions

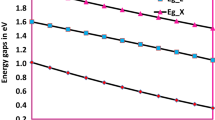
Table 1 and Fig. 1 show the effect of pressure from 0 to 120 kbar on the energy gaps of ${\text{InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y}$ lattice matched to InAs substrate for different compositional parameter y. It can be noticed that the energy gaps are increased at the $\Gamma ,L$ high symmetry points and decreased at X-point when the pressure increases. Due to this behavior, the quaternary alloy converts from a direct band gap semiconductor to an indirect one at a certain threshold value of pressure for every composition value. These inversion points were found to be (y = 0, p = 81 kbar), (y = 0.5, p = 78.5 kbar) and (y = 1, p = 74 kbar). The energy band gaps of ${\text{InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y}$/InAs as function of pressure were fitted by polynomials to give.

Fig. 1

The energy gaps of ${\text{InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y} /{\text{InAs}}$ as function of pressure at different values of y-composition parameter (0, 0.5, 1)

At y = 0

$$E_{L} \left( p \right) = 1.597 + 0.0058 p - 6 \times 10^{ - 6} p^{2}$$

(9)

$$E_{\Gamma } \left( p \right) = 1.012 + 0.0094 p - 9 \times 10^{ - 6} p^{2}$$

(10)

$$E_{X} \left( p \right) = 2.056 - 0.0042 p - 7 \times 10^{ - 7} p^{2}$$

(11)

At y = 0.5

$$E_{L} \left( p \right) = 1.329 + 0.0062 p - 7 \times 10^{ - 6} p^{2}$$

(12)

$$E_{\Gamma } \left( p \right) = 0.655 + 0.0105 p - 1 \times 10^{ - 5} p^{2}$$

(13)

$$E_{X} \left( p \right) = 1.761 - 0.0043 p - 1 \times 10^{ - 6} p^{2}$$

(14)

At y = 1

$$E_{L} \left( p \right) = 1.049 + 0.0068 p - 8 \times 10^{ - 6} p^{2}$$

(15)

$$E_{\Gamma } \left( p \right) = 0.359 + 0.0117 p - 1 \times 10^{ - 5} p^{2}$$

(16)

$$E_{X} \left( p \right) = 1.495 - 0.0043 p - 2 \times 10^{ - 6} p^{2}$$

(17)

Eqs. (9–17) can be used to predict the energy band gaps for the considered alloy for different pressures. Our calculated data at (y = 1 (InAs) & p = 0 kbar) of the E_L, E_Γ and E_X are in excellent agreement with the published values of 1.07 eV [45, 46], 0.36 eV [45, 46] and 1.37 eV [45, 46], respectively. Our calculated results of the energy band gaps for the ${\text{InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y}$/InAs system at high pressures may serve as reference for the experimental work.

The electronic band structure has been determined by using the (EPM) with including (VCA). Figure 2a shows the crystal structure for ${\text{InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y}$ alloy which has a face-centered cubic (FCC) lattice structure. The quaternary ${\text{InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y}$ alloy consists from four elements (In, P, As and Sb) or from three binary materials (InP, InAs and InSb). The electronic band structure of states for ${\text{InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y}$/InAs system for p = 0 kbar (solid lines) and p = 120 kbar (dashed lines) for composition y = 0.5 is displayed in Fig. 2b. It is observed from Fig. 2b that with increasing pressure, the minima of the conduction band at (Γ, L) points go up and at X-point go down. Consequently, the band gap at the Γ, L points increases, while at X-point decreases. It is seen that the conduction energy bands are more influenced by pressure than the valence energy bands. These properties of the alloy under investigation are desirable for the optoelectronic applications.

Fig. 2

The crystal structure (a) and electronic band structure for ${\text{InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y} /{\text{InAs}}$ system for p = 0 kbar (solid lines) and p = 120 kbar (dashed lines) for composition y = 0.5 (b)

The elastic constants are significant parameters that characterize the reaction to the macroscopic applied stress. The elastic constants C₁₁, C₁₂ and C₄₄ for the zinc blende $InP_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y}$/InAs system are listed in Table 2. Figure 3 displays the dependence of elastic constants $(10^{12} {\text{dyn}}/{\text{cm}}^{2} )$ on pressure for different constant values of y (0, 0.5, 1). It is noticed that all the elastic constants are increased with increasing pressure. The C₁₁ exhibits the largest values as compared to C₁₂ and C₄₄ and more affected by the pressure than C₁₂ and C₄₄. The calculated elastic constants are fitted by polynomials in 10¹² dyne cm⁻² that give the following relations:

Table 2 The elastic constants ${ }(10^{12} {\text{ dyn}}/{\text{cm}}^{2} )$ of ${\text{InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y} /{\text{InAs}}$ system for various pressures for different values of compositional parameter y

Fig. 3

The elastic constants ${ }(10^{12} {\text{ dyn}}/{\text{cm}}^{2} )$ of ${\text{ InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y} /{\text{InAs}}$ as function of pressure for different values of compositional parameter y

At y = 0

$$C_{11} \left( p \right) = 0.905 + 0.0004 p - 5 \times 10^{ - 8} p^{2}$$

(18)

$$C_{12} \left( p \right) = 0.391 + 0.0002 p - 5 \times 10^{ - 8} p^{2}$$

(19)

$$C_{44} \left( p \right) = 0.365 + 0.0002 p - 1 \times 10^{ - 7} p^{2}$$

(20)

At y = 0.5

$$C_{11} \left( p \right) = 0.918 + 0.0004 p - 2 \times 10^{ - 7} p^{2}$$

(21)

$$C_{12} \left( p \right) = 0.396 + 0.0002 p - 2 \times 10^{ - 7} p^{2}$$

(22)

$$C_{44} \left( p \right) = 0.371 + 0.0002 p + 9 \times 10^{ - 8} p^{2}$$

(23)

At y = 1

$$C_{11} \left( p \right) = 0.931 + 0.0005 p - 1 \times 10^{ - 7} p^{2}$$

(24)

$$C_{12} \left( p \right) = 0.402 + 0.0002 p - 9 \times 10^{ - 8} p^{2}$$

(25)

$$C_{44} \left( p \right) = 0.376 + 0.0002 p - 9 \times 10^{ - 8} p^{2}$$

(26)

Eqs. (18–26) can be used to predict the elastic constants for the ${\text{InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y}$/InAs system for different values of pressure. Our calculated values at (y = 1 & p = 0 kbar) of the $C_{11}$, $C_{12}$ and $C_{44}$ are in excellent accord with the published data of (0.943*10¹² ${\text{dyn}}/{\text{cm}}^{2}$) [47], (0.407*10¹² ${\text{dyn}}/{\text{cm}}^{2}$) [47] and (0.3819*10¹² ${\text{dyn}}/{\text{cm}}^{2}$) [47], respectively. Our calculated data of the elastic constants for the ${\text{InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y}$/InAs system at high pressures may serve as reference for the future experimental values.

Table 3 and Fig. 4 show the elastic moduli ($B_{u}$,$C_{s}$,$Y_{o}$) of ${\text{InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y}$ /InAs system under the effect of pressure for different values of compositional parameter y (0, 0.5 and 1). It can be noticed that all the mechanical moduli are increased with enhancing pressure. It is seen from Table 3 that the B_u/C_s ratio is greater than 1.75 which is the critical value that separates ductile and brittle materials [48]. The high values are associated with the ductility, whereas the low values correspond to the brittle nature. The calculated B_u/C_s values of ${\text{InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y}$ are greater than 1.75 for all values of pressure and for y = 0, 0.5 and 1. So, this alloy is classified as a ductile material over the whole range of pressure. There is an excellent agreement between our calculated values and the available published ones [46, 48, 49].

Table 3 The elastic moduli $\left( {10^{12} {\text{dyn}}/{\text{cm}}^{2} } \right)$ of InP_xAs_ySb_1−x−y/InAs at different values of pressure at constant values of compositional parameter y

Fig. 4

The mechanical moduli s ${ }(10^{12} {\text{ dyn}}/{\text{cm}}^{2} )$ of ${\text{InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y} /{\text{InAs}}$ as function of pressure for different values of compositional parameter y (0, 0.5, 1)

In the design and manufacturing of electronic devices such as photonic crystals, wave guides, solar cells and detectors, the refractive index and optical dielectric constants are important parameters. Table 4 and Fig. 5 display the effect of pressure on the refractive index n, the static dielectric constant ε₀ and the high frequency dielectric constant ε_∞ of ${\text{InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y}$ lattice matched to InAs substrate for different values of composition y (0, 0.5 and 1). It will be noted that the refractive index n, the static dielectric constant ε₀ and the high frequency dielectric constant ε_∞ are decreased with increasing pressure. Also, it can be seen that the refractive index n, the static dielectric constant ε₀ and the high frequency dielectric constant ε_∞ are increased with increasing the composition from 0 to 1. There is an excellent agreement between our calculated values and experimental ones [43, 45, 50,51,52].

Table 4 The refractive index, optical dielectric constant and static dielectric constant of ${\text{InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y} /{\text{InAs}}$ at different values of pressure at constant values of compositional parameter y

Fig. 5

The refractive index, optical dielectric constant and static dielectric constant of ${\text{InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y} /{\text{InAs}}$ as function of pressure at constant values of compositional parameter y (0, 0.5, 1)

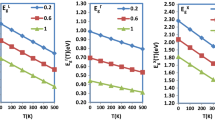
By solving the Lyddane–Sachs–Teller relationships, the longitudinal and the transversal optical phonon frequencies (ω_LO, ω_TO) of ${\text{InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y} /{\text{InAs}}$ system could be determined [43, 44]. Our calculated results are listed in Table 5 and illustrated in Fig. 6 which shows the dependence of the phonon frequencies on pressure at different compositions y (0, 0.5 and 1). Both $\omega_{{{\text{LO}}}}$ and $\omega_{{{\text{TO}}}}$ are increased by increasing pressure, and however, the separations between them are slightly affected with pressure. It is observed from this figure that the values of $\omega_{{{\text{LO}}}}$ and $\omega_{{{\text{TO}}}}$ are shifted downward when the concentration is increased along the range (0–1). On the other words, the $\omega_{{{\text{LO}}}}$ and $\omega_{{{\text{TO}}}}$ are decreased with enhancing composition. The following polynomials show the best fits of their pressure dependence.

Table 5 The phonon frequencies $\omega_{{{\text{LO}}}}$ and $\omega_{{{\text{TO}}}}$ (10¹³ s⁻¹) of ${\text{InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y} /{\text{InAs}}$ for different compositional alloys under the effect of pressure

Fig. 6

The phonon frequencies $\omega_{{{\text{LO}}}}$ and $\omega_{{{\text{TO}}}}$ of ${\text{ InP}}_{{\text{x}}} {\text{As}}_{{\text{y}}} {\text{Sb}}_{{1 - {\text{x}} - {\text{y}}}} /{\text{InAs}}$ as function of pressure at constant values of y-composition parameter (0, 0.5, 1)

At y = 0

$$\omega_{{{\text{LO}}}} \left( p \right) = 5.623 + 0.0161 p + 1 \times 10^{ - 5} p^{2}$$

(27)

$$\omega_{{{\text{TO}}}} \left( p \right) = 5.278 + 0.0163 p + 1 \times 10^{ - 5} p^{2}$$

(28)

At y = 0.5

$$\omega_{{{\text{LO}}}} \left( p \right) = 4.9591 + 0.0172 p + 2 \times 10^{ - 5} p^{2}$$

(29)

$$\omega_{{{\text{TO}}}} \left( p \right) = 4.6825 + 0.0174 p + 3 \times 10^{ - 5} p^{2}$$

(30)

At y = 1

$$\omega_{{{\text{LO}}}} \left( p \right) = 4.4597 + 0.0183 p + 4 \times 10^{ - 5} p^{2}$$

(31)

$$\omega_{{{\text{TO}}}} \left( p \right) = 4.2362 + 0.0185 p + 4 \times 10^{ - 5} p^{2}$$

(32)

The expressions (27–32) can be used to predict the phonon frequencies ω_LO and ω_TO for the alloys under investigation for various pressures. Our calculated results at (y = 1 (InAs) & p = 0 kbar) of the LO(Γ) and TO(Γ) modes are in good agreement with the experimental values of (4.5*10¹³ s⁻¹) [43], (4.1*10¹³ s⁻¹) [43] and theoretical values of (5.2*10¹³ s⁻¹) [45], (4.9*10¹³ s⁻¹) [45], respectively. The calculated values of $\omega_{{{\text{LO}}}}$ and $\omega_{{{\text{TO}}}}$ at high values of pressure may serve as reference for the experimental work.

4 Conclusions

In this paper, we have presented a study of electronic structure, elastic constants and the related mechanical properties for ${\text{InP}}_{x} {\text{As}}_{y} {\text{Sb}}_{1 - x - y}$ lattice matched to InAs in the zinc-blende structure using the empirical pseudo-potential approach under the virtual crystal approximation. The pressure dependence of the optical properties as refractive index, high frequency and static dielectric constants has been determined. The longitudinal and transverse phonon frequencies of the alloys of interest are also examined. All studied quantities are studied under pressure up to 120 kbar. Our calculated values of the optical, electronic, mechanical properties and phonons frequencies for the considered alloys are found to be in a reasonable agreement with the experimental values. The calculated results in the present work seem likely to be useful as reference to the quantities that are not easily experimentally obtained, especially at high pressure. The electronic, optical and mechanical properties of the InP_xAs_ySb_1−x−y/InAs system make it a successful candidate for optoelectronic applications such as thermo-photovoltaic cells.