Introduction

Pyroxenes are important minerals in the deep Earth. In the bulk mantle, according to pyrolitic compositional model, the top upper mantle is composed of ~65 % olivine, ~20 % pyroxene and ~15 % garnet by volume (Ringwood 1975; Ita and Stixrude 1992). As pressure and temperature increase, pyroxenes gradually dissolve into garnet and form majorite garnet at the base of the upper mantle (Ringwood 1967). Pyroxenes are also abundant in subducting slabs. The oceanic lithosphere is composed of basaltic crust (fine-grained basalt and coarse-grained gabbro), harzburgite, lherzolite and light rare earth element (LREE)-depleted pyrolite. There are ~22 and ~35 % pyroxenes in harzburgite and lherzolite, respectively (Ringwood 1982). As the slab subducts into mantle, the top basaltic crustal layer undergoes eclogitic metamorphism during which plagioclase and some of the clinopyroxenes are consumed to produce garnet (Ringwood 1982). However, recent high-pressure studies show that the dissolution of pyroxene into garnet is kinetically suppressed at temperatures below geotherm (<1000 °C); thus, they may be preserved to much higher pressure in the cool subducting slab (Nishi et al. 2008, 2013; Van Mierlo et al. 2013). Recently, anomalous seismic velocity structures have been observed by different seismic methods and are interpreted as metastable olivine wedge in cold subducting slabs (Jiang et al. 2008, 2015; Kaneshima et al. 2007). It is possible that metastable pyroxenes exist in the cold slabs and even in broader ranges than metastable olivine, due to the higher temperatures defining their metastability limits (Bina 2013).

Pyroxenes, with a general formula M2M1(Si, Al)2O6, belong to the inosilicate family, with crystal structures consisting of alternating layers of tetrahedral chains and layers of octahedrally coordinated cations. The ability of the tetrahedra along the silicate chains to rotate with respect to one another allows the structure to accommodate cations with different ionic radii and charges, leading to a wide range of possible compositions (Cameron and Papike 1981). Recent single-crystal X-ray diffraction studies on pyroxenes (enstatite, diopside, ferrosilite) reported a number of new high-pressure phases under ambient temperature (Plonka et al. 2012; Zhang et al. 2012; Dera et al. 2013a, b; Finkelstein et al. 2014). Hedenbergite is an important Ca, Fe end-member of clinopyroxenes family, and constraining its behavior and physical properties can be useful for modeling the behavior of clinopyroxene solid solutions. Hedenbergite can be found in chondrites (Krot et al. 2000) and skarn, a calcium-bearing rock (Meinert 1992). Hedenbergite has been the subject of several high-pressure studies. Its structure was determined up to ~10 GPa by single-crystal diffraction, and no discontinuous behavior was observed (Zhang et al. 1997). On the other hand, high-pressure nuclear forward scattering (synchrotron Mössbauer) studies up to 68 GPa reported discontinuities at 53 and 68 GPa (Zhang et al. 1999). High temperature and high-pressure phase transformation of hedenbergite were studied up to 40 GPa and ~1200 °C, and decomposition to multiple phases was also observed (Kim et al. 1989, 1991).

Single-crystal diffraction is a powerful tool for resolving variations in the crystal structure. The bond length changes inside the crystal can be useful for predicting crystal behavior at high pressure and calculating element partitioning, as well as thermodynamic properties (Wood and Blundy 1997; Chermak and Rimstidt 1989; Van Hinsberg et al. 2005a, b). Here, we determined the compressibility, structure and vibrational properties of natural hedenbergite by synchrotron single-crystal X-ray diffraction and Raman spectroscopy up to ~33 GPa in diamond anvil cell. Compressibilities and pressure evolution of bond lengths and polyhedral distortion are determined and discussed.

Experimental details

In this study, we used a sample of natural hedenbergite from the University of Arizona RRUFF collection #R070236, with composition Ca1.00(Fe.67Mn.16Mg.17)Si2O6, determined by means of electron microprobe analysis. The ambient unit cell parameters, determined by single-crystal X-ray diffraction at the University of Arizona, are a = 9.834(6) Å, b = 9.023(5) Å, c = 5.237(2) Å, β = 104.70(3)° and V 0 = 449.5(6) Å3. For comparison, the sample used by Zhang et al. (1997) was a synthetic CaFeSi2O6 hedenbergite.

A large approximately 1 mm3 specimen of hedenbergite was crushed into smaller pieces in a mortar under methanol. One small platelet-shaped crystal with approximate size 0.025 × 0.025 × 0.005 mm was loaded into a diamond anvil cell (DAC). The sample crystal was oriented with [1 1 0] direction along the DAC axis.

Diamond anvils with 0.250-mm culet size and Re gasket preindented to a thickness of ~0.042 mm were used. We utilized a combination of cubic boron nitride cBN (upstream) and tungsten carbide WC (downstream) backing plates. The angular access to the sample through the WC seat was ±13°; however, two additional rotation ranges, from −30° to −20° and from 20° to 30°, could be accessed thanks to the low X-ray absorption coefficient of the cBN seat. In the remaining two rotation ranges, from −20° to −13° and from 13° to 20°, strong powder diffraction signal caused by the transmitted beam touching the WC seat cone contaminated the diffraction images and this data were not used for further analysis. The gasket hole with initial diameter of ~0.160 mm was filled with Ne pressure medium, using the GSECARS/COMPRES gas loading apparatus (Rivers et al. 2008). Two small ruby spheres were placed in the sample chamber together with the sample crystal for pressure calibration. Pressure was calculated from the shift of the R1 ruby fluorescence line (Mao et al. 1986). The diffraction experiments were carried out at the GSECARS facility at APS, Argonne National Laboratory, in experimental station 13IDD. Monochromatic beam with incident energy of 37 keV was focused by a pair of Kirkpatrick–Baez mirrors to a spot of 0.003 by 0.005 mm. Diffraction images were collected using a MAR165 charge-coupled device (CCD) detector, placed at a sample-to-detector distance of approximately 200 mm. During the exposure, the sample was rotated about the vertical axis of the instrument (ω) in the three accessible rotation ranges, with a typical exposure time of 0.5 s/°. Diffraction images were collected at three different detector positions, differing by a translation of 70 mm perpendicular to the incident beam. The detector geometry parameters at each detector position were calibrated with a CeO2 NIST diffraction standard. In addition to the full-rotation exposures, a step scan with 1° rotation steps was performed at each pressure. The sample was compressed in approximately 5 GPa steps, with full data collection at each step. The pressure range covered was 0.0001–29.9(1) GPa.

Diffraction images were analyzed using the GSE_ADA/RSV software package (Dera et al. 2013a, b). Integrated peak intensities were corrected for Lorenz, polarization, DAC absorption and sample displacement effects using the methods implemented in GSE_ADA (Dera et al. 2013a, b). Because of high incident energy, negligible sample thickness and low X-ray absorption coefficient of the sample at 37 keV, the sample absorption effect was ignored. Corrected peak intensities were used for least-squares structure refinement with SHELXL (Sheldrick 2008), which started from the C2/c ambient pressure structure model of Cameron et al. (1973). Because of the limited number of unique observations, anisotropic atomic displacement parameters (ADPs) were used only for the non-oxygen atoms, whereas oxygen atoms were modeled with isotropic ADPs. According to the microprobe analysis, the M2 site was fully (within experimental uncertainty) occupied by Ca2+ and any attempts at refining the site occupancy factor (SOF) for this site resulted in convergence to SOF = 1; therefore, in the final refinement, this parameter was fixed to one. Occupancy model was also not refined for Si4+ and oxygen sites. For the M1 site, we assumed that Mn2+ and Fe2+, having almost the same atomic number, are undistinguishable with X-rays and treated both of these cations as Fe2+. We modeled cation disorder at the M1 site assuming substitution of Fe2+/Mn2+ with Mg2+ and constraining the sum of the two SOFs to one (no vacancy model). The SOF model of M1 site was only refined at the lowest pressure point and was fixed to be a constant at high pressures. The positions and ADPs of Fe2+ and Mg2+ were fixed to be the same. The substitution model for the M1 site at 1.9(1) GPa, based on refined SOFs for Mg2+ and Fe2+/Mn2+, is consistent with the microprobe results. Extinction parameter was also refined. Details of the crystal structure refinements, final fractional atomic coordinates and ADPs, as well as selected bond lengths and angles at the six pressures studied, are presented in Tables 1, 2 and 3. VESTA was used to calculate polyhedral volume.

Table 1 Refinement results of hedenbergite up to 29.9(1) GPa
Full size table
Table 2 Structural parameters of hedenbergite as a function of pressure
Full size table
Table 3 Atomic displacement parameters (Å2) of hedenbergite as a function of pressure
Full size table

Raman spectra were collected at GSECARS off-line system at the Advanced Phonon Source, Argonne National Laboratory. Raman scattering was excited with 514 nm green laser. The laser was focused on the sample by a 10× objective to a spot of few micrometers. Raman spectra were collected with a Horiba HR460 spectrometer equipped with 1800 lines/mm grating. A 30-μm spatial filter was used. The Roper Scientific LN/CCD-1340/100-EB/1 liquid nitrogen cooled CCD was used as detector.

Results

Axial compressibilities of hedenbergite

Unit cell parameters of hedenbergite up to 29.9(1) GPa are listed in Table 4. Normalized unit cell parameters of this study along with Zhang et al. (1997) are shown in Fig. 1. Our low-pressure results show good agreement with the previous study. No discontinuity in the pressure dependence of any of the parameters is found up to 29.9(1) GPa.

Table 4 Unit cell parameters of hedenbergite
Full size table
Fig. 1
figure 1

Pressure dependences of normalized unit cell parameters \(\frac{a}{{a_{0} }}\), \(\frac{b}{{b_{0} }}\), \(\frac{c}{{c_{0} }}\), \(\frac{\beta }{{\beta_{0} }}\), \(\frac{V}{{V_{0} }}\). Circles are data reported by Zhang et al. (1997). Triangles represent results of this study. Error bars are smaller than the symbol size

Full size image

Axial compressibility β l0 is defined as

$$\beta_{l0} = \frac{1}{{3K_{l0} }} = - \left( {\frac{1}{l}\frac{\partial l}{\partial P}} \right)_{T}$$
(1)

where K l0 is the linearized modulus at ambient pressure, l is axis length and P and T represent pressure and temperature, respectively. K l0 is obtained by weighted least-squares fit of the second-order linearized Birch–Murnaghan (BM2) equation of state as shown below (Hazen and Downs 2000):

$$P\left( l \right) = \frac{{3K_{l0} }}{2}\left[ {\left( {\frac{{l_{0} }}{l}} \right)^{7} - \left( {\frac{{l_{0} }}{l}} \right)^{5} } \right].$$
(2)

The three crystallographic axes a, b and c of hedenbergite exhibit different compressibilities. The results of linearized second-order Birch–Murnaghan equation of state fitting are shown as solid lines in Fig. 1. Axial compressibility of a, b and c are calculated to be 1.7(2), 4.9(5), 2.13(9) × 10−3 GPa−1 by BM2. β in Fig. 1 is fit with third-order polynomial fitting.

Equation of state of hedenbergite

The bulk modulus K T0 and its pressure derivative \(K_{T0}^{\prime }\) can be determined with a weighted nonlinear least-squares fitting of third-order Birch–Murnaghan equation of state (BM3):

$$P\left( V \right) = \frac{{3K_{T0} }}{2}\left[ {\left( {\frac{{V_{0} }}{V}} \right)^{7/3} - \left( {\frac{{V_{0} }}{V}} \right)^{5/3} } \right]\left\{ {1 + \frac{3}{4}\left( {K_{T0}^{{\prime }} - 4} \right)\left[ {\left( {\frac{{V_{0} }}{V}} \right)^{2/3} - 1} \right]} \right\},$$
(3)

where P is pressure and V and V 0 represent the volume at high pressure and ambient pressure, respectively (Hazen and Downs 2000). The bulk modulus K T0 and \(K_{T0}^{\prime }\) are determined to be 131(4) GPa and 3.8(3) in this study, as shown in Table 5. Considering the trade-off between K T0 and \(K_{T0}^{\prime }\), the confidence ellipses (with a confidence level of ±68.3 %) of this study and previous study are shown in Fig. 2. Previous study has a smaller bulk modulus K T0 and a wider range of \(K_{T0}^{\prime }\). Second-order Birch–Murnaghan equation of state (BM2) is also obtained with K = 128.3(5) GPa.

Table 5 Bulk modulus (K T0) and its pressure derivatives (\(K_{T0}^{\prime }\)) of hedenbergite
Full size table
Fig. 2
figure 2

Confidence ellipses in K T0 and \(K_{T0}^{\prime }\) for the fit of the third-order Birch–Murnaghan equation of state to the hedenbergite P–V data. Data with the filled circles are from this study yielding V 0 = 449.2(5) Å3, K T0 = 131(4) GPa and \(K_{T0}^{\prime } = 3.8\left( 3 \right)\). Data with the open circles represent Zhang et al.’s (1997) giving V 0 = 449.8(1) Å3, K T0 = 118(3) GPa and \(K_{T0}^{\prime } = 4.2\left( 7 \right)\)

Full size image

Figure 3 shows the f E –F E plot, depicting a relationship between Eulerian strain f E and Birch normalized stress F E ; thus, K T0 and \(K_{T0}^{\prime }\) can also be determined from a weighted linear least-squares fitting (Hazen and Downs 2000).

$$F_{E} = K_{T0} \left[ {1 + 1.5f_{E} \left( {K_{T0}^{{\prime }} - 4} \right)} \right]$$
(4)

where

$$f_{E} = {{\left[ {\left( {V/V_{0} } \right)^{{ - 2/3}} - 1} \right]} \mathord{\left/ {\vphantom {{\left[ {\left( {V/V_{0} } \right)^{{ - 2/3}} - 1} \right]} 2}} \right. \kern-\nulldelimiterspace} 2}$$
(5)

and

$$F_{E} = {P \mathord{\left/ {\vphantom {P {\left[ {3f_{E} \left( {1 + 2f_{E} } \right)^{{5/2}} } \right]}}} \right. \kern-\nulldelimiterspace} {\left[ {3f_{E} \left( {1 + 2f_{E} } \right)^{{5/2}} } \right]}}.$$
(6)
Fig. 3
figure 3

Birch’s normalized stress F E versus the Eulerian strain f E . Triangles represent the data from this study. Bulk modulus K and its pressure derivative calculated from linear fit of this study are K T0 = 128(2) GPa and \(K_{T0}^{\prime } = 4.0\left( 2 \right)\)

Full size image

In Eqs. (4)–(6), P is pressure and V and V 0 represent the volume at high and ambient pressures, respectively. K T0 = 128(2) GPa and \(K_{T0}^{\prime } = 4.0\left( 2 \right)\) are determined using weighted linear least-squares fitting and are in good agreement with the results of BM3 fitting discussed above.

Polyhedral compression in hedenbergite

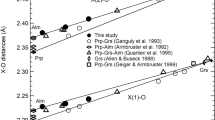
Figures 4, 5 and Table 6 report the bond lengths and polyhedron volumes of SiO4 tetrahedron, FeO6 octahedron and CaO8 polyhedron. The three polyhedra show anisotropic compressibility, usually shorter bond lengths show lower compressibility and smaller polyhedra show lower compressibility. It is interesting to notice that there are crossovers in bond lengths for all three polyhedra; however, they do not seem to cause any discontinuous changes in the compression mechanism.

Fig. 4
figure 4

Bond lengths of hedenbergite. Data with filled markers are from this study, and those with open markers are from Zhang et al.’s (1997). a Si–O bond lengths of hedenbergite. There are four different Si–O bond lengths, Si#0–O(3)#2, Si#0–O(3)#1, Si#0–O(2)#0 and Si#0–O(1)#0, which are represented by diamonds, pentagrams, right-pointing triangles and circles, respectively. The average Si–O bond lengths are marked with squares. The 12.7(1) GPa data points were excluded when doing second-order polynomial fitting for Si#0–O(3)#2 and Si#0–O(3)#1. b Fe–O bond lengths of hedenbergite. Six bonds with three different bond lengths are reported here. Fe(1)#0–O(1)#14 and Fe(1)#0–O(1)#13 have the same length and are represented by diamonds. Fe(1)#0–O(2)#4 and Fe(1)#0–O(2)#12 have the same bond length and are represented by circles. Triangle markers stand for Fe(1)#0–O(2)#11 and Fe(1)#0–O(2)#10, while the average bond lengths are represented by squares. c Ca–O bond lengths are reported. Ca(2)#0–O(3)#6 and Ca(2)#0–O(3)#3 are represented by pentagrams, and Ca(2)#0–O(3)#9 and Ca(2)#0–O(3)#8 are marked with diamonds. Data with circles are Ca(2)#0–O(1)#0 and Ca(2)#0–O(1)#7, while those of Ca(2)#0–O(1)#3 and Ca(2)#0–O(1)#6 are marked with triangles. Average values are represented by squares

Full size image
Table 6 Selected bond lengths (Å), angles (°), average bond length and polyhedral volume (Å3) of hedenbergite up to 29.9(1) GPa
Full size table

SiO4 tetrahedron

SiO4 tetrahedron has the smallest volume of ~2.1 Å3 and shows the lowest volume compression of 2.0(1) × 10−3 GPa−1 between 1.9(1) GPa and 29.9(1) GPa, as shown in Table 6 and Fig. 5. Four unique pairs of bond lengths along with the average bond length are shown in Fig. 4a and Table 6. The average Si–O bond length decreases continuously with a lowest compressibility of 1.07(8) × 10−3 GPa−1 among the three polyhedra, while individual bond behavior shows minor irregularities. Si#0–O(3)#2 is the longest bond between 0 GPa and 5.9(1) GPa, while Si#0–O(3)#1 is the second longest bond. These two bonds show anomalous behavior at 12.7(1) GPa; however, we attribute this anomaly to possible experimental error and omit that pressure point in polynomial fittings shown in Fig. 4a. A turnover is found in the two shorter Si–O bonds between 5.6(1) and 12.7(1) GPa.

Fig. 5
figure 5

Pressure dependences of SiO4 (circle), FeO6 (triangle) and CaO8 (asterisk) polyhedral volumes in hedenbergite

Full size image

M1 octahedron

Between 1.9(1) and 29.9(1) GPa, the volume of the M1 octahedron decreases from 12.48 to 10.72 Å3 and shows a compressibility of 5.2(2) × 10−3 GPa−1, as listed in Table 6 and Fig. 5. There are three different bond lengths in FeO6 octahedron, and they show some anisotropy of compression behavior as shown in Fig. 4b and Table 6. The shortest bond Fe(1)#0–O(1)#11 shows a medium compression. Fe(1)#0–O(1)#14 is the longest bond at low pressure and shows the largest compressibility, while the second longest bond, Fe(1)#0–O(1)#4, displays the smallest compression among the three bonds, thus leading to a crossover of the two bonds somewhere between 5.9(1) and 12.7(1) GPa. This crossover was also observed by Zhang et al. (1997). The average bond displays a compressibility of 3.7(1) × 10−3 GPa−1 which is medium among the three polyhedra.

M2 polyhedron

As shown in Table 6 and Fig. 5, the larger polyhedral site, CaO8, has a volume of 25.58 Å3 at 1.9(1) GPa and 21.96 Å3 at 29.9(1) GPa and is characterized by an average compressibility of 5.1(7) × 10−3 GPa−1 from 1.9(1) to 29.9(1) GPa. Four unique bond lengths are shown in Fig. 4c and Table 6. The longest bond Ca(2)#0–O(3)#6 shows the highest compressibility, while the second longest bond Ca(2)#0–O(3)#9 displays a second largest compressibility; therefore, a crossover is observed at ~5.9(1) GPa. The shortest bond is Ca(2)#0–O(2)#6 and shows a smallest compression, while the second shortest bond Ca(2)#0–O(2)#3 has a second smallest compression, with a turnover of these two bond lengths at ~5.5(1) GPa. The average bond length reveals a compressibility of 5.2(6) × 10−3 GPa−1 which is the highest among the three polyhedra.

Raman spectroscopy

Raman spectroscopy experiments were conducted on the same hedenbergite sample up to ~33 GPa. The Raman spectra of hedenbergite at 2.0(1), 17.4(1) and 32.8(1) GPa are shown in Fig. 6a. The peaks are fit with a Gaussian function and labeled ν 1–ν 13 (Fig. 6b). Our results show a good agreement with previous ambient study (Table 7) by Huang et al. (2000) and the data from University of Arizona RRUFF collection database. In our experiment, 13 Raman modes were observed between 200 and 1200 cm−1. Group theory analysis predicts 20 Raman active modes (Rutstein and White 1971). The reason why there are less observed modes in our experiments may be that some modes are too weak to be detected by our spectrometer or the spatial resolution is not sufficient to distinguish peaks that are very close to each other. Due to cation substitution in the M1 site, broadening of the M–O bend/stretch modes are expected (Gatta et al. 2005; Huang et al. 2000).

Fig. 6
figure 6

a Raman spectra of hedenbergite at 2.0(1), 17.4(1) and 32.8(1) GPa. b Raman peak positions of hedenbergite up to ~33 GPa

Full size image
Table 7 Assignment and frequencies (cm−1) of observed Raman modes up to 32.8(1) GPa with comparison of reference
Full size table

Discussion

Compression mechanism

Hedenbergite belongs to chain silicates, with single chains of SiO4 tetrahedron along the c axis, sandwiched by FeO6 octahedron and CaO8 polyhedron sheets, oriented in b–c plane. The SiO4 tetrahedron, FeO6 octahedron and CaO8 polyhedron constitute about 38 % volume of the unit cell. These polyhedra are compressed by ~15 % between 1.7(1) and 29.9(1) GPa.

In this study, the axial compressibilities of a, b and c are calculated to be 1.7(2), 4.9(5), 2.13(9) × 10−3 GPa−1. Zhang et al. (1997) adopted a different compressibility fitting algorithm, which causes an apparent discrepancy in values. Recalculating the axial compressibility of Zhang et al.’s (1997) with our method yields 2.3(9), 4(1) and 2.9(5) × 10−3 GPa−1 for a, b and c axis, respectively. Our results show similar pattern in the ratio of 1:2.83:1.22, as compared to 1:1.77:1.28 determined by the previous authors. In general, b shows the highest compressibility and c axis is more compressible than a. Brillouin scattering determined the elastic moduli C11, C22 and C33 to be 222(6), 176(5) and 249(5) GPa (Kandelin and Weidner 1988), which correlates well with our axial compressibility ratios. However, our results show that b axis is more compressible than indicated by the previous data. Diopside is a Ca, Mg end-member pyroxene, which is related to hedenbergite by substituting Fe2+ with Mg2+. We calculated the compressibility of diopside (Zhang et al. 1997) with our method and obtained 3(1), 4(1) and 3.0(5) × 10−3 GPa−1 for a, b and c axes. Similar to hedenbergite, also in diopside, the b axis has the highest compressibility. However, in contrast to hedenbergite, where c axis is more compressible than a, a and c have almost equal axial compressibility in diopside. The decrease in compressibility of c axis in diopside may be caused by the lower compressibility of MgO6 compared to FeO6 along the chain (Zhang et al. 1997). Different pressure range of data can also contribute to the discrepancy between our data and Zhang et al.’s (1997).

Figure 2 presents the confidence ellipses of this study and Zhang et al.’s (1997) data calculated by our method. We obtained K T0 = 131(4) GPa and \(K_{T0}^{\prime } = 3.8\left( 3 \right)\) for this study and K T0 = 118(3) GPa and \(K_{T0}^{\prime } = 4.2\left( 7 \right)\) for Zhang et al.’s (1997). Our results show higher bulk modulus K T0 but lower \(K_{T0}^{\prime }\). The discrepancy between these two data is likely due to the trade-off between K T0 and \(K_{T0}^{\prime }\), but could also be related to the compositional differences and different pressure ranges. For the diopside data (Zhang et al. 1997), the bulk modulus and its pressure derivative calculated with our method are K T0 = 108.3(3) GPa and \(K_{T0}^{\prime } = 5.4\left( 6 \right)\). Comparing hedenbergite and diopside, the latter has lower bulk modulus, which was also noted by Zhang et al. (1997).

The geometry of the SiO4 tetrahedral chain and pressure evolution of the O3–O3–O3 angle are shown in Fig. 7 and Table 6. The angle O3–O3–O3 (θ in Fig. 7a) is defined by the O3 atoms which form the two longest bonds with Si as shown by the red and magenta bonds in Fig. 7b, c (color of the bonds is consistent with Fig. 4a). The O3–O3–O3 angle is 163.7(2)° at 1.9(1) GPa and decreases, in general, as pressure increases which contributes to the axial compression along with the bond length decrease. The O3–O3–O3 angle remains almost unchanged from 5.9(1) to 12.7(1) GPa and even increases from 18.0(1) to 24.5(1) GPa; therefore, the decrease in Si–O3 bond lengths contributes mostly to the c axis compression in these pressure ranges.

Fig. 7
figure 7

a Polyhedral illustrations of the SiO4 tetrahedral chain, θ is O3–O3–O3 angle. b Illustration of bonds in SiO4 tetrahedron viewed along a axis. c Illustration of bonds in SiO4 tetrahedron viewed along b axis. Colors of the bonds are consistent with Fig. 4a

Full size image

Polyhedral distortion in hedenbergite

The polyhedral distortion can be described by angle variance and mean quadratic elongation parameters proposed by Robinson et al. (1971). The angle variance of the three polyhedra is shown in Fig. 8a. Angle variance is defined as \(\sigma^{2} = \mathop \sum \nolimits_{i = 1}^{n} \left[ {\left( {\theta_{i} - \theta_{0} } \right)^{2} /\left( {n - 1} \right)} \right]\), where θ i is the ith angle, θ 0 is the angle of ideal polyhedron of the same volume and n is the number of bonds. SiO4 tetrahedron shows a medium angle variance of ~30 and remains almost constant as pressure changes. FeO6 octahedron shows the smallest angle variance of ~14 at low pressure and starts to increase somewhere between 12.7(1) and 18.0(1) GPa and reaches ~33 at 29.9(1) GPa. The CaO8 polyhedron is already quite distorted at ambient conditions and has the largest angle variance of ~150; however, the distortion decreases linearly with pressure. It is interesting to notice that the drop in angle variance somewhere between 18.0(1) to 24.5(1) GPa can be the main factor that contributes to the decrease in CaO8 polyhedral compressibility starting from 18.0(1) GPa.

Fig. 8
figure 8

Angle variance (a) and quadratic elongation (b) of SiO4 (circle), FeO6 (triangle) and CaO8 (asterisk) in hedenbergite up to 29.9(1) GPa

Full size image

Mean quadratic elongation is defined as \(\left\langle \lambda \right\rangle = \mathop \sum \nolimits_{i = 1}^{n} \left[ {\left( {l_{i} /l_{0} } \right)^{2} /n} \right]\), where l 0 is the center-to-vertex distance of a regular polyhedron of the same volume, l i is the ith center-to-vertex distance and n is the number of bonds. In Fig. 8b, both SiO4 and FeO6 show a mean quadratic elongation >1. The mean quadratic elongation of SiO4 remains almost constant as pressure changes, just like in the case of the angle variance. However, the quadratic elongation of FeO6 starts to increase somewhere between 12.7(1) and 18.0(1) GPa, which indicates an increase in distortion. CaO8 has a mean quadratic elongation <1, which decreases gradually as pressure increases, also indicating increasing distortion.

Raman mode variation with pressure

Modes are assigned by comparing our results with previous ambient pressure data (Huang et al. 2000). Four modes (ν 1–ν 6) are assigned to M–O stretch or O–M–O bend (M = Ca2+, Fe2+, Mg2+, Mn2+), three modes (ν 7–ν 9) are assigned to O–Si–O bend, and four modes (ν 10–ν 13) are assigned to Si–O stretch. Two additional modes (ν 1*, ν 2*) are reported by Huang et al. (2000), but not in this study. The reason for this may be that these peaks are too weak or the difference in chemical composition and ordering causes them to be obscured. Our study also observed three modes ν 2, ν 6 and ν 9 that were not reported previously. These peaks are very weak at low pressure and as pressure increases, they start to diverge, as shown in Fig. 6. Such phenomenon was also observed by Gatta et al. (2005). The reason for the divergence of the two peaks may be the anisotropic compression making the crystal distorted, thus enhancing the difference between two peaks. In general, frequency increases as pressure increases, since compression generally strengthens bond, thus increasing the force constants. It is interesting to notice some discontinuities as frequencies increase. These phenomena are related to the discontinuous change in bond length and angles in the structure.

Conclusions

We reported results of high-pressure single-crystal X-ray diffraction and Raman spectroscopy experiments conducted on natural Ca, Fe end-member pyroxene (hedenbergite) up to ~33 GPa in diamond anvil cell. Unit cell parameters exhibit continuous decrease on compression. Axial compressibilities of hedenbergite up to ~30 GPa are calculated to be 1.7(2), 4.9(5) and 2.13(9) × 10−3 GPa−1, respectively, and show a highest compressibility of b and lowest compressibility of a. Our data show similar results in compressibilities with previous studies. Diopside shows a highest compressibility of b but almost equal compressibilities of a and c. The difference between hedenbergite and diopside may be caused by the different compressibilities of FeO6 and MgO6 polyhedra. Bulk modulus and its pressure derivate for hedenbergite are calculated to be 131(4) GPa and 3.8(3), respectively, by fitting third-order Birch–Murnaghan equation of state. The bulk modulus of hedenbergite is higher than that of diopside (108.3(3) GPa), but its pressure derivative is lower than that of diopside (5.4(6)).

Bond lengths are also discussed in this study. Generally, longer bonds show higher compressibilities except for some of the Si–O bonds. SiO4, the smallest polyhedron, shows the lowest compressibility. Angle and elongation distortions are observed in three polyhedra at high pressure. Thirteen vibrational modes are observed with Raman spectroscopy up to ~33 GPa. In general, mode frequencies increase as pressure increase.