Supply chain dynamics with assortative matching

Abstract

This article studies the evolutionarily stable equilibria of one-manufacturer and one-retailer supply chains. Each agent chooses to be either shareholder-oriented or stakeholder-oriented based on its own preference, then gives its pricing decision. Supply chains are formed by two types of matching processes: uniform random matching and assortative matching. Results indicate that, under uniform random matching, only one evolutionarily stable equilibrium exists, namely, the strict Nash equilibrium where both manufacturer and retailer choose shareholder strategy. Under assortative matching, the strict Nash equilibrium may not be evolutionarily stable under sign-compatible dynamics. The equilibrium where both manufacturer and retailer choose stakeholder strategy may be evolutionarily stable for certain values of the indices of assortativity. Furthermore, an interior equilibrium is observed with assortative matching, and the boundary equilibrium may be an evolutionarily stable equilibrium in some special cases.

1 Introduction

The classical model of the supply chain is one where firms are concerned singularly and solely with profits, and yet, we see that firms often devote considerable resources to their stakeholders (Lien 2002; Chai et al. 2018; Leppänen 2018). Yoshimori (1995) finds that 97% of managers in Japan, 83% in Germany, and 78% in France considered their stakeholders when making firm decisions. At the same time, being stakeholder-oriented does not always result in more profits in a competitive environment (Schaffer 1989). So, understanding how firms change their marketing objectives to respond to dynamic and complex changes in the markets is a question of academic and business relevance.

In the strategic interaction between firms, their strategy selection is a learning process, and over time the higher payoff strategies become more prevalent. The evolutionary theory defines the firm as a set of essential skills, gathered based on its learning ability. The evolutionary approach adopts the concept of bounded rationality; individuals and organizations have much to learn in a complex environment (Nicoleta et al. 2013). We study firms’ strategy choices in supply chains of manufacturers and retailers using an indirect evolutionary approach. In our model, firms choose between two types of strategies, and are either stakeholder-oriented or shareholder-oriented. The marketing objective of a shareholder-oriented firm is to maximize shareholder material payoff. On the other hand, a stakeholder-oriented firm seeks to maximize its material payoff plus a fraction of the interest of its stakeholders. Manufacturers and retailers are matched into pairs randomly.

A key feature of our model is that the random matching between manufacturers and retailers may not be uniform, which implies that the population of firms may not be well mixed. This non-uniform random matching is called assortative matching.¹ In assorttive matching, the probability of matching is determined by firm types and the proportions of types in the population. Assortative matching may arise for myriad reasons like geography, culture, socio-economics, preference, etc. (Alger and Weibull 2016). We extend the analysis in Bergstrom (2003) to a two-population game using the index of assortativity as a measure of the degree of assortment in the matching process. To the best of our knowledge, this article is the first attempt to investigate the index of assortativity for two populations.² The essence of biological evolution is that fitter genotypes increase relative to less fit genotypes. The economic analogue is that fitter strategies increase relative to less fit strategies in every population. This principle, Friedman (1991, 1998) calls compatibility. We show how matching rules determine the evolution of firm behavior with sign-compatible dynamics.

We present three main results in this paper. First, we discuss Stackelberg outcomes of manufacturers’ leadership in one single manufacturer-single retailer supply chain, and the stability of Nash equilibrium under uniform random matching. We find that the unique evolutionarily stable equilibrium is the strict Nash equilibrium under uniform random matching. Second, we completely analyze the evolutionary stability of equilibria in sign-compatible dynamics under assortative matching. We find that, unlike the case of uniform random matching, the strict Nash equilibrium may not be an evolutionarily stable equilibrium with assortative matching. The degree of assortment, or type correlation in the matching process in competition affects the selection of firms’ strategies, and may result in the non-selfish strategy being chosen. Our results are applicable in markets supporting different types of equilibria of firm types. Finally, we identity conditions under which positively and negatively assortative matchings exist in the long term.

The rest of the paper proceeds as follows. We review related literature in Section 2. Then, we introduce the basic model in Section 3 and present our analysis on assortative matching in Section 4. Section 5 concludes.

2 Related literature

In the stakeholder model of the corporation, a firm’s operations are guided by a broad sense of responsibility to its stakeholders, and not just shareholders. Being stakeholder oriented can lead to sustainable competitive advantage (Harrison et al. 2010) and long run advantage (Lusch and Laczniak 1987). While returns to being stakeholder-oriented can be determined by market structure (Kopel et al. 2014) and preference formation (Heifetz et al. 2007), stakeholder interests can be an opportunity for firm growth (Calabrese et al. 2013). Our work is related to the growing literature on the role of various stakeholder groups in firms; see, for example, Blinder (1993), Pagano and Volpin (2005), Lee (2008), Bhattacharya and Korschun (2008), Pascoe et al. (2009), and Boesso and Kumar (2009). Our contribution to this literature is in explicitly characterizing the nature of upstream and downstream stakeholder firms in a supply chain.

We also contribute to the literature on the dynamics of a supply chain with individual preferences. Previous research shows that a supply chain can be coordinated with preference for social responsibility, e.g., Amaeshi et al. (2008), Panda (2014), Goering (2012), Hua and Li (2008), Hsueh (2014), Ni et al. (2010), and Lau and Lau (2002). Xiao and Yu (2006a, b) study the evolutionarily stable strategy of retailers chosing between profit maximizing and revenue maximizing strategies. They find that four different strategy profiles may be evolutionarily stable under various conditions in a Cournot market. Chai et al. (2018) discuss evolutionary dynamics when firms choose to be either stakeholder-oriented or shareholder-oriented and analyze the evolution of firms’ preference for social responsibility using indirect evolutionary game theory. The evolution of firm behavior has also been studied by many others, such as, De Giovanni and Lamantia (2017), Blanco and Lozano (2015), and Yi and Yang (2017). These analyses consider games of uniform random matching. However, as Friedman and Sinervo (2016) point out, there are many other kinds of social structures in which matching departures from uniform random encounters affect payoffs and equilibrium. This paper adds to the literature by considering assortative matching in supply chains.

Assortative matching is a non-uniform random matching rule in which individuals of the same type match with each other more or less frequently than would be expected under uniform random matching. Shimer and Smith (2000) and Siow (2015) study the characteristics of assortative matching based on the results of Becker (1973), Legros and Newman (2007), and Schulhofer-Wohl (2006) discuss the dynamics of assortative matching in an economy where utility is not transferable between partners. Durlauf and Seshadri (2003) investigate the conditions under assortative matching that the total payoffs of agents can be maximized and show that characterizing distribution of evolution of types across agents is likely to be highly complex. Costinot et al. (2013), Sugita et al. (2016), and Bernard et al. (2018) show that the match between firms and suppliers in production and cross-border trade is positively assortative when production of final goods is sequential. Using data on US importers and their Indian exporters, Dragusanu (2014) finds that matching is affected by the proximity to the end user as well as demand elasticity. We contribute to this discussion by studying how assortative matching affects firms’ decisions to be shareholder-oriented or stakeholder-oriented.

We extend Bergstrom (2003) to a two-population game, and analyze the effect of the index of assortativity on firm behavior. To the best of our knowledge, this is the first attempt to investigate the impacts of the index of assortativity on firm behavior in a two-population game. Some scholars study the assortative matching with two populations in other contexts; Shirata (2012) studies the evolution of fairness in an ultimatum game with noise in learning under assortative matching and Atakan (2006) extends the results of Becker (1973) to heterogeneous agents and demonstrates that complementarities in joint production (supermodularity of the joint production function) lead to assortative matching. Further, Hoppe et al. (2009) consider two-sided markets with a finite number of agents on each side, and with two-sided incomplete information. While these previous articles study assortative matching with matching rules, our analysis is based on the index of assortativity. We find that the indices of assortativity determine whether the match between manufacturers and retailers is negatively assortative or positively assortative.

We contribute to the more recent literature on the application of the index of assortativity. Alger and Weibull (2013, 2016), and Alger (2010) study the evolution of preferences in a one-population game using the index of assortativity. Bergstrom (2013) shows the relationship between the index of assortativity and Wright’s F-statistic for a two-pool assortative matching process. Here, a member of any given population matches with either a member of an assortative pool comprised only of its own type or with a member from a random pool comprised of all who did not match in the assortative pool. Adding to this, our analysis compares two different populations (manufacturers and retailers), and further, each population has two types of agents. Matches are made across the population. Finally, we analyze the evolutionarily stable equilibrium under sign-compatible dynamics in supply chains using the index of assortativity, and compare the evolutionary dynamics of the supply chain under uniform random and assortative matchings.

3 The model

Consider an economic system that consists of two large populations: manufacturers and retailers. Manufacturers sell their product through an independent retailer in this vertically separated market by forming supply chains (Bonanno and Vickers 1988). A supply chain is a match between one manufacturer and one retailer. Each manufacturer/retailer chooses between two strategies of management: shareholder oriented (H/h) or stakeholder oriented (T/t). Manufacturer’s choices are denoted with uppercase letters and retailers’ choices with lowercase letters. We consider the simple set-up where every manufacturer sells to one retailer and every retailer sells the product of one manufacturer. We abstract away from the case of supply chains with multiple agents of any one population (like a manufacturer selling their product through multiple retailers) in order to focus on the impact of matching rules alone on firms’ choices. Our design can be interpreted as a setting with many markets that are separated by non-negligible distances, and each market is served by one manufacturer and one retailer (Xiao and Yu 2006a, b). The US-Mexican textile market is an example of a market that is broadly similar to our setting. Sugita et al. (2016) shows that the match between Mexican textile exporters and US textile importers is approximately one to one.

The time sequence of the game is as follows. First, manufacturers and retailers are matched according to a known matching rule to form single-manufacturer and single-retailer supply chains. Second, based on their strategy choice, each manufacturer chooses the wholesale price (w) that its retailer will face. Third, the retailer chooses its market price (p) based on the wholesale price and the retailer’s strategy choice. We assume there is a linear demand for the good sold through the supply chain.

$$ D = a - p. $$

(1)

The parameter a expresses market potential. Let manufacturers produce homogenous goods at marginal cost c, then a > c.

Because the manufacturer and the retailer each face two strategy choices, there are four possible strategy profiles for any given supply chain: (i) both manufacturer and retailer are stakeholder-oriented (Tt); (ii) both manufacturer and retailer are shareholder-oriented (Hh); (iii) manufacturer is shareholder-oriented and retailer is stakeholder-oriented (Ht); or, (iv) manufacturer is stakeholder-oriented and retailer is shareholder-oriented (Th). We denote a strategy by the second letter of the name of the strategy, and denote each strategy profile by a superscript; e.g., superscript Tt denotes case (i). We refer to Tt as symmetric stakeholder strategy profile, Hh as symmetric shareholder strategy profile, and Ht and Th as asymmetric strategy profiles.

The firms’ utilities are different according to their types. A shareholder-oriented firm seeks to maximize its own profit, which will then be distributed to its exogenously given shareholders. We model shareholder-oriented firms’ utilities with their profits. In our analysis, each firm’s stakeholder is its partner in the supply chain. We model stakeholder-oriented firms’ utilities by incorporating interests of all their stakeholders. Thus, a stakeholder-oriented manufacturer’s utility function incorporates a scaled version of its retailer’s profit function, and vice versa for a stakeholder-oriented retailer.

Next, we consider utility functions for each of the strategy choices in both the manufacturer and retailer populations. For tractability, all firms have the same information and are equally rational. Thus, all stakeholder-oriented firms share the same degree of concern for their stakeholders. Equations 2 and 3 are the utility functions of a stakeholder-oriented manufacturer and retailer, respectively.

$$ u_{m}(w)=(w-c)(a-p) + k_{m} (p-w)(a-p), $$

(2)

$$ u_{r}(p)=(p-w)(a-p) + k_{r} (w-c)(a-p). $$

(3)

In both equations, the first term is the firm’s profit function (π_m or π_r) and the second term is its stakeholder’s profit function. The parameter k_m ∈ (0,1) denotes manufacturers’ level of stakeholder concern. This parameter is how much the retailer’s profit factors into the manufacturer’s value-optimizing decision. Similarly, the parameter k_r ∈ (0,1) denotes the weight attached by the retailer to its stakeholder’s profit or retailers’ level of stakeholder concern.

In the supply chain, the manufacturer chooses its price first, and the retailer chooses its price in response. By backward induction, from the first-order condition of Eq. 3, we obtain the retailer’s best reply function as,

$$ p(w) = \frac{a+k_{r}c+w(1-k_{r})}{2}. $$

Substituting p(w) into the Eq. 2, the objective function of stakeholder-oriented manufacturer is

$$ u_{m}(w)=\frac{1}{4}[a-w+k_{r}(w-c)][(2-k_{m}k_{r})(w-c)+k_{m}(a-w)] $$

From the first order conditions,³ we obtain the wholesale price for stakeholder-oriented manufacturers as

$$w^{Tt} = \frac{a+c-2ck_{r}-k_{m}(a-c{k_{r}^{2}})}{(1-k_{r})[2-k_{m}(1+k_{r})]}.$$

Substituting w^Tt into the reaction function of the retailer, the retail price is

$$p^{Tt}=\frac{3a+c-k_{m}(2a+(a+c)k_{r})}{2[2-k_{m}(1+k_{r})]}.$$

Material payoffs of manufacturer and retailer with symmetric stakeholder strategy are, respectively,

$$\begin{array}{l} \pi_{m}^{Tt} = \frac{(a-c)^{2}(1-k_{m})(1-k_{m}k_{r})}{2(1-k_{r})[2-k_{m}(1+k_{r})]^{2}}, \\ \pi_{r}^{Tt} = \frac{(a-c)^{2}(1-k_{m}k_{r})[1-(3-k_{m})k_{r} + k_{m}{k_{r}^{2}}]}{4(1-k_{r})[2-k_{m} (1+k_{r})]^{2}}. \end{array}$$

Material payoffs for other strategy profiles can be derived similarly.

Table 1 captures the strategic interaction between manufacturer and retailer in the supply chain. In the bimatrix, we factor out $\frac {(a-c)^{2}}{2}$; rows denote manufacturer’s strategies and columns denote retailer’s strategies.

Table 1 Material payoff matrix

Comparing payoff functions in Table 1, it is straightforward to show that H is the dominant strategy for manufacturers, and h is the dominant strategy for retailers.⁴ Hence, (H, h) is a dominant strategy profile in the one shot game.

Furthermore, we analyze how material payoffs are affected by the firms’ level of stakeholder concern. As k_m and k_r are exogenous, firms observe the other population’s stakeholder concern with repeated interactions.

$$ \begin{array}{@{}rcl@{}} \frac{\partial \pi_{m}^{Tt}}{\partial k_{m}} &=& -\frac{(a-c)^{2}k_{m}(1-k_{r})}{2[(1-k_{r})^{2}[2-k_{m}(1+k_{r})]^{3}} < 0,\\ \frac{\partial \pi_{m}^{Tt}}{\partial k_{r}}&=&\frac{(a-c)^{2}(1-k_{m})[2-k_{m}(1+3k_{r})+{k_{m}^{2}}(1-k_{r}+2{k_{r}^{2}})]}{2(1-k_{r})^{2}[2-k_{m}(1+k_{r})]^{3}}>0.\\ \frac{\partial\pi_{r}^{Tt}}{\partial k_{r}}&=&-\frac{(a-c)^{2}(1-k_{m})[2-k_{m}k_{r}(5-k_{r})+{k_{m}^{2}}k_{r}(1+k_{r})]}{2(1-k_{r})^{2}[2-k_{m}(1+k_{r})]^{3}} <0 \quad(\text{as}\quad \pi_{r}^{Tt}>0), \\ \frac{\partial \pi_{r}^{Tt}}{\partial k_{m}}&=&\frac{(a-c)^{2}(1-k_{r})}{2[2-k_{m}(1+k_{r})]^{3}}>0. \end{array} $$

(4)

for all 0 < k_m < 1 and 0 < k_r < 1.

Both manufacturers and retailers face decreasing material payoffs form being more stakeholder-oriented. At the same time, both firm types enjoy higher material payoffs when paired with a stakeholder-oriented firm in the supply chain. From the material payoff equations, we have $\pi _{m}^{Tt} + \pi _{r}^{Tt}>\pi _{m}^{Hh} + \pi _{r}^{Hh}$.⁵ Channel material payoff, modeled as the sum of manufacturer and retailer material payoffs, is maximized when both manufacturer and retailer pursue a stakeholder strategy. However, it is in each agent’s own interest to be shareholder-oriented. Thus, a dilemma emerges in this game. Many scholars explain the evolution of non-selfish behavior in the Prisoners’ dilemma between kin. We are trying to explain this social dilemma using the matching rules between firms.

Up until now, our analysis was based on the assumption that the matching between manufacturers and retailers is uniform random, which indicates the distribution of firms is uniform. However, because the reasons of the geography, culture, socio-economics, preference, etc., the populations of manufacturers and retailers may not be well mixed in reality, which implies the random matching may not be uniform. We call this non-uniform random matching assortative matching. The Nash equilibria and evolutionary dynamics of the game under assortative matching are the focus in the rest of the paper.

4 Assortative matching

The index of assortativity, represents the degree of assortment, or type correlation, in the matching process. Next, we deduce the indices of assortativity of two populations: manufacturers and retailers.

4.1 Index of assortativity

Bergstrom (2003) defines the index of assortativity for a one-population, two-strategy game. It is the difference in probability of being matched with a given strategy-type if the agent is also of this strategy-type and the probability of being matched with that strategy-type if the agent is of the second strategy-type. We extend this concept to a two-population game by considering a game where agents’ type determines probability of encountering an agent of a specific type in the supply chain. Suppose that x is the proportion of stakeholder-oriented manufacturers, and y is the proportion of stakeholder-oriented retailers. Let p_m(x, y) be the conditional probability of a T-manufacturer encountering a t-retailer, and q_m(x, y) be the conditional probability of an H-manufacturer encountering a t-retailer (Fig. 1).⁶ We denote p_r(x, y) as the conditional probability of a t-retailer encountering a T-manufacturer, and q_r(x, y) as the conditional probability of an h-retailer encountering a T-manufacturer (Fig. 2).⁷

Fig. 1

Matching probability in manufacturer population: p_m = Pr(T meets t), q_m = Pr(H meets t)

Fig. 2

Matching probability in manufacturer population: p_r = Pr(t meets T), q_r = Pr(h meets T)

We define the index of assortativity for our two-population, two-strategy game as the difference in probability of a particular type of firm (either shareholder-oriented or stakeholder-oriented) matching with a firm of the same type and that of matching with a firm of the other type. We consider how strategy choices are affected by the probabilities of encountering a manufacturer or retailer of a specific type through a game of assortative matching. In the following analysis, we suppress arguments of the probability functions to simplify notation.

Let us define α₁ to be the difference between the probability of a T-manufacturer encountering a t-retailer and an H-manufacturer encountering an h-retailer. Thus, based on Fig. 1, we have

$$ \alpha_{1}=\text{Prob}(t|T)-\text{Prob}(t|H)=p_{m}(x,y)-q_{m}(x,y). $$

The difference in probability of an H-manufacturer encountering an h-retailer and a T-manufacturer encountering an h-retailer is given by

$$ \alpha_{2} = \text{Prob}(h|H)-\text{Prob}(h|T)=1-q_{m}(x,y) - (1-p_{m}(x,y))=p_{m}(x,y) - q_{m}(x,y). $$

Thus, for both types of retailers, the difference in probability of encountering a manufacturer of one’s own type and a manufacturer of the other type are identical. We write α₁ = α₂ = α.

Similarly, let us define β₁ to be the difference in probability between a t-retailer encountering a T-manufacturer, and an h-retailer encountering a T-manufacturer. Thus, based on Fig. 2, we have

$$ \beta_{1} = \text{Prob}(T|t)-\text{Prob}(T|h)=p_{r}(x,y) - q_{r}(x,y). $$

The difference in probability between an h-retailer encountering a manufacturer of the same type, and a t-retailer encountering an H-manufacturer is

$$ \beta_{2} =\text{Prob}(H|h)-\text{Prob}(H|t)=1- q_{r}(x,y) - (1-p_{r}(x,y)) = p_{r}(x,y) - q_{r}(x,y). $$

Again, for both types of manufacturers, the difference in probability of encountering a retailer of one’s own type and a retailer of the other type are identical. We write β₁ = β₂ = β.

Within each population, the difference in probability of one encountering one’s own type and an agent of the other type encountering one’s own type in the supply chain is equivalent. This result parallels that of a one-population, two-strategy game in Bergstrom (2003) where the difference between the probability of two agents of the same type encountering each other and the probability of two different types matching with each other are equivalent across both strategy-choices in the population.

In the rest of our analysis, we define α = p_m(x, y) − q_m(x, y) as the index of assortativity for manufacturers, and β = p_r(x, y) − q_r(x, y) as the index of assortativity for retailers.

Table 2 captures the combination of pairing and pairing probability under assortative matching. The rows define manufacturer’s conditional probabilities and columns define retailers’ conditional probabilities of matching.

Table 2 The conditional probability and pairs matrix

We assume equal sizes of manufacturer and retailer populations of N.⁸ We characterize the number of supply chains in which a T-manufacturer (H-manufacturer) matches with a t-retailer as N_Tt(N_Ht), and the number of supply chains in which a T-manufacturer (H-manufacturer) matches with an h-retailer as N_Th(N_Hh). Similarly, we define the number of supply chains in which a t-retailer (h-retailer) matches with a T-manufacturer as N_tT(N_hT), and the number of supply chains in which a t-retailer (h-retailer) matches with an H-manufacturer as N_tH(N_hH).

The number of supply chains of T-t pairings (N_Tt or N_tT) formed in a population of size N is the probability that a T-manufacturer meets with a t-retailer (p_m(x, y)) times the number of T-manufacturers (x ⋅ N), so N_Tt = N ⋅ x ⋅ p_m(x, y). Likewise, the number of supply chains in which a t-retailer encounters a T-manufacturer is N_Tt = N ⋅ y ⋅ p_r(x, y). The necessary balancing condition for the number of pairwise matches between T-manufacturers and t-retailers is N_Tt=N_tT, so we have x ⋅ p_m(x, y) = y ⋅ p_r(x, y). Similarly, the following equations hold. N_Th=N_hT, N_Ht=N_tH, N_Hh=N_hH.

Thus, we have Eqs. 5–8.

$$ \begin{array}{@{}rcl@{}} x \cdot p_m(x,y)&=&y \cdot p_r(x,y), \end{array} $$

(5)

$$ \begin{array}{@{}rcl@{}} x[1-p_m(x,y)] &=& [1-y]q_r(x,y), \end{array} $$

(6)

$$ \begin{array}{@{}rcl@{}} {[1-x]q_m(x,y)}&=&y[1-p_r(x,y)], \end{array} $$

(7)

$$ \begin{array}{@{}rcl@{}} {[1-x][1-q_m(x,y)]}&=&[1-y][1-q_r(x,y)]. \end{array} $$

(8)

The probabilities of encounter can thus be written as⁹

$$ \begin{array}{@{}rcl@{}} &&p_{m}(x,y)=y + \alpha[1-x],\\ &&q_{m}(x,y)=y-\alpha x,\\ &&p_{r}(x,y)=x+ \beta[1-y],\\ &&q_{r}(x,y)=x-\beta y. \end{array} $$

Next, we analyze the Nash equilibria of the Stackelberg models under assortative matching, and use an example to illustrate the evolution results of games of uniform random and assortative matching. We will then use these probabilities to analyze the evolution of firm behavior. The firm’s profitability determines whether it continues to operate with its current strategy as the market evolves. In the initial sub-game, firms choose prices based on their preferences alone. In the repeated interactions of later sub-games, firms dynamically adjust their strategies for higher material payoffs. While readjusting their strategies, firms optimize profitability through preference revision—this is the core idea of the indirect evolutionary approach (Güth and Yaari 1992).

4.2 The Nash equilibrium and an example

The unique Nash equilibrium under uniform random matching is the dominant strategy (H, h). In analyzing Nash equilibria under assortative matching, we use the material payoff matrix reproduced in Table 1 as below.

The population share weighted fitness functions for each population using the probability of encounters (as Table 2) and the strategy payoffs are

$$ \begin{array}{@{}rcl@{}} {w_{m}^{T}} &=& p_{m}(x,y)\pi_{m}^{Tt} + [1-p_{m}(x,y)]\pi_{m}^{Th}, \\ {w_{m}^{H}} &=& q_{m}(x,y)\pi_{m}^{Ht} + [1-q_{m}(x,y)]\pi_{m}^{Hh}, \\ {w_{r}^{t}} &=& p_{r}(x,y)\pi_{r}^{Tt} + [1-p_{r}(x,y)]\pi_{r}^{Ht},\\ {w_{r}^{h}}&=& q_{r}(x,y)\pi_{r}^{Th} + [1-q_{r}(x,y)]\pi_{r}^{Hh}. \end{array} $$

Substituting the expression of p_m, q_m, p_r, and q_r, we have

$$ \begin{array}{@{}rcl@{}} {w_{m}^{T}} &=& [y+\alpha (1-x)]\pi_{m}^{Tt} + [1-y-\alpha (1-x)]\pi_{m}^{Th}, \\ {w_{m}^{H}} &=& (y-\alpha x)\pi_{m}^{Ht} + (1-y +\alpha x)\pi_{m}^{Hh}, \\ {w_{r}^{t}} &=& [x+\beta (1-y)]\pi_{r}^{Tt} + [1-x-\beta (1-y)]\pi_{r}^{Ht},\\ {w_{r}^{h}}&=& (x-\beta y)\pi_{r}^{Th} + (1-x+\beta y)\pi_{r}^{Hh}. \end{array} $$

Unlike the situation with uniform random matching, the fitness of any strategy depends on the indices of assortativity α and β.

To characterize fitness of any one strategy, we look at the payoff advantage of that strategy. The payoff advantage in choosing to be stakeholder-oriented, for example, for manufacturers and retailers are, respectively,

$$ \begin{array}{@{}rcl@{}} {\varDelta} w_{m} &=&(y-\alpha x)(\pi_{m}^{Tt}+\pi_{m}^{Hh}-\pi_{m}^{Ht}-\pi_{m}^{Th})+(\pi_{m}^{Th}-\pi_{m}^{Hh})+\alpha(\pi_{m}^{Tt}-\pi_{m}^{Th}),\\ {\varDelta} w_{r} &= &(x-\beta y)(\pi_{r}^{Tt}+\pi_{r}^{Hh}-\pi_{r}^{Ht}-\pi_{r}^{Th})+(\pi_{r}^{Ht}-\pi_{r}^{Hh})+\beta(\pi_{r}^{Tt}-\pi_{r}^{Ht}). \end{array} $$

The proportions adopting the various possible strategies remain unchanged in a population when no agent can do any better by switching to another strategy, i.e., it has to be the case that the payoff advantage to either strategy is 0 (so, Δw_m = 0, Δw_r = 0). Consequently, the optimal shares of manufacturers and retailers choosing to be stakeholder-oriented are given by,

$$ \begin{array}{@{}rcl@{}} x^{*} =\frac{\beta[\pi_{m}^{Hh}-\pi_{m}^{Th}+\alpha(\pi_{m}^{Th}-\pi_{m}^{Tt})]}{{\varDelta} M (1-\alpha\beta)}+\frac{\pi_{r}^{Hh}-\pi_{r}^{Ht}+\beta(\pi_{r}^{Ht}-\pi_{r}^{Tt})}{{\varDelta} R(1-\alpha\beta)}, \end{array} $$

$$ \begin{array}{@{}rcl@{}} y^{*} =\frac{\pi_{m}^{Hh}-\pi_{m}^{Th}+\alpha(\pi_{m}^{Th}-\pi_{m}^{Tt})}{{\varDelta} M (1-\alpha\beta)}+\frac{\alpha[\pi_{r}^{Hh}-\pi_{r}^{Ht}+\beta(\pi_{r}^{Ht}-\pi_{r}^{Tt})]}{{\varDelta} R(1-\alpha\beta)}. \end{array} $$

Here ${\varDelta } M=\pi _{m}^{Hh}+\pi _{m}^{Tt}-\pi _{m}^{Ht}-\pi _{m}^{Th}, {\varDelta } R=\pi _{r}^{Hh}+\pi _{r}^{Tt}-\pi _{r}^{Th}-\pi _{r}^{Ht}$.

Let a = 4, c = 1, k_m = 0.55, k_r = 0.25, α = 0.4104, β = 0.8916, we get the payoff matrix in the one shot game as follows, based on Table 3:

$$ \begin{array}{@{}rcl@{}} \begin{pmatrix} 1.35184, 0.633673 & &0.963139, 1.07015 \\ 1.5, 0.1875 && 1.125, 0.5625 \\ \end{pmatrix} \end{array} $$

Table 3 The material payoff matrix in symbolic form

Obviously, the shareholder-oriented strategy dominates the stakeholder-oriented strategy for both manufacturers and retailers; the unique Nash equilibrium is (H, h) (point (0,0)) under uniform random matching. However, with assortative matching, there exists a mixed Nash equilibrium (0.8252,0.5095). We present the phase portrait of the replicator dynamics to illustrate evolutionary dynamics with each type of matching.

The continuous replicator dynamics of this game can be represented as

$$ \begin{array}{@{}rcl@{}} \dot{x} &=& x(1-x){\varDelta} w_{m}, \\ \dot{y} &=& y(1-y) {\varDelta} w_{r}. \end{array} $$

(9)

For these parameter values as above, the replicator equations under assortative matching are

$$ \begin{array}{@{}rcl@{}} \dot{x} = 0.0056x(1-x) (2.4367y-x-0.4161),\\ \dot{y}=0.0615y(1-y)(0.371-x+0.8916y). \end{array} $$

(10)

The replicator equations under uniform random matching are

$$ \begin{array}{@{}rcl@{}} \dot{x} &=& 0.0137x(1-x) (y-11.8167),\\ \dot{y}&=&-0.0615y(1-y)(6.0994+x). \end{array} $$

(11)

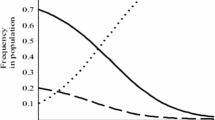
The evolutionary dynamics with uniform random matching are shown in Fig. 3, where the dominant strategy profile (H, h), i.e., the point (0,0), is evolutionarily stable. Figure 4 represents the phase portrait of Eq. 10. The strategy profile (H, h) is not supported under assortative matching in the long run. The point (1, 1), where both manufacturers and retailers choose stakeholder strategy, is evolutionarily stable. All agents, thus, eventually choose to be stakeholder-oriented in this game of assortative matching. These results imply that the non-selfish strategy may be chosen by firms in the evolutionary process under assortative matching even the selfish strategy is the dominant strategy under uniform random matching.

Fig. 3

Phase portrait of Eq. 11 with uniform random matching

Fig. 4

Phase portrait of Eq. 10 with assortative matching

However, the evolutionary dynamics of both pure strategy (H, h), (H, t), (T, h), (T, t), and the mix strategy (x^∗, y^∗) ∈ (0,1) × (0,1) under assortative matching is determined by parameter values.

4.3 Sign-compatible dynamics

A given payoff/fitness function can give rise to a variety of dynamics, but evolutionary dynamics are not completely arbitrary. The evolutionarily critical aspect of a strategic interaction is not some subjective utility but rather the objective material payoff/fitness it awards to a strategy. Therefore, for any dynamic compatible with a properly specified payoff/fitness function, fitter strategies should increase relative to less fit strategies. Friedman (1991) calls this dynamics as compatible dynamics.

Consider a set of interacting populations, index k = 1,⋯ , K. A member of each population k has available a finite number of strategies indexed i = 1,⋯ , N. Any point s^k in the N-simplex $S^{k}=\{x=(x_{1},\cdots , x_{N}): x_{i}\geq 0, \sum x_{i}=1\}$ represents the fraction of population k. The Cartesian product of K copies of the simplex, S = S¹ ×⋯ × S^K is the state space. Let $f: S\times S\rightarrow R^{K}$ be a fitness function. The relative fitness function for population k, $\hat {f}^{k}(s): S \rightarrow R^{N}$ is given by: $\hat {f}_{i}^{k}(s)={f_{i}^{k}}(s)-\sum {s_{i}^{k}}{f_{i}^{k}}(s), f=(f^{1}, \cdots , f^{k}), i =1,\cdots , N$. $G: S \rightarrow R^{NK}$. Compatibility implies some relationship between a payoff/fitness function f and the corresponding dynamics $\dot {s}=G(s)$, where $\dot {s}=(\dot {s}^{1}, \cdots , \dot {s}^{K})$ is the rate of change in the state and G(s) is a specified vector valued function, s ∈ S.

Definition 1

(Friedman 1991). Dynamics $\dot {s} = G(s)$ are admissible if

• (1) G is continuous and piecewise differentiable on S,

• (2) ${\sum }_{i=1}^{N} {G_{i}^{k}}(s) =0$ for all s ∈ S and k = 1,⋯ , K,

• (3) ${s_{i}^{k}} =0$ implies ${G_{i}^{k}}(s) =0$.

With sign-compatible dynamics, the change of $\dot {s}$ given by G have the same signs as relative fitness.

Definition 2

(Joosten 1996). Evolutionary dynamics are sign-compatible if they are admissible and for all i = 1,⋯ , N and k = 1,⋯ , K satisfy: $sign {G_{i}^{k}}(s) = sign \hat {f}_{i}^{k}(s)$ whenever s_i > 0.

In our models, firms’ earnings are related to their preferences regarding marketing objectives. Firms decide on their prices based on their preferences, and then later adjust these prices in response to competitors’ actions. As the market evolves, firms with high earnings eliminate those with lower earnings. Through this kind of repeated dynamic adjustment, firms make more material payoffs by revising preferences. For example, if stakeholder-oriented manufacturers earn higher material payoffs, then we would see the proportion of stakeholder-oriented manufacturers increasing over time. Thus, the evolution of manufacturers’ and retailers’ behavior satisfy the following sign-compatible dynamics.

$$ \begin{array}{c} \frac{\text{d}x}{dt}=G_{m}(x,y);\\ \frac{\text{d}y}{dt}=G_{r}(x,y). \end{array} $$

(12)

where signG_i(x, y) = signΔw_i(x, y) whenever (x, y) > (0,0), i = m, r.

The state (x, y) ∈ [0,1] × [0,1] is called an equilibrium/fixed point of Eq. 12 if G_m(x, y) = 0 and G_r(x, y) = 0; an equilibrium (x, y) ∈ (0,1) × (0,1) is called an interior equilibrium; and an equilibrium (x, y) ∈ (0,1) ×{0,1} or (x, y) ∈{0,1}× (0,1) is called a boundary equilibrium. Correspondingly, a strategy profile at the equilibrium is called an equilibrium strategy profile, and that at the interior equilibrium is the mixed strategy.

We use the following definition of an evolutionarily stable equilibrium to analyze the evolutionary dynamics of Eq. 12.

Definition 3

(Joosten 1996). The equilibrium (x, y) ∈ [0,1] × [0,1] of Eq. 12 is an evolutionarily stable equilibrium if and only if there exists an open neighborhood U ⊂ [0,1] × [0,1] of (x, y) satisfying

$$ ((x, y) - (\tilde{x}, \tilde{y}))(G_{m}(x,y), G_{r}(x, y))^{\text{T}} > 0\quad \text{for all}\quad (\tilde{x}, \tilde{y})\in U\backslash \{(x, y)\}.$$

Let,

$$ \begin{array}{@{}rcl@{}} A_{1}&=& 4 -4k_{m} + 3{k_{m}^{2}} - {k_{m}^{3}} -4k_{m} k_{r} + {k_{m}^{2}} k_{r} + {k_{m}^{2}} {k_{r}^{2}} > 0, \\ A_{2} &=& 8-4k_{m} - 12 k_{m} k_{r} + 7 {k_{m}^{2}} k_{r} - {k_{m}^{3}} k_{r} + 3{k_{m}^{2}} {k_{r}^{2}} - {k_{m}^{3}} {k_{r}^{2}} > 0, \\ A_{3} &=& (2-k_{m}-k_{m} k_{r})^{2}>0, \\ A_{4} &=& k_{m}(4-k_{m}-k_{m} k_{r})>0,\\ B_{1}&=&\frac{2A_{3}k_{r}}{A_{4}(1-k_{r})^{2}},\quad B_{2}=\frac{A_{3}{k_{m}^{2}}(1-k_{r})}{4A_{1}k_{r}(1-k_{m})},\\ B_{3}&=&\frac{{k_{m}^{2}}(1-k_{r})}{k_{r}(2-k_{m})^{2}},\quad B_{4}=\frac{4A_{2}k_{r}(1-k_{m})}{A_{4}(2-k_{m})^{2}(1-k_{r})^{2}},\\ B_{5}&=&\frac{2k_{r}(2-k_{m})^{2}}{k_{m}(4-k_{m})(1-k_{r})}, \quad B_{6}=\frac{{k_{m}^{2}}(2-k_{m})^{2}(1-k_{r})^{2}}{4A_{1}k_{r}(1-k_{m})},\\ B_{7}&=&\frac{{k_{m}^{2}}(1-k_{r})^{2}}{A_{3}k_{r}},\quad B_{8}=\frac{4A_{2}k_{r}(1-k_{m})}{A_{3}k_{m}(4-k_{m})(1-k_{r})}. \end{array} $$

The following results hold.

Proposition 1

The interior equilibrium (x^∗, y^∗) is a repellor, the stability of the other equilibria as follows:

• (1) When β < B₁ and α < B₂, the point (0, 0) is an evolutionarily stable equilibrium;

• (2) when α > B₃ and β < B₄, the point (1, 0) is an evolutionarily stable equilibrium;

• (3) when β > B₅ and α < B₆, the point (0, 1) is an evolutionarily stable equilibrium;

• (4) when α > B₇ and β > B₈, the point (1, 1) is an evolutionarily stable equilibrium.

For relatively small values of the index of assortativity, the probability of encountering an agent of one’s own type and the probability of encountering an agent of the other type are quite close. Our analysis shows that both types of firms choose to be shareholder-oriented when the index of assortativity is small (Proposition 1(1)). Thus, though firms benefit more from a Tt match than an Hh match, the relatively comparable probabilities of matching with a firm of one’s own type and a firm of the other type deters firms from being stakeholder-oriented. This result is presented in Fig. 5, where the parameter values are k_m = 0.2, k_r = 0.3, α = − 0.03, β = 0.03. With these low values of the indices of assortativity, both manufacturers and retailers choose to be shareholder-oriented in the long run.

Fig. 5

Phase portrait of Eq. 12 with Case (1)

The asymmetric strategy profile in which the manufacturer chooses to be shareholder-oriented (stakeholder-oriented) and the retailer chooses to be stakeholder-oriented (shareholder-oriented) is an evolutionarily stable equilibrium if the index of assortativity for the manufacturer is relatively low (relatively high), and the index of assortativity for the retailer is relatively high (relatively low). When the manufacturer’s index of assortativity is relatively low, the manufacturer’s game is similar to the game of uniform random matching. With a relatively high index of assortativity, a retailer is more likely to be matched with a manufacturer of its own type than that of the other type. The payoff functions show that the retailer benefits more from a symmetric match than an asymmetric match. Thus, with a relatively high index of assortativity, retailers choose to be stakeholder-oriented. Figures 6 and 7 show evolutionarily stable asymmetric strategy profiles when parameter values satisfy conditions in Proposition 1(2) and 1(3). The parameter values we use in these figures are k_m = 0.02, k_r = 0.1, α = 0.13, β = 0.3 and k_m = 0.5, k_r = 0.1, α = 0.55, β = 0.5, respectively.

Fig. 6

Phase portrait of Eq. 12 with Case (2)

Fig. 7

Phase portrait of Eq. 12 with Case (3)

When the probability of matching with one’s own type is higher than the probability of matching with the other type (Proposition 1(4)), firms choose to be stakeholder-oriented. This result is presented in Fig. 8, where parameter values are k_m = 0.4, k_r = 0.1, α = 0.6, β = 0.5. In our analysis, a = 4 and c = 1. The dynamics stability of the strategy profiles are generalizable beyond the values of a and c we present here.

Fig. 8

Phase portrait of Eq. 12 with Case (4)

In reality, we see that market often supports multiple types of matches between firms. For instance, some markets may reach an equilibrium that supports two evolutionarily stable matches, with some supply chains being comprised entirely of shareholder-oriented firms and others being comprised of shareholder-oriented upstream firms and stakeholder-oriented downstream; alternatively, a market could reach an evolutionarily stable profiles with some wholly shareholder-oriented supply chains and other supply chains with stakeholder-oriented upstream firms and shareholder-oriented downstream firms. What would cause markets to evolve into one of these and not the other type of market? While a detailed analysis of this question is beyond the scope of the current article, our succinct explanation is the initial conditions matter. These conditions include political, historical, or environmental factors, among many others. We use initial parameter values to describe aggregate initial conditions. In Corollary 1, we describe this simplified explanation by showing that for various initial parameter values, a game of assortative matching can support multiple types of supply chains in equilibrium.

Corollary 1

• (1) When B₃ < α < B₂ and $\beta < \min \limits \{B_{1}, B_{4}\}$, both (0, 0) and (1, 0) are evolutionarily stable equilibria;

• (2) when B₅ < β < B₁ and $\alpha < \min \limits \{B_{2}, B_{6}\}$, both (0, 0) and (0, 1) are evolutionarily stable equilibria;

• (3) when B₃ < α < B₆ and B₅ < β < B₄, both (1, 0) and (0, 1) are evolutionarily stable equilibria;

• (4) when B₈ < β < B₄ and $\alpha > \max \limits \{B_{3}, B_{7}\}$, both (1, 0) and (1, 1) are evolutionarily stable equilibria;

• (5) when $\beta > {\max \limits } \{B_{5}, B_{8}\}$ and B₇ < α < B₆, both (0, 1) and (1, 1) are evolutionarily stable equilibria;

• (6) when $B_{3} < \alpha <{\min \limits } \{B_{2}, B_{6}\}$ and $B_{5} < \beta < \min \limits \{ B_{1}, B_{4}\}$, (0, 0), (0, 1) and (1, 0) are evolutionarily stable equilibria;

• (7) when ${\max \limits } \{ B_{3}, B_{7} \} < \alpha < B_{6} \text {and} {\max \limits } \{B_{5}, B_{8} \} < \beta < B_{4}$, (1, 0), (0, 1) and (1, 1) are evolutionarily stable equilibria.

Figures 9 and 10 present two of the phase portraits of differential equation (12) with multiple evolutionarily stable strategy profiles. Figure 9 shows that for certain initial conditions, manufacturers’ index of assortativity determines whether manufacturers are shareholder-oriented or stakeholder-oriented in the evolutionarily stable profiles. For the range of initial conditions, identified in Corollary 1(2), retailers may be shareholder-oriented or stakeholder-oriented in the evolutionarily stable profiles, although all manufacturers are shareholder-oriented, as depicted in Fig. 10. The other dynamics of games with evolutionarily stable strategy profiles are shown in Appendix B.

Fig. 9

Phase portrait emerging from Corollary 1(1) when k_m = .8, k_r = .33, α = .95, β = .53

Fig. 10

Phase portrait emerging from Corollary 1(2) when k_m = .5, k_r = .3, α = .39, β = .7

Thus, when compared to a game of uniform random matching, the dynamics of the game under assortative matching yield the more interesting result of multiple evolutionarily stable strategy profiles.

Furthermore, we consider the special case where both manufacturers and retailers are equally concerned about their stakeholders’ utility, i.e., k_m = k_r. We find that, in this case, retailers adopt shareholder-oriented strategy in the long run. Manufacturers’ long-run strategy choice is determined by the index of assortativity in their population.

Corollary 2

When 0 ≤ k_m = k_r = k < 1/3, (0, 1) and (1, 1) are unstable; Furthermore, we have the following results:

• (1) If $\alpha < \hat {\alpha _{1}} = \frac {k(1-k)(2+k)^{2}}{4(4-k^{2}-k^{3})}$, (0, 0) is an evolutionarily stable equilibrium;

• (2) If $\alpha > \hat {\alpha _{2}}= \frac {k(1-k)} {(2-k)^{2}}$, (1, 0) is an evolutionarily stable equilibrium;

• (3) If $\hat {\alpha _{1}}\leq \alpha \leq \hat {\alpha _{2}}$, the boundary equilibrium (x^∗,0) is an evolutionarily stable equilibrium, where $x^{*}=\frac {\alpha (16-4k^{2}+4k^{3})-k(4-k+3k^{2})}{\alpha k^{2}(4-4k-k^{2})}$.

According to Corollary 2, if both manufacturers and retailers care about their stakeholders moderately and with comparable magnitudes (0 ≤ k_m = k_r = k < 1/3), retailers adopt a shareholder-oriented strategy in the long term. If the index of assortativity for manufacturer is small $(\alpha < \hat {\alpha _{1}})$, the strategy profile where both manufacturers and retailers choose a shareholder-oriented strategy is evolutionarily stable; when the index of assortativity for manufacturer is relatively high $(\alpha > \hat {\alpha _{2}})$, manufacturers choose to be stakeholder-oriented and retailers choose to be shareholder-oriented in the long run. When manufacturers’ index of assortativity is moderate ($\hat {\alpha _{1}}\leq \alpha \leq \hat {\alpha _{2}}$), some manufacturers use a stakeholder-oriented strategy, and the others use a shareholder-oriented strategy. Both types of manufacturers coexist in the long run (Fig. 11). Furthermore, we find that the degree to which manufacturer and retailer care about their stakeholders increases the critical value at which the manufacturer population switches to shareholder-oriented strategy.¹⁰

Fig. 11

Phase portrait of Corollary 2(3) with k = 0.32, a = 11, c = 1, α = 0.077, β = 0.2

As Proposition 1, Corollaries 1 and 2 show, the assortative matches may be symmetric, like (T, t),(H, h), or asymmetric, like (T, h),(H, t). Positively assortative matches refer to evolutionarily stable symmetric matches, and negatively assortative refer to evolutionarily stable asymmetric matches. We relate the indices of assortativity to these two types of matches and analyze the conditions that the assortative matching is positive or negative.

Proposition 2

The indices of assortativity for the manufacturer and retailer determine whether the match is positively assortative or negatively assortative; specifically,

• (1) when α > B₃ and β < B₄, or β > B₅ and α < B₆, there exists a negatively assortative matching in the long term;

• (2) when α < B₂ and β < B₁, or α > B₇ and β > B₄, there exists a positively assortative matching in the long term.

Proposition 2 provides sufficient conditions for positively assortative and negatively assortative matchings. Positively assortative matching occurs only when the indices of assortativity for both the manufacturer and the retailer populations are concurrently high or low enough. In other words, manufacturers and retailers of the same type are more likely to match with each other when the degree of type correlation in the matching is high or low enough for both populations. The match is negatively assortative when manufacturers’ (retailers’) index of assortativity is high and the index of assortativity for retailers (manufacturers) is moderate or low.

5 Conclusions

In this article, we analyze the effect of assortative matching on the evolution of firms’ strategies. Our primary contribution is using the indices of assortativity to establish evolutionary stability in a two-population, two-strategy game. In our basic model, we find that a firm’s material payoff increases with its stakeholder’s concern for it and decreases with the firm’s own concern for its stakeholder. These is no mixed Nash equilibrium, and the unique evolutionarily stable equilibrium is for both manufacturers and retailers to choose shareholder-oriented strategy with uniform random matching. This is also the dominant strategy for manufacturers and retailers.

With assortative matching, the game has a mixed Nash equilibrium. However, the mixed Nash equilibrium is unstable. All four pure strategy profiles considered in this article may be evolutionarily stable under sign-compatible dynamics for different values of the indices of assortativity. The symmetric shareholder strategy is evolutionarily stable when the indices of assortativity for both retailers and manufacturers are low. When the index of assortativity for manufacturers is high (moderate), and the index of assortativity for retailers is moderate (high), the asymmetric strategy profiles are evolutionarily stable, and matching is negatively assortative. The symmetric stakeholder strategy profile is evolutionarily stable and the match is positively assortative when the indices of assortativity for both manufacturers and retailers are concurrently high (or low) enough. In the special case where all firms (manufacturers and retailers) exhibit the same level of stakeholder concern, all retailers use shareholder-oriented strategy, some manufacturers choose stakeholder-oriented strategy in evolutionarily stable equilibrium.

Our article shows that the dynamics of the game is more varied under assortative matching than uniform random matching. The strict Nash equilibrium may not be an evolutionarily stable equilibrium, and the non-selfish behavior may be evolutionarily stable under assortative matching. Future work will consider games with continuous range of parameters of stakeholder concern, and games in which the probability of assortative matching is a specific function of the share of stakeholder-oriented manufacturers and retailers. Furthermore, we are also interested in analyzing the effect of stakeholder-oriented strategy on double marginalization in the channel, and in incorporating uncertainty into our model in the future. This is a more realistic setting and it would be interesting to study the impact of uncertainty, such as demand shocks or cost shocks, on the stability of equilibrium points.

Appendices

Appendix A

1.1 The proof of Proposition 1

According to Definition 3, an equilibrium $s^{*}=(s_{m}^{*}, s_{r}^{*})$ of differential equation (12) is an evolutionarily stable equilibrium if and only if there exists an open neighborhood O(s^∗) satisfying

$$ ((s_{m}^{*},s_{r}^{*})-(x, y))(G_{m}(x, y), G_{r}(x, y))^{\text{T}} > 0\quad \text{for all} (x, y)\in O(s^{*})\backslash\{s^{*}\}. $$

(13)

Due to the evolutionary dynamic (12) being sign-compatible, Eq. 13 holds if and only if

$$ ((s_{m}^{*},s_{r}^{*})-(x, y))({\varDelta} w_{m}(x, y), {\varDelta} w_{r}(x, y))^{\text{T}} > 0 \quad \text{for all}\quad (x, y)\in O(s^{*})\backslash\{s^{*}\}. $$

When s^∗ = (0, 0),

$$ \begin{array}{l} ((0, 0)-(x, y))({\varDelta} w_{m}, {\varDelta} w_{r})^{\text{T}}= -x{\varDelta} w_{m}-y{\varDelta} w_{r} \end{array} $$

(14)

Because (x, y) ∈ O((0, 0))∖{(0, 0)}, Eq. 14 is positive if $\pi _{m}^{Th}-\pi _{m}^{Hh}+\alpha (\pi _{m}^{Tt}-\pi _{m}^{Th}) < 0$ and $\pi _{r}^{Ht}-\pi _{r}^{Hh}+\beta (\pi _{r}^{Tt}-\pi _{r}^{Ht}) < 0$.

By the direct computation, as $\beta < \frac {2k_{r} A_{3}}{A_{4}(1-k_{r})^{2}}$ and $\alpha < \frac {{k_{m}^{2}} (1-k_{r})A_{3}}{4k_{r}(1-k_{m})A_{1}}$, the above equation (13) at s^∗ = (0, 0) holds. Hence, the equilibrium (0, 0) is an evolutionarily stable equilibrium.

Similarly, we can prove the other results in Proposition 1.

Appendix B

The other dynamics of games with multiple evolutionarily stable strategy profiles.

The asymmetric equilibrium derived in Corollary 1(3) is presented in Fig. 12, where manufacturers and retailers choose opposite strategies in the evolutionarily stable equilibrium. For the initial parameter values in Corollary 1(4), equilibrium is characterized by stakeholder-oriented manufacturers and both types of retailers (Fig. 13), whereas Corollary 1(5) characterizes the equilibrium with stakeholder-oriented retailers and both types of manufacturers (Fig. 14). Figures 15 and 16 present equilibria with three evolutionarily stable strategy profiles.

Fig. 12

Phase portrait emerging from Corollary 1(3) when k_m = .77, k_r = .31, α = .96, β = .55

Fig. 13

Phase portrait emerging from Corollary 1(4) when k_m = .72, k_r = .28, α = .92, β = .6

Fig. 14

Phase portrait emerging from Corollary 1(5) when k_m = .72, k_r = .28, α = .83, β = .62

Fig. 15

Phase portrait emerging from Corollary 1(6) when k_m = .782, k_r = .333, α = .883, β = .592

Fig. 16

Phase portrait emerging from Corollary 1(7) when k_m = .77, k_r = .31, α = .94, β = .57