1 Introduction

The face gear is a new type of staggered shaft drive gear applied in the aviation field, which has the advantages of a stable transmission ratio, high overlap, and a simple support structure [1]. Face gears used in helicopter power units can make the reducer more compact and torque splitting accuracy and gradually replace bevel gears as the core component of helicopter drive systems [2, 3].

Face gear grinding can significantly improve the machining accuracy and surface quality of tooth surfaces. The main processing methods are disc grinding and worm grinding [4]. In the field of disc wheel grinding research, Tang et al. [5] proposed a plan for grinding a modified face gear using a disc wheel, derived the equations for the modified face gear and the corresponding disc wheel tooth surface, calculated the range of swing angles for the entire tooth surface of the disc wheel ground face gear, and verified the correctness of the proposed method by Vericut machining simulation. Guo et al. [6,7,8] proposed a grinding plan for face gear manufactured by a disk wheel, investigated the envelope residuals of disc wheel grinding of face gears based on a five-coordinate machine tool, and proposed an optimization method to reduce tooth surface deviation. Wang et al. [9,10,11] analyzed the face gear drive principle, designed the disc wheel shape, developed a unique machine tool, proposed a motion control method for grinding face gears with disc wheels, established a mathematical model for roughness based on disc wheels, and studied the face gear generation grinding method using CBN wheels.

In the field of worm wheel grinding research, Litvin et al. [12, 13] proposed an analytical method for determining the worm thread surface, avoiding singularities and worm dressing, developed a computer program for generating face gears by grinding worm shafts, and proposed a new method for grinding face gears with specially shaped worm shafts. Tang et al. [14,15,16,17] suggested a multistep process for grinding face gears with worm gears when the working part of the gear tooth surface is not entirely covered. The worm wheel mounting error was analyzed, and the face gear grinding processing tooth surface error was studied and compared with the Vericut simulation. In addition, the method of changing the diamond wheel profile and modifying the face gear was proposed and verified by simulation. An accurate measurement method is proposed to obtain a tooth surface model for digital tooth contact analysis [18], and it is further applied in accurate and intelligent machining [19, 20]. Guo et al. [21] established a nonlinear contact equation for dressing grinding worm with standard double cone dressing and proposed an approximate method of grinding worm surface with variable meshing angle for grinding face gears. The continuous grinding process of the worm wheel can make each tooth surface of the face gear with high consistency, and has better tooth pitch control than the disc wheel spreading grinding tooth by tooth.

In addition to disc grinding and worm wheel grinding, some scholars have also studied other face gear processing methods. Tang et al. [22] proposed a method for planning spur face gears on a four-axis CNC planer. Guo et al. [23, 24] proposed a method for machining face gears with a circular cutter with a tool profile tilt angle. Chu et al. [25] offered a new way for grinding face gears along the contact trace using a disc CBN wheel. Zschippang et al. [26] described a general method for face gear with helix angle, axial angle, and axial offset of the tooth face generative approach. Wang et al. [27] proposed a shaving method for face gears. Zhang et al. [28] proposed a new way of tooth grinding based on continuous indexing, which uses a conical grinding tool to replace the blade on the face-hobbing cutter head. Yang et al. [29] proposed a face gear plunge milling method. However, these new machining methods are all in the theoretical research stage due to machine tools and equipment limitations.

All the above methods can realize the machining of face gears. However, with the increasing requirements of the application environment of face gears, the machining process is still dominated by worm grinding, and worm grinding can process face gear with high precision. In addition, worm grinding has high processing efficiency due to the principle of continuous generation. At present, some research institutions have carried out the development of face gear grinding machines. However, it is still in the laboratory prototype stage, and it is a special machine for face gears, which is not very versatile, and the maximum deviation of the face gear grinding measurement results is 52.6 μm [25].

A method for continuous generation grinding of face gears on a general cylindrical gear grinding machine is investigated to achieve precision generation grinding of face gears. The topology of the grinding machine and the dressing motion characteristics of the crown worm wheel are analyzed, and a forming dressing method based on the virtual center distance principle is proposed. The motion principle of grinding the face gear by the crown worm wheel is analyzed, and the motion trajectory of face gear grinding is replanned. The tooth surface errors caused by worm misalignment during grinding are analyzed, and the face gear grinding function is realized without affecting the ordinary cylindrical gear grinding process, which is of great significance for improving the versatility of gear machine tools and the development of face gear precision grinding processing technology.

2 Problem description

Due to the limitations of the machine structure, the large deflection of the diamond wheel cannot be achieved during the worm dressing motion when machining the face gear on a general cylindrical gear grinding machine, and the horizontal deflection of the worm cannot be achieved when the grinding of face gears. The dressing structure of the general cylindrical gear grinding machine is shown in Fig. 1. The main motion axes are radial slide X1, wheel axis slide Y1, vertical stroke slide Z1, wheel swivel axis A1, cutter spindle B1, and workpiece spindle C1, which can realize six axes linkage. Besides, there are dressing spindle B2 and roller swivel axis C2.

Fig. 1
figure 1

The structure of the general cylindrical gear grinding machine

Full size image

The movements of X1, Z1, A1, and B2 are independent, and only the linkage coupling exists between B1 and Y1 when dressing a cylindrical worm wheel on the grinding machine. In comparison, the dressing motion of the crown worm wheel requires not only the axial translation of the worm but also the circumferential deflecting motion of the diamond wheel. The deflected diamond wheel dresses both sides of the crown worm wheel, which changes the cone angle of the diamond wheel and increases the dressing range of the diamond wheel. The diamond wheel deflection motion cannot be realized on the machine tool during the crown worm wheel forming dressing. Consider the method to realize the forming dressing of the crown worm wheel without changing the topological relationship of each axis of the machine tool.

The normal section profile of the worm wheel is the same as the end face profile of the shaper cutter. Figure 2 shows the shaper cutter on the left side of the face gear, with the two axes perpendicular to each other. The worm wheel deflects the spiral angle λw to ensure that the normal section participates in the enveloping process. The worm wheel constantly rotates with the face gear, and the worm wheel radial feeds along the face gear, where the speed relationship between the worm wheel and the gear is shown in Eq. (1).

$$\frac{{n}_{2}}{{n}_{w}}={m}_{2w}=\frac{{N}_{w}}{{N}_{2}}$$
(1)

where n2 and nw are the face gear and worm wheel speed, m2w is the transmission ratio, N2 and Nw are the teeth number of the face gear and the head number of the worm wheel.

Fig. 2
figure 2

Worm wheel grinding motion

Full size image

The teeth of the face gear are distributed on the end face, the grinding stroke is completed by radial feed, and the grinding depth is changed by axial feed. The worm wheel needs to be deflected in the horizontal plane when it is radial feed in the direction shown in Fig. 2. According to the grinding machine structure shown in Fig. 1, the worm movement cannot be realized on the machine tool. To avoid additional machine axes, the grinding trajectory of the crown worm wheel must be redesigned to fit the current machine axis layout.

3 Forming dressing method of the crown worm wheel

3.1 Tooth surface of face gears

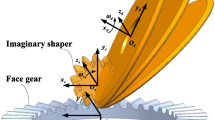
The meshing of a face gear drive is shown in Fig. 3. The face gear and the conjugate pinion mesh between two non-parallel shafts. The meshing process is simulated as the generation process of face gear, where the shaper cutter replaces the cylindrical gear [30].

Fig. 3
figure 3

The machining coordinate system of face gear

Full size image

The machining coordinate system of face gear is established as shown in Fig. 3. Sm is the fixed coordinate system on the shaper cutter, Ss is the moving coordinate system of the shaper cutter, Sp is the fixed coordinate system on the face gear, and S2 is the moving coordinate system of the face gear. The coordinate system of the shaper cutter is established at a position coincident with the origin of the face gear coordinate system. ϕs and ϕ2 are the rotation angles of the shaper cutter and the face gear.

$${\phi }_{2}={m}_{2s}{\phi }_{s}=\frac{{N}_{s}}{{N}_{2}}{\phi }_{s}$$
(2)

where m2s is the transmission ratio, and Ns and N2 are the teeth number of the shaper cutter and the face gear.

The transformation matrix from the Ss to the S2 as

$${M}_{2s}\left({\phi }_{s}\right)={M}_{2p}{M}_{pm}{M}_{ms}=\left[\begin{array}{cccc}cos{\phi }_{2}sin{\phi }_{s}& -cos{\phi }_{2}cos{\phi }_{s}& sin{\phi }_{2}& 0\\ sin{\phi }_{2}sin{\phi }_{s}& -sin{\phi }_{2}cos{\phi }_{s}& -cos{\phi }_{2}& 0\\ cos{\phi }_{s}& sin{\phi }_{s}& 0& 0\\ 0& 0& 0& 1\end{array}\right]$$
(3)

The shaper cutter is a standard involute gear as shown in Fig. 4. The tooth surface can be represented as [31]

$${\overrightarrow{\mathbf{r}}}_{s}\left({\theta }_{s},{u}_{s}\right)=\left[\begin{array}{c}{x}_{s}\\ {y}_{s}\\ {z}_{s}\\ 1\end{array}\right]=\left[\begin{array}{c}-{r}_{b}\left[cos\left({\theta }_{os}+{\theta }_{s}\right)+{\theta }_{s}sin\left({\theta }_{os}+{\theta }_{s}\right)\right]\\ \pm {r}_{b}\left[sin\left({\theta }_{os}+{\theta }_{s}\right)-{\theta }_{s}cos\left({\theta }_{os}+{\theta }_{s}\right)\right]\\ {u}_{s}\\ 1\end{array}\right]$$
(4)

where rb is the base circle radius of the shaper cutter, θs is the involute angle parameter, us is the parameter of the tooth width direction, ‘ + ’ is the right tooth shape, ‘ − ’ is the left tooth shape, θos is the half angle of the tooth groove.

$${\theta }_{os}=\frac{\pi }{2{N}_{s}}-inv{\alpha }_{0}$$
(5)

where α0 is the pressure angle of the indexing circle.

Fig. 4
figure 4

The involute tooth profile of the shaper cutter

Full size image

The working part and the fillet part of the face gear are shown in Fig. 5. The working part participates in the meshing in the transmission, which is the central part. According to the principle of tooth surface enveloping, the tooth surface of the face gear is shown in Eq. (6).

$$\left\{\begin{array}{c}{\overrightarrow{r}}_{2}\left({u}_{s},{\theta }_{s},{\phi }_{s}\right)={M}_{2s}\left({\phi }_{s}\right){\overrightarrow{r}}_{s}\left({\theta }_{s},{u}_{s}\right)\\ f\left({u}_{s},{\theta }_{s},{\phi }_{s}\right)={\overrightarrow{n}}_{s}\cdot {\overrightarrow{v}}_{s2}=0\end{array}\right.$$
(6)

where \({\overrightarrow{r}}_{2}\left({u}_{s},{\theta }_{s},{\phi }_{s}\right)\) is the tooth surface equation of the face gear, \(f\left({u}_{s},{\theta }_{s},{\phi }_{s}\right)\) is the meshing equation, \({\overrightarrow{n}}_{s}\) is the normal line of the shaper cutter, and \({\overrightarrow{v}}_{s2}\) is the relative velocity of the meshing point [31].

$${\overrightarrow{r}}_{2}\left({\theta }_{s},{\phi }_{s}\right)=\left[\begin{array}{c}{x}_{2}\\ {y}_{2}\\ {z}_{2}\\ 1\end{array}\right]=\left[\begin{array}{c}-{r}_{b}\left[sin{\phi }_{2}\left(sin{\phi }_{\theta }-{\theta }_{s}cos{\phi }_{\theta }\right)+\frac{cos{\phi }_{2}}{{m}_{2s}cos{\phi }_{\theta }}\right]\\ \pm {r}_{b}\left[cos{\phi }_{2}\left(sin{\phi }_{\theta }-{\theta }_{s}cos{\phi }_{\theta }\right)-\frac{sin{\phi }_{2}}{{m}_{2s}cos{\phi }_{\theta }}\right]\\ -{r}_{b}\left(cos{\phi }_{\theta }+{\theta }_{s}sin{\phi }_{\theta }\right)\\ 1\end{array}\right]$$
(7)

where ϕθ = ϕs + θos + θs, and ϕ2 = m2sϕs.

Fig. 5
figure 5

Face gear tooth surface

Full size image

The fillet part is at the inside tooth root of the face gear, and there is a common line of intersection between it and the working part. The fillet part is formed by the edge envelope of the shaper cutter top and does not participate in the meshing contact. The fillet part in the S2 is shown in Eq. (8) [31].

$${{\overrightarrow{r}}_{2}}^{*}\left({u}_{s},{\phi }_{s}\right)={M}_{2s}\left({\phi }_{s}\right){{\overrightarrow{r}}_{s}}^{*}\left({u}_{s},{{\theta }_{s}}^{*}\right)$$
(8)

The fillet part can be obtained by giving different values of us and ϕs. θs* is the involute parameter of the shaper cutter tooth top.

3.2 Crown worm wheel profile

The profile calculation of the worm is based on the conjugate surface envelope theory, and the worm profile is calculated by solving the meshing equation. The worm profile is formed by the single parameter envelope of the shaper cutter. Figure 6 shows the shaper cutter and the crown worm gear in a hypothetical internal mesh. The coordinate system Sm1 is the fixed coordinate system on the shaper cutter, Ss1 is the moving coordinate system of the shaper cutter, Sa is the fixed coordinate system on the worm wheel, Sw1 is the crown worm wheel motion coordinate system, and Sn1 is the auxiliary coordinate system. Ews is the shortest distance between the crown worm wheel's and shaper cutter’s axes. λw is the spiral angle of the crown worm wheel. ϕs and ϕw are the rotation angles of the shaper cutter and the crown worm wheel.

$${\phi }_{w}={m}_{ws}{\phi }_{s}=\frac{{N}_{s}}{{N}_{w}}{\phi }_{s}$$
(9)

where mws is the transmission ratio of the shaper cutter to the crown worm wheel.

Fig. 6
figure 6

Coordinate systems of the shaper cutter mesh with the crown worm wheel

Full size image

The transformation matrix from the Ss1 to the Sw1 is shown in Eq. (10).

$$\begin{array}{c}{M}_{w1 s1}\left({\varnothing }_{s}\right)={M}_{w1 a}{M}_{a n1}{M}_{n1 m1}{M}_{m1 s1}\\ =\left[\begin{array}{c}\begin{array}{c}\begin{array}{ccc}\begin{array}{c}{-\mathrm{cos}\varnothing }_{s}{\mathrm{sin\varnothing }}_{w}+\\ {\mathrm{sin\lambda }}_{w}{\mathrm{sin\phi }}_{s}{\mathrm{cos}\phi }_{w}\end{array}& \begin{array}{c}-{\mathrm{sin}\varnothing }_{s}{\mathrm{sin\varnothing }}_{w}-\\ {\mathrm{sin\lambda }}_{w}{\mathrm{cos\varnothing }}_{s}{\mathrm{cos}\varnothing }_{w}\end{array}& \begin{array}{cc}{\mathrm{cos}\lambda }_{w}{\mathrm{cos}\phi }_{w}& {E}_{ws}{\mathrm{sin}\phi }_{w}\end{array}\end{array}\\ \begin{array}{ccc}\begin{array}{c}{\mathrm{cos}\varnothing }_{s}{\mathrm{cos\varnothing }}_{w}+\\ {\mathrm{sin\lambda }}_{w}{\mathrm{sin\phi }}_{s}{\mathrm{sin}\phi }_{w}\end{array}& \begin{array}{c}{\mathrm{sin}\varnothing }_{s}{\mathrm{cos\varnothing }}_{w}-\\ {\mathrm{sin\lambda }}_{w}{\mathrm{cos\phi }}_{s}{\mathrm{sin}\phi }_{w}\end{array}& \begin{array}{cc}{\mathrm{cos}\lambda }_{w}{\mathrm{sin}\phi }_{w}& {-E}_{ws}{\mathrm{cos}\phi }_{w}\end{array}\end{array}\end{array}\\ \begin{array}{c}\begin{array}{ccc}{-\mathrm{cos}\lambda }_{w}{\mathrm{sin}\phi }_{s}& {\mathrm{cos}\lambda }_{w}{\mathrm{cos}\phi }_{s}& \begin{array}{cc}{sin\lambda }_{w}& 0\end{array}\end{array}\\ \begin{array}{ccc}0& 0& \begin{array}{cc}0& 1\end{array}\end{array}\\ \end{array}\end{array}\right]\end{array}$$
(10)

According to the conjugate envelope principle, the crown worm wheel profile \({\overrightarrow{\mathbf{r}}}_{\mathbf{w}1}\) in the Sw1 is shown in Eq. (11).

$$\left\{\begin{array}{c}{\overrightarrow{\mathbf{r}}}_{\mathbf{w}1}\left({\phi }_{s},{\theta }_{s},{u}_{s}\right)={\mathbf{M}}_{\mathbf{w}1\mathbf{s}1}\left({\phi }_{s}\right){\overrightarrow{\mathbf{r}}}_{\mathbf{s}1}\left({\theta }_{s},{u}_{s}\right)\\ {f}_{ws}\left({\phi }_{s},{\theta }_{s},{u}_{s}\right)={\overrightarrow{\mathbf{n}}}_{\mathbf{s}}\cdot {\overrightarrow{\mathbf{v}}}_{\mathbf{s}}^{\mathbf{s}\mathbf{w}}=0\end{array}\right.$$
(11)

where \({\overrightarrow{\mathbf{r}}}_{\mathbf{w}1}\left({\phi }_{s},{\theta }_{s},{u}_{s}\right)\) is the surface equation of the crown worm wheel, \({f}_{ws}\left({\phi }_{s},{\theta }_{s},{u}_{s}\right)\) is the meshing equation, \({\overrightarrow{\mathbf{n}}}_{\mathbf{s}}\) is the normal unit vector of the shaper cutter tooth surface, and \({\overrightarrow{\mathbf{v}}}_{\mathbf{s}}^{\mathbf{s}\mathbf{w}}\) is the relative velocity. The crown worm wheel profile is shown in Eq. (12).

$${\overrightarrow{\mathbf{r}}}_{\mathbf{w}1}\left({\theta }_{s},{\phi }_{s}\right)={\overrightarrow{\mathbf{r}}}_{\mathbf{w}1}\left({\phi }_{s},{\theta }_{s},{u}_{s}\left({\phi }_{s},{\theta }_{s}\right)\right)$$
(12)

3.3 Forming dressing method

The profile calculation of the crown worm wheel is based on the conjugate surface envelope theory, which is formed by the shaper cutter enveloped by a single parameter. Figure 7 shows that the profile of the shaft section of the design diamond wheel is consistent with the profile of the normal section of the crown worm wheel. The diamond wheel makes a spirally sweeping motion and maintains linear contact with the normal section of the crown worm wheel. The crown worm wheel rotates, and the eccentric deflection motion with eccentric Os as the center is the dressing stroke motion. The eccentric Os does not necessarily coincide with the wheel center Ow. The relationship between the speed of eccentric deflection motion nd and the rate of crown worm wheel rotation nw is shown in Eq. (13).

Fig. 7
figure 7

Crown worm wheel dressing motion

Full size image
$$\frac{{n}_{d}}{{n}_{w}}=\frac{1}{{m}_{ws}}=\frac{{N}_{w}}{{N}_{s}}$$
(13)

The deflection of the diamond wheel completes the dressing process instead of the deflection of the crowned worm wheel. This method avoids changing the topology of each axis of the machine and not adding the linkage axis. Figure 8 shows the crown worm wheel’s deflection motion with the eccentric Os as the center. This motion cannot be realized through the movement of a single axis. The principle of virtual center distance machining [32] regards any plane motion in the machining process as a combination of translational and rotational around the base point. The eccentric deflection motion of the crown worm wheel is decomposed into two movements based on the virtual center distance machining principle. One is the rotation of the crown worm wheel around the center OA, which the A1 realizes. The other is the translation of the crown worm wheel in the Y–Z plane, which is recognized by the linkage of the Y1 and the Z1.

Fig. 8
figure 8

Crown worm wheel dressing method based on virtual center distance

Full size image

Taking the crown worm wheel center Ow as the basis point, according to the structure and motion characteristics of the machine tool, the crown worm wheel motion in the dressing process is shown in Fig. 9. In Fig. 9 a), the crown worm wheel center Ow is in the same line as the diamond wheel center Od in the vertical direction. E is the distance between the eccentric Os and the crown worm wheel center Ow. There is an offset distance et between the wheel swivel axis center OA and the diamond wheel center Od in the horizontal direction, and an offset distance ez from the crown worm wheel center Ow in the vertical direction. The offset distance is constant and depends on the machine tool structure. Figure 9 b) shows the motion of the crown worm wheel on the machine tool during the dressing is decomposed, and the travel of each axis of the crown worm wheel from the reference point “0” to any point “i” is calculated.

Fig. 9
figure 9

The dressing motion diagram of the crown worm wheel

Full size image

The center of the crown worm wheel reaches the position of Ow΄ from Ow0, which requires A1 to drive the tool holder to rotate by an angle Δai. At the same time, the Y1 and Z1 respectively move the distances of Δyi and Δzi, and the center of the crown worm wheel reaches the Owi. The motion of the three axes is completed simultaneously. The Ow΄ is a virtual point, and the center of the crown worm wheel does not pass through this point in the actual dressing process. The relationship between the travel of the A1, Y1, and Z1 and the travel of the B1 is shown in Eq. (14).

$$\left\{\begin{array}{c}\Delta {a}_{i}=\Delta {b}_{i}/{N}_{s}\\ \Delta {y}_{i}=\left(E+{e}_{z}\right)tan\left(\Delta {b}_{i}/{N}_{s}\right)+{e}_{t}\left(1-1/cos\left(\Delta {b}_{i}/{N}_{s}\right)\right)\\ \Delta {z}_{i}=-{e}_{t}tan\left(\Delta {b}_{i}/{N}_{s}\right)-\left(E+{e}_{z}\right)\left(1-1/cos\left(\Delta {b}_{i}/{N}_{s}\right)\right)\end{array}\right.$$
(14)

3.4 Forming dressing simulation

The rotation of the diamond wheel does not change the shape and position of the profile of the shaft section involved in dressing. The diamond wheel is equivalent to a slice always in contact with the crown worm wheel. The coordinate transformation of the machine motion calculates the dressed crown worm wheel profile. Figure 10 shows the equivalent slice of the formed diamond wheel, the curve equation of the diamond wheel is shown in Eq. (15).

$${\overrightarrow{r}}_{d}=\left[\begin{array}{c}{x}_{d}\\ {y}_{d}\\ {z}_{d}\\ 1\end{array}\right]=\left[\begin{array}{c}-{r}_{bd}\left[cos\left({\theta }_{o\mathrm{s}}+{\theta }_{d}\right)+{\theta }_{d}sin\left({\theta }_{os}+{\theta }_{d}\right)\right]+{r}_{bd}+{R}_{d}\\ \pm {r}_{bd}\left[sin\left({\theta }_{os}+{\theta }_{d}\right)-{\theta }_{d}cos\left({\theta }_{os}+{\theta }_{d}\right)\right]\\ 0\\ 1\end{array}\right]$$
(15)

where rbd is the radius of the base circle of the diamond wheel, θd is an involute variable parameter, and Rd is the radius of the diamond wheel.

Fig. 10
figure 10

The equivalent slice of diamond wheel

Full size image

The crown worm wheel dressing motion coordinate system is based on the virtual center distance principle. The coordinate system Sd is the moving coordinate system of the diamond wheel, SC is the moving coordinate system of the C2, SB is the fixed coordinate system of the machine tool bed, SZ is the Z1 motion coordinate system, SA is the A1 motion coordinate system, SY’ is the Y1 fixed coordinate system, SY is the Y1 motion coordinate system, Sw is the crown worm wheel motion coordinate system (Fig. 11).

Fig. 11
figure 11

The dressing motion coordinate systems of the crown worm wheel

Full size image

The coordinate transformation from the diamond wheel to the crown worm wheel is shown in Eq. (16).

$${M}_{wd}\left(\Delta {b}_{i}\right)={M}_{wY}{M}_{{YY}^{^{\prime}}}{M}_{{Y}^{^{\prime}}A}{M}_{AZ}{M}_{ZB}{M}_{BC}{M}_{Cd}$$
(16)

where MWY, MYY’, MY’A, MAZ, MZB, MBC, and MCD are

$$\begin{array}{cc}{M}_{Cd}=\left[\begin{array}{cccc}1& 0& 0& 0\\ 0& cos{\lambda }_{w}& sin{\lambda }_{w}& 0\\ 0& -sin{\lambda }_{w}& cos{\lambda }_{w}& 0\\ 0& 0& 0& 1\end{array}\right]& {M}_{BC}=\left[\begin{array}{cccc}0& 0& 1& 0\\ 0& 1& 0& 0\\ -1& 0& 0& {E}_{t}\\ 0& 0& 0& 1\end{array}\right]\end{array}$$
(17)
$$\begin{array}{cc}{M}_{ZB}=\left[\begin{array}{cccc}1& 0& 0& 0\\ 0& 1& 0& 0\\ 0& 0& 1& \Delta {z}_{i}\\ 0& 0& 0& 1\end{array}\right]& {M}_{AZ}=\left[\begin{array}{cccc}1& 0& 0& 0\\ 0& cos\Delta {a}_{i}& sin\Delta {a}_{i}& 0\\ 0& -sin\Delta {a}_{i}& cos\Delta {a}_{i}& 0\\ 0& 0& 0& 1\end{array}\right]\end{array}$$
(18)
$$\begin{array}{cc}{M}_{{Y}^{^{\prime}}A}=\left[\begin{array}{cccc}1& 0& 0& 0\\ 0& 1& 0& -{e}_{t}cos\Delta {a}_{i}\\ 0& 0& 1& {e}_{t}sin\Delta {a}_{i}\\ 0& 0& 0& 1\end{array}\right]& {M}_{{YY}^{^{\prime}}}=\left[\begin{array}{cccc}1& 0& 0& 0\\ 0& 1& 0& -\Delta {y}_{i}\\ 0& 0& 1& 0\\ 0& 0& 0& 1\end{array}\right]\end{array}$$
(19)
$${M}_{wY}=\left[\begin{array}{cccc}cos\Delta {b}_{i}& 0& sin\Delta {b}_{i}& 0\\ 0& 1& 0& 0\\ -sin\Delta {b}_{i}& 0& cos\Delta {b}_{i}& 0\\ 0& 0& 0& 1\end{array}\right]$$
(20)

where Et = Rt + rbd + E + ez, Rt is the radius of the diamond wheel, Δzi is the travel of the Z1 in the dressing process, Δai is the rotation angle of the A1 in the dressing process, Δyi is the travel of the Y1 in the dressing process, Δbi is the rotation angle of the B1 in the dressing process.

There is only one independent motion parameter Δbi in the dressing process of the crown worm wheel. Calculating the profile of the crown worm wheel after the dressing is shown in Eq. (21).

$${\overrightarrow{r}}_{wg}\left({\theta }_{d},\Delta {b}_{i}\right)={M}_{wd}\left(\Delta {b}_{i}\right){\overrightarrow{r}}_{d}\left({\theta }_{d}\right)$$
(21)

Taking the design parameters in Table 1 as an example, the numerical simulation of the dressing process is carried out. The results are shown in Fig. 12. Fig. a) is the numerical simulation profile, and Fig. b) is the theoretical profile. The overall profile of the two is consistent, which preliminarily proves that the numerical simulation is correct.

Table 1 Design parameters of face gear and crown worm wheel
Full size table
Fig. 12
figure 12

Dressing numerical simulation results of the crown worm wheel

Full size image

Eight helixes are evenly selected along the tooth height direction on both sides of the surface. The normal deviation between the numerical simulation surface and the theoretical surface is calculated along the helix. The results are shown in Fig. 13. The deviation value increases linearly from the middle of the crown worm wheel surface to both sides, and the deviation values of the left and right profiles are opposite. Still, the deviation values are below 5 × 10−6 mm, within the allowable error range. It is verified that the decomposition of the dressing movement based on the principle of virtual center distance is correct. The dressing movement of the crown worm wheel can be completed by linking the B1, A1, Y1, and Z1.

Fig. 13
figure 13

The surface deviation between numerical simulation and theoretical calculation

Full size image

4 Face gear grinding method

4.1 Grinding method

The general cylindrical gear grinding machine cannot realize the deflection of the crown worm wheel in the horizontal plane. The trajectory of the crown worm wheel is replanned when the crown worm wheel axis is parallel to the Y1 of the gear grinding machine [33]. Figure 14 shows the angle between the crown worm wheel axis and the shaper cutter axis is always 90°\(-\) λw. The teeth of the face gear are distributed in a ring shape on the end face, and the shaper cutter can still mesh with the face gear when it is in the position shown in the figure.

Fig. 14
figure 14

The corresponding shaper of the worm without deflection

Full size image

Figure 15 shows the motion of each axis and the trajectory of the crown worm wheel when the face gear is grinding. The crown worm wheel and the face gear are rotated proportionally according to Eq. (1) relationship through the B1 and C1. At the same time, through the linkage of the X1 and Y1, the crown worm wheel radial feed from the starting position shown in the figure to the ending position along the shaper cutter axis, completing a full grinding travel, and there is no principal error in motion geometry. The relationship between the radial feed motion of the X1 and Y1 is shown in Eq. (22).

$$\Delta y=\Delta xtan{\lambda }_{w}$$
(22)

where Δx and Δy are the linkage travel of the X1 and Y1.

Fig. 15
figure 15

Crown worm wheel grinding trajectory

Full size image

4.2 Grinding simulation

The face gear is expressed by the spiral surface equation of the crown worm wheel and the motion of the machine. The constant ratio rotation of the crown worm wheel, the face gear, and the linear feed of the crown worm wheel complete the grinding process. Therefore, the numerical simulation of the grinding process of the crown worm wheel is the two-parameter envelope. The motion coordinate system of crown worm wheel grinding is shown in Fig. 16, Sw is the moving coordinate system of the crown worm wheel, SB is rigidly attached to the crown worm wheel (B1), Sa is the auxiliary coordinate system, SX and SY are the motion coordinate systems of X1 and Y1, SC is the fixed coordinate system fixed of the workpiece (C1), S2 is the moving coordinate system of face gear. Δb is the rotation angle of the B1, R is the minimum inner radius of the face gear, and Δc is the rotation angle of the C1. The face gear coordinate system is established at the same height as the shaper cutter axis corresponding to the crown worm wheel. The X of the SC is co-line with the shaper cutter axis, which facilitates the comparison between the numerical simulation and the theoretical calculation.

Fig. 16
figure 16

The worm grinding motion coordinate systems

Full size image

When grinding the face gear on the gear grinding machine, the coordinate transformation matrix from crown worm wheel to face gear is shown in Eq. (23).

$${M}_{2w}\left(\Delta b,\Delta x\right)={M}_{2C}{M}_{CY}{M}_{Ya}{M}_{aB}{M}_{Bw}$$
(23)

where M2C, MCY, MYa, MaB, and MBw are

$$\begin{array}{cc}{M}_{Bw}=\left[\begin{array}{cccc}cos\Delta b& -sin\Delta b& 0& 0\\ sin\Delta b& cos\Delta b& 0& 0\\ 0& 0& 1& 0\\ 0& 0& 0& 1\end{array}\right]& {M}_{aB}=\left[\begin{array}{cccc}1& 0& 0& 0\\ 0& 0& -1& 0\\ 0& 1& 0& E\\ 0& 0& 0& 1\end{array}\right]\end{array}$$
(24)
$$\begin{array}{cc}{M}_{Ya}=\left[\begin{array}{cccc}1& 0& 0& \Delta x\\ 0& 1& 0& -\Delta y\\ 0& 0& 1& 0\\ 0& 0& 0& 1\end{array}\right]& {M}_{CY}=\left[\begin{array}{cccc}-cos{\lambda }_{w}& sin{\lambda }_{w}& 0& -R\\ -sin{\lambda }_{w}& cos{\lambda }_{w}& 0& 0\\ 0& 0& 1& 0\\ 0& 0& 0& 1\end{array}\right]\end{array}$$
(25)
$${M}_{2C}=\left[\begin{array}{cccc}cos\Delta c& sin\Delta c& 0& 0\\ -sin\Delta c& cos\Delta c& 0& 0\\ 0& 0& 1& 0\\ 0& 0& 0& 1\end{array}\right]$$
(26)

The relationship between Δc and Δb is shown in Eq. (27).

$$\Delta c=\frac{{N}_{w}}{{N}_{2}}\cdot \Delta b$$
(27)

According to the coordinate transformation analysis, there are only two independent motion parameters, Δb and Δx, in the grinding process. The tooth surface of the face gear is shown in Eq. (28).

$${\overrightarrow{r}}_{2}\left({\theta }_{s},{\phi }_{s},\Delta b,\Delta x\right)={M}_{2w}\left(\Delta b,\Delta x\right){\overrightarrow{\mathbf{r}}}_{\mathbf{w}}\left({\theta }_{s},{\phi }_{s}\right)$$
(28)

The face gear grinding meshing equation is shown in Eq. (29).

$$\left\{\begin{array}{c}f\left({\theta }_{s},{\phi }_{s},\Delta b,\Delta x\right)=\left(\frac{\partial {\overrightarrow{r}}_{2}}{\partial {\theta }_{s}}\times \frac{\partial {\overrightarrow{r}}_{2}}{\partial {\phi }_{s}}\right)\cdot \frac{\partial {\overrightarrow{r}}_{2}}{\partial \Delta b}=0\\ g\left({\theta }_{s},{\phi }_{s},\Delta b,\Delta x\right)=\left(\frac{\partial {\overrightarrow{r}}_{2}}{\partial {\theta }_{s}}\times \frac{\partial {\overrightarrow{r}}_{2}}{\partial {\phi }_{s}}\right)\cdot \frac{\partial {\overrightarrow{r}}_{2}}{\partial \Delta x}=0\end{array}\right.$$
(29)

where \(\frac{\partial {\overrightarrow{r}}_{2}}{\partial {\theta }_{s}}\times \frac{\partial {\overrightarrow{r}}_{2}}{\partial {\phi }_{s}}\) is the normal vector of the instantaneous contact point and \(\frac{\partial {\overrightarrow{r}}_{2}}{\partial \Delta b}\) is the relative velocity of the instantaneous contact point in the grinding process. \(\frac{\partial {\overrightarrow{r}}_{2}}{\partial \Delta x}\) is the relative velocity of the instantaneous contact point when the crown worm wheel radial feed.

The tooth equations and meshing equations of the face gear are calculated, taking the design parameters shown in Table 1 as an example, using the meshing Eqs. (28) and (29) to eliminate the parameters ϕs and Δb and taking values for θs and Δx, a series of discrete points on the working part of the face gear are calculated. The results are shown in Fig. 17, where a) is the working part obtained from the double parameter envelope, and b) is the theoretical working part obtained from the single parameter envelope of the shaper cutter. The two profiles are the same, proving that the numerical simulation calculation is correct. The fillet part is enveloped by the crown worm wheel’s outer edge, and the numerical simulation process does not independently analyze the crown worm wheel's outer edge.

Fig. 17
figure 17

Worm grinding numerical simulation results of face gear

Full size image

The theoretical tooth surface points corresponding to the discrete points of the numerical simulation tooth surface are calculated. The discrete points of the numerical simulation tooth surface are si (xi(s), yi(s), zi(s)), and the corresponding points of the theoretical tooth surface are ti (xi(t), yi(t), zi(t)). Let xi(t) = xi(s), zi(t) = zi(s) be substituted into Eq. (7), and the corresponding yi(t) is solved. The coordinates of the corresponding points on the theoretical tooth surface are obtained, and the normal unit vector \({\overrightarrow{n}}_{i}\) of each point on the theoretical tooth surface is shown in Eq. (30).

$${\overrightarrow{n}}_{i}=\frac{\frac{\partial {\stackrel{\rightharpoonup }{r}}_{2}}{\partial {\theta }_{s}}\times \frac{\partial {\stackrel{\rightharpoonup }{r}}_{2}}{\partial {\phi }_{s}}}{\left|\frac{\partial {\stackrel{\rightharpoonup }{r}}_{2}}{\partial {\theta }_{s}}\times \frac{\partial {\stackrel{\rightharpoonup }{r}}_{2}}{\partial {\phi }_{s}}\right|}$$
(30)

where \({\stackrel{\rightharpoonup }{r}}_{2}\) is the tooth surface equation of the face gear enveloped by the shaper cutter.

The normal deviation of the corresponding point is shown in Eq. (31).

$${d}_{i}=\left({\overrightarrow{r}}_{{s}_{i}}-{\overrightarrow{r}}_{{t}_{i}}\right)\cdot {\overrightarrow{n}}_{i}$$
(31)

where \({\overrightarrow{r}}_{{s}_{i}}\) is the vector from the origin to the numerical tooth surface point, and \({\overrightarrow{r}}_{{t}_{i}}\) is the vector from the origin to the theoretical tooth surface point.

The normal deviation between the numerical simulation tooth surface and the theoretical tooth surface is shown in Fig. 18. The left and right tooth surface deviations are both negative, and the variation trend is consistent. In the tooth profile direction, the tooth top deviation is slightly larger than the tooth root deviation, and the departure from tooth top to tooth root decreases somewhat. In the tooth width direction, the tooth surface deviation near the inner diameter is less than near the outer diameter. The tooth surface deviation from the inner diameter to the outer diameter decreases first and then increases. Overall, the variation of the numerical simulation tooth surface relative to the theoretical tooth surface is less than 5 × 10−5 mm, within the allowable error range. The numerical simulation results prove the correct replanned crown worm wheel grinding trajectory.

Fig. 18
figure 18

Normal deviation of tooth surface between numerical simulation and theoretical calculation

Full size image

5 Experiment

5.1 Tool misalignment error analysis

The outer edge of the crown worm wheel is circular, and its cross profile varies periodically along the arc. In practice, due to the installation error or machine error, the crown worm wheel is offset along the Y-axis during grinding, causing changes in the tooth shape of the face gear, so the crown worm wheel must be strictly aligned when grinding the face gear. The grinding wheel is offset ey along the Y-axis during grinding, as shown in Fig. 19. The actual grinding trajectory of the crown worm wheel is shown as the blue line in the figure. The coordinate transformation relationship between Sw and SB is shown in Fig. 20. The error matrix is introduced as

Fig. 19
figure 19

Grinding trajectory of wheel misalignment

Full size image
Fig. 20
figure 20

Coordinate transformation relation of wheel misalignment

Full size image
$${M}_{e}=\left[\begin{array}{cccc}1& 0& 0& 0\\ 0& 1& 0& {e}_{y}\\ 0& 0& 1& 0\\ 0& 0& 0& 1\end{array}\right]$$
(32)

The coordinate transformation matrix from crown worm wheel to face gear is shown in Eq. (33).

$${M}_{2w}^{\left(e\right)}\left(\Delta b,\Delta x\right)={M}_{2C}{M}_{CY}{M}_{Ya}{M}_{aB}{M}_{Bw}{M}_{e}$$
(33)

The tooth surface of the face gear is shown in Eq. (34).

$${\overrightarrow{r}}_{2}^{\left(e\right)}\left(\theta ,\beta ,\Delta b,\Delta x\right)={M}_{2w}^{\left(e\right)}\left(\Delta b,\Delta x\right){\overrightarrow{\mathbf{r}}}_{\mathbf{w}}\left({\theta }_{s},{\phi }_{s}\right)$$
(34)

Taking Table 1 as the calculation example, set the wheel offset is 0.3 mm, the face gear tooth surface with wheel misalignment is calculated, as shown in Fig. 21, and the tooth surface is offset in the Y-axis. The normal deviation of the calculated error tooth face from the theoretical tooth face is shown in Fig. 22, and the maximum deviation of the tooth face does not exceed 0.03 mm. In the tooth profile direction, the left and right tooth faces vary less from the root to the top of the tooth. In the tooth width direction, the deviation of the left tooth face varies linearly from positive to negative from the inner diameter to the outer diameter, while the right tooth face is the opposite.

Fig. 21
figure 21

Error surface and theoretical surface

Full size image
Fig. 22
figure 22

Normal deviation between error tooth surface and theoretical tooth surface

Full size image

The tooth surfaces on both sides of the face gear will be offset in the same direction when the crown worm wheel is offset on the Y-axis. Figure 23 shows that the face teeth will be in a "non-orthogonal" state with its axis. Therefore, additional rotation of the C-axis and offset of the feed trajectory are used for reverse adjustment to improve the tooth grinding accuracy.

Fig. 23
figure 23

Variation diagram of face gear tooth surface

Full size image

5.2 Experimental verification

The YS7232 general cylindrical gear grinding machine was selected for crown worm wheel dressing and face gear grinding experiments. The effectiveness of the virtual center distance dressing method and diagonal feed continuous generation grinding method proposed in this study is verified. The face gear and crown worm wheel parameters are shown in Table 2. The machining process and results are shown in Fig. 24. The profile of the dressed crown worm wheel is consistent with the simulation results, and the tooth surface of the face gear after grinding is smooth.

Table 2 Design parameters of face gear and crown worm wheel
Full size table
Fig. 24
figure 24

(a) YS7232 gear grinding machine (b) Dressed crown worm wheel (c) Machined face gear

Full size image

The bevel gear measurement module of the Klingelnberg P26 gear measuring instrument was used to measure the machined face gear (as shown in Fig. 25). The machined tooth deviation is shown in Fig. 26. Analyzing the tooth error values of the machined face gear, the maximum error of the left tooth is − 6.0 μm, and the maximum error of the right tooth is 5.8 μm, both of which are within the error range allowed by the grade 5 accuracy. Considering the machining error of the grinding machine and the detection error of the measuring instrument, the tooth surface of the machined face gear is the same as the numerical simulation tooth surface, which verifies the effectiveness of the virtual center distance grinding wheel dressing method and the diagonal feed continuous spreading grinding method in this research, and the continuous generating grinding of face gears was realized on the general gear grinding machine.

Fig. 25
figure 25

Face gear measurement

Full size image
Fig. 26
figure 26

Tooth deviations of the face gear

Full size image

6 Conclusion

A method of continuously generating grinding the face gears on a general cylindrical gear grinding machine is proposed to realize the precise manufacture of face gears.

  1. 1)

    The forming dressing method of the crown worm wheel with virtual center distance is proposed. This method solves the problem that the general cylindrical gear grinding machine cannot realize the large deflection of the diamond wheel when dressing the crown worm wheel. The deflection of the worm wheel replaces the deflection of the diamond wheel, and the dressing stroke is realized through the linkage of A1, Y1, Z1, and B1 four axes.

  2. 2)

    The radial feed trajectory of the crown worm wheel for grinding the face gear is planned. This method solves the problem that the general cylindrical gear grinding machine cannot realize the horizontal deflection of the worm wheel when grinding the face gear. This trajectory makes the crown worm wheel feed along the radial oblique line of the face gear through the linkage of X1 and Y1 axes.

  3. 3)

    The tooth surface errors caused by the misalignment of the worm during grinding are analyzed. The numerical simulations and machining experiments are conducted for the crown worm wheel dressing and face gear grinding. The shape of the dressed crown worm wheel was consistent with the simulation results, the tooth surface of the face gear after continuous generating grinding was smooth, and the tooth surface error value is within the error range allowed for grade 5 accuracy.

The tooth surface configuration and precision grinding of face gears still need to be explored. Therefore, future work will modify the face gear tooth profile and study the continuous generating grinding processing method of complex modified face gears.