Urban wage inequality and economic agglomeration

Abstract

This paper explores the impact of interplay between transport costs and commuting costs on urban wage inequality and economic distribution within a new economic geography model. As in former studies, workers tend at the same time to agglomerate in order to limit transport costs of manufactured good and to disperse in order to alleviate the burden of urban costs. In this paper, we pay special attention to wages and spot light on how the urban wage inequality is determined by interplay between urban costs and transport costs. We also solve analytically the break points and the sustain points and disclose their relationships with transport costs and commuting costs in a general equilibrium model.

1 Introduction

Explaining the spatial organization of industrial activities across regions from the perspective of interplay between dispersion and agglomeration forces has been the main focus of the New Economic Geography (NEG). The canonical model of this field, developed by Krugman (1991) and known as the core–periphery model, relies on a dispersion force which origins from the expenditure of immobile workers who are employed by a homogeneous sector. But in practice, with the development of regional integration, workers migrate across regions. Nowadays, the main dispersion force seems to lie in the existence of urban costs borne by workers living in large urban agglomerations (Murata and Thisse 2005).

Regional scholars have long tried to integrate urban costs into the NEG models. In this vein of literature, Helpman (1998) first introduced a housing market into an economic geography model in which workers are mobile. However, commuting costs were not considered in his model because of the absence of spatial extension in his setting. As an extension of Helpman (1998), Pflüger and Tabuchi (2010) build a general equilibrium model in which land is used in production and demonstrate that a bell-shaped curve of spatial development can emerge. Independently, Tabuchi (1998) attempts to unify economics $\grave{a}$ la Alonso (1964) and new economic geography. He developed a model which allows for the interplay between urban commuting costs and transport costs in a general equilibrium model. However, his analysis is limited in two extreme cases of zero and infinite transport costs. Murata and Thisse (2005) overcome this difficulty by proposing a model with iceberg commuting costs that affect effective labor supplied by a worker, and thus her income. They show that when urban agglomeration occurs, commuting costs increase, workers spend more energy and time on commuting to CBD, and effective labor supply shrinks. Their results demonstrate that unlike the core–periphery model, low transport costs lead to dispersion while low commuting costs foster the agglomeration of economic activities.

Based on these pioneering works, recent theoretical studies have enriched our understanding of urban-space economy. For example, Allio (2016a) spot light on the role of urban congestion. His results show that urban congestion acts as a dispersion force and hampers workers from agglomerating in the same urban area. Wang and Yang (2014) present a modified Helpman (1998) model with an added tradable agriculture good and modify the manufacturing production according to Forslid and Ottaviano (2003) to identify all possible spatial configuration of a two-region economy. Inspired by Helpman (1998), Wang (2016) uses a solvable core–periphery model to study the issue that high housing prices are also strongly localized in large cities.

Though, existing studies seem to neglect an important question: how does the interplay between transport costs and urban costs affect wages and urban wage inequality? Partially due to the difficulties in obtaining an closed-form solution of wages in a general equilibrium model with both urban costs and transport costs, related studies remain limited. But actually, wages and wage differential are great concerns in NEG literature ever since the pioneering work by Krugman (1980) in which wages across regions are not equalized. Krugman (1980, Sections I and II) removes the agricultural sector of Helpman and Krugman (1985) and illustrates that wages in the larger region are higher. This result is called home market effect (HME) in terms of wages by Takahashi et al. (2013). They present a modified footloose capital model (Martin and Rogers 1995) and made a great contribution by showing that, in a model with mobile capital and endogenous wages, HME in terms of firm share (Krugman (1980, Section III)) and HME in terms of wages are equivalent. Wage rate has long been a great concern of regional studies and associated closely with HME in terms of firm share which is considered to be “the basic ingredient that lies at the heart of most models of agglomeration” (Ottaviano and Thisse 2004: 2566). On the other hand, empirical urban researchers have long recognized that wages in large city exceed those in smaller ones (Alonso 1971; Hoch 1972; Baum-Snow and Pavan 2013; Pan et al. 2016). Empirical studies attribute the wage advantage in large city to the compensating for the time and money costs of commuting costs or housing rents (Moses 1962; Rees and Schultz 1970), the economies in transport and communication (Segal 1976), the improved productivity from agglomeration (Combes et al. 2010, 2012), the spatial sorting of workers (Combes et al. 2008, 2010) and the learning by working in large cities (Gould 2007; Roca and Puga 2016).

In contrast, numerous theoretical studies have focused on how do urban costs alter existing spatial configuration results obtained by NEG models and, however, little address the impact of interplay between transport costs and urban costs on wages and urban wage inequality. This paper aims to fill this gap by building a simple general equilibrium model with iceberg commuting costs proposed by Murata and Thisse (2005).

First, we stress the increasing important role of capital in modern production and introduce mobile capital as a production factor into the framework of Murata and Thisse (2005). Specifically, we modify the manufacturing production according to Zhou et al. (2016) and solve the closed-form solution of wages. Further analyses show that wages in large city are higher than in the smaller one, and the wage differential involves in a bell-shaped pattern in terms of falling transport costs. It is consistent with existing results scattered in the NEG and the new trade theory (NTT) literature. We also illustrate how do commuting costs alter this U-shaped wage differential pattern.

On the other hand, we show a monotonic relationship between wage differential and commuting costs. This result confirms the empirical studies by Timothy and Wheaton (2001). Using microdata from the 1990 census for 2 large metropolitan areas in the USA, Timothy and Wheaton (2001) show that the wage differential across different employment zones is strongly and significantly correlated with the average commute time of workers. They further show that wages and average commuting times are found to be highly correlated with the aggregate number of workers (city size) in each zone. However, without transport costs of manufacturing product, considering only commuting costs in their model, they found inconclusive evidence as to whether wage differential results from agglomeration effects or from a disequilibrium distribution of employment. In contrast, this paper provides a tractable general equilibrium model to analytically address the impact of interplay between urban costs and transport costs on urban wage differential. Our results suggest that what matters is neither transport costs nor urban costs, but the interaction between them.

Borrowing the production technology from Zhou et al. (2016), the manufacturing product prices in our model are independent of wages. While simplifying the mathematical expressions, we retain the main properties of agglomeration forces originating from saving transport costs and dispersion forces originating from alleviation of urban costs in Murata and Thisse (2005). We solve analytically the break points and the sustain points and disclose their relationship with respect to commuting costs and transport costs, respectively. It demonstrates that, different from the core–periphery model, low transport costs lead to dispersion while low commuting costs foster the agglomeration of economic activities. It is well consistent with the main findings of Murata and Thisse (2005). However, while dispersion is a stable equilibrium regardless of transport costs when commuting costs are large enough in Murata and Thisse (2005), such result never occurs in our model where prices are independent of wages. It indicates that dispersion forces become weaker if prices are independent of wages, and the dispersion forces in Murata and Thisse (2005) originate not only from urban costs but also from higher labor costs and prices.

The rest part of this paper is organized as follows. Section 2 introduces the model setting. Section 3 examines the wage inequality. Section 4 examines the spatial equilibrium. Finally, Sect. 5 summarizes the conclusions.

2 The model

2.1 The spatial economy

Consider an economy involving two regions $(r=1, 2)$, one industrial sector producing a differentiated product by using labor and capital as input. The economy is endowed with a unit mass of identical and mobile workers, as well as a large amount of land in each region. Let $\lambda$ denote the fraction of workers residing in region 1 so that the mass of workers in regions 1 and 2 is, respectively, given by $L_1=\lambda$ and $L_2=1-\lambda$.¹

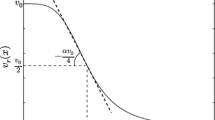
Each region is formed by a city spread along a one-dimensional space X. The amount of land available at each location $x\in X$ is equal to one. All firms located in region r are set up at the Central Business District (CBD) situated at the origin $x=0$ of X. Each worker consumes one unit of land, supplies one unit of labor, owns one unit of capital, and commutes to the CBD. Hence, in equilibrium, workers are equally distributed around the CBD of region r whose urban landscape is therefore given by $[-L_r/2, L_r/2]$. Following the assumption in Murata and Thisse (2005), the commuting costs have the nature of an iceberg and the effective labor supply by a worker living at a distance |x| from the CBD is given by

$$\begin{aligned} s(x)=1-2\theta |x|, \quad x\in [-L_r/2, L_r/2], \end{aligned}$$

where $\theta >0$ captures the efficiency loss due to commuting. For s(x) to be positive regardless of the spatial distribution of workers, we assume $\theta <1$ throughout the paper. Therefore, the total effective labor supply in region r is given by

$$\begin{aligned} S_r=\int _{-L_r/2}^{L_r/2} s(x)\,\mathrm{d}x=L_r(1-\theta L_r/2). \end{aligned}$$

(1)

We normalize the land rent at both city edges at zero. The wage net of commuting costs earned by a worker residing at either edge is such that:

$$\begin{aligned} s\left( \frac{-L_r}{2}\right) w_r=s\left( \frac{L_r}{2}\right) w_r=(1-\theta L_r)w_r. \end{aligned}$$

Because workers are identical, the wages net of both commuting costs and land rent must be equal across all locations. As a result, for a given distribution of workers across regions, the equilibrium land rent in region r is derived as

$$\begin{aligned} R^{*}_{r}(x)=\theta (L_r-2|x|)w_r. \end{aligned}$$

The aggregate land rent in region r is then given by

$$\begin{aligned} ALR_r=\int _{-L_r/2}^{L_r/2}R^{*}_{r}(x)\,\mathrm{d}x=\theta L_r^2 w_r/2. \end{aligned}$$

Here, we follow the assumption in Murata and Thisse (2005) that each region owns the land of its region only.² As a result, each worker living in region r owns an equal share of land in her region of residence. Accordingly, each worker receives an income $ALR_r/L_r=\theta L_r w_r/2$ from her land ownership.

2.2 Consumption

Regarding the consumption of the differentiated good, each worker in region r maximizes a CES-utility function given by

$$\begin{aligned} U_r=\left[ \int _{i\in \Omega _r}c_{rr}(i)^{\frac{\sigma -1}{\sigma }}\,\mathrm{d}i+ \int _{i\in \Omega _s}c_{sr}(i)^{\frac{\sigma -1}{\sigma }}\,\mathrm{d}i\right] ^{\frac{\sigma }{\sigma -1}} \end{aligned}$$

subject to the budget constraint

$$\begin{aligned} \int _{i\in \Omega _r}p_r(i)c_{rr}(i)\,\mathrm{d}i+\int _{i\in \Omega _s}p_s(i) \tau c_{sr}(i)\,\mathrm{d}i&=(1-\theta L_r)w_r+\theta L_r w_r/2+I_r\\&=(1-\theta L_r/2)w_r+I_r, \end{aligned}$$

where $\Omega _r$ is the set of varieties produced in region r, $c_{sr}(i)$ denotes the consumption of variety i produced in region s and consumed in region $r (r\ne s)$, and $I_r$ denotes capital return. Any variety of this good can be shipped from one region to the other according to an iceberg transportation technology $\grave{a}$ la Samuelson (1954): $\tau >1$ units of the variety must be sent from the origin for one unit to arrive at destination. Using the two-stage budgeting of Fujita et al. (1999, chapter 4), we can obtain the demand functions in region r as follows:

$$\begin{aligned} \begin{aligned}&c_{rr}(i)=p_r(i)^{-\sigma }P_{r}^{\sigma -1}[(1-\theta L_r/2)w_r+I_r], \\&c_{sr}(i)=p_s(i)^{-\sigma }\tau ^{-\sigma }P_{r}^{\sigma -1}[(1-\theta L_r/2)w_r+I_r], \end{aligned} \end{aligned}$$

(2)

where the price index in region r is given by

$$\begin{aligned} P_r=\left[ \int _{i\in \Omega _r}p_r(i)^{1-\sigma }\,\mathrm{d}i+\int _{i\in \Omega _s}p_s(i)^{1-\sigma }\tau ^{1-\sigma }\,\mathrm{d}i \right] ^{\frac{1}{1-\sigma }}. \end{aligned}$$

It is then readily verified that her indirect utility is as follows:

$$\begin{aligned} V_r=\frac{(1-\theta L_r/2)w_r+I_r}{P_r}. \end{aligned}$$

(3)

2.3 Production

Existing studies in this vein of literature mainly use labor as the only producing factor. It simplifies the mathematical expressions and captures well the urban models with labor mobility. However, in the age of booming artificial intelligences which allow machines to replace workers (Fernald and Jones 2014) and fast development of automatic production, this kind of production functions does not seem to fit well with the modern production technology took place today. Karabarbounis and Neiman (2013) demonstrate the global labor share has significantly declined since the early 1980s. They show the decrease in the relative price of investment goods, often attributed to advances in information technology and the computer age, induced firms to shift away from labor and toward capital. Another notable study by Frey and Osborne (2017) estimates that, especially for manufacturing routine intensive occupations, employment is increasingly replaced by automatic production.

No doubt, capital takes essential and increasing important roles in production today. Even more speculatively, artificial intelligence and machine learning could allow computers and robots to increasingly replace labor in the production function for goods (Fernald and Jones 2014). Therefore, here, we introduce capital into production and choose capital as marginal input (depreciation of machines, input of raw materials, etc.) while labor is used as fixed input (entrepreneur, human capital, etc.).³ Specifically, we choose units of labor and manufactured goods so that a fixed input of one unit of labor and a marginal input of $(\sigma -1)/\sigma$ units of capital are required to produce one unit of a variety. This production technology describes the situation that labor is used to “design the production line” (Peng et al. 2006) that captures the diversity of differential products, while capital is used to produce each unit of the product, whose amount is dependent on the quantity of firms’ output.

A representative firm in region r sets its price to maximizes its profit:

$$\begin{aligned} \pi _r(i)=(p_r(i)-\frac{\sigma -1}{\sigma }I_r)(c_{rr}(i)L_r+\tau c_{rs}L_s)-w_r, \end{aligned}$$

where $c_{rr}(i)L_r+\tau c_{rs}L_s$ is the total output. Taking the first-order condition yields the profit-maximizing price

$$\begin{aligned} p_r^*(i)=I_r. \end{aligned}$$

Due to the free mobility of capital, the capital returns in two countries are equalized at equilibrium.⁴ We take this capital return as numeraire and obtain $p_r^*(i)=I_r=1$. In addition, the CBD usually includes not only office space but also financial institutions, government, medical centers, entertainment, etc. Therefore, the entrepreneurs here are assumed to commute to the CBD, even though they seem to be able to start production anywhere by our production setting.

2.4 Labor market equilibrium

Let $n_r$ denote the mass of firms located in region r. Since each firm employs one unit of effective labor, the labor market equilibrium condition is given by

$$\begin{aligned} n_r=S_r=L_r(1-\theta L_r/2). \end{aligned}$$

(4)

Proposition 1 of Murata and Thisse (2005) shows the total mass of varieties is maximized at $L_r=1/2$, and illustrates that the more symmetric the spatial distribution of workers, the larger the total mass of varieties in the economy. On the other hand, for given population distribution, an increase of commuting costs $\theta$ would further decrease the range of available varieties. As proposed by Murata and Thisse (2005), agglomeration generates two types of costs for workers: higher commuting costs and narrower range of varieties. Intuitively, when the economy is dispersed, commuting costs are lower, thus implying that more effective labor is available for manufacturing production.

3 Wage inequality

The market clearing conditions for the differentiated product are as follows:

$$\begin{aligned} c_{11}(i) L_1+\tau c_{12}(i)L_2=\sigma w_1, \end{aligned}$$

(5)

$$\begin{aligned} c_{22}(i) L_2+\tau c_{21}(i)L_1=\sigma w_2. \end{aligned}$$

(6)

The LHS are total sales, whereas the RHS are fixed costs. Free entry of firms ensures the zero profit condition. The price indexes are then given by

$$\begin{aligned} P_1=(n_1+\phi n_2)^{\frac{1}{1-\sigma }},\quad P_2=(\phi n_1+ n_2)^{\frac{1}{1-\sigma }}, \end{aligned}$$

(7)

where $\phi \equiv \tau ^{1-\sigma }\in (0,1)$. In the following analyses, a higher $\phi$ implies smaller transport costs across regions, and vice versa.

Plugging Eqs. (2) and (7) into (5) and (6), we solve wages of two regions in closed-form (see Appendix A) and yield wage differential as follows:

$$\begin{aligned} \frac{w_1}{w_2}=\frac{ L_2\left( 2-\theta L_2\right) \left[ \sigma -L_1 (\sigma -1)\right] \phi ^2+ L_1 \left( 2-\theta L_1\right) \sigma \phi + L_1 L_2(\sigma -1)\left( 2-\theta L_2\right) }{ L_1\left( 2-\theta L_1\right) \left[ \sigma -L_2 (\sigma -1)\right] \phi ^2+L_2 \left( 2-\theta L_2\right) \sigma \phi + L_1 L_2(\sigma -1)\left( 2-\theta L_1\right) }, \end{aligned}$$

(8)

where $L_1=\lambda$, $L_2=1-\lambda$.

Proposition 1

For given population distribution $\lambda >1/2$ and commuting costs $1>\theta >0$, wages in large region are higher. Furthermore, the wage differential $w_1/w_2$ involves in a bell-shaped pattern in terms of transport costs.

Proof

See “Appendix A”. $\square$

Urban scholars have long recognized that wages in large city exceed those in smaller ones (Alonso 1971; Hoch 1972; Baum-Snow and Pavan 2013; Pan et al. 2016). Some attribute the wage advantage in large city to the compensating for the time and money costs of commuting costs or housing rents (Moses 1962; Rees and Schultz 1970). Segal (1976) indicates that the wage advantage comes from the “economies exist in transport and communication in the very largest cities with the result that the benefits from agglomeration more than offset congestion costs.” It is consistent with the market access effect in the NEG literature: Firms in large region can save on transport costs and enjoy a higher local sales.

The results of Proposition 1 exhibit the wage advantages in large region and are called the HME in terms of wages by Takahashi et al. (2013) which provides a model with one manufacturing sector and two factors. It is also consistent with section II of Krugman (1980) which removes the agricultural sector of Helpman and Krugman (1985) and shows the wages in the larger country are higher. Meanwhile, with multiple industries, Amiti (1998), Hanson and Xiang (2004), Laussel and Paul (2007), and Zhou et al. (2016) also demonstrate that large region provides higher wages. Based on a one-factor model, Laussel and Paul (2007) show that the wage differential declines in a monotone way with falling transport costs. In contrast, employing two-factor model, Amiti (1998), Takahashi et al. (2013), and Zhou et al. (2016) show that the wage differential involves in a bell-shaped pattern in terms of falling transport costs.

Intuitively, the difference comes from the capital mobility. In one-factor models, all rewards are distributed among immobile labor, which results in a stronger market access effect. By contrast, in a two-factor model, some of rewards are paid to the mobile capital, which decreases the market access effect. Specifically, when transport costs are high ($\phi$ is small), the production location matters, and the market access effect dominates. Higher demand in large region increases local wage rate and further pushes up the wage differential. When transport costs are low ($\phi$ is large), location advantage becomes less obvious, wages in large region decrease to sustain the production.

What worth noting is that existing studies yield such a bell-shaped pattern of wage differential by considering transport costs of manufacturing products only. In contrast, this article incorporates with both transport costs of products and urban costs of living residents. The difference could be shown more clearly when we consider the autarky case ($\phi =0$): The wage differential disappears in Amiti (1998), Takahashi et al. (2013), and Zhou et al. (2016); however, in our model with both transport costs and commuting costs, the wage differential increases in the commuting costs.⁵ This could be clarified more clearly in the following analysis.

Proposition 2

For given population distribution $\lambda >1/2$ and transport costs $1>\phi >0$, there exists one and only one $\phi ^{\sharp }\equiv \sqrt{\frac{\lambda (1-\lambda )(\sigma -1)^2}{[(\sigma -1)\lambda +1][\sigma -(\sigma -1)\lambda ]}}\in (0,1)$. For $\phi \in (0,\phi ^{\sharp })$, the wage differential $w_1/w_2$ increases in commuting costs $\theta$; for $\phi \in (\phi ^{\sharp },1)$, the wage differential $w_1/w_2$ decreases in commuting costs $\theta$.

Proof

See “Appendix B”. $\square$

Existing theoretical studies have paid much attention to how does the interaction between urban costs (commuting costs, housing costs, congestion costs, etc.) and transport costs change the results of economic distribution across regions (Tabuchi 1998; Helpman 1998; Murata and Thisse 2005). However, little ink has been spent on how this interaction affects the mechanism that determines urban wages and wage inequality. In our model, by employing imperfect competition model $\grave{a}$ la  Dixit and Stiglitz (1977), wages equal a fixed proportion of total sale revenues. On the one hand, an increase of commuting costs $\theta$ reduces the range of varieties available (Eq. (4)), thus increases the price index, shifts demand curves for existing products upward (Because consumers value variety, they have to consume more to attain a given utility level.), and increases the sale revenues. On the other hand, an increase of commuting costs reduces the effective labor supply for each worker thus their labor incomes. For given transport costs, wages are determined by the trade-off between these two opposite forces. When transport costs are relatively high ($\phi <\phi ^{\sharp }$), an increase of commuting costs pushes up the price index in large region higher, and thus a relatively higher revenue improvement than in the smaller region, and the wage differential increases in commuting costs. When the transport costs are relatively low ($\phi >\phi ^{\sharp }$), the price index in the small region raises more as commuting costs increase, and thus the wage differential decreases in commuting costs.

The monotonic relationship between wage differential and commuting costs confirms the empirical study by Timothy and Wheaton (2001). Using microdata from the 1990 census for 2 large metropolitan areas in the USA, their results indicate that the wage differential across different employment zones is strongly and significantly correlated with the average commute time of the workers. Interestingly, Timothy and Wheaton (2001) further show that wages and average commuting times are found to be highly correlated with the aggregate number of workers in each zone. However, without transport costs of products, considering only commuting costs in their model, they find inconclusive evidence as to whether wage differences result from equilibrium agglomeration effects or from a disequilibrium distribution of employment. Segal (1976) provides evidences to explain the wage advantage in large region by “agglomeration effect,” but failed to investigate the impacts of falling transport costs on this effect.

Instead, our results disclose that what really matters in the determination of wages and wage differential is the interplay between transport costs and commuting costs. The market access effect is strong when transport costs are high, the agglomeration economies enjoyed in large region encourage firms to pay higher wages to local workers who bear much higher commuting costs than their counterparts living in a smaller city. On the other hand, when transport costs are low, the market access effect decreases in magnitude, firms in large region pay a lower wage rate than before to sustain the production, and an increase of commuting costs would further diminish the wage differential.

4 The spatial equilibrium

In this section, workers migrate across regions driven by utility differential. As in Murata and Thisse (2005), migration is governed by the migration equation:

$$\begin{aligned} \dot{\lambda }=(V_1-V_2)(1-\lambda )\lambda . \end{aligned}$$

We determine the relative utility across regions and have analyses as follows.

4.1 Symmetry

Proposition 3

For $\theta \in (0,1)$ and $\sigma \in (1,+\infty )$, there always exists a unique $\phi$-break point given by

$$\begin{aligned} \phi ^{b}\equiv \frac{\theta +4\sigma -3\theta \sigma +\sqrt{8 (2-\theta ) (\sigma -1) (3\sigma -\theta \sigma -1)+(\theta +4 \sigma -3 \theta \sigma )^2}}{4 (3\sigma -\theta \sigma -1)} \in (0,1), \end{aligned}$$

and the symmetric equilibrium is stable if and only if $\phi >\phi ^{b}$. Furthermore, $\phi ^{b}$ decreases in commuting costs $\theta$.

Proof

See “Appendix C”. $\square$

Alternatively, we can derive the break point in terms of commuting costs, which is called $\theta$-break point by Murata and Thisse (2005).

Proposition 4

If $\phi >\frac{\sigma +1+\sqrt{17\sigma ^2 -22\sigma +9}}{4( 2\sigma -1)}$, there exists a unique $\theta$-break point given by

$$\begin{aligned} \theta ^{b}\equiv \frac{-2 \phi ^2(3\sigma -1)+4 \sigma \phi +2 (\sigma -1)}{-2 \sigma \phi ^2+(3 \sigma -1)\phi +\sigma -1}, \end{aligned}$$

and the symmetric equilibrium is stable if and only if $\theta >\theta ^{b}.$ Furthermore, $\theta ^{b}$ decreases in $\phi$. If $\phi <\frac{\sigma +1+\sqrt{17\sigma ^2 -22\sigma +9}}{4( 2\sigma -1)}$, there exists no $\theta$-break point, and the symmetric equilibrium is always unstable regardless of commuting costs.

Proof

See “Appendix D”. $\square$

Unlike what we observe in the standard core–periphery model, the symmetric configuration is stable when the transport costs are sufficiently low $(\phi >\phi ^b)$. The difference comes from the existence of commuting costs. Agglomeration causes higher commuting costs and narrower range of varieties (See Eq. (4)). When commuting costs are high, or transport costs are low, workers disperse to maximize their utilities. It is consistent with Murata and Thisse (2005) showing that the symmetric configuration is stable when transport costs are low. Furthermore, our model even able to disclose the relationship between $\phi ^b$ ($\theta ^b$) and $\theta$ ($\phi$): Higher commuting costs or lower transport costs would further enlarge the range of symmetric configuration.

However, we contrast with them in the sense that the dispersion forces in our model are weaker. Specifically, the symmetric configuration is always a stable equilibrium in Murata and Thisse (2005) if varieties are sufficiently differentiated and commuting costs are sufficiently high. However, in our model with mobile capital as marginal input, such results never appear. The differences origin from the production technology which use mobile capital as marginal input, and thus the prices are independent of wages. It reminds us that the higher wages and thus higher product prices result from agglomeration serve as another dispersion force that discourages agglomeration. Therefore, the dispersion forces in Murata and Thisse (2005) may associate not only with urban costs, but also with wages and prices. However, in their model, wages are not tractable and thus difficult to explicitly separate the dispersion force that comes from higher labor costs and prices. Here, product prices are independent of wages and thus enable us to investigate the effects of interplay between commuting costs and transport costs on economic activity distribution more clearly. Note the reciprocal relationship between $\phi ^b$ and $\theta ^b$, Proposition 4 may be viewed as the counterpart of Proposition 3 in terms of commuting costs.

4.2 Agglomeration

Proposition 5

For $\theta \in (0,1)$, there exists a unique $\phi ^s$ which is defined by

$$\begin{aligned} \frac{(\sigma -1)}{\sigma }(\phi ^s)^{\frac{1}{\sigma -1}}+\frac{2}{ (2-\theta ) \sigma }(\phi ^s)^{\frac{\sigma }{\sigma -1}}-1=0. \end{aligned}$$

Agglomeration is a stable equilibrium if and only if $\phi <\phi ^s$. Furthermore, $\phi ^s$ decreases in $\theta$.

Proof

See “Appendix E”. $\square$

It differs from the standard core–periphery model which shows that agglomeration is stable when transport costs are low ($\phi$ is large). Intuitively, this difference origins from the higher commuting costs and narrower range of varieties available workers bear when they agglomerate. Our results are consistent with models considering urban costs associated with agglomeration (Helpman 1998; Murata and Thisse 2005). Intuitively, workers bear higher commuting costs and narrower range of varieties available when they agglomerate in a single city. Agglomeration occurs only if transport costs are so high that buying varieties from the other city becomes too expensive. Furthermore, the range of transport costs for agglomeration becomes wider when commuting costs decrease. In the same vein, the $\theta$-sustain point can be derived as follows.

Proposition 6

There exists a unique $\underline{\phi }\in (0,1)$ which satisfies $2\underline{\phi }^{\frac{\sigma }{\sigma -1}}+(\sigma -1)\underline{\phi }^{\frac{1}{\sigma -1}}-\sigma =0$. If $\phi \in (\underline{\phi },1)$, agglomeration is a stable equilibrium if and only if

$$\begin{aligned} \theta <\theta ^s\equiv 2\left[ \frac{(\sigma -1) \left( 1-\phi ^{\frac{1}{\sigma -1}}\right) +1- \phi ^{\frac{\sigma }{\sigma -1}}}{\sigma -(\sigma -1) \phi ^{\frac{1}{\sigma -1}}}\right] >0. \end{aligned}$$

Furthermore, $\theta ^s$ decreases in $\phi$. If $\phi \in (0,\underline{\phi })$, there exists no $\theta$-sustain point, and the agglomeration equilibrium is sustainable for all commuting costs.

Proof

See “Appendix F”. $\square$

As we mentioned before, the dispersion forces in our model are weaker than in Murata and Thisse (2005) in the sense that higher wages associated with agglomeration do not affect prices of varieties here. Therefore, it is not surprising that this result is consistent with Murata and Thisse (2005) showing that agglomeration is sustainable for all commuting costs when transport costs are sufficiently large. Note the reciprocal relationship between $\phi ^s$ and $\theta ^s$, Proposition 6 may be viewed as the counterpart of Proposition 5 in terms of commuting costs.

4.3 Break point versus sustain point

Proposition 7

When they exist, the $\theta$-sustain point is larger than the $\theta$-break point; on the other hand, the $\phi$-sustain point is larger than the $\phi$-break point.

Proof

See “Appendix G”. $\square$

The forgoing analyses, however, do not provide a full characterization of spatial equilibria. Since it is difficult to solve the closed-form solution of $\lambda$, we appeal to numerical solutions in the next section.

Fig. 1

Real wage differentials and economic distribution

4.4 The set of equilibria: numerical examples

The Fig. 1 is depicted for $\sigma =3$, and the columns show the situation when $\theta$ is given while $\phi$ is increasing. In the first column, for given $\theta =0.1$ we have $\phi ^b=0.98$. There are three equilibria for each different $\phi$, but dispersion is unstable while full agglomeration within a single city is the only stable equilibrium as $\phi$ is smaller than $\phi ^b$.

In the second column, for given $\theta =0.75$, we have $\phi ^b=0.80$ and $\phi ^s=0.82$. Three equilibria exist for each different $\phi$. As $\phi$ increases to 0.9, dispersion becomes the only stable equilibrium as $\phi$ is larger than $\phi ^s$.

In the third column, for given $\theta =0.9$, we have $\phi ^b=0.74$ and $\phi ^s=0.77$. There are also three equilibria when $\phi =0.1$ or $\phi =0.9$: Full agglomeration is the only stable equilibrium when $\phi <\phi ^b$ while dispersion is the only stable equilibrium when $\phi >\phi ^s$. Furthermore, there are five equilibria when $\phi =0.75$: The two partial equilibria involving partial agglomeration in two cities of unequal size are unstable, whereas the other three equilibria, corresponding to dispersion or full agglomeration, are stable because the value of $\phi$ belongs to the interval of $[\phi ^b,\phi ^s]$.

The forgoing results are somewhat reminiscent of those derived in the standard core–periphery model, but the most striking difference is that the sequence of configurations is reversed. In Krugman (1991), the cost associated with agglomeration comes from the provision of the manufactured goods to the immobile workers residing in the periphery (Murata and Thisse 2005). In contrast, in this paper with urban costs, agglomeration causes higher commuting costs and even narrower range of varieties. Thus, mobile workers are unwilling to bear these costs by being dispersed unless the transport costs across regions are so high that the net benefit of having all varieties locally produced is sufficiently large to outweigh the higher urban costs that workers must bear by being agglomerated.

Our spatial equilibrium results confirm the findings by Murata and Thisse (2005) which proposes a simple model of economic geography integrating both transport costs and commuting costs. The difference between this paper and Murata and Thisse (2005) origins from the production function. It enables us to separate the influence of wages on prices and thus clearly analyze the effect of interplay between transport costs and commuting costs on spatial equilibrium. As we mentioned above, dispersion is a stable equilibrium regardless of transport costs when commuting costs are large enough in Murata and Thisse (2005); however, such result never occurs in our model where prices are independent of wages.

5 Concluding remarks

This paper explores the impact of interplay between commuting costs and transport costs on urban wage inequality and economic distribution. We show that integrating urban costs into NEG models does not only affect how economic activities distribute across regions, but also the mechanism that determines urban wages and wage inequality. The analyses of wages here are limited to the situation when population distribution is given; nonetheless, it does provide insights as how the urban wages and wage differential are determined by the interactions between urban costs and transport costs. We show the bell-shaped pattern between wage differential and transport costs that are scattered in existing NEG and NTT literature. We also demonstrate the monotonic relationship between urban wage differential and commuting costs that is consistent with empirical urban studies. While several empirical studies provide inconclusive evidence as to whether wage differences result from agglomeration effect or from a disequilibrium distribution of employment, our model analytically discloses how this monotonic relationship is determined. Our results imply that what matters is neither agglomeration effect nor commuting costs, but the interaction between them.

Second, we stress the essential and increasing important roles of capital in modern production and introduce capital as marginal input into the framework of Murata and Thisse (2005). Our spatial equilibrium results confirm their findings by showing that, when agglomeration generates higher urban costs and narrower range of varieties, low transport costs lead to the dispersion whereas low commuting costs foster the agglomeration of economic activities. Certainly, some different results are also generated. Dispersion is a stable equilibrium regardless of transport costs when commuting costs are large enough in Murata and Thisse (2005); however, such result never occurs in our model where prices are independent of wages. It indicates that the dispersion forces in Murata and Thisse (2005) may come not only from urban costs and narrower rang of varieties, but also from higher prices. On the other hand, we determine four threshold value of break points and sustain points and analytically disclose their relationships with commuting costs or transport costs. By showing how the interplay between transport costs and commuting costs shapes the spatial equilibrium, this paper emphasizes the impacts of interaction between urban costs and transport costs on the determination of urban wages and economic distribution.

Appendices

Appendices

1.1 Appendix A: Proof of Proposition 1

Plugging Eqs. (2) and (7) into (5) and (6), we solve wages as follows:

$$\begin{aligned} w_1&=\frac{2 (1-\lambda )(2+\theta \lambda -\theta )[(\sigma -\lambda \sigma +\lambda ) \phi ^2+\lambda (\sigma -1)]+2 \lambda (2-\theta \lambda ) \sigma \phi }{\lambda (1-\lambda )(2+\theta \lambda -\theta )(2-\theta \lambda ) \left( \sigma ^2-1\right) \phi ^2+{\mathscr {A}}_1},\\ w_2&=\frac{2\lambda (2-\theta \lambda )[(1+\lambda \sigma -\lambda ) \phi ^2+(1-\lambda )(\sigma -1)] +2(1-\lambda )(2+\theta \lambda -\theta ) \sigma \phi }{\lambda (1-\lambda )(2+\theta \lambda -\theta )(2-\theta \lambda ) \left( \sigma ^2-1\right) \phi ^2+{\mathscr {A}}_1}, \end{aligned}$$

where

Treating $w_1/w_2$ as a function of $\phi$, we define $f(\phi )\equiv w_1/w_2$ and have

$$\begin{aligned} f(0)=\frac{2-\theta (1-\lambda )}{2-\theta \lambda }>1 \quad \text {and} \quad f(1)=1, \end{aligned}$$

(A1)

where the inequality comes from $\lambda >1/2.$

Differentiating $f(\phi )$ with respect to $\phi$, we have

$$\begin{aligned} \frac{\partial f}{\partial \phi } \biggr |_{\phi =0}&=\left( \frac{\sigma }{\sigma -1}\right) \left\{ \frac{\left[ 4(1-\theta ) +\theta ^2\left( 1-\lambda +\lambda ^2\right) \right] (2 \lambda -1)}{\lambda (1-\lambda )(2-\theta \lambda )^2}\right\} >0, \end{aligned}$$

(A2)

$$\begin{aligned} \frac{\partial f}{\partial \phi } \biggr |_{\phi =1}&= \left( \frac{2 \lambda -1}{\sigma }\right) \left[ \frac{2 \theta \lambda (1-\lambda )}{ 2-\theta \left( 1-2 \lambda +2\lambda ^2\right) }-\sigma \right] <0, \end{aligned}$$

(A3)

where the first inequality comes from $\lambda >1/2$ and the second inequality comes from $\frac{2 \theta \lambda (1-\lambda )}{ 2-\theta \left( 1-2 \lambda +2\lambda ^2\right) }<1<\sigma .$

On the other hand, the numerators of $w_r$ are quadratic functions of $\phi$. Evidently, for any given parameter ${\mathscr {A}}$, equation $f(\phi )={\mathscr {A}}$ has at most two solutions of $\phi$. In other words, in the $\phi -f(\phi )$ panel, the curve $f(\phi )$ crosses any horizontal line $w_1/w_2={\mathscr {A}}$ at most twice. Given inequalities (A2) and (A3), we know $f(\phi )$ involves in a bell-shaped pattern in terms of $\phi$. Furthermore, we have $w_1/w_2>1$ at $\phi =0$ and $w_1/w_2=1$ at $\phi =1$. Therefore, for $\phi \in (0,1)$, we have $w_1>w_2$.

1.2 Appendix B: Proof of Proposition 2

Differentiating $w_1/w_2$ with respect to $\theta$, we have

$$\begin{aligned}&\frac{\partial f}{\partial \theta }=\frac{ \lambda (1-\lambda )(2\lambda -1) \left( 1-\phi ^2\right) {\mathscr {B}}(\phi )}{\{\lambda (2-\theta \lambda )[(1+\lambda \sigma -\lambda ) \phi ^2+(1-\lambda )(\sigma -1)] +(1-\lambda )(2+\theta \lambda -\theta ) \sigma \phi \}^2}, \end{aligned}$$

where

$$\begin{aligned} {\mathscr {B}}(\phi )\equiv -[\lambda (\sigma -1)+1] [\sigma -\lambda (\sigma -1)] \phi ^2+\lambda (1-\lambda )(\sigma -1)^2. \end{aligned}$$

Evidently, the sign of $\partial (w_1/w_2)/\partial \theta$ depends on ${\mathscr {B}}(\phi )$ which is a quadratic function of $\phi$. Easy to find that ${\mathscr {B}}(0)>0$ and ${\mathscr {B}}(1)=-\sigma <0$, for $\phi \in (0,1)$. There exists one and only one

$$\begin{aligned} \phi ^\sharp \equiv \sqrt{\frac{\lambda (1-\lambda )(\sigma -1)^2}{[(\sigma -1)\lambda +1][\sigma -(\sigma -1)\lambda ]}}, \end{aligned}$$

${\mathscr {B}}(\phi )>0$ if $\phi \in (0,\phi ^\sharp )$ and ${\mathscr {B}}(\phi )<0$ if $\phi \in (\phi ^\sharp ,1)$. Therefore, we have $\partial (w_1/w_2)/\partial \theta >0$ if $\phi \in (0,\phi ^\sharp )$ and $\partial (w_1/w_2)/\partial \theta <0$ if $\phi \in (\phi ^\sharp ,1)$.

1.3 Appendix C: Proof of Proposition 3

Differentiating $V_1/V_2$ with respect to $\lambda$, we have

$$\begin{aligned} \frac{\partial (V_1/V_2)}{\partial \lambda }\biggr |_{\lambda =\frac{1}{2}}=\frac{8 {\mathscr {C}}(\phi )}{(4-\theta ) (\sigma -1) (1+\phi ) (\sigma -1+\phi +\sigma \phi )}, \end{aligned}$$

(C1)

where

$$\begin{aligned} {\mathscr {C}}(\phi )\equiv -2 (3\sigma -\theta \sigma -1) \phi ^2+(\theta +4 \sigma -3 \theta \sigma ) \phi +(2-\theta ) (\sigma -1). \end{aligned}$$

(C2)

Evidently, the sign of Eq. (C1) depends on ${\mathscr {C}}(\phi )$ which is a quadratic function of $\phi$. Easy to find that ${\mathscr {C}}(0)=(2-\theta )(\sigma -1)>0, {\mathscr {C}}'(0)>0$ and ${\mathscr {C}}(1)=-2\theta (\sigma -1)<0$, therefore, for $\phi \in (0,1)$, there exists one and only one

$$\begin{aligned} \phi ^b\equiv \frac{\theta +4 \sigma -3 \theta \sigma +\sqrt{8 (2-\theta ) (\sigma -1) (3\sigma -\theta \sigma -1)+(\theta +4 \sigma -3 \theta \sigma )^2}}{4 (3\sigma -\theta \sigma -1)}. \end{aligned}$$

If $\phi >\phi ^b$, we have $\frac{\partial (V_1/V_2)}{\partial \lambda }\biggr |_{\lambda =\frac{1}{2}}<0$; if $0<\phi <\phi ^b$, we have $\frac{\partial (V_1/V_2)}{\partial \lambda }\biggr |_{\lambda =\frac{1}{2}}>0$.

Furthermore, differentiating $\phi ^b$ with respect to $\theta$ yields

$$\begin{aligned} \frac{\partial \phi ^b}{\partial \theta }&=\frac{(\sigma -1)\left[ -(32-19 \theta ) \sigma ^2+4 (5-3 \theta ) \sigma -4+\theta -(5 \sigma -1){\mathscr {C}}_1 \right] }{4 (1-3\sigma +\theta \sigma )^2 {\mathscr {C}}_1}\\&\le \frac{(\sigma -1)\left[ \left( \frac{-28-12\theta +17 \theta ^2}{32-19 \theta }\right) -(5 \sigma -1){\mathscr {C}}_1 \right] }{4 (1-3\sigma +\theta \sigma )^2 {\mathscr {C}}_1}\\&<0, \end{aligned}$$

where ${\mathscr {C}}_1\equiv \sqrt{8 (2-\theta ) (\sigma -1) (3\sigma -\theta \sigma -1)+(\theta +4 \sigma -3 \theta \sigma )^2}$ and the second inequality comes from $\frac{-28-12\theta +17 \theta ^2}{32-19 \theta }<0.$

1.4 Appendix D: Proof of Proposition 4

For $\sigma >1$ and $1>\phi >0$, solving ${\mathscr {C}}(\phi )<0$ yields

$$\begin{aligned} \theta>\theta ^b\equiv \frac{-2 \phi ^2(3\sigma -1)+4 \sigma \phi +2 (\sigma -1)}{-2 \sigma \phi ^2+(3 \sigma -1)\phi +\sigma -1}>0. \end{aligned}$$

Using properties of quadratic function, we have $\theta ^b<1$ if and only if

$$\begin{aligned} \phi >\frac{\sigma +1+\sqrt{17\sigma ^2 -22\sigma +9}}{4( 2\sigma -1)}\in (0,1). \end{aligned}$$

Differentiating $\theta ^b$ with respect to $\phi$ yields

$$\begin{aligned} \frac{\partial \theta ^b}{\partial \phi }=-\frac{2 (\sigma -1) \left[ (5 \sigma -1) \phi ^2+2(\sigma -1) \phi +\sigma -1\right] }{\left[ 2\sigma \phi ^2+(1-3\sigma )\phi +1-\sigma \right] ^2}<0, \end{aligned}$$

where the inequality comes from $(5 \sigma -1) \phi ^2+2(\sigma -1) \phi +\sigma -1>0.$

1.5 Appendix E: Proof of Proposition 5

Agglomeration is a stable equilibrium if and only if $\frac{V_2}{V_1}\biggr |_{\lambda =1}<1$ that implies

$$\begin{aligned} F(\phi )\equiv \frac{(\sigma -1)}{\sigma }\phi ^{\frac{1}{\sigma -1}}+\frac{2}{ (2-\theta ) \sigma }\phi ^{\frac{\sigma }{\sigma -1}}-1<0. \end{aligned}$$

(E1)

Evidently, $F(\phi )$ is an increasing function of $\phi$, and $F(0)=-1<0$, $F(1)=\frac{\theta }{\sigma (2-\theta )}>0$. Therefore, there exists one and only one $\phi ^s\in (0,1)$ which satisfies $F(\phi ^s)=0$. We have $F(\phi )<0$ if and only if $\phi <\phi ^s$. On the other hand, by using properties of implicit function, we have

$$\begin{aligned} \frac{\partial \phi ^s}{\partial \theta }=\frac{-2 (\sigma -1) \phi ^2}{(2-\theta ) [2 (\sigma -1+\sigma \phi )-\theta (\sigma -1)]}<0. \end{aligned}$$

Therefore, $\phi ^s$ decreases in $\theta$.

1.6 Appendix F: Proof of Proposition 6

Solving $\frac{V_2}{V_1}\biggr |_{\lambda =1}<1$, we obtain

$$\begin{aligned} \theta <\theta ^s\equiv 2\left[ \frac{(\sigma -1) \left( 1-\phi ^{\frac{1}{\sigma -1}}\right) +1- \phi ^{\frac{\sigma }{\sigma -1}}}{\sigma -(\sigma -1) \phi ^{\frac{1}{\sigma -1}}}\right] >0. \end{aligned}$$

To make sure $\theta ^s<1$, it must be hold that

$$\begin{aligned} {\mathscr {F}}(\phi )\equiv 2\phi ^{\frac{\sigma }{\sigma -1}}+(\sigma -1)\phi ^{\frac{1}{\sigma -1}}-\sigma >0. \end{aligned}$$

The above inequality holds if and only if $\phi >\underline{\phi }$ with $\underline{\phi }$ determined by ${\mathscr {F}}(\underline{\phi })=0$. Easy to find that ${\mathscr {F}}(\phi )$ is an increasing function of $\phi$, ${\mathscr {F}}(0)=-\sigma <0$ and ${\mathscr {F}}(1)=1>0$. Therefore, $\underline{\phi }$ is uniquely determined.

On the other hand, differentiating $\theta ^s$ with respect to $\phi$ yields

$$\begin{aligned} \frac{\partial \theta ^s}{\partial \phi }=-\frac{\sigma ^2 \phi ^{\frac{1}{1-\sigma }}-(\sigma -1)^2}{(\sigma -1) \left( 1+\sigma \phi ^{\frac{1}{1-\sigma }}-\sigma \right) ^2}<0, \end{aligned}$$

where the inequality comes from $\phi ^{\frac{1}{1-\sigma } }>1$ and $\sigma >1$. Therefore, $\theta ^s$ decreases in $\phi$. If $\phi <\underline{\phi }$, we know the agglomeration is always stable regardless of commuting costs $\theta$.

1.7 Appendix G: Proof of Proposition 7

We have

$$\begin{aligned} \theta ^s-\theta ^b=\frac{2\sigma \phi ^{\frac{\sigma }{\sigma -1}} {\mathscr {G}}(\phi )}{\left[ \sigma -(\sigma -1) \phi ^{\frac{1}{\sigma -1}}\right] \left[ -2 \sigma \phi ^2+(3 \sigma -1)\phi +\sigma -1\right] }, \end{aligned}$$

where ${\mathscr {G}}(\phi )\equiv (\sigma -1) (1+\phi )\phi ^{\frac{-1}{\sigma -1}}- \left( \sigma -1+\phi +\sigma \phi -2 \phi ^2\right) .$ For $\sigma >1$ and $1>\phi >0$, the denominator is positive, and the sign of $\theta ^s-\theta ^b$ depends on ${\mathscr {G}}(\phi )$. We have ${\mathscr {G}}(0)>0$, ${\mathscr {G}}(1)=0$ and ${\mathscr {G}}'(1)=0$. Furthermore, the twice differential of ${\mathscr {G}}(\phi )$ is

$$\begin{aligned} {\mathscr {G}}''(\phi )=4+\frac{\phi ^{\frac{1}{1-\sigma }-2} (\sigma -\sigma \phi +2 \phi )}{\sigma -1}>0. \end{aligned}$$

Therefore, in the interval of $\phi \in (0,1)$, ${\mathscr {G}}(\phi )$ is convex and ${\mathscr {G}}(\phi )>0$. Then, we have $\theta ^s-\theta ^b>0.$

By construction, $\phi ^s$ is the reciprocal of Eq. (E1), whereas $\phi ^b$ is the reciprocal of Eq. (C2). From Popositions 3 and 5, it follows that $\phi ^s$ and $\phi ^b$ are monotonic with respect to $\theta$. Therefore, it must be that $\phi ^s>\phi ^b$; otherwise, it would have $\theta ^s<\theta ^b$ for some $\phi$, thus contradicting $\theta ^s-\theta ^b>0.$