Geometrical Proportion in the Sixteenth Century: Methods and Constraints

Abstract

Common conceptions about Gothic and Renaissance architectural proportion systems contrast the mediaeval geometrical methods with the arithmetical, rational ones of the Renaissance. In this paper, the authors analyze the geometrical proportion systems in Chapter V of Compendio de Arquitectura y simetría de los templos, generally attributed to the sixteenth-century Spanish architect Rodrigo Gil de Hontañón. This shows that geometrical proportioning system of the Compendio generally leads to rational proportions. However, on some occasions, irrational proportions arise from the geometrical properties of the figures used in the layout of the churches, or from deliberate choices of the author, with a remarkable disregard for the notions of commensurable or incommensurable dimensions. This is consistent with the notion, put forward by Shelby, of constructive geometry. Medieval design methods were based on a compass and ruler geometry, with no concern for rational and irrational proportions.

Introduction

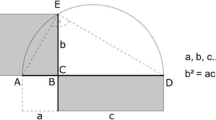
Rudolf Wittkower, in his classic Architectural Principles in the Age of Humanism (1949), set the arithmetical, rational, commensurable systems of proportion of Renaissance architecture in opposition to the geometrical, irrational and incommensurable practices of the Middle Ages. However, he warned that Renaissance graphical methods with an apparent geometrical basis could lead to rational proportions, citing an example taken from Serlio (1545: 23r). A few years later, Otto von Simson (1956) argued that rational systems of proportion were frequently used in the Gothic period. In order to gauge the use of geometrical and arithmetical proportion systems in the first half of the sixteenth century, we shall analyze the proportion systems for churches included in Compendio de Arquitectura y Simetría de los templos, that is, “Summary of Architecture and Temple Symmetry” (Fig. 1). This manuscript, written by Simón García (1681), states in its introduction that most of its content derives from Rodrigo Gil de Hontañón, the main designer of the cathedrals of Segovia and Salamanca in the mid-sixteenth century.

Fig. 1

First page of Compendio de arquitectura y simetría de los templos, by Simón García, 1681, with portions taken from Rodrigo Gil de Hontañón, c. 1550. Biblioteca Nacional de España, MSS/8884. The image is property of Biblioteca Nacional de España

Marcelino Menéndez Pelayo (1883–1889: 2, 377, note 2) identified Rodrigo’s contribution with the first six chapters of the treatise. This stance has been followed by most scholars who have dealt with the manuscript, in light of the strong difference between these chapters, which deal with traditional church plans and rib vaults, and the rest of the manuscript, which focuses on the orders and classical plane geometry, although there are some contradictions and disagreements in the details (see Camón 1941: 301, 305; Gómez-Moreno 1949: 11–12; Hoag 1958: 406; Bonet 1991: 14–15; Sanabria 1982: 282). Further, Hoag (1958: 410) placed the start of the planning of Rodrigo’s contribution in the early 1560s, while Sergio Luis Sanabria (1984: 149–150) argued for an earlier date, between 1544 and 1554.

Chapter V of the Compendio, traditionally ascribed to Rodrigo Gil as mentioned, includes four geometrical schemes for the general layout of churches of different sizes. These diagrams have been analyzed by Chanfón (1991) and Sanabria (1984: 107–110); however, these authors do not examine the drawings exhaustively and do not furnish complete proofs for their assertions. Rodríguez et al. (2012) have carried out a thorough analysis of the first drawing, leaving aside the other three; their presentation does not show clearly the proportions of the nave bays. Thus, it is worthwhile to present here a detailed geometrical study of all four drawings.

The Double-Aisle Church in fols. 11 v–12v of the Compendio

The first drawing, found on fol. 12r of the Compendio, represents a double-aisle church, with a semicircular ambulatory (Figs. 2, 3).

Fig. 2

Church with double aisles in fol. 12r of Compendio de arquitectura y simetría de los templos. Biblioteca Nacional de España, MSS/8884. The image is property of Biblioteca Nacional de España. Reproduced by permission

Fig. 3

Church with double aisles in fol. 12r of Compendio de arquitectura y simetría de los templos, BNE, MSS/8884. Drawing by the authors

The plan resembles the cathedral of Segovia, where Rodrigo Gil acted as master mason (Hoag 1958: 160–170, 345–355; Casaseca 1988: 85–95). The whole temple is enclosed in a square. In order to determine the total width of the church, the author places points G and B at the middle of two sides of this square. The intersection of BG with the diagonal of the square, located at X, determines the width of the church. Next, he draws lines from points R and O, which divide the sides of the square into fourths, to B. Points 4 and Y, located at the intersections of these lines with a parallel to the side of the square drawn by X, determine the position of the arcades of the church, dividing the nave and the first and second aisles.

It is easy to see that CX is the diagonal of a square whose side equals 1/4 of the larger enclosing square, and thus the total width of the church is 1/2 the side of the enclosing square. Since the sanctuary is a semicircle, the proportion of the rest of the church is 3/2 or sesquialtera, as stated by Chanfón (1991: 39). The rest of the procedure seems to lead to complex and irregular results.¹ However, a detailed analysis shows different conclusions. For the sake of clarity, we will use the side of the enclosing square as a basic reference unit, U. It is clear that BG is the diagonal of a small square, that the sides of this small square equal 1/2 of the sides of the larger square, and that the intersection of BG and CN is the centre of the smaller square. Thus XT, which represents 1/2 the width of the church, equals 1/4 of the basic unit.

In order to compute the combined width of half the nave and the first aisle, we can take into account that triangles BT4 and BγR are similar, and the former measures 1/3 of the dimensions of the latter. Thus, T4, the combined width of half the nave and the first aisle, measures 1/3 of Rγ, that is, 1/6 of the basic unit. Similar triangles can also be used to measure the width of the nave. The dimensions of the small triangle BTY are 1/4 of those of the larger triangle BδO; thus, TY, half the width of the nave, equals 1/4 of δO, that is, 1/16 of the reference unit.

Unifying these measures through their least common multiple, we get 6/48 of the basic unit for the width of the nave, 5/48 for the first aisle and 4/48 for the second aisle. That is, an apparently capricious geometrical construction results in a neat series of simple arithmetical proportions, 4, 5, 6; even the proportion between nave and second aisle equals 2/3, that is, a perfect fifth, diapente or proportio sesquialtera.

Bay lengths do not follow such a neat scheme. Point 5, placed at the intersection of the line drawn from point B to point R and the diagonal of the enclosing square, NC, gives the division between the presbytery and the crossing. This is a typical trait of Spanish cathedrals and large churches; since the half-circle 1Y is usually insufficient for the presbytery, while the choir is placed at the other side of the crossing, a short rectangle is added in order to enlarge the sanctuary. Next, points S, Δ, and X, located at the intersections of the main diagonals CN and AN with the arcades, give the depths of the crossing, first and second bays of the nave. According to the text, the depths of subsequent bays equal that of the second one.

The depth of the shallow rectangle added to the presbytery can again be computed using similar triangles. Considering that the proportion between both catheti of triangles Bε5 and BγR is 2/3, and C5 is the diagonal of a square, it can be proved that Tε equals U/20 (see Appendix “Depth of the shallow rectangle added to the presbytery”). The rest is simpler, although there are some surprises. The depth of the crossing, given by the intersection of the diagonal with the first arcade, equals 11U/80. Since the width of the nave is U/8, the crossing is not square, departing from usual practice (see Appendix “Depth of the crossing”). In contrast, the first bay of the nave, which usually houses the choir in Spanish churches, is square; its depth, SΔ, is given by the intersections of the diagonals with the main arcades, at both sides of the nave. Thus, the depth of the first bay equals 1/8 of the basic unit, that is, 6U/48. The depth of the second bay is given by the intersection of the diagonal with the arcades, so it equals 5U/48, the width of the first aisle; this depth is used also for the third, fourth and fifth bays.²

There is another surprise. In the drawing, the aggregated depths of the ambulatory, the presbytery extension, the crossing and all five bays equal the length of the side of the enclosing square. However, when adding the numerical values of these depths, the result amounts to 47/48 of this basic unit; that is, the total length of the church does not fill the enclosing square, in contradiction with the drawing. It is not easy to tell whether this inconsistency between text and drawing comes from the original text of the Compendio, or was introduced by Simón García or an intermediate copyist. Hopefully, future studies on the authorship of the manuscript, which are out of the scope of this paper, will shed some light on this puzzling issue.³

As a final stage, the author of the Compendio explains a peculiar method for the division of the ambulatory into five bays. One of the division points, 8, is placed at the intersection of the line BR with the semicircle that divides the inner from the outer ambulatory. Then, the angle T84 is divided again into halves. Repeating the procedure at the other side of the church, the ambulatory is divided into five sections. As a result of bisection and axial symmetry, the first, second, fourth and fifth bays are equal in length; by contrast, nothing guarantees that the third, central section equals the other bays.

The position of the dividing point 8 can be computed today by writing the equations of line B4 and the semicircle that divides the inner and the outer ambulatory. Somewhat surprisingly, the coordinates of 8 are rational, namely (5/78, 2/13). Using this result, the magnitude of angle T84 can be determined as 1.176 radians or 67°22′47″. The angular measure of the four outer bays of the ambulatory equal 1/2 this value, that is, 33°41′24″, while the central bay measures 45°14′25″, appreciably larger than the side bays (see Appendix “Angular dimensions in the ambulatory”). The author of the Compendio mentions the retablo, or altarpiece, of the church in connection with this bay; this suggests that he is deliberately giving a larger span to the central bay.

The Single-Aisle Church in fols. 12v–13r of the Compendio

The second church in Chapter V features a single-aisle with a characteristic chamfered sanctuary, known in Spanish as ochavo (Figs. 4, 5).

Fig. 4

Church with single aisles in fol. 13r of Compendio de arquitectura y simetría de los templos. Biblioteca Nacional de España, MSS/8884. The image is property of Biblioteca Nacional de España. Reproduced by permission

Fig. 5

Church with single aisles in fol. 13r of Compendio de arquitectura y simetría de los templos, BNE, MSS/8884. Drawing by the authors

First, the author instructs the reader to construct an auxiliary square BBDD, as in the preceding example, but this time the side of the square equals the width of the church, not its length; in this way, the square will enclose only the chancel, the crossing and the first bay, not the whole church. In this section of the paper, we shall use the side of this square, U, as the basic reference unit.

After drawing the diagonals, the side of the square is again divided into four parts, so that a line can be drawn from point C to A. A crossing pier is placed at point F, where this line meets the diagonal of the square. Repeating this operation four times, the original square is divided into nine unequal portions. The central one will furnish the crossing; the four lateral ones will be used as the sanctuary, transepts and first bay of the nave. As to the four corner sections, two will be used for the first bay of the aisles, another one will house the sacristy, and the fourth one will be left uncovered.

Once again, such apparently haphazard methods conceal rational, arithmetical proportions. Considering that AαF and ABC are similar triangles, the proportion between their catheti is 2/3, and αBLF is a square, it can be proved that the width of the crossing and the nave equals 2/5 of the width of the church (see Appendix “Width of the crossing and aisles and depth of transepts”). The remainder of the side, 3/10 of the width, is given to the depth of the transepts and the width of the aisles.

The sanctuary chamfers are constructed using a peculiar method: a line is drawn from the upper right corner of the auxiliary square, B, to the upper left corner of the transept, L, while another one is drawn from the midpoint A of the upper side of the auxiliary square, to point C, on the right side of the auxiliary square. Then a line is drawn from the midpoint of the bottom side of the auxiliary square, E, through the intersection ε of lines LB and AC. The intersection K of this line and the upper side of the auxiliary square gives a corner of the chamfered sanctuary or ochavo. The other corner is given by the intersection H of the presbytery wall with line LB.

The shortest way for us to compute the positions of the sanctuary corners is to write the equations of lines LB and AC and determine the coordinates of point ε. This allows us to write the equation of line Eε; the coordinates of its intersection with the side of the enclosing square give a dimension of 4/21 basic units for the flat side of the ochavo. The position of the other corner in the chamfer, H, can be computed using the equation of line LB; the coordinates of its intersection with line HF are (7/10, 91/100). As we may expect, the length of the chamfer is irrational (see Appendix “Position of corners and length of chamfer in the sanctuary”).

While the first bay of the nave is included in the first auxiliary square, the remaining three bays are part of another auxiliary square. The depth of each bay is determined, again, by an apparently capricious geometrical tracing, using the intersection P of line BE with the arcade. Then, a line is drawn from point A through point P until it meets the side of the enclosing square at point R. Next, the distance between E and R is transferred to the axis of the church in order to determine the length of the second bay; the length of the third and fourth bays equals that of the second bay.

Taking into account that the rectangle γPδE is similar to ABDE, which is a half-square, and that both triangles AγP and PδR are also similar, it is easy to prove that ER equals 1/3 of the width of the church. This measure is rotated in order to compute the length of the second, third and fourth bays of the church. Thus, these three bays together exactly fill the second auxiliary square; there is no gap at the end of the church, in contrast with the preceding example (see Appendix “Length of the second, third and fourth bays of the church”). However, this neat result comes together with a surprise. The first bay, with a depth of 3/10 (0.30) of the side of the auxiliary squares, is actually shorter than the second bay, with 1/3 (0.33) of the side, in contrast with the solution of the preceding example.⁴

The Single-Aisle Church in fols. 13v–14r of the Compendio

Once again, the third temple in Chapter V is constructed through a geometrical procedure (Figs. 6, 7), which in this case involves rotating squares as in some schemes in the thirteenth-century portfolio of Villard de Honnecourt, drawn by a Hand IV, (Honnecourt 2009: 19v, 20r; see also Barnes’s study in the same volume, pp. 13, 127–129, 134–136), or the late fifteenth-century booklets of Mathes Roriczer (Shelby 1977: 85, 108, 109, 111).

Fig. 6

Church with single aisles in fol. 14r of Compendio de arquitectura y simetría de los templos. Biblioteca Nacional de España, MSS/8884. The image is property of Biblioteca Nacional de España. Reproduced by permission

Fig. 7

Church with single aisles in fol. 14r of Compendio de arquitectura y simetría de los templos, BNE, MSS/8884. Drawing by the authors

A first auxiliary square CCDD is drawn and then divided using the diagonals; their intersection divides the crossing from the sanctuary. A secondary set of diagonals is traced; its intersections, in turn, divide the nave and the aisles.

Although these geometrical constructions give incommensurable measures, these irrationals are not used for actual built members. Thus, the resulting forms feature simple arithmetical proportions as in the preceding examples. Of course, first-order diagonals divide the auxiliary square into halves, while second-order ones divide it into fourths. The edge of the crossing area equals 1/2 of the auxiliary square or the width of the church. The transepts are defined by rectangles measuring 1/2 by 1/4 of the width of the church. The width of the nave measures 1/2 of the width of the temple, while the aisles equal 1/4.

As in the preceding example, the depth of the second, third and fourth bays, which lie outside this first auxiliary square, is computed using a geometrical construction carried out inside the first auxiliary square. A line is drawn, starting from point A and passing through the intersection of the second-order diagonal MB and the arcade; where this line meets the edge of the auxiliary square, DD, a point L is marked. Then, the distance ML is rotated in order to compute the depth of the first bay of the nave; the second and third bays should equal the second one. It is quite easy to see that the proportion between the catheti of AαK and AML is the same, namely 1/3, so ML equals 1/3 of side of the enclosing square, that is, 1/3 of the width of the church. This dimension gives the depth of the nave bays; once again, they fit neatly inside a second auxiliary square.

One of these second-order diagonals, BA, is used together with the line CG in order to place points H and H, which give the position of the corners of the chamfered sanctuary. As in the preceding example, the position of these corners may be computed writing the equations of lines GC and EA (see Appendix “The Single-Aisle Church in fols. 13v–14r of the Compendio. Position of the corners and length of chamfer in the sanctuary”). As expected, the length of the chamfer is irrational, measuring √(9/200). Thus, except for the sanctuary chamfers, the proportions are simple arithmetical, even musical ones. The ratio of width to depth of the nave bays is 2/3, that is, sesquialtera, a perfect fifth; the shape of the crossings is a double square; even the enclosing rectangle of the sanctuary is 8/10, or 4/5.⁵

The Church with Side Chapels in fols. 14v–15r of the Compendio

Similar solutions, with one important exception, are used in the last temple in Chapter V (Figs. 8, 9).

Fig. 8

Church with side chapels in fol. 15r of Compendio de arquitectura y simetría de los templos. Biblioteca Nacional de España, MSS/8884. The image is property of Biblioteca Nacional de España. Reproduced by permission

Fig. 9

Church with side chapels in fol. 15r of Compendio de arquitectura y simetría de los templos, BNE, MSS/8884. Drawing by the authors

Again, the crossing and sanctuary are enclosed in an auxiliary square that is divided three times using diagonals; their intersection points give the position of the corners of the transepts and the chamfers in the sanctuary, and indirectly, the crossing, the single nave, and the typically Iberian side chapels, known as capillas hornacinas. The depth of the nave bays is computed using the length of one of these diagonals.

As for the crossing, transepts and sanctuary, in spite of the Gothic character of the tracing method, the fact is that the diagonals divide the auxiliary square into halves, fourths and eights, and the actual lengths of the diagonals are not used, except for the sanctuary chamfers. Thus, the side of the square crossing measures 1/2 of the auxiliary square, the depth of the transepts equals 1/4 of this basic unit, the depth of the chamfered portion of the sanctuary measures 1/8, and so on.

These rational, arithmetical measures are used also for the width of the nave and the side chapels, which equal 1/2 and 1/8 of the auxiliary square. However, when computing the depth of the nave bays, the author of the Compendio rotates a second-order diagonal, RN and brings it to the axis of the church. Of course, the result of such operation is an irrational, incommensurable proportion between width and depth of the nave, namely 1/√2 of the basic unit, while the proportion of the side chapels is √2 divided by four.⁶

It is evident, when seeing the whole ensemble of the church, that the nave does not fit into a second auxiliary square, nor does the church fit into a double square, as in the preceding examples. The proportion system of the church is therefore inconsistent. What is startling is that this could have been quite easily avoided, by making, for example, the depth of the nave bays equal to the combined width of half the nave and the depth of the side chapels, which equals 1/4 plus 1/8, that is, 3/8 of the basic unit. This dimension equals 0.375 in decimal notation, while the depth of the nave bays, as constructed in the Compendio, is 1/(2√2), that is, 0.35355, or 6% shorter; the difference would have been barely noticeable in practice.⁷

We can thus surmise that the author of the Compendio is completely indifferent to the notions of geometrical or arithmetical proportion, commensurability or inconmensurability. He uses a geometrical layout method that usually furnishes commensurable dimensions, but he does not eschew irrational proportions where they are brought about by geometrical logic—in particular, in sanctuary chamfers—or when he chooses them out of whimsy or, what seems more likely, out of his unawareness of the notion of commensurable proportions.

Conclusion: Graphical Rather than Geometrical

These examples should caution us against thinking in terms of a simplistic opposition between the arithmetical, rational, commensurable proportional systems of the Renaissance and the geometrical, irrational, incommensurable results of the tracing methods of the Middle Ages. The proportioning system described in Chapter V of the Compendio leads to three different situations. In most cases, simple, arithmetical proportions arise from two different mechanisms. In the double-aisle church and the single-aisle church on fols. 12v–13r, similar triangles foster simple proportions such as 4/5, 5/6 or 3/4. The use of enclosing squares, even when they are not strictly necessary and may encumber the physical execution of the full-scale layout, may derive from medieval design methods. This is much clearer in the single-aisle church in fols. 13v–14r and the church with side chapels, where the enclosing square is divided into halves using diagonals, the smaller squares resulting from this division are divided again into fourths of the enclosing square, and so on. All this leads to proportions such as 1/2, 1/4 or 2/5.

In most occasions, the irrational dimensions arising from this network of rotating squares are not used in order to design actual built members. There are exceptions, however. In some cases, the traditional chamfered layout of sanctuaries leads to irrational proportions; it is worthwhile to remark that the proportions between the flat sections, parallel to the enclosing squares, and other members of the layout, are generally rational. Thus, the irrational proportions of the chamfered sections should be seen as an unavoidable consequence of the use of this traditional layout, not a deliberate choice.

It is quite revealing to compare these ochavos with the proportions of the crypt of the chapel of the palace of Charles V in Granada, which features an octagonal plan and is covered by a lunette vault. The proportion between the width of the lunette and the radius of the octagon is 3/5, while the proportion between the depth and the width of the lunette is 1/2. Up to this moment, everything is rational and precise. However, the geometry of such a Classical form as the octagon brings about irrational dimensions; its side equals 2sin(π/8) times the radius of the octagon; as a result, the distance between a corner of the octagon and the starting point of the lunette is also an irrational. That is, the geometrical constraints of many figures, in particular the central plans that feature so prominently in Wittkower’s study, conspire to re-introduce the irrational proportions that Renaissance artists intended to exclude.

However, the irrationals in both medieval ochavos and classical octagons arise from unavoidable geometrical constraints. In contrast, the irrational dimensions of the bays in the last church in Chapter V are perfectly avoidable, as we have shown. This raises a puzzling question: since Rodrigo Gil was using mostly simple, numerical proportions, why should he bother with geometrical constructions?

Sergio Sanabria (1984: 109) argued that such methods are “as convoluted a mode of thinking as reading the Tarot or casting a horoscope”. It seems that Rodrigo Gil uses geometrical proportioning schemes following an established medieval tradition, for didactic and practical reasons; this also explains the use of geometrical methods by Serlio. Transferring—as opposed to reproducing—a reduced-scale drawing to a full-scale layout with the instruments of the period leads to errors of significant magnitude. Besides, it is not easy to divide a dimension into thirds or fifths, in particular when tracing at full scale on the ground.⁸ At the same time, it is essential to take into account that this tradition adhered to neither that of classical abstract geometry, nor to the practical geometry tradition of the Middle Ages, which was rooted in learned geometry. It was rather a “constructive geometry” or “geometria fabrorum” (Shelby 1972: 409; Sanabria 1984: 39; Ruiz de la Rosa: 2005), based in an empiric use of rulers and compasses, or better still, ropes, eschewing abstract concepts. Rodrigo Gil’s proportional system may be aptly described as “graphical”, rather than arithmetical or geometrical; he seems to have believed that the network of lines, drawn with pens or ropes, justifies in some way the proportions of any element of his churches, either rational or irrational. This explains his indifference to the notions of commensurability and incommensurability; paradoxically both Wittkower’s and Von Simson’s opposing stances are coherent with this graphical approach to proportion, since it furnishes both rational and irrational proportions.

Appendix

In this Appendix, we shall maintain the notation in the drawings of the Compendio, which uses both numbers and Latin letters. In order to avoid confusion with actual numbers, the numbers used in the Compendio as notation will be followed by a prime symbol (′); a few points that do not carry notation in the Compendio will be marked with Greek letters.

The Double-Aisle Church in fols. 11 v–12v of the Compendio

In this section, we shall take the length AC of the side of the enclosing square in Fig. 3 as a basic reference unit, U.

Depth of the Shallow Rectangle Added to the Presbytery

In order to compute the depth of the shallow rectangle, Tɛ, we should take into account that C5′ is the diagonal of a square, and thus

$$5 ' {\text{F}} = {\text{CF}} = {\text{B}}\upvarepsilon ;\quad 5 '\upvarepsilon = {\text{BC}} - 5 ' {\text{F}}; 5 '\upvarepsilon = {\text{BC}} - {\text{B}}\upvarepsilon .$$

Further, since triangles 5′ε B and RγB are similar,

$$\frac{5 '\upvarepsilon }{{{\text{B}}\upvarepsilon }} = \frac{{{\text{R}}\upgamma }}{{{\text{B}}\upgamma }} = 2/3;$$

$$\frac{{{\text{BC}} - {\text{B}}\upvarepsilon }}{{{\text{B}}\upvarepsilon }} = \frac{{5^{ '} \upvarepsilon }}{{{\text{B}}\upvarepsilon }} = \frac{2}{3}; {\text{BC}} - {\text{B}}\upvarepsilon = \frac{2}{3}\cdot {\text{B}}\upvarepsilon ;\quad {\text{BC}} = \frac{5}{3}\cdot {\text{B}}\upvarepsilon ;$$

$${\text{B}}\upvarepsilon = \frac{3 }{5}\cdot {\text{BC}} = \frac{3}{5} \cdot \frac{1}{2} \cdot {\text{U }} = \frac{3}{10} \cdot {\text{U}}.$$

Since point D divides CN into 1/4 and 3/4 and BT = CD = 1/4U,

$${\text{T}}\upvarepsilon = {\text{B}}\upvarepsilon - {\text{BT}} = \left[ {\frac{3 }{10}{-} \frac{1}{4}} \right] {\text{U }} = \frac{1}{20} {\text{U}}.$$

Depth of the Crossing

The depth of the crossing, FH, can be computed taking into account that

$${\text{FH}} = {\text{CH }}\,{-}\, {\text{BE}} = {\text{CH}} - {\text{CF}} = {\text{HS}} - {\text{CF}}.$$

Now, HS is the combined length of NO, which equals 1/4 of the reference unit, since O divides the side of the enclosing square into 1/4 and 3/4; plus PO, the width of the second aisle, which measures 4U/48, as seen in the text; plus Y4, the width of the first aisle, which equals 5U/48, also seen in the text. Besides, CF equals Bε, measuring 3U/10, as seen before. Thus, the depth of the crossing equals

$$\begin{aligned} {\text{FH}} = {\text{NO }} + {\text{PO }} + {\text{Y}}4 ' {-} {\text{CF}} = 12/48 {\text{U }} + 4/48 {\text{U }} + 5/48 {\text{U }}{-} 3/10 {\text{U = }} \hfill \\ \left( {\frac{21}{48} - \frac{3}{10}} \right)\cdot {\text{U}} = \frac{11}{80}\cdot {\text{U}}. \hfill \\ \end{aligned}$$

Angular Dimensions in the Ambulatory

Placing the origin of coordinates at point T and taking into account that BT equals 1/4U and T4 equals 8/48U, and thus BT = 3/2 T4, the equation of line B4′ can be written as

$$y = 1/4 {-} 3/2 x,$$

(1)

while taking into account that T4 = 8U/48 = U/6, the equation of the semicircular arcade between the outer and inner ambulatory can be expressed as

$$x^{2} + y^{2} = 1/36.$$

(2)

Substituting y in the Eq. (2),

$$x^{2} + \left( {\frac{1}{4} - \frac{3}{2}x} \right)^{2} = \frac{1}{36}; \frac{13}{4}x^{2} - \frac{3}{4}x + \frac{5}{144} = 0;$$

$$\begin{aligned} x & = \frac{{\frac{3}{4} \pm \sqrt {\left( {\frac{9}{{16}}} \right) - \left( {4\cdot\frac{{13}}{4}\cdot\frac{5}{{144}}} \right)} }}{{\frac{{13}}{2}}} = \frac{{\frac{3}{4} \pm \sqrt {\frac{{81 - 65}}{{144}}} }}{{\frac{{13}}{2}}} \\ & = \frac{{\frac{3}{4} \pm \sqrt {\frac{{16}}{{144}}} }}{{\frac{{13}}{2}}} = \frac{{\frac{3}{4} \pm \frac{4}{{12}}}}{{\frac{{13}}{2}}} = \frac{{\frac{3}{4} \pm \frac{1}{3}}}{{\frac{{13}}{2}}} = \frac{{\frac{3}{2} \pm \frac{2}{3}}}{{13}} = \frac{{9 \pm 4}}{{78}}. \\ \end{aligned}$$

The first solution of this equation,

$$x = \frac{13}{78} = \frac{1}{6},$$

corresponds to the abscissa of point 4′. The second solution

$$x = \frac{5}{78}$$

gives the abscissa of point 8′. Substituting this value in the equation of line B4′, the ordinate of point 8′ equals

$$y = \frac{1}{4} - \frac{3}{2}\cdot\frac{5}{78} = \frac{1}{4} - \frac{5}{52} = \frac{2}{13}.$$

The dimension of angle 8′T4′ equals

$$arc tg (2/13)/(5/78),$$

that is, 1.176 radians or 67º22′48″.

The Single-Aisle Church in fols. 12v–13r of the Compendio

In this section, we shall take the length of the side of the square BBDD in Fig. 5 as a basic reference unit, U.

Width of the Crossing and Aisles and Depth of Transepts

From Fig. 5, since BF is the diagonal of a square,

$${\text{BL}} = {\text{B}}\upalpha = {\text{AB}} - {\text{A}}\upalpha = 1/2 \cdot {\text{U}} - {\text{A}}\upalpha .$$

Also, since triangles AαF and ABC are similar and C divides BD into 1/3 and 2/3,

$$\frac{{{\text{A}}\upalpha }}{\text{BL}} = \frac{{{\text{A}}\upalpha }}{{\upalpha {\text{F}}}} = \frac{\text{AB}}{\text{BC}} = 2/3,$$

$${\text{A}}\upalpha = \frac{2}{3}{\text{BL}} = \frac{2}{3}{\text{B}}\upalpha = \frac{2}{3}\left( {\frac{1}{2}\cdot {\text{U}} - {\text{A}}\upalpha } \right) = \frac{1}{3}\cdot {\text{U }}{-}\frac{2}{3}{\text{A}}\upalpha ;$$

$$\frac{5}{3}{\text{A}}\upalpha = \frac{1}{3}\cdot {\text{U}}; {\text{A}}\upalpha = \frac{1}{5}\cdot {\text{U}}.$$

This dimension equals half the width of the crossing, so the full width of the crossing and the nave measures 2/5 of the basic unit. As for the depth of the transepts, which equals the width of the aisles,

$${\text{B}}\upalpha = \frac{1}{2}\cdot {\text{U}} - \frac{1}{5}\cdot {\text{U}} = \frac{3}{10}\cdot {\text{U}} .$$

Position of Corners and Length of Chamfer in the Sanctuary

From Fig. 5, placing the origin of coordinates at the lower left corner D, the equation of line LB, going from point L at the left side to B in the upper right corner, is

$$y = \frac{ 7}{10} + \frac{3}{10} x.$$

(3)

The equation of line AC, from A in the middle of the upper side to C on the right side, is,

$$y = 1 - \frac{3 }{2}\left[ {x {-} \frac{1}{2}} \right] = 1 {-} \frac{3}{2} x + \frac{3}{4} = \frac{7 }{4}{-} \frac{3}{2} x.$$

(4)

The coordinates of point ε, at the intersection of both lines, must fulfill both equations.

$$\frac{7}{10} + \frac{3}{10}x = \frac{7}{4}{-}\frac{3}{2}x; \left( {\frac{3}{10} + \frac{3}{2}} \right) x = \frac{7}{4}{-}\frac{7}{10};$$

$$\frac{9}{5}x = \frac{70 - 28}{40} = \frac{21}{20}; x = \frac{21 \cdot 5}{20 \cdot 9} = \frac{7}{12}$$

Substituting x in Eq. (3),

$$y = (7/10) + (3/10) \cdot (7/12) = (7/10) + (21/120) = 7/8;$$

thus, the coordinates of ε are (7/12, 7/8).

Using these coordinates, the equation of line Eε can be written as:

$$y = \left[ {x {-} \frac{1}{2}} \right] \cdot \frac{{\frac{7}{8}}}{{\frac{1}{12}}} = \frac{21 }{2}(x {-} 1/2) .$$

The equation of line BB is y = 1. Thus,

$$\frac{21 }{2}\left[ {x - \frac{1}{2}} \right] = 1; x = \frac{2}{21} + \frac{1}{2} = \frac{25}{42} ,$$

and the coordinates of K are (25/42, 1). Since the abscissa of A is 1/2, which equals 21/42, the distance between A and K is 4/42 = 2/21; the dimension of the flat side of the ochavo is twice this distance, that is, 4/21.

As seen before in Eq. (3), the equation of line LB is

$$y = 7/10 + 3/10 x.$$

The abscissa of point H equals 3/10 + 4/10 = 7/10. Substituting this value,

$$y = 7/10 + (3/10) \cdot (7/10) = 7/10 \cdot 13/10 = 91/100.$$

Thus, the coordinates of point H are (7/10, 91/100). Taking this into account, the length of the chamfer equals

$$\begin{aligned} \sqrt {\left[ {\frac{7}{10} - \frac{25}{42}} \right]^{2} + \left[ {1 - \frac{91}{100}} \right]^{2} } &= \sqrt {\left[ {\frac{11}{105}} \right]^{2} + \left[ {\frac{9}{100}} \right]^{2} } \hfill \\ = \sqrt {\frac{121}{11025} + \frac{81}{10000}} &= \sqrt {\frac{84121}{4410000}} . \hfill \\ \end{aligned}$$

Length of the second, third and fourth bays of the church

In Fig. 5, both Eδ and Pγ equal half the width of the nave, that is, 1/5 the basic unit, as seen in Sect. “Width of the crossing and aisles and depth of transepts”. Besides, triangles BDE and PδE are similar; thus

$$\frac{{{\text{P}}\updelta }}{{{\text{E}}\updelta }} = \frac{\text{BD}}{\text{DE}} = 2; {\text{P}}\updelta = 2 \cdot {\text{E}}\updelta = 2/5 \cdot {\text{U}}.$$

Also,

$${\text{A}}\upgamma = {\text{U}} - {\text{P}}\updelta = {\text{U}} - \frac{2}{5}\cdot {\text{U}} = \frac{3}{5}\cdot {\text{U}}.$$

Further, since triangles AγP and PδR are also similar,

$$\frac{\text{ER}}{\text{AE}} = \frac{{\upgamma {\text{P}}}}{{{\text{A}}\upgamma }}; {\text{ER}} = {\text{U }}\frac{{\upgamma {\text{P}}}}{{{\text{A}}\upgamma }} = {\text{U }}\frac{{\frac{1}{5}{\text{U}}}}{{\frac{3}{5}{\text{U}}}} = \frac{1}{3}{\text{U}} .$$

This dimension is rotated in order to construct ES, the depth of the second bay of the church, and used also to lay out the third and fourth bays; thus, the combined depth of the second, third and fourth bays equals the reference unit, that is, BD, and the whole church is inscribed in a double square.

The Single-Aisle Church in fols. 13v–14r of the Compendio: Position of the corners and length of chamfer in the sanctuary

From Fig. 7, using the side of square CCDD as a reference unit and placing the origin of coordinates at point C at the upper left corner of the enclosing square, the equation of line CH, from the upper left corner to point G in the right side, can be written as

$$y = - 1/4 x,$$

(5)

while the one for line BEA, from B in the left side to A in the upper side is

$$y = x - 1/2.$$

(6)

Setting the two equations equal to each other,

$$\begin{aligned} - \frac{1}{4}x = x - \frac{1}{2}; \frac{5}{4}x = \frac{1}{2}; x = \frac{1}{2}\cdot\frac{4}{5} = \frac{2}{5}; \hfill \\ y = - \frac{1}{4}x = - \frac{1}{10} \hfill \\ \end{aligned}$$

Thus, the coordinates of H are (2/5, −1/10). The length of the flat section of the sanctuary equals

$$2 \cdot \left[ {\frac{1}{2} - \frac{2}{5}} \right] \cdot {\text{U }} = \frac{1}{5} \cdot {\text{U}},$$

while the length of the chamfer equals

$$\sqrt {\left( {\frac{3}{20}} \right)^{2} + \left( {\frac{3}{20}} \right)^{2} } = \sqrt {\frac{9}{200}} \cdot {\text{U}} .$$

The Church with Side Chapels in fols. 14v–15r of the Compendio. Proportion of the side chapels

From Fig. 9, taking as reference unit U the side of the square CCDD, the front of each side chapel measures 1/2√2 of the basic unit, as seen in the text, while the depth of the chapel is 1/8 of the reference unit; thus, the proportion of the chapel is

$$\frac{1/8}{1/2\sqrt 2 } = \frac{2\sqrt 2 }{8} = \frac{\sqrt 2 }{4} .$$