Synchronization Phenomena in Coupled Hyperchaotic Oscillators with Hidden Attractors Using a Nonlinear Open Loop Controller

Abstract

In recent years the study of dynamical systems with hidden attractors, namely systems in which their basins of attraction do not intersect with small neighborhoods of equilibria, is a great challenge due to their application in many research fields such as in mechanics, secure communication and electronics. Especially, the investigation of hyperchaotic systems with hidden attractors plays a crucial role in this research approach. Motivated by the very complex dynamical behavior of hyperchaotic systems and the unusual features of hidden attractors, a bidirectionally and unidirectionally coupling scheme of systems of this family, by using a nonlinear open loop controller, is studied in this chapter. For this reason, a recently new proposed hyperchaotic system with hidden attractors, the four-dimensional modified Lorenz system, which is structurally the simplest hyperchaotic system with hidden attractors, is used. The simulation results show that the proposed scheme drives the coupled system either to complete synchronization or anti-synchronization depending on the choice of the signs of the error function’s parameters. In addition, an electronic circuit emulating the control scheme of the coupled hyperchaotic systems with hidden attractors is also presented to verify the feasibility of the proposed model.

1 Introduction

In the last three decades the phenomenon of synchronization between coupled chaotic systems has attracted the interest of the scientific community because it is a rich and multi-disciplinary phenomenon with broad range applications, such as in secure communications [19] and cryptography [14, 60], in broadband communications systems [7] and in a variety of complex physical, chemical, and biological systems [17, 37, 41, 51, 54, 57, 62]. In general, synchronization of chaos is a process, where two or more chaotic systems adjust a given property of their motion to a common behavior, such as equal trajectories or phase locking, due to coupling or forcing. Because of the exponential divergence of the nearby trajectories of a chaotic system, having two chaotic systems being synchronized, might be a surprise. However, today the synchronization of coupled chaotic oscillators is a phenomenon well established experimentally and reasonably well understood theoretically.

The history of chaotic synchronization’s theory began with the study of the interaction between coupled chaotic systems in the 1980s and early 1990s by Fujisaka and Yamada [11], Pikovsky [49], Pecora and Carroll [48]. Since then, a wide range of research activity based on synchronization of nonlinear systems has risen and a variety of synchronization’s forms depending on the nature of the interacting systems and of the coupling schemes has been presented. Complete or full chaotic synchronization [9, 24–26, 28, 39, 55, 63], phase synchronization [8, 45], lag synchronization [52, 56], generalized synchronization [53], anti-synchronization [22, 36], anti-phase synchronization [1, 5, 6, 27, 58, 64], projective synchronization [38], anticipating [61] and inverse lag synchronization [34] are the most interesting types of synchronization, that have been investigated numerically and experimentally by many research groups.

This work is referred to complete synchronization and to anti-synchronization. In the first case, which is the most studied type of synchronization, two identical coupled chaotic systems leads to a perfect coincidence of their chaotic trajectories i.e., $x_{1}(t)=x_{2}(t)$ as $t \ \rightarrow \ \infty$. In the anti-synchronization, on the other hand, which is also a very interesting type of synchronization, two systems $x_{1}$ and $x_{2}$, can be synchronized in amplitude, but with opposite sign, for initial conditions chosen from large regions in the phase space, that is $x_{1}(t) = -x_{2}(t)$ as $t \ \rightarrow \ \infty$.

As it is known, nonlinear systems and especially chaotic systems exhibit high sensitivity on initial conditions or system’s parameters and thus, if they are identical and, possibly, starting from almost the same initial conditions, following trajectories which rapidly become uncorrelated. For this reason, many techniques have been set up to obtain the aim of chaotic synchronization. So, many of these techniques to couple two or more nonlinear chaotic systems can be mainly divided into two classes: bidirectional or mutual coupling and unidirectional coupling [13]. In the mutual coupling both the systems are connected and each system’s dynamic behavior influences the dynamics of the other, while on the contrary in unidirectional coupling, only the first system drives the second one.

Recently, a great interest for dynamical systems with hidden attractors has been raised. The term “hidden attractor” is referred to the fact that in this class of systems the attractor is not associated with an unstable equilibrium and thus often remains undiscovered because it may occur in a small region of parameter space and with a small basin of attraction in the space of initial conditions [23, 31–33, 46, 47]. In 2010, for the first time, a chaotic hidden attractor was discovered in the most well-known nonlinear circuit, in Chua’s circuit, which is described by a three-dimensional dynamical system [23, 31].

The problem of analyzing hidden oscillations arose for the first time in the second part of Hilbert’s 16th problem (1900) for two-dimensional polynomial systems [16]. The first nontrivial results were obtained in Bautin’s works [2, 3], which were devoted to constructing nested limit cycles in quadratic systems and showed the necessity of studying hidden oscillations for solving this problem.

Later, in the middle of the 20th century, Kapranov studied [21] the qualitative behavior of Phase-Locked Loop (PLL) systems, which are used in telecommunications and computer architectures, and estimated stability domains. In that work, Kapranov assumed that in PLL systems there were self-excited oscillations only. However, in 1961, Gubar’ [15] revealed a gap in Kapranov’s work and showed analytically the possibility of the existence of hidden oscillations in two-dimensional system of PLL, thus, from a computational point of view, the system considered was globally stable, but, in fact, there was only a bounded domain of attraction.

Also, in the same period, the investigations of widely known Markus–Yamabe [40] and Kalman [20] conjectures on absolute stability have led to the finding of hidden oscillations in automatic control systems with a unique stable stationary point and with a nonlinearity, which belongs to the sector of linear stability [4, 10, 30].

Furthermore, systems with hidden attractors have received attention due to their practical and theoretical importance in other scientific branches, such as in mechanics (unexpected responses to perturbations in a structure like a bridge or in an airplane wing) [18, 29]. So, the study of these systems is an interesting topic of a significant importance.

In this work a hyperchaotic four-dimensional modified Lorenz system with hidden attractors, is used for studying the bidirectional or unidirectional coupling by using the nonlinear open loop controller. The simulation results from system’s numerical integration as well as the circuital implementation of the proposed system in SPICE, confirm the appearance of complete synchronization and anti-synchronization phenomena depending on the signs of the parameters of the error functions.

The chapter is organized as follows. In Sect. 2 the four-dimensional modified Lorenz system, which is used in this work, is presented. The scheme, by using the nonlinear open loop controller, in both coupling ways (bidirectional and unidirectional) as well as the simulation results are discussed in Sect. 3. Section 4 presents the circuital implementation of the unidirectional coupling system and the simulation results which are obtained by using SPICE. Finally, the conclusive remarks are drawn in the last section.

2 The Four-Dimensional Modified Lorenz System

In this work the simplest four-dimensional hyperchaotic Lorenz-type system, which has been proposed by Gao and Zhang [12], is used. This system is an extension of a modified Lorenz system, which was studied by Schrier and Maas as well as by Munmuangsaen and Srisuchinwong [42, 59]. The proposed system is described by the following set of differential equations.

$$\begin{aligned} \left\{ {\begin{array}{l} \dot{x}=y-x \\ \dot{y}=-xz+u \\ \dot{z}=xy-c \\ \dot{u}=-dy \\ \end{array}} \right. \end{aligned}$$

(1)

Fig. 1

Bifurcation diagram of y versus d for $c = 5$, with initial conditions ($x_0$, $y_0$, $z_0$, $u_0) = (0.55, -0.49, -0.08, 0.50)$

Fig. 2

Bifurcation diagram of y versus d for $c = 4$, with initial conditions ($x_{0}$, $y_{0}$, $z_{0}$, $u_{0}) = (0.55, -0.49, -0.08, 0.50)$

Fig. 3

Bifurcation diagram of y versus d for $c = 3.5$, with initial conditions ($x_{0}$, $y_{0}$, $z_{0}$, $u_{0}) = (0.55, -0.49, -0.08, 0.50)$

Fig. 4

Bifurcation diagram of y versus d for $c = 2.97$, with initial conditions ($x_{0}$, $y_{0}$, $z_{0}$, $u_{0}) = (0.55, -0.49, -0.08, 0.50)$

Fig. 5

Bifurcation diagram of y versus d for $c = 2.9$, with initial conditions ($x_{0}$, $y_{0}$, $z_{0}$, $u_{0}) = (0.55, -0.49, -0.08, 0.50)$

Fig. 6

Bifurcation diagram of y versus d, for $c = 2.7$, with initial conditions ($x_{0}$, $y_{0}$, $z_{0}$, $u_{0}) = (0.55, -0.49, -0.08, 0.50)$

Fig. 7

Bifurcation diagram of y versus d, for $c = 1$, with initial conditions ($x_{0}$, $y_{0}$, $z_{0}$, $u_{0}) = (0.55, -0.49, -0.08, 0.50)$

Fig. 8

Phase portrait of z versus x for $c = 2.7$ and $d = 0.9$ (period-1 state), with initial conditions ($x_{0}$, $y_{0}$, $z_{0}$, $u_{0}) = (0.55, -0.49, -0.08, 0.50)$

Fig. 9

Quasi-periodic attractor for $c = 2.7$ and $d = 0.75$, in x–y plane, with initial conditions ($x_{0}$, $y_{0}$, $z_{0}$, $u_{0}) = (0.55, -0.49, -0.08, 0.50)$

Fig. 10

Quasi-periodic attractor for $c = 2.7$ and $d = 0.75$, in x–z plane, with initial conditions ($x_{0}$, $y_{0}$, $z_{0}$, $u_{0}) = (0.55, -0.49, -0.08, 0.50)$

Fig. 11

Quasi-periodic attractor for $c = 2.7$ and $d = 0.75$, in x–u plane, with initial conditions ($x_{0}$, $y_{0}$, $z_{0}$, $u_{0}) = (0.55, -0.49, -0.08, 0.50)$

Fig. 12

Quasi-periodic attractor for $c = 2.7$ and $d = 0.75$, in y–z plane, with initial conditions ($x_{0}$, $y_{0}$, $z_{0}$, $u_{0}) = (0.55, -0.49, -0.08, 0.50)$

Fig. 13

Quasi-periodic attractor for $c = 2.7$ and $d = 0.75$, in y–u plane, with initial conditions ($x_{0}$, $y_{0}$, $z_{0}$, $u_{0}) = (0.55, -0.49, -0.08, 0.50)$

Fig. 14

Phase portrait of z versus x for $c = 2.7$ and $d = 0.2$ (chaotic state), with initial conditions ($x_{0}$, $y_{0}$, $z_{0}$, $u_{0}) = (0.55, -0.49, -0.08, 0.50)$

Fig. 15

Hyperchaotic attractor for $c = 2.7$ and $d = 0.44$, in x–y plane, with initial conditions ($x_{0}$, $y_{0}$, $z_{0}$, $u_{0}) = (0.55, -0.49, -0.08, 0.50)$

Fig. 16

Hyperchaotic attractor for $c = 2.7$ and $d = 0.44$, in x–z plane, with initial conditions ($x_{0}$, $y_{0}$, $z_{0}$, $u_{0}) = (0.55, -0.49, -0.08, 0.50)$

Fig. 17

Hyperchaotic attractor for $c = 2.7$ and $d = 0.44$, in x–u plane, with initial conditions ($x_{0}$, $y_{0}$, $z_{0}$, $u_{0}) = (0.55, -0.49, -0.08, 0.50)$

It is structurally a very simple four-dimensional dynamical system having only two independent parameters (c, d). Also, as it is mentioned in [35], it has many interesting properties not found in other proposed systems, such as:

• (i) It has very few terms, only seven with two quadratic nonlinearities, and two parameters.

• (ii) All its attractors are hidden.

• (iii) It exhibits hyperchaos over a large region of parameter space.

• (iv) Its Jacobian matrix has rank less than four everywhere in the space of the parameters.

• (v) It exhibits a quasi-periodic route to chaos with an attracting torus for some choice of parameter values.

• (vi) It has regions in which the torus coexists with either a symmetric pair of strange attractors or a symmetric pair of limit cycles and other regions where three limit cycles coexist.

• (vii) The basins of attraction have an intricate fractal structure.

• (viii) There is a series of Arnold tongues [43] within the quasi-periodic region where the two fundamental oscillations mode-lock and form limit cycles of various periodicities.

All the afore-mentioned reasons make the dynamical system (1) an ideal candidate for the coupling scheme which is used in this work. Especially, the existence of hidden attractors and the hyperchaotic nature of a system like this have played a crucial role in our decision.

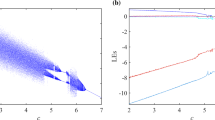
In this section the system’s dynamic behavior is investigated numerically by employing a fourth order Runge–Kutta algorithm. For this reason, the bifurcation diagram, which is a very useful tool from nonlinear theory, is used. In Figs. 1, 2, 3, 4, 5, 6 and 7 the bifurcation diagrams of the variable y versus the parameter d, for various values of the parameter c, reveal the richness of system’s dynamical behavior. Besides limit cycles, system (1) has quasi-periodicity, chaos, and hyperchaos, which can make the control of the system a difficult case in practical applications where a particular dynamic is desired. In more, details, as the value of d is decreased from $d = 0.9$ the system goes from a period-1 steady state (Fig. 8), through a quasi-periodic route (Figs. 9, 10, 11, 12 and 13), to a chaotic state, which is confirmed by the chaotic attractor in x–z plane, that is shown in Fig. 14. However, a very interesting feature of the specific system is the existence of hyperchaos for a range of parameters as it is shown in the phase portraits of Figs. 15, 16, 17, 18 and 19. Figure 20 shows the Lyapunov exponents’ spectra for chosen value of the parameter c ($c = 2.7$). The system’s hyperchaotic behavior is found for $c = 2.7$ in the range of $d\in [0.388, 0.49]$ (Figs. 15, 16, 17, 18 and 19), where the system has two positive Lyapunov exponents, as it is shown in the embedded diagram in Fig. 20.

Fig. 18

Hyperchaotic attractor for $c = 2.7$ and $d = 0.44$, in y–z plane, with initial conditions ($x_{0}$, $y_{0}$, $z_{0}$, $u_{0}) = (0.55, -0.49, -0.08, 0.50)$

Fig. 19

Hyperchaotic attractor for $c = 2.7$ and $d = 0.44$, in y–u plane, with initial conditions ($x_{0}$, $y_{0}$, $z_{0}$, $u_{0}) = (0.55, -0.49, -0.08, 0.50)$

Fig. 20

The diagrams of Lyapunov exponents ($\lambda$ $_{i})$ versus the parameter d, for $c = 2.7$

3 The Coupling Scheme

Two identical coupled chaotic systems can be described by the following system of differential equations:

$$\begin{aligned} \left\{ {\begin{array}{l} \dot{x}=f(x)+U_X \\ \dot{y}=f(y)+U_Y \\ \end{array}} \right. \end{aligned}$$

(2)

where $(f(x), f(y))\in R^{n}$ are the flows of the systems. The coupling of the systems is defined by the Nonlinear Open Loop Controllers (NOLCs), $U_{X}$ and $U_{Y}$ [44]. The error function is given by $e = {\beta y} - {\alpha x}$, where $\alpha$ and $\beta$ are constants. If one applies the Lyapunov Function Stability (LFS) technique, a stable synchronization state will be realized when the error function of the coupled system follows the limit

$$\begin{aligned} \mathop {\mathrm{lim}}\limits _{t\rightarrow \infty } \left| {\left| {e(t)} \right| } \right| \rightarrow 0 \end{aligned}$$

(3)

so that $\alpha x = \beta y$.

As it is mentioned, the design process of the coupling scheme, is based on the Lyapunov function

$$\begin{aligned} V(e)=\frac{1}{2}e^{T}e \end{aligned}$$

(4)

where T denotes transpose of a matrix and V(e) is a positive definite function. For known system’s parameters and with the appropriate choice of the controllers $U_{X}$ and $U_{Y}$, the coupled system has $\dot{V}(e)<0$. This ensures the asymptotic global stability of synchronization and thereby realizes any desired synchronization state.

By using the appropriate NOLCs functions $U_{X}, U_{Y}$ and error function’s parameters $\alpha$, $\beta$, a unidirectional or bidirectional (mutual) coupling scheme can be implemented. In more details, for ($U_{X} = 0, \beta = 1)$ or ($U_{Y}$ = 0, $\alpha$ = 1), a unidirectional coupling scheme is realized, while for $U_{X,Y} \ne 0$ and $\alpha$, $\beta$ $\ne$ 0, a bidirectional coupling scheme is realized, respectively. The signs of $\alpha$, $\beta$ play a crucial role to the type of synchronization (complete synchronization or anti-synchronization), which is observed in this work. On the other hand, the ratio of $\alpha$ over $\beta$ decides the amplification or attenuation of one oscillator relative to another one.

Next, the results of the simulation process in the two coupling (bidirectional and unidirectional) schemes and for various values of parameters $\alpha$ and $\beta$ are presented.

3.1 Bidirectional Coupling

Systems of chaotic oscillators bidirectionally (mutually) coupled are frequently found not only in the simulation environment or the laboratory but also in the natural world [41, 50]. This way of coupling, which is the simplest, is very interesting because it displays much of the phenomenology that is observed in more complex networks. Asymptotically stable synchronization between the coupled oscillators happens to be one of the basic phenomena that is observed.

As it is mentioned, the synchronization of coupled chaotic systems is a process where two or more systems adjust a given property of their motion to a common behavior, such as identical trajectories, due to coupling.

So, in the first case, the bidirectional coupling scheme of two coupled systems of Eq. (1), which is described by the following systems (5) and (6), is studied.

Coupled System-1:

$$\begin{aligned} \left\{ {\begin{array}{l} \dot{x}_1 =x_2 -x_1 +U_{X1} \\ \dot{x}_2 =-x_1 x_3 +x_4 +U_{X2} \\ \dot{x}_3 =x_1 x_2 -c+U_{X3} \\ \dot{x}_4 =-dx_2 +U_{X4} \\ \end{array}} \right. \end{aligned}$$

(5)

Coupled System-2:

$$\begin{aligned} \left\{ {\begin{array}{l} \dot{y}_1 =y_2 -y_1 +U_{Y1} \\ \dot{y}_2 =-y_1 y_3 +y_4 +U_{Y2} \\ \dot{y}_3 =y_1 y_2 -c+U_{Y3} \\ \dot{y}_4 =-dy_2 +U_{Y4} \\ \end{array}} \right. \end{aligned}$$

(6)

where $U_{X }= [U_{X1}, U_{X2}, U_{X3}, U_{X4}]^{\text {T}}$ and $U_{Y }= [U_{Y1}, U_{Y2}, U_{Y3}, U_{Y4}]^{\text {T}}$ are the NOLCs functions. The error function is defined by ${\varvec{e}} = \beta {\varvec{y}} - \alpha {\varvec{x}}$, with ${\varvec{e}} = [e_{1}, e_{2}, e_{3}, e_{4}]^{\text {T}}$, ${\varvec{x}} = [x_{1}, x_{2}, x_{3}, x_{4}]^{\text {T}}$ and ${\varvec{y}} = [y_{1}, y_{2}, y_{3}, y_{4}]^{\text {T}}$. So, the errors dynamics, by taking the difference of Eqs. (5) and (6), are written as:

$$\begin{aligned} \left\{ {\begin{array}{l} \dot{e}_1 =e_2 -e_1 +\beta U_{Y1} -\alpha U_{X1} \\ \dot{e}_2 =\alpha x_1 x_3 -\beta y_1 y_3 +e_4 +\beta U_{Y2} -\alpha U_{X2} \\ \dot{e}_3 =-\alpha x_1 x_2 +\beta y_1 y_2 -c(\beta -\alpha )+\beta U_{Y3} -\alpha U_{X3} \\ \dot{e}_4 =-de_2 +\beta U_{Y4} -\alpha U_{X4} \\ \end{array}} \right. \end{aligned}$$

(7)

For stable synchronization $e \rightarrow 0$ as $t \rightarrow \infty$. By substituting the conditions in Eq. (7) and taking the time derivative of Lyapunov function

$$\begin{aligned} \dot{V}(e)&=e_1 \dot{e}_1 +e_2 \dot{e}_2 +e_3 \dot{e}_3 +e_4 \dot{e}_4 \nonumber \\&=e_1 \left( {e_2 -e_1 +\beta U_{Y1} -\alpha U_{X1} } \right) \nonumber \\&\quad +e_2 \left( {\alpha x_1 x_3 -\beta y_1 y_3 +e_4 +\beta U_{Y2} -\alpha U_{X2} } \right) \nonumber \\&\quad +e_3 \left[ {-\alpha x_1 x_2 +\beta y_1 y_2 -c\left( {\beta -\alpha } \right) +\beta U_{Y1} -\alpha U_{X1} } \right] \nonumber \\&\quad +e_4 \left( {-de_2 +\beta U_{Y1} -\alpha U_{X1} } \right) \end{aligned}$$

(8)

we consider the following NOLC controllers:

$$\begin{aligned} \left\{ {\begin{array}{l} U_{X1} =\frac{1}{2\alpha }e_2 \\ U_{X2} =\frac{1}{\alpha }\left( {\alpha x_1 x_3 +e_2 +e_4 } \right) \\ U_{X3} =\frac{1}{\alpha }\left( {-\alpha x_1 x_2 +e_3 } \right) \\ U_{X4} =\frac{1}{\alpha }\left( {-\frac{d}{2}e_2 +e_4 } \right) \\ \end{array}} \right. \end{aligned}$$

(9)

and

$$\begin{aligned} \left\{ {\begin{array}{l} U_{Y1} =-\frac{1}{2\beta }e_2 \\ U_{Y2} =\frac{1}{\beta }\left( {\beta y_1 y_3 } \right) \\ U_{Y3} =\frac{1}{\beta }\left[ {-\beta y_1 y_2 +c(\beta -\alpha )} \right] \\ U_{Y4} =\frac{1}{2\beta }\left( {de_2 } \right) \\ \end{array}} \right. \end{aligned}$$

(10)

such that

$$\begin{aligned} \dot{V}(e)=-e_1^2 -e_2^2 -e_3^2 -e_4^2 <0 \end{aligned}$$

(11)

So, Eq. (11) ensures the asymptotic global stability of synchronization.

Next, the simulation results, in this coupling scheme, for three different cases of system’s parameters ($\alpha$, $\beta$), are presented.

3.1.1 The symmetric case ($\alpha =\beta )$

Firstly, the parameters $\alpha$, $\beta$ are chosen to be equal ($\alpha$ $=$ $\beta$ $=$ 1). This is the most studied type of mutual coupling and also the most interesting due to its applications in a variety of scientific fields. Also, by choosing, in this case, the systems’ parameters as $c = 2.7$ and $d = 0.44$, each one of the coupled systems is in a hyperchaotic state. In this case of coupled identical systems with the proposed coupling scheme, only the complete synchronization is observed. This type of synchronization is confirmed by the $y_{1}$ versus $x_{1}$ plot of Fig. 21. Furthermore, the time-series of the variables $x_{2}$, $y_{2}$ as well as the errors $e_{i}$ (i $=$ 1, 2, 3, 4) show the exponential convergence to zero which confirms the expected system’s complete synchronization (Figs. 22 and 23).

Fig. 21

The phase portrait of $y_{1}$ versus $x_{1}$, for $\alpha = \beta = 1$, $c = 2.7$ and $d = 0.44$

Fig. 22

The time-series of $x_{2}, y_{2}$, for $\alpha = \beta = 1$, $c = 2.7$ and $d = 0.44$

Fig. 23

The plot of errors $e_{i} ({=}{\beta y_{i}} - \alpha x_{i})$, for $\alpha = \beta = 1$, $c = 2.7$ and $d = 0.44$

Fig. 24

The phase portraits of $x_{2}$ versus $x_{1}$ and $y_{2}$ versus $y_{1}$, for $\alpha = 2$, $\beta = 1$, $c = 2.7$ and $d = 0.44$

Fig. 25

The phase portrait of $y_{1}$ versus $x_{1}$, for $\alpha = 2$, $\beta = 1$, $c = 2.7$ and $d = 0.44$

3.1.2 The case $\alpha = 2$, $\beta = 1$

In this case, the parameters of the error functions are chosen to be $\alpha = 2$ and $\beta = 1$. By choosing again the systems’ parameters as $c = 2.7$, $d = 0.44$ and for $\alpha = 2$ the hyperchaotic attractor of the second system is enlarged by two times, as it is shown with red color in Fig. 24, as well as by the time-series of signals $y_{1}$ and $y_{2}$ in regard to the signals $x_{1}$ and $x_{2}$ respectively (Figs. 26 and 27). The $y_{1}$ versus $x_{1}$ plot in Fig. 25 confirms that the coupled system is in complete synchronization state independently of the values of the error’s parameters $\alpha$, $\beta$. The error plot $e_{i}=y_{i} - 2x_{i}$ (i $=$ 1, 2, 3, 4) in Fig. 28 shows the exponential convergence to zero that confirms the realization of system’s complete synchronization state.

Fig. 26

The time-series of $x_{1}, y_{1}$, for $\alpha = 2$, $\beta = 1$, $c = 2.7$ and $d = 0.44$

Fig. 27

The time-series of $x_{2}, y_{2}$, for $\alpha = 2$, $\beta = 1$, $c = 2.7$ and $d = 0.44$

Fig. 28

The plot of errors $e_{i} ({=}{\beta y_{i}}- \alpha x_{i})$, for $\alpha = 2$, $\beta = 1$, $c = 2.7$ and $d = 0.44$

Fig. 29

The phase portraits of $x_{2}$ versus $x_{1}$ and $y_{2}$ versus $y_{1}$, for $\alpha = -1$, $\beta = 2$, $c = 2.7$ and $d = 0.44$

Fig. 30

The phase portrait of $y_{1}$ versus $x_{1}$, for $\alpha = -1$, $\beta = 2$, $c = 2.7$ and $d = 0.44$

3.1.3 The Case $\alpha = -1$, $\beta = 2$

By choosing the parameters of the error functions as $\alpha = -1$ and $\beta = 2$, the attractor of the first coupled system has been enlarged by factor two, while the attractor of the second coupled system has been inverted in regard to the first one, as it is shown in Fig. 29. In this case the systems’ parameters are chosen again as $c = 2.7$ and $d = 0.44$ so as both of the coupled systems are in hyperchaotic state. This process is shown more clearly in the plots of the time-series of $x_{1}, y_{1}$ and $x_{2}, y_{2}$ (Figs. 31 and 32). The phase portrait of $y_{1}$ versus $x_{1}$ in Fig. 30 indicates that the coupled system is in anti-synchronization state, which is also confirmed by the error plot $e_{i} = 2y_{1}+x_{1}$ (i $=$ 1, 2, 3, 4) in Fig. 33.

Fig. 31

The time-series of $x_{1}, y_{1}$, for $\alpha = -1$, $\beta = 2$, $c = 2.7$ and $d = 0.44$

Fig. 32

The time-series of $x_{2}, y_{2}$, for $\alpha = -1$, $\beta = 2$, $c = 2.7$ and $d = 0.44$

Fig. 33

The plot of errors $e_{i} ({=}{\beta y_{i}} - \alpha x_{i})$, for $\alpha = -1$, $\beta = 2$, $c = 2.7$ and $d = 0.44$

3.2 Unidirectional Coupling

In this section, the unidirectional coupling scheme, $U_{X}$ = 0, for $\beta$ = 1, given by Eq. (1), is presented.

Master System:

$$\begin{aligned} \left\{ {\begin{array}{l} \dot{x}_1 =x_2 -x_1 \\ \dot{x}_2 =-x_1 x_3 +x_4 \\ \dot{x}_3 =x_1 x_2 -c \\ \dot{x}_4 =-dx_2 \\ \end{array}} \right. \end{aligned}$$

(12)

Slave System:

$$\begin{aligned} \left\{ {\begin{array}{l} \dot{y}_1 =y_2 -y_1 +U_{Y1} \\ \dot{y}_2 =-y_1 y_3 +y_4 +U_{Y2} \\ \dot{y}_3 =y_1 y_2 -c+U_{Y3} \\ \dot{y}_4 =-dy_2 +U_{Y4} \\ \end{array}} \right. \end{aligned}$$

(13)

where ${\varvec{U}_{Y}}= [U_{Y1}, U_{Y2}, U_{Y3}, U_{Y4}]^{\text {T}}$ are the Nonlinear Open Loop Controller (NOLC). The error function is defined by ${\varvec{e}} = \beta {\varvec{y}} - \alpha {\varvec{x}}$, with ${\varvec{e}} = [e_{1}, e_{2}, e_{3}, e_{4}]^{\text {T}}$, ${\varvec{x}} = [x_{1}, x_{2}, x_{3}, x_{4}]^{\text {T}}$ and ${\varvec{y}} = [y_{1}, y_{2}, y_{3}, y_{4}]^{\text {T}}$. So, the error dynamics, by taking the difference of Eqs. (12) and (13), are written as:

$$\begin{aligned} \left\{ {\begin{array}{l} \dot{e}_1 =e_2 -e_1 +\beta U_{Y1} \\ \dot{e}_2 =\alpha x_1 x_3 -\beta y_1 y_3 +e_4 +\beta U_{Y2} \\ \dot{e}_3 =-\alpha x_1 x_2 +\beta y_1 y_2 +c(\alpha -\beta )+\beta U_{Y3} \\ \dot{e}_4 =-de_2 +\beta U_{Y4} \\ \end{array}} \right. \end{aligned}$$

(14)

For stable synchronization $e \rightarrow 0$ with $t \rightarrow \infty$. By substituting the conditions in Eq. (14) and taking the time derivative of Lyapunov function

$$\begin{aligned} \dot{V}(e)&=e_1 \dot{e}_1 +e_2 \dot{e}_2 +e_3 \dot{e}_3 +e_4 \dot{e}_4 \nonumber \\&= e_1 \left( {e_2 -e_1 +\beta U_{Y1} } \right) +e_2 \left( {\alpha x_1 x_3 -\beta y_1 y_3 +e_4 +\beta U_{Y2} } \right) \nonumber \\&\quad +e_3 \left[ {-\alpha x_1 x_2 +\beta y_1 y_2 +c\left( {\alpha -\beta } \right) +\beta U_{Y3} } \right] +e_4 \left( {-de_2 +\beta U_{Y4} } \right) \nonumber \\ \end{aligned}$$

(15)

we consider the following NOLC controllers

$$\begin{aligned} \left\{ {\begin{array}{l} U_{Y1} =-\frac{1}{\beta }e_2 \\ U_{Y2} =-\frac{1}{\beta }\left( {e_2 +\alpha x_1 x_3 -\beta y_1 y_3 +e_4 } \right) \\ U_{Y3} =-\frac{1}{\beta }\left[ {e_3 -\alpha x_1 x_2 +\beta y_1 y_2 +c(\alpha -\beta )} \right] \\ U_{Y4} =-\frac{1}{\beta }\left( {e_4 -de_2 } \right) \\ \end{array}} \right. \end{aligned}$$

(16)

such that

$$\begin{aligned} \dot{V}(e)=-e_1^2 -e_2^2 -e_3^2 -e_4^2 <0 \end{aligned}$$

(17)

Equation (17) ensures the asymptotic global stability of synchronization.

3.2.1 The Case $\alpha =\beta = 1$

In this case, as it occurs in the mutual coupling, the phenomenon of complete synchronization is achieved for every value of $\alpha = \beta$. Especially, for $\alpha = \beta = 1$, the two coupled systems are in the same hyperchaotic state, due to the chosen values of system’s parameters ($c = 2.7$ and $d = 0.44$). The goal of complete synchronization is achieved as it is shown from the plots of $y_{1}$ versus $x_{1}$, the time-series of $x_{2}, y_{2}$ and the errors $e_{i}$ in Figs. 34, 35 and 36.

Fig. 34

The phase portrait of $y_{1 }$ versus $x_{1}$, for $\alpha = \beta = 1$, $c = 2.7$ and $d = 0.44$

Fig. 35

The time-series of $y_{2}, x_{2}$, for $\alpha = \beta = 1$, $c = 2.7$ and $d = 0.44$

Fig. 36

The plot of errors $e_{i} ({=}{\beta y_{i}}- \alpha x_{i})$, for $\alpha = \beta = 1$, $c = 2.7$ and $d = 0.44$

Fig. 37

The phase portrait of $y_{1 }$ versus $x_{1}$, for $\alpha = -\beta = -1$, $c = 2.7$ and $d = 0.44$

3.2.2 The Case for $\alpha = -\beta = -1$

By using opposing values for the parameters $\alpha = -\beta = -1$ the phenomenon of anti-synchronization is achieved, as it is shown in Fig. 37. Initially, the coupled systems are in different hyperchaotic states but the unidirectional coupling leads the slave system to an opposite hyperchaotic attractor in regard to the master system. This conclusion is derived from the phase portrait of $y_{1 }$ versus $x_{1}$ (Fig. 37), as well as from the time-series of $x_{2}$, $y_{2}$ (Fig. 38). Also, the plot of errors $e_{i}=y_{i}+x_{i}$ in Fig. 39 confirms the anti-synchronization of the coupled system.

Fig. 38

The time-series of $y_{2}, x_{2}$, for $\alpha = -\beta = -1$, $c = 2.7$ and $d = 0.44$

Fig. 39

The plot of errors $e_{i} ({=}{\beta y_{i}}$ – $\alpha x_{i})$, for $\alpha = -\beta = -1$, $c = 2.7$ and $d = 0.44$

Fig. 40

The phase portraits of $x_{2}$ versus $x_{1}$ and $y_{2}$ versus $y_{1}$, for $\alpha = 2$, $\beta = 1$, $c = 2.7$ and $d = 0.44$

Fig. 41

The phase portrait of $y_{1}$ versus $x_{1}$, for $\alpha = 2$, $\beta = 1$, $c = 2.7$ and $d = 0.44$

Fig. 42

The time-series of $x_{1}, y_{1}$, for $\alpha = 2$, $\beta = 1$, $c = 2.7$ and $d = 0.44$

3.2.3 The Case $\alpha = 2$, $\beta = 1$

In this case, the parameters of the error functions are chosen as $\alpha = 2$ and $\beta = 1$. By choosing the systems’ parameters as $c = 2.7$, $d = 0.44$ and for $\alpha = 2$ the chaotic attractor of the second system is enlarged by two times, as it is shown with red color in Fig. 40, as well as by the time-series of signals $y_{1}$ and $y_{2}$ in regard to the signals $x_{1}$ and $x_{2}$ respectively (Figs. 42 and 43). The $y_{1}$ versus $x_{1}$ plot in Fig. 41 confirms that the coupled system is in complete synchronization state independently of the values of the error’s parameters $\alpha$, $\beta$. The error plot $e_{i}=y_{1} - 2x_{1}$ (i $=$ 1, 2, 3, 4) in Fig. 44 shows the exponential convergence to zero that confirms the realization of system’s complete synchronization state.

Fig. 43

The time-series of $x_{2}$, $y_{2}$, for $\alpha$ = 2, $\beta = 1$, $c = 2.7$ and $d = 0.44$

Fig. 44

The plot of errors $e_{i} ({=}{\beta y_{i}}- \alpha x_{i})$, for $\alpha = 2$, $\beta = 1$, $c = 2.7$ and $d = 0.44$

Fig. 45

The phase portrait of $x_{2}$ versus $x_{1}$, for $\alpha = -2$, $\beta = 1$, $c = 2.7$ and $d = 0.44$

Fig. 46

The phase portrait of $y_{1}$ versus $x_{1}$, for $\alpha = -2$, $\beta = 1$, $c = 2.7$ and $d = 0.44$

Fig. 47

The time-series of $x_{1}, y_{1}$, for $\alpha = -2$, $\beta = 1$, $c = 2.7$ and $d = 0.44$

3.2.4 The Case $\alpha = -2$, $\beta = 1$

In the last case the parameters of the error function are chosen as $\alpha = -2$ and $\beta = 1$. So, the attractor of the first coupled system has been enlarged again by factor two, while the attractor of the second coupled system has been inverted in regard to the first one, as it is shown in Fig. 45. In this case the systems’ parameters are chosen as $c = 2.7$ and $d = 0.44$ so as both of the coupled systems are in hyperchaotic state. This process is shown more clearly in the plots of the time series of $x_{1}, y_{1}$ and $x_{2}, y_{2}$ of Figs. 47 and 48. The phase portrait of $y_{1}$ versus $x_{1}$ in Fig. 46 indicates that the coupled system is in anti-synchronization state, which is also confirmed by the error plot $e_{i} = 2y_{i}+x_{i}$ (i $=$ 1, 2, 3, 4) in Fig. 49.

4 Circuit’s Implementation of the Proposed Scheme

In this section the circuit implementation of the proposed scheme, with the electronic simulation package Cadence OrCAD, in the case of unidirectional coupling systems with $a = \beta$ is presented, in order to prove the feasibility of this method. The coupling system is realized by common electronic components. The system’s circuit consists of three sub-circuits, which are the master circuit, the slave circuit and the coupling circuit.

Fig. 48

The time-series of $x_{2}, y_{2}$, for $\alpha = -2$, $\beta = 1$, $c = 2.7$ and $d = 0.44$

Fig. 49

The plot of errors $e_{i} ({=}{\beta y_{i}} - \alpha x_{i})$, for $\alpha = -2$, $\beta = 1$, $c = 2.7$ and $d = 0.44$

Figure 50 depicts the schematic of the master circuit. It has four integrators (U$_{1}$, U$_{2}$, U$_{3}$ and U$_{4})$ and two differential amplifiers (U$_{7}$, U$_{8})$, which are implemented with the TL084, as well as two signals multipliers (U$_{5}$, U$_{6})$ by using the AD633. By applying Kirchhoff’s circuit laws, the corresponding circuital equations of designed master circuit can be written as:

$$\begin{aligned} \left\{ {\begin{array}{l} \dot{x}_1 =\frac{1}{RC}\left( {x_2 -x_1 } \right) \\ \dot{x}_2 =\frac{1}{RC}\left( {-\frac{R}{R_1 10V}x_1 x_3 +x_4 } \right) \\ \dot{x}_3 =\frac{1}{RC}\left( {\frac{R}{R_1 10V}x_1 x_2 -c} \right) \\ \dot{x}_4 =\frac{1}{RC}\left( {-\frac{R}{R_d }x_2 } \right) \\ \end{array}} \right. \end{aligned}$$

(18)

where $x_{i} (i = 1, \ldots , 4)$ are the voltages in the outputs of the operational amplifiers U$_{1}$, U$_{2}$, U$_{3}$ and U$_{4}$. Normalizing the differential equations of system (18) by using $\tau =t/{RC}$ we could see that this system is equivalent to the system (12). The circuit components have been selected as: R $=$ 10 k$\Omega$, R$_{1} = 1$ k$\Omega$, R$_{\text {d}} = 22.727$ k$\Omega$, C $=$ 10 nF, $\text {V}_{\text {C}} = 2.7$ V, while the power supplies of all active devices are $\pm$15 ${\text {V}}_{\text {DC}}$. For the chosen set of components the master system’s parameters are: c $=$ 2.7 and d $=$ 0.44. In Figs. 51, 52, 53, 54 and 55 the hyperchaotic attractors, which are obtained from Cadence OrCAD in various phase planes, are proved to be in a very good agreement with the respective phase portraits from system’s simulation process (Figs. 15, 16, 17, 18 and 19). So, the proposed circuit emulates well the master system.

Fig. 50

The schematic of the master circuit

Fig. 51

Hyperchaotic attractor of the designed master circuit obtained from Cadence OrCAD in the $(x_{1}, x_{2})$ phase plane

Fig. 52

Hyperchaotic attractor of the designed master circuit obtained from Cadence OrCAD in the $(x_{1}, x_{3})$ phase plane

Fig. 53

Hyperchaotic attractor of the designed master circuit obtained from Cadence OrCAD in the $(x_{1}, x_{4})$ phase plane

Fig. 54

Hyperchaotic attractor of the designed master circuit obtained from Cadence OrCAD in the ($x_{2}, x_{3}$) phase plane

Fig. 55

Hyperchaotic attractor of the designed master circuit obtained from Cadence OrCAD in the ($x_{2}, x_{4}$) phase plane

Fig. 56

The schematic of the slave circuit

Fig. 57

The schematic of the coupling circuit

Fig. 58

The phase portrait in the ($x_{1}, y_{1})$ phase plane, for $\alpha = \beta = 1$, $c = 2.7$ and $d = 0.44$, obtained from Cadence OrCAD

Fig. 59

The phase portrait in the ($x_{2}, y_{2})$ phase plane, for $\alpha = \beta = 1$, $c = 2.7$ and $d = 0.44$, obtained from Cadence OrCAD

Fig. 60

The phase portrait in the ($x_{3}$, $y_{3})$ phase plane, for $\alpha =\beta = 1$, $c = 2.7$ and $d = 0.44$, obtained from Cadence OrCAD

Fig. 61

The phase portrait in the ($x_{4}$, $y_{4})$ phase plane, for $\alpha = \beta = 1, c = 2.7$ and $d = 0.44,$ obtained from Cadence OrCAD

In Fig. 56 the schematic of the slave circuit, which is similar to the master circuit, is shown. The difference of this circuit in comparison to the previous one are the signals mu $_{2}$, mu $_{3}$ and mu $_{4}$, which are the opposites of the signals $U_{Y2}$, $U_{Y3}$ and $U_{Y4}$, produced by the controllers of Eq. (16). Also, $e_{2}$ is the difference signal ($\beta y_{2} - \alpha x_{2})$. Next, the design of the coupling circuit as well as the simulation results obtained from SPICE in the case of $\alpha = \beta$ is discussed in details.

In the case of $\alpha = \beta = 1$ and by considering the achievement of synchronization between the coupled systems (12) and (13), the NOLCs take the following forms.

$$\begin{aligned} \left\{ {\begin{array}{l} U_{Y1} =-e_2 \\ U_{Y2} =-\left( {e_2 +e_4 } \right) \\ U_{Y3} =-e_3 \\ U_{Y4} =-\left( {e_4 -de_2 } \right) \\ \end{array}} \right. \end{aligned}$$

(19)

The units from which the coupling circuit is consisted, are shown in the schematic of Fig. 57. In this schematic $u_{2}$ and $u_{4}$ are the control signals $U_{Y2}$ and $U_{Y4}$ of Eq. (19) respectively, while $mu_{2}$ and mu $_{4}$ are the opposite of these signals. Also, $e_{i}$, (i $=$ 2, 3, 4) are the difference signals ($\beta y_{i}- \alpha x_{i}$, i $=$ 2, 3, 4) and $me_{2}$ is the opposite of $e_{2}$.

Figures 58, 59, 60 and 61 depict the phase portraits in ($x_{i}, y_{i})$ phase plane, with $i = 1, \ldots , 4,$ for $\alpha = \beta$ = 1, $c = 2.7$ and $d = 0.44$, obtained from Cadence OrCAD. These figures confirm the achievement of complete synchronization in the case of unidirectionally coupled circuits with the proposed method.

5 Conclusion

In this chapter, the case of bidirectional and unidirectional coupling scheme of hyperchaotic dynamical systems with hidden attractors was studied. The proposed system is a four-dimensional modified Lorenz system, which is the simplest hyperchaotic system of this family. Furthermore, the coupling method was based on a recently new proposed scheme based on the nonlinear open loop controller.

According to the simulation results from system’s numerical integration as well as the circuital implementation of the proposed system in SPICE, in the case of unidirectional coupling, the appearance of complete synchronization and anti-synchronization, depending on the signs of the parameters of the error functions, was investigated in various cases. So, by choosing an appropriate sign for the error functions one could drive the coupling system either in complete synchronization or anti-synchronization behavior.

As it is known, the complex behavior of hyperchaotic systems, like the aforementioned, makes the control difficult in practical applications where a particular dynamic is desired. So, this chapter presents an interesting research result for the family of hyperchaotic systems with hidden attractors, because this method could be very useful in many potential applications of these systems. As a next step in this direction is the application of the proposed method in non-identical coupling systems in order to satisfy the goal of control of systems, which are in totally different dynamical behaviors.