Keywords

1 Introduction

Rolling element bearing is one of the essential components in rotating equipments. In present scenario, bearing applications require understanding of nonlinear dynamic effects. Nonlinearity often arises due to Hertzian force deformation relationship, the varying compliance nature, the internal clearance, varying number of balls etc. The behavior of nonlinear system often demonstrates unexpected behavior patterns that are extremely sensitive to initial conditions. The excitation due to unbalance force in rotor bearing system cannot be eliminated completely [1, 2] even with good balancing of rotor and therefore it is the major parameter to consider for study. Due to consideration of unbalance force the system is bi-periodically excited. The amount of unbalanced force changes continuously with operating parameters and thus changing the dynamic behavior of the whole system. Moreover, when ball bearing operates at high speed the effect of centrifugal force cannot be neglected. Jang and Jeong [3] considered the effect of centrifugal force of ball with waviness on races for balanced rotor. However effect of centrifugal force of ball along with unbalanced rotor and nonlinear spring-damping model has not been considered so far.

The aim of present study is to analyze the nonlinear dynamic response of unbalanced rotor supported on ball bearings considering centrifugal force. Sources of nonlinearity such as Hertzian contact force, varying compliance frequency are considered and formulate in Mathematical model. Effect of number of balls is considered at constant rotational speed.

2 Mathematical Modeling

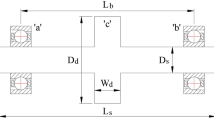
A rolling element bearing supporting horizontal rotor considering unbalance force is shown in Fig. 1. To analyze nonlinear dynamic response of ball bearing the bearing components are modeled as non-linear spring-mass-damper system, in which the outer race of the bearing is fixed rigidly and inner race, is fixed rigidly with shaft. A constant radial force is assumed to be acting on the system.

Fig. 1
figure 1

Rolling element bearing model considering Mass-spring-damper

Full size image

It is assumed that the spring damper model considered here has equally spaced balls rotating on the surfaces of the inner and outer races. When the rolling element set and the cage rotates with a constant angular velocity (ωcage), a parametrically excited vibration is generated and transmitted through the outer race.

$$ \omega_{cage} = \omega_{rotor} \frac{r}{(R + r)} $$
(1)

The characteristic frequency of this vibration is called the varying compliance frequency (VC) and is given by

$$ \omega_{VC} = \omega_{cage} \times N_{b} $$
(2)

where N b is number of balls.

For the ith ball, local Hertzian contact force and deflection are related as [4]

$$ P_{{\theta_{i} }} = k\left( {r_{{\theta_{i} }} } \right)^{{{\raise0.7ex\hbox{$3$} \!\mathord{\left/ {\vphantom {3 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}}} $$
(3)

where, k is the nonlinear stiffness due to point contact, \( \theta_{i} \) is the angular position ith ball and \( r_{{\theta_{i} }} \) is radial displacement of the ith ball which is given by [5]

$$ r_{{\theta_{i} }} = x\cos \theta_{i} + y\sin \theta_{i} $$
(4)

Considering the internal radial clearance, \( \gamma_{0} \), the contact deformation becomes,

$$ r_{{\theta_{i} }} = x\cos \theta_{i} + y\sin \theta_{i} - \left( {\gamma_{0} } \right) $$
(5)

Substituting \( r_{{\theta_{i} }} \) in the Eq. (3), we get

$$ P_{{\theta_{i} }} = k.H\left\{ {\left( {x\cos \theta_{i} + y\sin \theta_{i} } \right) - \left( {\gamma_{0} } \right)} \right\}^{{{\raise0.7ex\hbox{$3$} \!\mathord{\left/ {\vphantom {3 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}}} $$
(6)

where, \( H\left\{ {\left( {x\cos \theta_{i} + y\sin \theta_{i} } \right) - \left( {\gamma_{0} } \right)} \right\}^{{{\raise0.7ex\hbox{$3$} \!\mathord{\left/ {\vphantom {3 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}}} \) is a Heaviside step function.

Total restoring force in x and y direction considering Hetzian contact force \( \left( {P_{{\theta_{i} }} } \right) \) and centrifugal force \( \left( {P_{c} } \right) \) is written as

$$ P_{x} = \sum\limits_{i = 1}^{{N_{b} }} {\left[ {k.H\left\{ {\left( {x\cos \theta_{i} + y\sin \theta_{i} } \right) - \left( {\gamma_{0} } \right)} \right\}^{{{\raise0.7ex\hbox{$3$} \!\mathord{\left/ {\vphantom {3 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}}} -\,P_{c} } \right]\cos \theta_{i} } $$
(7)
$$ P_{y} = \sum\limits_{i = 1}^{{N_{b} }} k \left[ {k.H\left\{ {\left( {x\cos \theta_{i} + y\sin \theta_{i} } \right) - \left( {\gamma_{0} } \right)} \right\}^{{{\raise0.7ex\hbox{$3$} \!\mathord{\left/ {\vphantom {3 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}}} -\,P_{c} } \right]\sin \theta_{i} $$
(8)

The centrifugal force is given by [6]

$$ P_{c} = 2.26 \times 10^{ - 11} \times D_{b}^{2} \times \omega_{cage} \times D_{m} $$
(9)

where, \( D_{b} \) is ball diameter, \( \omega_{cage} \) is angular velocity of the cage and \( D_{m} \) is pitch circle diameter of the bearing.

2.1 Nonlinear Damping Force

A viscous damping model is considered in present formulation in which the dissipative forces are proportional to the time derivate. The resulting equation [7] is given by

$$ P_{{d_{i} }} = c\left( {r_{{\theta_{i} }} } \right)\dot{r}_{{\theta_{i} }}^q $$
(10)

where, \( c(r_{{\theta_{i} }} ) \) depends on contact geometry, material properties of elastic bodies, lubricant property and contact surface velocities.

2.2 Equation of Motion

The equation considering effect of inertia, restoring and damping force and constant vertical force acting on the inner race are represented as [5]

$$ \begin{aligned} & M_{R} \textit{\"{x}} + \sum\limits_{i = 1}^{{N_{b} }} {P_{{d_{i} }} } \cos \theta_{i}\,+ \\ & \sum\limits_{i = 1}^{{N_{b} }} {\left[ {k.H\left\{ {\left( {x\cos \theta_{i} + y\sin \theta_{i} } \right) - \left( {\gamma_{0} } \right)} \right\}^{{{\raise0.7ex\hbox{$3$} \!\mathord{\left/ {\vphantom {3 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}}} -\,P_{c} } \right]\cos \theta_{i} } = P_{r} + P_{u} \cos \left( {\omega t} \right) \\ \end{aligned} $$
(11)
$$ \begin{aligned} & M_{R}\, \textit{\"{y}} + \sum\limits_{i = 1}^{{N_{b} }} {P_{{d_{i} }} } \sin \theta_{i}\,+ \\ & \sum\limits_{i = 1}^{{N_{b} }} k \left[ {k.H\left\{ {\left( {x\cos \theta_{i} + y\sin \theta_{i} } \right) - \left( {\gamma_{0} } \right)} \right\}^{{{\raise0.7ex\hbox{$3$} \!\mathord{\left/ {\vphantom {3 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}}} -\,P_{c} } \right]\sin \theta_{i} = P_{u} \sin \left( {\omega t} \right) \\ \end{aligned} $$
(12)

where, \( M_{R} \) is the rotor mass supported on bearings, \( P_{r} \) is the radial load on bearing and \( P_{u} \) is force due to unbalance.

The system is defined by Eqs. (11) and (12) which are two coupled non-linear second order differential equations.

3 Results and Discussion

In present study, ball bearing 6205 is considered whose parameters are shown in Table 1. Shaft is assumed to be running at 5000 rpm and an unbalance force of 10 % of radial load is considered in the current study. The Runge-Kutta methods of fourth order are used to solve coupled equations. The time step for the investigation is taken as ∆t = 10−5 s with the initial displacements as x 0 = 10−6 m, y 0 = 10−6 m and initial velocities are assumed to be zero i.e. \( \dot{x}_{0} =0\,{\text{m/s}},\dot{y}_{0} = 0\;{\text{m/s}} \). Poincaré maps and frequency responses are used to illustrate and study the effect of system diversity.

Table 1 Parameters of bearing 6205 (SKF)
Full size table

For number of balls N b = 6, peak amplitude of vibration for X direction response appears at rotational speed (X = 83 Hz) as shown in Fig. 2a. Other major peaks appears at the interaction of varying compliance frequency and rotational speed (X = 83.33 Hz) and their harmonics at VC − X(=116 Hz), 2X, VC + X(= 282 Hz).Fractal structure is observed through Poincaré map which reveals chaotic nature. For Y Direction response, peak amplitude appears at rotational speed (X = 83.33 Hz) and other peak appears at VC − X(=116 Hz), VC(= 199 Hz) shown in Fig. 2b. The Poincaré map shows fractal structure for N b = 6 as shown Fig. 2b.

Fig. 2
figure 2

FFT and poincare map at N b = 6 a X direction b Y direction

Full size image

Figures 3, 4 and 5 shows quasi periodic response from Poincaré map for N b = 7, 8 and 9 respectively. Major peak is obtained corresponding to shaft rotational frequency (X = 83 Hz) and amplitude decreases as number of balls increases. This is due to increase in stiffness of the system since number of balls coming in load zone increases. System further stabilizes when number of balls is changed to 10, which is shown in Fig. 6. At this moment, systems exhibits multi orbit periodic response which is clearly indicated in Poincaré map. The results are summarized in Table 2.

Fig. 3
figure 3

FFT and poincare map at Nb = 7 a X direction b Y direction

Full size image
Fig. 4
figure 4

FFT and poincare map at N b = 8 a X direction b Y direction

Full size image
Fig. 5
figure 5

FFT and poincare map at N b = 9 a X direction b Y direction

Full size image
Fig. 6
figure 6

FFT and poincare map at N b = 10 a X direction b Y direction

Full size image
Table 2 Influence of number of balls on stability
Full size table

4 Concluding Remarks

This study presents an analytical model to investigate the effects of unbalance and centrifugal force acting on ball bearing model. Unbalance force corresponding to 10 % magnitude of radial load \( \left( {P_{r} } \right) \) with internal clearance of 10 µm are considered in the present study. Number of balls are varied and effect of variation has been analyzed at constant rotational speed of shaft. Following conclusions can be drawn from present study.

  • Nonlinear dynamic responses are found to be associated with rotational frequency (X) and its super harmonics due to unbalance force.

  • Results show interaction of rotational frequency (X) due to unbalance force and varying compliance frequency (VC) due to parametric excitation.

  • When number of balls are increased peak amplitude decreases implying a stiffer system and same was reported by Harsha et al. [4] and Kankar et al. [8]. It can be predicted from the results that increasing number of balls reduces the effect of frequency modulation.

  • For N b = 5–6, chaotic nature is observed from poincare map. When number of balls increases to N b = 7–9, quasi-periodic behavior is observed and further increase in the number of balls results in entering of system in stable zone. This can be attributed to increase in stiffness due to increase in number of balls.

  • From this analysis an insight is obtained to choose appropriate number of balls so that system can be operated in stable zone and severe amplitude of vibration (chaotic) can be avoided.