Skip to main content
SpringerLink
Log in

Representation Theory in Clifford Analysis

  • Living reference work entry
  • First Online:
Operator Theory

Abstract

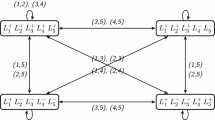
This chapter introduces contemporary Clifford analysis as a local function theory of first-order systems of PDEs invariant under various Lie groups. A concept of a symmetry of a system of partial differential equations is the key point of view, it makes it possible to use many efficient tools from the theory of representations of simple Lie groups. A systematic approach is based on a choice of a Klein geometry (a homogeneous space \(M \simeq G/P\) with \(P\) being a Lie subgroup of a Lie group G ) and on a notion of a homogeneous (invariant) differential operator acting among sections of associated homogeneous vector bundles. The main example is the conformal group G = Spi n ( m + 1 , 1) acting on the sphere S m and the Dirac operator.The chapter contains a description of basic properties of solutions of such systems and lists many various examples of the aforementioned scheme. The introductory sections describe the Clifford algebra, its spinor representations, the conformal group of the Euclidean space, the Fegan classification of the conformally invariant first order differential operators, and a series of examples of such operators appearing in the Clifford analysis. They include the Dirac equation for spinor-valued functions, the Hodge and Moisil–Théodoresco systems for differential forms, the Hermitian Clifford analysis, the quaternionic Clifford analysis, the (generalized) Rarita–Schwinger equations, and the massless fields of higher spin.A different point of view to these first-order systems presents them as special solutions of the (twisted) Dirac equation. The second part of this chapter contains a description of basic properties of solutions of the Dirac equation, including the Fischer decomposition of spinor-valued polynomials, the Howe duality, the Taylor and the Laurent series for monogenic functions. The last two sections contains a description of the Gelfand–Tsetlin bases for the spaces of (solid) spherical monogenics and a discussion of possible future direction of research in Clifford analysis.

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution

Institutional subscriptions

Similar content being viewed by others

References

  1. Abreu Blaya, R., Bory Reyes, J., Delanghe, R., Sommen, F.: Generalized Moisil– Théodoresco systems and Cauchy integral decompositions. Int. J. Math. Math. Sci.2008, 19 (2008). Article ID746946

    Google Scholar 

  2. Abreu Blaya, R., Bory Reyes, J., De Schepper, H., Sommen, F.: Cauchy integral formulae in Hermitian quaternionic Clifford analysis. Complex Anal. Oper. Theory6(5), 971–985 (2012)

    Article  MATH  MathSciNet  Google Scholar 

  3. Abreu Blaya, R., Bory Reyes, J., De Schepper, H., Sommen, F.: Matrix Cauchy and Hilbert transforms in Hermitean quaternionic Clifford analysis. Comp. Var. Elliptic Equ.58(8), 1057–1069 (2013)

    Article  MATH  Google Scholar 

  4. Adams, W.W., Loustaunau, P., Palamodov, V.P., Struppa, D.C.: Hartog’s phenomenon for polyregular functions and projective dimension of related modules over a polynomial ring. Ann. Inst. Fourier47, 623–640 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  5. Adams, W.W., Berenstein, C.A., Loustaunau, P., Sabadini, I., Struppa, D.C.: Regular functions of several quaternionic variables. J Geom. Anal.9(1), 1–15 (1999)

    Article  MATH  MathSciNet  Google Scholar 

  6. Ahlfors, L.: Möbius ransformations in \(\mathbb{R}^{n}\) expressed through 2 × 2 matrices of Clifford numbers. Complex Var. Theory Appl.5, 215–224 (1986)

    Article  MATH  MathSciNet  Google Scholar 

  7. Andrews, G.E., Askey, R., Roy, R.: Special Functions. Encyclopedia of Mathematics and Its Applications, vol. 71. Cambridge University Press, Cambridge (1999)

    Google Scholar 

  8. Bock, S., Gürlebeck, K.: On generalized Appell systems and monogenic power series. Math. Methods Appl. Sci.33(4), 394–411 (2009)

    Google Scholar 

  9. Brackx, F., Delanghe, R., Sommen, F.: Clifford Analysis. Pitman, London (1982)

    MATH  Google Scholar 

  10. Brackx, F., Delanghe, R., Sommen, F.: Differential forms and/or multivector functions. Cubo7(2), 139–169 (2005)

    MATH  MathSciNet  Google Scholar 

  11. Brackx, F., Bureš, J., De Schepper, H., Eelbode, D., Sommen, F., Souček, V.: Fundaments of Hermitean Clifford analysis, part I: complex structure. Complex Anal. Oper. Theory1(3), 341–365 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  12. Brackx, F., De Schepper, H., Eelbode, D., Souček, V.: The Howe dual pair in Hermitean Clifford analysis. Rev. Mat. Iberoamericana26(2), 449–479 (2010)

    Article  MATH  Google Scholar 

  13. Brackx, F., De Schepper, H., Souček, V.: Fischer decompositions in Euclidean and Hermitean Clifford analysis. Arch. Math.46(5), 301–321 (2010)

    MATH  MathSciNet  Google Scholar 

  14. Brackx, F., De Schepper, H., Lávička, R., Souček, V.: Fischer decompositions of kernels of Hermitean Dirac operators. In: Simos, T.E., Psihoyios, G., Tsitouras, Ch. (eds.) ICNAAM 2010, Rhodes, Greece, 2010. AIP Conf. Proc., vol. 1281, pp. 1484–1487 (2010)

    Google Scholar 

  15. Brackx, F., De Schepper, H., Lávička, R., Souček, V.: Gel’fand–Tsetlin procedure for the construction of orthogonal bases in Hermitean Clifford analysis. In: Simos, T.E., Psihoyios, G., Tsitouras, Ch. (eds.) ICNAAM 2010, Rhodes, Greece, 2010. AIP Conf. Proc., vol. 1281, pp. 1508–1511 (2010)

    Google Scholar 

  16. Brackx, F., De Schepper, H., Lávička, R., Souček, V.: Orthogonal basis of Hermitean monogenic polynomials: an explicit construction in complex dimension 2. In: Simos, T.E., Psihoyios, G., Tsitouras, Ch. (eds.) ICNAAM 2010, Rhodes, Greece, 2010. AIP Conf. Proc., vol. 1281, pp. 1451–1454 (2010)

    Google Scholar 

  17. Brackx, F., De Schepper, H., Lávička, R., Souček, V.: The Cauchy–Kovalevskaya Extension Theorem in Hermitean Clifford analysis. J. Math. Anal. Appl.381, 649–660 (2011)

    Article  MATH  MathSciNet  Google Scholar 

  18. Brackx, F., De Schepper, H., Lávička, R., Souček, V.: Gelfand–Tsetlin bases of orthogonal polynomials in Hermitean Clifford analysis. Math. Methods Appl. Sci.34, 2167–2180 (2011)

    Article  MATH  MathSciNet  Google Scholar 

  19. Brackx, F., De Schepper, H., Lávička, R., Souček, V.: Branching of monogenic polynomials. In: Simos, T.E., Psihoyios, G., Tsitouras, Ch. (eds.) ICNAAM 2012, Kos, Greece, 2012. AIP Conf. Proc., vol. 1479, pp.  304–307 (2012)

    Google Scholar 

  20. Brackx, F., De Schepper, H., Lávička, R., Souček, V.: Embedding Factors for Branching in Hermitian Clifford Analysis, Complex Anal. Oper. Theory, 2014, doi:10.1007/s11785-014-0365-3

    Google Scholar 

  21. Brackx, F., De Schepper, H., Eelbode, D., Lávička, R., Souček, V.: Fundaments of quaternionic Clifford analysis I: quaternionic structure, preprint arXiv:1403.2922

    Google Scholar 

  22. Branson, T.: Stein–Weiss operators and ellipticity. J. Funct. Anal.151(2), 334–383 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  23. Budinich, P., Trautman, A.: The Spinorial Chessboard. Springer, Berlin (1988)

    Book  Google Scholar 

  24. Bump, D.: Authomorphic Forms and Representations. Cambridge Studies in Adv. Mathematics, vol. 55. CUP, Cambridge (1996)

    Google Scholar 

  25. Bureš, J., Souček, V.: Regular spinor valued mappings, Seminarii di Geometria, Bologna 1984. In: Coen, S. (ed.) pp. 7–22. Bologna (1986)

    Google Scholar 

  26. Bureš, J., Souček, V.: Complexes of invariant differential operators in several quaternionic variables. Complex Var. Elliptic Equ.51(5–6), 463–487 (2006)

    MATH  MathSciNet  Google Scholar 

  27. Bureš, J., Damiano, A., Sabadini, I.: Explicit invariant resolutions for several Fueter operators. J. Geom. Phys.57, 765–775 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  28. Bureš, J., Van Lancker, P., Sommen, F., Souček, V.: Symmetric analogues of Rarita–Schwinger equations. Ann. Global Anal. Geom.21, 215–240 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  29. Bureš, J., Lávička, R., Souček, V.: Elements of Quaternionic Analysis and Radon Transform. Textos de Matematica, vol. 42. Universidade de Coimbra, Coimbra (2009)

    Google Scholar 

  30. Cacao, I.: Constructive Approximation by Monogenic Polynomials. PhD thesis, Univ. Aveiro (2004)

    Google Scholar 

  31. David, M.J.: Calderbank: Geometrical Aspects of Spinor and Twistor Analysis. PhD Thesis, Warwick (1995). Available at web page of the author

    Google Scholar 

  32. Čap, A., Slovák, J., Souček, V.: The BGG sequences. Ann. Math.154(1), 97–113 (2001)

    Article  MATH  Google Scholar 

  33. Čap, A., Slovák, J.: Parabolic Geometries I: Background and General Theory. Mathematical Surveys and Monographs, vol. 154. Amer. Math. Soc., Providence (2009)

    Google Scholar 

  34. Cerejeiras, P., Khler, U., Ren, G.: Clifford analysis for finite reflection groups. Complex Var. Elliptic Equ.51, 487–495 (2006)

    Article  MATH  MathSciNet  Google Scholar 

  35. Cnops, J.: An Introduction to Dirac Operators on Manifolds. Prog. Math. Phys., vol. 24. Birkhäuser, Boston (2002)

    Google Scholar 

  36. Colombo, F., Sabadini, I., Sommen, F., Struppa, D.C.: Analysis of Dirac Systems and Computational Algebra. Progress in Math. Physics, vol. 39. Birkhauser, Boston (2004)

    Google Scholar 

  37. Colombo, F., Souček, V., Struppa, D.: Invariant resolutions for several Fueter operators. J. Geom. Phys.56(7), 1538–1543 (2006)

    Article  Google Scholar 

  38. Coulembier, K.: The orthosymplectic superalgebra in harmonic analysis. J. Lie Theory23, 55–83 (2013)

    MATH  MathSciNet  Google Scholar 

  39. Coulembier, K.: Bernstein–Gelfand–Gelfand resolutions for basic classical Lie superalgebras. J. Algebra399, 131–169 (2014)

    Article  MATH  MathSciNet  Google Scholar 

  40. Coulembier, K., Somberg, P., Souček, V.: Joseph ideals and harmonic analysis for osp(m—2n). Int. Math. Res. Not. (2013). Doi:10.1093/imrn/rnt074

    Google Scholar 

  41. Crumeyrolle, A.: Orthogonal and Symplectic Clifford Algebras. Kluwer Academic, Dordrecht (1989)

    Google Scholar 

  42. Damiano, A., Eelbode, D., Sabadini, I.: Quaternionic Hermitian spinor systems and compatibility conditions. Adv. Geom.11, 169–189 (2011)

    Article  MATH  MathSciNet  Google Scholar 

  43. De Bie, H., Sommen, F.: Spherical harmonics and integration in superspace. J. Phys. A Math. Theory40, 7193–7212 (2007)

    Article  MATH  Google Scholar 

  44. De Bie, H., Eelbode, D., Sommen, F.: Spherical harmonics and integration in superspace II. J. Phys. A: Math. Theory42, 245204 (2009)

    Article  Google Scholar 

  45. Delanghe, R.: Clifford analysis: History and perspective. Complex Methods Funct. Theory1(1), 107–153 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  46. Delanghe, R.: On homogeneous polynomial solutions of the Riesz system and their harmonic potentials. Complex Var. Elliptic Equ.52, 1047–1061 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  47. Delanghe, R., Sommen, F., Souček, V.: Clifford Analysis and Spinor Valued Functions. Kluwer Academic, Dordrecht (1992)

    Book  Google Scholar 

  48. Delanghe, R., Lávička, R., Souček, V.: The Howe duality for Hodge systems. In: Grlebeck, K., Könke, C. (eds.) Proceedings of 18th International Conference on the Application of Computer Science and Mathematics in Architecture and Civil Engineering, Bauhaus-Universität Weimar, Weimar (2009)

    Google Scholar 

  49. Delanghe, R., Lávička, R., Souček, V.: The Fischer decomposition for Hodge–de Rham systems in Euclidean spaces. Math. Methods Appl. Sci.35, 10–16 (2012)

    Article  MATH  MathSciNet  Google Scholar 

  50. Delanghe, R., Lávička, R., Souček, V.: The Gelfand–Tsetlin bases for Hodge–de Rham systems in Euclidean spaces. Math. Methods Appl. Sci.35(7), 745–757 (2012)

    Article  MATH  MathSciNet  Google Scholar 

  51. Delanghe, R., Lávička, R., Souček, V.: On polynomial solutions of generalized Moisil–Théodoresco systems and Hodge systems. Adv. Appl. Clifford Alg.21(3), 521–530 (2011)

    Article  MATH  Google Scholar 

  52. Dostalová, M., Somberg, P.: Symplectic twistor operator and its solution space on \(\mathbb{R}^{2n}\). Complex Anal. Oper. Theory4 (2013). Doi:10.1007/s11785-013-0300-z

    Google Scholar 

  53. Dunkl, C.F.: Differential-difference operators asociated to reflection groups. Trans. MAS311, 167–183 (1989)

    MATH  MathSciNet  Google Scholar 

  54. Eastwood, M., Ryan, J.: Monogenic functions in Conformal geometry. SIGMA3, 084, 14 pp. (2007)

    Google Scholar 

  55. Eelbode, D.: A Clifford algebraic framework forsp( m )-invariant differential operators. Adv. App. Clifford Alg.17, 635–649 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  56. Eelbode, D.: Clifford analysis for higher spin operators. In Handbook of Operator Theory, Springer, (2014)

    Google Scholar 

  57. Eelbode, D., Souček, V.: Conformally invariant powers of the Dirac operator in Clifford analysis. Math. Method Appl. Sci.33(13), 1011–1023 (2010)

    Google Scholar 

  58. Fegan, H.D.: Conformally invariant first order differential operators. Quart. J. Math.27, 513–538 (1976)

    Article  MathSciNet  Google Scholar 

  59. Fei, M., Cerejeiras, P., Kähler, U.: Fueter’s theorem and its generalizations in Dunkl–Clifford analysis. J. Phys. A42(39), 395209, 15 pp. (2009)

    Google Scholar 

  60. Franek, P.: Generalized Dolbeault sequences in parabolic geometry. J. Lie Theory18(4), 757–774 (2008)

    MATH  MathSciNet  Google Scholar 

  61. Fulton, W., Harris, J.: Representation Theory. Springer, New York (1991)

    MATH  Google Scholar 

  62. Gilbert, J., Murray, M.: Clifford Algebras and Dirac Operators in Harmonic Analysis. Cambridge University Press, Cambridge (1991)

    Book  MATH  Google Scholar 

  63. Goodman, R., Wallach, N.R.: Representations and Invariants of the Classical Groups. Cambridge University Press, Cambridge (1998)

    MATH  Google Scholar 

  64. Graham, C.R., Jenne, R., Mason, L.J., Sparling, G.A.J.: Conformally invariant powers of the Laplacian. I. Existence. J. Lond. Math. Soc.46(2), 557–565 (1992)

    Article  MATH  MathSciNet  Google Scholar 

  65. Gürlebeck, K., Sprössig, W.: Quaternionic and Clifford Calculus for Physicists and Engineers. Wiley, Chichester (1997)

    MATH  Google Scholar 

  66. Habermann, K., Habermann, L.: Introduction to Symplectic Dirac Operators. Springer, Heidelberg (2006)

    Book  MATH  Google Scholar 

  67. Humphreys, J.: Introduction to Lie Algebras and Representation Theory. GTM, vol. 9. Springer, New York (1980)

    Google Scholar 

  68. Kac, V.G.: Lie superalgebras. Adv. Math.26, 8–96 (1977)

    Article  MATH  Google Scholar 

  69. Knapp, A.: Representation Theory of Semisimple Groups. An Overview Based on Examples. Princeton University Press, Princeton (1986)

    MATH  Google Scholar 

  70. Kostant, B.: Symplectic Spinors, Symposia Mathematica, vol. XIV, pp. 139–152. Cambridge University Press, Cambridge (1974)

    Google Scholar 

  71. Krump, L.: A resolution for the Dirac operator in four variables in dimension 6. Adv. Appl. Clifford Alg.19, 365–374 (2009)

    Article  MATH  MathSciNet  Google Scholar 

  72. Krump, L., Salač, T.: Exactness of the generalized Dolbeault complex for k-Dirac operators in the stable rank. In: AIP Conf. Proc., vol. 1479, p. 300 (2012)

    Google Scholar 

  73. Krýsl, S.: Symplectic spinor valued forms and operators acting between them. Arch. Math. Brno42, 279–290 (2006)

    MATH  MathSciNet  Google Scholar 

  74. Krýsl, S.: Classification of 1st order symplectic spinor operators in contact projective geometries. Differ. Geom. Appl.26(3), 553–565 (2008)

    Article  MATH  Google Scholar 

  75. Krýsl, S.: Complex of twistor operators in spin symplectic geometry. Monatshefte fuer Mathematik161(4), 381–398 (2010)

    Article  MATH  Google Scholar 

  76. Krýsl, S.: Howe duality for the metaplectic group acting on symplectic spinor valued forms. J. Lie Theory22(4), 1049–1063 (2012)

    MATH  MathSciNet  Google Scholar 

  77. Lávička, R.: On the structure of monogenic multi-vector valued polynomials. In: Simos, T.E., Psihoyios, G., Tsitouras, Ch. (eds.) ICNAAM 2009, Rethymno, Crete, Greece, 18–22 September 2009. AIP Conf. Proc., vol. 1168, pp. 793–796 (2009)

    Google Scholar 

  78. Lávička, R.: Canonical bases for sl(2,C)-modules of spherical monogenics in dimension 3. Arch. Math. Brno46(5), 339–349 (2010)

    MathSciNet  Google Scholar 

  79. Lávička, R.: The Fischer decomposition for the H-action and its applications. In: Sabadini, I., Sommen, F. (eds.) Hypercomplex Analysis and Applications. Trends in Mathematics, pp. 139–148. Springer, Basel (2011)

    Chapter  Google Scholar 

  80. Lávička, R.: Generalized Appell property for the Riesz system in dimension 3. In: Simos, T.E., Psihoyios, G., Tsitouras, Ch. (eds.) ICNAAM 2011, Halkidiki, Greece, 2011. AIP Conf. Proc., vol. 1389, pp. 291–294 (2011)

    Google Scholar 

  81. Lávička, R.: Complete orthogonal Appell systems for spherical monogenics. Complex Anal. Oper. Theory6(2), 477–489 (2012)

    Article  MATH  MathSciNet  Google Scholar 

  82. Lávička, R., Souček, V., Van Lancker, P.: Orthogonal basis for spherical monogenics by step two branching. Ann. Glob. Anal. Geom.41(2), 161–186 (2012)

    Article  MATH  Google Scholar 

  83. Lávička, R.: Orthogonal Appell bases for Hodge–de Rham systems in Euclidean spaces. Adv. Appl. Clifford Alg.23(1), 113–124 (2013)

    Article  MATH  Google Scholar 

  84. Liu, H., Ryan, J.: Clifford analysis techniques for spherical PDE. J. Four. Anal. Appl.8(6), 535–563 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  85. Lounesto, P.: Clifford algebras and spinors. London Math. Soc. LNS, vol. 238. CUP, Cambridge (1997)

    Google Scholar 

  86. Ørsted, B., Somberg, P., Souček, V.: The Howe duality for the Dunkl version of the Dirac operator. Adv. Appl. Clifford Alg.19(2), 403–415 (2009)

    Article  Google Scholar 

  87. Peetre, J., Qian, T.: Möbius covariance of iterated Dirac operators. J. Aust. Math. Soc. Ser. A56, 403–414 (1994)

    Article  MATH  MathSciNet  Google Scholar 

  88. Peña-Peña, D., Sabadini, I., Sommen, F.: Quaternionic Clifford analysis: the Hermitian setting. Complex Anal. Oper. Theory1, 97–113 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  89. Rarita, W., Schwinger, J.: On a theory of particles with half-integer spin. Phys. Rev.60, 61 (1941)

    Article  MATH  Google Scholar 

  90. Ryan, J.: Clifford Algebras in Analysis and Related Topics. Studies in Advanced Mathematics. CRC Press, Boca Raton (1996)

    MATH  Google Scholar 

  91. Sabadini, I., Struppa, D.C.: Some open problems on the Cauchy–Fueter system in several variables. Surikaisekikenkyusho Kokyuroku, Kyoto University1001, 1–21 (1997)

    MATH  MathSciNet  Google Scholar 

  92. Sabadini, I., Sommen, F.: Hermitian Clifford analysis and resolutions. Math. Methods Appl. Sci.25, 1395–1413 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  93. Salač, T.: Penrose transform and monogenic sections. Arch. Math.48(5), 399 (2012)

    MATH  MathSciNet  Google Scholar 

  94. Salač, T.: k-Dirac operator and parabolic geometries. Complex Anal. Oper. Theory8, 383–408 (2014)

    MATH  MathSciNet  Google Scholar 

  95. Sharpe, R.W.: Differential geometry. Cartan’s generalization of Klein’s Erlangen program. GTM, vol. 166. Springer, New York (1997)

    Google Scholar 

  96. Slovák, J.: Natural operators on conformal manifolds. Hab. dissertation, Masaryk Univeristy, Brno (1993)

    Google Scholar 

  97. Slovák, J., Souček, V.: Invariant operators of the first order on manifolds with a given parabolic structure. In: Proc. of the Conference, Luminy (1999)

    Google Scholar 

  98. Souček, V.: Clifford analysis for higher spins. In: Brackx, F., Delanghe, R., Serras, H. (eds.) Clifford Algebras and Their Applications in Mathematical Physics. In: Proc. of the Third Conference held at Deinze, pp. 223–232. Belgium (1993)

    Google Scholar 

  99. Souček, V.: Analogues of the Dolbeault complex and the separation of variables. In: Eastwood, M., Miller, V. (eds.) Symmetries and Overdetermined Systems of Partial Differential Equations. The IMA Volumes in Math. and Its Appl., pp. 537–550. Springer, New York (2007)

    Google Scholar 

  100. Stein, E., Weiss, G.: Generalization of the Cauchy–Riemann equations and representations of the rotation group. Am. J. Math.90, 163–196 (1968)

    Article  MATH  MathSciNet  Google Scholar 

  101. Sudbery, A.: Quaternionic analysis. Proc. Cambr. Phil. Soc.85, 199–225 (1979)

    Article  MATH  MathSciNet  Google Scholar 

  102. Vahlen, K.: Über Bewegungen un Complexe Zahlen. Math. Ann.55, 585–593 (1902)

    Article  MATH  MathSciNet  Google Scholar 

  103. Van Lancker, P.: Spherical monogenics: an algebraic approach. Adv. Appl. Clifford Alg.19, 467–496 (2009)

    Article  MATH  Google Scholar 

Download references

Acknowledgements

The work was supported by the grant GA CR P201/12/G028.

Author information

Authors and Affiliations

  1. Mathematical Institute, Charles University, Sokolovská 83, 186 75, Prague, Czech Republic

    Vladimír Souček

Authors
  1. Vladimír Souček
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Vladimír Souček .

Editor information

Editors and Affiliations

  1. Ben-Gurion University of the Negev, Department of Mathematics, Beer-Sheva, Israel

    Daniel Alpay

Rights and permissions

Reprints and permissions

Copyright information

© 2014 Springer Basel

About this entry

Cite this entry

Souček, V. (2014). Representation Theory in Clifford Analysis. In: Alpay, D. (eds) Operator Theory. Springer, Basel. https://doi.org/10.1007/978-3-0348-0692-3_22-1

Download citation

  • DOI: https://doi.org/10.1007/978-3-0348-0692-3_22-1

  • Received:

  • Accepted:

  • Published:

  • Publisher Name: Springer, Basel

  • Online ISBN: 978-3-0348-0692-3

  • eBook Packages: Springer Reference MathematicsReference Module Computer Science and Engineering

Publish with us

Policies and ethics

Search

Navigation