Pseudo-Hermitian Geometry in 3D

Abstract

This chapter concerns CR geometry, a research field for which there is an extremely fruitful interaction of different ideas, ranging from Differential Geometry, Partial Differential Equations and Complex Analysis. First, some basic concepts of the subject are introduced, as well as some conformally covariant operators. Then some surprising relations are shown between the embeddability of abstract CR structures, the spectral properties of the Paneitz operator and the attainment of the Yamabe quotient. Finally, some surface theory is treated, in relation to the isoperimetic problem, the prescribed mean curvature problem and to some Willmore-type functionals.

4.1 Introduction

In this series of lectures I plan to discuss several geometric/analytic problems in CR-geometry. They are, in order of presentation: the embedding problem, the CR Yamabe problem, the Q-prime curvature equation and finally the geometry of surfaces in the Heisenberg group. After a brief introduction to the local invariants defined by Tanaka and Webster we will follow the following table of contents. I would like to thank Matt Gursky and Andrea Malchiodi for the invitation to give this series of lectures at the Cetraro Summer School.

• 1. Local invariants: the Webster connection, torsion and curvature.

• 2. Conformally covariant operators, the CR conformal Laplacian, the CR Paneitz operator and the Q-curvature.

• 3. Pluriharmonic functions, Fefferman equations, psuedo-Einstein contact form, the P-prime operator and the Q-prime curvature, the Burns-Epstein invariant.

• 4. The embedding problem and the Kohn Laplacian, sign of the Paneitz operator.

• 5. Positive mass theorem.

• 6. The Q-prime curvature equation: a sharp inequality for the total Q-prime curvature, an existance theorem for the Q-prime curvature.

• 7. Isoperimetric inequality for the total Q-prime curvature.

• 8. Geometry of surfaces in the Heisenberg group, the mean curvature and the angle function α, the analysis of the Codazzi equation.

• 9. Analogues of the Willmore functional, renormalized area, examples and open problems.

In these notes we will follow the notational conventions in [26].

4.2 Definitions

A pseudo-Hermitian 3-manifold is a triple (M, θ, J) where θ is a 1-form satisfying θ ∧ dθ ≠ 0 and letting ξ = KerJ, the almost complex structure is given by J : ξ → ξ satisfying J ² = −I. The Reeb vector field T is uniquely determined by θ(T) = 1 and dθ(T, ⋅) = 0.

Let H denote the + i eigenspace of $\mathbb {C} \otimes \xi$ and Z ₁ is a local frame for H normalized by the condition $d\theta (Z_1, \bar {Z}_1)=1.$ Let {θ, θ ¹, θ ^T} be the dual 1-form to $\{T, Z_1, Z_{\bar 1}\}$.

$$\displaystyle \begin{aligned}d\theta = i\theta^1 \wedge a^{\bar{1}}.\end{aligned}$$

The Webster connection is characterized by

where $\omega _1^1$ is the 1-form uniquely determined by

$$\displaystyle \begin{aligned}d\theta^1= \theta^1 \wedge \omega _1^1 + A_{\bar{1}}^1 \theta \wedge \theta^{\bar{1}}, \omega _1^1 + \omega_{\bar{1}}^{\bar{1}} = 0.\end{aligned}$$

$A_{\bar {1}}^1$ is called the torsion tensor.

$$\displaystyle \begin{aligned}d\omega_1^1 = R \theta^1 \wedge \theta^{\bar{1}} + A_{1, \bar{1}}^{\bar{1}}\theta^1 \wedge \theta - A_{\bar{1},1}^1 \theta^{\bar{1}}\wedge \theta\end{aligned}$$

where R is the Webster scalar curvature.

Examples

• 1. The Heisenberg $\mathbb {H}^1 = \{(x,y,t) \in \mathbb {R}^3 | \theta = dt + xdy - ydx\}$Z ₁ = ∂ _x − i∂ _y + (ix + y)∂ _t, T = ∂ _t. This is the flat model: A = 0 and R = 0.

• 2. The unit sphere $S^3 = \{(z_1, z_2)\in \mathbb {C}^2 | |z_1|{ }^2 + |z_2|{ }^2 = 1\}$

  $$\displaystyle \begin{aligned}\theta = i \sum_{k=1}^2{({\bar z}^k dz^k - z^kd\bar{z}^k)}, z_1 = \bar{z}^2\partial_{z^1} - \bar{z}^1 \partial_{z^2}\end{aligned}$$

  A = 0 and R = 1.

The Cayley transform: F : S ³∖{(0, −1)}→ H ¹

$$\displaystyle \begin{aligned}x=Re \frac{z_1}{1+z_2}, y= Im \frac{z_1}{1+z_2}, t=\frac{1}{2} Re \left\{i \frac{1-z_2}{1+z_2}\right\}.\end{aligned}$$

It is elementary to observe that the nondegeneracy condition θ ∧ dθ ≠ 0 means the distribution ξ = Kerθ is not integrable. That is if X and Y  are vector fields satisfying θ(X) = θ(Y ) = 0 then 0 ≠ dθ(X, Y ) = Xθ(Y ) − Y θ(X) − θ([X, Y ]) if X, Y  are independent.

The basic regularity theory of the $\bar {\partial }_b$ equation on the Heisenberg group is established by Folland and Stein [21] making use of Fourier analysis on the Heisenberg group. Explicit kernels are given. In this way they recover the basic estimates of Kohn: $C||u||{ }_{\frac {1}{2}} \leq ||\bar {\partial }_b u||{ }_{L^2} + ||u||{ }_{L^2}.$

By introducing the space $S^p_k=\{$ function with k tangential derivatives in L ^p}, they obtained the more precise result:

Let L _αu = f where L _αu = △_bu − iαT when ± α ≠ n, n + 2, n _x, … then the following local estimates hold if $f \in S^p_k(U)$ and V ⊂⊂ U then $u\in S^P_{k+2}(V).$

Definition

A CR structure is locally spherical if there is a local biholomorphic map to the standard sphere.

Definition

The Cartan tensor [18] is defined as

$$\displaystyle \begin{aligned}Q_J=iQ_1^{\bar{1}}\theta^1 \otimes Z{\bar{1}} - iQ^1_{\bar{1}} \theta^{\bar{1}}\otimes Z_1\end{aligned}$$

where

$$\displaystyle \begin{aligned}Q^{\bar{1}}_1 = \frac{1}{6}R_{,1}^{\bar{1}} + \frac{i}{2}RA_1^{\bar{1}}-A_{1,0}^{\bar{1}} - \frac{2i}{3}A_{1,T}^{\bar{1}\bar{1}}.\end{aligned}$$

The vanishing of Q _J is necessary and sufficient for the structure to be locally spherical.

4.3 Conformally Covariant Operators

The CR conformal Laplacian is given by:

It is elementary to check that under a conformal change of contact form $\tilde {\theta } = u^2\theta$

$$\displaystyle \begin{aligned} L_{\tilde{\theta}} f = u^{-3}L_\theta (uf)\end{aligned}$$

and

$$\displaystyle \begin{aligned} L_\theta u = \tilde{R}u^3\end{aligned}$$

where $\tilde {R}$ is the scalar curvature of $\tilde {\theta }.$ Just like the Yamabe problem, there is a variational functional

$$\displaystyle \begin{aligned}q[u]=\frac{\int{Lu\cdot u \theta \wedge d\theta }}{||u||{}^2_4}.\end{aligned} $$

The Euler equation for critical points of q[u] is the CR Yamabe equation

$$\displaystyle \begin{aligned}L_\theta u = \lambda u^3\end{aligned}$$

where λ is the Lagrange multiplier.

The CR Paneitz operator is given by

$$\displaystyle \begin{aligned}P \varphi = 4(\varphi^{\bar{1}}_{\bar{1}1}+ iA_{11} \varphi^\prime)^1 = (P_3\varphi)^1.\end{aligned} $$

The operator P is real, self-adjoint operator and satisfies the covariance property: for $\tilde {\theta }=u^2\theta$

$$\displaystyle \begin{aligned}P_{\tilde{\theta}}\varphi=u^{-4}P_\theta \varphi.\end{aligned}$$

The third order operator P ₃ characterizes pluriharmonic functions [26]. The Paneitz operator is closely related to Kohn’s Laplacian

we have

$$\displaystyle \begin{aligned} P\phi & = \frac{1}{4} (\square_b \circ \bar{\square}_b \varphi - 4i(A^{11}\varphi_1)_1)\\ & = \frac{1}{4} (\bar{\square} \circ \square_b \varphi + 4i (A^{\bar{1}\bar{1}}\varphi_{\bar{1}})_{\bar{1}}) \end{aligned} $$

When the torsion vanishes, the operators □_b and $\bar {\square }_b$ commute, hence they are simultaneously diagonalizable, and in this case it follows that eigenvalues of P are non-negative. The Paneitz operator is closely connected with Q curvature

which appears [23] as the coefficient of the logarithm term in the asymptotic expansion of the Szego kernel for a C ^∞ strictly pseudoconvex domain $\Omega \in \mathbb {C}^2$ with respect to a volume element θ ∧ dθ on ∂ Ω

$$\displaystyle \begin{aligned}S(z,\bar{z}) = \varphi (z) \rho(z)^{-2} + \psi (z) \log \rho(z)\end{aligned}$$

with $\varphi , \psi , \in C^\infty (\bar {\Omega })$ where ρ is a defining function ρ > 0 on Ω. Under change of contact form $\tilde {\theta } = e^{2f}\theta , \psi _0 = \psi |{ }_{\partial \Omega }$ transforms as

$$\displaystyle \begin{aligned}\tilde{\psi}_0 = e^{-4f}\left(\psi_0+\frac{1}{12}Pf\right).\end{aligned} $$

Closely related is the 1-form

$$\displaystyle \begin{aligned}W_1\theta^1 = (R_{,1}-iA_{11}^1)\theta^1,\end{aligned} $$

so that

and W ₁ transforms as

$$\displaystyle \begin{aligned}\tilde{W}_1 = e^{-3f} (W_1-6P_3f)\end{aligned}$$

where $P_3f = f_{\bar {1}1}^{\bar {1}}+iA_{11}f^1$ is the operator that defines pluriharmonic functions.

4.4 Fefferman’s Equation, Pseudo-Einstein Contact Form, P ^′ and Q ^′

The question whether there is a non-zero coefficient ψ ₀ in the Szego kernel expansion is answered by the work of Fefferman [20] where he considered the following Monge Ampere equation for a defining function u of a strictly pseudo convex domain Ω in $\mathbb {C}^2$:

(4.4.1)

A smooth solution of this equation in the interior [17] gives a complete Kahler–Einstein metric g whose Kahler form is $i\partial \bar {\partial } \log (u).$ In general, the solution may not be smooth at the boundary. C. Fefferman provides an iterative process to compute approximate solutions to (4.4.1). Let ψ be any smooth defining function in ∂ Ω, let

Then u _s satisfies J[u _s] = 1 + O(ψ ^s).

It turns out that for the contact form $\theta = Im \bar {\partial }u_3$ satisfies the equation W ₁ = 0 and hence has Q = 0.

Contact forms θ satisfying the condition $R_{,1}-iA_{11,}^1=0$ are called pseudo-Einstein on account of the following consequence of the Bianchi identity in M ²ⁿ⁺¹

$$\displaystyle \begin{aligned}\nabla^{\bar{\beta}}\left(R_{\alpha \bar{\beta}} - \frac{1}{n}Rh_{\alpha\bar{\beta}}\right) = \frac{n-1}{n}\left(\nabla_\alpha R-in\nabla^\beta A_{\alpha\beta}\right).\end{aligned}$$

Thus when n = 1, the pseudo-Einstein condition is the “residue in n ” of the general pseudo-Einstein condition.

The pseudo-Einstein contact forms are in 1 −to− 1 correspondence with pluriharmonic functions according to

Lemma

If θ is pseudo-Einstein then $\tilde {\theta } = e^{2f}\theta$ is pseudo-Einstein iff f is pluriharmonic.

This is evident from the transformation rule for W ₁ at the end of Sect. 4.3.

P-prime operator and Q-prime curvature:

In general dimensions there is the CR-Paneitz operator of order 4 on (M ²ⁿ⁺¹, θ, τ) (Gover/Graham)

where

where

$$\displaystyle \begin{aligned} P_{\alpha\bar{\beta}} &= \ \frac{1}{n+2}(R_{\alpha\bar{\beta}} - \frac{1}{2(n+1)}Rh_{\alpha\bar{\beta}} )\\ P &= tr P = \frac{R}{2(n+1)} \end{aligned} $$

P ₄ is conf. covariant of degree $(-\frac {n-1}{2}, \frac {n+3}{2}).$

Definition ([7])

and

Observe

So

(4.4.2)

The totalQ ^′-curvature and the Burns–Epstein invariant

In case M ³ = ∂X where X is a strictly pseudoconvex domain in $\mathbb {C}^2$ the solution of Fefferman’s equation gives X a complete Kahler Einstein metric and there is a Gauss Bonnet type formula

$$\displaystyle \begin{aligned}C \chi(X)= \int_X (c_2-\frac{1}{3}c_1^2)+\oint_M Q^\prime \theta \wedge d\theta\end{aligned}$$

where Q ^′ is the Q ^′ curvature, and $\oint Q^\prime \theta \wedge d\theta$ agrees with the Burns–Epstein invariant [4] given by the integral of the 3-form

$$\displaystyle \begin{aligned} i\left[\left(-\frac{2i}{3}\omega^1_1 \wedge d\omega^1_1 + \frac{1}{6}(R\theta) \wedge d\omega^1_1 - 2|A|{}^2 \theta \wedge d\theta\right)\right] \end{aligned} $$

(4.4.3)

where $\omega ^1_1$ is the connection form, R the scalar curvature, A the torsion.

Lemma

A contact form θ is pseudo-Einstein iff there exists frames θ, θ ¹so that $h_{\alpha _{{\beta }}} = \delta _{\alpha \bar {\beta }}$and $\omega ^1_1 + (\frac {i}{n})R\theta =0$

Proof

This is elementary.

It then follows that (4.4.3) yields (4.4.2).

Remark

For KE metrics the integrand $c_2-\frac {1}{3}c^2_1$ is a sum of squares, and its integral is always finite. No renormalization is needed.

4.5 The Embedding Problem

The almost complex structure J gives a splitting

$$\displaystyle \begin{aligned}d_b = \partial_b + \bar{\partial}_b,\end{aligned}$$

where $\partial _b f= Z_1(f)\theta ^1, \bar {\partial }_b f= Z_{\bar {1}}(f) \theta ^{\bar {1}}$

The usual integrability condition

$$\displaystyle \begin{aligned}\bar{\partial}_b^2=0\end{aligned}$$

becomes vacuous in dimension 2n + 1 = 3. In dimensions 2n + 1 ≥ 5, under this integrability condition, closed CR manifolds may be embedded holomorphically in $\mathbb {C}^N$ [2]. Indeed Kohn showed that if □_b on functions has closed range, then the CR structure is embeddable.

Theorem ([6])

If (M ³, θ, J) satisfy the condition P ≥ 0 and the Webster scalar curvature R ≥ c > 0, then the non-zero eigenvalues λ of □_bhave the lower bound

$$\displaystyle \begin{aligned}\lambda \geq \min R \geq c > 0.\end{aligned}$$

It follows that □_b has closed range. Hence the CR structure is embeddable.

The proof is a simple application of the following Bochner formula

$$\displaystyle \begin{aligned} -\frac{1}{2} \square_b | \bar{\partial}_b \varphi|{}^2 &= (\varphi_{\bar{1}\bar{1}} \bar{\varphi}_{11} + \varphi_{\bar{1}1}\bar{\varphi}_{1\bar{1}}) - \frac{1}{2} \langle \bar{\partial}_b \varphi, \bar{\partial}_b \square_b \varphi \rangle - \langle \bar{\partial}_b \square_b \varphi, \bar{\partial}_b \varphi \rangle\\ &- \langle \bar{P}_3 \varphi, \bar{\partial}_b \varphi \rangle + R|\bar{\partial}_b \varphi|{}^2 . \end{aligned} $$

Apply this to an eigenfunction φ with non-zero eigenvalue. Integrating this Bochner formula we find

$$\displaystyle \begin{aligned}0=\int \varphi_{\bar{1}{\bar{1}}} \bar{\varphi}_{11} + \int \varphi_{\bar{1}1}\bar{\varphi}_{1\bar{1}} - \frac{3}{2} \lambda \int | \bar{\partial}_b \varphi|{}^2 + \int\langle P\varphi, \varphi\rangle + \int{R|\bar{\partial}_b\varphi|{}^2}.\end{aligned}$$

Rewriting

$$\displaystyle \begin{aligned}\int \varphi_{\bar{1}1} \bar{\varphi}_{1\bar{1}} = \frac{1}{4} \int \langle \square, \varphi, \square_b \varphi \rangle = \frac{\lambda}{2}\int |\bar{\partial}_b \varphi|{}^2,\end{aligned}$$

we obtain

$$\displaystyle \begin{aligned}\lambda \int |\bar{\partial}_b \varphi|{}^2 = \int |\varphi_{\bar{1}\bar{1}}|{}^2 + \int P\varphi \cdot \bar{\varphi} + \int R|\bar{\partial}_b \varphi|{}^2 \geq \int P\varphi \cdot \bar{\varphi} + \int R |\bar{\partial}_b \varphi |{}^2 .\end{aligned}$$

Example of Rossi [3]

On (S ³, θ ₀, J ₀) let $Z_1 = \bar {z}_2 \partial _{z_1} - \bar {z}_1 \partial _{z_2}$.

Change the CR structure to

$$\displaystyle \begin{aligned}Z_{1(t)} = Z_1 + t \bar{Z}_1\end{aligned}$$

where |t| < 1, keeping the same contract form.

An elementary argument using spherical harmonics shows that all holomorphic functions with respect to $\bar {Z}_{1(t)} f = 0$ are even, i.e. f(z ₁, z ₂) = f(−z ₁, −z ₂). Hence it is not possible to separate points by holomorphic functions.

However, a relatively easy computation shows

$$\displaystyle \begin{aligned}R(t)=\frac{2(1+t^2)}{1-t^2} \geq 2.\end{aligned}$$

On the other hand, $(S^3/\mathbb {Z}_2, \theta _\lambda J_t)$ does embed [10].

Kohn’s solution of □_b on (M ³, θ, J) if M ³ = ∂ Ω in a Stein manifold. There exists an solution operator K on L ²(M ³)

$$\displaystyle \begin{aligned}\square_b \circ K = K \circ \square_b = Id -S\end{aligned}$$

where S is the Szego projection with respect to θ ∧ dθ. Such K exists when $\bar \partial _b : L^2\to L^2_{(0,1)}$ has closed range and $||u||{ }_{1/2} \leq C||\bar {\partial }_b u ||{ }^2$ if $u \bot N(\bar {\partial }_b).$

When Is P ≥ 0?

Recall Pφ = δ _bP ₃φ where $P_3\varphi = (\varphi _{\bar {1}1}^{\bar {1}} + i A_{11} \varphi ^1 ) \theta ^1$ and P ₃φ = 0 is equivalent to φ being pluriharmonic written $\varphi \in \cal {P}$. It follows that $Ker P \subset \cal {P}$.

Typically $\cal {P}$ is an infinite dimensional space if (M ³, θ, J) is embeddable.

Let us write $Ker{ P} = \mathcal {P} \oplus W$.

For an embeddable CR structure, W is at most finite dimensional.

When Is W Empty?

About the supplementary space W the following estimate is useful:

Lemma

Suppose λ ₁(□_b) ≥ C > 0, for $f \in S^{4,2} \cap \cal {P}^{\bot }$there exists C ₁:

$$\displaystyle \begin{aligned} C_1 ||f||{}_{s^{4,2}}\leq ||Pf||{}_2 + ||f||{}_2. \end{aligned}$$

Proof

$f \in \cal {P}^{\bot }$ implies f⊥Ker∂ _b. Hence there exists solution ψ to $\bar {\square }_b \psi =f$.

Let h be an antiholomorphic function

$$\displaystyle \begin{aligned} \langle {\square}_b f, h \rangle &= \langle \square_b \bar{\square}_b \psi, h \rangle \\ &= \langle \psi, (\bar{\square}_b \square_b - 2 \bar{Q}) h\rangle + 2 \langle \psi, \bar{Q} h \rangle, \end{aligned} $$

where

$$\displaystyle \begin{aligned}P = \bar{\square}_b \square_b - 2 \bar{Q},\end{aligned}$$

therefore

$$\displaystyle \begin{aligned} \langle \square_b f - 2 Q \psi, h \rangle = 0.\end{aligned}$$

Also

$$\displaystyle \begin{aligned}\lambda_1 (\square_b) \geq c > 0 \Rightarrow\end{aligned}$$

$$\displaystyle \begin{aligned}C_1 || f ||{}_{S^{4,2}} \leq || \square_b f ||{}_{S^{2,2}} + ||f||{}_2\end{aligned}$$

$$\displaystyle \begin{aligned} \leq || \square_b f - 2 Q \psi ||{}_{S^{2,2}} + 2||Q \psi ||{}_{S^{2,2}} + ||f||{}_2 . \end{aligned} $$

(*)

Since $(\square f - 2Q \psi ) \bot$ antiholomorphic

$$\displaystyle \begin{aligned} C_2 || \square_b f - 2 Q \psi ||{}_{S^{2,2}} &\leq || \bar{\square_b}(\square_b f - 2Q \psi)||{}_2 + || \square_b f- 2 Q \psi ||{}_2\\ \Rightarrow C_3 ||f ||{}_{S^{4,2}} &\leq || P f ||{}_2 + || \bar{Q} f ||{}_2 + ||Q \psi ||{}_{S^{2,2}} + ||f||{}_{S^{2,2}} + ||f||{}_2\\ \Rightarrow C || \psi ||{}_{S^{4,2}} &\leq ||f||{}_{S^{2,2}} + ||f||{}_2\\ \Rightarrow C_3 || f||{}_{S^{4,2}} &\leq ||Pf||{}_2 + \underbrace{||f||{}_{S^{2,2}}}_{\leq} + ||f||{}_2\\ &\quad \times \epsilon ||f||{}_{S^{4,2}}+ C_\epsilon ||f||{}_2. \end{aligned} $$

(**)

□ □

Corollary

For an embedded CR structure $Ker P = \mathcal {P} + W$ , where $\dim W<\infty$

Definition

For a one-parameter-family of CR structures (M ³, θ, J _t) we say that $\mathcal {P} ^t$ is stable if for any $\varphi \in \mathcal {P} ^t$, and 𝜖 > 0, there exists δ > 0 so that for |t − s| < δ there is a $f_s \in \mathcal {P}^s$ so that

$$\displaystyle \begin{aligned}||\varphi - f_s||{}_2 < \epsilon.\end{aligned}$$

Theorem ([9])

Let (M ³, θ, J ^t) be a family of embedded CR structures for t ∈ [−1, 1] with the following

• 1. J ^tis real analytic in the parameter t

• 2. The Szego projection $S^t=F^{2,0} \to Ker \bar {\partial _b}^t \subset F^{2,0}$vary continuously in the parameter t (def F ^{2, 0}later)

• 3. For J ⁰we have P ⁰ ≥ 0 and $Ker P^0 = \mathcal {P}^0$

• 4. There exists a uniform c > 0 s.t.

  $$\displaystyle \begin{aligned}\min_{-1 \leq t \leq 1} R^t \geq c > 0\end{aligned}$$

• 5. The pluriharmonics $\mathcal {P}^t$are stable with respect to t

then

$$\displaystyle \begin{aligned}P^t \geq 0 \mathit{\mbox{ and }} Ker P^t = \mathcal{P} ^t \,\,\mathit{\text{for all}}\,\, t \in [-1,1]. \end{aligned}$$

Proof

By continuity:

Let

$$\displaystyle \begin{aligned}S=\{t\in[-1,1] | P^t \geq 0 \, \,and \,\, Ker P^t = \mathcal{P}^t\}.\end{aligned}$$

S is open:

The small eigenvalues of P ^t are finitely many and parameterized by λ _i(t) real analytic in t, i = 1, ⋯ , k. To be precise let there be no eigenvalues of P ⁰ in the intervals (−r, 0) ∨ (0, r), and the eigenvalue of P ^t in these intervals are called small eigenvalues of P ^t, |t| small. Assume to the contrary u _t is an eigenfunction for P ^t with small eigenvalue. Write u _t = u ₀ + f _t where u ₀ ∈ KerP ⁰ and ||f _t||₂ = o(1), ||u ₀||₂ = 1. Stability implies that there is g _t ∈ KerP ^t such that ||u ₀ − g _t|| < 𝜖

$$\displaystyle \begin{aligned} 0&= \langle u_t, g_t\rangle\\ &= \langle u_t, u_0\rangle + \langle u_t, g_t - u_0\rangle\\ &= 1+ \langle f_t, u_0 \rangle + \langle u_t, g_t-u_0 \rangle = 1 + o(1) . \end{aligned} $$

(*)

Next we check W ^t = {0} for |t| small. Observe the constant c in the Lemma can be taken uniformly in t. So if to the contrary, there are $f_{t_k} \in W_{t_k} \,\,|| f_{t_k}||{ }_1 =1, t_k \to t_0$ the Lemma implies $||f_{t_u}||{ }_{W^{4,2}} \leq c.$ Hence a subsequence converges strongly in L ².

$$\displaystyle \begin{aligned}P^{t_0} f_0 = 0 \Rightarrow f_0 \in \mathcal{P}^{t_\circ}\end{aligned}$$

Stability implies that given 𝜖 > 0 ∃δ > 0 s.t.

$$\displaystyle \begin{aligned}|t-t_0|<\delta \Rightarrow \exists \psi_t \in \mathcal{P}^t\end{aligned}$$

$$\displaystyle \begin{aligned}||f_0-\psi_t ||{}_2 < \epsilon\end{aligned}$$

$$\displaystyle \begin{aligned}1 = ||f_0||{}^2_2 = \underset{\underset{0}{\downarrow}}{\langle f_0-\psi_{t_k}, f_0 \rangle} + \underset{\underset{0}{\downarrow}}{\langle \psi_{t_k}, f_0 - f_{t_k} \rangle} + \underset{\underset{0}{||}}{\langle \psi_{t_k}, f_{t_k} \rangle}\end{aligned}$$

$$\displaystyle \begin{aligned} =o(1) \end{aligned}$$

(*)

For closedness of S we need to introduce the Garfield-Lee complex. Let

$$\displaystyle \begin{aligned}E^{0,0} = \langle 1 \rangle, E^{1,0} = \langle \theta^1 \rangle, E^{0,1} = \langle \theta^{\bar{1}} \rangle, F^{2,0} = \langle \theta^1 \wedge \theta \rangle, F^{1,1} = \langle \theta^{\bar{1}} \wedge \theta \rangle\end{aligned}$$

F ^{2, 1} = 〈θ ∧ dθ〉 be the line bundles

Here $d^\prime = \partial _b, d^{\prime \prime } = \bar {\partial }_b$ and D ^′, D ^′′andD ⁺ are the second order operators introduced by Rumin [29]

$$\displaystyle \begin{aligned} D^\prime (\sigma_1, \theta^1) &= (-i\sigma_{1, \bar{1}1} -\sigma_{1,0} )\theta^1 \wedge \theta\\ D^{\prime\prime} (\sigma_1 \theta^1) &= (-i \sigma_{1,\bar{1}\bar{1}} - A_{\bar{1}{\bar1}} \sigma_1) \theta^{\bar{1}} \wedge \theta\\ D^\prime (\sigma_{\bar{1}} \theta^{\bar{1}}) &= \langle i \sigma_{\bar{1},1\bar{1}} -\sigma_{\bar{1},0} \rangle \theta^1 \wedge \theta\\ D^+ (\sigma_{\bar{1}} \theta^{\bar{1}}) &= \langle i \sigma_{\bar{1},11} -A_{11} \sigma_{\bar{1}} \rangle \theta^1 \wedge \theta \end{aligned} $$

It follows that

$$\displaystyle \begin{aligned} P_3 &= -i D^+ d^{\prime\prime}\\ P&= -id^{\prime\prime} D^+ d^{\prime\prime} \end{aligned} $$

The supplementary subspace W may be realized as

$$\displaystyle \begin{aligned} W &\cong Ker d^{\prime\prime} \cap im D^+d^{\prime\prime} \subset F^{2,0}\\ &\cong Ker d^{\prime\prime} \cap Im P_3 \subset F^{2,0} \end{aligned} $$

Let

$$\displaystyle \begin{aligned}F^t = Ker \bar{\partial}_b^ {t} \cap Im P_3^t \subset F^{2,0},\end{aligned}$$

so that

$$\displaystyle \begin{aligned}dim F^t = dim W^t .\end{aligned}$$

ClaimdimF ^t is a lower semicontinuous function of t.

Let S ^t be the Szego projector in F ^{2, 0}. Then rank $(S^t_0 P^t_3) = dim F^t < \infty .$ For fixed φ ∈ E ⁰⁰ψ ∈ (F ^{2, 0})^∗⊗ L ²,

$$\displaystyle \begin{aligned}h(t) = \langle S^t_0 P_3^t (\varphi), \psi \rangle \end{aligned}$$

is continuous. It suffices to check that

$$\displaystyle \begin{aligned} G = \{ t \in [-1,1]| \mbox{ rank } (S^t_0P_3^t)>a\}\end{aligned}$$

is open for any a.

Set r =  rank $S^{t_{0_0}} P_3^{t_0} > a$.

There exist $\{\varphi _i\}_{i=1}^r$ and functions $\{\psi _i\}_{i=1}^r,$ s.t.

$$\displaystyle \begin{aligned}h_{ij} (t_0) = \langle S^{t_0} \circ P_3^{t_0} \varphi_i, \psi_j \rangle\end{aligned}$$

is continuous:

To finish the proof,

Let t _n ∈ S, t _n → t ₀. We have $P^{t_0} \geq 0$ and

$$\displaystyle \begin{aligned}\bar{\lim_{t_n \to t_0}} dim W^{t_n} \leq dim W^{t_0}\end{aligned}$$

Lower semi-continuity implies then $dim W^{t_0} \leq \liminf dim W^{t_n} =0$. □

A typical family would start with J ⁰ so that the torsion vanishes. For example, the ellipsoids form such a family with J ⁰ the standard sphere.

In [1] Bland determined embeddable deformations of J on the standard 3-sphere in terms of conditions on the Fourier coefficients of $\dot J$.

In a recent preprint [30], Takeuchi showed that the embeddedness implies P ≥ 0 and the kernel of P consist of pluriharmonics.

4.6 The Positive Mass Theorem

For (M ³, θ, J) we consider the variational problem to find the minimizer of the Sobolev quotient

$$\displaystyle \begin{aligned}q[u] = \frac{\int L u\cdot u}{||u||{}_4^2}.\end{aligned}$$

The interesting case is when the CR conformal Laplacian L _θ is a positive operator. The same argument as in the case of conformal geometry applies to reduce this problem to finding a test function u for which q[u] is strictly smaller than the corresponding constant as the standard 3-sphere

$$\displaystyle \begin{aligned}q_0 [1] = \frac{\int (L_0 \cdot 1 \cdot)\cdot 1}{(\mbox{vol } S^3)^{\frac{1}{2}}}.\end{aligned}$$

It turns out that this may not be possible for some CR structures (M, θ, J) with L _θ > 0. What is required is a positive mass theorem which we describe.

Given (M, θ, J) with L _θ > 0, the Green’s function for L _θG(p, ⋅) = δ _p exists with pole at p ∈ M. To describe the asymptotic behaviour of G(p, ⋅) near the pole, Jerison and Lee [25] showed that after making suitable conformal change of contact form, there will exists local coordinate system (x, y, t) with p at the origin so that denoting by

the new contact form $\hat {\theta }$ and local frame $\hat {\theta }^1$ are of the form:

and the Green’s function satisfies

$$\displaystyle \begin{aligned}G_p = \frac{1}{2\pi \rho^2} + A + O(\rho) \mbox{ near } p.\end{aligned}$$

Consider the “blowup” of M∖{p} with

$$\displaystyle \begin{aligned}\theta = G^2_\rho \hat{\theta}.\end{aligned}$$

Making use of the inversion

$$\displaystyle \begin{aligned}z_* = \frac{z}{v}, \,\,t_* = \frac{t}{|v|{}^2}, \,\,v = t+i|z|{}^2,\,\, \rho_* = \frac{1}{\rho},\end{aligned}$$

we find

(AF1 )

Rotate θ ¹ by $e^{i\psi }:= -\frac {v_*^3}{\rho _*^6}$ we find

(AF2 )

This motivates the definition of an asymptotically Heisenberg end i.e. (N ³, J, θ) is called asymptotically flat if N = N ₀ ∪ N _∞ where N ₀ compact and N _∞ is diffeomorphic to $H|{ }^\prime \backslash B_{\rho _0}$ in which (J, θ) is close to in this sense i.e. AF ₁ and AF ₂ hold.

Define the mass for AF manifold N to be

where S _∧ = {ρ = ∧}.

Remarks

• 1. It follows that, for a family J ^(s) of CR-structures which are AF with fixed θ,

  $$\displaystyle \begin{aligned}\frac{d}{ds}|{}_{s=0} \left(-\int_N R(s) \theta \wedge d\theta + m (J(S), \theta)\right) = \int_N (A_{11} E_{\bar{1}\bar{1}}+A_{\bar{1}\bar{1}}E_{11}) \theta \wedge d\theta\end{aligned}$$

  where

  $$\displaystyle \begin{aligned}\overset{\cdot}{J} = 2E = 2E_{11}\theta^1 \otimes Z_{\bar{1}} + 2E_{\bar{1}\bar{1}}\theta^{\bar{1}} \otimes Z_1.\end{aligned}$$

• 2. If (N, θ, J) arises out of a compact (M, θ, J) as we did then

  $$\displaystyle \begin{aligned}m(J,\theta) = 48\pi^2 A.\end{aligned}$$

Theorem ([14])

Let (N, θ, J) be an asymptotically flat CR manifold, suppose L _θ > 0, andP ≥ 0 then the mass m ≥ 0 and equality can hold only if (N, θ, J) is biholomorphic to the Heisenberg group $\mathbb {H}^1$.

Idea of Proof

Look for a function $\beta : N \to \mathbb {C}$ smooth such that

$$\displaystyle \begin{aligned}\beta = \bar{z}+ \beta_{-1} + O (\rho^{-2+\epsilon}) \mbox{ near } \infty\end{aligned}$$

and

$$\displaystyle \begin{aligned}\square_b \beta = O(\rho^{-4}),\end{aligned}$$

where β ₋₁ is a term of homogeneity ρ ⁻¹ s.t.

$$\displaystyle \begin{aligned} (\beta_{-1})_{,\bar{1}} = -2 \sqrt{2} \frac{\pi A}{\rho^2} - \frac{\sqrt{2}A}{|z|{}^2+it} \end{aligned}$$

(*)

then

$$\displaystyle \begin{aligned}\frac{2}{3} m(J,\theta) = \int_N \{ - |\square_b \beta |{}^2 + z| \beta_{,\bar{1}\bar{1}}|{}^2 + 2R|\beta_{1\bar{1}}|{}^2 + \frac{1}{2} P \beta \cdot \bar{\beta} \} \theta \wedge d \theta.\end{aligned}$$

We find β ₋₁ by “hand”: choose a cut off function $\eta : \mathbb {H}^1 \to \mathbb {R}$

$$\displaystyle \begin{aligned} \eta(z,t)=0 & \mbox{ in a neighborhood of } (0,0) \end{aligned} $$

(4.6.1)

$$\displaystyle \begin{aligned} \eta(z,t)=1 & \mbox{ in a neighborhood of } \infty \end{aligned} $$

(4.6.2)

to solve for $\overset {\circ }{\square _b} \beta _{-1} = -\eta f$ near ∞ where

$$\displaystyle \begin{aligned}f=4\pi A \frac{\bar{z}(|z|{}^2 + it)}{\rho^6} = \square_b \bar{z} + O (\rho^{-4})\end{aligned}$$

making use of solvability of $\overset {\circ }{\square _b}$ on $\mathbb {H}^1$:

$$\displaystyle \begin{aligned}\overset{\circ}{\square_b}K = K\overset{\circ}{\square_b} = I - S \mbox{ on } L^2(\mathbb{H}^1)\end{aligned}$$

where

$$\displaystyle \begin{aligned}Kh =- h*\Phi, \Phi = \frac{1}{8\pi^2} \log (\frac{|z|{}^2 - it}{|z|{}^2 + it}) \cdot (|z|{}^2 - it)^{-1}.\end{aligned}$$

Then it follows that

$$\displaystyle \begin{aligned}\beta_{-1} = K(-\eta f)+O(\rho^{-2+\epsilon}).\end{aligned}$$

and (*) holds.

Existence of a Solution □_bβ = 0

This follows from the work of Hsiao and Yung [24] where they studied a weighted □_b problem

$$\displaystyle \begin{aligned}\square_{b,1}:= G_\rho^2 \square_b , m_1 := G_\rho^{-2} \theta \wedge d\theta\end{aligned}$$

$$\displaystyle \begin{aligned} \square_{b,1} : \mbox{Dom }(\square_{b,1}) \subseteq L^2(m_1) \to L^2(m_1) \mbox{ has closed range} \end{aligned}$$

and

$$\displaystyle \begin{aligned} \square_{b,1} K+S = I \mbox{ on } L^2(m_1) \end{aligned}$$

for each 𝜖 in (0, 2) such that

$$\displaystyle \begin{aligned} \square_{b,1} K+S = Id \mbox{ on } {\cal E} (\rho^{-2+\epsilon}) \end{aligned}$$

$$\displaystyle \begin{aligned} K: {\cal E}(\rho^{-2+\epsilon}) \to {\cal E}(\rho^{\epsilon}) \ \ S: {\cal E}(\rho^{-2+\epsilon}) \to {\cal E} (\rho^{-2+\epsilon}) \end{aligned}$$

where $\cal {E}(\rho ^\mu )$ consists of functions u satisfying |Z ^(α)u|≤ c _α,μρ ^μ−|α|.

This shows m(J, θ) ≥ 0.

When m(J, θ) = 0 the mass formula shows

$$\displaystyle \begin{aligned}\beta_{\bar{1}\bar{1}}=0\,, \beta_{,\bar{1}1} = 0, \, P(\beta)= 0\end{aligned}$$

We conclude R ≡ 0 but still need to show A ₁₁ = 0.

Let φ _s be the flow generated by the Reeb vector field T, and let

$$\displaystyle \begin{aligned}J(s) = \varphi_s^* J \ \mbox{ i.e. } \overset{.}{J} = 2A_{J,\theta}.\end{aligned}$$

Since this deformation is pull back by φ _s, its underlying CR structure is biholomorphic to the original (J, θ), hence the condition P _(s) ≥ 0 is preserved.

Making use of $A_{11, \bar {1}\bar {1}} = O(s\rho ^{-8})$ for s small and ρ large we find

$$\displaystyle \begin{aligned} \frac{d}{ds} R_{J_s, \theta} &= -2 |A_{11}|{}^2 + i(A_{11, \bar{1}\bar{1}}-A_{\bar{1}\bar{1},11})\\ \frac{d}{ds} (A_{11})_{J_{(s)},\theta} &= -i A_{11,0}. \end{aligned} $$

We see that

$$\displaystyle \begin{aligned} R_{J_{(s)},\theta} &\geq -cs \ \mbox{ on } N\\ &\geq -c \frac{s}{\rho^8} \ \mbox{ near } \infty. \end{aligned} $$

Therefore we can solve v _s, decaying to zero near ∞

let u _s = 1 − v _s, then $(N, J_{(s)}, u^2_s \theta )$ is scalar flat and asymptotic flat.

$$\displaystyle \begin{aligned} \Rightarrow u_s &= 1- \frac{1}{32\pi \rho^2} \int_N R_{J_{(s)},\theta} u_s \theta \wedge d\theta + O(\rho^{-3})\\ \Rightarrow m_s &= -\frac{3}{4}\int_N{ R_{J_{(s)}, \theta} u_s} \theta \wedge d\theta . \end{aligned} $$

Differentiating in s:

$$\displaystyle \begin{aligned}\frac{d}{ds} \underset{s=0}{|} m_s = \frac{3}{2} \int |A_{11}|{}^2 \theta \wedge d\theta >0 \ \mbox{ unless } A_{11} \equiv 0.\end{aligned}$$

This implies for s < 0 and small m _s < 0. This is a contradiction to the first part of this theorem.

Remarks

• 1. It is possible to show that ∃ small perturbation J _(s) of the standard CR structure on S ³ which has negative mass. Indeed, in a recent preprint [15], it is shown that the Rossi sphere has negative mass and that the Sobolev quotient is never attained.

• 2. We believe that the Rossi sphere with $Z_1^{(t)} = Z_1 +t\bar {Z_1}$ for t small have negative mass. In fact in on-going work with Cheng and Malchiodi that for t small, every minimizing sequence for the Sobolev quotient $\frac {\int L_\epsilon u\cdot u}{||u||{ }^2_4}$ must blow up. So that the infimum for the Sobolev quotient is never attained.

• 3. It is quite likely this is true for a large class of perturbations of J.

• 4. In dimensions 2n + 1 ≤ 7, and locally spherical CR structures. Cheng, Chiu and I showed [11] the developing map is injective, and the CR mass is positive along the same lines as the Schoen–Yau argument.

4.7 The Q-Prime Curvature Equation

In Sect. 4.4 we introduced the P-prime operator and Q-prime curvature equation. For a pseudo-Einstein manifold (M ³, J, θ) the Q ^′ curvature is given by

under conformal change of contact form

$$\displaystyle \begin{aligned}\tilde{\theta} = e^\sigma \theta,\end{aligned}$$

where σ is pluriharmonic, we have

$$\displaystyle \begin{aligned}e^{2\sigma} \tilde{Q^\prime} = Q^\prime + P^\prime \sigma + \frac{1}{2} P(\sigma^2).\end{aligned}$$

In analogy with Gursky’s result [22] about total Q-curvature on a Riemannian 4-manifold we have the following:

Theorem ([8])

Let $(M^3, \bar {\theta }, J)$be a pseudo-Einstein manifold with positive CR Yamabe constant and non-negative Paneitz operator. Given any p ∈ M, it holds that

$$\displaystyle \begin{aligned}\int_M Q^\prime = 16 \pi^2 - 4 \int_M G_L^4 |A_{11}|{}_\theta^2 - 12\int \log (G_L)P_4 \log (G_L)\end{aligned}$$

where G _L is the Green’s function for the CR conformal Laplacian with pole at p and $\tilde {\theta } = G_L^2 \theta$ . In particular,

$$\displaystyle \begin{aligned}\int_M Q^\prime \leq 16\pi^2\end{aligned}$$

with equality if and only if (M ³, J) is CR equivalent to the standard 3-sphere.

We indicate two proofs of this result, the first one depends on the positive mass theorem and an identity relating the change of the Q ^′-curvature under conformal change of contact form $\tilde {\theta } = e^\sigma \theta$ where σ is not necessarily pluriharmonic. While the second argument is elementary and hence more transparent.

If the background contact form θ is pseudo-Einstein, and $\tilde {\theta } = e^\sigma \theta$ is the minimizer of the Sobolev quotient so that normalizing the values of $\tilde {\theta }$ to be that of the standard S ³, then

$$\displaystyle \begin{aligned}R(\tilde{\theta}) = \mbox{ constant } \leq 2.\end{aligned}$$

Hence the formal expression

$$\displaystyle \begin{aligned}\int R^2 (\tilde{\theta}) - 4 |A_{11} (\tilde{\theta})|{}^2 \tilde{\theta}d\tilde{\theta} \leq \int R^2(\tilde{\theta}) \tilde{\theta}d\tilde{\theta} \leq \int R^2 (\mbox{ standard sphere }) dV = 16\pi^2.\end{aligned}$$

On the other hand in [7] we found

$$\displaystyle \begin{aligned}\int Q^\prime (\tilde{\theta}) \hat{\theta} \wedge d \tilde{\theta} = \int Q^\prime (\theta) \theta \wedge d \theta + 3 \int \sigma P \sigma \theta \wedge d\theta.\end{aligned}$$

Therefore we have

$$\displaystyle \begin{aligned}\int Q^\prime(\theta) \theta \wedge d\theta \leq 16\pi^2,\end{aligned}$$

with equality holding iff the Yamabe invariant of (M ³, J) agrees with the standard sphere, hence it is biholomorphic to the standard sphere.

The second argument works for boundaries of strictly pseudoconvex domains in $\mathbb {C}^2$ which have a contact form θ defined $\theta =Im \bar {\partial } u$ where u is an approximate solution of Fefferman’s equation:

$$\displaystyle \begin{aligned} J[u]=\det \left\lgroup\begin{array}{cc} u&\frac{\partial u}{\partial \bar{z}_{\bar{j}}}\\ \frac{\partial u}{\partial z_i} & \frac{\partial^2u}{\partial z_i\partial\bar{z}_j}\end{array}\right\rgroup = 1 + O(u^3). \end{aligned}$$

We will consider the contact form $\tilde {\theta } = G_p^2\theta$ where G _p is the Green’s function for L _θ with pole at p. Instead of conformal normal coordinates, we work with Moser’s coordinates [19]. That is, there is a local biholomorphic change of coordinate near p, say (z, w) with p at the origin so that ∂ Ω is given by, writing w = u + iv, ,

$$\displaystyle \begin{aligned}v = |z|{}^2 + E (u, z, \bar{z}),\end{aligned}$$

where

$$\displaystyle \begin{aligned}E(u, z, \bar{z}) = + c_{42} (u) z^4 \bar{z}^2 + c_{24} (u) z^2 \bar{z}^4 + c_{33}(u) z^3 \bar{z}^3 + O(\rho^7).\end{aligned}$$

Let r denote

$$\displaystyle \begin{aligned}\frac{1}{2i} (w-\bar{w}) - |z|{}^2 - E(u, z, \bar{z}):\end{aligned}$$

then

$$\displaystyle \begin{aligned}J[r] = 1 + O(\rho^4).\end{aligned}$$

Lee-Melrose’s asymptotic expansion [27] reads

$$\displaystyle \begin{aligned}u \backsim r \sum_{k\geq 0} \eta_k (r^3 \log r)^k \mbox{ near } \partial \Omega = \{r=0\},\end{aligned}$$

with

$$\displaystyle \begin{aligned}\eta_k \in \mathcal{C}^{\infty }(\bar{\Omega})..\end{aligned}$$

Thus for N large, $u \backsim r \sum _{n=0}^N \eta _k (r^3 \log r )^k$ has many continuous derivatives in Ω and vanish to high order at ∂ Ω. Hence

$$\displaystyle \begin{aligned}J[r\eta_0] = 1+O(\rho^4)\end{aligned}$$

$$\displaystyle \begin{aligned}\eta_0 = 1+O(\rho^4)\end{aligned}$$

$$\displaystyle \begin{aligned}u \backsim r \eta_0 + \eta_1 r^4 \log r + \mbox{ h.o.t}\end{aligned}$$

$$\displaystyle \begin{aligned}\backsim r + O(\rho^6).\end{aligned}$$

We compute

(4.7.1)

Claim 1

$P^\prime (\log G_L)= 8 \pi ^2 S_p +$ a bounded function, where S _p = S(p, ⋅) is the kernel of the orthogonal projection $\pi : L^2 \to L^2 \cap \cal {P}$ onto pluriharmonics.

Making use of a similar expansion for △_b, write

$$\displaystyle \begin{aligned}G_L = \frac{1}{2\pi\rho^2} + \omega,\end{aligned}$$

we find that Lω is bounded function near p.

It then follows that

The smoothness of ω then implies that the third term is bounded.

The second term is also bounded by (4.7.1). This proves Claim 1.

Claim 2

$P((\log G_L)^2) = \delta \pi ^2 (\delta _p - S_p) +$ a bounded function. This follows from:

$$\displaystyle \begin{aligned}\breve{P}_3(\log \rho) = 0\end{aligned}$$

because ρ is the absolute value of a holomorphic function. Hence

In addition,

Claim 3

$(\log G_L)P(\log G_L)$ blows up like $\log \rho$ near p.

Therefore, it is integrable.

The transformation rule shows that away from the pole p:

$$\displaystyle \begin{aligned} -4G^4_L | \hat{A}_{11}|{}^2 &= Q^\prime + 2 P^\prime (\log G_L) + 2 P ((\log G_L)^2) - 4(\log G_L) P(\log G_L)\\ &\quad - 64 Re (J^1 \log G_L) (P_3 (\log G_L))_1, \end{aligned} $$

so we find

$$\displaystyle \begin{aligned}G_L^4|\hat{A}_{11}|{}^2 = O(\rho^4).\end{aligned}$$

Thus in the distribution sense

$$\displaystyle \begin{aligned} 2P^\prime (\log G_L) + 2P((\log G_L)^2) &= 16 \pi^2 \delta_p - Q^\prime - 4G_L^4 | \tilde{A}_{11}|{}^2 + 4 (\log G_L) P (\log G_L)\\ &\quad + 64 Re (\nabla^1 \log G_L) (P_3(\log G_L))_1. \end{aligned} $$

Apply this equation to the constant function 1 gives

$$\displaystyle \begin{aligned}\int Q^\prime = 16 \pi^2 - 4 \int G_L^4|\tilde{A}_{11}|{}^2 - 12 \int (\log G_L) P (\log G_L) \leq 16 \pi^2.\end{aligned}$$

Observe finally equality holds iff $\tilde {A}_{11} \equiv 0$ and $\log G_L$ is pluriharmonic. Since $\tilde {R}\equiv 0$, the space $(M\setminus {p}, \tilde {\theta })$ is CR flat, and is simply connected at ∞, hence is globally isometric to the Heisenberg group.

4.7.1 An Existence Result for the Q ^′-Curvature Equation

There is a variational functional whose critical point when restricted to the pluriharmonics is the Q ^′-curvature equation, modulo a Lagrange multiplier. Currently we do not know how to determine the Lagrange multiplier. To get around this difficulty we introduce the modified P-prime operator $\bar {P}^\prime := \tau P^\prime : \cal {P} \to \cal {P}$ where τ is the L ² projection to $\cal {P}.$ Consider the functional $II: \cal {P} \to \mathbb {R}$

$$\displaystyle \begin{aligned}II (\sigma) = \int \sigma \bar{\mathcal{P}}^\prime \sigma + 2 \int \bar{Q}^\prime \sigma - (\int \bar{Q}^\prime)\log \int e^{2\sigma}.\end{aligned}$$

Theorem ([5])

Let (M ³, θ, J) be a compact pseudo-Einstein 3-manifold with L _θ > 0 and P _θ ≥ 0. Suppose

$$\displaystyle \begin{aligned}\int \bar{Q}^\prime \theta \wedge d\theta < 16 \pi^2,\end{aligned}$$

then there exists a function $\sigma \in \cal {P}$ minimizing the functional II. Moreover, the contact form $\tilde {\theta } = e^\sigma \theta$ has $\bar {Q}^\prime$ equal to a constant.

Remark

The conclusion cannot be strengthened to Q ^′ =  constant, in fact there are contact forms on S ¹ × S ² with its spherical CR structure such that will have $\bar {Q}^\prime = 0$ but Q ^′ is non-constant.

The main analysis is a Moser–Trudinger inequality which is deduced from the asymptotics for the Green’s function of the pseudo-differential operator $(\bar {P}^\prime )^{\frac {1}{2}}.$ Fix a point p ∈ M. Let (z, t) be the CR normal coordinates defined in a neighborhood of p = (0, 0).

Let E(ρ ^k) denote the class of functions g ∈ C ^∞(M ∖{p}) satisfying

$$\displaystyle \begin{aligned} |\partial_Z^p \partial_{\bar{Z}}^q \partial_t^r g(z,t)| \leq C\rho(z,t)^{k-p-q-2r} \mbox{ near } 0.\end{aligned}$$

The bulk of the work is to establish the following:

Claim

There exists a B _p ∈ C ^∞(M∖{p}) s.t.

$$\displaystyle \begin{aligned}B_p - \frac{1}{\rho^2} \in {E} (\rho^{-1-\epsilon})\end{aligned}$$

for all 0 < 𝜖 < 1 and

$$\displaystyle \begin{aligned}G_p = \tau B_p\tau .\end{aligned}$$

Idea of Proof

One has

$$\displaystyle \begin{aligned}(\bar{P}^\prime)^{-\frac{1}{2}} = c \int_0^\infty t^{-\frac{1}{2}}(\bar{P}^\prime + t + \pi)^{-1}dt \end{aligned}$$

from spectral theory and

$$\displaystyle \begin{aligned}G_p = \bar{P}^{-\frac{1}{2}} \tau \delta_p \tau.\end{aligned}$$

The analysis involved a detailed study of the family G _t of PDO of order -2 depending continuously in t s.t.

$$\displaystyle \begin{aligned}(E_2 + t ) G_t = I + F_t\end{aligned}$$

where E ₂ satisfies $\bar {P}^\prime = \tau E_2, E_2$ being a classical PDO of order 2 and F _t a smoothing operator depending continuously on t.

Proof of Theorem

Making use of the claim, we established the following Moser–Trudinger inequality

$$\displaystyle \begin{aligned}\log \int e^{2(\sigma-\bar{\sigma})} \leq c + \frac{1}{16\pi^2} \int \sigma \bar{P}^\prime \sigma.\end{aligned}$$

This in turn yields estimate for minimizing sequence of the functional II.

4.8 An Isoperimetric Inequality

An interesting application of the Q-prime curvature integral is the following [32]:

Theorem

On $\mathbb {H}^1$, let σ be a pluriharmonic function on $\mathbb {H}^1$, such that e ^σθ is a complete pseudo-Einstein contact form, suppose that lim_p→∞R(e ^σθ) ≥ 0 and Q ^′(e ^σθ) ≥ 0 and $\int Q^\prime \theta \wedge d\theta < C_1$. Then for any bounded domain $\Omega \subset \mathbb {H}^1$

$$\displaystyle \begin{aligned}\mathit{\mbox{ vol }} (\Omega) \leq C \mathit{\mbox{ area }} (\partial \Omega)^{\frac{4}{3}}\end{aligned}$$

where the constant C depends only on the difference $C_1 - \int Q^\prime \theta \wedge d\theta .$

Remarks

• 1. This is a weaker version of an analogous result of Wang [31] about Q-curvature integral on $\mathbb {R}^n$, where the Q-curvature is given by

  

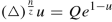
  for the conformal metric g = e ^2u|dx|².

• 2. The constant C ₁ is critical in that there exists example where $\int _{\mathbb {H}^\prime } Q^\prime \theta \wedge d\theta = C_1$ and no isoperimetric constant exists.

• 3. This is a result in harmonic analysis where the assumptions imply that ω = e ^2σ is an A ₁-weight, i.e. as a measure

  

Idea of Proof

The first step is to show that $\tilde {\theta } = e^\sigma \theta$ is a normal contact form i.e.

$$\displaystyle \begin{aligned}\sigma(x) = \frac{1}{C_1} \int_{\mathbb{H}^\prime} \frac{\log \rho(y)}{\rho(y^{-1}\cdot x)} Q^\prime (y) e^{2\sigma (y)}dv(y) + C.\end{aligned}$$

The rest of the argument is to show that ω = e ^2σ is an A ₁-weight.

A Counterexample

The contact form $\hat {\theta } = \frac {1}{\rho ^2}\theta$ on $\mathbb {H}^1 \setminus \{0\}$ is clearly not an A ₁ weight and it does not satisfy an isoperimetric inequality. It is easy to construct a smoothing of $\tilde {\theta }$ so that it satisfies the assumptions of the theorem. This shows that the constant C ₁ is sharp.

4.9 Geometry of Surfaces in the Heisenberg Group

In this last topic we discuss the local invariants of a surface Σ² in the Heisenberg group $\mathbb {H}^1$, the p-mean curvature equation and several global questions of interest to analysts.

In the following I will summarize briefly the local invariants of a surface in the Heisenberg group $\mathbb {H}^1$. Given a smooth surface $\Sigma \subset \mathbb {H}^1$ at a generic point p the tangent space and the contact plane are distinct. We let e ₁ be a unit vector whose span 〈e ₁〉 = T _ρ Σ ∩ γ ₁ and p will be called a regular point. Nearby point q will also be regular and the e ₁ vector field exists locally. It determines integral curves $\gamma , (\overset {\cdot }{\gamma } = e_1)$ called characteristic curves. The equation $\nabla _{ \overset {\cdot }{\gamma } } \overset {\cdot }{\gamma }= HJ\overset {\cdot }{\gamma }$ defines the p-mean curvature H for the surface. Along the regular part of the surface we have the local framing {e ₁, e ₂ = Je ₁, T}. Since T and e ₂ are both transverse to T _p Σ, there exist unique α so that

$$\displaystyle \begin{aligned}T+ \alpha e_2 \in T_p \Sigma.\end{aligned}$$

α is called the angle function. The associated dual frame {e ¹, e ², θ} exists in a neighborhood of p. It is natural to define the area element as |e ¹ ∧ θ|, in fact its integral gives the 3-dimensional Hausdorff measure of Σ.

The p-mean curvature equation for a graph t = u(x, y) read as

$$\displaystyle \begin{aligned}\frac{(u_y +x)^2 u_{xx} - 2 (u_y+x)(u_x-y)u_{xy} + (u_x - y)^2u_{yy}}{((u_x-y)^2+ (u_y+x)^2)^{\frac{3}2}} = H.\end{aligned}$$

It is a degenerate hyperbolic equation.

Notice that the p-mean curvature is not defined at a characteristic point p on the surface, i.e. where T _p Σ = ξ _p or in the case of a graph

$$\displaystyle \begin{aligned}u_y+x=0 \ \mbox{ and } u_x-y=0.\end{aligned}$$

We summarize the key facts below [12].

When Σ is C ²-smooth and the p-mean curvature satisfy the condition near a singular point $p:|H(q)|=O (\frac {1}{|p-q|})$ then there is the classification:

• 1. either p is an isolated singular point and the e ₁-line field has an isolated singularity at p, and its topological degree is 1; or

• 2. p is part of a C ¹-curve γ consisting of the singular points near p and the characteristic line field e ₁ pass transversally through the singular curve; and as a line field, it is not singular at the curve γ.

If Σ² is a closed surface in $\mathbb {H}^1$ with bounded p-mean curvature then χ( Σ²) ≥ 0. This is the direct consequence of the Hopf index theorem.

Pansu’s sphere is obtained by rotating the following curve around the z-axis:

$$\displaystyle \begin{aligned} X(s) &= \frac{1}{2\lambda} \sin (2\lambda s)\\ Y(s) &= \frac{1}{2\lambda} (-1 + \cos (2\lambda s))\\ Z(s)& = \frac{1}{2\lambda} (s- \frac{1}{2\lambda} \sin (2\lambda s)) \end{aligned} $$

Theorem ([28])

If Σ ²is smooth C ²solution of the p-mean curvature equation with H = constant > 0, then it is congruent to the Pansu sphere. It has two singular points (0, 0, 0) and (0, 0, π).

Elliptic Approximation

One way to deal with the lack of ellipticity in this problem is to approximate the Heisenberg geometry by a family of Riemannian metrics, and then to understand the limiting behavior of the solutions of the Riemannian mean curvature equation. This is difficult to do because the Heisenberg is not the metric limit of Riemannian spaces with Ricci bounded from below.

Conditions for a Weak Solution

(II)

We say u ∈ W ¹( Ω) is a weak solution of the p-mean curvature equation if

$$\displaystyle \begin{aligned}\int_{S[u]} |\nabla \varphi| + \int_{\Omega\backslash S[u]} N(u) \cdot \nabla \varphi + \int H \varphi \geq 0, \text{for all test functions}\,\, \phi.\end{aligned} $$

One surprising feature of this equation is the following equivalence:

Theorem

u ∈ W ^{1, 1}( Ω) is a weak solution if and only if u is a minimizing solution.

Analogue of the Codazzi Equation

Let V  denote the vector field T + αe ₂: then [13]

$$\displaystyle \begin{aligned}e_1e_1(\alpha) = - 6 \alpha e_1 (\alpha) + V(H) - \alpha H^2 - 4\alpha^3\end{aligned} $$

along each characteristic curve.

It is the analogue of the Codazzi equation because it is part of a system of equations that characterize a smooth surface in the Heisenberg group as an abstract surface with a smooth foliation of curves just like the Gauss and Codazzi equation characterized a smooth surface in $\mathbb {R}^3$

Invariant Surface Area Functionals

In a pseudo-Hermitian manifold (M ³, θ, J) let Σ² be a smooth surface without singular points. The following two area functionals are conformally invariant:

$$\displaystyle \begin{aligned} dA_1 &= | e_1 (\alpha) + \frac{1}{2} \alpha^2 - Im A_{11} + \frac{1}{4} R + \frac{1}{6} H^2|{}^{\frac{3}{2}} \theta \wedge e^1\\ dA_2 &= \left\{V(\alpha) + \frac{2}{3} \left[e_1(\alpha) + \frac{1}{2} \alpha^2 - Im A_{11} + \frac{1}{4}R \right] H + \frac{2}{27}H^3\right.\\ &\left.\quad + Im \left(\frac{1}{6}R_{\bar{1}}+ \frac{2}{3} i A_{\bar{1}\bar{1}, 1}\right) + \alpha Re A_{\bar{1}\bar{1}}\right\} \theta \wedge e^1 \end{aligned} $$

They remain pointwise conformally invariant under conformal change of contact form $\hat {\theta } = e^{2u}\theta .$

These invariants were first given by J. Cheng in terms of the Chern connection, and more recently by Cheng et al. [16] in terms of the local invariants developed more recently.

Examples in the Heisenberg Group

• 1. The vertical plane y = 0 it is easy to see that H = 0 and α = 0, hence it is a minimizer for the energy integral ∫_ΣdA ₁ = E ₁[ Σ], it is also critical for E ₂.

• 2. The shifted sphere: $(|z|{ }^2 + \frac {\sqrt {3}}{2}\rho _0^2)^2 + 4t^2 = \rho _0^4$ (for any positive p ₀). It is an easy computation to show dA ₁ ≡ 0 hence it is a closed example having the topology of the 2-sphere. It is an open question whether this is the unique minimizer (up to a biholomorphic transformation of the Heisenberg group).

• 3.
  

  Again

  $$\displaystyle \begin{aligned}e_1(\alpha) + \frac{1}{2}\alpha^2 + \frac{1}{6} H^2 = 0\end{aligned}$$

• 4. The Clifford Torus

  $$\displaystyle \begin{aligned}\rho_1 = \rho_2 = \frac{\sqrt{2}}{2} \subset S^3\end{aligned}$$

  where

  $$\displaystyle \begin{aligned}z_1 = \rho_1e^{i\varphi_1}, z_2 = \rho_2e^{i\varphi_1}.\end{aligned}$$

  This surface is p-minimal in S ³, i.e. H = 0. It is, after a bit of computation, a critical point for E. Again it is an open question whether this is the unique minimizer for E ₁ under the topological constraint to be a torus.

Connections to the Singular Yamabe Problem

Consider change of contact form $\hat {\theta } = u^{-2}\theta ,$ we consider $\Omega \subset \mathbb {H}^\prime$ the singular CR Yamabe problem:

(4.9.1)

It is relatively easy to see the existence of solutions to this equation by means of the strong maximum principle.

The interesting question is when is the solution smooth at the boundary.

We develop the solution u in Taylor series expansion in powers of ρ, a defining function for ∂ Ω.

Thus

$$\displaystyle \begin{aligned}u(x,p) = c(x)\rho + v(x) \rho^2 + w (x) \rho^3 + z(x)\rho^4 + l(x)\rho^5 \log \rho + h(x)\rho^5 +O(\rho^6)\end{aligned}$$

Similar to the expansion for the Riemannian singular Yamabe solution expansion of R. Graham we are able to determine, after an extended calculation, these coefficients c, v, w, z, l.

It turns out that l is related to the volume renormalization coefficient L and the functional E ₂.

Consider the volume expansion

$$\displaystyle \begin{aligned} \mbox{Vol} \{\rho>\epsilon\} &= \int_{\{\rho>\epsilon\}} u^{-4} \langle e_2, v \rangle d\mu_{\Sigma_\rho} d\rho.\\ \mbox{Vol} (\{\rho>\epsilon\}) &= c_0 \epsilon^{-3} + c_1\epsilon^{-2} + c_2\epsilon^{-1} + L \log \frac{1}{\epsilon} + V_0 + o(1) \end{aligned} $$

with

$$\displaystyle \begin{aligned} c_0 &= \frac{1}{3}\int_\Sigma \theta \wedge \theta^1\\ c_1 &= -\frac{1}{6} \int_\Sigma H \theta \wedge \theta^1\\ c_2 &= \frac{1}{3} \int (5e_1(\alpha)+10\alpha^2+ \frac{1}{6}H^2-a_2) \theta \wedge \theta^1\\ L &= \int_\Sigma \underbrace{\frac{1}{6}e_1e_1(H)+4V(\alpha) + \alpha e_1 (H) + 2 H e_1(\alpha)+ \frac{4}{27} H^3}_{V^{(3)}} \end{aligned} $$

and where

$$\displaystyle \begin{aligned}{}[T,e_1] &= -a_1 e_1 + [a_2 + \omega (T)]e_2\\ {} [e_2, T] &= -a_1e_2 + (-a_2+\omega (T)]e_1 \end{aligned} $$

It turns out $\frac {1}{2}V^{(3)}$ differs from dA ₂ by an exact form

$$\displaystyle \begin{aligned}dA_2 - \frac{1}{2} V^{(3)} = \frac{1}{12} d (e_1(H)\theta) - d(\alpha e^1) + \frac{1}{3} d(\alpha H \theta) + \frac{1}{2} d (a_1 \theta)\end{aligned}$$

and l is the Euler equation in L.

Therefore we find

Theorem ([16])

The solution u to the equation (δ, 1) is smooth up to order 5 if and only if ∂ Ω =  Σ is a critical point for the energy functional E ₂.

It is an interesting question to ask where does the energy functional E ₁ come from.

Example

The upper Heisenberg $\tilde {\theta }=\frac {1}{y^2} \theta$. In this case, the function u = y is smooth at the boundary.

Euler Equations of E ₁ and E ₂

$$\displaystyle \begin{aligned} \delta E_1 &= l = He_1e_1H + 3e_1V(H)+e_1(H)^2 + \frac{1}{3} H^4 + 3 e_1(\alpha)^2 + 12 \alpha^2e_1(\alpha)+12\alpha^4 \\ &- \alpha H e_1 (H)+ 2H^2e_1(\alpha) + 5\alpha^2H^2 + \frac{3}{2}R(e_1(\alpha)) + \frac{2}{3} H^2 + 5 \alpha^2 + \frac{1}{2} R.\\ \delta E_2 &= |H_{cr}|{}^{-\frac{1}{2}} \left\{-\frac{1}{4} sqn (H_{cr}) f e_1 (H_{cr}) + \frac{1}{2}e_1 (|H_{cr}|f) + \frac{3}{2} (H_{cr})f\alpha\right.\\ &\left.\quad + H_{cr} \left[\frac{9}{2} V(\alpha) + 3 HH_{cr} - \frac{1}{6}H^3\right]\right\}. \end{aligned} $$

where

$$\displaystyle \begin{aligned} H_{cr} &= e_1(\alpha) + \frac{1}{2} \alpha^2 + \frac{1}{6} H^2\\ f& = \frac{\frac{1}{2}e_1 (H) H_{cr} + \frac{3}{4} V(H_{cr} + \frac{1}{4} H e_1 (H_{cr}) - \frac{1}{2} \alpha HH_{cr}}{2|H_{cr}|}. \end{aligned} $$

Open Questions

In these lectures we dealt with the extremals of the following two Sobolev inequalities:

$$\displaystyle \begin{aligned} c_1 ||u||{}_{\frac{4}{3}} &\leq \int |\nabla_b u|\\ c_2 ||u||{}_4 &\leq \int | \nabla_b u|{}^2 \end{aligned} $$

It is natural to wonder about

$$\displaystyle \begin{aligned}c_1 || u ||{}_q \leq \int|\nabla_b u|{}^p \,?\end{aligned}$$

and what are the level sets of critical functions? It appears likely that there are fractional order conformally covariant operators which may shed light on sharp form of inequalities of these type.

As for geometric applications for some of the analysis discussed here we hope to be able to study the following type of questions. A basic question is:

$$\displaystyle \begin{aligned}0 < \int_{M^3} Q^\prime \theta \wedge d\theta \Rightarrow M^3 = S^3/\Gamma, \quad where\, \Gamma \,is \,a \,finite \,subgroup \,of \,U(2),\end{aligned}$$

and whether (S ³∕ Γ, θ _can, J _can) realizes maximal value of $\int Q^\prime .$ for this topology.

Similarly if M ³ is the unit tangent bundle of a hyperbolic surface Σ, it is expected that it also realizes the maximal value of

$$\displaystyle \begin{aligned}\int Q^{\prime} \theta \wedge d\theta \end{aligned}$$

for the given topology.