Keywords

1 Introduction

“... we have to decide what is the most fundamental nature of matter. Does it stick or not?”. This question was posed by professor Kevin Kendall in his fascinating book “Molecular adhesion and its applications: the sticky universe” [1]. He introduced the so-called adhesion paradox: objects do not often stick together but the matter they form is sticky at the molecular scale. The main cause of this paradox is that objects surface is not smooth. Surface roughness widely reduces the effective contact area and destroys adhesion [2]. In this respect, a tricky challenge is to find a criterion for stickiness of rough surfaces [3, 4].

In the last years, the leading role of adhesion has been recognized in several fields. Interfacial forces dominates the contact mechanics of micro and nano electro-mechanical devices, because of their high surface to volume ratio [5]. In biomedical applications it is often required to optimize adhesive properties and, for example, the design of polymers surface is as important as their chemical composition [6]. Sticky skills of animals, e.g. insects and gecko, are studied by engineers with the aim of manufacturing adhesive robots [7] and dry reversible adhesives [8,9,10,11]. Moreover, many tribological aspects, like friction, leak-rate of seals, lubrication [12, 13], are influenced by surface interactions.

One of the first work on the adhesion of elastic bodies is due to Johnson, Kendall & Roberts (JKR) [14]. The JKR theory faithfully depicts adhesion between soft bodies. The model assumes the presence of short-range attractive interactions acting inside the contact zone. The contact shape is modified by these strong surface forces and adhesion leads to large elastic deformations.

Derjaguin, Muller & Toporov (DMT) proposed a different model [15]. Moving from the Hertzian solution, the DMT theory predicts long-range interactions outside the contact area. Contrary to what happens in the JKR model, adhesion does not alter the contact shape, which remains equal to the Hertzian one.

The JKR and DMT theories describe the same phenomenon in two different limits. Tabor [16] introduced the so-called Tabor parameter \(\mu =\left[ R\Delta \gamma ^{2}/\left( E^{*2}\epsilon ^{3}\right) \right] ^{1/3}\) (where \(E^{*}\) is the composite elastic modulus of the spheres and \(\epsilon \) is the range of attractive forces) to describe the transition between the JKR and DMT solutions. Specifically, the JKR theory applies for soft elastic spheres with large radius of curvature (\(\mu \gg 1\)), while the DMT theory for hard elastic spheres with small radius of curvature (\(\mu \ll 1\)).

Later, Muller et al. [17] describing the adhesive interactions by means of the Lennard-Jones (LJ) law, showed that the pull-off force also depends on the elastic properties of the contacting bodies and the values predicted by the JKR and DMT theories occur for soft and hard media, respectively.

Even in the simple case of spherical contact, there exist complex phenomena, such us instability at the contact jump-in and jump-off, that can be predicted only by numerical simulations.

Both the JKR and DMT theories are formulated in the simple case of the contact between elastic smooth spheres. However, real surfaces show roughness that may extend on several length scales. Multiasperity models, multiscale theories and advanced numerical codes are the main instruments to extend adhesion theory to the contact mechanics of rough solids.

In this work, we discuss an extension of the DMT and JKR theory to the adhesion of rough surfaces. To this aim, we use a recent advanced multiasperity model, the Interacting and Coalescing Hertzian Asperities (ICHA) model [18,19,20]. Typically, a DMT based solution consists in solving the contact problem neglecting the effect of adhesion on surface deformations, but simply scaling the total load by a tensile attractive contribution. In a JKR based solution it is instead necessary to model surface deformations due to adhesion.

The ICHA model differs from the pioneering Greenwood and Williamson (GW) model [21] because it takes account of (i) the effective curvature of the asperities, (ii) the lateral interaction between asperities, (iii) the coalescence of merging contact regions.

The results of the ICHA model are compared with data taken from the literature, finding a very good agreement in both DMT and JKR limit.

2 Adhesion of Rough Elastic Media

The first attempt to study the effect of roughness on adhesion is due to Fuller & Tabor (FT) [22]. They incorporated the JKR theory in the Greenwood & Williamson (GW) model [21], comparing theoretical predictions of the pull-off force to experimental measurements on rubbers.

In the GW-type models, roughness is described by spherical asperities with the same radius of curvature. The height distribution of the asperities follows a Gaussian law (or an exponential one). Moreover, asperities are assumed independent of each other.

Recently, it has been proved that modelling the elastic coupling of contact regions is fundamental to correctly simulate the contact problem [19, 23]. Real surfaces often show a more complex roughness than modeled in the GW model and small deviations from the nominally Gaussian case can also occur (Ref. [24]).

In general, roughness of real surfaces can be described with a self-affine geometry with power spectral density (PSD) of the form

$$\begin{aligned} C(q)= & {} C_{0}\qquad \qquad \qquad \qquad \text {for }q_{L}\le q<q_{0} \nonumber \\ C(q)= & {} C_{0}\left( q/q_{0}\right) ^{-2(H+1)}\qquad \text {for }q_{0}\le q<q_{1} \end{aligned}$$
(1)

and zero otherwise.

In Eq. (1), \(C_{0}\) is the amplitude of the power spectrum, H is the Hurst exponent, which is related to the fractal dimension \(D_{f}=3-H\), \(q=\sqrt{q_{x}^{2}+q_{y}^{2}}\) is the modulus of the wave vector, \(q_{L}\) and \(q_{1}\) are the short and long cut-off frequencies, and \(q_{0}\) is the roll-off frequency. In the simple case of the sphere, the Tabor parameter is very useful to identify the fields of application of the DMT and JKR theories. For rough surfaces several authors [25,26,27] agree in defining a modified Tabor parameter by replacing the radius R of the sphere with the mean radius of curvature \(\rho \) of the surface

$$\begin{aligned} \mu _{T}=\left[ \rho \Delta \gamma ^{2}/\left( E^{*2}\epsilon ^{3}\right) \right] ^{1/3} \end{aligned}$$
(2)

For a fractal surface, \(\rho =2/h_{\mathrm {rms}}^{^{\mathrm {\prime \prime } }} \), being \(h_{\mathrm {rms}}^{^{\mathrm {\prime \prime }}}\) its rms curvature.

Other authors have suggested different ideas in the definition of the Tabor parameter. For example, in Ref. [28], a generalization of the Tabor parameter to non-spherical geometries is proposed, by exploiting an analogy with the ‘small-scale yielding’ criterion. Specifically, if we define the width \(s_{0} \) of the zone where the traction exceeds the theoretical strength \(\sigma _{0}=\Delta \gamma /\epsilon \) by the equation

$$\begin{aligned} s_{0}=\frac{E^{*}\Delta \gamma }{\pi \sigma _{0}^{2}}, \end{aligned}$$
(3)

the JKR solution is expected to give a good approximation if \(s_{0}\ll a_{\min }\), where \(a_{\min }\) is the smallest linear dimension of the contact geometry. Therefore, the Tabor parameter could be alternatively defined as

$$\begin{aligned} \mu _{T}=\sqrt{\frac{0.21a_{\min }}{s_{0}}} \end{aligned}$$
(4)

where the numerical factor is introduced to ensure coincidence between the above definition and the conventional one in the case of the sphere, with \(a_{\min }\) being the radius of the circular contact area at pull-off.

Moreover, near full contact, Ciavarella et al. [29] suggested to use in the definition of the Tabor parameter the size of separation areas as characteristic dimension, rather than the contact areas.

2.1 Modeling Adhesion with the Advanced Asperity Model ICHA in the DMT and JKR Limits

In the following, we discuss DMT- and JKR-type solutions for rough contacts, comparing results of the Interacting and Coalescing Hertzian Asperities (ICHA) model [18] with data taken from the literature. For details of the model the reader is referred to the works [18, 19, 23].

Here, we recall that the problem is solved under controlled displacement conditions. When an asperity gets into contact, the first estimate of the contact radius is done by inverting the \(\delta (a)\) relation, which is

$$\begin{aligned} \delta =\frac{a^{2}}{R} \end{aligned}$$
(5)

in the DMT limit, and

$$\begin{aligned} \delta =\frac{a^{2}}{R}-\sqrt{\frac{2\pi a\Delta \gamma }{E^{*}}} \end{aligned}$$
(6)

in the JKR limit.

In the ICHA-DMT model [23, 27, 30], contact raises when the distance between an asperity and the half-space vanishes; on the contrary, in the ICHA-JKR model [31], jump into contact occurs when the approach reaches a critical gap [32]

$$\begin{aligned} \Delta _{ON}=\left( 1-2.641\mu _{T}^{3/7}\right) \epsilon , \end{aligned}$$
(7)

where \(\epsilon \) is the range of attraction of adhesive forces, close to atomic distance.

When the approach is further increased of a quantity \(\eta _{i}\), the contact radius is correspondingly increased of \(\Delta a_{i}=\eta _{i}/(d\delta /da)\).

The total contact area and the total load are calculated by summing up the contributions of all the asperities in contact. However, we observe that in the JKR limit the total force is computed as the sum of the forces on each contacting asperity

$$\begin{aligned} F=\frac{4}{3}\sum _{i=1}^{n_{ac}}\left( \frac{E^{*}a_{i}^{3}}{R_{i}}- \sqrt{8\pi E^{*}\Delta \gamma a_{i}^{3}}\right) . \end{aligned}$$
(8)

In the DMT limit, according to the classical DMT force approach [33], we first calculate the total force due to the Hertzian loads on each asperity

$$\begin{aligned} F_{H}=\frac{4E^{*}}{3}\sum _{i=1}^{n_{ac}}\frac{a_{i}^{3}}{R_{i}}, \end{aligned}$$
(9)

then the effective total load F is obtained by subtracting to \(F_{H}\) the total adhesive load \(F_{ad}\), which is computed by the convolution of the gap probability distribution P(u) and a convenient traction-separation law \(\sigma (u)=\frac{8\Delta \gamma }{3\epsilon }\left[ \left( \frac{\epsilon }{u+\epsilon }\right) ^{3}-\left( \frac{\epsilon }{u+\epsilon }\right) ^{9}\right] \) (see Ref. [26])

$$\begin{aligned} F_{ad}=A_{0}\int _{0}^{\infty }\sigma (u)P(u)du\text {.} \end{aligned}$$
(10)
Fig. 1.
figure 1

(a) The relative contact area \(\bar{A}\) as a function of the normalized contact pressure \(p^{*}=p/(E^{*}h_{\mathrm {rms}}^{^{\mathrm {\prime }}})\). (b) The interfacial mean separation as a function of the normalized contact pressure \(p^{*}=p/(E^{*}h_{\mathrm {rms}}^{^{\mathrm {\prime }}})\). The ICHA-JKR model predictions are compared with the GFMD solution, used as a reference. Calculations are performed on a self-affine fractal surface with \(H=0.8,\) \(q_{L}=\pi /50\) \(\upmu \mathrm {m}^{-1}\), \(q_{0}=\pi /10\) \(\upmu \mathrm {m}^{-1},\) \(q_{1}=20\pi \) \(\upmu \mathrm {m}^{-1}\), rms roughness amplitude \(h_{\mathrm {rms}}=0.762\) \({\upmu }\mathrm {m}\), and Tabor coefficient \(\mu _{T}=3\).

Full size image

Finally, the interfacial mean separation \(\bar{u}\) is calculated by subtracting the total approach \(\delta \) to the initial separation \(\bar{u}_{0}\) as a self-balanced load distribution is considered on the problem domain.

2.2 Results

Figure 1 shows predictions of the ICHA-JKR model compared with the Green Function Molecular Dynamics (GFMD) solution by Müser [34, 35], here used as a reference. Specifically, Fig. 1a shows a comparison in a double-logarithmic representation about the area vs. load relation; in Fig. 1b the comparison concerns the predictions of the interfacial mean separation.

We recall that calculations are performed on a self-affine fractal surface with Hurst exponent \(H=0.8\), roll-off frequency \(q_{0}=\pi /10\) \(\upmu \mathrm {m}^{-1}\), short frequency cut-off \(q_{L}=\pi /50\) \(\upmu \mathrm {m}^{-1}\), and long frequency cut-off \(q_{1}=20\pi \) \(\upmu \mathrm {m}^{-1}\). Moreover, the root mean square (rms) height fluctuation is \(h_{\mathrm {rms}}=0.762\) \(\upmu \mathrm {m}\).

Fig. 2.
figure 2

(a) The relative contact area \(\bar{A}\) as a function of the normalized contact pressure \(p/E^{*}\). (b) The interfacial mean separation as a function of the normalized contact pressure \(p/E^{*}\). The ICHA-DMT and ICHA-JKR model predictions are compared with the numerical solution by Ref. [26], used as a reference. Calculations are performed on a self-affine fractal surface with \(H=0.8,\) \(q_{L}=0.25\) \(\upmu \mathrm {m}^{-1}\), \(q_{0}=1\) \(\upmu \mathrm {m}^{-1}\) and for \(q_{1}=16q_{0},32q_{0},64q_{0}\). The marker size increases with the ratio \(q_{1}/q_{0}\). The rms roughness amplitude is \(h_{\mathrm {rms}}=0.52\) nm. The Tabor coefficient for the three cases is \(\mu _{T}=0.172,0.132,0.102\).

Full size image

Results are obtained for a surface energy \(\Delta \gamma =50\) \(\mathrm {mJ/m}^{2}\) and a range of attractive interactions \(\epsilon =2.071\) nm. Consequently, according to the definition of the Tabor coefficient given in (2), we obtain \(\mu _{T}=3\). This value can be classified as short-range adhesion [36] and hence close to the JKR limit of infinitely short-range adhesion. We found the same behavior observed in the contact of smooth elastic spheres, i.e., when the surface energy \(\Delta \gamma \) is sufficiently high (\(\mu _{T}\gg 1\)) and elastic deformations due to the adhesion forces may not be neglected, the JKR solution is accurate.

On the contrary, DMT-type solutions can return accurate solutions in the opposite limit (\(\mu _{T}\ll 1\)). In this respect, Violano and Afferrante [27] showed that predictions with DMT-type models [23, 26] of the effective interfacial binding energy \(\gamma _{\mathrm {eff}}\), which takes account of the elastic repulsive energy, are very accurate when compared with calculations of an “exact” theoretical solution [37].

The above arguments find confirmation in the comparison shown in Fig. 2. Results are obtained for a self-affine fractal surface with \(q_{L}=0.25\) \(\upmu \mathrm {m}^{-1}\), \(q_{0}=1\) \(\upmu \mathrm {m}^{-1}\) and rms roughness amplitude \(h_{\mathrm {rms}}=0.52\) nm. We show three cases corresponding to \(q_{1}=16q_{0}\), \(32q_{0}\), \(64q_{0}\) and Tabor coefficient \(\mu _{T}=0.172,0.132,0.102\). In Fig. 2 the marker size increases with the ratio \(q_{1}/q_{0}\). We stress that, in the DMT limit, the maximum tensile load, i.e. the pull-off force, is not influenced by the truncation frequency of the power spectrum, as deeply discussed in a very recent note [38]. In this case, we notice a very good agreement between the ICHA-DMT predictions with the “exact” numerical ones taken from Ref. [26] (Fig. 2a). On the contrary, results of the ICHA-JKR model are much less accurate as we are far from the limit of infinitely short-range interaction forces.

Fig. 3.
figure 3

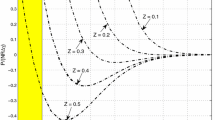
Adhesion-induced (relative) excess contact area \(\Delta \bar{A}=\bar{A}(\Delta \gamma )-\bar{A}(0)\), at a reduced pressure of \(p^{*}=p/(E^{*}h_{\mathrm {rms}}^{^{\mathrm {\prime }}})=0.02\). The dotted line represents the fit of the numerical predictions of the GFMD code by Muser [25]. Results are obtained for systems with \(H=0.8\), and different values of the Tabor parameter. For \(\mu _{T}=0.657,0.885,1.072\) we used the ICHA-DMT model (red squares), while for \(\mu _{T}=5.772,14.541,26.793\) the ICHA-JKR one (green disks).

Full size image

Müser showed that “short-range adhesion or JKR-like adhesion compactifies contact patches and smoothes contact lines” [25]. He found that the JKR regime occurs at \(\mu _{T}>1\), when adhesive forces are predominant within the contact zone. He introduced a measure of the surface energy for randomly rough surfaces (rrs), introducing the quantity

$$\begin{aligned} \gamma _{\mathrm {rrs}}=\frac{\Delta \gamma }{E^{*}\rho }\frac{\tanh (\mu _{T})}{\left( h_{\mathrm {rms}}^{\prime }\right) ^{3}}\text {.} \end{aligned}$$
(11)

Adhesion certainly leads to an increase of the relative contact area \(\bar{A}=A/A_{0}.\) For testing if \(\gamma _{\mathrm {rrs}}\) is a useful quantity to estimate adhesion-induced extra contact area \(\Delta \bar{A}\), Müser conducted several numerical simulations on self-affine fractal surfaces. He fixed the Hurst exponent and varied the Tabor parameter as well as the surface energy. In his results, he found that \(\Delta \bar{A}\) collapses “quite nicely over several decades in surface energies for systems including both short-range (JKR) and long-range (DMT) adhesion”. In Fig. 3, where \(\Delta \bar{A}\) is plotted in terms of \(\gamma _{\mathrm {rrs}} \), we show the fit of the results obtained by Müser with the GFMD code, for the case \(H=0.8\) and \(\mu _{T}\) ranging from 0.1 to 2.0. In particular, one can observe the existence of a proportionality relation between \(\Delta \bar{A}\) and \(\gamma _{\mathrm {rrs}}\)

$$\begin{aligned} \Delta \bar{A}\propto \gamma _{\mathrm {rrs}}^{0.7}, \end{aligned}$$
(12)

that in a range in which \(\bar{A}\) scales approximately linearly with the normalized pressure \(p^{*}=p/(E^{*}h_{\mathrm {rms}}^{^{\mathrm {\prime }}})\) becomes

$$\begin{aligned} \Delta \bar{A}\approx 17\gamma _{\mathrm {rrs}}^{0.7}p^{*}. \end{aligned}$$
(13)

In order to assess the capability of the ICHA model for reproducing the above trends, we conducted numerical simulations at different values of \(\Delta \gamma \) on the surface of the contact mechanics challenge [20]. In particular, we used the ICHA-DMT model for the lower values of \(\mu _{T} \) \(\left( \mu _{T}=0.657,0.885,1.072\right) \), and the ICHA-JKR one for the higher ones (\(\mu _{T}=5.772,14.541,26.793\)). Results are in very good agreement with the trend obtained by Müser [25].

3 Conclusions

In this paper, we investigated the applicability of the DMT and JKR theories to the contact mechanics of randomly rough surfaces. To this purpose, we used a recently developed advanced multiasperity model (ICHA model) conveniently modified to include the effect of adhesion in both the DMT and JKR limits.

We fundamentally found that, like in the contact of smooth spheres, the DMT theory is appropriate to study the contact of hard solids with low surface energy, while accurate results are obtained in the opposite limit (soft media with high surface energy) with a JKR-type approach. In particular, in adhesive contact problems involving roughness, an equivalent Tabor parameter \(\mu _{T}\) can be defined by considering the mean radius of curvature of the surface. We found that the JKR theory is valid only for \(\mu _{T}>1\).

Results are compared with data taken from the literature and confirm the ICHA model is efficient and yields very accurate predictions of the main contact quantities. In particular, it correctly reproduces the dependence of the adhesion-induced extra contact area \(\Delta \bar{A}\) on the parameter \(\gamma _{\mathrm {rrs}}\) introduced by Müser [25] as a unique measure for surface energy of randomly rough surfaces.